A Relationship between the Schrödinger and Klein–Gordon Theories and Continuity Conditions for Scattering Problems
Abstract
:1. Introduction
2. Materials and Methods
2.1. Systematic Approach to Lagrangians with Galilean Invariance
2.1.1. General Scheme for Lagrangians with Galilean Invariance
2.1.2. Madelung’s Picture of Schrödinger’s Theory
2.2. Modified Scheme for Lagrangians with Lorentz/Poincaré Invariance
2.3. Inclusion of External Forces
2.4. Noether Balances for Mass, Particle Number or Charge, and Energy
- Lagrangians being in accordance to the general scheme (1) or its extended form (16) are invariant with respect to the phase shift . The related density and flux density result from Noether’s theorem for the relativistic case asand in Galilean case, the same formula with in place of , respectively. The physical interpretation of is different in both cases: in Schrödinger’s theory, is the mass or particle density, while in Klein–Gordon theory, it is interpreted as charge density.
- In general, translation invariance is violated if an external potential V is considered according to (16). However, if V depends only on spatial coordinates, i.e., , then the time translation remains as a symmetry transformation of the Lagrangian. The energy density and associated energy flux density (Poynting vector) result from Noether’s theorem as
2.5. Transition Conditions at Discontinuities
3. Results
3.1. Relationships between Schrödinger’s and Klein–Gordon’s Theory
3.1.1. Discovery of a Common ‘Blueprint’ of Schrödinger’s and Klein–Gordon’s Lagrangian
3.1.2. Noether Density and Flux Associated to a Phase Shift
3.1.3. Energy Density and Poynting Vector
3.1.4. Discussion
3.2. Continuity Conditions at Discontinuities of the Potential
3.2.1. Transition Conditions Resulting Directly from Hamilton’s Principle
3.2.2. Conservation Conditions Resulting from Extended Noether’s Theorem
3.2.3. Discussion
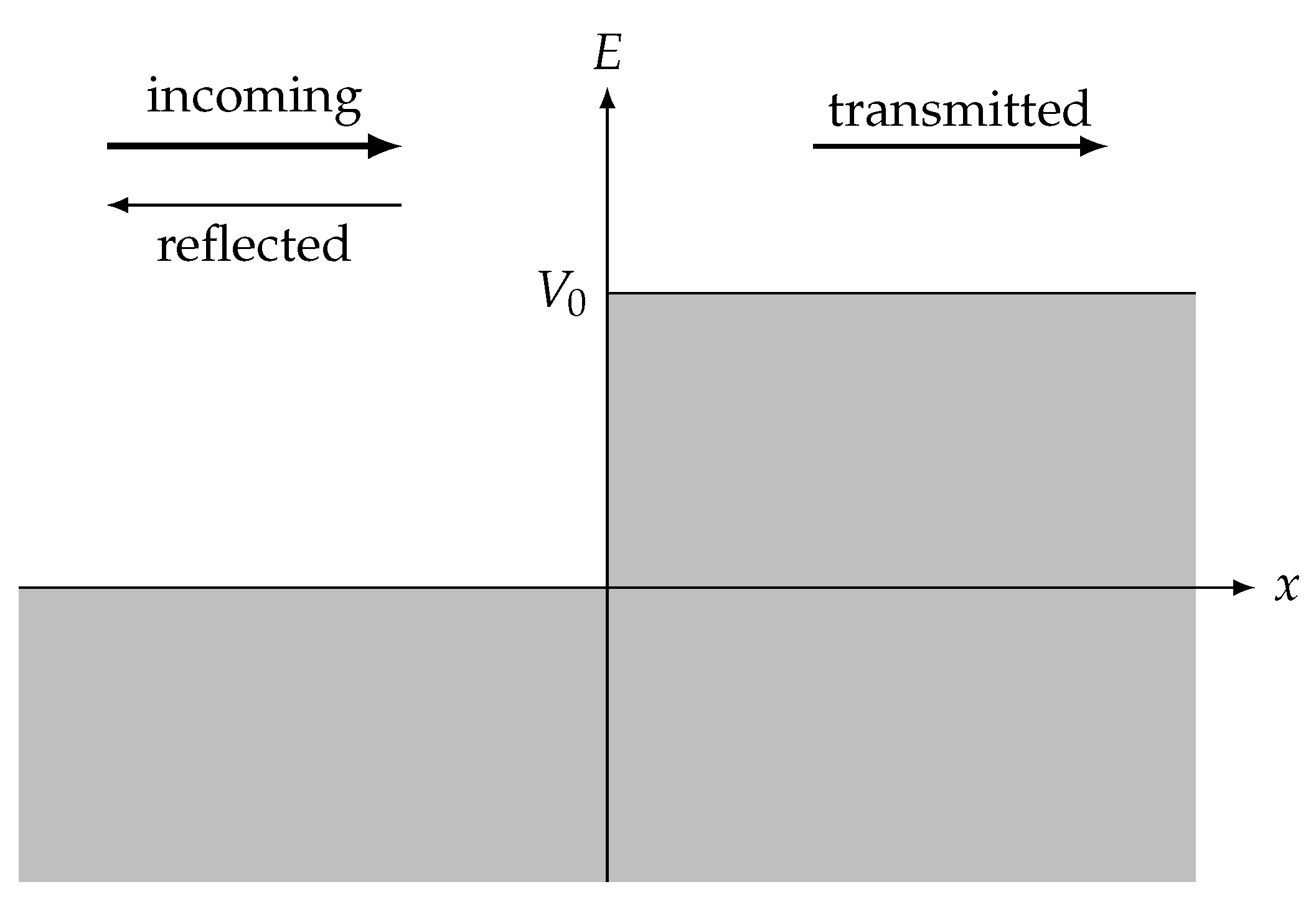
3.3. Scattering of a Bimodal Particle Beam at a Step Potential
3.3.1. State Function for Bimodal Particle Beam
3.3.2. Relationships between , and
3.3.3. Transition Conditions and Solutions
3.3.4. Considerations about Non-Bimodal Particle Beams
3.3.5. Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| c.c. | complex conjugate |
| PDE | partial differential equation |
| NTC | natural transition condition |
| MCC | methodical continuity condition |
| EBSD | electron backscatter diffraction |
References
- Kluwick, A. Shock discontinuities: From classical to non-classical shocks. Acta Mech. 2018, 229, 515–533. [Google Scholar] [CrossRef]
- Gavrilyuk, S.L.; Gouin, H. Rankine-Hugoniot conditions for fluids whose energy depends on space and time derivatives of density. Wave Motion 2020, 98. [Google Scholar] [CrossRef]
- Jordan, P.M.; Saccomandi, G.; Parnell, W.J. The sesquicentennial of Rankine’s On the Thermodynamic Theory of Waves of Finite Longitudinal Disturbance: Recent advances in nonlinear acoustics and gas dynamics. Wave Motion 2021, 102. [Google Scholar] [CrossRef]
- Davydov, A.; Haar, D. Quantum Mechanics; International series in natural philosophy; Elsevier Science & Technology Books: Amsterdam, The Netherlands, 1976. [Google Scholar]
- Branson, D. Continuity conditions on Schrödinger wave functions at discontinuities of the potential. Am. J. Phys. 1979, 47, 1000–1003. [Google Scholar] [CrossRef]
- Andrews, M. Matching conditions on wave-functions at discontinuities of the potential. Am. J. Phys. 1981, 49, 281–282. [Google Scholar] [CrossRef]
- Noether, E. Invariante Variationsprobleme. Nachr. d. König. Gesellsch. d. Wiss. zu Göttingen 1918, 235–257. Available online: https://gdz.sub.uni-goettingen.de/id/PPN252457811_1918 (accessed on 26 August 2023).
- Anthony, K.H. Hamilton’s action principle and thermodynamics of irreversible processes—A unifying procedure for reversible and irreversible processes. J. Non-Newt. Fluid Mech. 2001, 96, 291–339. [Google Scholar] [CrossRef]
- Scholle, M. Construction of Lagrangians in continuum theories. Proc. R. Soc. Lond. Math. Phys. Eng. Sci. 2004, 460, 3241–3260. [Google Scholar] [CrossRef]
- Scholle, M.; Marner, F. A non-conventional discontinuous Lagrangian for viscous flow. R. Soc. Open Sci. 2017, 4, 160447. [Google Scholar] [CrossRef]
- Mellmann, M.; Scholle, M. Symmetries and Related Physical Balances for Discontinuous Flow Phenomena within the Framework of Lagrange Formalism. Symmetry 2021, 13, 1662. [Google Scholar] [CrossRef]
- Marner, F.; Scholle, M.; Herrmann, D.; Gaskell, P.H. Competing Lagrangians for incompressible and compressible viscous flow. R. Soc. Open Sci. 2019, 6, 181595. [Google Scholar] [CrossRef]
- Scholle, M. A discontinuous variational principle implying a non-equilibrium dispersion relation for damped acoustic waves. Wave Motion 2020, 98, 102636. [Google Scholar] [CrossRef]
- Scholle, M. A weakly nonlinear wave equation for damped acoustic waves with thermodynamic non-equilibrium effects. Wave Motion 2022, 109, 102876. [Google Scholar] [CrossRef]
- Rund, H. The Hamilton-Jacobi Theory in the Calculus of Variations: Its Role in Mathematics and Physics; Krieger Publishing Company: Malabar, FL, USA, 1966. [Google Scholar]
- Madelung, E. Quantentheorie in hydrodynamischer Form. Z. für Phys. 1927, 40, 322–326. [Google Scholar] [CrossRef]
- Scholle, M. Das Hamiltonsche Prinzip in der Kontinuumstheorie Nichtdissipativer und Dissipativer Systeme: Ein Neues Konzept zur Konstruktion von Lagrangedichten. Ph.D. Thesis, University of Paderborn, Paderborn, Germany, 1999. Available online: https://worldcat.org/de/title/76110070 (accessed on 26 August 2023).
- Noether, E. Invariant variation problems. Transp. Theory Stat. Phys. 1971, 1, 186–207. [Google Scholar] [CrossRef]
- Schmutzer, E. Symmetrien und Erhaltungssätze der Physik; 75: Reihe Mathematik und Physik, Akad.-Verl.: Berlin, Germany, 1972. [Google Scholar]
- Corson, E.M. Introduction to Tensors, Spinors and Relativistic Wave-Equations: Relation Structure; Hafner: New York, NY, USA, 1953. [Google Scholar]
- Greiner, W.; Bromley, D. Relativistic Quantum Mechanics. Wave Equations; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Becker, H.; Hielscher, R.; Leineweber, A. Interplay between Habit Plane and Orientation Relationship in an Electron Backscatter Diffraction Analysis: Using the Example of η′-Al8Fe3 in η-Al5Fe2. Crystals 2022, 12, 813. [Google Scholar] [CrossRef]
- Náraigh, L.Ó.; O’Kiely, D. Homogenization theory for periodic potentials in the Schrödinger equation. Eur. J. Phys. 2012, 34, 19. [Google Scholar] [CrossRef]
- Sun, R.H. A study of imaging in the existence of resonance with multiple scattering in isotropic homogeneous media. In Proceedings of the Computational Imaging VII; Petruccelli, J.C., Preza, C., Eds.; International Society for Optics and Photonics, SPIE: Bellingham, WA, USA, 2023; Volume 12523, p. 1252307. [Google Scholar] [CrossRef]
- Ritz, W. Über eine neue Methode zur Lösung gewisser Variationsprobleme der mathematischen Physik. J. für die Reine Angew. Math. 1908, 135, 1–61. [Google Scholar] [CrossRef]
- Lin, C.C. Hydrodynamics of Helium II. In Proceedings of the International School of Physics “Enrico Fermi”; Academic Press: New York, NY, USA, 1963; Volume 21. [Google Scholar]
- Seliger, R.L.; Whitham, G.B. Variational principles in continuum mechanics. Proc. R. Soc. Lond. Ser. Math. Phys. Sci. 1968, 305, 1–25. [Google Scholar]
- Zuckerwar, A.J.; Ash, R.L. Variational approach to the volume viscosity of fluids. Phys. Fluids 2006, 18, 047101. [Google Scholar] [CrossRef]
- Salmon, R. Hamiltonian fluid mechanics. Annu. Rev. Fluid Mech. 1988, 20, 225–256. [Google Scholar] [CrossRef]
- Andersson, N.; Comer, G.L. Relativistic Fluid Dynamics: Physics for Many Different Scales. Living Rev. Relativ. 2007, 10, 1. [Google Scholar] [CrossRef] [PubMed]
- Yang, X.; Wu, L.; Zhang, H. A space-time spectral order sinc-collocation method for the fourth-order nonlocal heat model arising in viscoelasticity. Appl. Math. Comput. 2023, 457, 128192. [Google Scholar] [CrossRef]
- Prix, R. Variational description of multifluid hydrodynamics: Uncharged fluids. Phys. Rev. D 2004, 69, 043001. [Google Scholar] [CrossRef]
- Jiang, X.; Wang, J.; Wang, W.; Zhang, H. A Predictor-Corrector Compact Difference Scheme for a Nonlinear Fractional Differential Equation. Fractal Fract. 2023, 7, 521. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Scholle, M.; Mellmann, M. A Relationship between the Schrödinger and Klein–Gordon Theories and Continuity Conditions for Scattering Problems. Symmetry 2023, 15, 1667. https://doi.org/10.3390/sym15091667
Scholle M, Mellmann M. A Relationship between the Schrödinger and Klein–Gordon Theories and Continuity Conditions for Scattering Problems. Symmetry. 2023; 15(9):1667. https://doi.org/10.3390/sym15091667
Chicago/Turabian StyleScholle, Markus, and Marcel Mellmann. 2023. "A Relationship between the Schrödinger and Klein–Gordon Theories and Continuity Conditions for Scattering Problems" Symmetry 15, no. 9: 1667. https://doi.org/10.3390/sym15091667
APA StyleScholle, M., & Mellmann, M. (2023). A Relationship between the Schrödinger and Klein–Gordon Theories and Continuity Conditions for Scattering Problems. Symmetry, 15(9), 1667. https://doi.org/10.3390/sym15091667






