Kirchhoff Index and Degree Kirchhoff Index of Tetrahedrane-Derived Compounds
Abstract
1. Introduction
2. Preparations
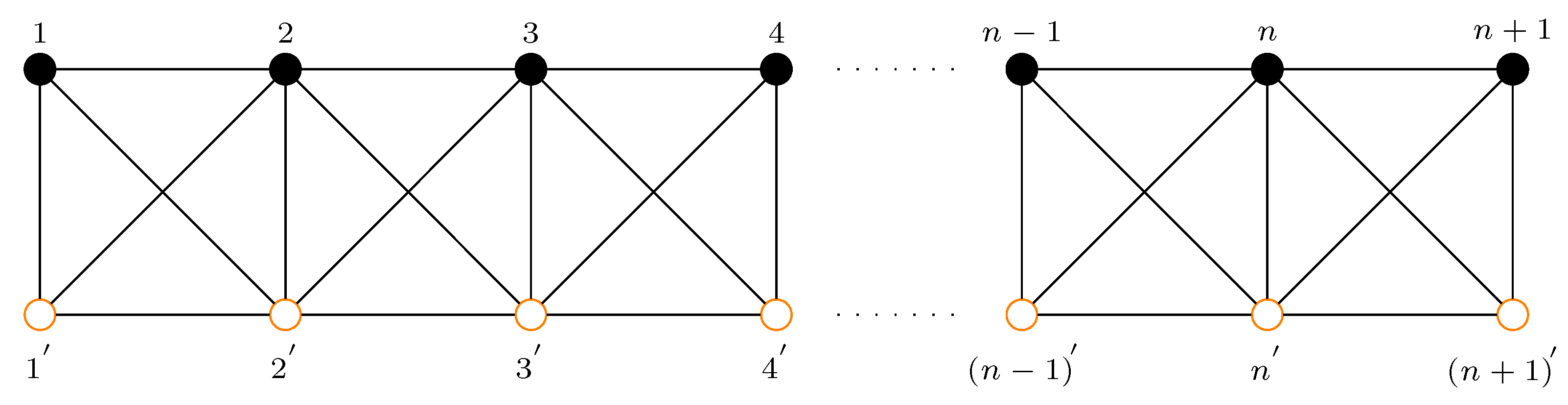
3. Kirchhoff Indexes for Linear Crossed Quadrilateral Chains
4. Degree Kirchhoff Index of Linear Crossing Quadrilateral Chains
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Klein, D.J.; Randi, M. Resistance distance. J. Math. Chem. 1993, 12, 81–95. [Google Scholar] [CrossRef]
- Ye, L. On the Kirchhoff index of some toroidal lattices. Linear Multilinear Algebra 2011, 59, 645–650. [Google Scholar] [CrossRef]
- Jing, Z.; JiaBao, L.; Sakander, H. Resistance distance-based graph invariants and the number of spanning trees of linear crossed octagonal graphs. Appl. Math. Comput. 2020, 63, 1865–2085. [Google Scholar]
- Wenrui, W. Kirchhoff Index and Degree Kirchhoff Index of Complete Multipartite Graph; Anhui University: Hefei, China, 2016. [Google Scholar]
- Liu, J.B.; Zhao, J.; Zhu, Z.; Cao, J. On the Normalized Laplacian and the Number of Spanning Trees of Linear Heptagonal Networks. Mathematics 2019, 7, 314. [Google Scholar] [CrossRef][Green Version]
- Wang, Y.; Zhang, W. Kirchhoff Index of Cyclopolyacenes. Z. Naturforschung A 2010, 65, 865–870. [Google Scholar] [CrossRef]
- Liu, H.; WU, R.; You, L. Three Types of Kirchhoff Indices in the Random Cyclooctane Chains. J. South China Norm. Univ. 2021, 53, 96–103. [Google Scholar]
- Guanghui, W.; Jiabao, L.; Jinde, C.; XiangFeng, P.; Ahmed, E. The Kirchhoff Index of Hypercubes and Related Complex Networks. Discret. Dyn. Nat. Soc. 2007, 2013, 543189. [Google Scholar]
- Yang, Y.; Zhang, H. and Klein, Douglas J. New Nordhaus-Gaddum-type results for the Kirchhoff index. J. Math. Chem. 2011, 49, 1587–1598. [Google Scholar] [CrossRef]
- Mitsuhashi, H.; Morita, H.; Sato, I. Kirchhoff Index and Additive Kirchhoff Index Based on Multiplicative Degree for a Random Polyomino Chain. Symmetry 2023, 15, 718. [Google Scholar]
- Brualdi, R.A.; Ryser, H.J. Combinatorial Matrix Theory; Cambridge University Press: Cambridge, UK, 1991. [Google Scholar]
- Huang, J.; Li, S.; Li., X. The normalized Laplacian, degree—Kirchhoff index and spanning trees of the linear polyomino chains. Appl. Math. Utation 2016, 289, 324–334. [Google Scholar] [CrossRef]
- Liu, J.; Zheng, Q.; Cai, Z.; Hayat, S. On the Laplacians and Normalized Laplacians for Graph Transformation with Respect to the Dicyclobutadieno Derivative of [n] Phenylenes. Polycycl. Aromat. Compd. 2022, 42, 1413–1434. [Google Scholar] [CrossRef]
- Feng, Z.; Huang, S.; Yan, L. On the Kirchhoff Indices and the Numbers of Spanning Trees of Two Types of Molecular Graphs. Polycycl. Aromat. Compd. 2022, 42, 316–331. [Google Scholar] [CrossRef]
- Tu, Y.; Ma, X.; Zhang, Y.; Ren, J. On the Laplacian, the Kirchhoff Index, and the Number of Spanning Trees of the Linear Pentagonal Derivation Chain. Axioms 2022, 11, 278. [Google Scholar] [CrossRef]
- Wei, S.L.; Shiu, W.C.; Ke, X.L.; Huang, J.W. Comparison of the Wiener and Kirchhoff Indices of Random Pentachains. J. Math. 2021, 2021, 7523214. [Google Scholar] [CrossRef]
- Massimo, F.; Yang, Y.J. Some Bounds for the Kirchhoff Index of Graphs. Abstr. Appl. Anal. 2013, 2014, 1085–3375. [Google Scholar]
- Sahir, M.A.; Nayeem, S.M.A. On the degree-Kirchhoff index, Gutman index and the Schultz index of pentagonal cylinder/Möbius chain. arXiv 2023, arXiv:2302.07170. [Google Scholar]
- Aouchiche, M.; Hansen, P. Distance spectra of graphs: A survey. Linear Algebra Its Appl. 2014, 458, 301–386. [Google Scholar] [CrossRef]
- Muthuraman, S.; Rajkumar, R. Spectral analysis of weighted neighborhood networks. Discret. Math. Algorithms Appl. 2022, 2022, 2250141. [Google Scholar] [CrossRef]
- Jia, Y.; Du, J.; Zhang, W. Proceedings of the 2018 Chinese Intelligent Systems Conference Volume II; Springer: Singapore, 2019. [Google Scholar]
- Li, Z.; Xie, Z.; Li, J.; Pan, Y. Resistance distance-based graph invariants and spanning trees of graphs derived from the strong prism of a star. Appl. Math. Comput. 2020, 382, 125335. [Google Scholar] [CrossRef]
- Zeng, Y.; Zhang, Z. Spectra, Hitting Times and Resistance Distances of q- Subdivision Graphs. Comput. J. 2019, 64, 76–92. [Google Scholar] [CrossRef][Green Version]
- Pan, Y.; Liu, C.; Li, J. Kirchhoff Indices and Numbers of Spanning Trees of Molecular Graphs Derived from Linear Crossed Polyomino Chain. Polycycl. Aromat. Compd. 2022, 42, 218–225. [Google Scholar] [CrossRef]
| G | K f(G) | G | K f(G) | G | K f(G) | G | K f(G) | G | K f(G) |
|---|---|---|---|---|---|---|---|---|---|
| 3.00 | 49.00 | 201.67 | 525.00 | 1083.00 | |||||
| 8.00 | 74.64 | 264.00 | 640.00 | 1266.67 | |||||
| 16.67 | 108.00 | 338.00 | 770.67 | 1470.00 | |||||
| 30.00 | 150.00 | 424.67 | 918.00 | 1694.00 |
| G | |||
|---|---|---|---|
| 16 | 331,776 | ||
| 192 | 3,981,312 | ||
| 2304 | 47,775,744 | ||
| 27,648 | 573,308,928 |
| G | G | G | |||
|---|---|---|---|---|---|
| 18 | 341.3 | 1171.3 | |||
| 51.3 | 501.3 | 1458 | |||
| 116.3 | 693 | 1776.3 | |||
| 213 | 916.3 | 2126.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, D.; Zhao, Y.; Wang, Z.; Li, X.; Zhou, K. Kirchhoff Index and Degree Kirchhoff Index of Tetrahedrane-Derived Compounds. Symmetry 2023, 15, 1122. https://doi.org/10.3390/sym15051122
Zhao D, Zhao Y, Wang Z, Li X, Zhou K. Kirchhoff Index and Degree Kirchhoff Index of Tetrahedrane-Derived Compounds. Symmetry. 2023; 15(5):1122. https://doi.org/10.3390/sym15051122
Chicago/Turabian StyleZhao, Duoduo, Yuanyuan Zhao, Zhen Wang, Xiaoxin Li, and Kai Zhou. 2023. "Kirchhoff Index and Degree Kirchhoff Index of Tetrahedrane-Derived Compounds" Symmetry 15, no. 5: 1122. https://doi.org/10.3390/sym15051122
APA StyleZhao, D., Zhao, Y., Wang, Z., Li, X., & Zhou, K. (2023). Kirchhoff Index and Degree Kirchhoff Index of Tetrahedrane-Derived Compounds. Symmetry, 15(5), 1122. https://doi.org/10.3390/sym15051122






