Topology and Emergent Symmetries in Dense Compact Star Matter
Abstract
1. Introduction
2. Hidden Symmetries and Hadron Resonances
2.1. Hidden Scale Symmetry
2.2. Hidden Local Flavor Symmetry
3. Topology Change and Hadron-Quark Continuity
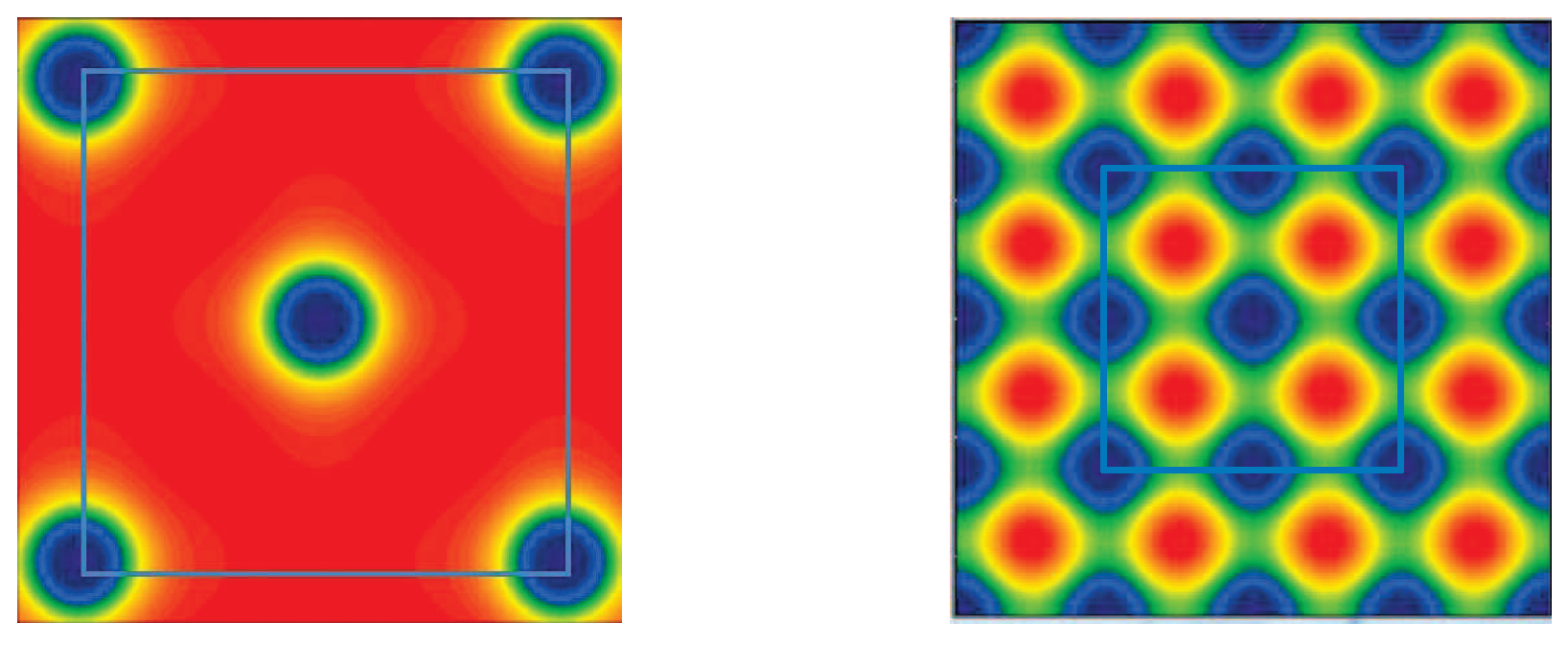
3.1. Baryons as Topology Objects and Topology Change
- Quark condensate: In skyrmion matter, the space-average of the normalized quark condensate iswith V being the volume of the crystal cell. It is found that in the skyrmion matter but in the half-skyrmion state. This implies that the quark condensate in medium—here in skyrmion matter— in the half-skyrmion matter when space-averaged.
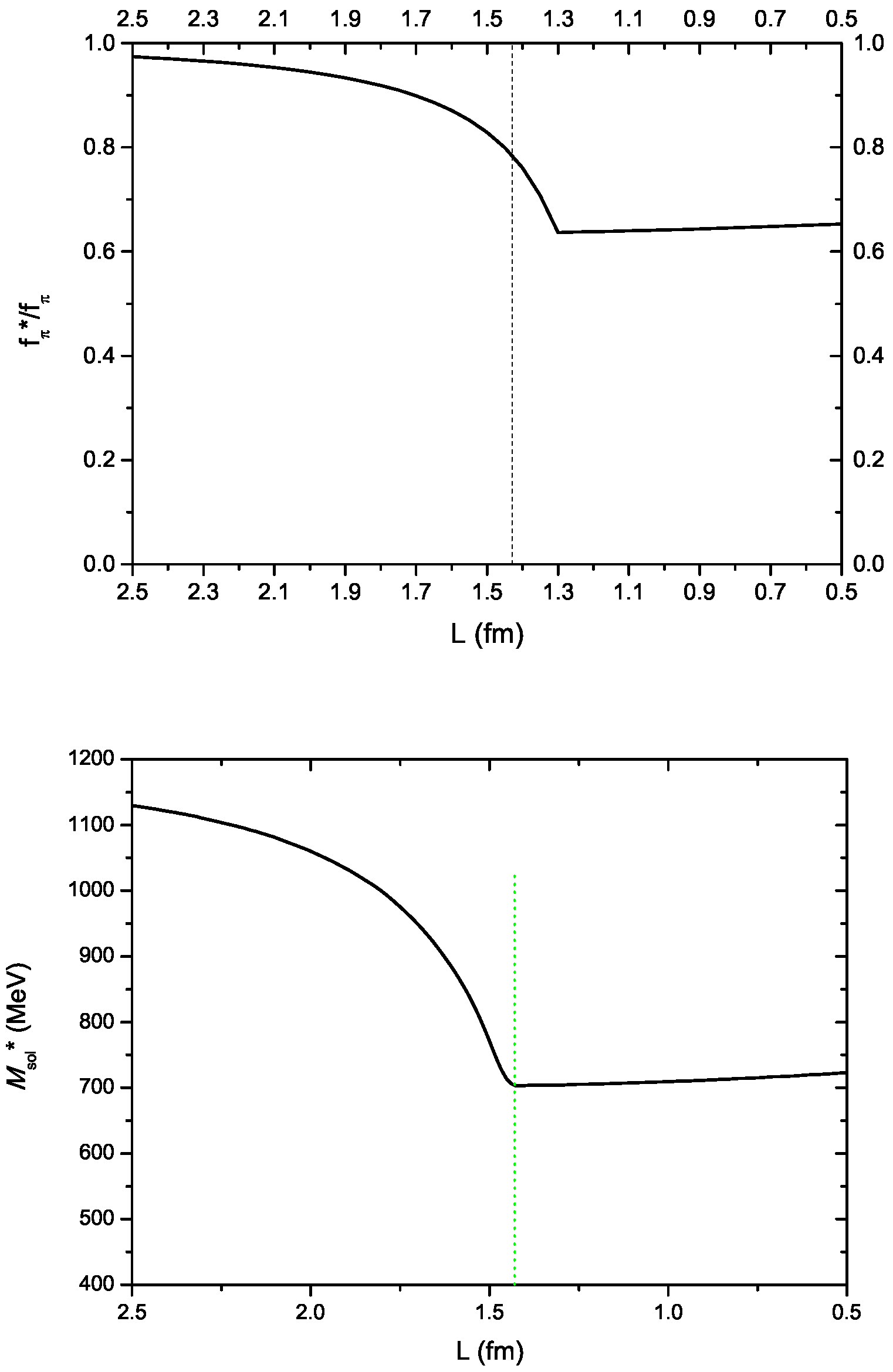
- Pion decay constant: In the skyrmion crystal approach, it is found that the medium modified pion decay constant first decreases with density until but after stays as a constant. We plot a typical result of as a function of crystal size calculated by using the HLS up to the next leading order including the homogeneous Wess–Zumino terms [84] in Figure 2. This means that in the half-skyrmion matter, although the space-averaged quark condensate vanishes, the chiral symmetry is not restored and it is still in the Nambu–Goldstone mode. Actually, in the half-skyrmion matter, the inhomogeneous quark condensate persists [85].
- Nucleon mass: By using the medium modified pion decay constant , one can calculate the density dependence of nucleon mass and obtain the scaling relationwhich, as discussed later, is consistent with the Brown–Rho scaling from the LOSS [46]. It is found that, as shown in Figure 2, similar to , —regarded as soliton mass in the large limit—first decreases with density until , after which it stays as a constant. This is predominantly, if not entirely, due to the space-averaged quark condensate going to zero at . Since in the half-skyrmion matter, , this observation indicates that the nucleon mass has decompositionthat is, there is a chiral invariant part in the nucleon mass and the parity doubling of nucleons may emerge in dense nuclear matter [86,87].
3.2. Topological Baryon for
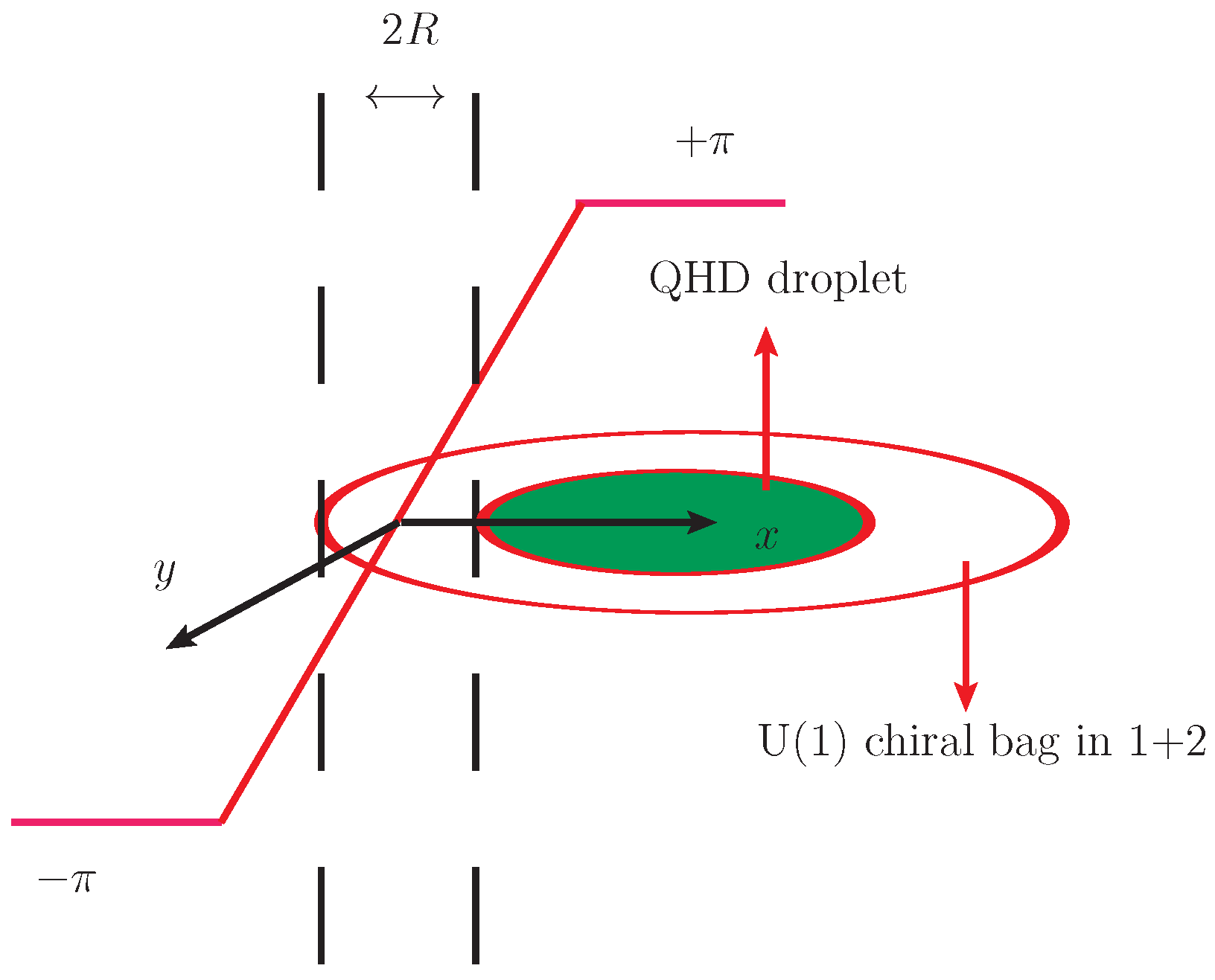
3.3. Cheshire Cat Principle and Quark-Hadron Continuity
4. Generalized Nuclear Effective Field Theory
4.1. Generalized Brown–Rho Scaling
4.2. Quenching of in Nuclei Transition
5. Equation of State of Nuclear Matter
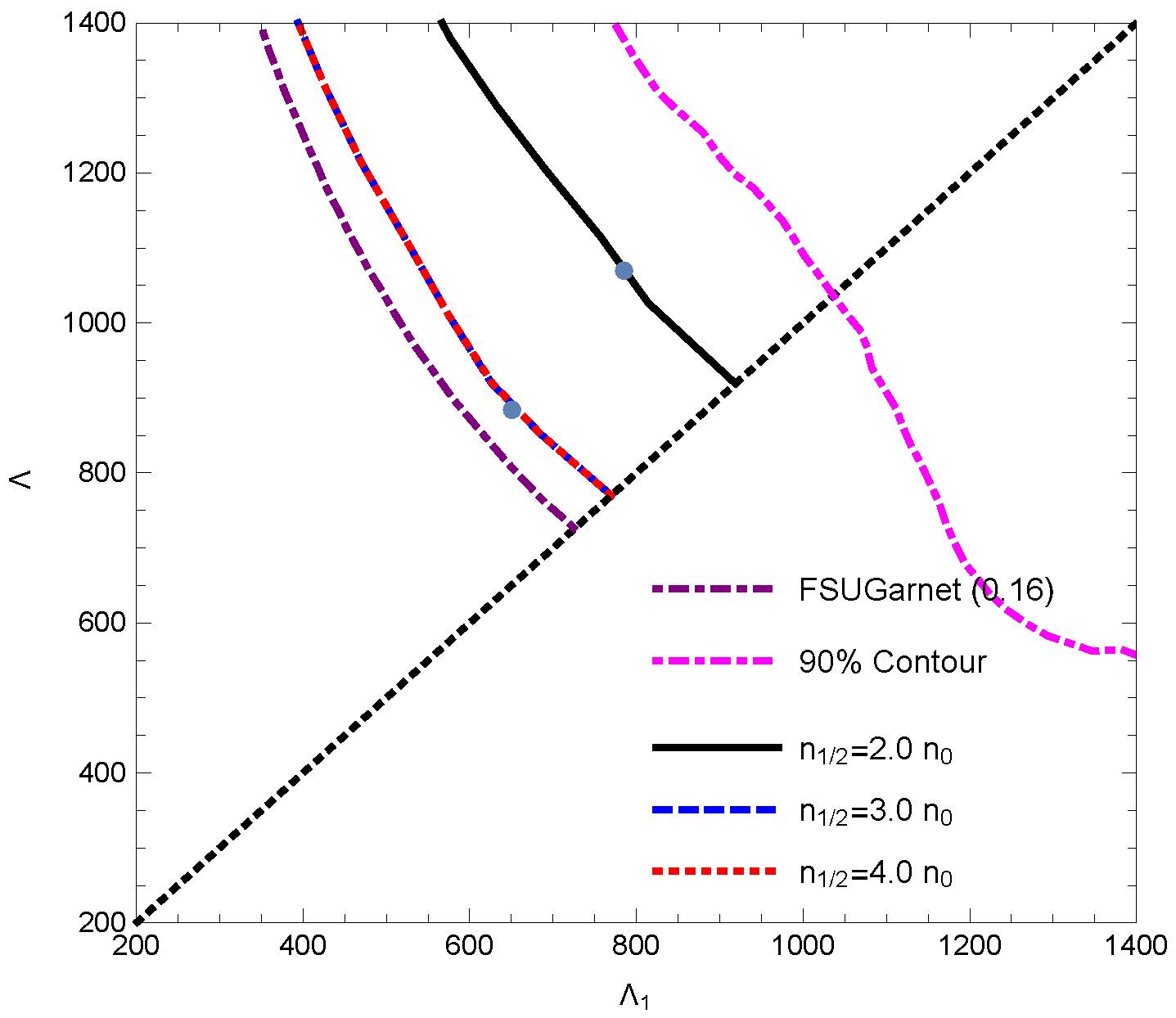
- R-I: In this region, the scaling function in the master formulism (30) decreases with density. Without first principle information on the explicit form of , we parameterize it aswith being a constant. With respect to the nuclear matter properties around saturation density [16] and the measured pion decay constant [111], the range of is found to beIn practice, to reproduce the nuclear matter properties around saturation density, it is easy to imagine that there should be fine-tuning within the range (41).
- R-II: Due to the topology change—which is one of the most robust inputs from skyrmion matter—at 2n0 the scaling behaviours of some parameters in R-II are drastically different from that in R-I. The scaling behaviours of the parameters are quite involved.
- −
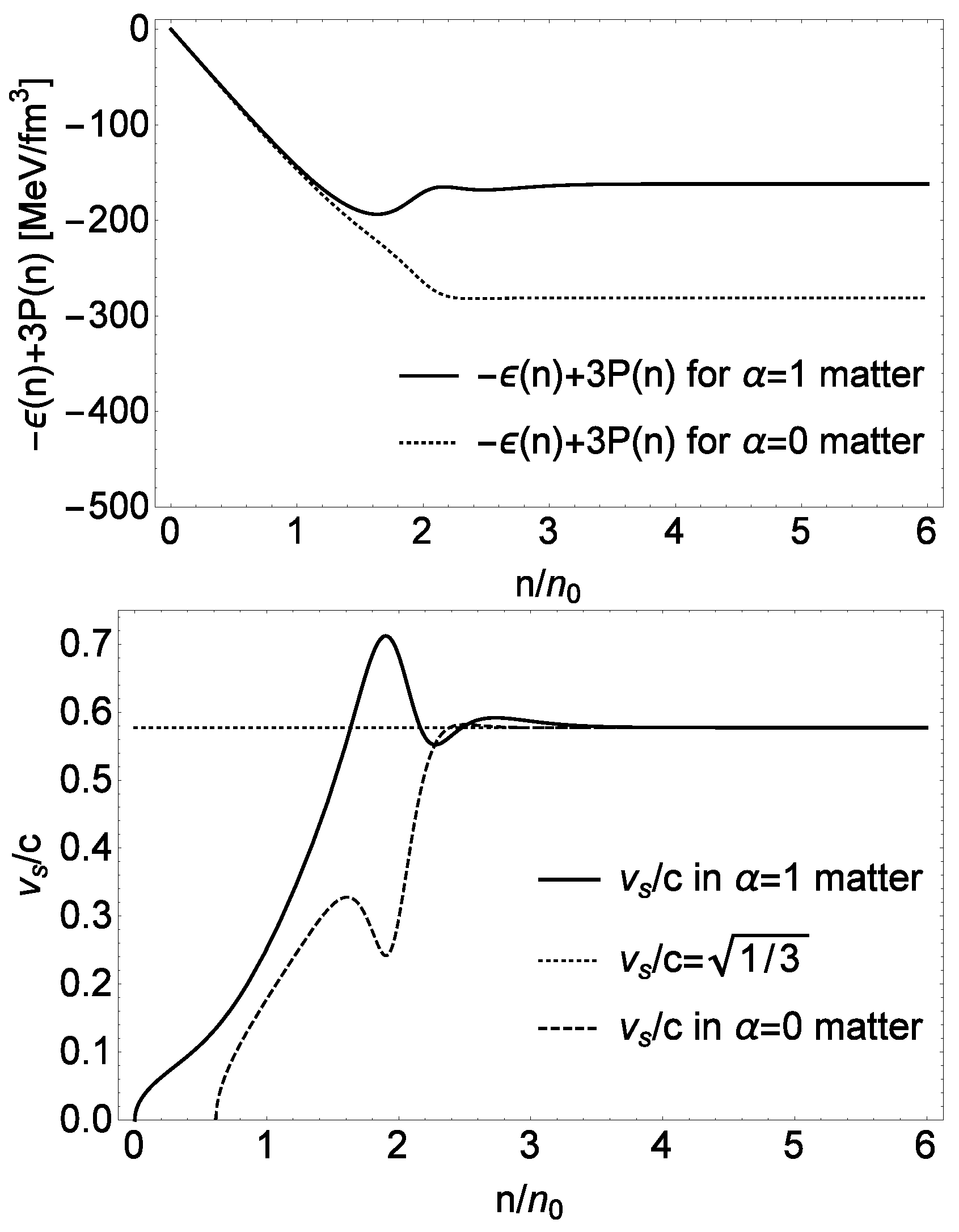
- and ρ mass: The hidden local gauge coupling related to the mass through the KSRF relation. Combined with the vector manifestation(VM) fixed-point structure of HLS this leads to the fact that for the coupling should drop to zero toward the putative VM fixed point . We take the simple form [77]where h is determined by the location of theVMfixed point , e.g., for . Where is located is not known in QCD. In compact stars, whether it is or ≳ 20n0 does not make noticeable differences with one possible exception, namely, the star sound velocity, as we will see below.
- −
- Nucleon mass: As we learned from the 1/2-skyrmion phase, the parity doubling emerges giving rise to the chiral-invariant mass m0, and the pion decay constant becomes density invariant. In the chiral-scale effective theory, they both lock to the dilaton condensate . Therefore we have
- −
- Dilaton mass: Since from the partially conserved dilatation current (PCDC) the dilaton mass is also proportional to the dilaton condensate [33], we then have
- −
- meson: The nuclear matter density dependences of the meson properties are subtle. Using the HLS, the mass is locked to the hidden gauge coupling constant. Since the HLS which works well in R-I breaks in R-II [9,71], some sort of fine-tuning is needed in the density-scaling of mass and hidden gauge coupling constant. We take it asIn the numerical calculation, we takewith .
5.1. Vector Manifestation
5.2. Pseudoconformal Structure
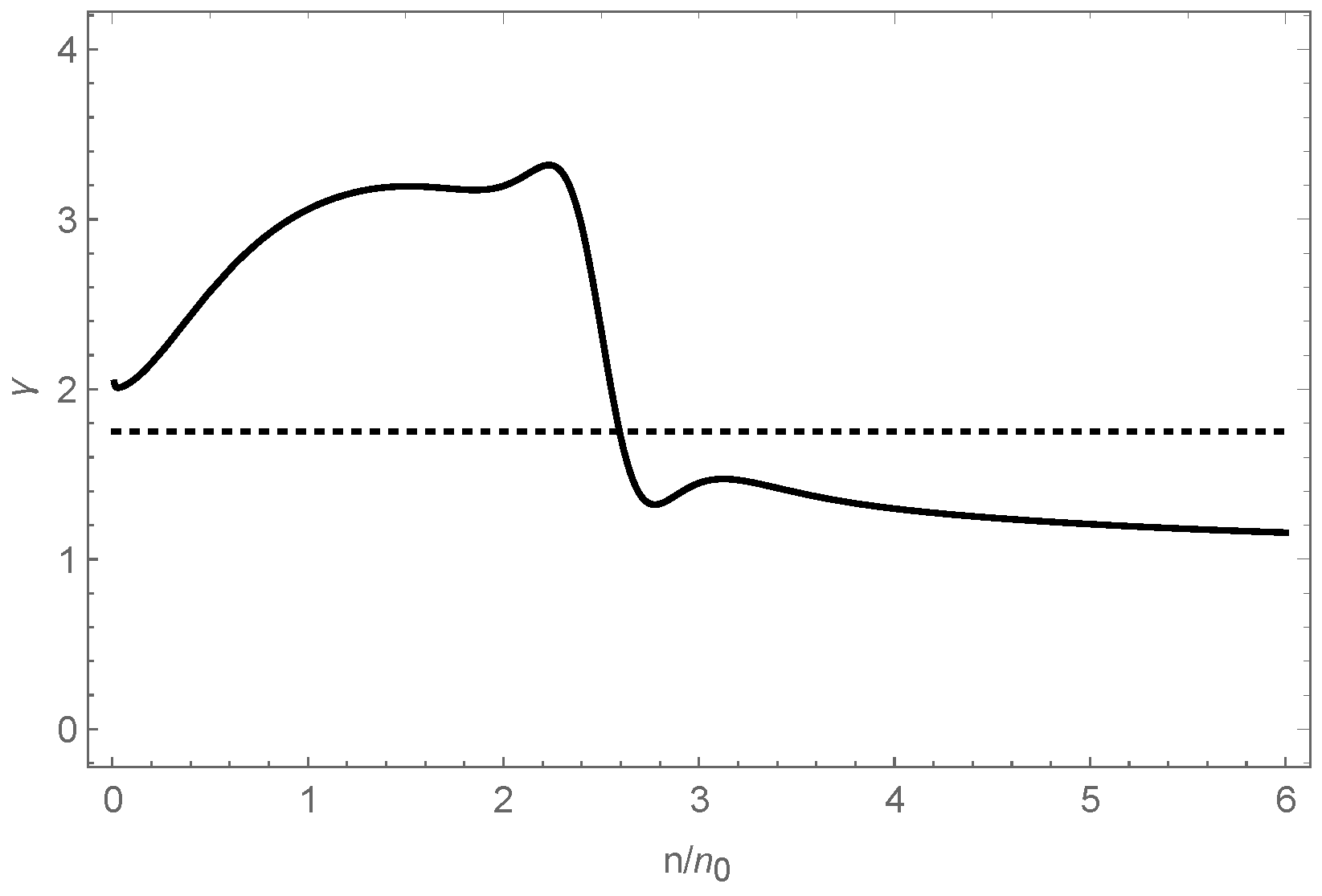
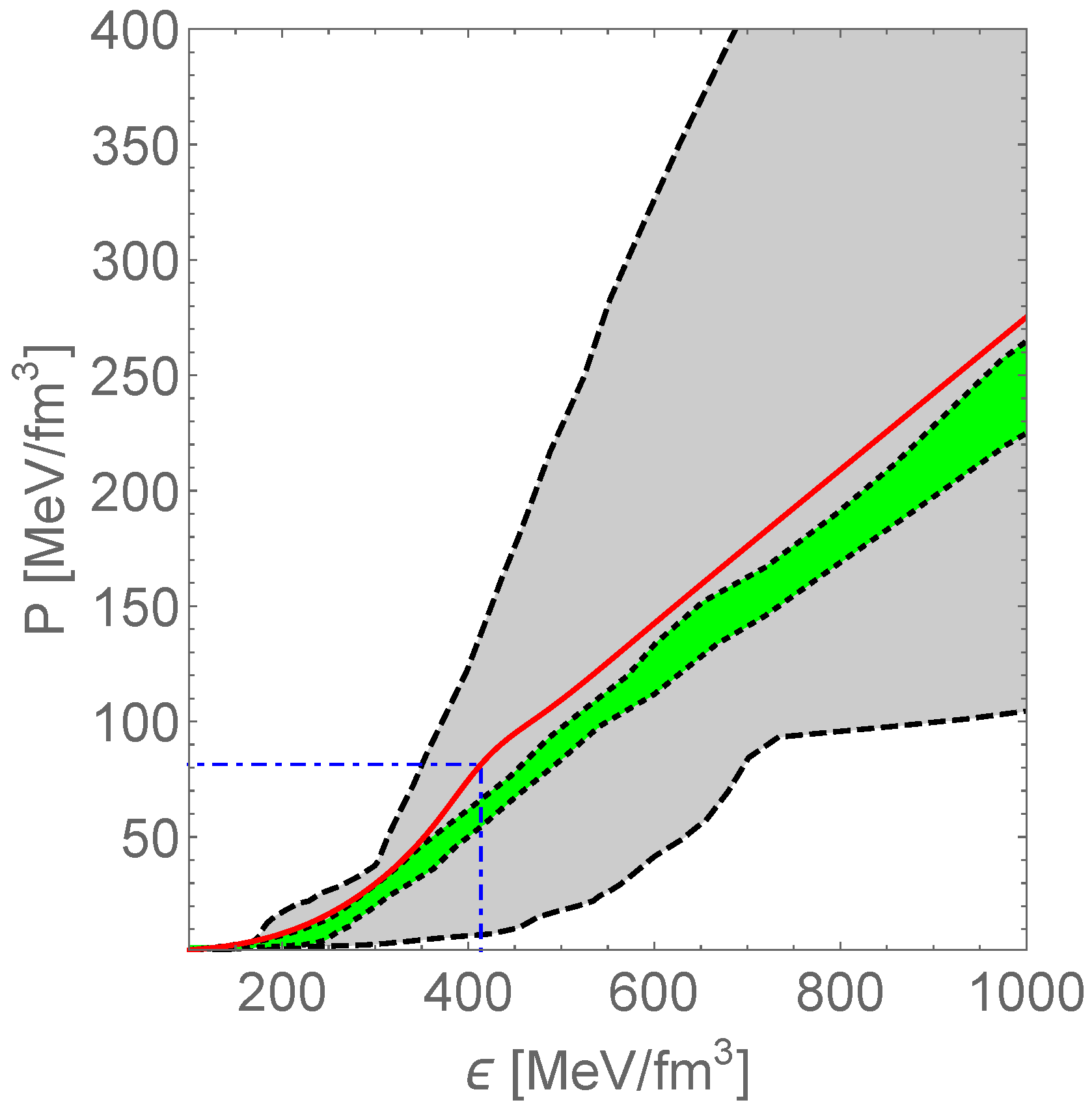
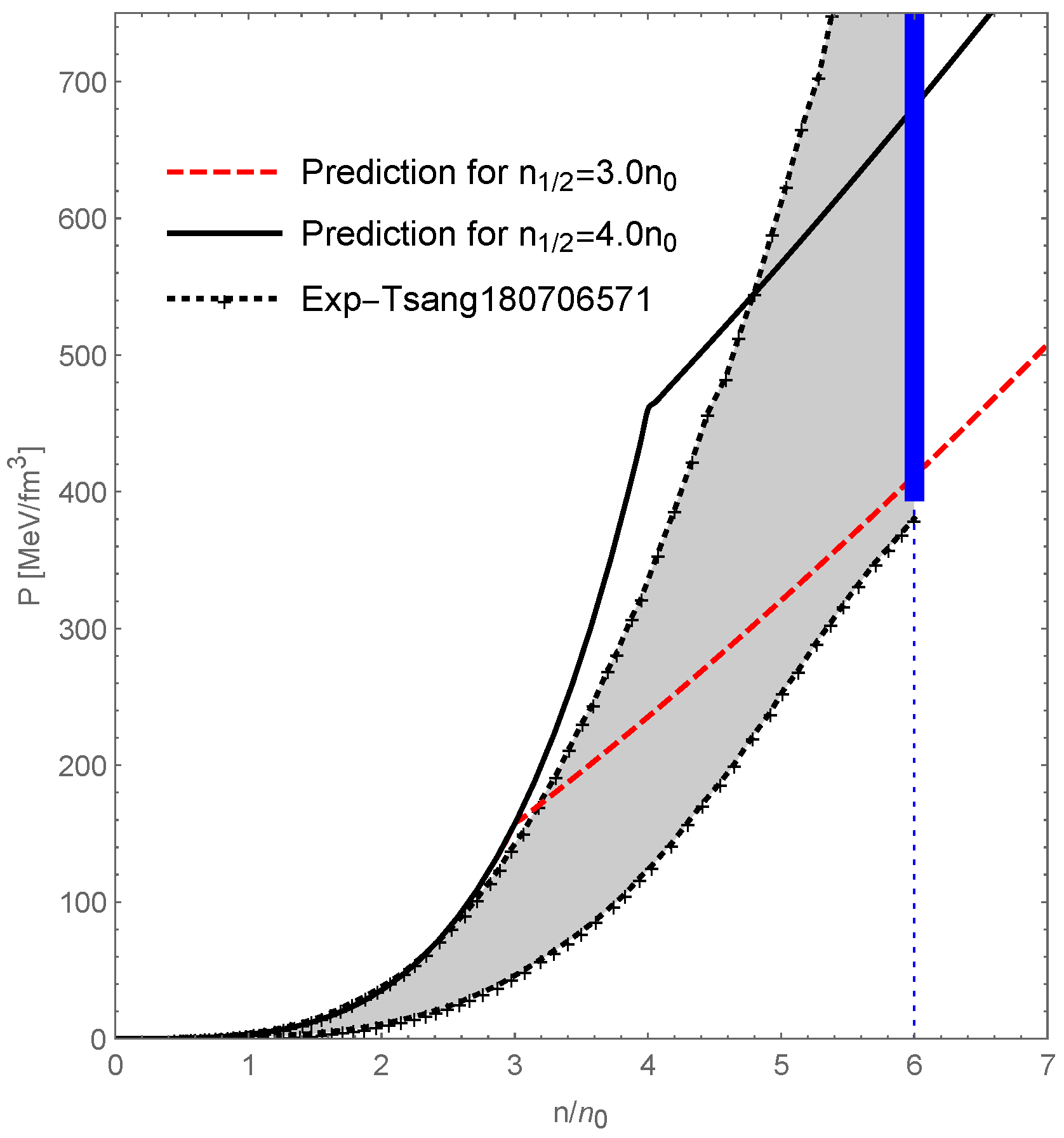
5.3. Equation of State
6. Star Properties and Gravitational Waves
7. Summary and Perspective
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brown, G.E.; Rho, M. On the manifestation of chiral symmetry in nuclei and dense nuclear matter. Phys. Rep. 2002, 363, 85–171. [Google Scholar] [CrossRef]
- Holt, J.W.; Rho, M.; Weise, W. Chiral symmetry and effective field theories for hadronic, nuclear and stellar matter. Phys. Rept. 2016, 621, 2–75. [Google Scholar] [CrossRef]
- Drews, M.; Weise, W. Functional renormalization group studies of nuclear and neutron matter. Prog. Part. Nucl. Phys. 2017, 93, 69–107. [Google Scholar] [CrossRef]
- Baym, G.; Hatsuda, T.; Kojo, T.; Powell, P.D.; Song, Y.; Takatsuka, T. From hadrons to quarks in neutron stars: A review. Rep. Prog. Phys. 2018, 81, 056902. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Towards the hadron–quark continuity via a topology change in compact stars. Prog. Part. Nucl. Phys. 2020, 113, 103791. [Google Scholar] [CrossRef]
- Li, B.A.; Krastev, P.G.; Wen, D.H.; Zhang, N.B. Towards Understanding Astrophysical Effects of Nuclear Symmetry Energy. Eur. Phys. J. A 2019, 55, 117. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Dichotomy of Baryons as Quantum Hall Droplets and Skyrmions: Topological Structure of Dense Matter. Symmetry 2021, 13, 1888. [Google Scholar] [CrossRef]
- Lovato, A.; Dore, T.; Pisarski, R.D.; Schenke, B.; Chatziioannou, K.; Read, J.S.; Landry, P.; Danielewicz, P.; Lee, D.; Pratt, S.; et al. Long Range Plan: Dense matter theory for heavy-ion collisions and neutron stars. arXiv 2022, arXiv:2211.02224. [Google Scholar]
- Paeng, W.G.; Kuo, T.T.S.; Lee, H.K.; Rho, M. Scale-Invariant Hidden Local Symmetry, Topology Change and Dense Baryonic Matter. Phys. Rev. C 2016, 93, 055203. [Google Scholar] [CrossRef]
- Paeng, W.G.; Kuo, T.T.S.; Lee, H.K.; Ma, Y.L.; Rho, M. Scale-invariant hidden local symmetry, topology change, and dense baryonic matter. II. Phys. Rev. D 2017, 96, 014031. [Google Scholar] [CrossRef]
- Ma, Y.L.; Lee, H.K.; Paeng, W.G.; Rho, M. Pseudoconformal equation of state in compact-star matter from topology change and hidden symmetries of QCD. Sci. China Phys. Mech. Astron. 2019, 62, 112011. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Pseudoconformal structure in dense baryonic matter. Phys. Rev. D 2019, 99, 014034. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Sound velocity and tidal deformability in compact stars. Phys. Rev. D 2019, 100, 114003. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. What’s in the core of massive neutron stars? arXiv 2020, arXiv:2006.14173. [Google Scholar]
- Rho, M.; Ma, Y.L. Manifestation of Hidden Symmetries in Baryonic Matter: From Finite Nuclei to Neutron Stars. Mod. Phys. Lett. A 2021, 36, 2130012. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Topology change, emergent symmetries and compact star matter. AAPPS Bull. 2021, 31, 16. [Google Scholar] [CrossRef]
- Lee, H.K.; Ma, Y.L.; Paeng, W.G.; Rho, M. Cusp in the symmetry energy, speed of sound in neutron stars and emergent pseudo-conformal symmetry. Mod. Phys. Lett. A 2022, 37, 2230003. [Google Scholar] [CrossRef]
- Rho, M. Mapping Topology of Skyrmions and Fractional Quantum Hall Droplets to Nuclear EFT for Ultra-Dense Baryonic Matter. Symmetry 2022, 14, 994. [Google Scholar] [CrossRef]
- Bedaque, P.; Steiner, A.W. Sound velocity bound and neutron stars. Phys. Rev. Lett. 2015, 114, 031103. [Google Scholar] [CrossRef]
- Tews, I.; Carlson, J.; Gandolfi, S.; Reddy, S. Constraining the speed of sound inside neutron stars with chiral effective field theory interactions and observations. Astrophys. J. 2018, 860, 149. [Google Scholar] [CrossRef]
- Moustakidis, C.C.; Gaitanos, T.; Margaritis, C.; Lalazissis, G.A. Bounds on the speed of sound in dense matter, and neutron star structure. Phys. Rev. C 2017, 95, 045801, Erratum: Phys. Rev. C 2017, 95, 059904. [Google Scholar] [CrossRef]
- Alsing, J.; Silva, H.O.; Berti, E. Evidence for a maximum mass cut-off in the neutron star mass distribution and constraints on the equation of state. Mon. Not. R. Astron. Soc. 2018, 478, 1377–1391. [Google Scholar] [CrossRef]
- McLerran, L.; Reddy, S. Quarkyonic Matter and Neutron Stars. Phys. Rev. Lett. 2019, 122, 122701. [Google Scholar] [CrossRef] [PubMed]
- Jeong, K.S.; McLerran, L.; Sen, S. Dynamically generated momentum space shell structure of quarkyonic matter via an excluded volume model. Phys. Rev. C 2020, 101, 035201. [Google Scholar] [CrossRef]
- Kapusta, J.I.; Welle, T. Neutron stars with a crossover equation of state. Phys. Rev. C 2021, 104, L012801. [Google Scholar] [CrossRef]
- Zhao, T.; Lattimer, J.M. Quarkyonic Matter Equation of State in Beta-Equilibrium. Phys. Rev. D 2020, 102, 023021. [Google Scholar] [CrossRef]
- Margueron, J.; Hansen, H.; Proust, P.; Chanfray, G. Quarkyonic stars with isospin-flavor asymmetry. Phys. Rev. C 2021, 104, 055803. [Google Scholar] [CrossRef]
- Fujimoto, Y.; Fukushima, K.; McLerran, L.D.; Praszalowicz, M. Trace anomaly as signature of conformality in neutron stars. Phys. Rev. Lett. 2022, 129, 252702. [Google Scholar] [CrossRef]
- Marczenko, M.; McLerran, L.; Redlich, K.; Sasaki, C. Reaching percolation and conformal limits in neutron stars. Phys. Rev. C 2022, 107, 025802. [Google Scholar] [CrossRef]
- Bando, M.; Kugo, T.; Uehara, S.; Yamawaki, K.; Yanagida, T. Is rho Meson a Dynamical Gauge Boson of Hidden Local Symmetry? Phys. Rev. Lett. 1985, 54, 1215. [Google Scholar] [CrossRef]
- Bando, M.; Kugo, T.; Yamawaki, K. Nonlinear Realization and Hidden Local Symmetries. Phys. Rep. 1988, 164, 217–314. [Google Scholar] [CrossRef]
- Harada, M.; Yamawaki, K. Hidden local symmetry at loop: A New perspective of composite gauge boson and chiral phase transition. Phys. Rep. 2003, 381, 1–233. [Google Scholar] [CrossRef]
- Crewther, R.J.; Tunstall, L.C. ΔI = 1/2 rule for kaon decays derived from QCD infrared fixed point. Phys. Rev. D 2015, 91, 034016. [Google Scholar] [CrossRef]
- Catà, O.; Crewther, R.J.; Tunstall, L.C. Crawling technicolor. Phys. Rev. D 2019, 100, 095007. [Google Scholar] [CrossRef]
- Crewther, R.J. Genuine Dilatons in Gauge Theories. Universe 2020, 6, 96. [Google Scholar] [CrossRef]
- Skyrme, T.H.R. A Nonlinear field theory. Proc. R. Soc. Lond. A 1961, 260, 127–138. [Google Scholar] [CrossRef]
- Kugler, M.; Shtrikman, S. A New Skyrmion Crystal. Phys. Lett. B 1988, 208, 491–494. [Google Scholar] [CrossRef]
- Kugler, M.; Shtrikman, S. Skyrmion Crystals and Their Symmetries. Phys. Rev. D 1989, 40, 3421. [Google Scholar] [CrossRef] [PubMed]
- Lee, H.J.; Park, B.Y.; Rho, M.; Vento, V. Sliding vacua in dense skyrmion matter. Nucl. Phys. A 2003, 726, 69–92. [Google Scholar] [CrossRef]
- Park, B.Y.; Rho, M.; Vento, V. Vector mesons and dense Skyrmion matter. Nucl. Phys. A 2004, 736, 129–145. [Google Scholar] [CrossRef]
- Park, B.Y.; Rho, M.; Vento, V. The Role of the Dilaton in Dense Skyrmion Matter. Nucl. Phys. A 2008, 807, 28–37. [Google Scholar] [CrossRef]
- Ma, Y.L.; Harada, M.; Lee, H.K.; Oh, Y.; Park, B.Y.; Rho, M. Dense baryonic matter in the hidden local symmetry approach: Half-skyrmions and nucleon mass. Phys. Rev. D 2013, 88, 014016, Erratum: Phys. Rev. D 2013, 88, 079904. [Google Scholar] [CrossRef]
- Ma, Y.L.; Harada, M.; Lee, H.K.; Oh, Y.; Park, B.Y.; Rho, M. Dense baryonic matter in conformally-compensated hidden local symmetry: Vector manifestation and chiral symmetry restoration. Phys. Rev. D 2014, 90, 034015. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Recent progress on dense nuclear matter in skyrmion approaches. Sci. China Phys. Mech. Astron. 2017, 60, 032001. [Google Scholar] [CrossRef]
- Shao, L.Q.; Ma, Y.L. Scale symmetry and composition of compact star matter. Phys. Rev. D 2022, 106, 014014. [Google Scholar] [CrossRef]
- Brown, G.E.; Rho, M. Scaling effective Lagrangians in a dense medium. Phys. Rev. Lett. 1991, 66, 2720–2723. [Google Scholar] [CrossRef] [PubMed]
- Bogner, S.K.; Kuo, T.T.S.; Schwenk, A.; Entem, D.R.; Machleidt, R. Towards a model independent low momentum nucleon nucleon interaction. Phys. Lett. B 2003, 576, 265–272. [Google Scholar] [CrossRef]
- Bogner, S.K.; Schwenk, A.; Kuo, T.T.S.; Brown, G.E. Renormalization group equation for low momentum effective nuclear interactions. arXiv 2001, arXiv:nucl-th/0111042. [Google Scholar]
- Bogner, S.K.; Kuo, T.T.S.; Schwenk, A. Model independent low momentum nucleon interaction from phase shift equivalence. Phys. Rep. 2003, 386, 1–27. [Google Scholar] [CrossRef]
- Serot, B.D.; Walecka, J.D. The Relativistic Nuclear Many Body Problem. Adv. Nucl. Phys. 1986, 16, 1–327. [Google Scholar]
- Li, F.; Cai, B.J.; Zhou, Y.; Jiang, W.Z.; Chen, L.W. Effects of Isoscalar- and Isovector-scalar Meson Mixing on Neutron Star Structure. Astrophys. J. 2022, 929, 183. [Google Scholar] [CrossRef]
- Miyatsu, T.; Cheoun, M.K.; Saito, K. Asymmetric Nuclear Matter in Relativistic Mean-field Models with Isoscalar- and Isovector-meson Mixing. Astrophys. J. 2022, 929, 82. [Google Scholar] [CrossRef]
- Workman, R.L. Review of Particle Physics. Prog. Theor. Exp. Phys. 2022, 2022, 083C01. [Google Scholar] [CrossRef]
- Isham, C.J.; Salam, A.; Strathdee, J.A. Broken chiral and conformal symmetry in an effective-lagrangian formalism. Phys. Rev. D 1970, 2, 685–690. [Google Scholar] [CrossRef]
- Ellis, J.R. Aspects of conformal symmetry and chirality. Nucl. Phys. B 1970, 22, 478–492, Erratum: Nucl. Phys. B 1971, 25, 639. [Google Scholar] [CrossRef]
- Schechter, J. Effective Lagrangian with Two Color Singlet Gluon Fields. Phys. Rev. D 1980, 21, 3393–3400. [Google Scholar] [CrossRef]
- Golterman, M.; Shamir, Y. Low-energy effective action for pions and a dilatonic meson. Phys. Rev. D 2016, 94, 054502. [Google Scholar] [CrossRef]
- Brodsky, S.J.; de Teramond, G.F.; Deur, A. Nonperturbative QCD Coupling and its β-function from Light-Front Holography. Phys. Rev. D 2010, 81, 096010. [Google Scholar] [CrossRef]
- Horsley, R.; Perlt, H.; Rakow, P.E.L.; Schierholz, G.; Schiller, A. The SU(3) Beta Function from Numerical Stochastic Perturbation Theory. Phys. Lett. B 2014, 728, 1–4. [Google Scholar] [CrossRef]
- Yu, Q.; Zhou, H.; Huang, X.D.; Shen, J.M.; Wu, X.G. Novel and Self-Consistency Analysis of the QCD Running Coupling αs(Q) in Both the Perturbative and Nonperturbative Domains. Chin. Phys. Lett. 2022, 39, 071201. [Google Scholar] [CrossRef]
- Alexandru, A.; Horváth, I. Possible New Phase of Thermal QCD. Phys. Rev. D 2019, 100, 094507. [Google Scholar] [CrossRef]
- Freund, P.G.O.; Nambu, Y. Scalar field coupled to the trace of the energy-momentum tensor. Phys. Rev. 1968, 174, 1741–1743. [Google Scholar] [CrossRef]
- Fubini, S. A New Approach to Conformal Invariant Field Theories. Nuovo Cim. A 1976, 34, 521. [Google Scholar] [CrossRef]
- Serra, J. A higgs-like dilaton: Viability and implications. EPJ Web Conf. 2013, 60, 17005. [Google Scholar] [CrossRef]
- Goldberger, W.D.; Grinstein, B.; Skiba, W. Distinguishing the Higgs boson from the dilaton at the Large Hadron Collider. Phys. Rev. Lett. 2008, 100, 111802. [Google Scholar] [CrossRef]
- Li, Y.L.; Ma, Y.L.; Rho, M. Chiral-scale effective theory including a dilatonic meson. Phys. Rev. D 2017, 95, 114011. [Google Scholar] [CrossRef]
- Catà, O.; Müller, C. Chiral effective theories with a light scalar at one loop. Nucl. Phys. B 2020, 952, 114938. [Google Scholar] [CrossRef]
- Li, Y.L.; Ma, Y.L.; Rho, M. Nuclear Axial Currents from Scale-Chiral Effective Field Theory. Chin. Phys. C 2018, 42, 094102. [Google Scholar] [CrossRef]
- Li, Y.L.; Ma, Y.L.; Rho, M. Nonquenching of gA in nuclei, Landau-Migdal fixed-point theory, and emergence of scale symmetry in dense baryonic matter. Phys. Rev. C 2018, 98, 044318. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Quenched gA in Nuclei and Emergent Scale Symmetry in Baryonic Matter. Phys. Rev. Lett. 2020, 125, 142501. [Google Scholar] [CrossRef]
- Paeng, W.G.; Lee, H.K.; Rho, M.; Sasaki, C. Dilaton-Limit Fixed Point in Hidden Local Symmetric Parity Doublet Model. Phys. Rev. D 2012, 85, 054022. [Google Scholar] [CrossRef]
- Georgi, H. New Realization of Chiral Symmetry. Phys. Rev. Lett. 1989, 63, 1917–1919. [Google Scholar] [CrossRef] [PubMed]
- Georgi, H. Vector Realization of Chiral Symmetry. Nucl. Phys. B 1990, 331, 311–330. [Google Scholar] [CrossRef]
- Harada, M.; Yamawaki, K. Vector manifestation of the chiral symmetry. Phys. Rev. Lett. 2001, 86, 757–760. [Google Scholar] [CrossRef] [PubMed]
- Beane, S.R.; van Kolck, U. The Dilated chiral quark model. Phys. Lett. B 1994, 328, 137–142. [Google Scholar] [CrossRef]
- Suzuki, M. Inevitable emergence of composite gauge bosons. Phys. Rev. D 2017, 96, 065010. [Google Scholar] [CrossRef]
- Yang, W.C.; Ma, Y.L. Vector manifestation, conformality and sound velocity in compact star matter. In manuscript in preparation.
- Witten, E. Baryons in the 1/n Expansion. Nucl. Phys. B 1979, 160, 57–115. [Google Scholar] [CrossRef]
- Brown, G.E.; Rho, M. (Eds.) The Multifaceted Skyrmion; World Scientific: Singapore, 2010. [Google Scholar] [CrossRef]
- Manton, N.S. Skyrmions—A Theory of Nuclei; World Scientific: Singapore, 2022. [Google Scholar] [CrossRef]
- Klebanov, I.R. Nuclear Matter in the Skyrme Model. Nucl. Phys. B 1985, 262, 133–143. [Google Scholar] [CrossRef]
- Goldhaber, A.S.; Manton, N.S. Maximal Symmetry of the Skyrme Crystal. Phys. Lett. B 1987, 198, 231–234. [Google Scholar] [CrossRef]
- Ma, Y.L.; Harada, M. Lecture notes on the Skyrme model. arXiv 2016, arXiv:1604.04850. [Google Scholar]
- Ma, Y.L.; Harada, M.; Lee, H.K.; Oh, Y.; Rho, M. Skyrmions, half-skyrmions and nucleon mass in dense baryonic matter. Int. J. Mod. Phys. Conf. Ser. 2014, 29, 1460238. [Google Scholar] [CrossRef]
- Harada, M.; Lee, H.K.; Ma, Y.L.; Rho, M. Inhomogeneous quark condensate in compressed Skyrmion matter. Phys. Rev. D 2015, 91, 096011. [Google Scholar] [CrossRef]
- Detar, C.E.; Kunihiro, T. Linear σ Model with Parity Doubling. Phys. Rev. D 1989, 39, 2805. [Google Scholar] [CrossRef] [PubMed]
- Motohiro, Y.; Kim, Y.; Harada, M. Asymmetric nuclear matter in a parity doublet model with hidden local symmetry. Phys. Rev. C 2015, 92, 025201, Erratum: Phys. Rev. C 2017, 95, 059903. [Google Scholar] [CrossRef]
- Komargodski, Z. Baryons as Quantum Hall Droplets. arXiv 2018, arXiv:1812.09253. [Google Scholar]
- Hsin, P.S.; Seiberg, N. Level/rank Duality and Chern-Simons-Matter Theories. J. High Energy Phys. 2016, 9, 95. [Google Scholar] [CrossRef]
- Gaiotto, D.; Komargodski, Z.; Seiberg, N. Time-reversal breaking in QCD4, walls, and dualities in 2 + 1 dimensions. J. High Energy Phys. 2018, 1, 110. [Google Scholar] [CrossRef]
- Benini, F. Three-dimensional dualities with bosons and fermions. J. High Energy Phys. 2018, 2, 68. [Google Scholar] [CrossRef]
- Tong, D. Lectures on the Quantum Hall Effect. arXiv 2016, arXiv:1606.06687. [Google Scholar]
- Karasik, A. Skyrmions, Quantum Hall Droplets, and one current to rule them all. SciPost Phys. 2020, 9, 8. [Google Scholar] [CrossRef]
- Bigazzi, F.; Cotrone, A.L.; Olzi, A. Hall Droplet Sheets in Holographic QCD. J. High Energ. Phys. 2023, 194. [Google Scholar] [CrossRef]
- Liu, X.H.; Ma, Y.L.; Rho, M. Topology change and nuclear symmetry energy in compact-star matter. Phys. Rev. C 2019, 99, 055808. [Google Scholar] [CrossRef]
- Ma, Y.L.; Nowak, M.A.; Rho, M.; Zahed, I. Baryon as a Quantum Hall Droplet and the Cheshire Cat Principle. Phys. Rev. Lett. 2019, 123, 172301. [Google Scholar] [CrossRef] [PubMed]
- Callan, C.G., Jr.; Harvey, J.A. Anomalies and Fermion Zero Modes on Strings and Domain Walls. Nucl. Phys. B 1985, 250, 427–436. [Google Scholar] [CrossRef]
- Pisarski, R.D.; Wilczek, F. Remarks on the Chiral Phase Transition in Chromodynamics. Phys. Rev. D 1984, 29, 338–341. [Google Scholar] [CrossRef]
- Park, B.Y.; Paeng, W.G.; Vento, V. The Inhomogeneous Phase of Dense Skyrmion Matter. Nucl. Phys. A 2019, 989, 231–245. [Google Scholar] [CrossRef]
- Canfora, F. Ordered arrays of Baryonic tubes in the Skyrme model in (3 + 1) dimensions at finite density. Eur. Phys. J. C 2018, 78, 929. [Google Scholar] [CrossRef]
- Rho, M. Probing Fractional Quantum Hall Sheets in Dense Baryonic Matter. arXiv 2022, arXiv:2211.14890. [Google Scholar]
- Sulejmanpasic, T.; Shao, H.; Sandvik, A.; Unsal, M. Confinement in the bulk, deconfinement on the wall: Infrared equivalence between compactified QCD and quantum magnets. Phys. Rev. Lett. 2017, 119, 091601. [Google Scholar] [CrossRef]
- Senthil, T.; Vishwanath, A.; Balents, L.; Sachdev, S.; Fisher, M.P.A. Deconfined Quantum Critical Points. Science 2004, 303, 1490–1494. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.L.; Ma, Y.L. Derivation of Brown–Rho Scaling from Scale-Chiral Perturbation Theory. 2017. Available online: https://arxiv.org/abs/1710.02839 (accessed on 6 February 2023).
- Ma, Y.L.; Rho, M. Scale-chiral symmetry, ω meson and dense baryonic matter. Phys. Rev. D 2018, 97, 094017. [Google Scholar] [CrossRef]
- Wilkinson, D.H. Renormalization of the Axial-Vector Coupling Constant in Nuclear beta Decay. Phys. Rev. C 1973, 7, 930–936. [Google Scholar] [CrossRef]
- Suhonen, J.T. Value of the Axial-Vector Coupling Strength in β and ββ Decays: A Review. Front. Phys. 2017, 5, 55. [Google Scholar] [CrossRef]
- Engel, J.; Menéndez, J. Status and Future of Nuclear Matrix Elements for Neutrinoless Double-Beta Decay: A Review. Rep. Prog. Phys. 2017, 80, 046301. [Google Scholar] [CrossRef] [PubMed]
- Rho, M. How and How Much is gA\it Fundamentally Quenched in Nuclei? arXiv 2022, arXiv:2212.05558. [Google Scholar]
- Friman, B.; Rho, M. From chiral Lagrangians to Landau Fermi liquid theory of nuclear matter. Nucl. Phys. A 1996, 606, 303–319. [Google Scholar] [CrossRef]
- Kienle, P.; Yamazaki, T. Pions in nuclei, a probe of chiral symmetry restoration. Prog. Pa. Nucl. Phys. 2004, 52, 85–132. [Google Scholar] [CrossRef]
- Lubos, D.; Park, J.; Faestermann, T.; Gernhäuser, R.; Kruecken, R.; Lewitowicz, M.; Nishimura, S.; Sakurai, H.; Ahn, D.S.; Baba, H.; et al. Improved Value for the Gamow-Teller Strength of the 100Sn Beta Decay. Phys. Rev. Lett. 2019, 122, 222502. [Google Scholar] [CrossRef]
- van Kolck, U. Nuclear physics with an effective field theory around the unitarity limit. Nuovo Cim. C 2019, 42, 52. [Google Scholar] [CrossRef]
- Tews, I.; Lattimer, J.M.; Ohnishi, A.; Kolomeitsev, E.E. Symmetry Parameter Constraints from a Lower Bound on Neutron-matter Energy. Astrophys. J. 2017, 848, 105. [Google Scholar] [CrossRef]
- Dong, H.; Kuo, T.T.S.; Lee, H.K.; Machleidt, R.; Rho, M. Half-Skyrmions and the Equation of State for Compact-Star Matter. Phys. Rev. C 2013, 87, 054332. [Google Scholar] [CrossRef]
- Annala, E.; Gorda, T.; Kurkela, A.; Nättilä, J.; Vuorinen, A. Evidence for quark-matter cores in massive neutron stars. Nat. Phys. 2020, 16, 907–910. [Google Scholar] [CrossRef]
- Glendenning, N.K. First order phase transitions with more than one conserved charge: Consequences for neutron stars. Phys. Rev. D 1992, 46, 1274–1287. [Google Scholar] [CrossRef] [PubMed]
- Macher, J.; Schaffner-Bielich, J. Phase transitions in compact stars. Eur. J. Phys. 2005, 26, 341–360. [Google Scholar] [CrossRef]
- Alford, M.G.; Han, S.; Prakash, M. Generic conditions for stable hybrid stars. Phys. Rev. D 2013, 88, 083013. [Google Scholar] [CrossRef]
- Han, S.; Steiner, A.W. Tidal deformability with sharp phase transitions in (binary) neutron stars. Phys. Rev. D 2019, 99, 083014. [Google Scholar] [CrossRef]
- Hippert, M.; Fraga, E.S.; Noronha, J. Insights on the peak in the speed of sound of ultradense matter. Phys. Rev. D 2021, 104, 034011. [Google Scholar] [CrossRef]
- Tsang, C.Y.; Tsang, M.B.; Danielewicz, P.; Lynch, W.G.; Fattoyev, F.J. Constraining neutron-star equation of state using heavy-ion collisions. arXiv 2018, arXiv:1807.06571. [Google Scholar]
- Demorest, P.; Pennucci, T.; Ransom, S.; Roberts, M.; Hessels, J. Shapiro Delay Measurement of a Two Solar Mass Neutron Star. Nature 2010, 467, 1081–1083. [Google Scholar] [CrossRef]
- Antoniadis, J.; Freire, P.C.; Wex, N.; Tauris, T.M.; Lynch, R.S.; Van Kerkwijk, M.H.; Kramer, M.; Bassa, C.; Dhillon, V.S.; Driebe, T.; et al. A Massive Pulsar in a Compact Relativistic Binary. Science 2013, 340, 6131. [Google Scholar] [CrossRef] [PubMed]
- Cromartie, H.T.; Fonseca, E.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Blumer, H.; Brook, P.R.; DeCesar, M.E.; Dolch, T.; Ellis, J.A.; et al. Relativistic Shapiro delay measurements of an extremely massive millisecond pulsar. Nature Astron. 2019, 4, 72–76. [Google Scholar] [CrossRef]
- Raaijmakers, G.; Riley, T.E.; Watts, A.L.; Greif, S.K.; Morsink, S.M.; Hebeler, K.; Schwenk, A.; Hinderer, T.; Nissanke, S.; Guillot, S.; et al. A NICER view of PSR J0030+0451: Implications for the dense matter equation of state. Astrophys. J. Lett. 2019, 887, L22. [Google Scholar] [CrossRef]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Harding, A.K.; Ho, W.C.G.; Lattimer, J.M.; et al. PSR J0030+0451 Mass and Radius from NICER Data and Implications for the Properties of Neutron Star Matter. Astrophys. J. Lett. 2019, 887, L24. [Google Scholar] [CrossRef]
- Fattoyev, F.J.; Piekarewicz, J.; Horowitz, C.J. Neutron Skins and Neutron Stars in the Multimessenger Era. Phys. Rev. Lett. 2018, 120, 172702. [Google Scholar] [CrossRef]
- Shankar, R. Renormalization group approach to interacting fermions. Rev. Mod. Phys. 1994, 66, 129–192. [Google Scholar] [CrossRef]
- Lee, H.K.; Rho, M. Topology Change and Tensor Forces for the EoS of Dense Baryonic Matter. Eur. Phys. J. A 2014, 50, 14. [Google Scholar] [CrossRef]
- Kaplunovsky, V.; Melnikov, D.; Sonnenschein, J. Baryonic Popcorn. J. High Energy Phys. 2012, 11, 47. [Google Scholar] [CrossRef]
- Weise, W. Dense Baryonic Matter and Strangeness in Neutron Stars. JPS Conf. Proc. 2019, 26, 011002. [Google Scholar] [CrossRef]
- Takeda, Y.; Kim, Y.; Harada, M. Catalysis of partial chiral symmetry restoration by Δ matter. Phys. Rev. C 2018, 97, 065202. [Google Scholar] [CrossRef]
- Marczenko, M.; Redlich, K.; Sasaki, C. Reconciling Multi-messenger Constraints with Chiral Symmetry Restoration. Astrophys. J. Lett. 2022, 925, L23. [Google Scholar] [CrossRef]
- Sedrakian, A.; Li, J.J.; Weber, F. Heavy Baryons in Compact Stars. arXiv 2022, arXiv:2212.01086. [Google Scholar] [CrossRef]
- Yang, W.C.; Ma, Y.L.; Wu, Y.L. Topology change and emergent scale symmetry in compact star matter via gravitational wave detection. Sci. China Phys. Mech. Astron. 2021, 64, 252011. [Google Scholar] [CrossRef]
- Hotokezaka, K.; Kyutoku, K.; Shibata, M. Exploring tidal effects of coalescing binary neutron stars in numerical relativity. Phys. Rev. D 2013, 87, 044001. [Google Scholar] [CrossRef]
- Hotokezaka, K.; Kyutoku, K.; Okawa, H.; Shibata, M.; Kiuchi, K. Binary Neutron Star Mergers: Dependence on the Nuclear Equation of State. Phys. Rev. D 2011, 83, 124008. [Google Scholar] [CrossRef]
- Bauswein, A.; Stergioulas, N. Unified picture of the post-merger dynamics and gravitational wave emission in neutron star mergers. Phys. Rev. D 2015, 91, 124056. [Google Scholar] [CrossRef]
- Fujimoto, Y.; Fukushima, K.; Hotokezaka, K.; Kyutoku, K. Gravitational Wave Signal for Quark Matter with Realistic Phase Transition. Phys. Rev. Lett. 2022, 130, 091404. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, Y.-L.; Yang, W.-C. Topology and Emergent Symmetries in Dense Compact Star Matter. Symmetry 2023, 15, 776. https://doi.org/10.3390/sym15030776
Ma Y-L, Yang W-C. Topology and Emergent Symmetries in Dense Compact Star Matter. Symmetry. 2023; 15(3):776. https://doi.org/10.3390/sym15030776
Chicago/Turabian StyleMa, Yong-Liang, and Wen-Cong Yang. 2023. "Topology and Emergent Symmetries in Dense Compact Star Matter" Symmetry 15, no. 3: 776. https://doi.org/10.3390/sym15030776
APA StyleMa, Y.-L., & Yang, W.-C. (2023). Topology and Emergent Symmetries in Dense Compact Star Matter. Symmetry, 15(3), 776. https://doi.org/10.3390/sym15030776