Chiral Magnetic Interactions in Small Fe Clusters Triggered by Symmetry-Breaking Adatoms

Abstract

The chirality of the interaction between the local magnetic moments in small transition-metal alloy clusters is investigated in the framework of density-functional theory. The Dzyaloshinskii–Moriya (DM) coupling vectors ${\mathit{D}}_{ij}$ between the Fe atoms in Fe₂X and Fe₃X with X = Cu, Pd, Pt, and Ir are derived from independent ground-state energy calculations for different noncollinear orientations of the local magnetic moments. The local-environment dependence of ${\mathit{D}}_{ij}$ and the resulting relative stability of different chiral magnetic orders are analyzed by contrasting the results for different adatoms X and by systematically varying the distance between the adatom X and the Fe clusters. One observes that the adatoms trigger most significant DM couplings in Fe₂X, often in the range of 10–30 meV. Thus, the consequences of breaking the inversion symmetry of the Fe dimer are quantified. Comparison between the symmetric and antisymmetric Fe-Fe couplings shows that the DM couplings are about two orders of magnitude weaker than the isotropic Heisenberg interactions. However, they are in general stronger than the anisotropy of the symmetric couplings. In Fe₃X, alloying induces interesting changes in both the direction and strength of the DM couplings, which are the consequence of breaking the reflection symmetry of the Fe trimer and which depend significantly on the adatom-trimer distance. A local analysis of the chirality of the electronic energy shows that the DM interactions are dominated by the spin-orbit coupling at the adatoms and that the contribution of the Fe atoms is small but not negligible.

1. Introduction

Systematic investigations of the magnetism of small clusters and low-dimensional systems over past decades have unfolded a wealth of remarkable possibilities of tuning and optimizing the magnetic characteristics of materials for specific technological and scientific purposes. Besides the most basic average magnetization and magnetic order, a major focus of attention has been dedicated to understanding the magneto-anisotropic effects, which originate in subtle relativistic contributions to the electronic structure such as spin-orbit coupling (SOC) [1]. A central property in this context is the magnetic anisotropy energy (MAE) which measures the spin-orientation dependence of the total electronic energy, which determines the low-temperature direction of the magnetic monodomains with respect to the lattice structure as well as its stability in the face of finite-temperature fluctuations and external fields [2,3,4,5,6,7,8]. Furthermore, recent studies have highlighted the importance of relativistic corrections on the magnetic interactions among the local atomic magnetic moments. Indeed, the spin-orbit-driven anisotropy of the magnetic interactions is known to condition the shape and stability of both ground-state and metastable magnetic configurations. Particularly interesting are competing interactions and antisymmetric Dzyaloshinskii–Moriya (DM) couplings that favor the development of noncollinear magnetic orders, such as extended spin-density waves and localized chiral magnetic textures [9,10,11,12,13]. Consequently, understanding the microscopic origin of the anisotropy and chirality of the interactions between local magnetic moments represents one of the major challenges in this field.

Symmetry and the absence thereof are obviously indissociable from magnetic anisotropy. Manipulating the composition, structure, and interfaces of magnetic systems has always been a major route in the development of artificially made magnetic materials [14,15,16,17,18,19,20,21,22,23,24,25,26,27,28]. Most previous theoretical studies of the electronic origin of chiral magnetism have been focused on extended low-dimensional systems (e.g., wires, stripes, thin films, and multilayers) [10,11,12,13,29,30,31,32,33,34,35,36,37,38]. Yang et al. analyzed the DM interaction between nearest neighbors in Co/Pt bilayers using first principles calculations [12]. The DM interaction was found to be the largest at the interfacial Co layer, where the effects of the spin-orbit energy associated with the adjacent Pt atoms are strongest. The authors concluded that the DM interaction is an indirect 3-site coupling in agreement with [9,39]. Within this interpretation, the mechanism behind the DM coupling can be regarded as analogous to the indirect Ruderman–Kittel–Kasuya–Yosida (RKKY) interaction [40,41,42]. However, the role of the 5d substrates in the chiral properties of the 3d coatings is still not quite clear [43]. For instance, Kashid et al. have performed a theoretical analysis of the chiral magnetic order in free-standing planar 3d–5d biatomic metallic chains using a tight-binding model and ab initio calculations [11]. In the case of FePt and CoPt chains, they found that the DM interaction can compete with the symmetric Heisenberg exchange interaction and with the local magnetocrystalline anisotropy. They also confirmed that the strength of the DM interaction is proportional to the strength of the SOC at the 5d atoms, which confirms the dominant role of the latter. Recently, Meyer et al. have successfully mapped the quantum electronic calculations onto an effective atomistic spin model appropriate for ultrathin Co and Mn films [35,44,45,46,47]. Thus, by combining electronic structure theory and real space modeling, the stability of magnetic skyrmions in the absence of external magnetic fields could be theoretically predicted and subsequently experimentally confirmed [35].

Studies of the magnetic anisotropy of finite clusters have been mostly focused on the dependence of the total energy on the orientation of the magnetization of monodomains [1,2,15,22,23,24,25,48]. These investigations have revealed a complicated, non-perturbative behavior of the magnetic anisotropy energy (MAE) as a function of cluster size, structure, bond length, and d-band filling. In particular, a considerable size-dependent enhancement of the cluster MAE with respect to the corresponding crystals and thin films has been observed, together with remarkable multiaxial energy landscapes. As in the case of extended nanostructures, alloying has been a most valuable means of optimizing the MAE of finite clusters. For instance, alloying magnetic 3d transition metals (TMs) with the highly polarizable and heavier 4d and 5d elements has allowed one to optimize the MAE of nanoalloys as a function of size and composition [14,15,16,21,22,23,24,25]. However, very little quantitative information is currently available on the degree of anisotropy of the magnetic interactions, particularly concerning the chiral DM couplings. It is the purpose of the present paper to address this problem by calculating the DM interactions in small Fe clusters. There is a particular emphasis on assessing how the DM interactions can be triggered by breaking the point group symmetry of the clusters and quantifying the actual changes in these chiral coupling as a function of the cluster structure.

The reminder of the paper is organized as follows. In the following Section 2, the bilinear expansion of the electronic energy as a function of the orientations ${\widehat{\mathbf{\mu }}}_i$ of the local magnetic moments at the different cluster atoms i is presented, from which the DM pseudovectors ${\mathit{D}}_{ij}$ characterizing the anisotropic antisymmetric couplings between the moments ${\mathbf{\mu }}_i$ and ${\mathbf{\mu }}_j$ at nearest neighbor (NN) atoms are derived. In Section 3, the method used for obtaining ${\mathit{D}}_{ij}$ is outlined, including the main technical details on the underlying density-functional calculations for different noncollinear orientations of ${\widehat{\mathbf{\mu }}}_i$ and ${\widehat{\mathbf{\mu }}}_j$. Our results for the DM couplings in Fe₂X and Fe₃X clusters with X = Cu, Pd, Pt, and Ir are presented and discussed in Section 4. The importance of the dimer-adatom hybridizations to the chiral interactions between the Fe atoms is quantified by varying the dimer-adatom distance systematically. The results for ${\mathit{D}}_{ij}$ are correlated to the various local contributions to the spin-orbit (SO) energy. In addition, the DM interactions are contrasted with the corresponding isotropic Heisenberg couplings, as well as with the anisotropy of the symmetric couplings. Finally, Section 5 summarizes our conclusions.

2. Classical Spin Model: Symmetric and Antisymmetric Couplings

In order to derive the effective pairwise interaction between the local magnetic moments at the Fe atoms, we expand the total electronic energy of the clusters to second order in the orientations of the local magnetic moments ${\mathbf{\mu }}_i$. The most general quadratic approximation of the interaction energy adopts the form of the classical spin Hamiltonian

$$\begin{array}{c}\hfill H=-\frac{1}{2}\sum _{i,j}{\widehat{\mathbf{\mu }}}_i·{\mathit{W}}_{ij}{\widehat{\mathbf{\mu }}}_j,\end{array}$$

(1)

where ${\mathit{W}}_{ij}\in {\mathbb{R}}^{3\times 3}$ are the interaction matrices and ${\widehat{\mathbf{\mu }}}_i={\mathbf{\mu }}_i/\left|{\mathbf{\mu }}_i\right|\in {\mathbb{R}}^3$ are the orientations of the local magnetic moments ${\mathbf{\mu }}_i$ [49]. Notice that Equation (1) takes into account not only all possible pair interactions between local magnetic moments ${\mathbf{\mu }}_i$ and ${\mathbf{\mu }}_j$ but also the local contribution to the electronic energy for $i=j$, which is often referred to as a local contribution to the magnetocrystalline anisotropy energy. Although fully unrestricted at the quadratic (i.e., pairwise interaction) level, the model can in principle be extended in order to incorporate three-spin interactions and beyond [50,51,52].

Without a loss of generality, H can be expressed in terms of irreducible contributions as the sum

$$\begin{array}{c}\hfill H=H_{\mathrm{H}}+H_{\mathrm{S}}+H_{\mathrm{DM}},\end{array}$$

(2)

where the rotational invariant Heisenberg term is given by

$$\begin{array}{c}\hfill H_{\mathrm{H}}=-\frac{1}{2}\sum _{i,j}{J}_{ij}{\widehat{\mathbf{\mu }}}_i·{\widehat{\mathbf{\mu }}}_j\end{array}$$

(3)

with $J_{ij}=\mathrm{Tr}\left\{{\mathit{W}}_{ij}+{\mathit{W}}_{ji}\right\}/6$, the anisotropic symmetric term is given by

$$\begin{array}{c}\hfill H_{\mathrm{S}}=-\frac{1}{2}\sum _{i,j}{\widehat{\mathbf{\mu }}}_i·{\mathit{W}}_{ij}^{\mathrm{S}}{\widehat{\mathbf{\mu }}}_j,\end{array}$$

(4)

with ${\mathit{W}}_{ij}^{\mathrm{S}}=\frac{1}{2}\left({\mathit{W}}_{ij}+{\mathit{W}}_{ji}\right)-J_{ji}𝟙={\mathsf{\Delta }\mathit{J}}_{ij}$ and the anisotropic antisymmetric term is given by

$$\begin{array}{c}\hfill H_{\mathrm{DM}}=-\frac{1}{2}\sum _{i\ne j}{\widehat{\mathbf{\mu }}}_i·{\mathit{W}}_{ij}^{\mathrm{A}}{\widehat{\mathbf{\mu }}}_j,\end{array}$$

(5)

with ${\mathit{W}}_{ij}^{\mathrm{A}}=\frac{1}{2}\left({\mathit{W}}_{ij}-{\mathit{W}}_{ji}\right)$. The focus of this work is on the antisymmetric contribution $H_{\mathrm{DM}}$ known as the Dzyaloshinskii–Moriya (DM) interaction [53,54,55,56]. This term can be written in a geometrically more transparent form as

$$\begin{array}{c}\hfill H_{\mathrm{DM}}=\frac{1}{2}\sum _{i,j}{\mathit{D}}_{ij}·\left({\widehat{\mathbf{\mu }}}_i\times {\widehat{\mathbf{\mu }}}_j\right),\end{array}$$

(6)

where ${\mathit{D}}_{ij}=-{\mathit{D}}_{ji}\in {\mathbb{R}}^3$ is a pseudovector known as the DM vector. The magnitude of ${\mathit{D}}_{ij}$ depends on the details of the electronic structure and on the strength of the SOC of the compounds [12], whereas the orientation of ${\mathit{D}}_{ij}$ is to a large extent conditioned by the point-group symmetry of the nanostructure around the bond connecting i and j [54]. The orientation of ${\mathit{D}}_{ij}$ (direction and sense) defines both the favored rotation sense as well as the optimal rotation plane of the local magnetic moments. According to Equation (6) a positive (negative) value of ${\mathit{D}}_{ij}·\left({\widehat{\mathbf{\mu }}}_i\times {\widehat{\mathbf{\mu }}}_j\right)$ means that the sense of rotation of the local magnetic moments from ${\mathbf{\mu }}_i$ toward ${\mathbf{\mu }}_j$ is an unfavorable (favorable) configuration. For example, if ${\mathit{D}}_{ij}$ is pointing along the positive z axis, then a counterclockwise $(+)$ [clockwise $(-)$] sense of rotation from ${\mathbf{\mu }}_i$ toward ${\mathbf{\mu }}_j$ within the $xy$ plane yields the highest [lowest] pair-interaction energy.

The relation between the point-group symmetry of the lattice and the possible orientation of the DM vector has been discussed by Moriya who established a number of useful symmetry rules [54]. Three of them are particularly relevant for the following discussion. Consider two local magnetic moments ${\mathbf{\mu }}_i$ and ${\mathbf{\mu }}_j$ located at ${\mathit{r}}_i$ and ${\mathit{r}}_j$, with ${\mathit{r}}_{ij}={\mathit{r}}_j-{\mathit{r}}_i$ being the vector connecting them and C denoting the point bisecting the segment ${\mathit{r}}_{ij}$. Moriya has shown that the DM interaction satisfies the following symmetry constraints [54]:

(i)

If the structure has an inversion symmetry with respect to C then ${\mathit{D}}_{ij}=0$.

(ii)

If the structure has a mirror symmetry plane perpendicular to ${\mathit{r}}_{ij}$ passing through C then ${\mathit{D}}_{ij}$ is perpendicular to ${\mathbf{r}}_{ij}$.

(iii)

${\mathit{D}}_{ij}$ is perpendicular to any mirror symmetry plane of the structure that includes the bond ${\mathit{r}}_{ij}$ connecting ${\mathit{r}}_i$ and ${\mathit{r}}_j$.

In order to verify the validity of these rules, it suffices to recall that the local magnetic moments, having spin and angular momentum origin, are pseudovectors while the DM energy ${\mathit{D}}_{ij}·({\widehat{\mathbf{\mu }}}_i\times {\widehat{\mathbf{\mu }}}_j)$ is of course a scalar. Let us finally underscore that the antisymmetric nature of the DM coupling favors noncollinear magnetic order with a precise local chirality, thus providing the driving force that stabilizes spiral magnetic orders and vortex magnetic patterns.

3. Electronic Calculations

In order to determine the local-environment dependence of the DM interaction in small Fe clusters with non-magnetic adatoms, we perform independent density-functional theory (DFT) calculations for different noncollinear magnetic configurations that are imposed by constraining the directions of the local magnetic moments ${\mathbf{\mu }}_i$ at each Fe atom. The DM pseudovectors ${\mathit{D}}_{ij}$ between the Fe atoms are then obtained as a function of the geometry of the cluster by building appropriate total energy differences between equivalent configurations with opposite chiralities. 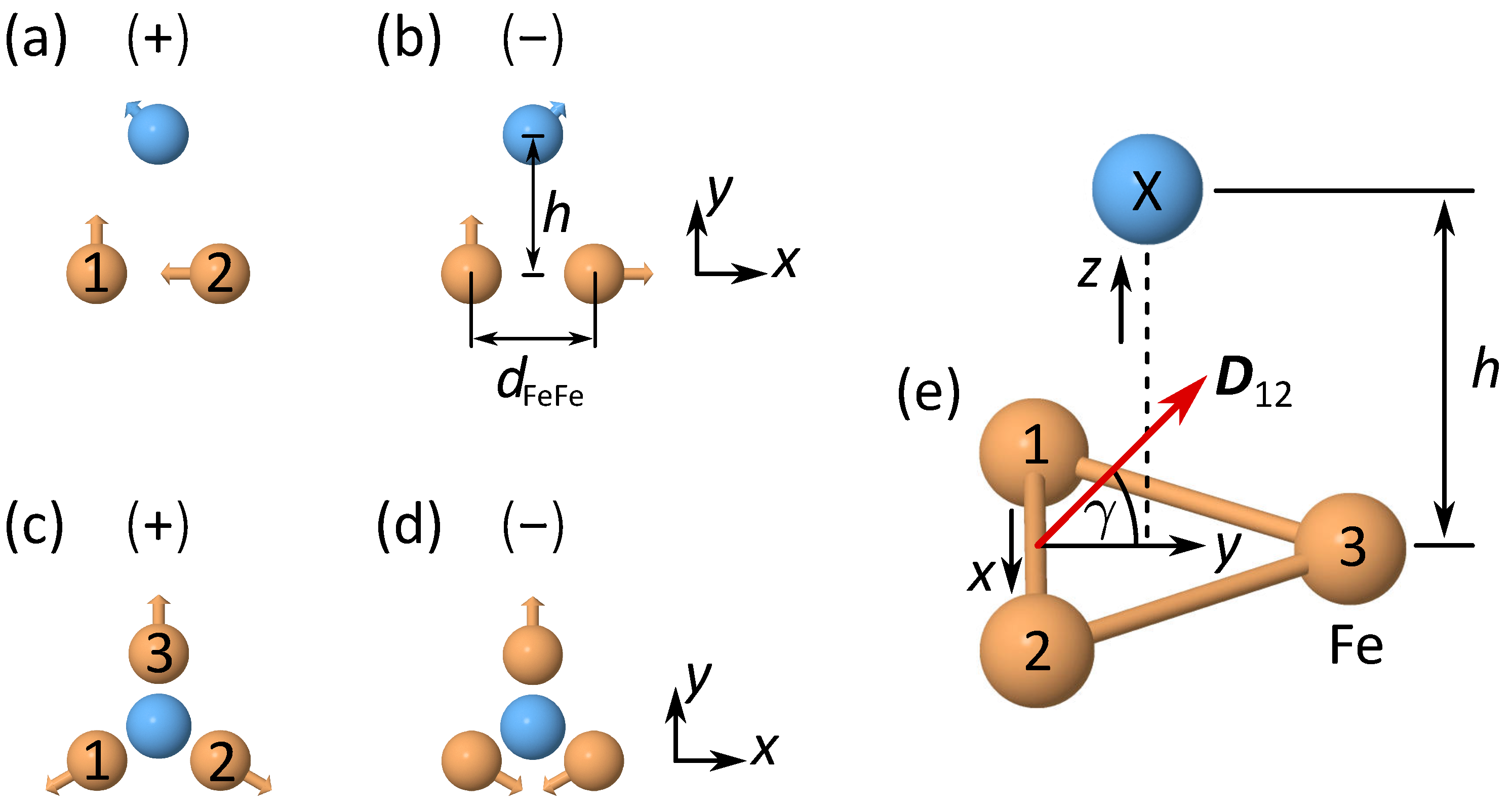As an example, Figure 1a,b show two representative magnetic configurations of Fe₂X, one exhibiting counterclockwise $(+)$ and the other clockwise $(-)$ chiralities, from which the z component of the DM vector $D_{12}^z=\left(E_{+}-E_{-}\right)/2$ is obtained. The contribution of scalar and symmetric magnetic interactions $J_{ij}$ and ${\mathit{W}}_{ij}^{\mathrm{S}}$ cancel out in this energy difference, since they are independent of the chirality of otherwise identical magnetic configurations [see Section 2]. In the case of Fe₃X the interactions between all the pairs of Fe atoms have to be taken into account. One possibility to determine $D_{12}^z$ is then to consider the magnetic configurations displayed in Figure 1c,d, from which $D_{12}^z=\left(E_{+}-E_{-}\right)/3\sqrt{3}$ follows. The remaining components $D_{12}^x$ and $D_{12}^y$ are determined in an analogous way by considering further magnetic configurations in which the local magnetic moments point out of the Fe₂X or Fe₃ plane. The symmetric couplings ${\mathit{W}}_{ij}^{\mathrm{S}}$, the isotropic part $J_{ij}$, and the anisotropies ${\mathsf{\Delta }\mathit{J}}_{ij}$ are obtained by performing independent selfconsistent calculations for parallel and antiparallel orientations of the local Fe moments along the x, y and z axes. Thus, the antisymmetric DM contributions to the total energy vanish and the symmetric couplings can be derived by building the energy differences between the corresponding antiparallel and parallel alignments.

The spin polarization at the adatoms, as well as the modulus of the local magnetic moments at the Fe atoms, remain unconstrained in all the calculations. In this way, these electronic degrees of freedom are optimized for each orientation of the Fe magnetic moments and the most stable solution of the Kohn–Sham equations is obtained [57,58]. In practice, in the case of Fe, the sizes of local magnetic moments are largely independent of their orientation, since changing the intra-atomic spin-density redistribution involves relatively large energies. Indeed, the intra-atomic exchange energy $\Delta E_X$ is of the order of 1 eV, which is significantly larger than the exchange coupling constants controlling the orientations of ${\mathbf{\mu }}_i$ [59,60,61,62,63,64]. This also justifies our analysis in terms of the classical spin model introduced in Section 2.

No constraints are imposed on the magnetic moments ${\mathbf{\mu }}_i^{\mathrm{X}}$ of the adatoms X, since our focus lies on the Fe atoms, their magnetic configurations, and their effective interactions. Furthermore, we do not intend to determine the magnetic couplings involving the adatoms, whose magnetic moments are small and strongly dependent on the cluster geometry. This by no means implies that the adatom contribution to the magnetic coupling is unimportant. In fact, significant local magnetic moments ${\mathbf{\mu }}_i^{\mathrm{X}}$ are induced at the adatoms X as a result of the proximity with the Fe atoms, which tend to increase as the adatom-cluster distance decreases. Although the orientations of ${\mathbf{\mu }}_i$ are in general noncollinear, the tendency thereby is to align ${\mathbf{\mu }}_i^{\mathrm{X}}$ parallel to ${\mathbf{\mu }}_i^{\mathrm{Fe}}$. Consequently, the adatom contribution to the changes in the total energy as a function of the orientation of the Fe moments is central to our study. Moreover, as already discussed, the adatoms break the inversion symmetry of the Fe dimer, thus triggering the appearance of DM interaction between Fe atoms [54]. In the case of Fe₃, the adatom-induced symmetry breaking unfolds new components of ${\mathit{D}}_{ij}$. Furthermore, heavy open d-shell adatoms such as Pd, Pt, and Ir exhibit a particularly large SOC, which enhances all magneto-anisotropic phenomena and, in particular, the DM couplings [9,12,54].

The first-principles calculations have been performed in the framework of Hohenberg–Kohn–Sham’s density functional theory [65,66] as implemented in the Vienna ab initio simulation package (VASP) [67,68]. The spin-polarized Kohn–Sham equations are solved using an augmented plane-wave basis set, taking into account the interaction between the valence electrons and ionic cores through the projector-augmented wave (PAW) method [69]. The exchange and correlation energy-functional is described by the Perdew–Burke–Ernzerhof (PBE) parameterization of the generalized-gradient approximation (GGA) [70]. In order to gain insight into the role of electron correlations on the DM interactions, we have also performed the calculations by using the local-density approximation (LDA) [71,72,73] and the GGA proposed by Perdew and Wang (PW) [74,75,76,77].

From a technical perspective, the numerical convergence and stability of the calculations is significantly improved by considering the fractional occupations of Kohn–Sham orbitals with a Gaussian smearing. The width of the Gaussian is subsequently decreased stepwise in the range $0.7\mathrm{eV}\ge \sigma \ge 0.01\mathrm{eV}$ until the entropy contribution to the free energy is less than 10⁻³ eV/atom. Concerning the expansion of the Kohn–Sham orbitals, a cut-off energy $E_{\max }=500$ eV has been used for the plane-wave basis set. The self-consistent relaxation of the electronic degrees of freedom is pursued until the total electronic-energy change between subsequent optimization steps is smaller than 10⁻⁶ eV. This is sufficient for our purposes, as we are interested in determining the energy differences of the order of 10⁻⁴–10⁻³ eV. The dimensions of the supercell are chosen to be large enough (at least 12 Å) so that any spurious interactions between the cluster images are negligible. As in any finite-cluster calculation, the wave vector is set to $\mathit{k}=0$, since no integration in the Brillouin zone is necessary.

In order to constrain the orientation of the local magnetic moments ${\mathbf{\mu }}_i$ at each Fe atom i along specific directions ${\widehat{\mathit{n}}}_i$ ($|{\widehat{\mathit{n}}}_i|=1$), the penalty function

$$\begin{array}{c}\hfill \Delta E_{\mathrm{p}}=\sum _i\zeta {\left[{\mathbf{\mu }}_i-{\widehat{\mathit{n}}}_i\left({\widehat{\mathit{n}}}_i·{\mathbf{\mu }}_i\right)\right]}^2\end{array}$$

(7)

is added to the usual Hohenberg–Kohn energy functional, where $\zeta \in \mathbb{R}$ specifies the weight of the penalty term $\Delta E_{\mathrm{p}}$ in the Hamiltonian [78,79]. The local magnetic moments ${\mathbf{\mu }}_i$ entering Equation (7) are obtained by integrating the magnetization density $\mathit{m}\left(\mathit{r}\right)$ within the corresponding Wigner–Seitz sphere ${\mathsf{\Omega }}_i$, i.e.,

$$\begin{array}{c}\hfill {\mathbf{\mu }}_i={\int }_{{\mathsf{\Omega }}_i}\mathit{m}\left(\mathit{r}\right)\mathrm{d}{r}^3.\end{array}$$

(8)

Once self-consistency is reached, the spin-orbit interactions are introduced by using the method implemented in Ref. [80].

The equilibrium geometry of the clusters are determined by performing unconstrained relaxations of all atomic positions until the forces are smaller than 0.01 eV/Å. The resulting equilibrium distances are listed in

Table 1. Equilibrium configurations of Fe₂X and Fe₃X clusters with X = Cu, Pd, Pt, and Ir. The equilibrium distances $d_{\mathrm{FeFe}}$ and $d_{\mathrm{FeX}}$, as well as the height $h_0$ between the adatom X and the center of the Fe₂ dimer or the centre of the Fe₃ triangle, are given in Å. In the case of Fe₃X, average values are shown ignoring minor distortions of the Fe trimer.

	Fe2Cu	Fe2Pd	Fe2Pt	Fe2Ir	Fe3Cu	Fe3Pd	Fe3Pt	Fe3Ir
$d_{\mathrm{FeFe}}$	2.00	2.04	2.10	2.25	2.26	2.31	2.27	2.35
$d_{\mathrm{FeX}}$	2.49	2.41	2.38	2.27	2.40	2.37	2.35	2.36
$h_0$	2.28	2.18	2.14	1.97	2.02	2.07	2.06	1.93

. In the case of Fe₃X, the Fe atoms actually form an isosceles triangle whose shape changes somewhat as the height h between the Fe₃ plane and the adatom X is varied. For simplicity, we ignore in the following these minor distortions and consider equilateral Fe₃ with the adatom X located above its center. The effects of symmetry breaking and adatom-cluster hybridizations on the DM interaction are then investigated as a function of the height between X and the Fe₃ plane.

4. Results

This section presents and discusses our results on Fe₂X and Fe₃X clusters for X = Cu, Pd, Pt, and Ir. In Section 4.1, the effects of the different adatoms as the triggering source of the DM coupling in Fe₂X are quantified by systematically varying the adatom-dimer distance. The trends for different adatoms are contrasted. The microscopic origin of the DM interaction is analyzed from a local perspective in Section 4.2 by computing the adatom-resolved contributions of the SOC to the chirality energy $\Delta E_{\pm }$. In Section 4.3 the symmetric isotropic and anisotropy interactions in Fe₂X are quantified and the results are compared to the corresponding antisymmetric DM couplings. Finally, in Section 4.4, the changes in the modulus and orientation of the DM vector ${\mathit{D}}_{12}$ between two Fe atoms, which are induced by the adatom in Fe₃X are quantified as a function of the distance between the adatom and the Fe₃ plane. The trends for different X are identified and compared with the behavior found in Fe₂X.

4.1. DM Interaction in Fe₂X Clusters

The presence of the adatom breaks the inversion symmetry of the pure dimer and unlocks the DM interaction between the Fe atoms. In accordance with Moriya’s symmetry considerations (see Section 2 and [54]) we obtain that ${\mathit{D}}_{12}$ is perpendicular to the plane defined by the Fe₂X triangle (i.e., ${\mathit{D}}_{12}^x={\mathit{D}}_{12}^y=0$). 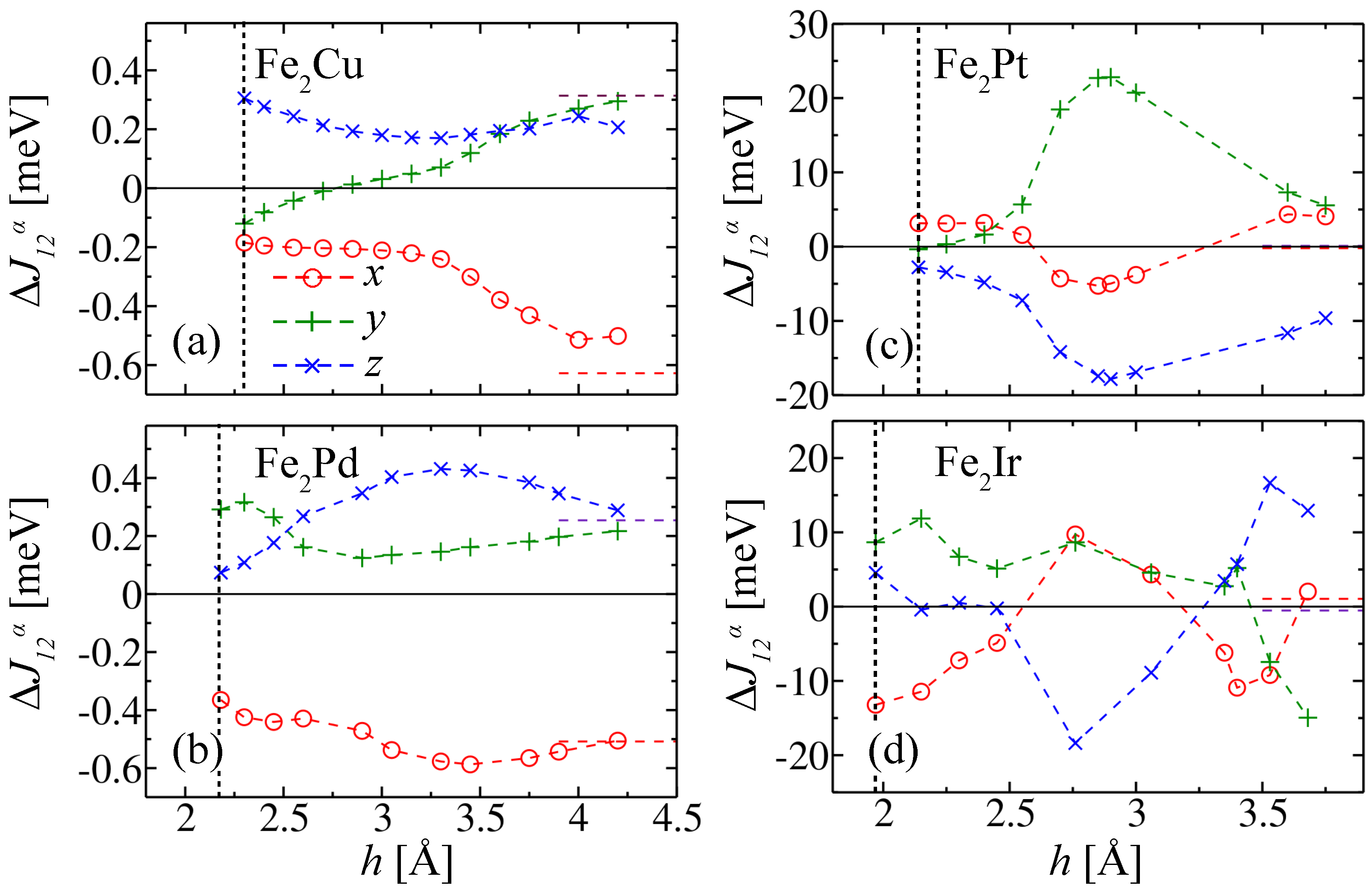The calculated z component $D_{12}^z$ for X = Cu, Pd, Pt, and Ir are shown in Figure 2 as a function of the distance h between X and the center of the Fe₂ dimer bond. A rich variety of behaviors is found for the considered adatoms, which have different chemical properties, d-band fillings, and the SOC strengths.

Probably the simplest or softest way of breaking the symmetry of the Fe dimer and thereby trigger a possible DM coupling is by adding a non-magnetic light atom with a closed d shell similar to Cu. Figure 2a shows that the effects are, already in this case, quite remarkable. The induced DM coupling between the Fe atoms is significant, reaching values of about 2 meV at the equilibrium distance. Stronger effects are expected by considering heavier 4d atoms, particularly if the d-band is no longer completely filled. This is indeed the case when a Pd atom is added. Figure 2b shows an enhancement by about a factor 10 of the strength of the induced DM coupling between the Fe atoms, as compared to Fe₂Cu. It is, however, remarkable that the dependence of $D_{12}^z$ on the adatom-dimer distance is qualitatively similar in these two cases. In Fe₂Cu, the z component $D_{12}^z$ is positive and largest around the equilibrium configuration ($h_0\simeq 2.28$Å). This means that an in-plane clockwise $(-)$ rotation of the local magnetic moments ${\mathbf{\mu }}_i$ at the Fe atoms is favored with respect to a counterclockwise $(+)$ rotation [see also Figure 1a,b]. In contrast, at large distances, where the adatom-dimer interaction is weak, $(h>3$–3.5 Å) $D_{12}^z$ changes sign and goes over a minimum before vanishing in the limit of $h\to +\infty$, where the consequences of breaking the inversion symmetry become negligible. In the case of Fe₂Pd a qualitatively similar trend is observed. However, the strength of the DM coupling is significantly larger, particularly at short distances, where the adatom-dimer hybridization is strong. This can be qualitatively understood by recalling that, in Pd, the 4d shell is open and the nuclear charge is larger, which both results in a much stronger and more effective SOC.

The trend to stronger DM couplings is confirmed when the even heavier Pt and Ir adatoms are considered. In these cases, $D_{12}^z$ reaches values of up to 20–30 meV. Furthermore, the dependence on h and on the adatom-dimer hybridization is far richer. Indeed, in Fe₂Pt and Fe₂Ir, $D_{12}^z$ oscillates as a function of h, which demonstrates interesting possibilities of manipulating the chiral magnetic coupling through alloying. For example, in Fe₂Pt, the favored chirality changes twice as h increases: First, at a short height $h\simeq 1.9$ Å, from a clockwise rotation $(-)$ of ${\mathbf{\mu }}_i$, which corresponds to $D_{12}^z\ge 0$, to a counterclockwise rotation $(+)$, which corresponds to $D_{12}^z\le 0$. Second, at larger h, the favored sense of rotation changes again from counterclockwise $(+)$ to clockwise $(-)$ at $h\simeq 2.3$–2.5 Å. Notice that the oscillations of $D_{12}^z$ are quite important in Fe₂Pt, their values range from $D_{12}^z\simeq -15$ meV to 10–20 meV in the counterclockwise, respectively, and clockwise sense of rotation.

The importance of d-band fillings becomes clear by comparing the results for Pt and Ir, which have similar atomic numbers and thus comparably strong SOCs. In the case of Fe₂Ir, starting from a large negative value $D_{12}^z\simeq -20$meV at the smallest considered height $h=1.9$Å, $D_{12}^z$ becomes even stronger at larger distances reaching its maximum absolute value $|D_{12}^z|\simeq 30$meV at a height $h=2.3$–2.6 Å, which is larger than the equilibrium height $h_0=1.97$Å (see Table 1). Around $h_0$, $D_{12}^z$ depends linearly on h, with an important derivative $\partial D_{12}^z/\partial h$, which indicates the possibility of interesting couplings between magnetic chirality and stress or lattice vibrations. For heights beyond the largest $|D_{12}^z|$, $D_{12}^z$ increases monotonically ($D_{12}^z<0$) approaching zero in the limit of large h. In this context, it is interesting to note that a similar oscillation of the orientation of the DM vector and an exponential decrease in the DM-interaction strength as a function of interatomic distance have been derived from low-temperature inelastic scanning tunneling spectroscopy on a pair of Fe atoms deposited on Pt(111) [32].

Three different local and gradient-corrected approximations to the exchange and correlation (XC) functional have been considered in order to assess the role of correlations on the SO energies and DM interactions. The results shown in Figure 2 show that they all yield similar trends as a function of the distance between the nonmagnetic adatom and the Fe dimer. The quantitative differences between the functionals are in almost all cases clearly smaller than the changes in $D_{12}^z$ as a function of h or composition. This is quite remarkable since the behaviors found for the various considered adatoms differ widely (see Figure 2). Notice, however, that in the case of Fe₂Pt and Fe₂Ir, where the absolute values of $D_{12}^z$ are particularly large, the quantitative differences between the results for different functionals can be significant (up to 15 meV), which demonstrates that electron correlations can have a significant impact on the DM couplings.

4.2. Microscopic Origin of the Dzyaloshinskii–Moriya Interactions in Fe₂X Clusters

Besides quantifying the strength and orientation of the DM interactions, understanding their microscopic origin is also of considerable interest. Indeed, a more detailed insight on this subtle relativistic effect from a theoretical point of view should be helpful to the experimental search for new materials relevant for technological applications. In this context, it has been proposed [9] that the DM coupling can be regarded as an indirect interaction between two magnetic atoms with local magnetic moments ${\mathbf{\mu }}_i$ and ${\mathbf{\mu }}_j$ (for example Fe or Co atoms) mediated by a third often non-magnetic atom exhibiting strong SOC (for example Pt). According to this picture, different relative cantings of the local magnetic moments ${\mathbf{\mu }}_i$ and ${\mathbf{\mu }}_j$ result in changes of the SOC energy, which occur for the most part at the heavy non-magnetic atoms. This dominant dependence of the contribution of the nonmagnetic, though strongly spin-polarizable atoms, to the SO energy is regarded as the driving force behind the DM interaction [12]. A further interesting alternative perspective has been proposed in [13]. In this case, the DM interaction is regarded as the result of spin-flip hoppings of electrons between the magnetic moments ${\mathbf{\mu }}_i$ and ${\mathbf{\mu }}_j$, whereby the spin flips are triggered by spin-orbit scattering at the non-magnetic nearby atoms [13]. Moreover, previous theoretical studies on 3d TM chains, monolayers and multilayers deposited on 5d substrates have shown that the chiral coupling between 3d TM atoms are often associated with changes in the SOC energy originating mainly at the adjacent 5d atoms of the support, rather than at the actual magnetic materials [9,11,13,43]. It is therefore very interesting to investigate if a similar situation applies to the chiral properties triggered on Fe dimers by adatoms.

In order to clarify the origin of the DM interaction in the Fe₂X clusters, we have analyzed our ab initio calculations from a local perspective. In Figure 3, the results are given for the changes in the SO energy $\Delta E^{\mathrm{SO}}=E_{+}^{\mathrm{SO}}-E_{-}^{\mathrm{SO}}$ upon changing the chirality of the magnetic configuration in Fe₂X from clockwise [$(-)$, Figure 1b] to counterclockwise [$(+)$, Figure 1a]. First of all, one observes that, in the case of Cu and Pd, the chirality of the spin-orbit energy $\Delta E^{\mathrm{SO}}$ accurately accounts for the corresponding chirality of the total electronic energy $\Delta E_{\pm }=E_{+}-E_{-}$. Some deviations are found in the case of the Pt adatom while, in the case of Ir, the quantitative discrepancies between $\Delta E^{\mathrm{SO}}$ and $\Delta E_{\pm }$ are significant. This demonstrates that the changes in the kinetic and Coulomb energies of the electrons as a function of chirality, possibly related to redistributions of the spin- and orbital-polarized density, cannot be neglected when heavy TM atoms are involved. Particularly, in the case of Fe₂Ir at a relatively large Ir-dimer distance ($h\ge 2.8$ Å), the actual DM couplings derived from $\Delta E_{\pm }$ are orders of magnitude larger than those derived from the spin-orbit contributions $\Delta E^{\mathrm{SO}}$ alone. Nevertheless, it also true that, except in Fe₂Ir, $\Delta E^{\mathrm{SO}}$ is in general able to account for both the sign and approximate strength of $D_{12}^z$, including the non-monotonic behavior as a function of h found in Fe₂Pt. Concerning the atom specific contributions to $\Delta E^{\mathrm{SO}}$, one observes that the adatoms dominate the chirality of the spin-orbit energy, not only for 4d and 5d TMs but also in the case of Cu. Notice, moreover, that the contribution of the Fe atoms is sometimes significant. In particular, in the case of Cu, the chirality of the SO energy at the Fe atoms is responsible for the change of sign of $D_{12}^z$ for $h>3.5$Å.

The results shown in Figure 3 also provide a useful insight on the role of the d-band filling of the adatoms X regarding the total energy asymmetry $\Delta E_{\pm }$. One observes that Fe₂Cu, the lightest adatom with a closed 3d shell, exhibits the smallest $|\Delta E_{\pm }|\le 5$meV among the considered clusters. Pd and Pt, which have a similar d-band filling, yield comparable largest values of $|\Delta E_{\pm }|\simeq 25$–35 meV, despite Pt being much heavier than Pd. In contrast, Ir, which has one more d hole than Pt, shows a greatly increased maximum $|\Delta E_{\pm }|\simeq 63$meV. This is consistent with the conclusions drawn by Belabbes et al., in whose work the DM interactions are regarded as the result of spin-flip hoppings between magnetic compounds triggered by spin-orbit scattering at often heavy non-magnetic elements [13]. As these authors point out, active d-band states at both the magnetic and intermediate nonmagnetic alloy components are necessary for the spin-flip processes to occur. Therefore, a lower d-band filling at the adatoms facilitates the indirect spin-flip hoppings between the Fe atoms, thus resulting in a larger DM coupling.

4.3. Symmetric Magnetic Interactions in Fe₂X Clusters

The stability of noncollinear magnetic configurations and the morphology of spin textures such as domain walls, spin-density waves, and skyrmions are conditioned by the importance of the antisymmetric DM couplings ${\mathit{D}}_{ij}$, which favor noncollinear spin arrangements [see Equation (6)], relative to the symmetric isotropic couplings $J_{ij}$, which, in the absence of frustrations, favor collinear alignments of the local moments [see Equation (3)]. Furthermore, the anisotropies ${\mathsf{\Delta }\mathit{J}}_{ij}$ of the symmetric couplings including the local anisotropies ${\Delta \mathit{J}}_{ii}$ define, together with ${\mathit{D}}_{ij}$, the shape and orientation of the magnetic configurations in both collinear and noncollinear situations [see Equation (4)]. It is therefore very interesting to analyze the dependence of $J_{ij}$ and ${\mathsf{\Delta }\mathit{J}}_{ij}$ on the local environment of the magnetic moments in Fe₂X and to compare them with the corresponding DM couplings.

In Figure 4, the symmetric Heisenberg interactions $J_{12}^{\alpha \alpha }$ in Fe₂X are shown as a function of the distance h between Fe₂ and the adatom X = Cu, Pd, Pt, and Ir for the different orientations $\alpha =x,y$ and z of the Fe moments ${\mathbf{\mu }}_i$. These couplings are given by the difference between the electronic energies obtained in independent calculations for parallel and antiparallel alignments of the local magnetic moments along $\alpha$ [see Equation (4)]. The corresponding isotropic couplings $J_{ij}=\frac{1}{3}{\sum }_{\alpha }{J}_{ij}^{\alpha \alpha }$ are shown as well. In Fe₂Cu and Fe₂Pd, the dependencies of $J_{12}^{\alpha \alpha }$ on h are qualitatively similar. In contrast to the results for $D_{12}^z$ shown in Figure 2a,b, $J_{12}^{\alpha \alpha }$ decreases with decreasing dimer-adatom distance. In other words, $J_{12}^{\alpha \alpha }$ decreases as the hybridization between the Fe d-orbitals and the adatom increases. This trend to decouple the Fe local magnetic moments by the adatom contrasts strongly with the behavior found for the DM interaction, where the adatom plays the role of a mediator, enhancing $D_{12}^z$ as it approaches the dimer. In the case of Fe₂Pt and Fe₂Ir, the symmetric couplings are further reduced in comparison with Fe₂Cu and Fe₂Pd. Moreover, one observes that $J_{12}^{\alpha \alpha }$ oscillates strongly as a function of h deviating by about 10% from its large-h value. Similar strong oscillations have been observed in $D_{12}^z$ of Fe₂Pt and Fe₂Ir [Figure 2c,d], which reflects a much stronger modification of the electronic properties due to the presence of these adatoms. However, note that the presence of the adatom does not result in a clear enhancement of the symmetric Heisenberg coupling $J_{12}^{\alpha \alpha }$, as it is the case for $D_{12}^z$. In fact, the symmetric couplings $J_{12}^{\alpha \alpha }$ are not triggered, despite being certainly affected by the symmetry breaking adatoms. They are already present in the isolated Fe clusters [2,81,82].

For large h, in the limit of isolated Fe₂, one observes that $J_{12}$ is finite and positive, which corresponds to the known ferromagnetic alignment of the Fe moments in the dimer ground state [81,82,83]. Notice that the large-h value of $J_{12}$ decreases as we move from X = Cu to X = Ir. This is a consequence of the fact that the considered Fe-Fe distance, assumed for simplicity to be independent of h, is larger for the heavier adatoms (see Table 1). The quantitative values of $J_{12}$ are in the range of 0.4–0.6 eV. They are larger than typical Fe-bulk values, which is in agreement with the temperature dependence of the average magnetic moment of Fe₂ obtained in functional-integral d-band-model calculations [82]. The comparison with the DM couplings reported in Figure 2, which are of the order of 1–30 meV, shows that a misalignment of Fe moments by an angle $\beta =2D_{12}^z/J_{12}$ of only 1/100–1/10 radians yields exchange energies comparable to the DM energy. Previous theoretical studies have shown that the ratio $\beta$ between the DM and exchange couplings depends strongly on the considered magnetic material and its interfaces. The values of $\beta$ of the order of 1/100, as in this work, are not uncommon [44,84,85,86]. However, the situations have been also found in which $\beta$ is of the order of 1 or even larger [32,87,88].

The anisotropy of the symmetric couplings ${\mathsf{\Delta }\mathit{J}}_{12}^{\alpha }=J_{12}^{\alpha \alpha }-J_{12}$, shown in Figure 5 reveals the SOC effects the exchange couplings. One observes that ${\mathsf{\Delta }\mathit{J}}_{12}^{\alpha }$ is quantitatively comparable, though it is in general weaker than $D_{12}^z$ for the corresponding adatom. Although both symmetric and antisymmetric anisotropic interactions are a consequence of SOC, the effect of the adatoms is clearly stronger on the DM couplings than on the anisotropy of the Heisenberg couplings, particularly in the case of the heavier adatoms having strong SOC (e.g., Pt and Ir). In the case of Cu and Pd, the anisotropies ${\mathsf{\Delta }\mathit{J}}_{12}^{\alpha }$ are very small, of the order of 0.2–0.6 meV, with an even weaker dependence on h. In fact, they are very similar to the anisotropies in the isolated dimer: ${\mathsf{\Delta }\mathit{J}}_{12}^x\simeq -0.6$ meV and ${\mathsf{\Delta }\mathit{J}}_{12}^y={\mathsf{\Delta }\mathit{J}}_{12}^z\simeq 0.25$ meV. In contrast, ${\mathsf{\Delta }\mathit{J}}_{12}^{\alpha }$ becomes orders of magnitude stronger when the heavier Pt and Ir atoms are added (see Figure 5). Accordingly, the distance dependence of ${\mathsf{\Delta }\mathit{J}}_{12}^{\alpha }$ is also much stronger baring no relation to the values in the isolated dimer even at a relatively large adatom-dimer distance.

4.4. DM Interaction in Fe₃X Clusters

The Fe₃X clusters with an equilateral basis exhibit mirror symmetry planes perpendicular to the bonds connecting the Fe atoms and passing through the middle of the vectors ${\mathit{r}}_{ij}$ connecting them [see Figure 1]. In accordance with Moriya’s symmetry considerations (see Section 2 and [54]), we obtain that ${\mathit{D}}_{12}$ is perpendicular to ${\mathit{r}}_{12}$ (i.e., $D_{12}^x=0$). In contrast to Fe₂X, the adatom affects not only the absolute value of the ${\mathit{D}}_{12}$ but also its orientation. 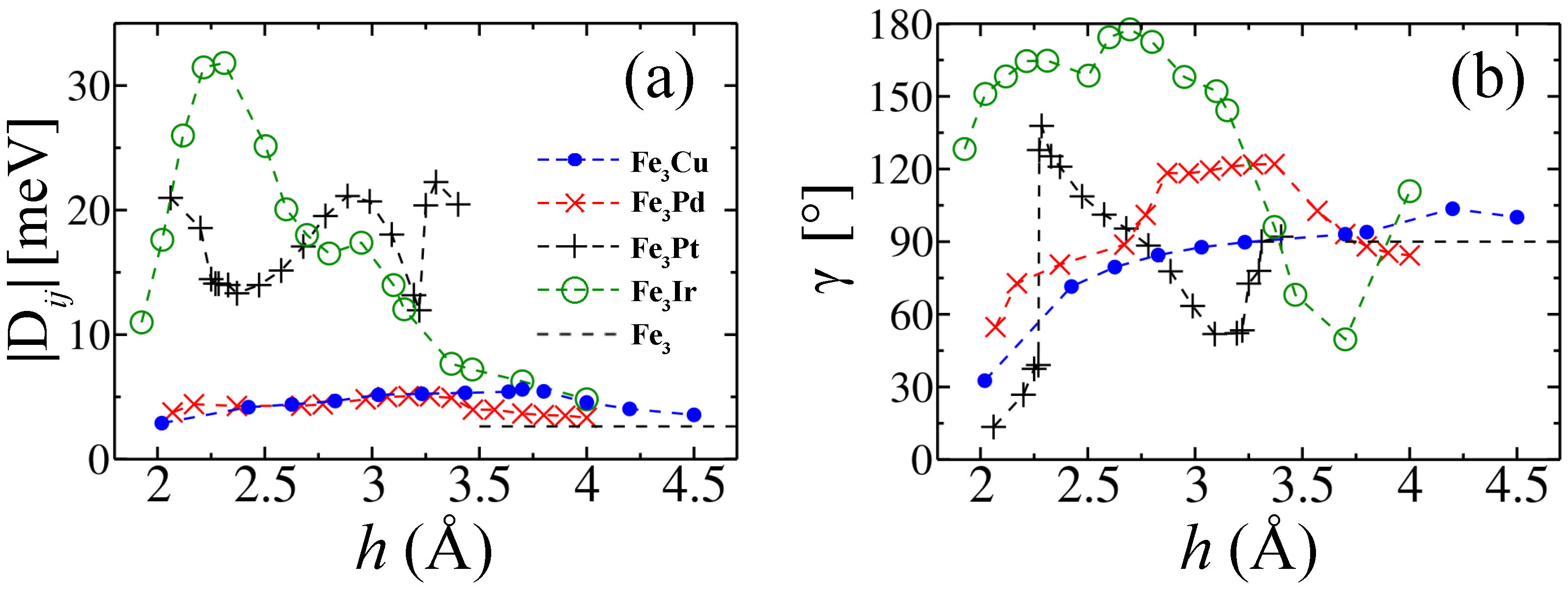In Figure 6, the absolute value $|{\mathit{D}}_{12}|$ and the angle $\gamma$ between ${\mathit{D}}_{12}$ and the positive y axis are shown as a function of the distance h between X and the center of the Fe₃ triangle. As shown in Figure 1e, the positive y axis is the direction perpendicular to the bond between Fe(1) and Fe(2) pointing toward Fe(3) within the Fe-trimer plane.

In Fe₃Cu and Fe₃Pd, the absolute value of the DM vector depends weakly on h remaining in the range $|{\mathit{D}}_{12}|$ = 3–6 meV. Notice that in Fe₃X $|{\mathit{D}}_{12}|$ does not vanish for large h, as in Fe₂X, since the inversion symmetry with respect to the center of the bond between Fe(1) and Fe(2) is already broken by the presence of Fe(3). In fact, calculations for Fe₃ yield $D_{12}^z\simeq 2.6$meV in good agreement with the results for Fe₃X at large h. The DM coupling strengths in Fe₃Cu and Fe₃Pd are very similar, although the spin-orbit coupling in Pd is much larger than in Cu. This behavior is completely different from what is observed in Fe₂Cu and Fe₂Pd, where $|{\mathit{D}}_{12}|$ is much stronger in the case of Pd [see Figure 2a,b]. One concludes that the hybridization with the additional Fe atom in Fe₃X critically modifies the DM interaction between the other two Fe atoms. Therefore, besides the properties of the adatoms, the DM interaction is strongly affected by the local environment of the atoms, as given by the geometry of the nanoclusters and the resulting hybridizations.

Concerning the orientation of ${\mathit{D}}_{12}$, the differences between Fe₃Cu and Fe₃Pd become more significant quantitatively, although some common qualitative trends are shared. At short distances, ${\mathit{D}}_{12}$ is tilted pointing toward the adatom and forming an angle $\gamma =33$° with the y axis for Cu and $\gamma =54$° for Pd [see Figure 6b as well as Figure 1e]. As the distance h increases, ${\mathit{D}}_{12}$ move rapidly toward the normal to the Fe₃ plane in the direction of the adatom, showing some oscillations and, in particular, a maximum $\gamma =122$° for Fe₃Pd at $h\simeq 3.4$Å. Finally, $\gamma \simeq 90$° is reached at large adatom-cluster distances, which corresponds to the result obtained for ${\mathit{D}}_{12}$ in the case of pure Fe₃. The geometry of the clusters qualitatively conditions the orientation of ${\mathit{D}}_{12}$, whereas the Cu or Pd adatoms are important in order to define the precise direction of the DM vector with respect to the cluster structure. It is important to note that the orientation of ${\mathit{D}}_{12}$ (direction and sense) defines both the favored rotation sense as well as the optimal rotation plane of the local magnetic moments of the interacting atoms. For example, if ${\mathit{D}}_{12}$ is pointing along the z axis, a clockwise $(-)$ [counterclockwise $(+)$] rotation direction within the $xy$ plane is the most [least] favorable.

The significantly heavier Pt and Ir adatoms yield distinctive strong distance dependencies of ${\mathit{D}}_{12}$. In the case of Fe₃Pt, the absolute value $|{\mathit{D}}_{12}|$ exhibits oscillations between the maxima $|{\mathit{D}}_{12}|\simeq 20$–22 meV at $h=2.1$Å, 2.9 Å and 3.3 Å and the minima $|{\mathit{D}}_{12}|\simeq 13$–14 meV at $h=2.3$Å and 3.2 Å. In the case of Fe₃Ir, $|{\mathit{D}}_{12}|$ increases for small h from $|{\mathit{D}}_{12}|\simeq 11$ meV at $h=1.9$Å to ${\mathit{D}}_{12}\simeq 32$ meV at $h=2.3$Å. As in the case of Fe₂X, Ir yields the strongest DM couplings among the considered Fe₃X. For large distances, $|{\mathit{D}}_{12}|$ rapidly decreases to ${\mathit{D}}_{12}\simeq 7$meV at $h=3.5$Å with an intermediate local maximum ${\mathit{D}}_{12}\simeq 17$meV at $h=2.9$Å. Notice that the influence of the Pt and Ir adatoms is still significant, even at the largest considered h. The fact that Pt and Ir show such different behaviors, although the atomic numbers are similar, emphasizes the role of the d-band filling on the DM coupling, in agreement with the conclusion drawn for Fe₂X clusters.

The Pt and Ir adatoms also have a major influence on the orientation of ${\mathit{D}}_{12}$. In the case of Fe₃Pt and at short distances $h\simeq 2.1$Å, ${\mathit{D}}_{12}$ is close to the positive y axis with $\gamma \simeq 14$°. As the distance h is increased, ${\mathit{D}}_{12}$ rapidly rotates to $\gamma \simeq 138$° at $h\simeq 2.3$Å, with its component $D_{12}^y$ now being negative. For even larger h, ${\mathit{D}}_{12}$ rotates back, first in the direction of the normal to the Fe₃ plane and then further toward the positive y axis, with $\gamma$ almost linearly decreasing until $\gamma \simeq 53$° is reached at $h\simeq 3.2$Å. Finally, this is followed by an increase toward $\gamma \simeq 92$° at $h\simeq 3.4$Å. In the case of Fe₃Ir, the orientation dependence is far smoother. Starting from $\gamma \simeq 128$° at $h\simeq 1.9$Å, ${\mathit{D}}_{12}$ gradually rotates toward the negative y axis reaching $\gamma \simeq 178$° at $h\simeq 2.7$Å, i.e., almost parallel to the negative y axis. Subsequently, ${\mathit{D}}_{12}$ reorients itself toward the z axis and beyond it, until $\gamma \simeq 50$° is reached at $h\simeq 3.7$Å. At even larger h, ${\mathit{D}}_{12}$ rotates again approaching the normal to the Fe₃ plane with $\gamma \simeq 104$° at $h\simeq 4$Å. In summary, both the absolute value as well as the orientation of ${\mathit{D}}_{12}$ exhibit non-monotonous behaviors as a function of h. Therefore, they open the most interesting possibilities of manipulating the chirality in a magnetic nanostructure.

5. Conclusions

The chirality of the magnetic interactions between Fe atoms in Fe₂X and Fe₃X clusters with X = Cu, Pd, Pt, and Ir has been investigated in the framework of density-functional theory. In Fe₂X clusters, the adatoms trigger the DM interaction by breaking the inversion symmetry of the dimer, thereby unraveling a rich variety of chiral magnetic behaviors that strongly depend on the nature of the adatom as well as on their distance to the Fe atoms. Three different local and gradient-corrected approximations to the exchange and correlation functional have been considered, which yield qualitatively very similar trends, as well some significant quantitative differences. The microscopic origin of the adatom-induced DM coupling has been investigated by computing the contributions of the different atoms to the chirality of the spin-orbit energy in Fe₂X. The results show that the DM couplings originate primarily on the spin-orbit energy of the adatoms, even in the case of a light atom such as Cu, which has a complete d shell. These trends are consistent with previous interpretations of the DM couplings as the result of indirect interactions with heavy open-d-shell atoms [9].

Adatoms showing stronger spin-orbit coupling generally result in stronger and more interesting DM couplings. Furthermore, the d-band filling of the adatom has also been found to be a most relevant parameter for controlling the chiral magnetic properties of the clusters. In fact, the strength of spin-orbit coupling alone is not enough in order to define the significance of the symmetry breaking effect. In particular, we have found that increasing the number of d holes upon changing Pt by Ir results in stronger and more complex DM interactions. Comparison between the symmetric and antisymmetric Fe-Fe couplings in Fe₂X shows that the DM couplings are about two orders of magnitude weaker than the isotropic Heisenberg interactions. However, they are in general stronger and more sensitive to dimer-adatom hybridizations than the anisotropy of the symmetric couplings.

The results reported in this work motivate further investigations on larger clusters and nanoalloys in order to understand the size and environment dependence of the DM interaction. The role of cluster symmetry and the possibilities of tuning it by means of alloying should be particularly emphasized. Furthermore, in order to stress the role of the d-band filling, it is interesting to expand the investigation to other magnetic transition metals, for example, Ni, Co, Mn, and Cr. In particular, Mn and Cr, with a nearly half-filled d-band, are promising candidates for an exceptionally strong DM coupling [13]. Finally, a more thorough analysis of the anisotropy of the magnetic interactions in small clusters requires the examination of all contributions to the interaction energy, including the symmetric and thus nonchiral interactions $J_{ij}$ and ${\mathit{W}}_{ij}^{\mathrm{S}}$ for different adatoms. Using these ab initio-derived interactions in the framework of phenomenological spin models, it would be possible to investigate the magnetic energy landscapes of the clusters, including metastable magnetic configurations as well as the relevant transition states connecting them. In this way, a quantitative material specific study of dynamical processes such as magnetic relaxation and magnetization reversals would be possible.