Spin and Orbital Symmetry Breakings Central to the Laser-Induced Ultrafast Demagnetization of Transition Metals
Abstract
:1. Introduction
2. Theory
3. Results and Discussion
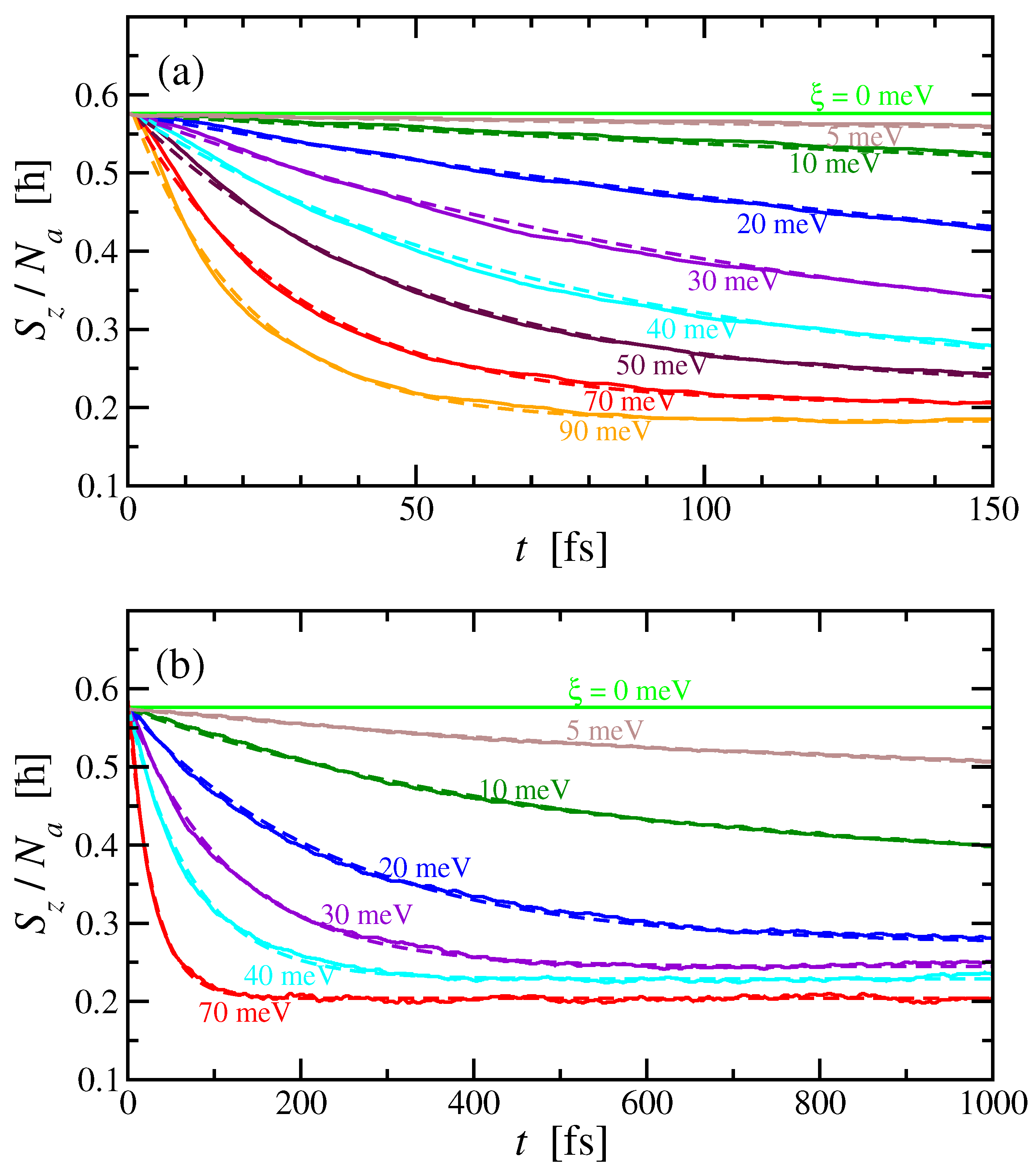
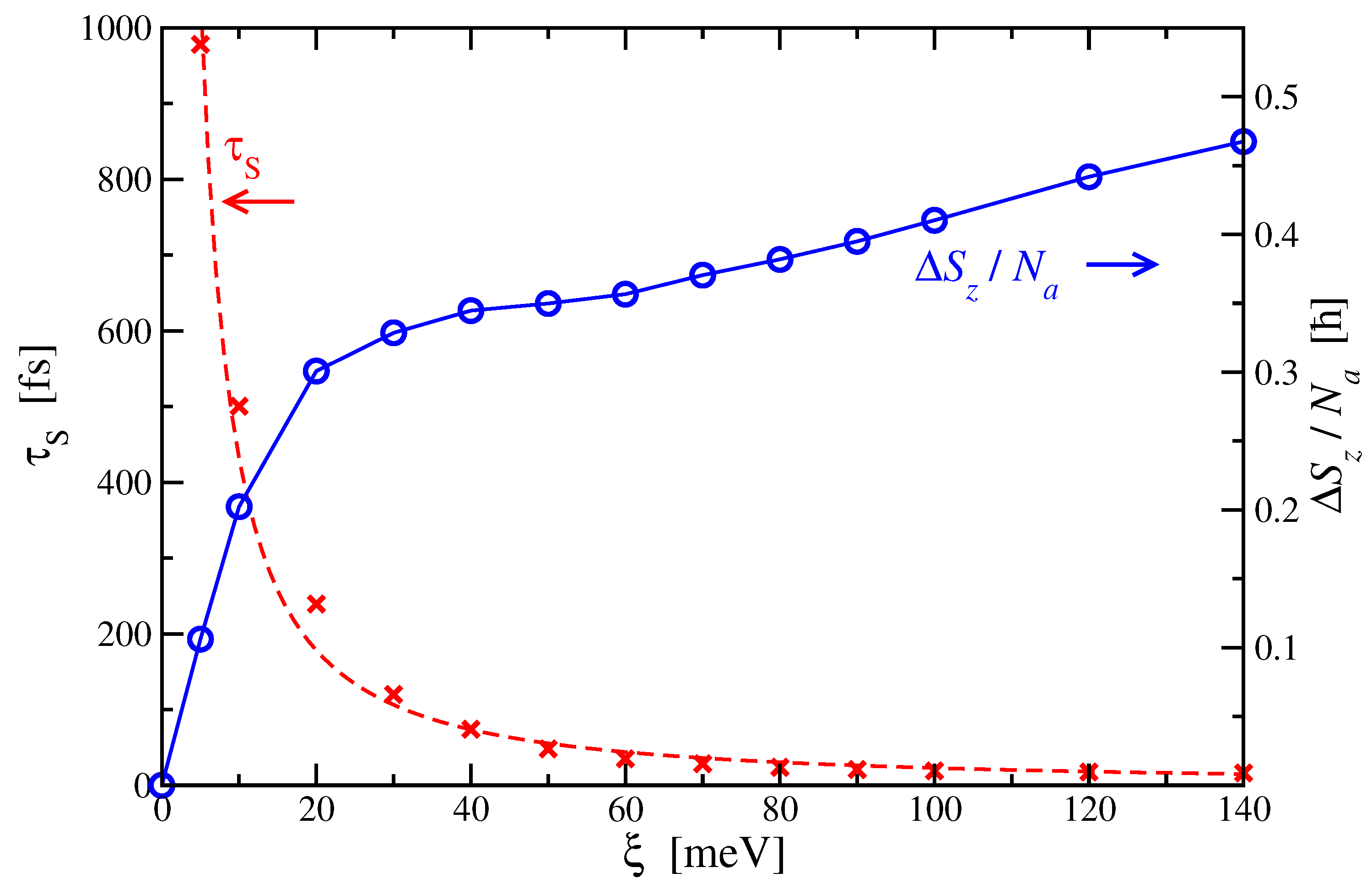
3.1. Breaking Spin-Rotational Symmetry
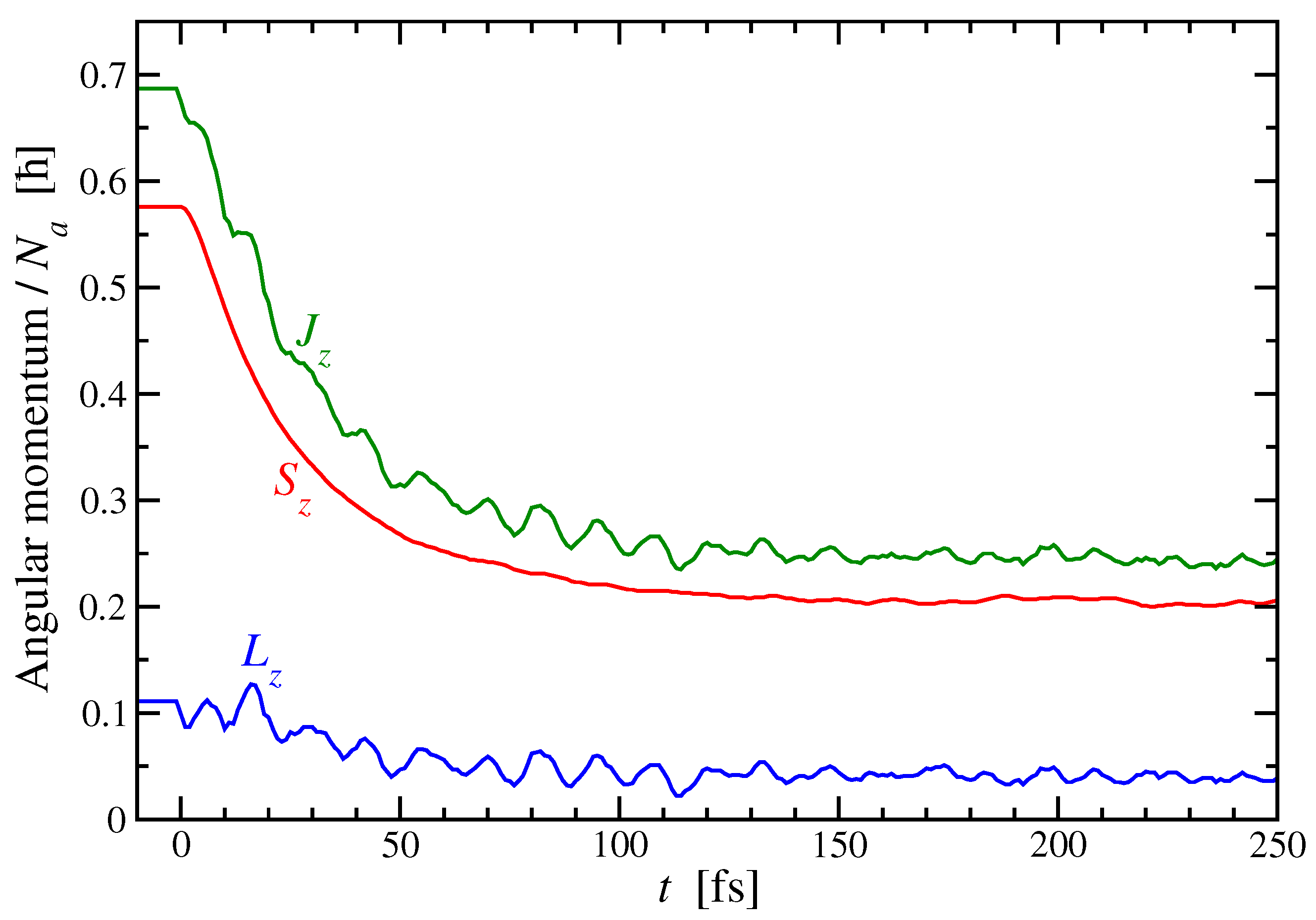
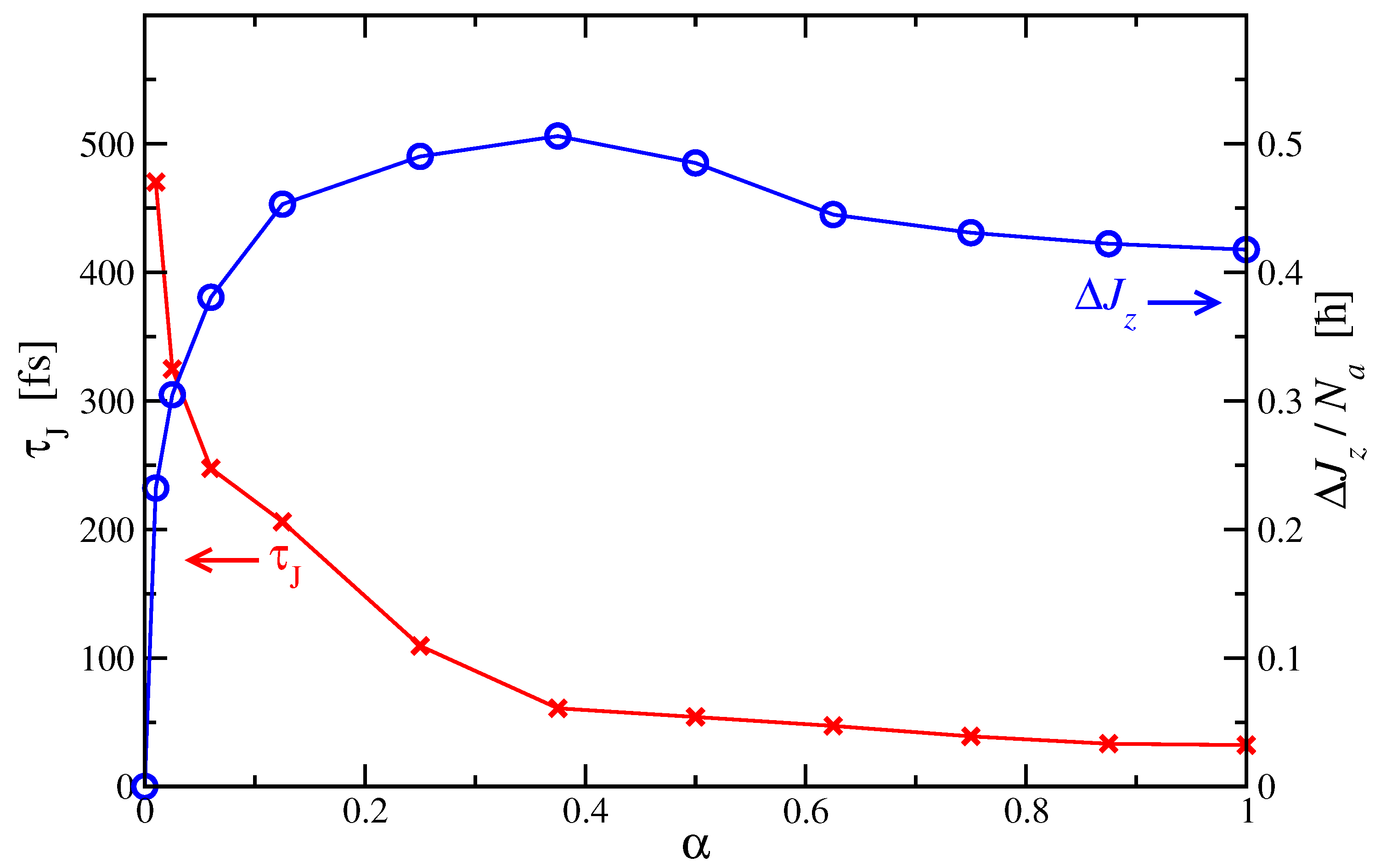
3.2. Breaking Orbital Rotational Symmetry
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mermin, N.D.; Wagner, H. Absence of Ferromagnetism or Antiferromagnetism in One- or Two-Dimensional Isotropic Heisenberg Models. Phys. Rev. Lett. 1966, 17, 1133–1136. [Google Scholar] [CrossRef]
- Beaurepaire, E.; Merle, J.C.; Daunois, A.; Bigot, J.Y. Ultrafast Spin Dynamics in Ferromagnetic Nickel. Phys. Rev. Lett. 1996, 76, 4250–4253. [Google Scholar] [CrossRef]
- Rhie, H.S.; Dürr, H.A.; Eberhardt, W. Femtosecond Electron and Spin Dynamics in Ni/W(110) Films. Phys. Rev. Lett. 2003, 90, 247201. [Google Scholar] [CrossRef]
- Stamm, C.; Kachel, T.; Pontius, N.; Mitzner, R.; Quast, T.; Holldack, K.; Khan, S.; Lupulescu, C.; Aziz, E.F.; Wietstruk, M.; et al. Femtosecond modification of electron localization and transfer of angular momentum in nickel. Nat. Mater. 2007, 6, 740. [Google Scholar] [CrossRef]
- Stamm, C.; Pontius, N.; Kachel, T.; Wietstruk, M.; Dürr, H.A. Femtosecond X-ray absorption spectroscopy of spin and orbital angular momentum in photoexcited Ni films during ultrafast demagnetization. Phys. Rev. B 2010, 81, 104425. [Google Scholar] [CrossRef]
- Boeglin, C.; Beaurepaire, E.; Halté, V.; López-Flores, V.; Stamm, C.; Pontius, N.; Dürr, H.A.; Bigot, J.Y. Distinguishing the ultrafast dynamics of spin and orbital moments in solids. Nature 2010, 465, 458. [Google Scholar] [CrossRef]
- Turgut, E.; Zusin, D.; Legut, D.; Carva, K.; Knut, R.; Shaw, J.M.; Chen, C.; Tao, Z.; Nembach, H.T.; Silva, T.J.; et al. Stoner versus Heisenberg: Ultrafast exchange reduction and magnon generation during laser-induced demagnetization. Phys. Rev. B 2016, 94, 220408. [Google Scholar] [CrossRef]
- You, W.; Tengdin, P.; Chen, C.; Shi, X.; Zusin, D.; Zhang, Y.; Gentry, C.; Blonsky, A.; Keller, M.; Oppeneer, P.M.; et al. Revealing the Nature of the Ultrafast Magnetic Phase Transition in Ni by Correlating Extreme Ultraviolet Magneto-Optic and Photoemission Spectroscopies. Phys. Rev. Lett. 2018, 121, 077204. [Google Scholar] [CrossRef]
- Tengdin, P.; You, W.; Chen, C.; Shi, X.; Zusin, D.; Zhang, Y.; Gentry, C.; Blonsky, A.; Keller, M.; Oppeneer, P.M.; et al. Critical behavior within 20 fs drives the out-of-equilibrium laser-induced magnetic phase transition in nickel. Sci. Adv. 2018, 4, eaap9744. [Google Scholar] [CrossRef]
- Eschenlohr, A.; Battiato, M.; Maldonado, P.; Pontius, N.; Kachel, T.; Holldack, K.; Mitzner, R.; Föhlisch, A.; Oppeneer, P.M.; Stamm, C. Ultrafast spin transport as key to femtosecond demagnetization. Nat. Mater. 2013, 12, 332. [Google Scholar] [CrossRef]
- Vodungbo, B.; Tudu, B.; Perron, J.; Delaunay, R.; Müller, L.; Berntsen, M.H.; Grübel, G.; Malinowski, G.; Weier, C.; Gautier, J.; et al. Indirect excitation of ultrafast demagnetization. Sci. Rep. 2016, 6, 18970. [Google Scholar] [CrossRef]
- Bergeard, N.; Hehn, M.; Mangin, S.; Lengaigne, G.; Montaigne, F.; Lalieu, M.L.M.; Koopmans, B.; Malinowski, G. Hot-Electron-Induced Ultrafast Demagnetization in Co/Pt Multilayers. Phys. Rev. Lett. 2016, 117, 147203. [Google Scholar] [CrossRef]
- Krauß, M.; Roth, T.; Alebrand, S.; Steil, D.; Cinchetti, M.; Aeschlimann, M.; Schneider, H.C. Ultrafast demagnetization of ferromagnetic transition metals: The role of the Coulomb interaction. Phys. Rev. B 2009, 80, 180407. [Google Scholar] [CrossRef]
- Krieger, K.; Dewhurst, J.K.; Elliott, P.; Sharma, S.; Gross, E.K.U. Laser-Induced Demagnetization at Ultrashort Time Scales: Predictions of TDDFT. J. Chem. Theory Comput. 2015, 11, 4870–4874. [Google Scholar] [CrossRef]
- Töws, W.; Pastor, G.M. Many-Body Theory of Ultrafast Demagnetization and Angular Momentum Transfer in Ferromagnetic Transition Metals. Phys. Rev. Lett. 2015, 115, 217204. [Google Scholar] [CrossRef]
- Shokeen, V.; Sanchez Piaia, M.; Bigot, J.Y.; Müller, T.; Elliott, P.; Dewhurst, J.K.; Sharma, S.; Gross, E.K.U. Spin Flips versus Spin Transport in Nonthermal Electrons Excited by Ultrashort Optical Pulses in Transition Metals. Phys. Rev. Lett. 2017, 119, 107203. [Google Scholar] [CrossRef]
- Dewhurst, J.K.; Elliott, P.; Shallcross, S.; Gross, E.K.U.; Sharma, S. Laser-Induced Intersite Spin Transfer. Nano Lett. 2018, 18, 1842–1848. [Google Scholar] [CrossRef]
- Töws, W.; Pastor, G.M. Tuning the laser-induced ultrafast demagnetization of transition metals. Phys. Rev. B 2019, 100, 024402. [Google Scholar] [CrossRef]
- Koopmans, B.; Kicken, H.; van Kampen, M.; de Jonge, W. Microscopic model for femtosecond magnetization dynamics. J. Magn. Magn. Mater. 2005, 286, 271–275. [Google Scholar] [CrossRef]
- Koopmans, B.; Malinowski, G.; Dalla Longa, F.; Steiauf, D.; Fähnle, M.; Roth, T.; Cinchetti, M.; Aeschlimann, M. Explaining the paradoxical diversity of ultrafast laser-induced demagnetization. Nat. Mater. 2010, 9, 259. [Google Scholar] [CrossRef]
- Battiato, M.; Carva, K.; Oppeneer, P.M. Superdiffusive Spin Transport as a Mechanism of Ultrafast Demagnetization. Phys. Rev. Lett. 2010, 105, 027203. [Google Scholar] [CrossRef]
- Battiato, M.; Carva, K.; Oppeneer, P.M. Theory of laser-induced ultrafast superdiffusive spin transport in layered heterostructures. Phys. Rev. B 2012, 86, 024404. [Google Scholar] [CrossRef]
- Steiauf, D.; Illg, C.; Fähnle, M. Extension of Yafet’s theory of spin relaxation to ferromagnets. J. Magn. Magn. Mater. 2010, 322, L5–L7. [Google Scholar] [CrossRef]
- Fähnle, M.; Illg, C. Electron theory of fast and ultrafast dissipative magnetization dynamics. J. Phys. Condens. Matter 2011, 23, 493201. [Google Scholar] [CrossRef]
- Carva, K.; Battiato, M.; Oppeneer, P.M. Ab Initio Investigation of the Elliott-Yafet Electron-Phonon Mechanism in Laser-Induced Ultrafast Demagnetization. Phys. Rev. Lett. 2011, 107, 207201. [Google Scholar] [CrossRef]
- Garibay-Alonso, R.; Dorantes-Dávila, J.; Pastor, G.M. Noncollinear spin-fluctuation theory of transition-metal magnetism: Role of transverse spin fluctuations in Fe. Phys. Rev. B 2015, 91, 184408. [Google Scholar] [CrossRef]
- Hubbard, J. The magnetism of iron. Phys. Rev. B 1979, 19, 2626–2636. [Google Scholar] [CrossRef]
- Hubbard, J. Magnetism of iron. II. Phys. Rev. B 1979, 20, 4584–4595. [Google Scholar] [CrossRef]
- Hasegawa, H. Single-Siet Spin Fluctuation Theory of Itinerant-Electron Systems with Narrow Bands. J. Phys. Soc. Jpn. 1980, 49, 178–188. [Google Scholar] [CrossRef]
- Hasegawa, H. Single-Site Spin Fluctuation Theory of Itinerant-Electron Systems with Narrow Bands. II. Iron and Nickel. J. Phys. Soc. Jpn. 1980, 49, 963–971. [Google Scholar] [CrossRef]
- Bruno, P. Physical origins and theoretical models of magnetic anisotropy. In Magnetismus von Festkörpern und Grenzflächen; Forschungszentrum Jülich: Jülich, Germany, 1993; Chapter 24. [Google Scholar]
- Victora, R.H.; Falicov, L.M. Exact Solution of a Four-Site d-Electron Problem: The Nickel-Metal Photoemission Spectrum. Phys. Rev. Lett. 1985, 55, 1140–1143. [Google Scholar] [CrossRef]
- Hüfner, S.; Wertheim, G. Multielectron effects in the XPS spectra of nickel. Phys. Lett. A 1975, 51, 299–300. [Google Scholar] [CrossRef]
- Eberhardt, W.; Plummer, E.W. Angle-resolved photoemission determination of the band structure and multielectron excitations in Ni. Phys. Rev. B 1980, 21, 3245–3255. [Google Scholar] [CrossRef]
- Feldkamp, L.A.; Davis, L.C. X-ray photoemission spectra of core levels in Ni metal. Phys. Rev. B 1980, 22, 3644–3653. [Google Scholar] [CrossRef]
- Papaconstantopoulos, D.A. Handbook of the Band Structure of Elemental Solids; Plenum Press: New York, NY, USA, 1986. [Google Scholar]
- Slater, J.C.; Koster, G.F. Simplified LCAO Method for the Periodic Potential Problem. Phys. Rev. 1954, 94, 1498–1524. [Google Scholar] [CrossRef]
- Tannor, D.J. Introduction to Quantum Mechanics: A time-Dependent Perspective; University Science Books: Sausalito, CA, USA, 2007. [Google Scholar]
- Guirado-López, R.A.; Dorantes-Dávila, J.; Pastor, G.M. Orbital Magnetism in Transition-Metal Clusters: From Hund’s Rules to Bulk Quenching. Phys. Rev. Lett. 2003, 90, 226402. [Google Scholar] [CrossRef]
- Ashcroft, N.; Mermin, N. Solid State Physics; Thomson Learning: London, UK, 1976. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Töws, W.; Stegmann, G.; Pastor, G.M. Spin and Orbital Symmetry Breakings Central to the Laser-Induced Ultrafast Demagnetization of Transition Metals. Symmetry 2023, 15, 457. https://doi.org/10.3390/sym15020457
Töws W, Stegmann G, Pastor GM. Spin and Orbital Symmetry Breakings Central to the Laser-Induced Ultrafast Demagnetization of Transition Metals. Symmetry. 2023; 15(2):457. https://doi.org/10.3390/sym15020457
Chicago/Turabian StyleTöws, Waldemar, Gunnar Stegmann, and G. M. Pastor. 2023. "Spin and Orbital Symmetry Breakings Central to the Laser-Induced Ultrafast Demagnetization of Transition Metals" Symmetry 15, no. 2: 457. https://doi.org/10.3390/sym15020457
APA StyleTöws, W., Stegmann, G., & Pastor, G. M. (2023). Spin and Orbital Symmetry Breakings Central to the Laser-Induced Ultrafast Demagnetization of Transition Metals. Symmetry, 15(2), 457. https://doi.org/10.3390/sym15020457




