Effects of Joule Heating, Viscous Dissipation, and Activation Energy on Nanofluid Flow Induced by MHD on a Vertical Surface
Abstract
1. Introduction
2. Mathematical Formulation
3. Numerical Method
4. Results and Discussion
5. Conclusions
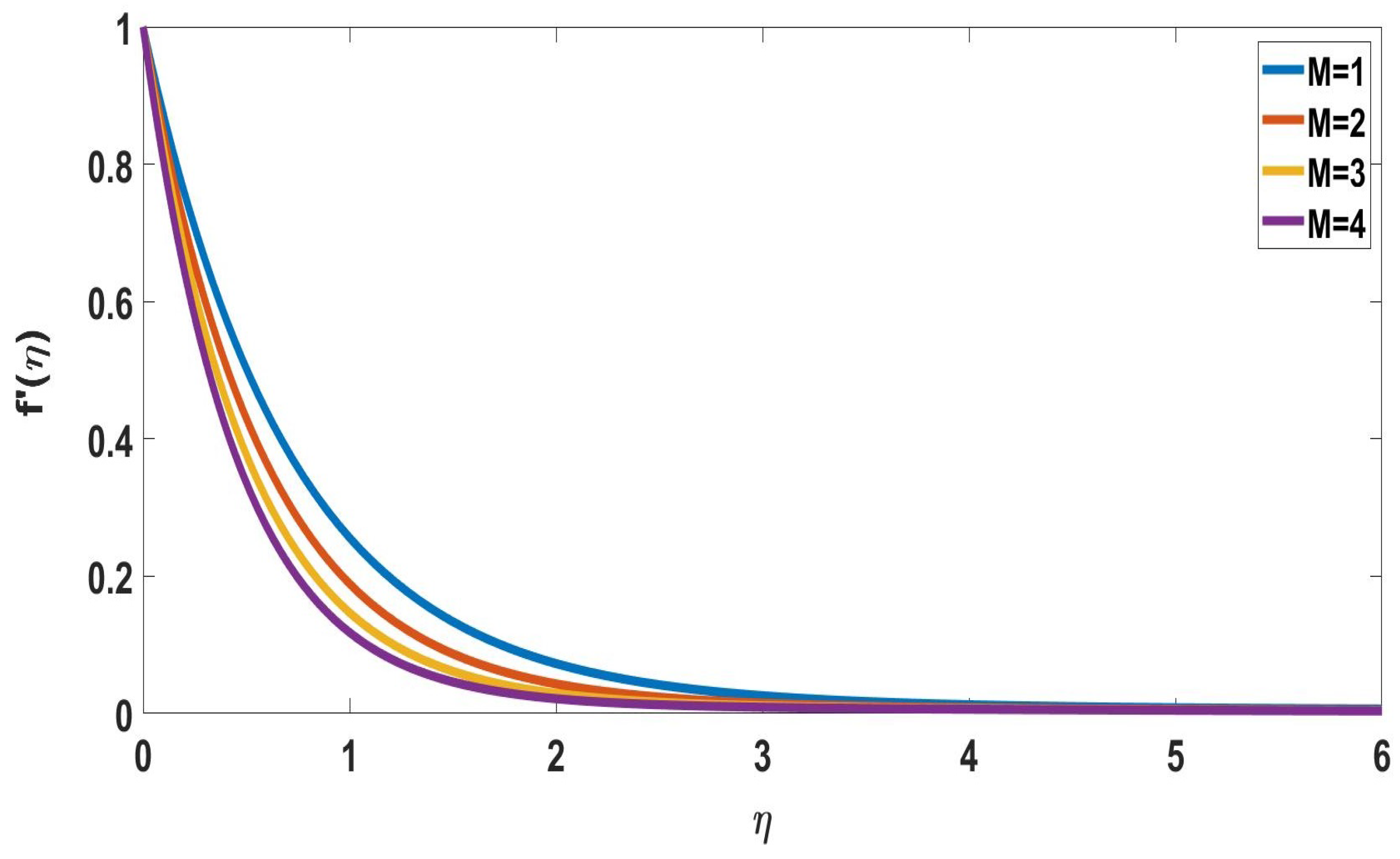
- As the magnetic parameter increases, the velocity profile over the vertical surface decreases.
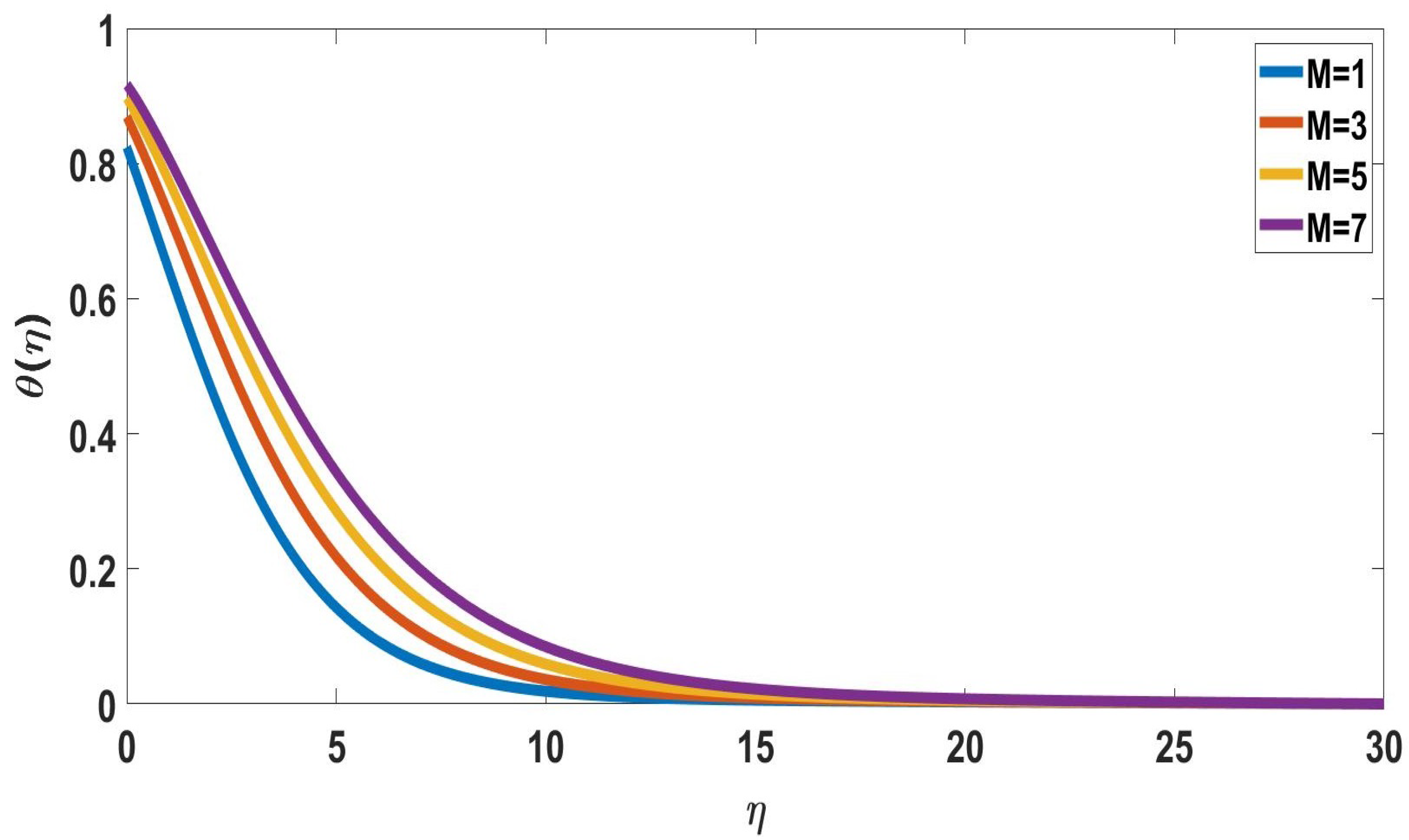
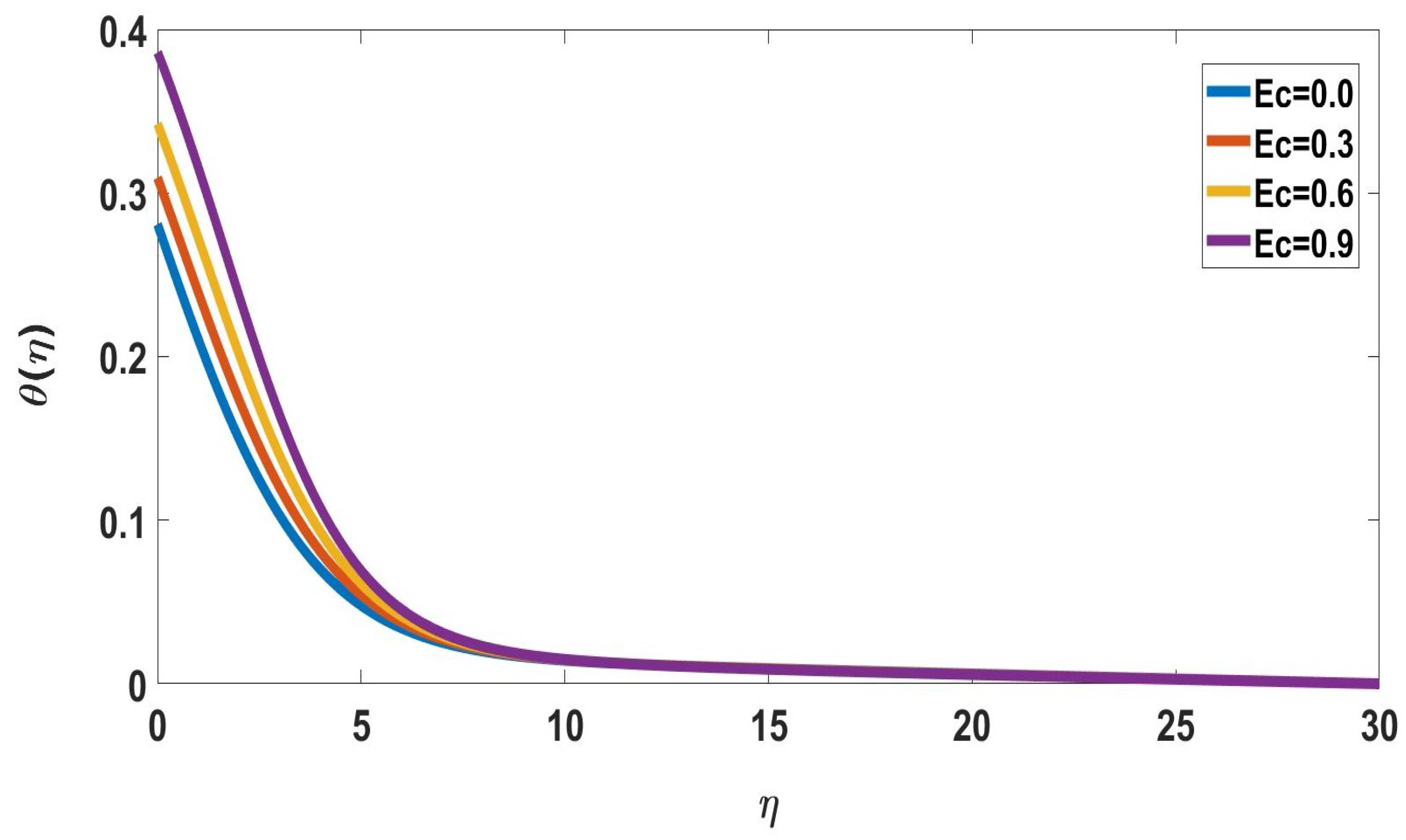
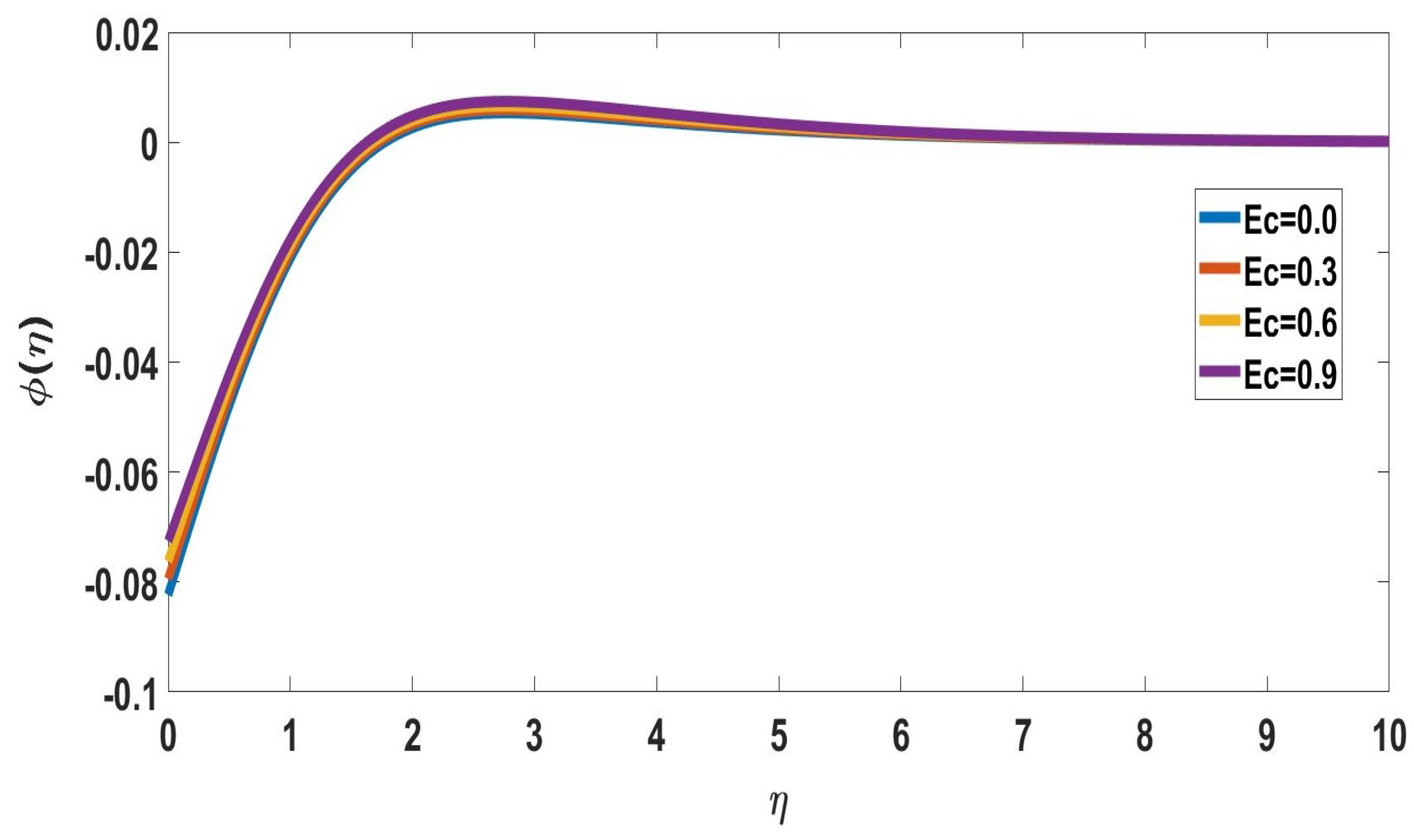
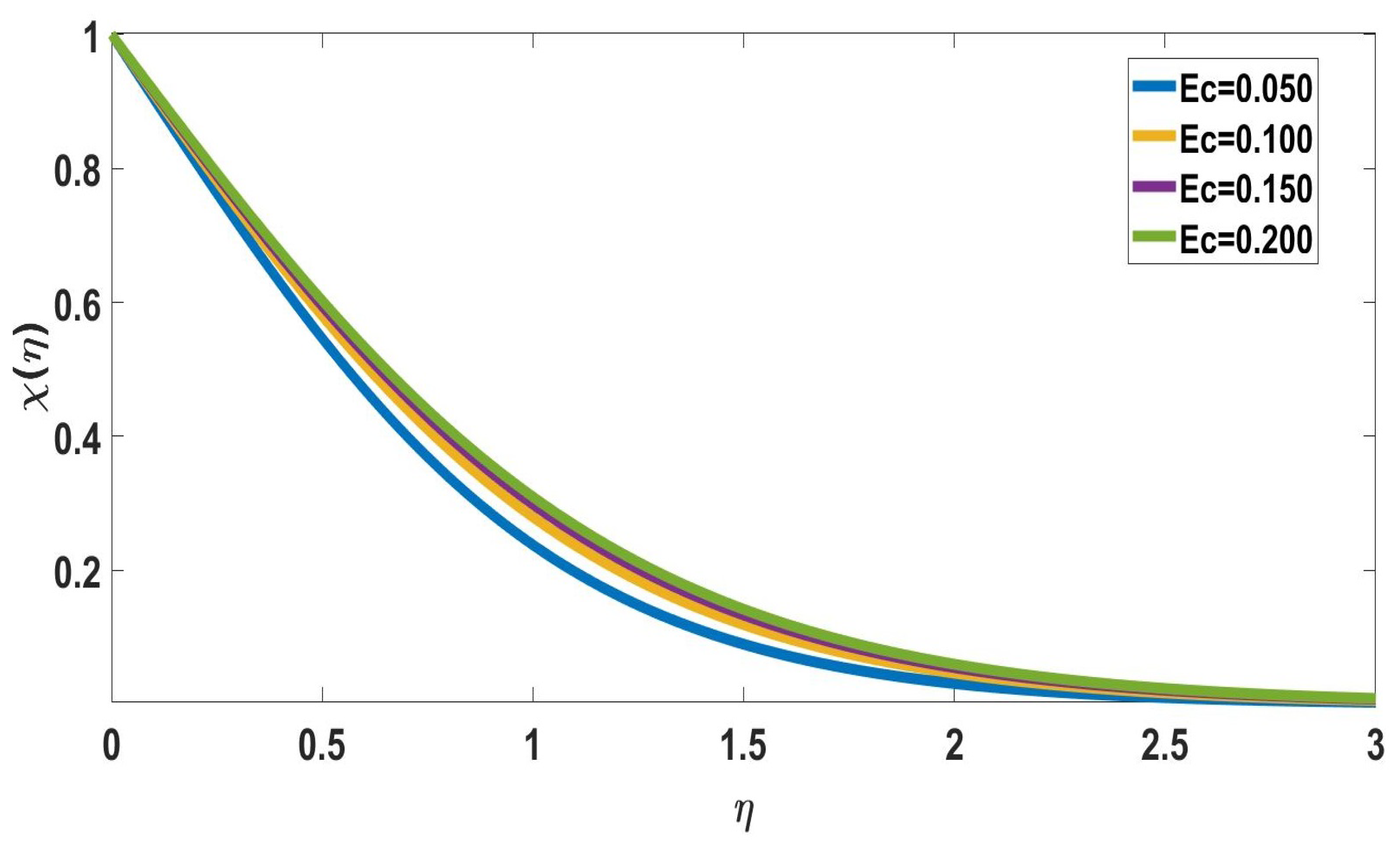
- The temperature of the fluid rises as the magnetic parameters and Eckert number increases.
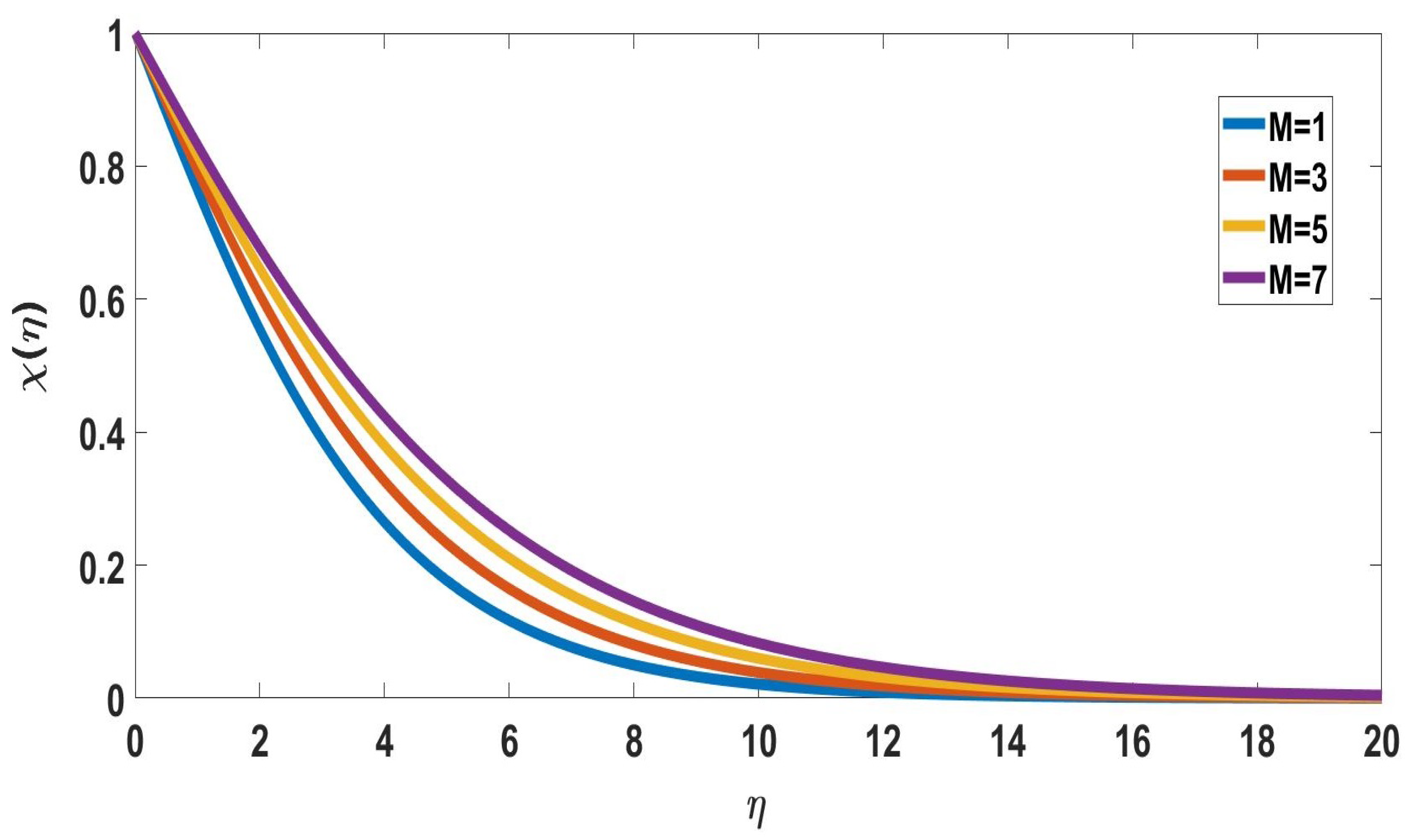
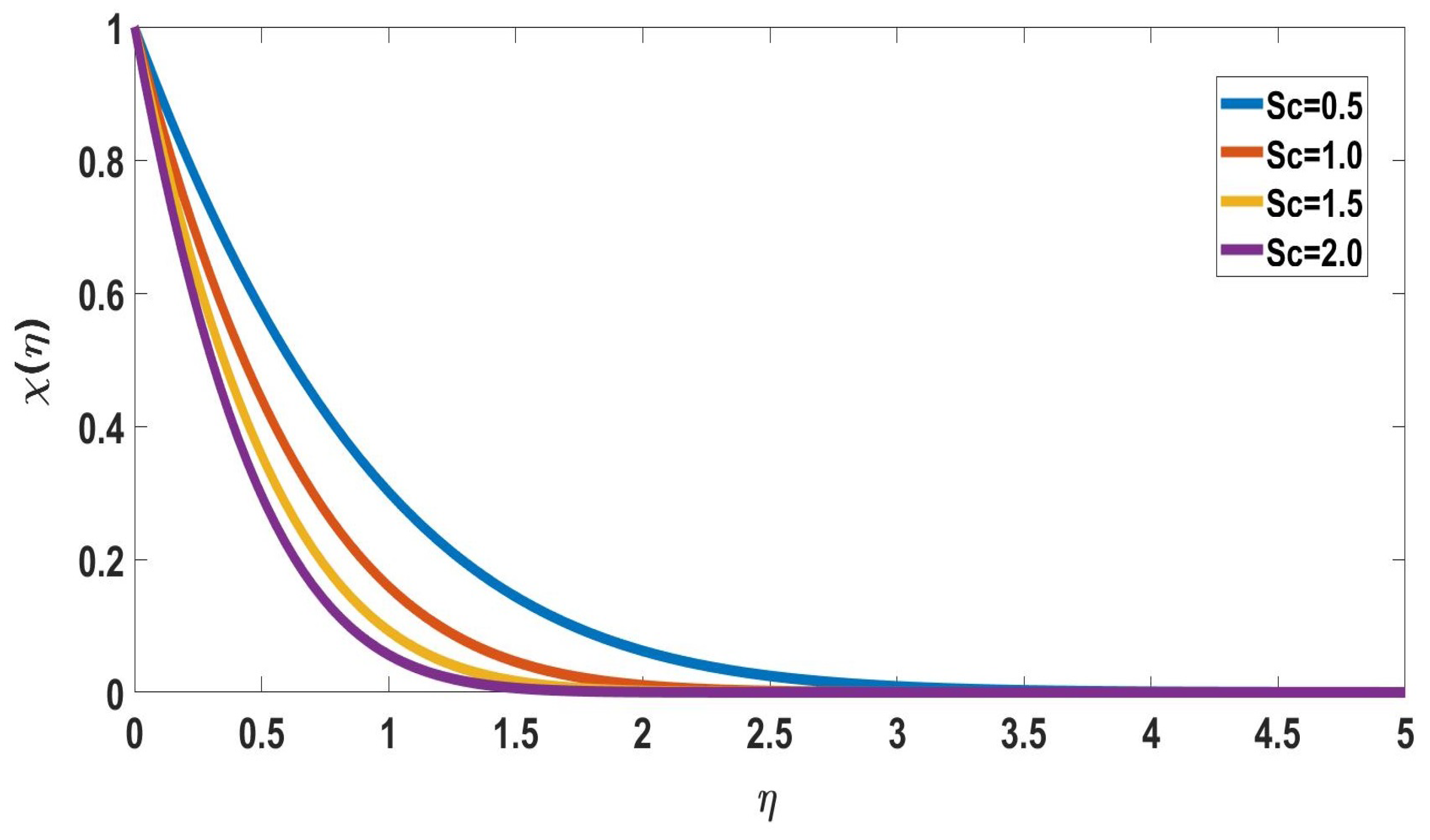
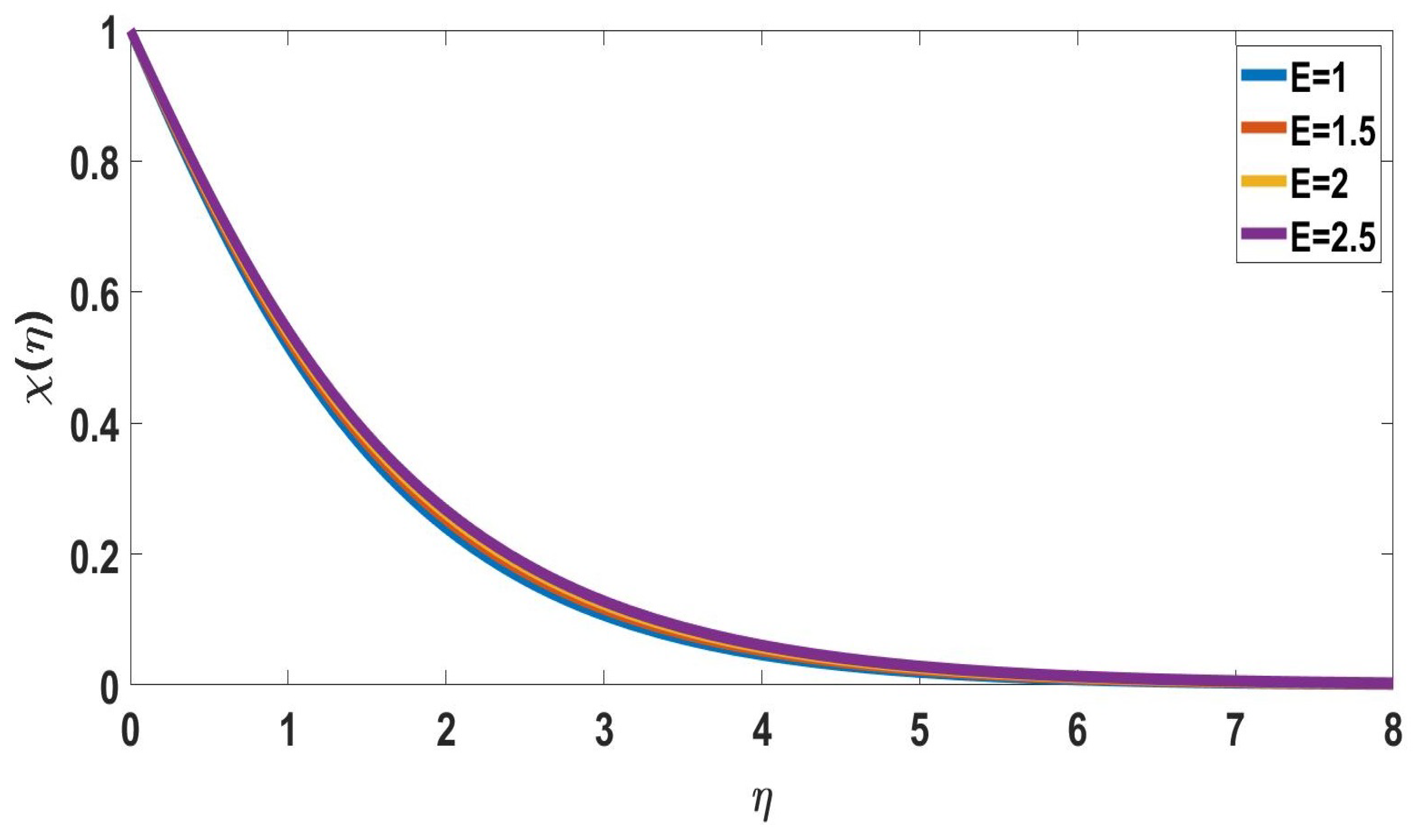
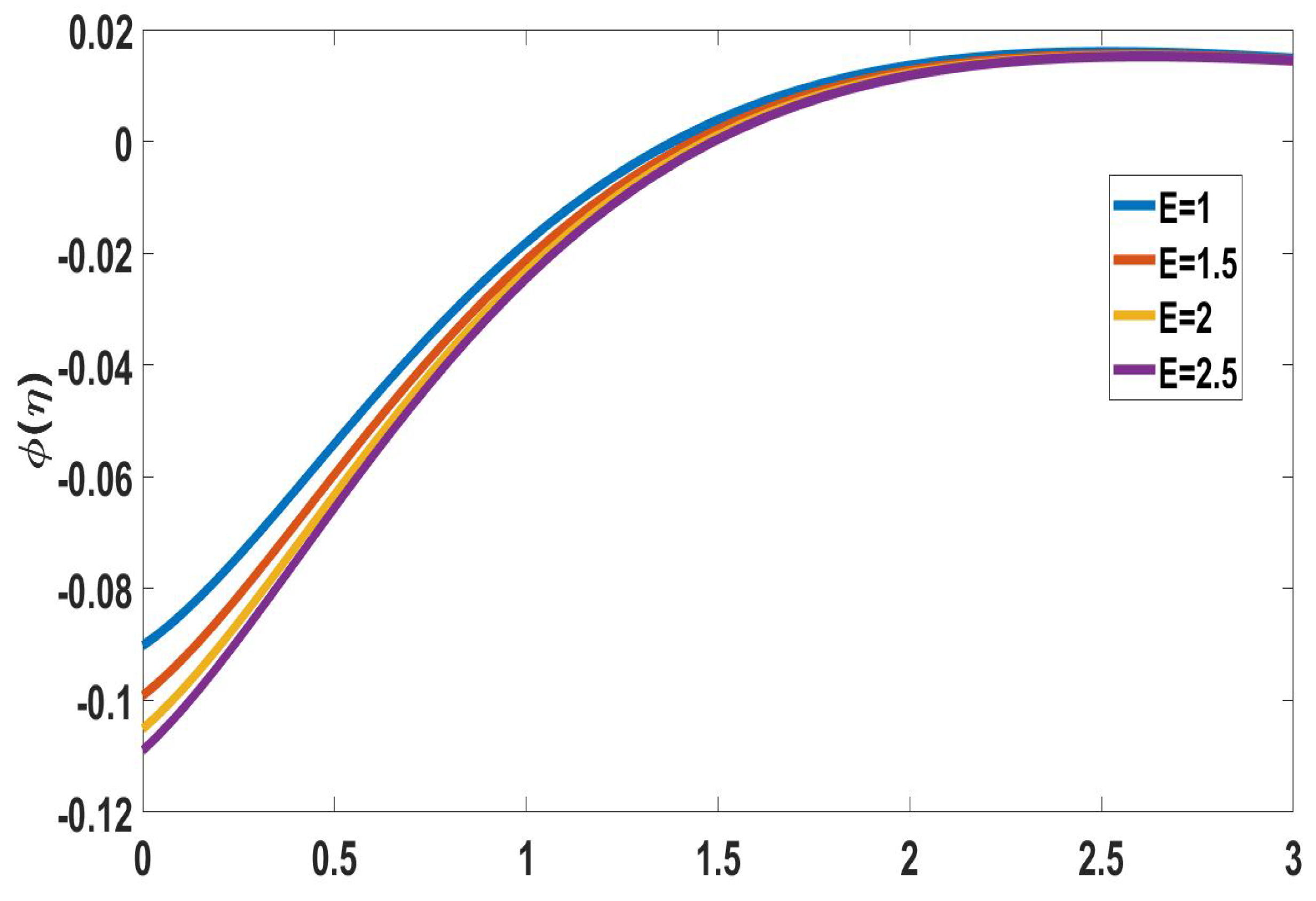
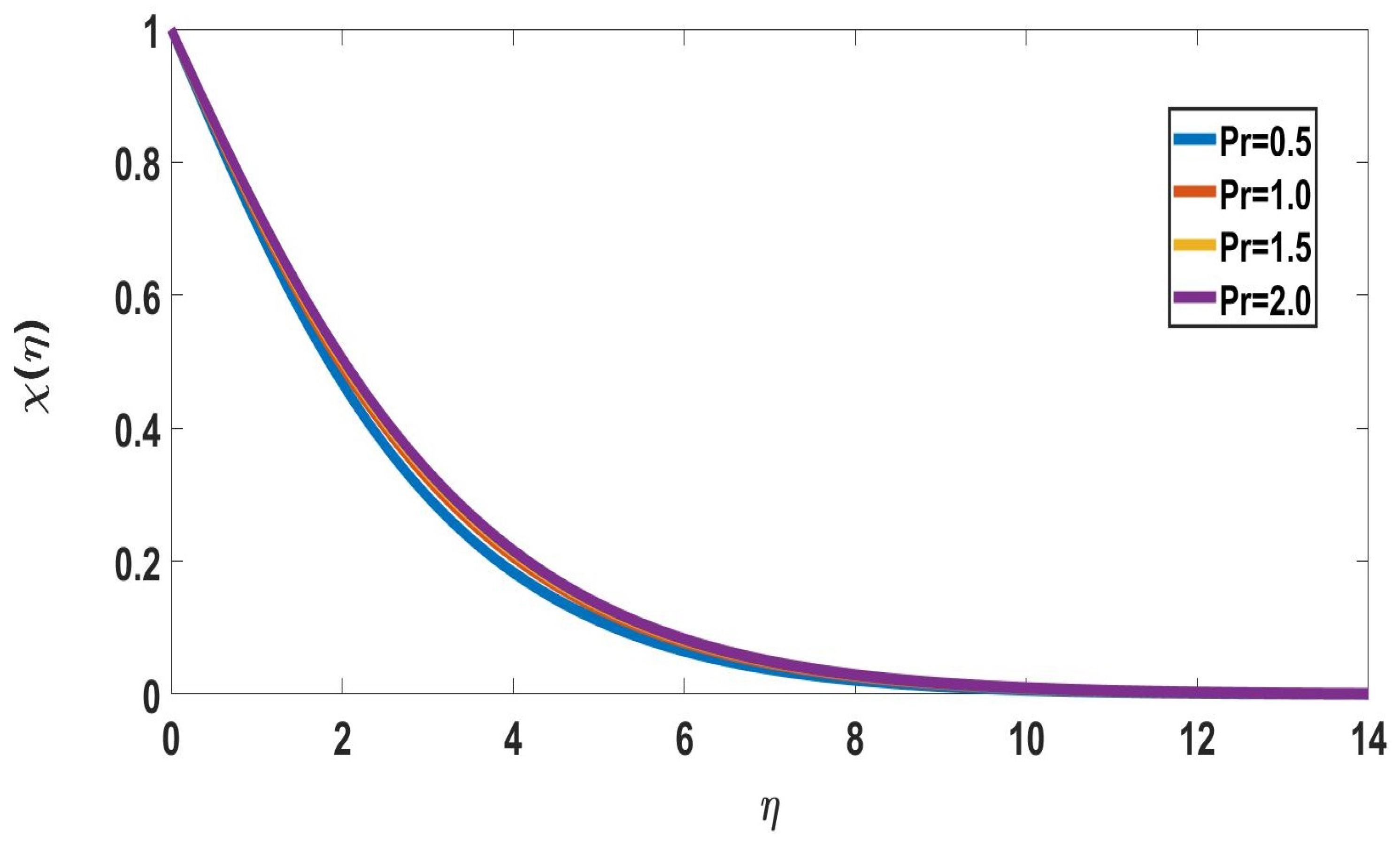
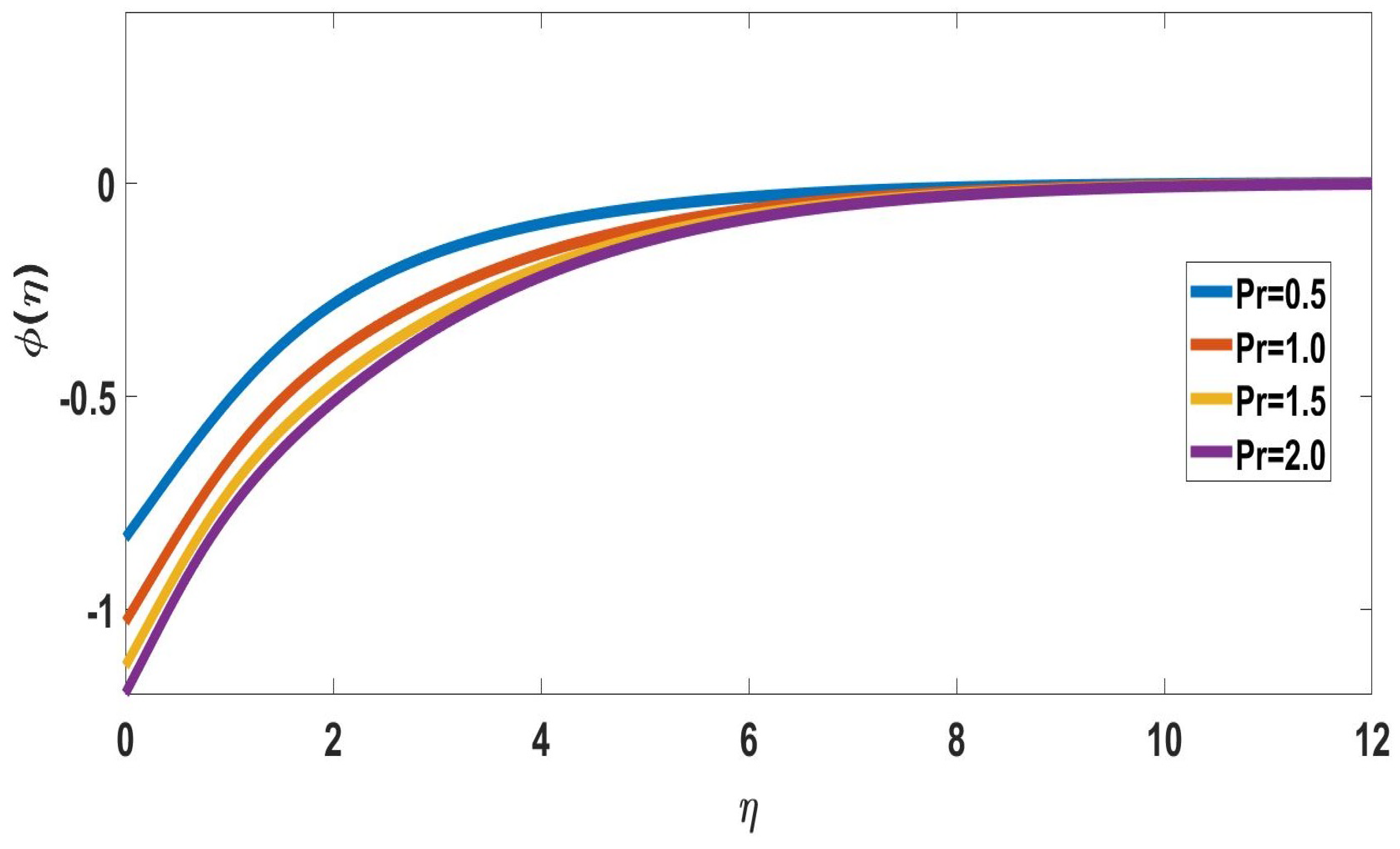
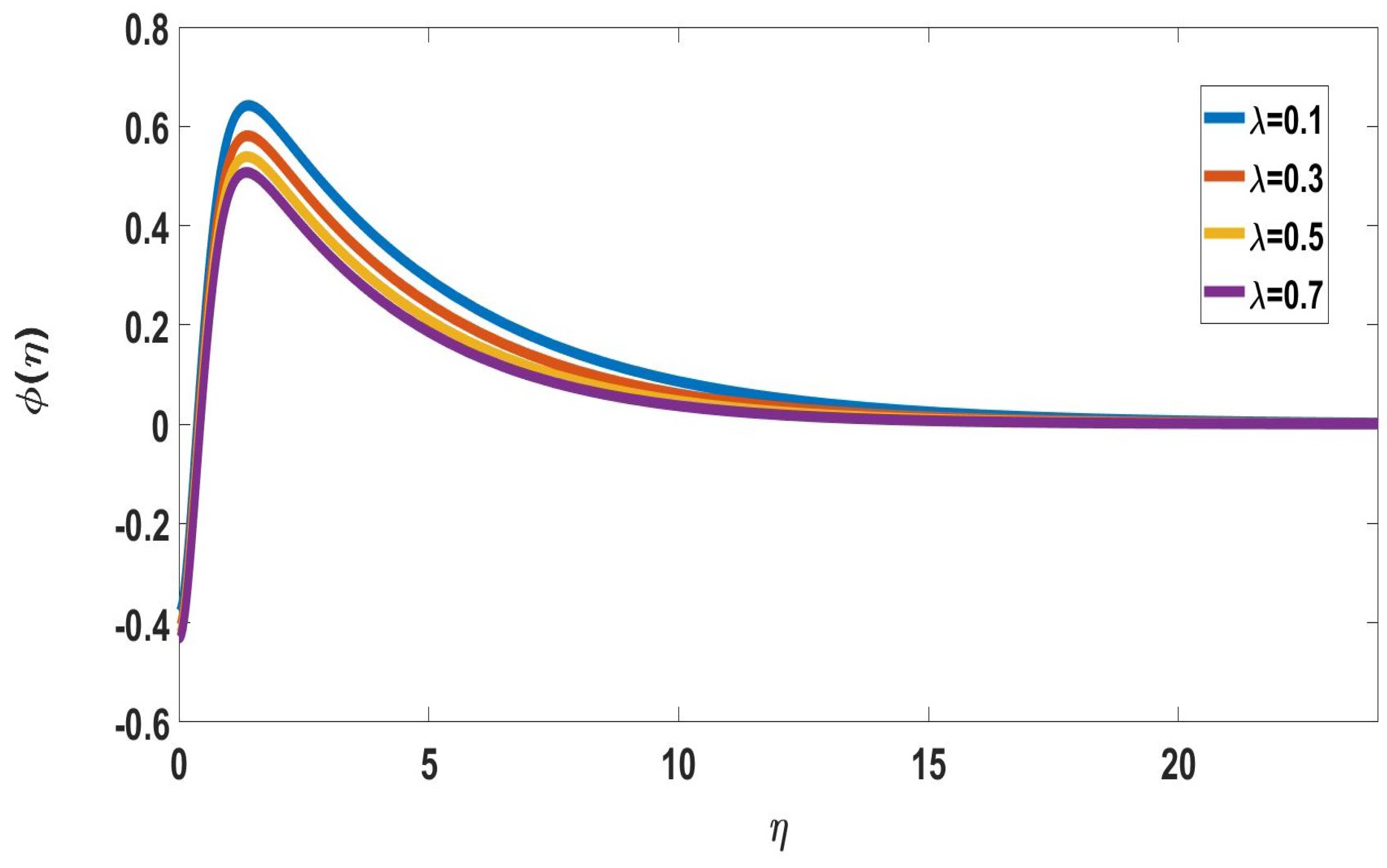
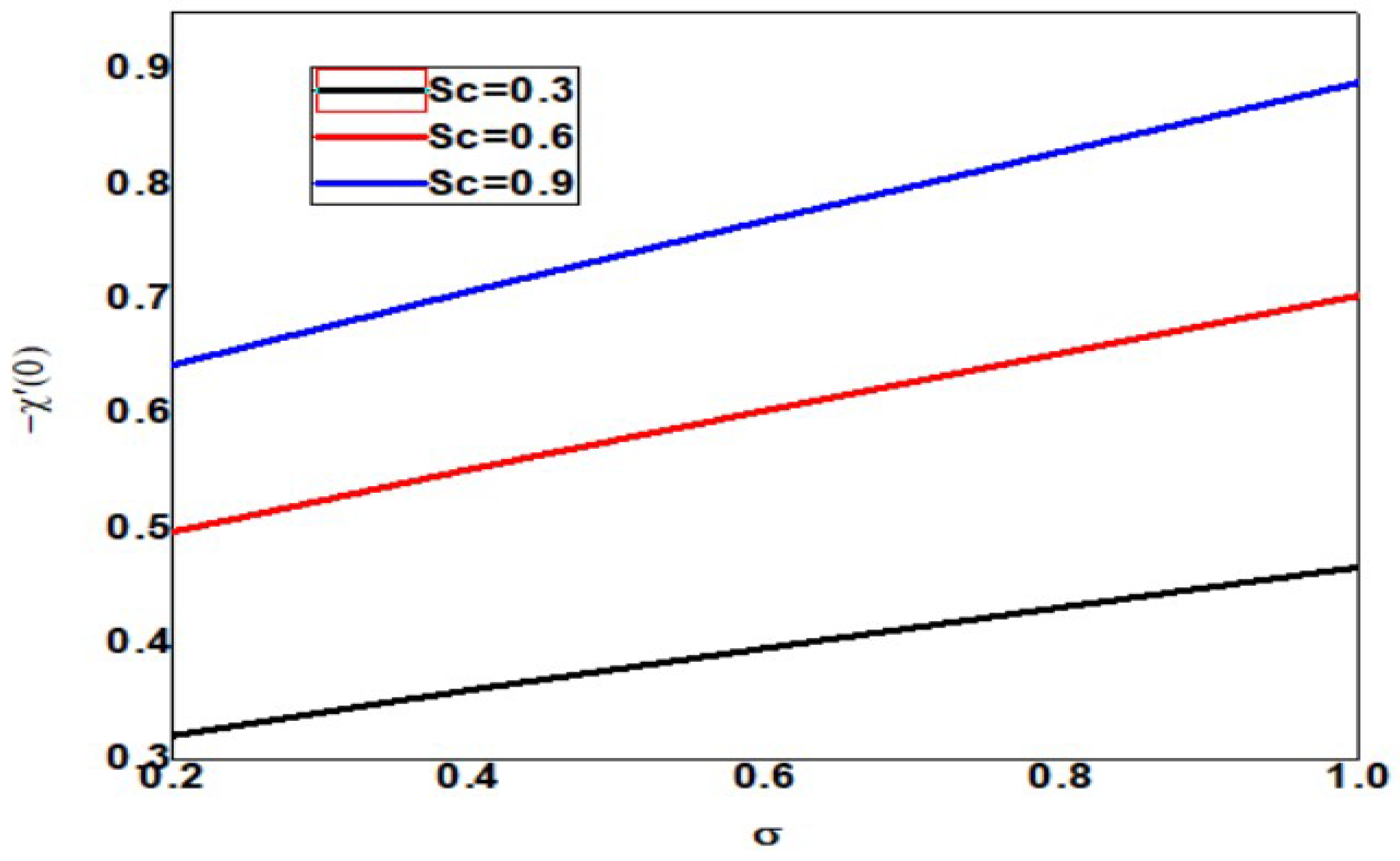
- The magnetic parameter, Eckert number, activation energy, Schmidt number, thermal radiation, and Prandtl number all rise as the solute concentration profile rises.
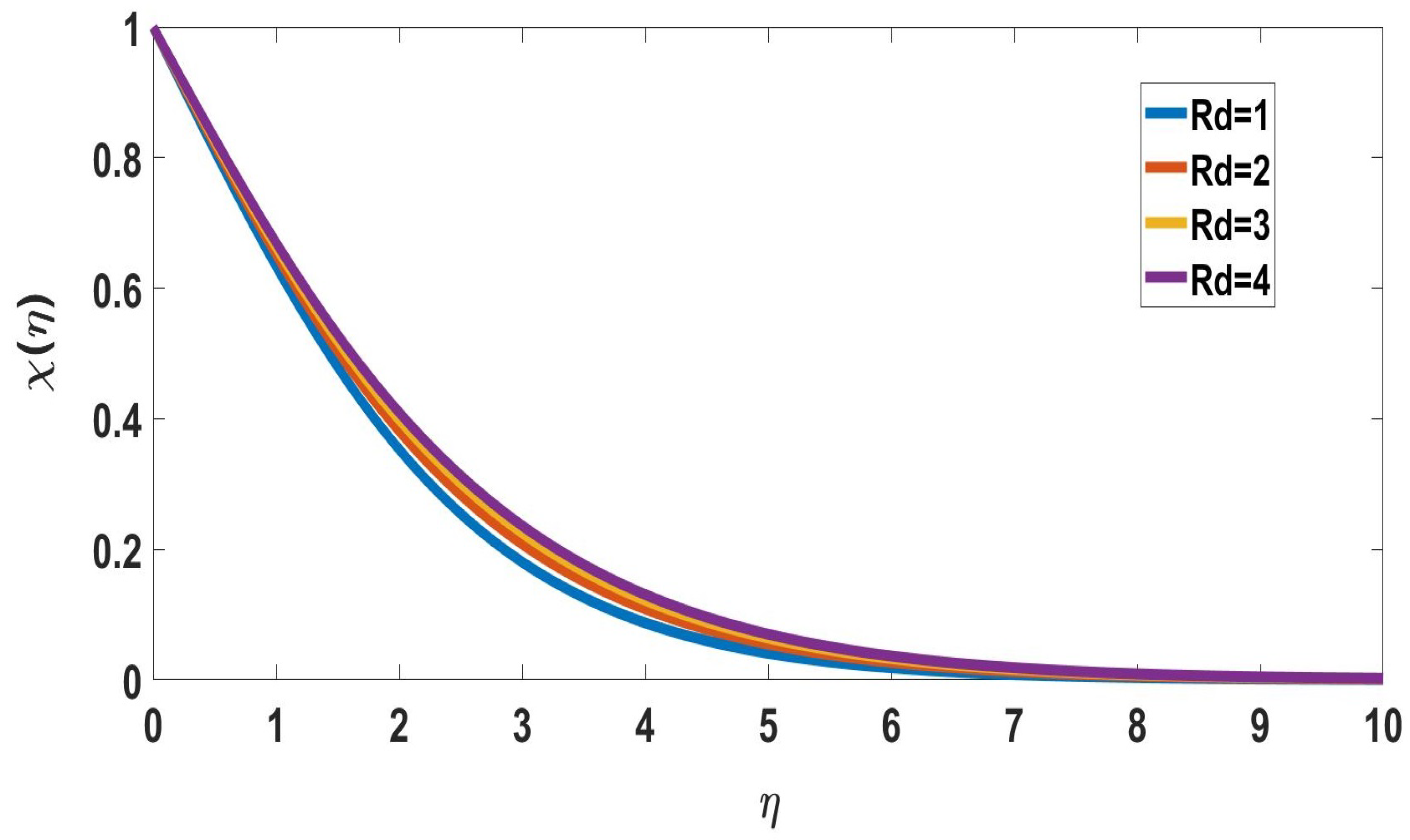
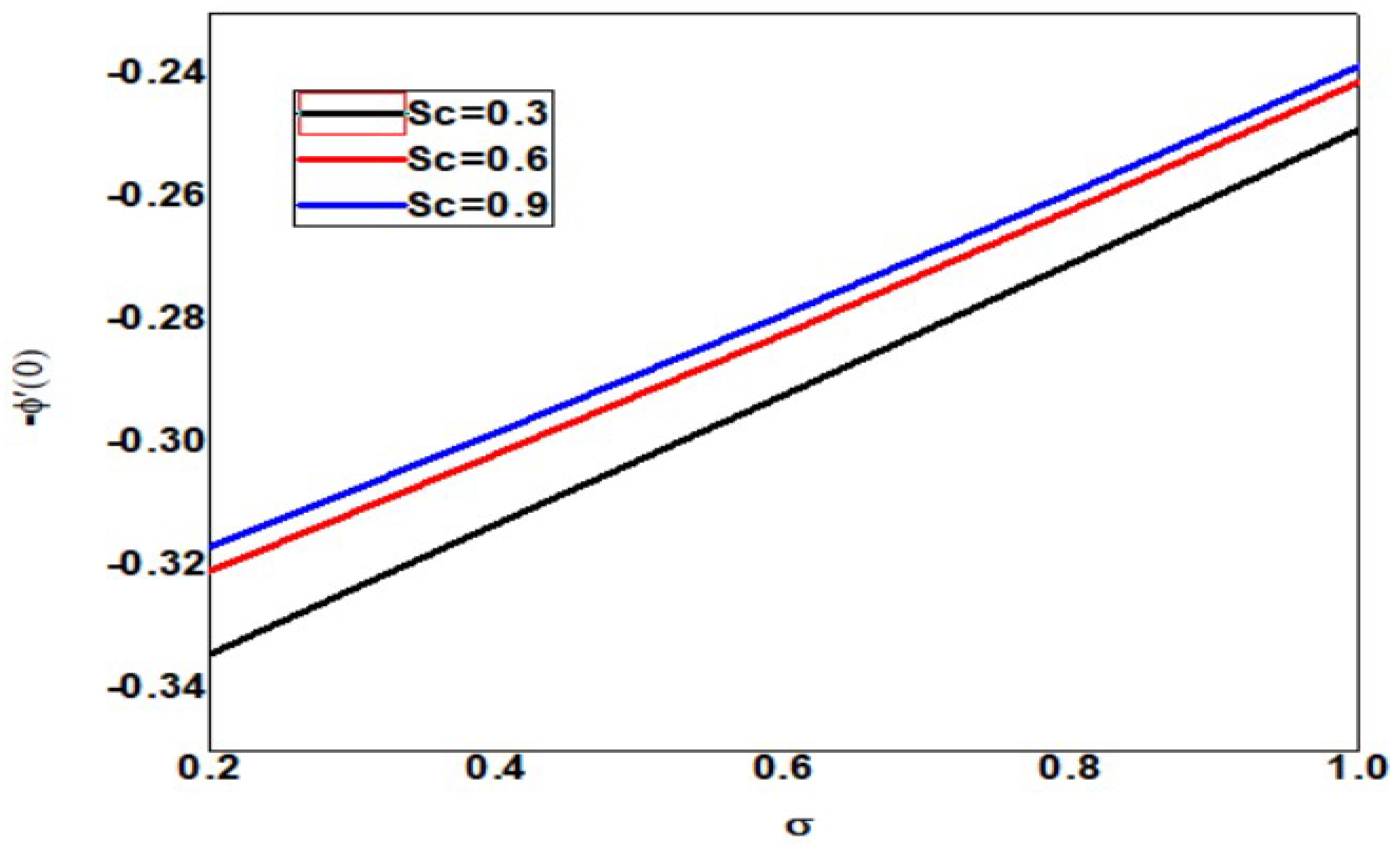
- When the Schmidt number, activation energy, radiation parameter, Prandtl number, and Eckert number increases, the fluid’s nanoparticle concentration decreases.
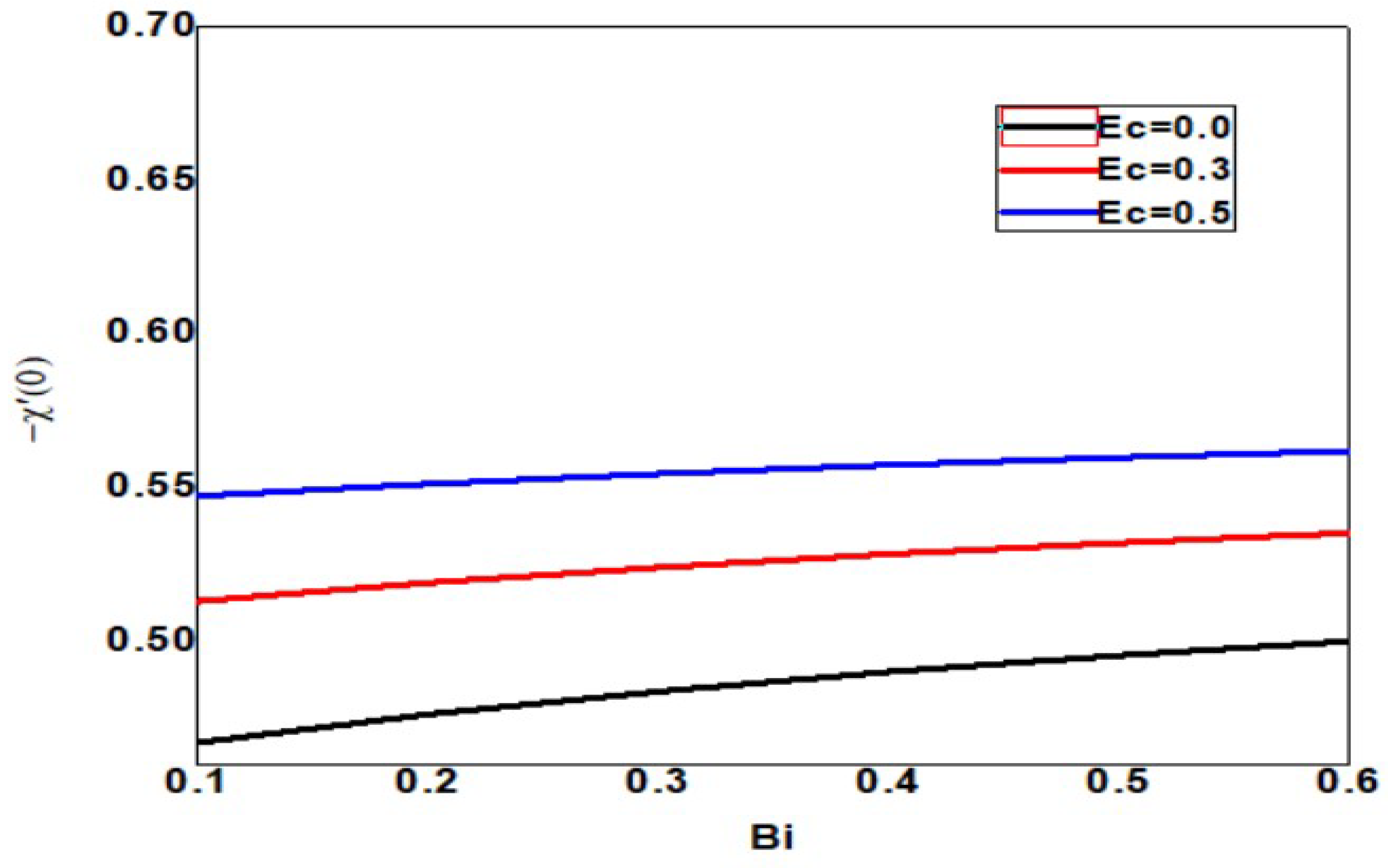
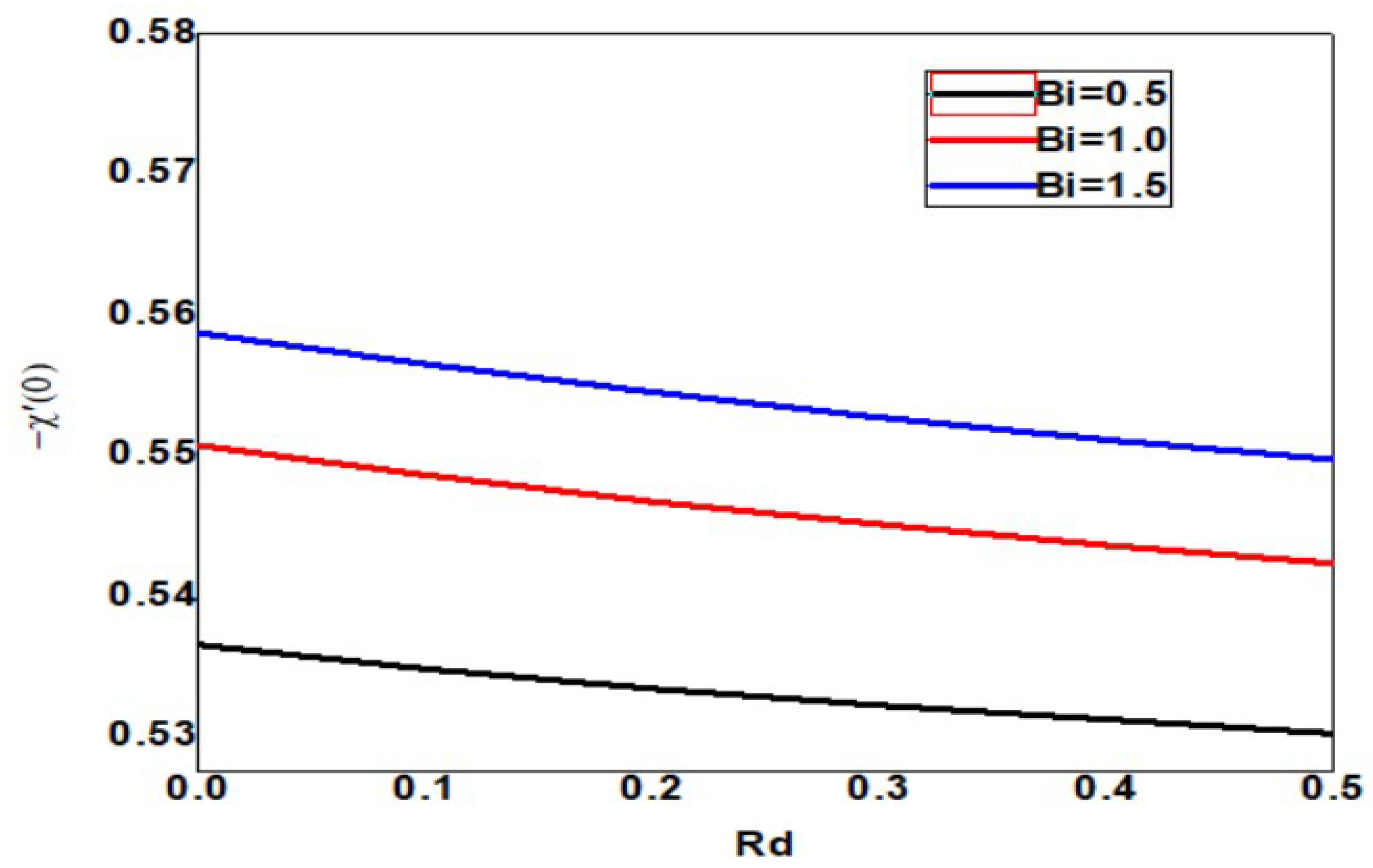
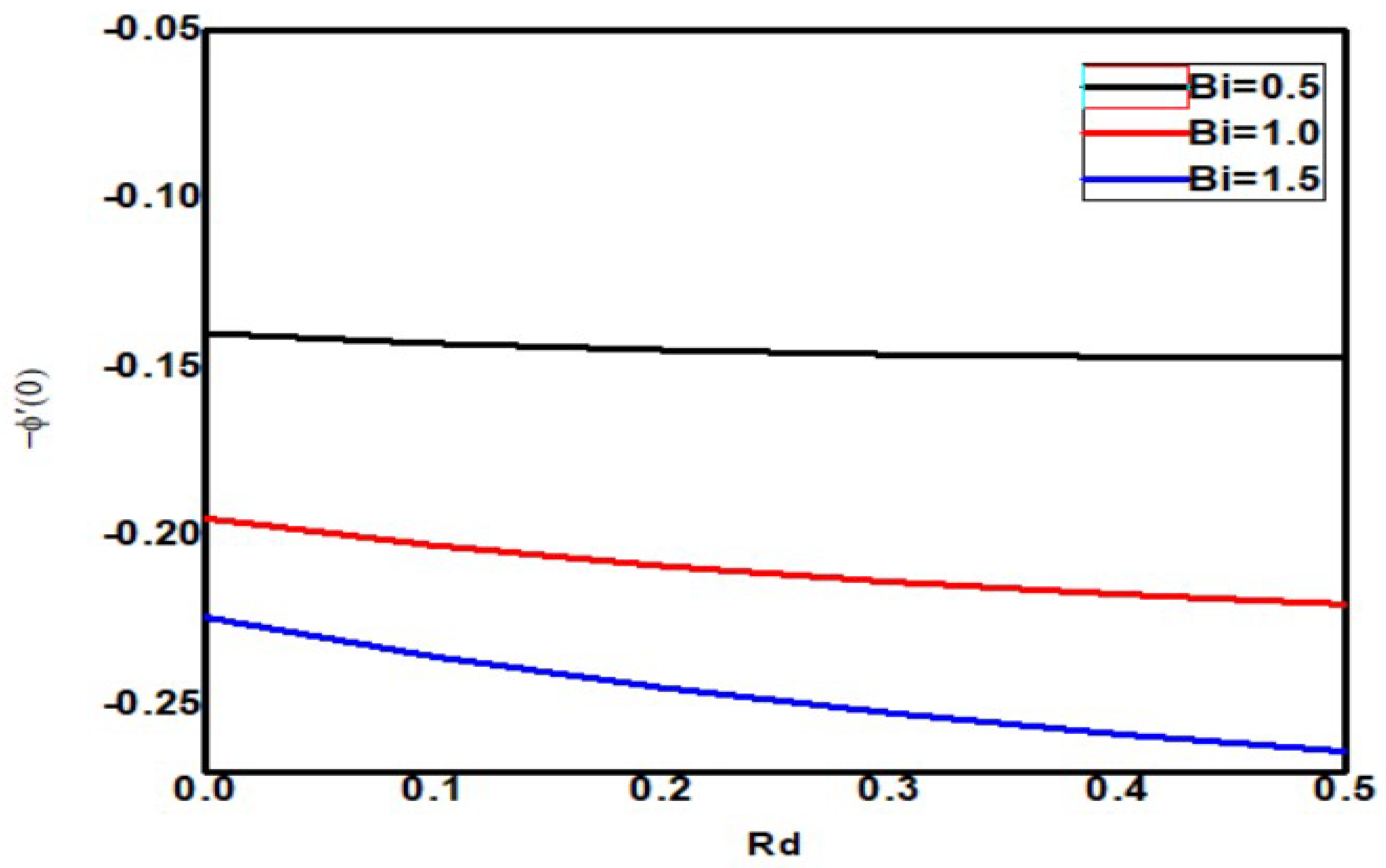
- Skin friction, local Sherwood number increase the Eckert number values as the Biot number increases.
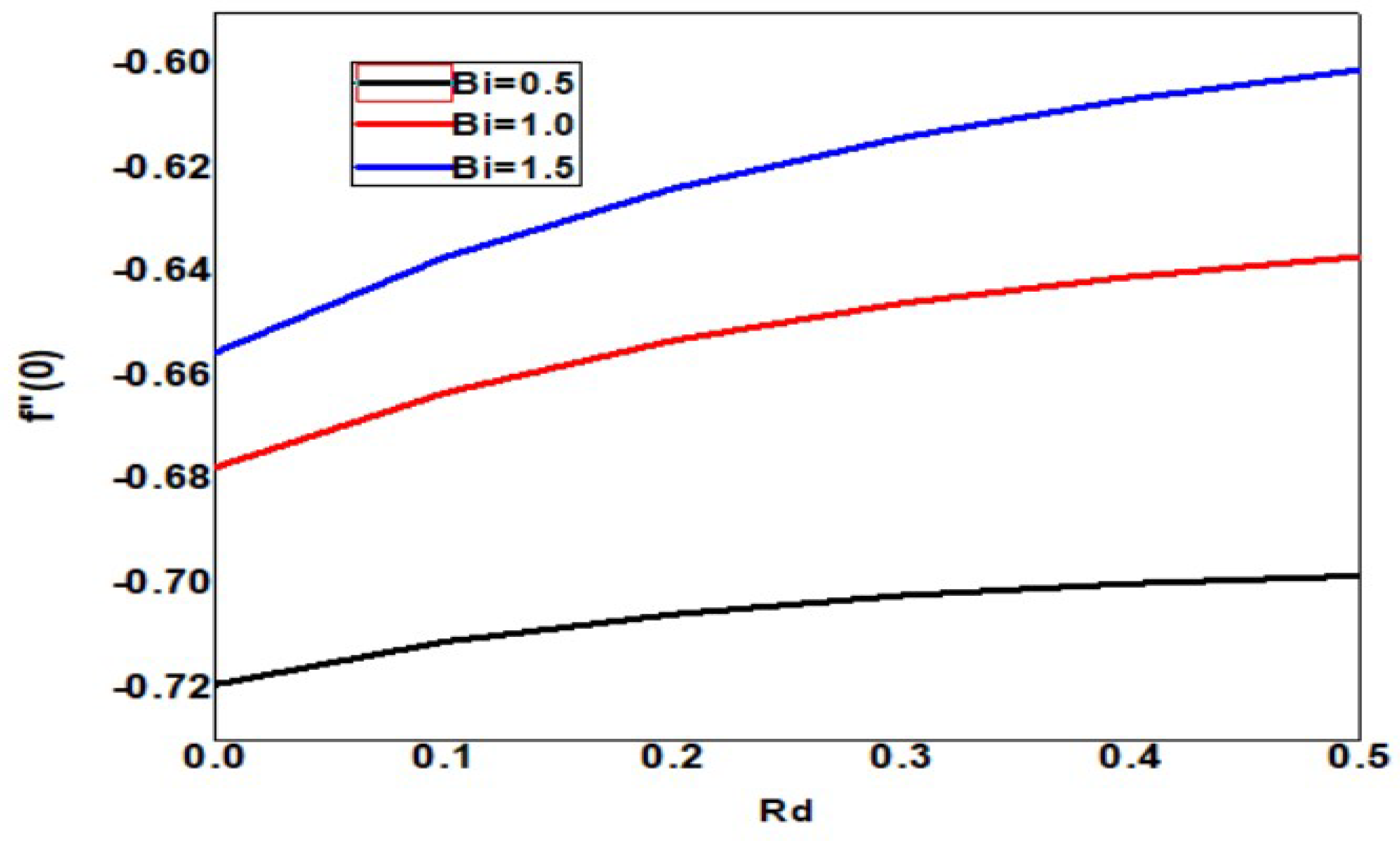
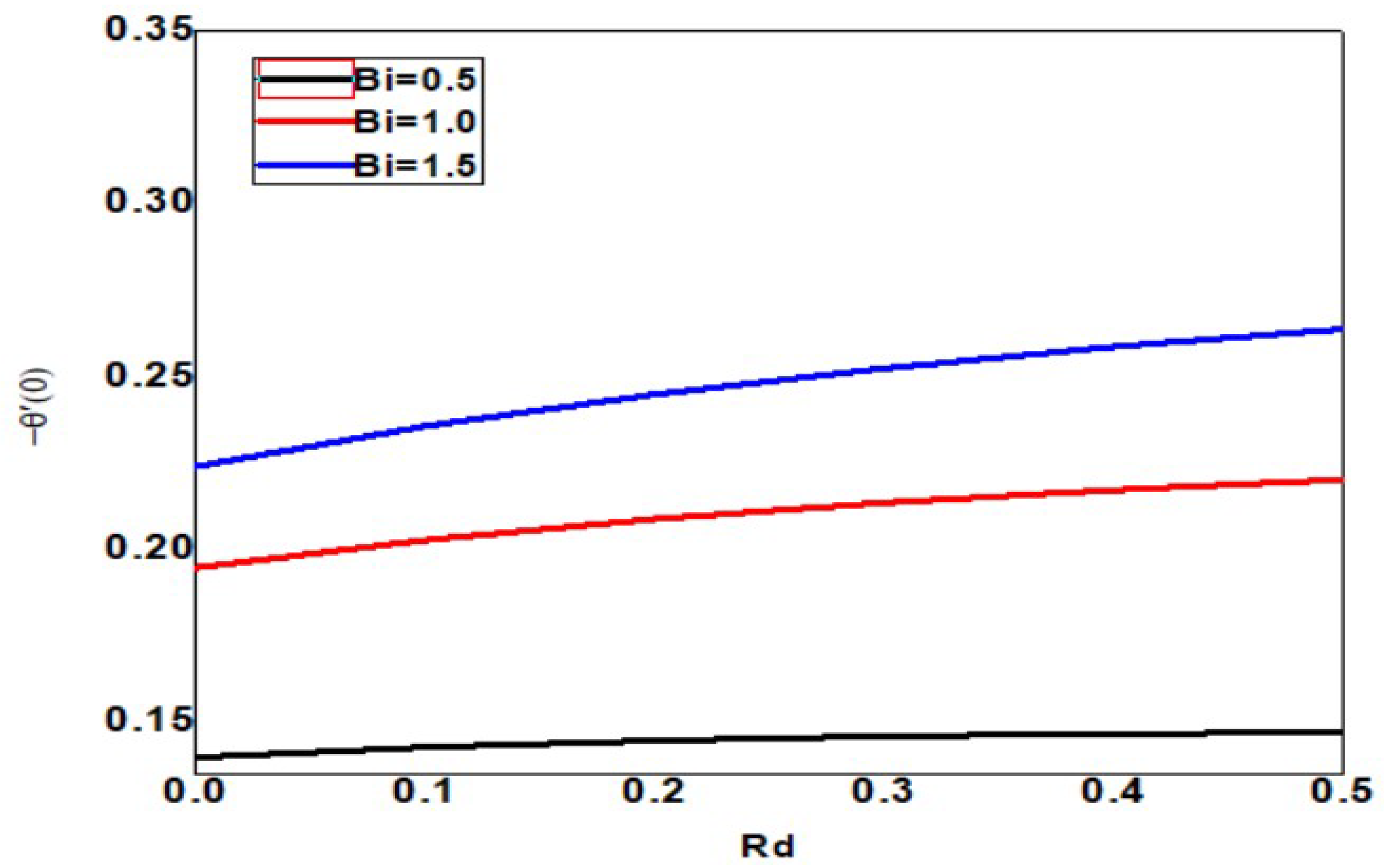
- As Bi and Rd are increased, skin friction and the local Nusselt number increased.
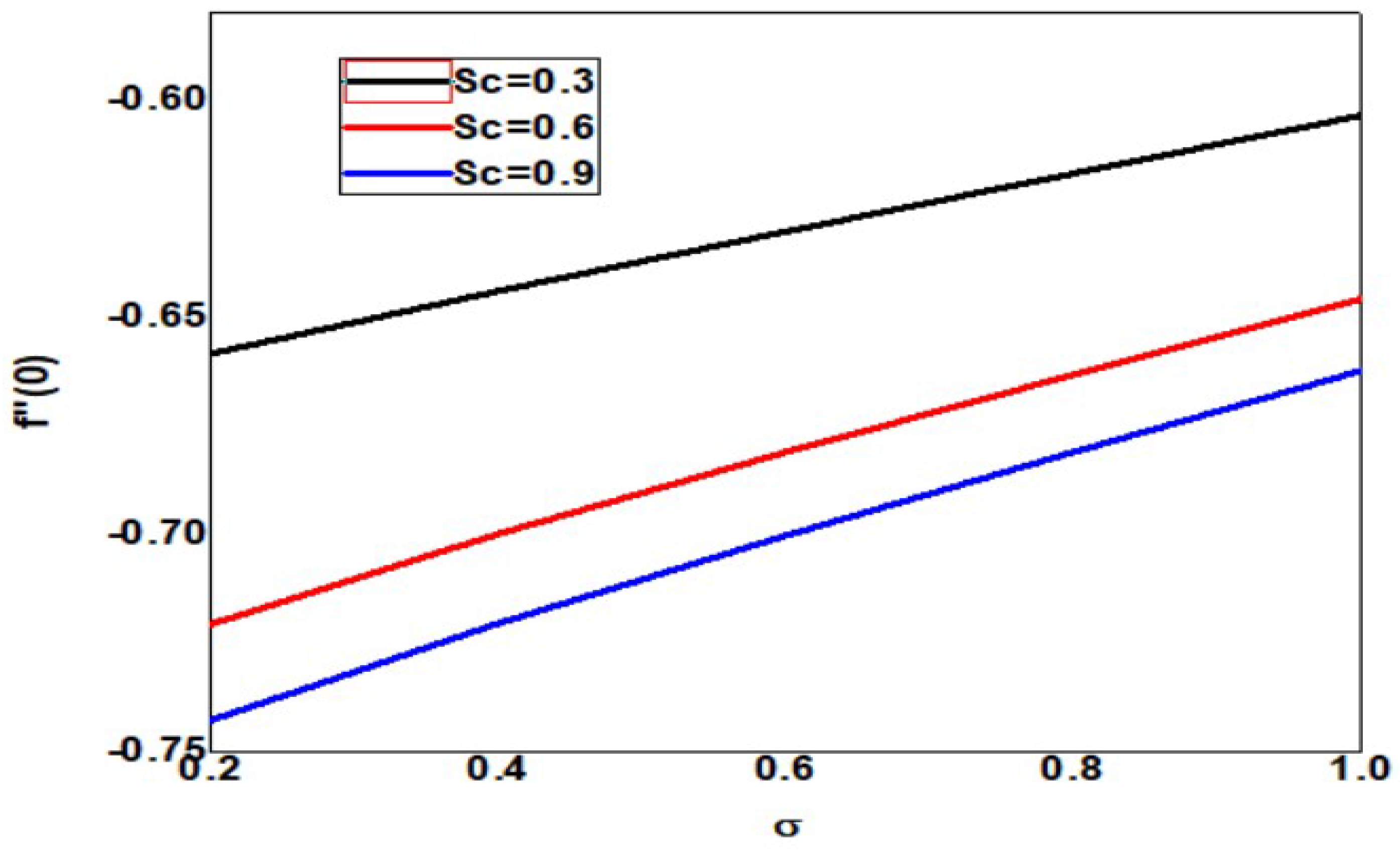
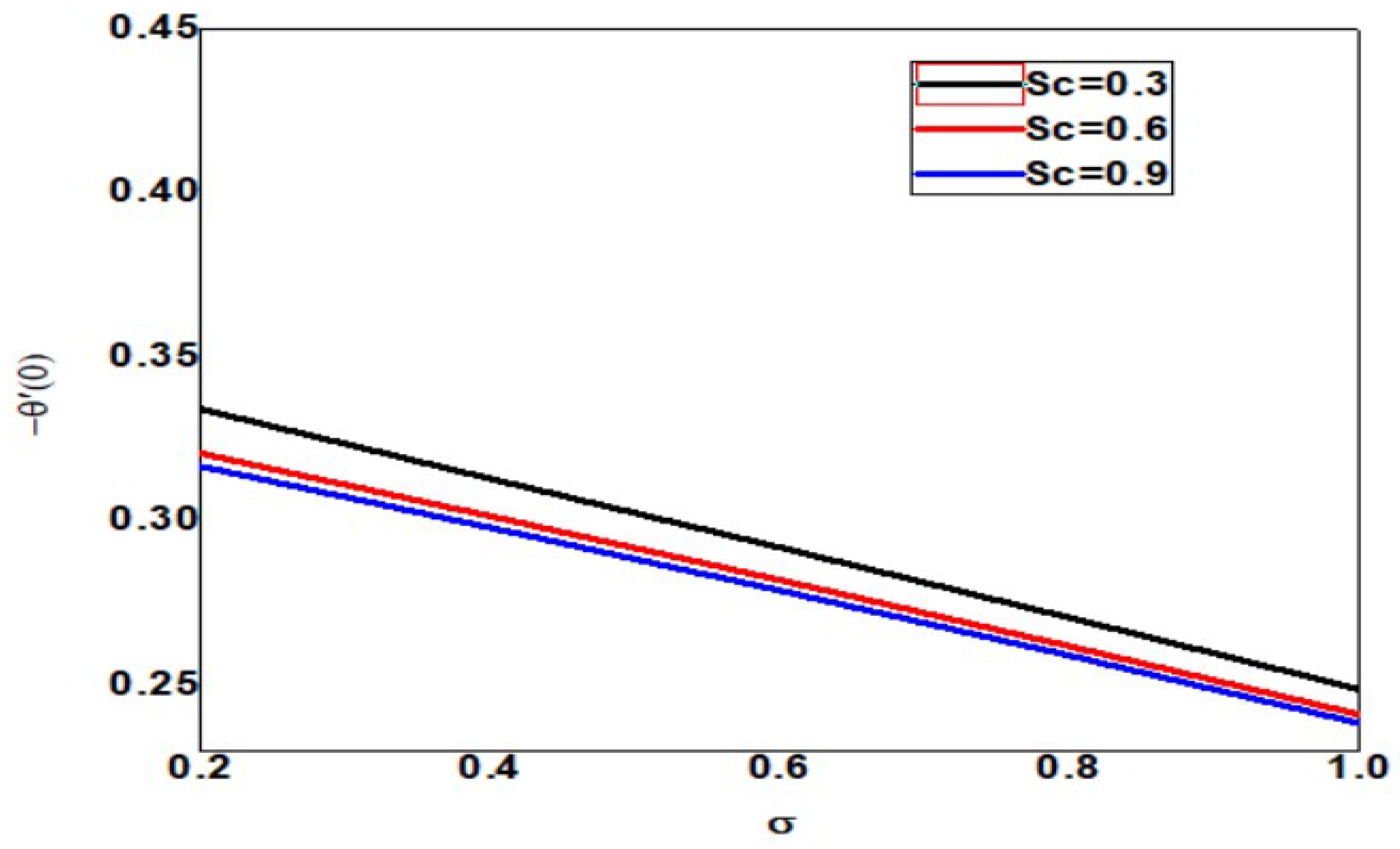
- Skin friction and Nusselt number decrease upon increasing , Sc; nanoparticle Sherwood number increases as Sc increases.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Strength of magnetic field (kgsm) | |
| C | Concentration |
| Local Reynolds number | |
| Schmidt number | |
| Thermal radiation parameter | |
| T | Temperature(K) |
| Radiative heat flux | |
| Brownian diffusion coefficient | |
| Thermophoretic diffusion coefficient (ms) | |
| Prandtl number of base fluid | |
| E | Nondimensional activation energy |
| n | Fitted rate constant |
| Activation energy | |
| Dimensionless velocity | |
| Thermophoresis parameter | |
| Local Grashof number | |
| Buoyancy ratio parameter | |
| g | Gravitational acceleration (ms) |
| K | Boltzmann constant |
| Regular buoyancy parameter | |
| K’ | Mean absorption coefficient |
| k | Thermal conductivity (mkgsK) |
| Reaction rate | |
| M | Magnetic field parameter |
| Brownian diffusion parameter |
Greek Symbols
| Mixed convection parameter | |
| Dimensionless reaction rate | |
| Dimensionless solute concentration | |
| Temperature difference parameter | |
| Dimensionless temperature | |
| Kinematic viscosity (ms) | |
| Effective heat capacity of the nanoparticle material | |
| Heat capacity of the base fluid | |
| Electrical conductivity | |
| T | Ratio of the effective heat capacity of the fluid and the heat capacity of |
| the nanoparticle material | |
| Stefan–Boltzmann constant (WmK) | |
| Thermal diffusivity of the base fluid (ms) | |
| Density of the fluid (kgm) | |
| Coefficient of thermal expansion (K) | |
| Nanoparticle density | |
| Dimensionless nanoparticle concentration |
Subscripts
| w | Condition at a wall |
| ∞ | Condition at free stream |
References
- Jabeen, S.; Hayat, T.; Alsaedi, A.; Alhodaly, M.S. Consequences of activation energy and chemical reaction in radiative flow of tangent hyperbolic nanoliquid. Sci. Iran. 2019, 26, 3928–3937. [Google Scholar] [CrossRef]
- Niranjan, H.; Sivasankaran, S.; Bhuvaneswari, M. Chemical reaction, Soret and Dufour effects on MHD mixed convection stagnation point flow with radiation and slip condition. Sci. Iran. 2017, 24, 698–706. [Google Scholar] [CrossRef]
- Hayat, T.; Ullah, I.; Alsaedi, A.; Asghar, S. Flow of magneto Williamson nanoliquid towards stretching sheet with variable thickness and double stratification. Radiat. Phys. Chem. 2018, 152, 151–157. [Google Scholar] [CrossRef]
- Lakshmi Devi, G.; Niranjan, H. The Novelty of Thermo-Diffusion and Diffusion-Thermo, Slip, Temperature and Concentration Boundary Conditions on Magneto–Chemically Reactive Fluid Flow Past a Vertical Plate with Radiation. Symmetry 2022, 14, 1496. [Google Scholar] [CrossRef]
- Asha, S.K.; Sunitha, G. Thermal radiation and Hall effects on peristaltic blood flow with double diffusion in the presence of nanoparticles. Case Stud. Therm. Eng. 2020, 17, 100560. [Google Scholar] [CrossRef]
- Kumaran, G.; Sandeep, N.; Ali, M.E. Computational analysis of magnetohydrodynamic Casson and Maxwell flows over a stretching sheet with cross diffusion. Results Phys. 2017, 7, 147–155. [Google Scholar] [CrossRef]
- Kodi, R.; Mopuri, O. Unsteady MHD oscillatory Casson fluid flow past an inclined vertical porous plate in the presence of chemical reaction with heat absorption and Soret effects. Heat Transf. 2022, 51, 733–752. [Google Scholar] [CrossRef]
- Swain, B.K.; Parida, B.C.; Kar, S.; Senapati, N. Viscous dissipation and joule heating effect on MHD flow and heat transfer past a stretching sheet embedded in a porous medium. Heliyon 2020, 6, e05338. [Google Scholar] [CrossRef]
- Ramesh, K.; Riaz, A.; Dar, Z.A. Simultaneous effects of MHD and Joule heating on the fundamental flows of a Casson liquid with slip boundaries. Propuls. Power Res. 2021, 10, 118–129. [Google Scholar] [CrossRef]
- Ahmed, K.; Akbar, T. Numerical investigation of magnetohydrodynamics Williamson nanofluid flow over an exponentially stretching surface. Adv. Mech. Eng. 2021, 13, 16878140211019875. [Google Scholar] [CrossRef]
- Abbas, A.; Shafqat, R.; Jeelani, M.B.; Alharthi, N.H. Significance of Chemical Reaction and Lorentz Force on Third-Grade Fluid Flow and Heat Transfer with Darcy–Forchheimer Law over an Inclined Exponentially Stretching Sheet Embedded in a Porous Medium. Symmetry 2022, 14, 779. [Google Scholar] [CrossRef]
- Rasool, G.; Shafiq, A.; Baleanu, D. Consequences of Soret–Dufour effects, thermal radiation, and binary chemical reaction on Darcy Forchheimer flow of nanofluids. Symmetry 2020, 12, 1421. [Google Scholar] [CrossRef]
- Sharma, B.K.; Gandhi, R.; Bhatti, M.M. Entropy analysis of thermally radiating MHD slip flow of hybrid nanoparticles (Au-Al2O3/Blood) through a tapered multi-stenosed artery. Chem. Phys. Lett. 2022, 790, 139348. [Google Scholar] [CrossRef]
- Asjad, M.I.; Zahid, M.; Inc, M.; Baleanu, D.; Almohsen, B. Impact of activation energy and MHD on Williamson fluid flow in the presence of bioconvection. Alex. Eng. J. 2022, 61, 8715–8727. [Google Scholar] [CrossRef]
- Rehman, S.U.; Mariam, A.; Ullah, A.; Asjad, M.I.; Bajuri, M.Y.; Pansera, B.A.; Ahmadian, A. Numerical computation of buoyancy and radiation effects on MHD micropolar nanofluid flow over a stretching/shrinking sheet with heat source. Case Stud. Therm. Eng. 2021, 25, 100867. [Google Scholar] [CrossRef]
- Naseem, T.; Fatima, U.; Munir, M.; Shahzad, A.; Kausar, N.; Nisar, K.S.; Saleel, C.A.; Abbas, M. Joule heating and viscous dissipation effects in hydromagnetized boundary layer flow with variable temperature. Case Stud. Therm. Eng. 2022, 35, 102083. [Google Scholar] [CrossRef]
- Biswas, R.; Hossain, M.S.; Islam, R.; Ahmmed, S.F.; Mishra, S.R.; Afikuzzaman, M. Computational treatment of MHD Maxwell nanofluid flow across a stretching sheet considering higher-order chemical reaction and thermal radiation. J. Comput. Math. Data Sci. 2022, 4, 100048. [Google Scholar] [CrossRef]
- Bejawada, S.G.; Reddy, Y.D.; Jamshed, W.; Nisar, K.S.; Alharbi, A.N.; Chouikh, R. Radiation effect on MHD Casson fluid flow over an inclined non-linear surface with chemical reaction in a Forchheimer porous medium. Alex. Eng. J. 2022, 61, 8207–8220. [Google Scholar] [CrossRef]
- Gopal, D.; Jagadha, S.; Sreehari, P.; Kishan, N.; Mahendar, D. A numerical study of viscous dissipation with first order chemical reaction and ohmic effects on MHD nanofluid flow through an exponential stretching sheet. Mater. Today Proc. 2022, 59, 1028–1033. [Google Scholar] [CrossRef]
- Lakshmi Devi, G.; Niranjan, H.; Sivasankaran, S. Effects of chemical reactions, radiation, and activation energy on MHD buoyancy induced nano fluidflow past a vertical surface. Sci. Iran. 2022, 29, 90–100. [Google Scholar]
- Patel, H.R.; Singh, R. Thermophoresis, Brownian motion and non-linear thermal radiation effects on mixed convection MHD micropolar fluid flow due to nonlinear stretched sheet in porous medium with viscous dissipation, joule heating and convective boundary condition. Int. Commun. Heat Mass Transf. 2019, 107, 68–92. [Google Scholar] [CrossRef]
- Ramana, R.M.; Raju, K.V.; Kumar, J.G. Multiple slips and heat source effects on MHD stagnation point flow of casson fluid over a stretching sheet in the presence of chemical reaction. Mater. Today Proc. 2022, 49, 2306–2315. [Google Scholar] [CrossRef]
- Kotnurkar, A.S.; Talawar, V.T. Impact of electroosmosis and joule heating effects on peristaltic transport with thermal radiation of hyperbolic tangent fluid through a porous media in an endoscope. Partial. Differ. Equations Appl. Math. 2022, 5, 100340. [Google Scholar] [CrossRef]
- Khan, S.A.; Hayat, T.; Alsaedi, A. Irreversibility analysis in hydromagnetic Reiner-Rivlin nanofluid with quartic autocatalytic chemical reactions. Int. Commun. Heat Mass Transf. 2022, 130, 105797. [Google Scholar] [CrossRef]
- Thammanna, G.T.; Gireesha, B.J.; Mahanthesh, B. Partial slip and Joule heating on magnetohydrodynamic radiated flow of nanoliquid with dissipation and convective condition. Results Phys. 2017, 7, 2728–2735. [Google Scholar] [CrossRef]
- Mahanthesh, B.; Gireesha, B.J.; Gorla, R.S. Unsteady three-dimensional MHD flow of a nano Eyring-Powell fluid past a convectively heated stretching sheet in the presence of thermal radiation, viscous dissipation and Joule heating. J. Assoc. Arab. Univ. Basic Appl. Sci. 2017, 23, 75–84. [Google Scholar] [CrossRef]
- Zhang, X.H.; Abidi, A.; Ahmed, A.E.; Khan, M.R.; El-Shorbagy, M.A.; Shutaywi, M.; Issakhov, A.; Galal, A.M. MHD stagnation point flow of nanofluid over a curved stretching/shrinking surface subject to the influence of Joule heating and convective condition. Case Stud. Therm. Eng. 2021, 26, 101184. [Google Scholar] [CrossRef]
- Khan, W.A.; Makinde, O.D.; Khan, Z.H. MHD boundary layer flow of a nanofluid containing gyrotactic microorganisms past a vertical plate with Navier slip. Int. J. Heat Mass Transf. 2014, 74, 285–291. [Google Scholar] [CrossRef]
- Bestman, A.R. Natural convection boundary layer with suction and mass transfer in a porous medium. Int. J. Energy Res. 1990, 14, 389–396. [Google Scholar] [CrossRef]
- RamReddy, C.; Murthy, P.V.; Chamkha, A.J.; Rashad, A.M. Soret effect on mixed convection flow in a nanofluid under convective boundary condition. Int. J. Heat Mass Transf. 2013, 64, 384–392. [Google Scholar] [CrossRef]
- Hussain, A.; Malik, M.Y. MHD nanofluid flow over stretching cylinder with convective boundary conditions and Nield conditions in the presence of gyrotactic swimming microorganism: A biomathematical model. Int. Commun. Heat Mass Transf. 2021, 126, 105425. [Google Scholar] [CrossRef]
- Qayyum, S.; Hayat, T.; Kanwal, M.; Alsaedi, A.; Khan, M.I. Transportation of entropy optimization in radiated chemically dissipative flow of Prandtl–Eyring nanofluid with activation energy. Comput. Methods Programs Biomed. 2020, 184, 105130. [Google Scholar] [CrossRef]
- Kumbhakar, B.; Nandi, S.; Chamkha, A.J. Unsteady hybrid nanofluid flow over a convectively heated cylinder with inclined magnetic field and viscous dissipation: A multiple regression analysis. Chin. J. Phys. 2022, 79, 38–56. [Google Scholar] [CrossRef]
- Parveen, N.; Awais, M.; Awan, S.E.; Shah, S.A.; Yuan, A.; Nawaz, M.; Akhtar, R.; Malik, M.Y. Thermophysical properties of chemotactic microorganisms in bio-convective peristaltic rheology of nano-liquid with slippage, Joule heating and viscous dissipation. Case Stud. Therm. Eng. 2021, 27, 101285. [Google Scholar] [CrossRef]
- Hayat, T.; Imtiaz, M.; Alsaedi, A. Melting heat transfer in the MHD flow of Cu–water nanofluid with viscous dissipation and Joule heating. Adv. Powder Technol. 2016, 27, 1301–1308. [Google Scholar] [CrossRef]
- Mustafa, M.; Khan, J.A.; Hayat, T.; Alsaedi, A. Buoyancy effects on the MHD nanofluid flow past a vertical surface with chemical reaction and activation energy. Int. J. Heat Mass Transf. 2017, 108, 1340–1346. [Google Scholar] [CrossRef]
- Gürdal, M.; Pazarlıoğlu, H.K.; Tekir, M.; Arslan, K.; Gedik, E. Numerical investigation on turbulent flow and heat transfer characteristics of ferro-nanofluid flowing in dimpled tube under magnetic field effect. Appl. Therm. Eng. 2022, 200, 117655. [Google Scholar] [CrossRef]
- Tekir, M.; Gedik, E.; Arslan, K.; Pazarlıoğlu, H.K.; Aksu, B.; Taskesen, E. Hydrothermal behavior of hybrid magnetite nanofluid flowing in a pipe under bi-directional magnetic field with different wave types. Therm. Sci. Eng. Progress. 2022, 34, 101399. [Google Scholar] [CrossRef]
- Akbar, A.A.; Ahammad, N.A.; Awan, A.U.; Hussein, A.K.; Gamaoun, F.; Tag-ElDin, E.M.; Ali, B. Insight into the role of nanoparticles shape factors and diameter on the dynamics of rotating water-based fluid. Nanomaterials 2022, 12, 2801. [Google Scholar] [CrossRef]
- Khan, I.; Saeed, K.; Khan, I. Nanoparticles: Properties, applications and toxicities. Arab. J. Chem. 2019, 12, 908–931. [Google Scholar] [CrossRef]






| Pr | Nt | E | n | Mustafa et al. [36] | Devi et al. [20] | Present Study | ||
|---|---|---|---|---|---|---|---|---|
| 2 | 0.5 | 1 | 1 | 0.5 | 0.5 | 0.706605 | 0.706831 | 0.706708 |
| 4 | 0.935952 | 0.936056 | 0.936052 | |||||
| 7 | 1.132787 | 1.132900 | 1.132892 | |||||
| 10 | 1.257476 | 1.257032 | 1.257374 | |||||
| 5 | 0.1 | 1 | 1 | 0.5 | 0.5 | 1.426267 | 1.426012 | 1.426112 |
| 0.5 | 1.013939 | 1.038594 | 1.038649 | |||||
| 0.7 | 0.846943 | 0.846593 | 0.846547 | |||||
| 1.0 | 0.649940 | 0.649752 | 0.649733 | |||||
| 10 | 0.5 | 1 | 2 | 0.5 | 0.0 | 1.032281 | 1.032085 | 1.032264 |
| 0.5 | 1.056704 | 1.056294 | 1.056327 | |||||
| 3.0 | 1.154539 | 1.154956 | 1.154851 | |||||
| 5.0 | 1.215937 | 1.216012 | 1.216242 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jayanthi, S.; Niranjan, H. Effects of Joule Heating, Viscous Dissipation, and Activation Energy on Nanofluid Flow Induced by MHD on a Vertical Surface. Symmetry 2023, 15, 314. https://doi.org/10.3390/sym15020314
Jayanthi S, Niranjan H. Effects of Joule Heating, Viscous Dissipation, and Activation Energy on Nanofluid Flow Induced by MHD on a Vertical Surface. Symmetry. 2023; 15(2):314. https://doi.org/10.3390/sym15020314
Chicago/Turabian StyleJayanthi, Senthil, and Hari Niranjan. 2023. "Effects of Joule Heating, Viscous Dissipation, and Activation Energy on Nanofluid Flow Induced by MHD on a Vertical Surface" Symmetry 15, no. 2: 314. https://doi.org/10.3390/sym15020314
APA StyleJayanthi, S., & Niranjan, H. (2023). Effects of Joule Heating, Viscous Dissipation, and Activation Energy on Nanofluid Flow Induced by MHD on a Vertical Surface. Symmetry, 15(2), 314. https://doi.org/10.3390/sym15020314







