A Scalar Product for Computing Fundamental Quantities in Matter
Abstract
:1. Introduction and Summary
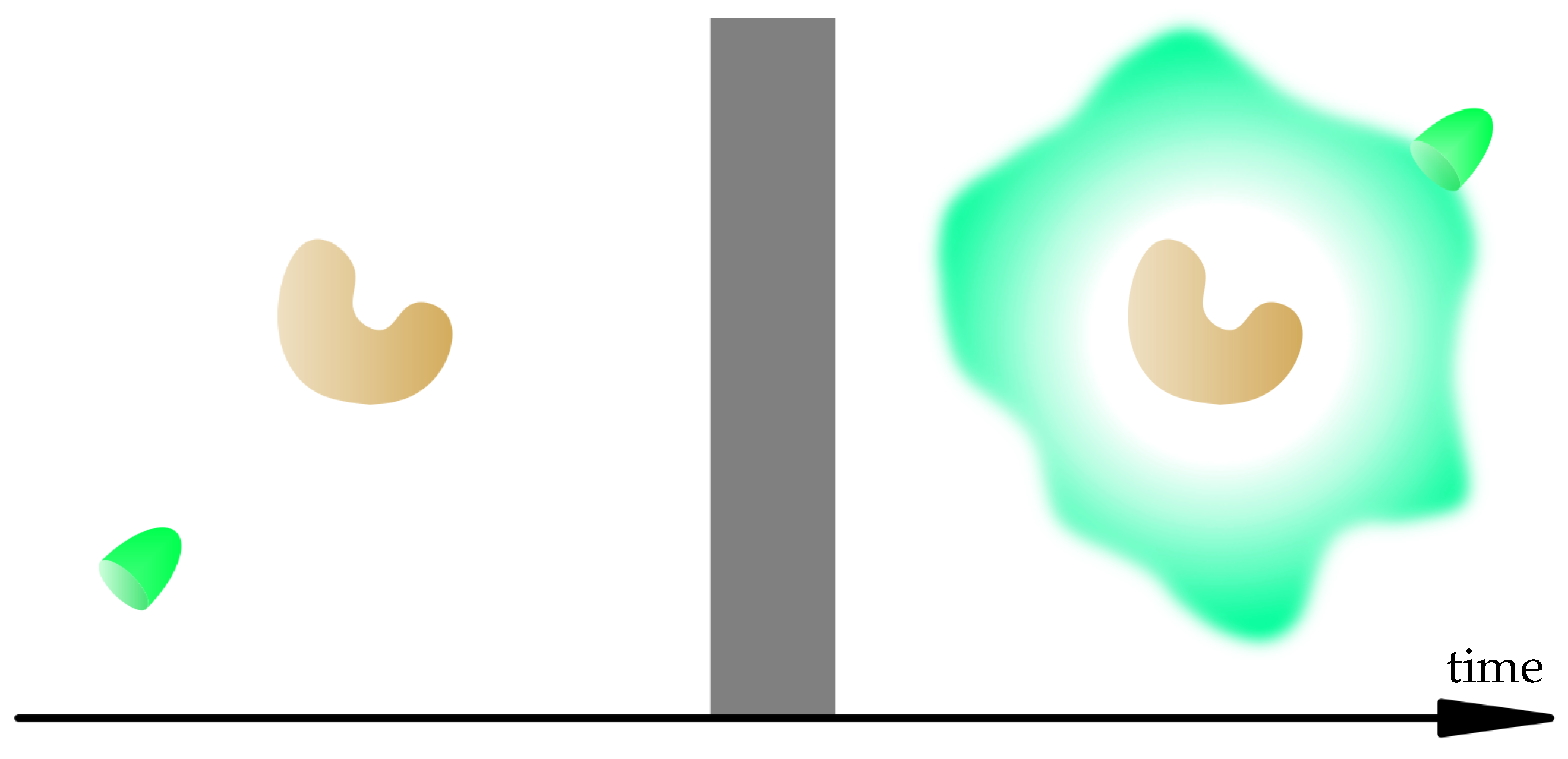
2. Motivation and Problem Setting
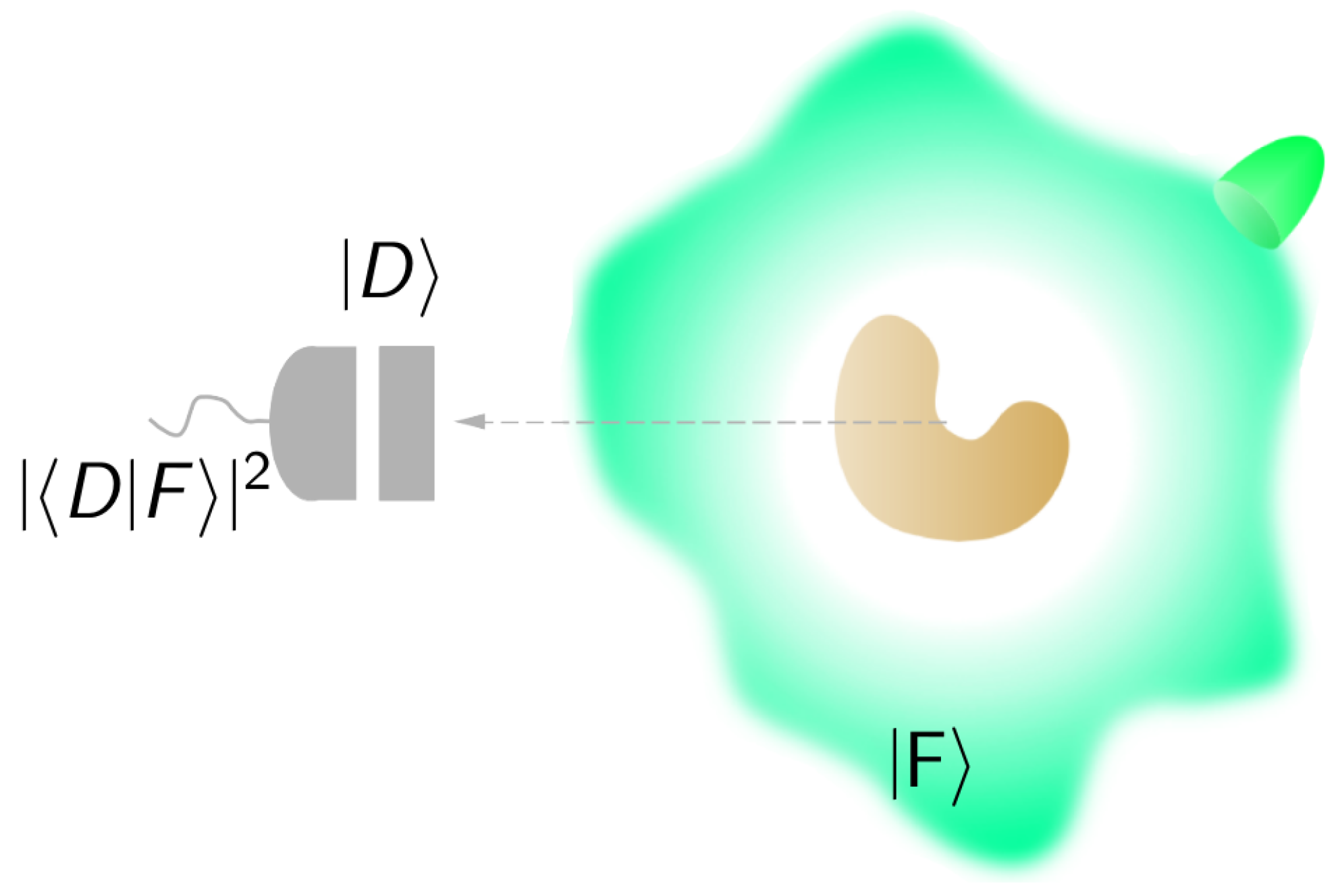
3. The Importance of Invariant Scalar Products in the Consistent Definition of Measurements
4. Conformally Invariant Scalar Product for Static Matter
4.1. Representation of Matter
4.2. Group of Transformations and Invariant Scalar Product for the Static Case
5. Helicity and Angular Momentum in Magnetization
5.1. Discrepancies with Existing Results
5.2. Helicity and Angular Momentum of a Hopfion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. The Inversion R Is Unitary
Appendix B. The Scalar Product in the Angular Momentum Basis
Appendix C. The Linear and Angular Momenta Vanish
Appendix C.1. Angular Momentum
Appendix C.2. Linear Momentum
Appendix D. Angular Momentum Content of the Hopfion
References
- Wigner, E.P. Group Theory and Its Application to the Quantum Mechanics of Atomic Spectra; Academic Press: New York, NY, USA, 1959. [Google Scholar]
- Wigner, E. On Unitary Representations of the Inhomogeneous Lorentz Group. Ann. Math. 1939, 40, 149–204. [Google Scholar] [CrossRef]
- Gross, L. Norm Invariance of Mass-Zero Equations under the Conformal Group. J. Math. Phys. 1964, 5, 687–695. [Google Scholar] [CrossRef]
- Moses, H.E. Photon Wave Functions and the Exact Electromagnetic Matrix Elements for Hydrogenic Atoms. Phys. Rev. A 1973, 8, 1710–1721. [Google Scholar] [CrossRef]
- Bialynicki-Birula, I.; Bialynicka-Birula, Z. Quantum Electrodynamics; Pergamon: Oxford, UK, 1975. [Google Scholar]
- Bialynicki-Birula, I. Photon Wave Function. Prog. Opt. 1996, 36, 245–294. [Google Scholar]
- Fernandez-Corbaton, I.; Rockstuhl, C. Unified theory to describe and engineer conservation laws in light-matter interactions. Phys. Rev. A 2017, 95, 053829. [Google Scholar] [CrossRef]
- Bateman, H. The Transformation of the Electrodynamical Equations. Proc. Lond. Math. Soc. 1910, s2-8, 223–264. [Google Scholar] [CrossRef]
- Cunningham, E. The Principle of Relativity in Electrodynamics and an Extension Thereof. Proc. Lond. Math. Soc. 1910, s2-8, 77–98. [Google Scholar] [CrossRef]
- Dirac, P.A.M. Wave Equations in Conformal Space. Ann. Math. 1936, 37, 429–442. [Google Scholar] [CrossRef]
- Fernandez-Corbaton, I. A Conformally Invariant Derivation of Average Electromagnetic Helicity. Symmetry 2019, 11, 1427. [Google Scholar] [CrossRef]
- Grafarend, E.W.; Krumm, F.W. Map Projections; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Sutcliffe, P. Hopfions in chiral magnets. J. Phys. A Math. Theor. 2018, 51, 375401. [Google Scholar] [CrossRef]
- Liu, Y.; Lake, R.K.; Zang, J. Binding a hopfion in a chiral magnet nanodisk. Phys. Rev. B 2018, 98, 174437. [Google Scholar] [CrossRef]
- Tai, J.S.B.; Smalyukh, I.I. Static Hopf Solitons and Knotted Emergent Fields in Solid-State Noncentrosymmetric Magnetic Nanostructures. Phys. Rev. Lett. 2018, 121, 187201. [Google Scholar] [CrossRef] [PubMed]
- Kent, N.; Reynolds, N.; Raftrey, D.; Campbell, I.T.G.; Virasawmy, S.; Dhuey, S.; Chopdekar, R.V.; Hierro-Rodriguez, A.; Sorrentino, A.; Pereiro, E.; et al. Creation and observation of Hopfions in magnetic multilayer systems. Nat. Commun. 2021, 12, 1562. [Google Scholar] [CrossRef] [PubMed]
- Khodzhaev, Z.; Turgut, E. Hopfion dynamics in chiral magnets. J. Phys. Condens. Matter 2022, 34, 225805. [Google Scholar] [CrossRef]
- Verba, R.V.; Navas, D.; Bunyaev, S.A.; Hierro-Rodriguez, A.; Guslienko, K.Y.; Ivanov, B.A.; Kakazei, G.N. Helicity of magnetic vortices and skyrmions in soft ferromagnetic nanodots and films biased by stray radial fields. Phys. Rev. B 2020, 101, 064429. [Google Scholar] [CrossRef]
- Peralta, J.E.; Scuseria, G.E.; Frisch, M.J. Noncollinear magnetism in density functional calculations. Phys. Rev. B 2007, 75, 125119. [Google Scholar] [CrossRef]
- Coppens, P. Charge Densities Come of Age. Angew. Chem. Int. Ed. 2005, 44, 6810–6811. [Google Scholar] [CrossRef] [PubMed]
- Donnelly, C.; Guizar-Sicairos, M.; Scagnoli, V.; Gliga, S.; Holler, M.; Raabe, J.; Heyderman, L.J. Three-dimensional magnetization structures revealed with X-ray vector nanotomography. Nature 2017, 547, 328–331. [Google Scholar] [CrossRef]
- Stanciu, C.D.; Hansteen, F.; Kimel, A.V.; Kirilyuk, A.; Tsukamoto, A.; Itoh, A.; Rasing, T. All-Optical Magnetic Recording with Circularly Polarized Light. Phys. Rev. Lett. 2007, 99, 047601. [Google Scholar] [CrossRef]
- Schwinger, J.; Deraad, L.L.J.; Milton, K.A. Classical Electrodynamics; Westview Press: Boulder, CO, USA, 1998. [Google Scholar]
- Jackson, J.D. Classical Electrodynamics; Wiley: New York, NY, USA, 1998. [Google Scholar]
- Bialynicki-Birula, I.; Newman, E.T.; Porter, J.; Winicour, J.; Lukacs, B.; Perjes, Z.; Sebestyen, A. A note on helicity. J. Math. Phys. 1981, 22, 2530–2532. [Google Scholar] [CrossRef]
- Budinich, P.; Raczka, R. Global properties of conformally flat momentum space and their implications. Found. Phys. 1993, 23, 599–615. [Google Scholar] [CrossRef]
- Fuschchich, W.; Nikitin, A. Symmetries of Equations of Quantum Mechanics; Allerton Press: New York, NY, USA, 1994. [Google Scholar]
- Kastrup, H.A. Zur physikalischen Deutung und darstellungstheoretischen Analyse der konformen Transformationen von Raum und Zeit. Ann. Der Phys. 1962, 464, 388–428. [Google Scholar] [CrossRef]
- Barut, A.; Haugen, R.B. Theory of the conformally invariant mass. Ann. Phys. 1972, 71, 519–541. [Google Scholar] [CrossRef]
- Kastrup, H. On the advancements of conformal transformations and their associated symmetries in geometry and theoretical physics. Ann. Der Phys. 2008, 520, 631–690. [Google Scholar] [CrossRef]
- Sorkin, R.D. Quantum mechanics as a quantum measure theory. Mod. Phys. Lett. A 1994, 9, 3119–3127. [Google Scholar] [CrossRef]
- Hardy, L. Quantum theory from five reasonable axioms. arXiv 2001, arXiv:quant-ph/0101012. [Google Scholar]
- Rajantie, A. The search for magnetic monopoles. Phys. Today 2016, 69, 40–46. [Google Scholar] [CrossRef]
- Fortson, N.; Sandars, P.; Barr, S. The Search for a Permanent Electric Dipole Moment. Phys. Today 2003, 56, 33–39. [Google Scholar] [CrossRef]
- Barut, A.O. Electrodynamics and Classical Theory of Fields & Particles; Dover: New York, NY, USA, 1980. [Google Scholar]
- Frenkel, J. Die Elektrodynamik des rotierenden Elektrons. Z. Für Phys. 1926, 37, 243–262. [Google Scholar] [CrossRef]
- Bargmann, V.; Michel, L.; Telegdi, V.L. Precession of the Polarization of Particles Moving in a Homogeneous Electromagnetic Field. Phys. Rev. Lett. 1959, 2, 435–436. [Google Scholar] [CrossRef]
- Fernandez-Corbaton, I. Total helicity of electromagnetic fields and matter. Phys. Rev. B 2021, 103, 054406. [Google Scholar] [CrossRef]
- Brown, W.F. Magnetostatic Principles in Ferromagnetism; North-Holland Publishing Company: Amsterdam, The Netherlands, 1962; Volume 1. [Google Scholar]
- Moses, H.E.; Quesada, A.F. The expansion of physical quantities in terms of the irreducible representations of the scale-euclidean group and applications to the construction of scale-invariant correlation functions. Arch. Ration. Mech. Anal. 1973, 50, 194–221. [Google Scholar] [CrossRef]
- Kastrup, H.A.; Mayer, D.H. On Some Classes of Solutions of the Wave Equation. J. Math. Phys. 1970, 11, 1041–1047. [Google Scholar] [CrossRef]
- Moses, H.E. Transformation from a Linear Momentum to an Angular Momentum Basis for Particles of Zero Mass and Finite Spin. J. Math. Phys. 1965, 6, 928–940. [Google Scholar] [CrossRef]
- Moses, H.E. The role of the irreducible representations of the Poincaré group in solving Maxwell’s equations. J. Math. Phys. 2004, 45, 1887–1918. [Google Scholar] [CrossRef]
- Woltjer, L. A theorem on force-free magnetic fields. Proc. Natl. Acad. Sci. USA 1958, 44, 489–491. [Google Scholar] [CrossRef]
- Moffatt, H.K. The degree of knottedness of tangled vortex lines. J. Fluid Mech. 1969, 35, 117–129. [Google Scholar] [CrossRef]
- Ranada, A.F. On the magnetic helicity. Eur. J. Phys. 1992, 13, 70–76. [Google Scholar] [CrossRef]
- Varshalovich, D.A.; Moskalev, A.N.; Khersonskii, V.K. Quantum Theory of Angular Momentum; World Scientific: Singapore, 1988. [Google Scholar]
- Stoll, S.; Schweiger, A. EasySpin, a comprehensive software package for spectral simulation and analysis in EPR. J. Magn. Reson. 2006, 178, 42–55. [Google Scholar] [CrossRef]
- Lomont, J.S.; Moses, H.E. The Representations of the Inhomogeneous Lorentz Group in Terms of an Angular Momentum Basis. J. Math. Phys. 1964, 5, 294–298. [Google Scholar] [CrossRef]
- Dzyloshinskii, I.; Ivanov, B. Localized topological solitons in a ferromagnet. Sov. J. Exp. Theor. Phys. Lett. 1979, 29, 540. [Google Scholar]
- Volovik, G.E. Linear momentum in ferromagnets. J. Phys. C Solid State Phys. 1987, 20, L83–L87. [Google Scholar] [CrossRef]
- Papanicolaou, N.; Tomaras, T. Dynamics of magnetic vortices. Nucl. Phys. B 1991, 360, 425–462. [Google Scholar] [CrossRef]
- Papanicolaou, N. Dynamics of magnetic vortex rings. In Singularities in Fluids, Plasmas and Optics; Springer: Berlin/Heidelberg, Germany, 1993; pp. 151–158. [Google Scholar]
- Cooper, N.R. Propagating Magnetic Vortex Rings in Ferromagnets. Phys. Rev. Lett. 1999, 82, 1554–1557. [Google Scholar] [CrossRef]
- Kosevich, A.; Ivanov, B.; Kovalev, A. Magnetic Solitons. Phys. Rep. 1990, 194, 117–238. [Google Scholar] [CrossRef]
- Borisov, A.B.; Rybakov, F.N. Stationary precession topological solitons with nonzero Hopf invariant in a uniaxial ferromagnet. JETP Lett. 2008, 88, 264–267. [Google Scholar] [CrossRef]
- Borisov, A.B.; Rybakov, F.N. Dynamical toroidal Hopfions in a ferromagnet with easy-axis anisotropy. JETP Lett. 2009, 90, 544–547. [Google Scholar] [CrossRef]
- Yan, P.; Kamra, A.; Cao, Y.; Bauer, G.E.W. Angular and linear momentum of excited ferromagnets. Phys. Rev. B 2013, 88, 144413. [Google Scholar] [CrossRef]
- Tchernyshyov, O. Conserved momenta of a ferromagnetic soliton. Ann. Phys. 2015, 363, 98–113. [Google Scholar] [CrossRef]
- Tung, W.K. Group Theory in Physics; World Scientific: Singapore, 1985. [Google Scholar]
- Rose, M.E. Elementary Theory of Angular Momentum; Wiley: New York, NY, USA, 1957. [Google Scholar]
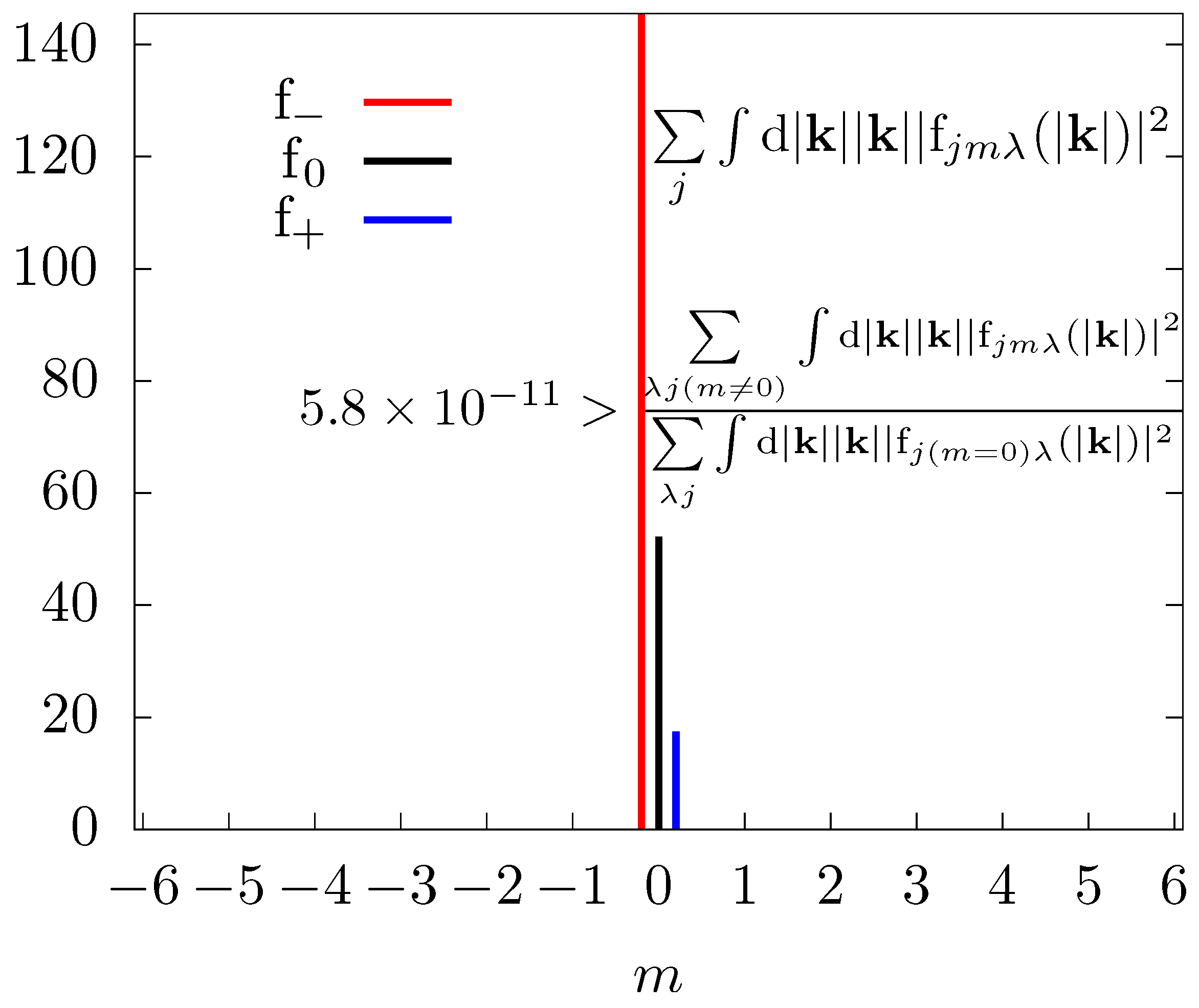
| −129.1 ℏ | 1.30 × |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fernandez-Corbaton, I.; Vavilin, M. A Scalar Product for Computing Fundamental Quantities in Matter. Symmetry 2023, 15, 1839. https://doi.org/10.3390/sym15101839
Fernandez-Corbaton I, Vavilin M. A Scalar Product for Computing Fundamental Quantities in Matter. Symmetry. 2023; 15(10):1839. https://doi.org/10.3390/sym15101839
Chicago/Turabian StyleFernandez-Corbaton, Ivan, and Maxim Vavilin. 2023. "A Scalar Product for Computing Fundamental Quantities in Matter" Symmetry 15, no. 10: 1839. https://doi.org/10.3390/sym15101839
APA StyleFernandez-Corbaton, I., & Vavilin, M. (2023). A Scalar Product for Computing Fundamental Quantities in Matter. Symmetry, 15(10), 1839. https://doi.org/10.3390/sym15101839








