Analytical Results for Classical Axially Symmetric States of Relativistic Hydrogenic Ions in Collinear Electric and Magnetic Fields of Arbitrary Strengths
Abstract
:1. Introduction
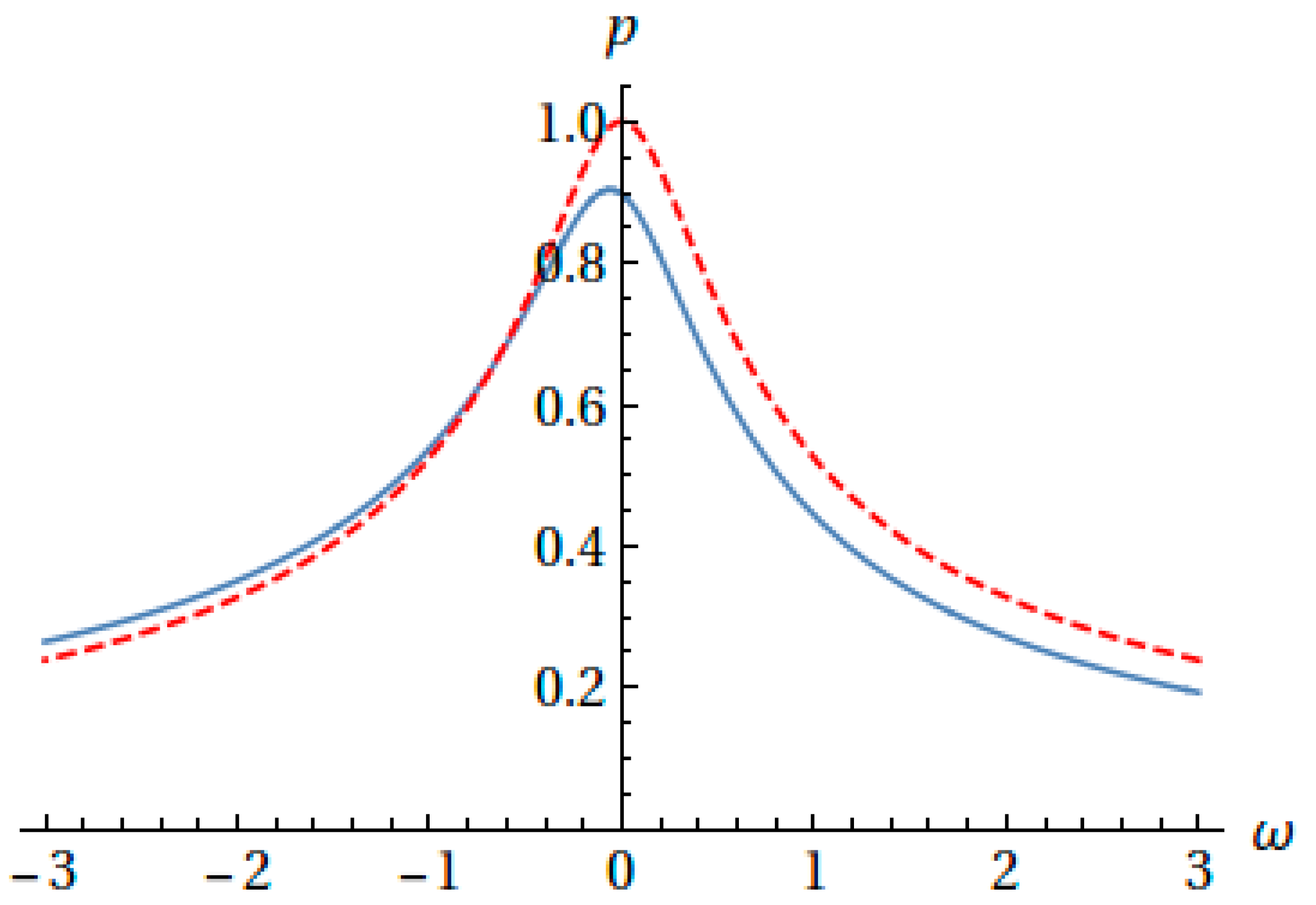
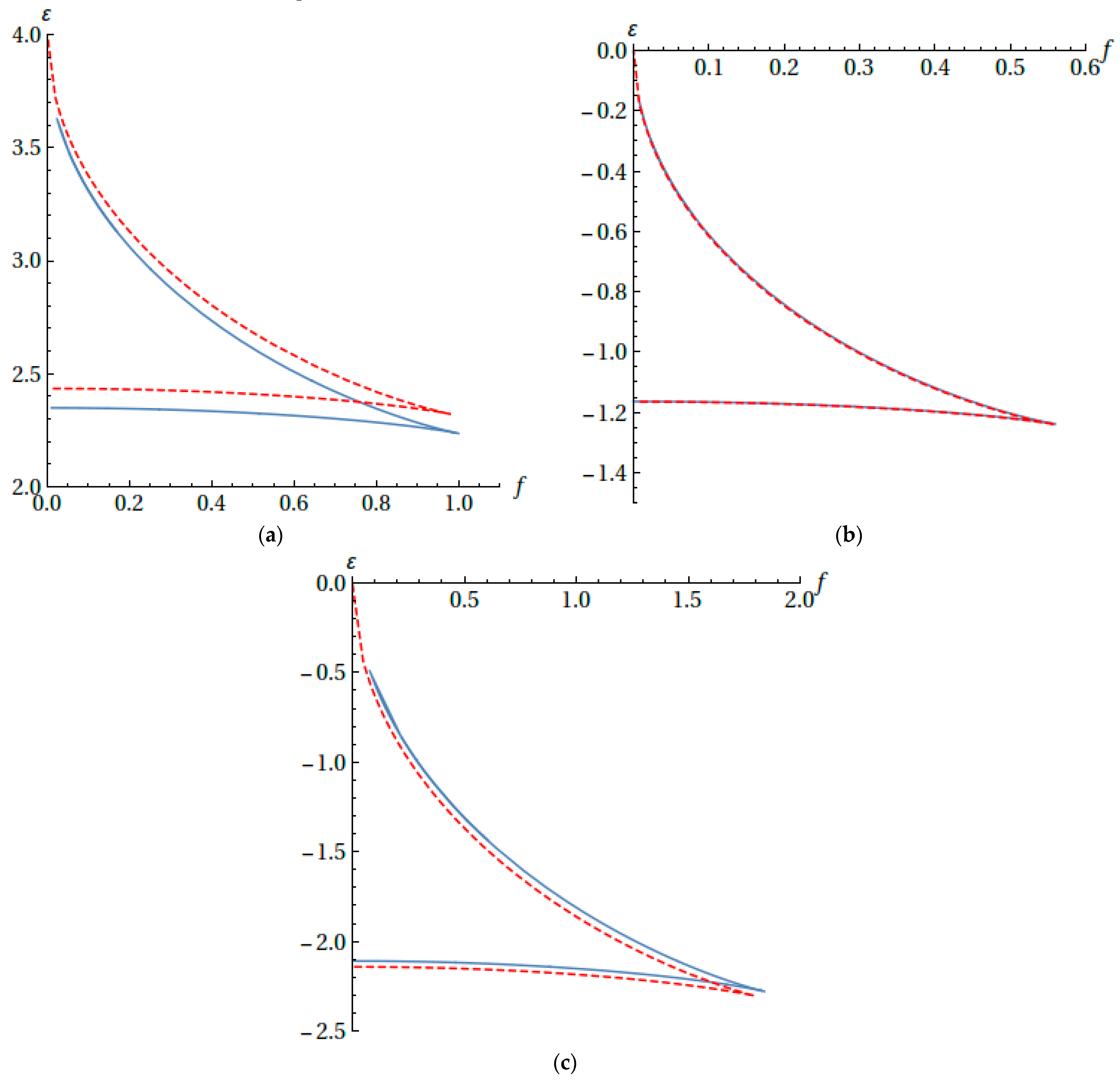
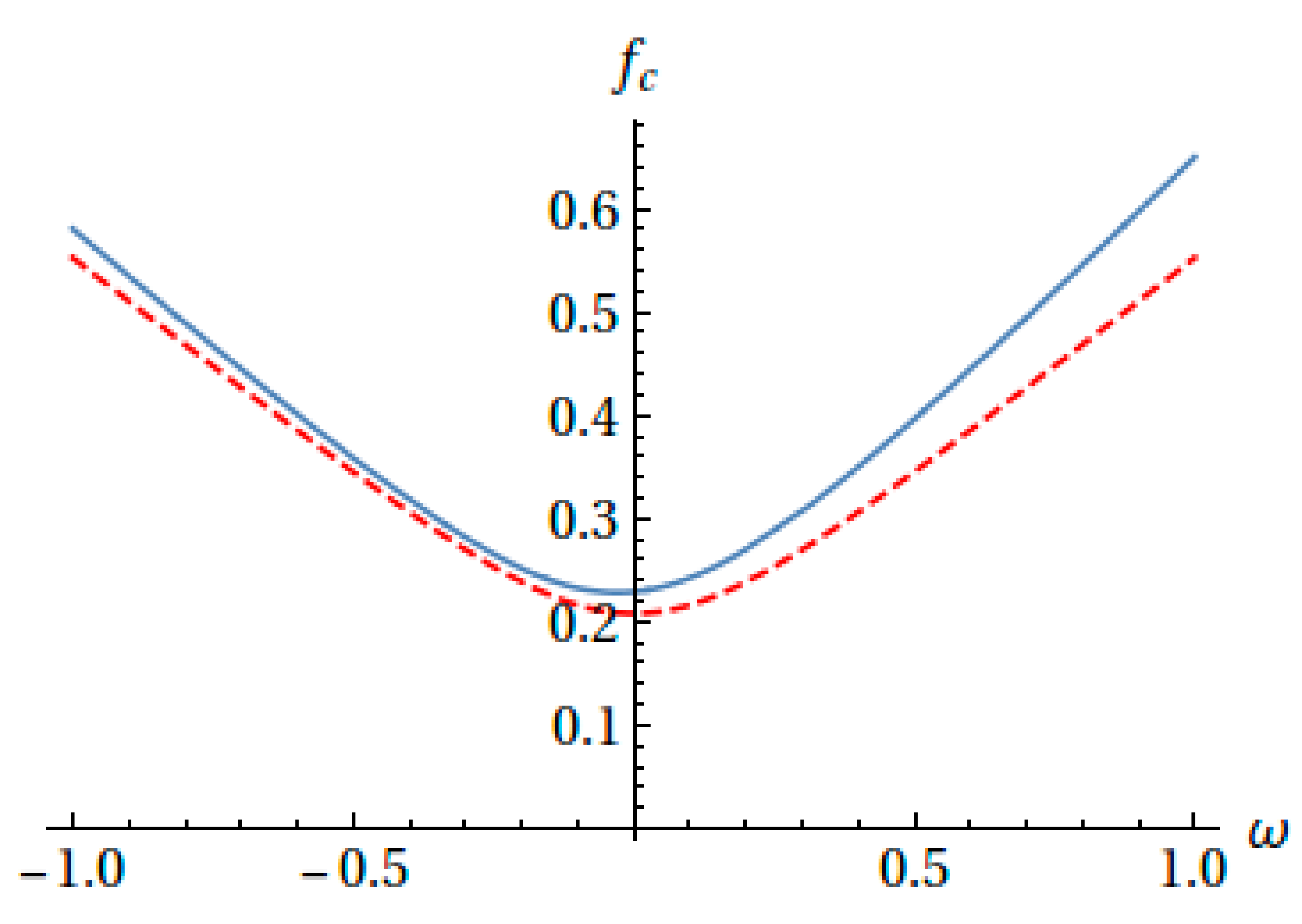
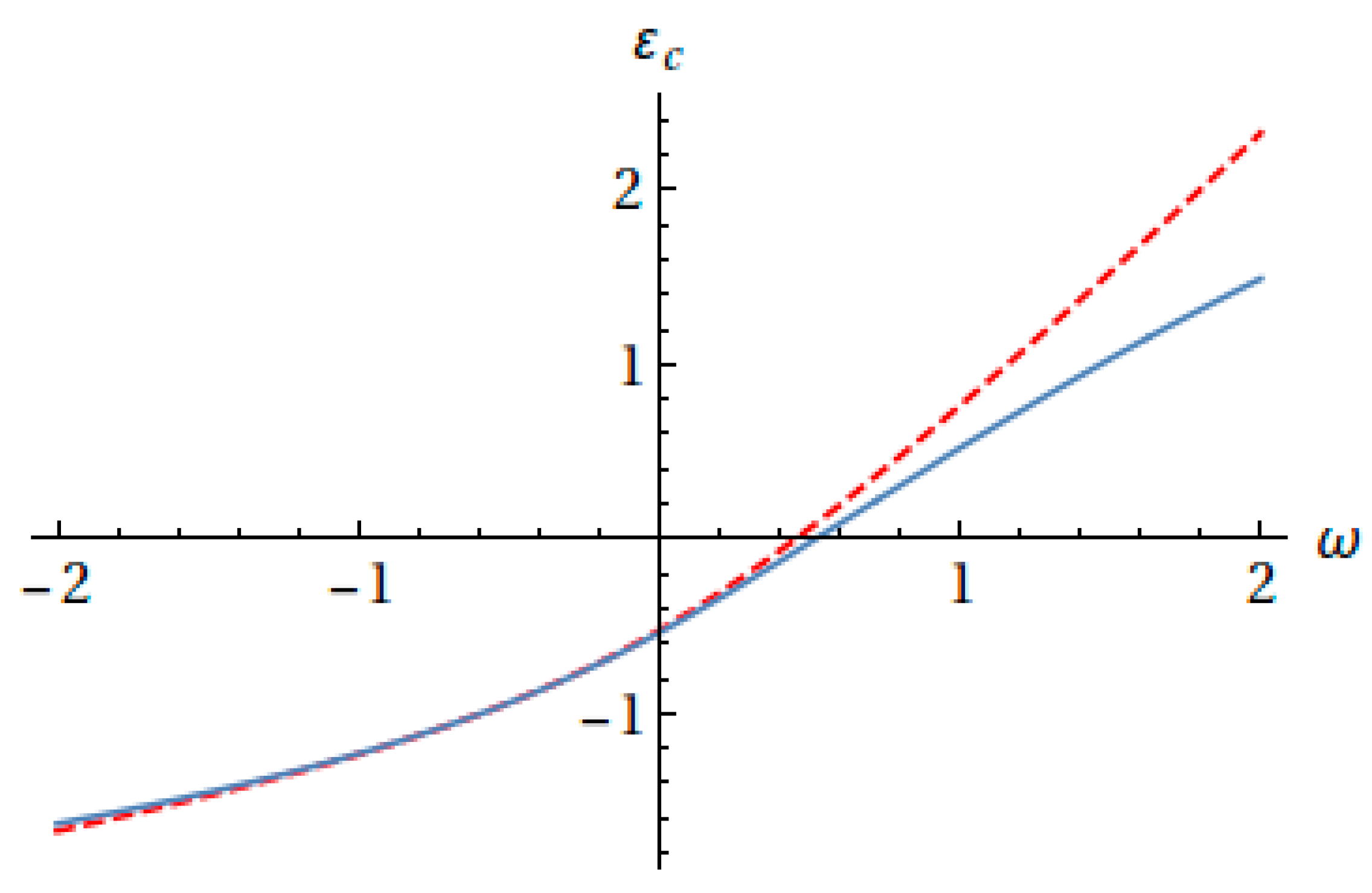
2. Results
3. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lee, E.; Farrelly, D.; Uzer, T. A Saturnian atom. Opt. Express 1997, 1, 221–228. [Google Scholar] [CrossRef] [PubMed]
- Germann, T.C.; Herschbach, D.R.; Dunn, M.; Watson, D.K. Circular Rydberg states of the hydrogen atom in a magnetic field. Phys. Rev. Lett. 1995, 74, 658. [Google Scholar] [CrossRef] [PubMed]
- Cheng, C.H.; Lee, C.Y.; Gallagher, T.F. Production of circular Rydberg states with circularly polarized microwave fields. Phys. Rev. Lett. 1994, 73, 3078. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.; Cheret, M.; Roussel, F.; Spiess, G. New scheme for producing circular orbital states in combined RF and static fields. J. Phys. B At. Mol. Opt. Phys. 1993, 26, L437. [Google Scholar] [CrossRef]
- Dutta, S.K.; Feldbaum, D.; Walz-Flannigan, A.; Guest, J.R.; Raithel, G. High-angular-momentum states in cold Rydberg gases. Phys. Rev. Lett. 2001, 86, 3993. [Google Scholar] [CrossRef] [PubMed]
- Hulet, R.G.; Hilfer, E.S.; Kleppner, D. Inhibited spontaneous emission by a Rydberg atom. Phys. Rev. Lett. 1985, 55, 2137. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- MacAdam, K.B.; Horsdal-Pedersen, E. Charge transfer from coherent elliptic states. J. Phys. B At. Mol. Opt. Phys. 2003, 36, R167. [Google Scholar] [CrossRef] [Green Version]
- Vainberg, V.M.; Popov, V.S.; Sergeev, A.V. The 1/n expansion for a hydrogen atom in an external field. Sov. Phys. JETP 1990, 71, 470–477. [Google Scholar]
- Oks, E. Circular Rydberg states of hydrogenlike systems in collinear electric and magnetic fields of arbitrary strengths: An exact analytical classical solution. Eur. Phys. J. D-At. Mol. Opt. Plasma Phys. 2004, 28, 171–179. [Google Scholar] [CrossRef]
- Haba, Y.; Nagaoka, K.; Tsumori, K.; Kisaki, M.; Takahashi, K.; Nakano, H.; Ikeda, K.; Yoshimura, S.; Osakabe, M. Response of beam focusing to plasma fluctuation in a filament-arc-type negative ion source. Jpn. J. Appl. Phys. 2020, 59, SHHA01. [Google Scholar] [CrossRef]
- Zhu, C.; Gao, Z.F.; Li, X.D.; Wang, N.; Yuan, J.P.; Peng, Q.H. Modified Fermi energy of electrons in a superhigh magnetic field. Mod. Phys. Lett. A 2016, 31, 1650070. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Gao, Z.F.; Jia, H.Y.; Wang, N.; Li, X.D. Estimation of electrical conductivity and magnetization parameter of neutron star crusts and applied to the high-braking-index pulsar PSR J1640-4631. Universe 2020, 6, 63. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kryukov, N.; Oks, E. Analytical Results for Classical Axially Symmetric States of Relativistic Hydrogenic Ions in Collinear Electric and Magnetic Fields of Arbitrary Strengths. Symmetry 2022, 14, 1515. https://doi.org/10.3390/sym14081515
Kryukov N, Oks E. Analytical Results for Classical Axially Symmetric States of Relativistic Hydrogenic Ions in Collinear Electric and Magnetic Fields of Arbitrary Strengths. Symmetry. 2022; 14(8):1515. https://doi.org/10.3390/sym14081515
Chicago/Turabian StyleKryukov, N., and E. Oks. 2022. "Analytical Results for Classical Axially Symmetric States of Relativistic Hydrogenic Ions in Collinear Electric and Magnetic Fields of Arbitrary Strengths" Symmetry 14, no. 8: 1515. https://doi.org/10.3390/sym14081515
APA StyleKryukov, N., & Oks, E. (2022). Analytical Results for Classical Axially Symmetric States of Relativistic Hydrogenic Ions in Collinear Electric and Magnetic Fields of Arbitrary Strengths. Symmetry, 14(8), 1515. https://doi.org/10.3390/sym14081515






