New Comparison Results for Oscillation of Even-Order Delay Differential Equations
Abstract
:1. Introduction
- (H1)
- is an even natural number, is a natural number, and ;
- (H2)
- and for
- (H3)
- and
- (H4)
- and for
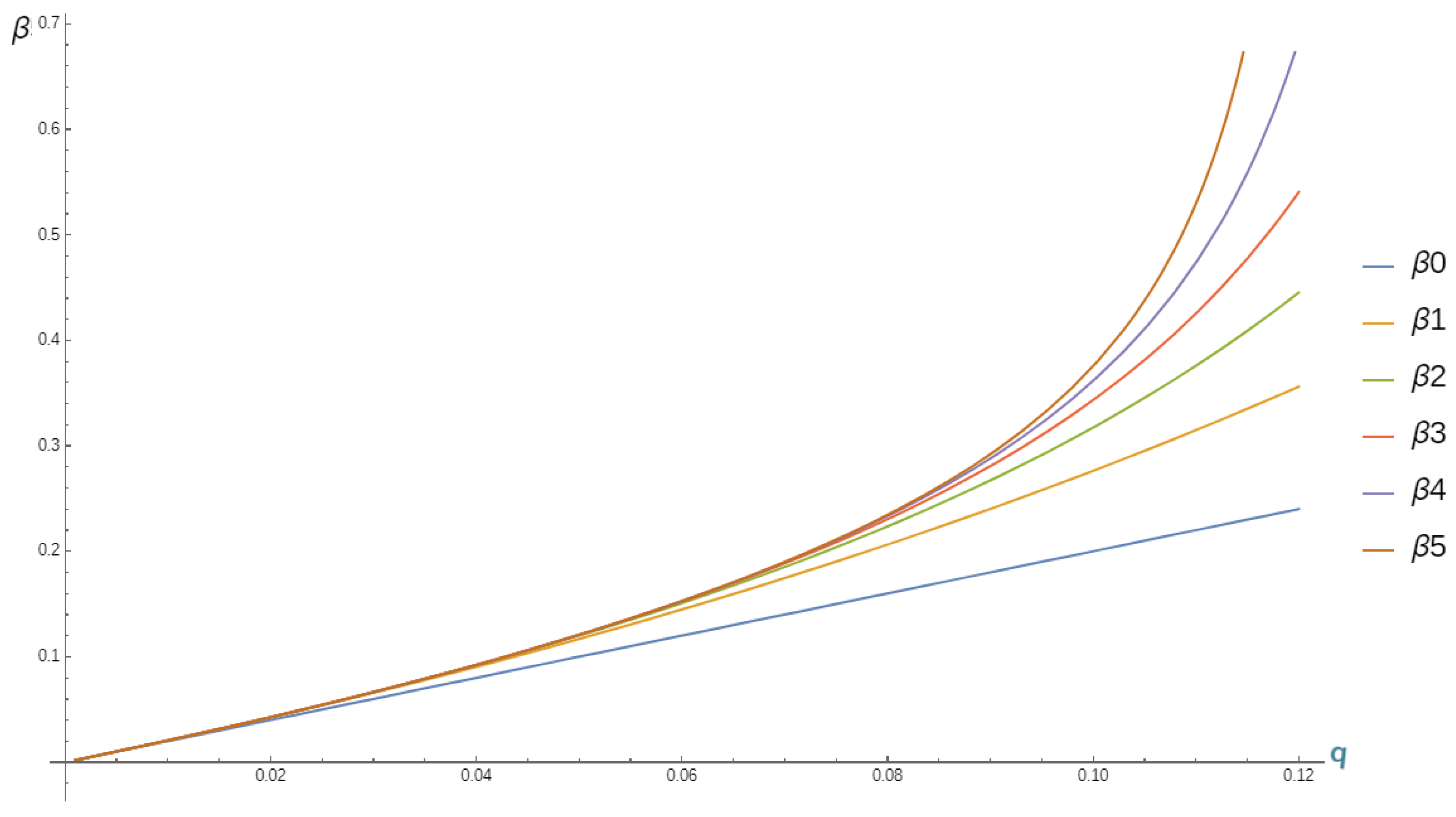
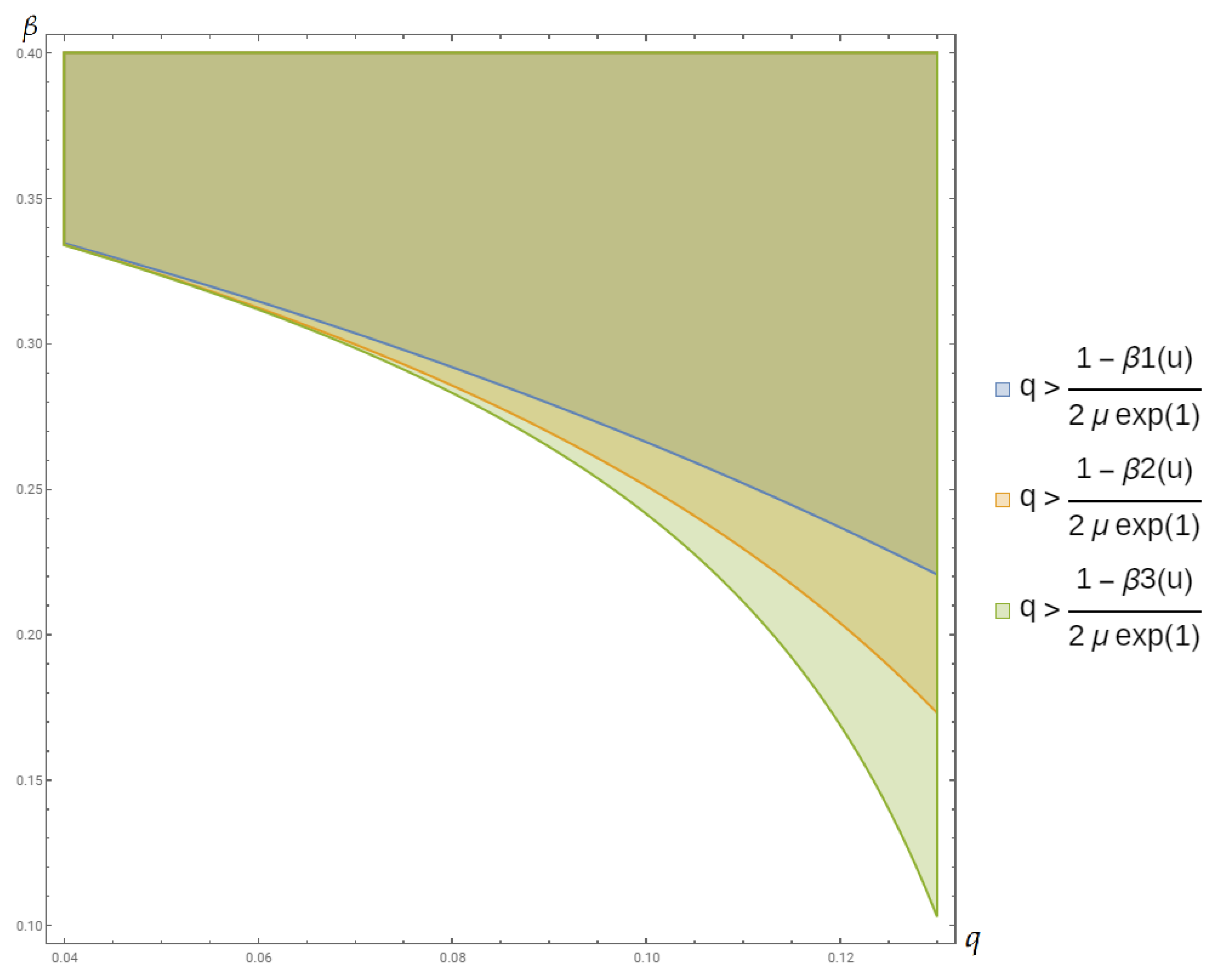
2. Criteria for Nonexistence of Positive Decreasing Solution
3. Oscillation Theorem
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Moaaz, O.; Chalishajar, D.; Bazighifan, O. Some qualitative behavior of solutions of general class of difference equations. Mathematics 2019, 7, 585. [Google Scholar] [CrossRef] [Green Version]
- Mukhin, R.R. Legacy of Alexander Mikhailovich Lyapunov and nonlinear dynamics. Appl. Nonlinear Dyn. 2018, 26, 95–120. [Google Scholar] [CrossRef]
- Myshkis, A.D. On certain problems in the theory of differential equations with deviating argument. Russ. Math. Surv. 1977, 32, 181. [Google Scholar] [CrossRef]
- Myshkis, A.D. Linear Differential Equations with Retarded Argument: Russian Book on Linear Differential Delay Equations Covering Solvability Theorems, Solution Properties, Stable and Unstable Equations, First and Second Order Equations, Periodic Equations, Etc; Izdatel’stvo Nauka: Moscow, Russia, 1972; p. 352. [Google Scholar]
- Agarwal, R.P.; Grace, S.R.; O’Regan, D. Oscillation Theory for Second Order Linear, Half-Linear, Superlinear and Sublinear Dynamic Equations; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2002. [Google Scholar] [CrossRef] [Green Version]
- Agarwal, S.R.; Grace, S.R.; O’Regan, D. Oscillation Theory for Second Order Dynamic Equations; Series in Mathematical Analysis and Applications; Taylor & Francis Group: London, UK, 2003; Volume 5. [Google Scholar]
- Agarwal, R.P.; Bohner, M.; Li, W.T. Nonoscillation and Oscillation: Theory for Functional Differential Equations; Marcel Dekker, Inc.: New York, NY, USA, 2004. [Google Scholar] [CrossRef]
- Agarwal, S.R.; Grace, S.R.; O’Regan, D. Oscillation Theory for Difference and Functional Differential Equations; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2000. [Google Scholar] [CrossRef]
- Došlý, O.; Rehák, P. Half-Linear Differential Equations, North-Holland Mathematics Studies; Elsevier: Amsterdam, The Netherlands, 2005; p. 202. [Google Scholar]
- Gyori, I.; Ladas, G. Oscillation Theory of Delay Differential Equations with Applications; Oxford University Press: New York, NY, USA, 1991. [Google Scholar]
- Saker, S.H. Oscillation Theory of Delay Differential and Difference Equations: Second and Third Orders; LAP Lambert Academic Publishing: Chisinau, Moldova, 2010. [Google Scholar]
- Agarwal, R.P.; Grace, S.R.; O’Regan, D. Oscillation criteria for certain nth order differential equations with deviating arguments. J. Math. Appl. Anal. 2001, 262, 601–622. [Google Scholar] [CrossRef] [Green Version]
- Agarwal, R.P.; Grace, S.R.; O’Regan, D. The oscillation of certain higher-order functional differential equations. Math. Comput. Model. 2003, 37, 705–728. [Google Scholar] [CrossRef]
- Grace, S.R. Oscillation theorems for nth-order differential equations with deviating arguments. J. Math. Appl. Anal. 1984, 101, 268–296. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z.; Xia, Y. Integral averaging technique and oscillation of certain even order delay differential equations. J. Math. Appl. Anal. 2004, 292. [Google Scholar] [CrossRef]
- Moaaz, O.; Kumam, P.; Bazighifan, O. On the oscillatory behavior of a class of fourth-order nonlinear differential equation. Symmetry 2020, 12, 524. [Google Scholar] [CrossRef] [Green Version]
- Park, C.; Moaaz, O.; Bazighifan, O. Oscillation results for higher order differential equations. Axioms 2020, 9, 14. [Google Scholar] [CrossRef] [Green Version]
- Baculíková, B.; Džurina, J.; Graef, J.R. On the oscillation of higher-order delay differential equations. J. Math. Sci. 2012, 187, 387–400. [Google Scholar] [CrossRef]
- Zhang, C.; Li, T.; Suna, B.; Thandapani, E. On the oscillation of higher-order half-linear delay differential equations. Appl. Math. Lett. 2011, 24, 1618–1621. [Google Scholar] [CrossRef] [Green Version]
- Moaaz, O.; Muhib, A. New oscillation criteria for nonlinear delay differential equations of fourth-order. Appl. Math. Comput. 2020, 377, 125192. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Zhang, C.; Li, T. Some remarks on oscillation of second order neutral differential equations. Appl. Math. Comput. 2016, 274, 178–181. [Google Scholar] [CrossRef]
- Baculíková, B. Oscillation of second-order nonlinear noncanonical differential equations with deviating argument. Appl. Math. Lett. 2019, 91, 68–75. [Google Scholar] [CrossRef]
- Baculíková, B. Oscillatory behavior of the second order noncanonical differential equations. Electron. J. Qual. Theory Differ. Equ. 2019, 89, 1–11. [Google Scholar] [CrossRef]
- Koplatadze, R. Criteria for the oscillation of solutions of differential inequalities and second-order equations with retarded argument. Tbiliss. Gos. Univ. Inst. Prikl. Mat. Trudy 1986, 17, 104–121. [Google Scholar]
- Dzurina, J.; Jadlovská, I. A note on oscillation of second-order delay differential equations. Appl. Math. Lett. 2017, 69, 126–132. [Google Scholar] [CrossRef]
- Baculíková, B.; Dzurina, J. Oscillation theorems for higher order neutral differential equations. Appl. Math. Comput. 2012, 219, 3769–3778. [Google Scholar] [CrossRef]
- Philos, C.G. On the existence of nonoscillatory solutions tending to zero at ∞ for differential equations with positive delays. Arch. Math. 1981, 36, 168–178. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almarri, B.; Masood, F.; Muhib, A.; Moaaz, O. New Comparison Results for Oscillation of Even-Order Delay Differential Equations. Symmetry 2022, 14, 946. https://doi.org/10.3390/sym14050946
Almarri B, Masood F, Muhib A, Moaaz O. New Comparison Results for Oscillation of Even-Order Delay Differential Equations. Symmetry. 2022; 14(5):946. https://doi.org/10.3390/sym14050946
Chicago/Turabian StyleAlmarri, Barakah, Fahd Masood, Ali Muhib, and Osama Moaaz. 2022. "New Comparison Results for Oscillation of Even-Order Delay Differential Equations" Symmetry 14, no. 5: 946. https://doi.org/10.3390/sym14050946
APA StyleAlmarri, B., Masood, F., Muhib, A., & Moaaz, O. (2022). New Comparison Results for Oscillation of Even-Order Delay Differential Equations. Symmetry, 14(5), 946. https://doi.org/10.3390/sym14050946







