Topological Superconducting Transition Characterized by a Modified Real-Space-Pfaffian Method and Mobility Edges in a One-Dimensional Quasiperiodic Lattice
Abstract
:1. Introduction
2. Model and Hamiltonian
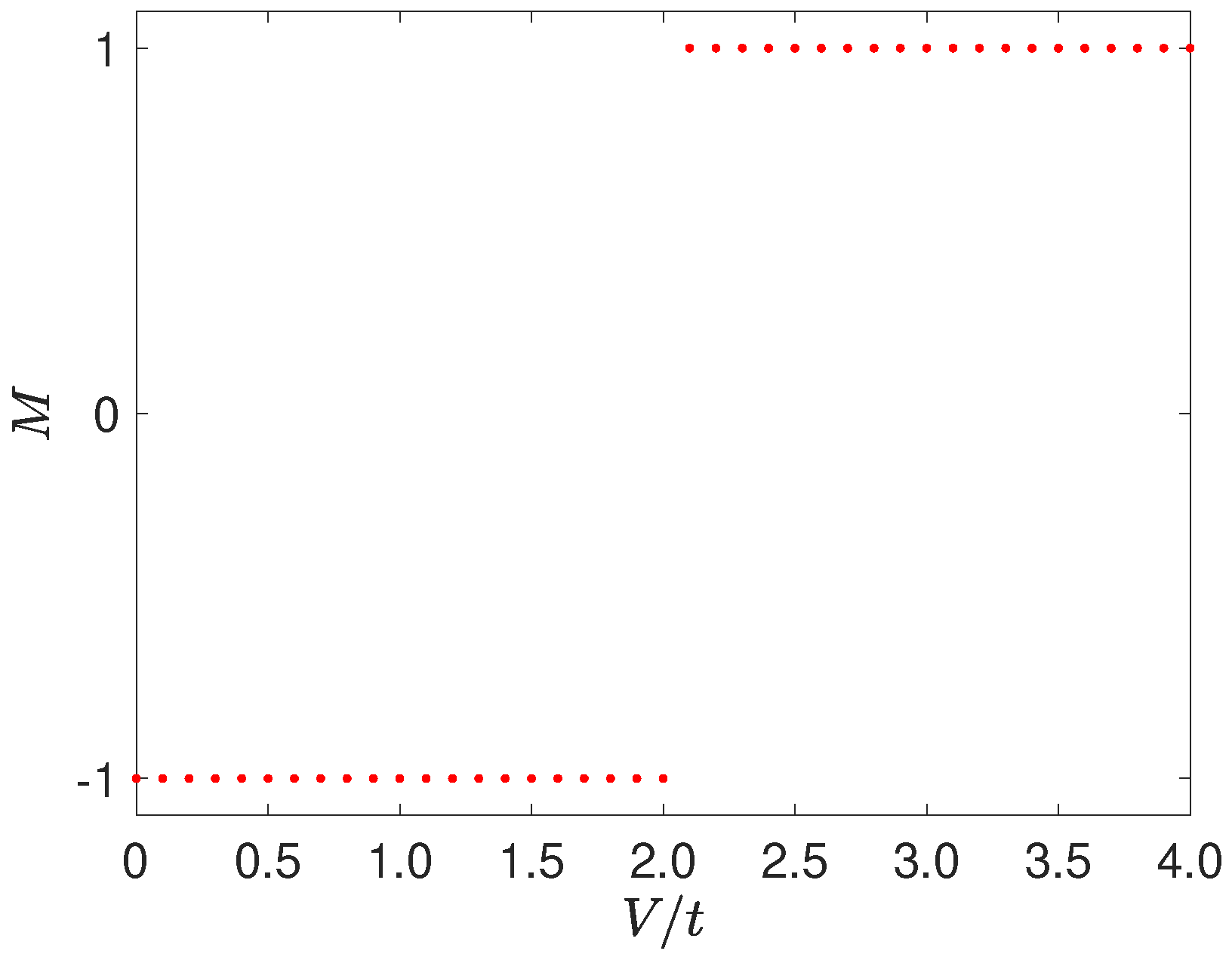
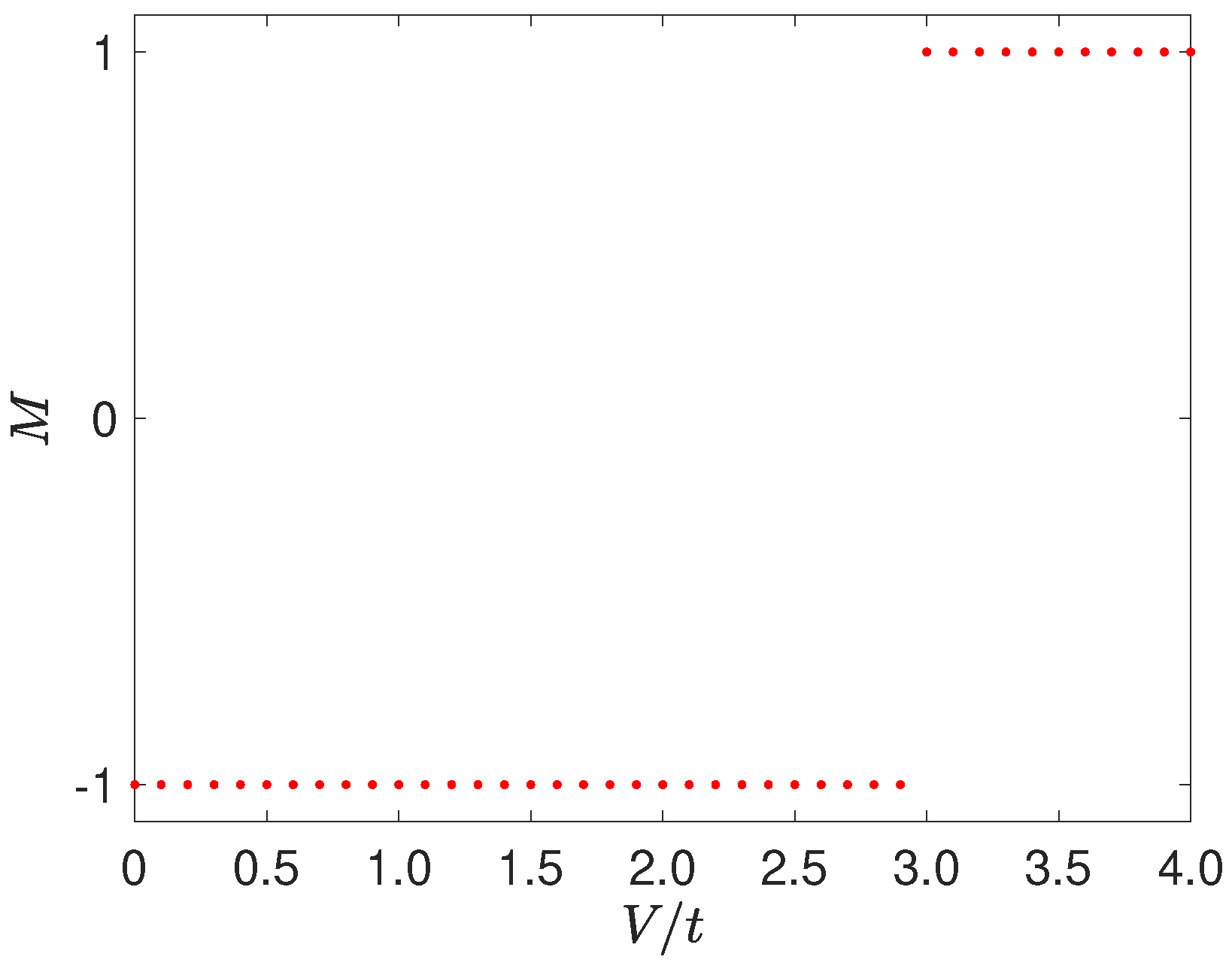
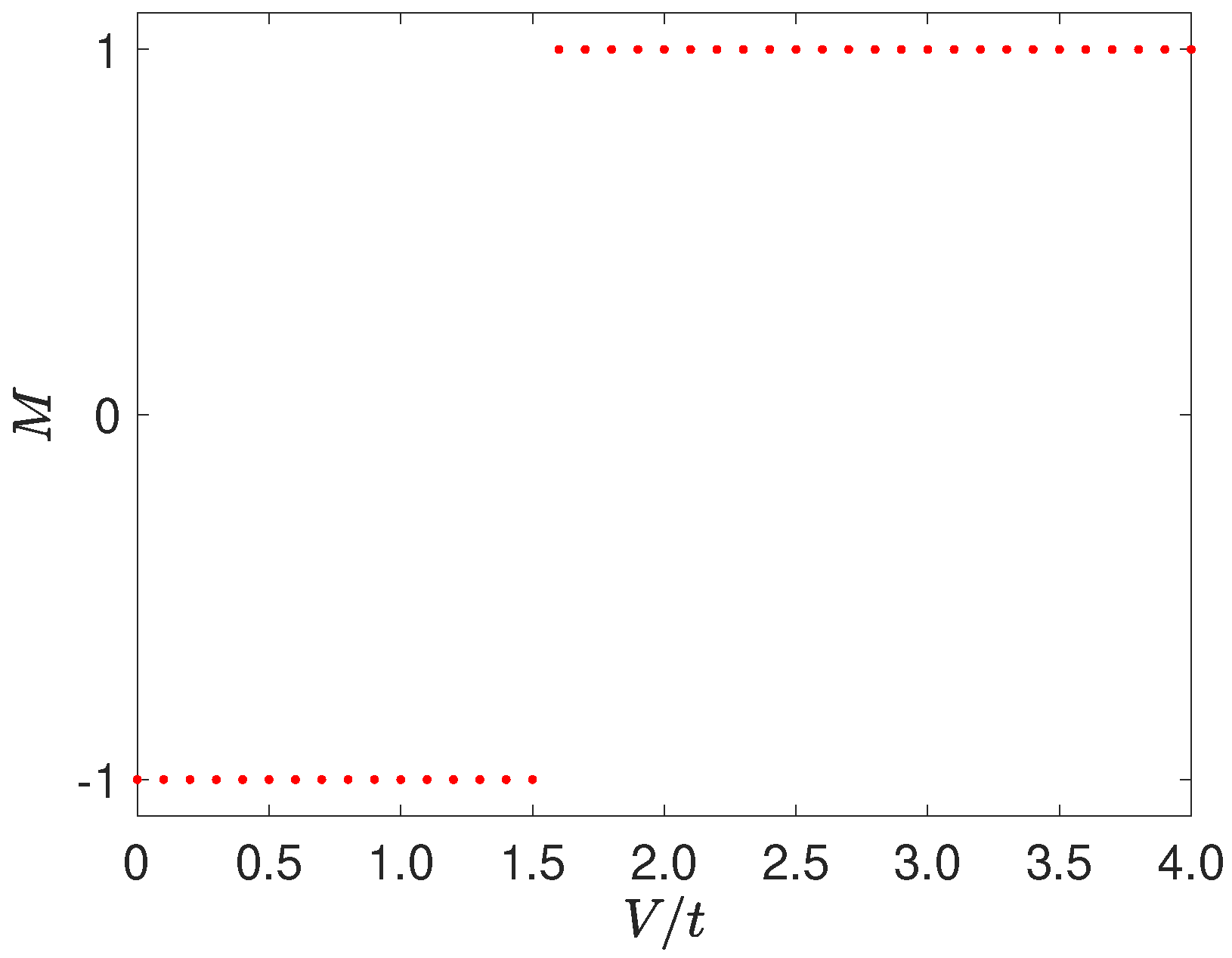
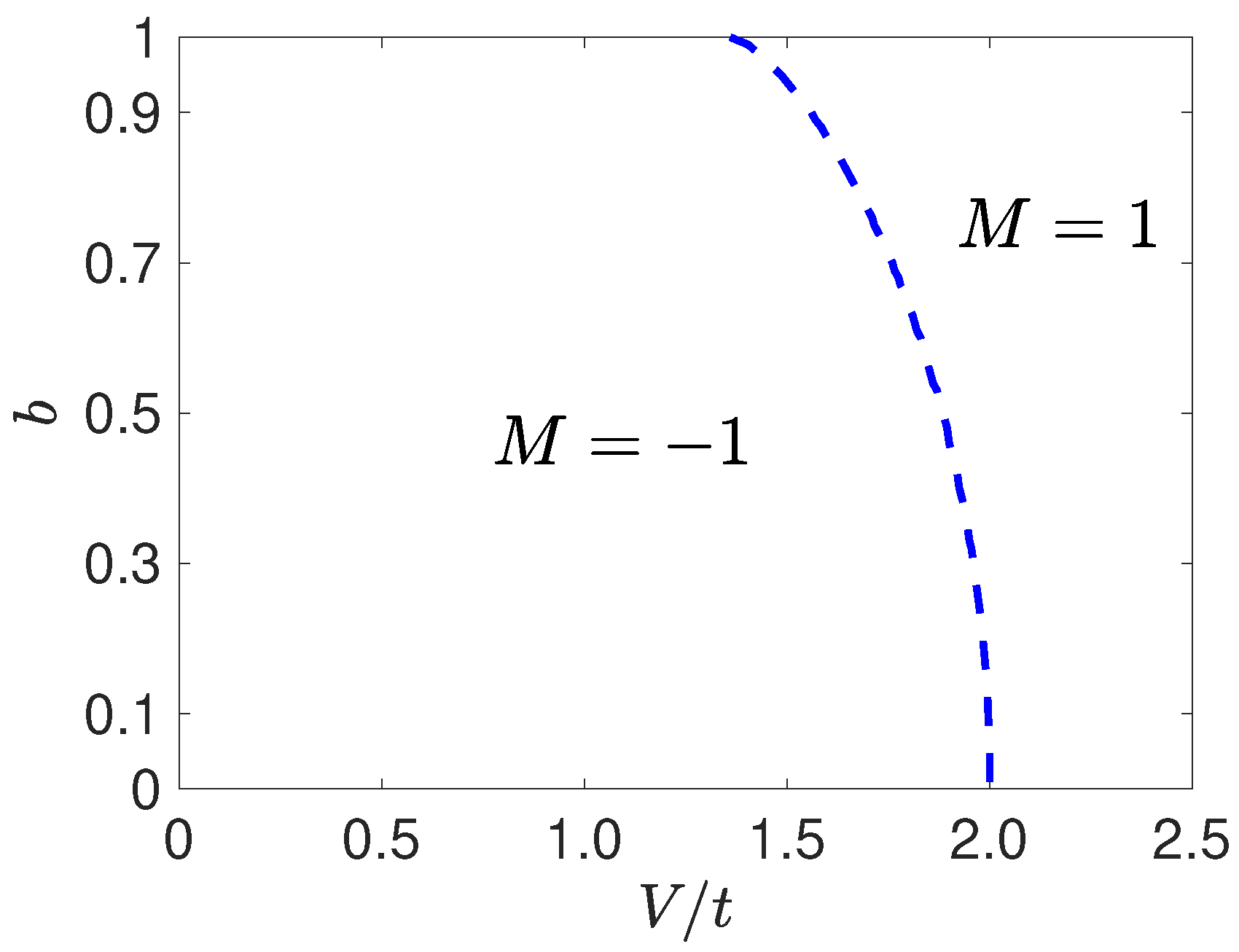
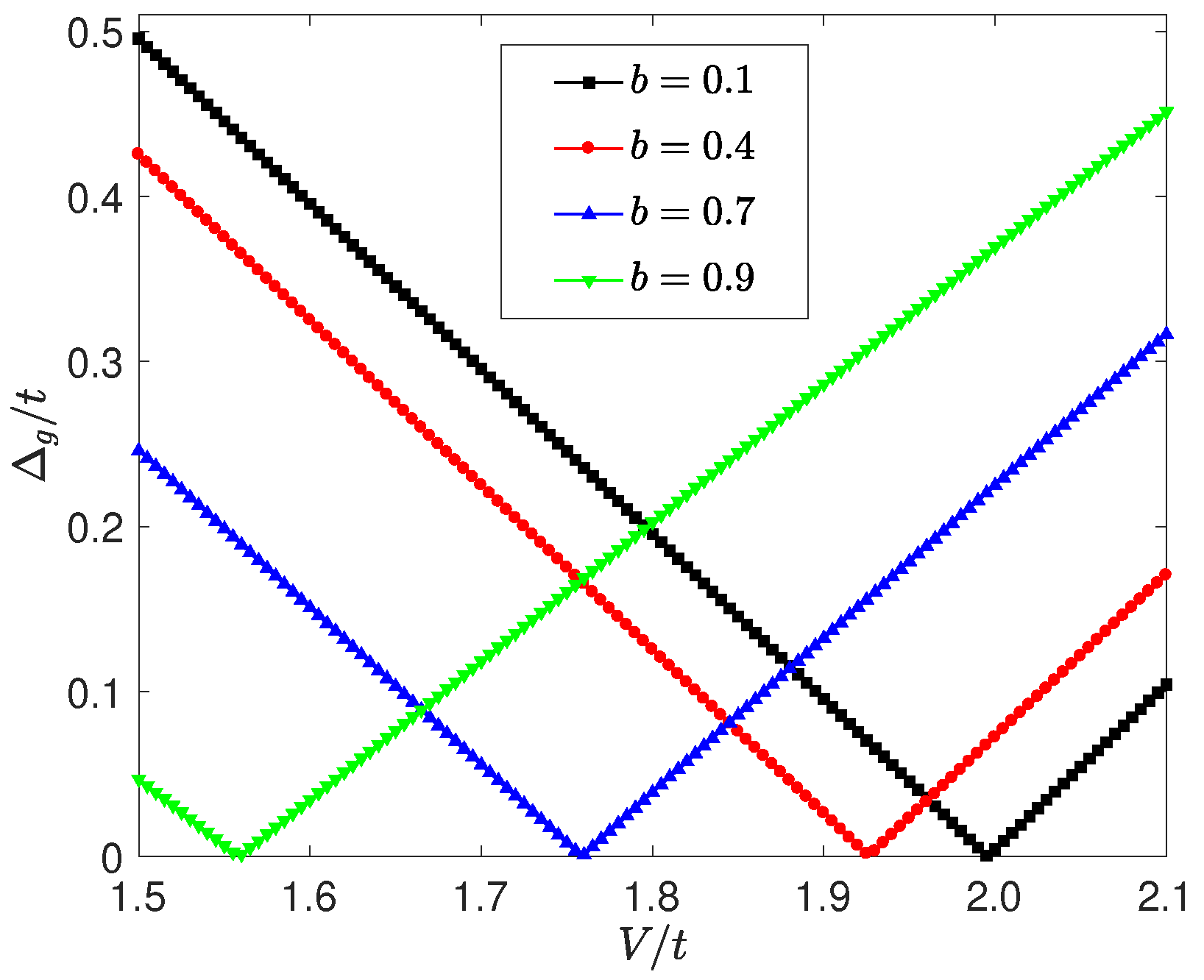
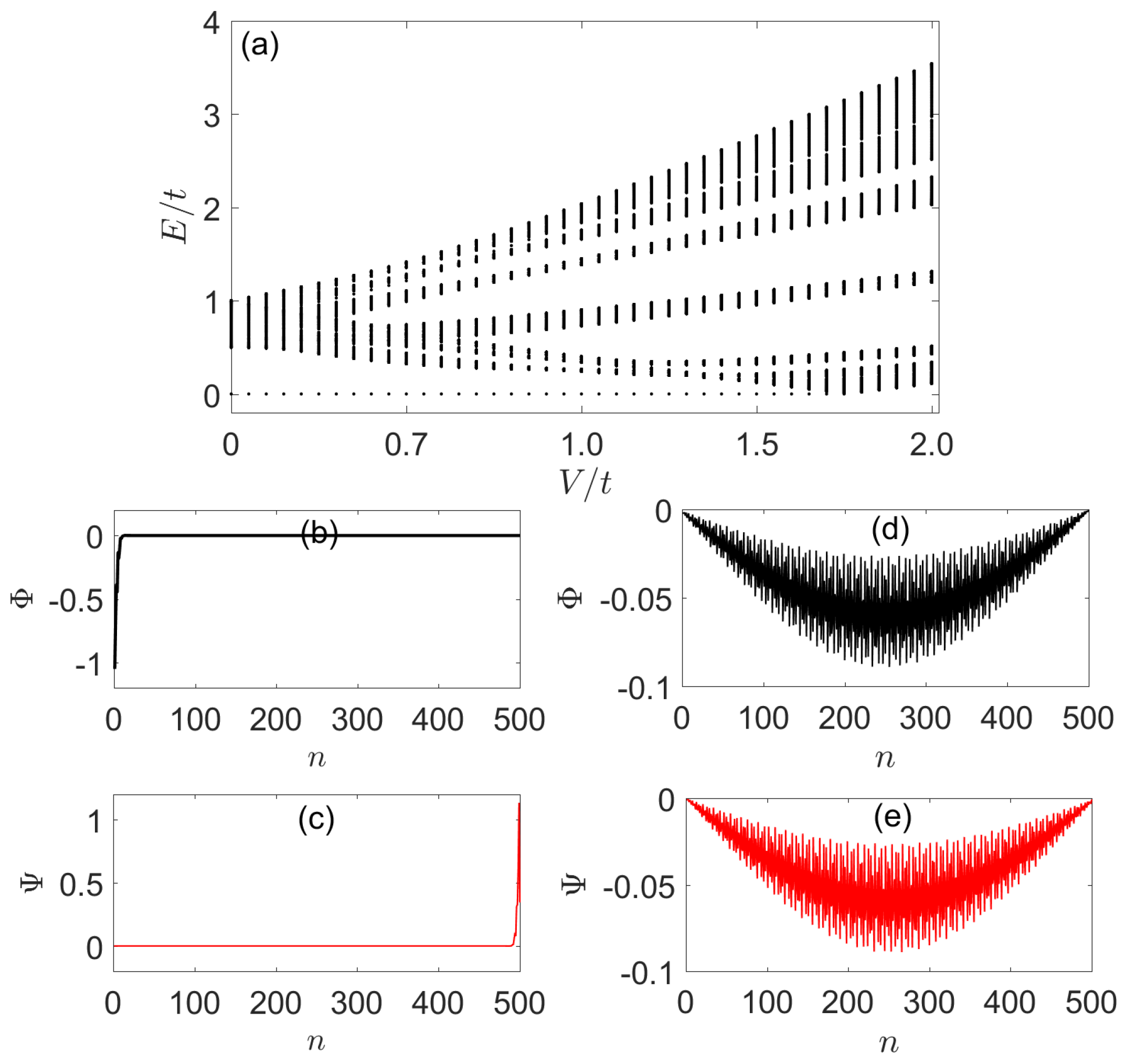
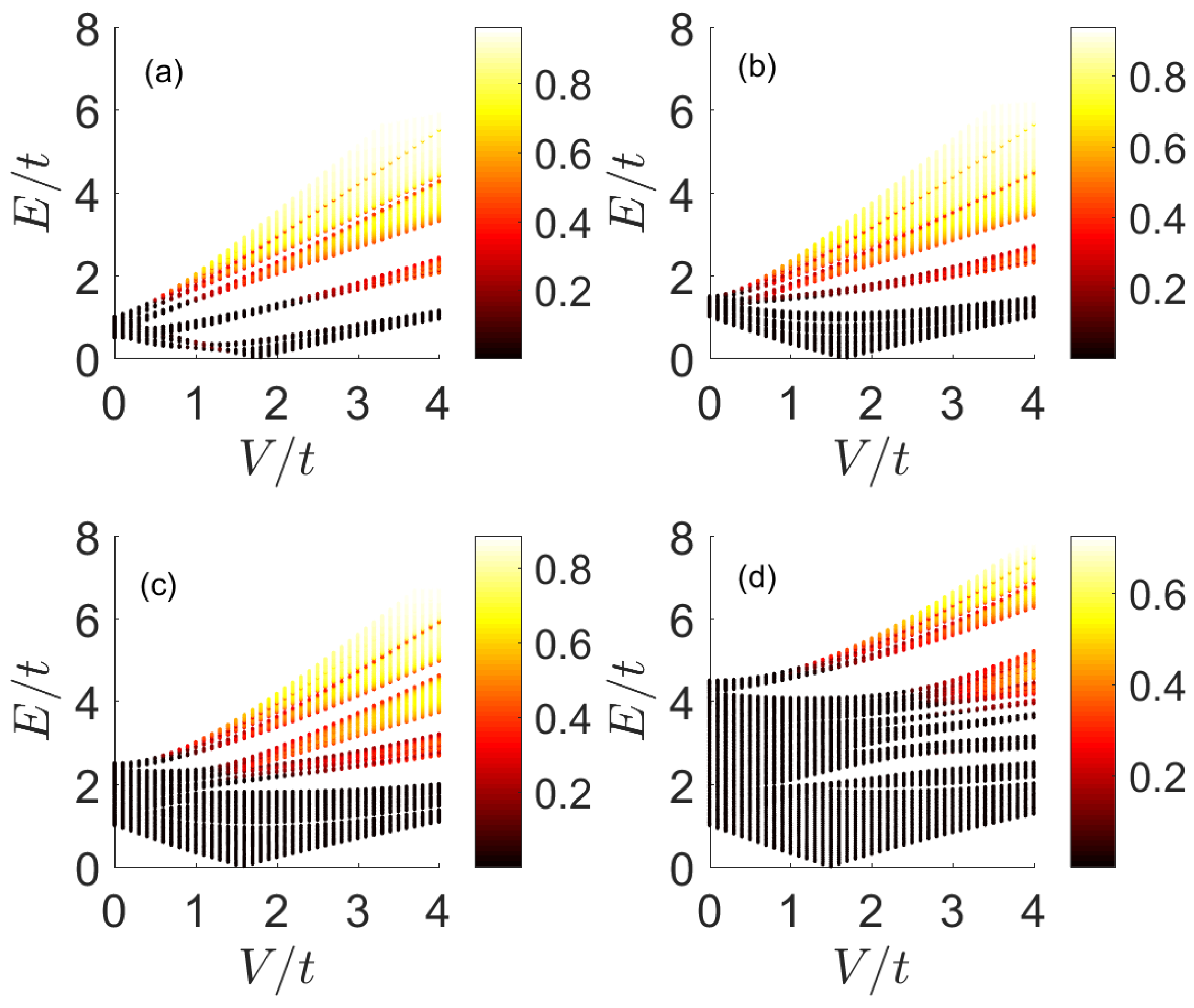
3. Results
4. Summary
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Modified Real-Space-Pfaffian Method
References
- Qi, X.L.; Zhang, S.C. Topological insulators and superconductors. Rev. Mod. Phys. 2011, 83, 1057. [Google Scholar] [CrossRef] [Green Version]
- Shen, S.Q. Topological Insulators; Springer: Berlin/Heidelberg, Germany, 2012; pp. 1–221. [Google Scholar] [CrossRef] [Green Version]
- Aguado, R. Majorana quasiparticles in condensed matter. Riv. Nuovo Cim. 2017, 40, 523. [Google Scholar] [CrossRef]
- Mourik, V.; Zuo, K.; Frolov, S.M.; Plissard, S.R.; Bakkers, E.P.A.M.; Kouwenhoven, L.P. Signatures of Majorana Fermions in Hybrid Superconductor-Semiconductor Nanowire Devices. Science 2012, 336, 1003. [Google Scholar] [CrossRef] [Green Version]
- Albrecht, S.M.; Higginbotham, A.P.; Madsen, M.; Kuemmeth, F.; Jesperson, T.S.; Nygard, J.; Krogstrup, P.; Marcus, C.M. Exponential protection of zero modes in Majorana islands. Nature 2016, 531, 206. [Google Scholar] [CrossRef] [Green Version]
- Deng, M.T.; Vaitiekenas, S.; Hansen, E.B.; Danon, J.; Leijnse, M.; Flensberg, K.; Nygard, J.; Krogstrup, P.; Marcus, C.M. Majorana bound state in a coupled quantum-dot hybrid-nanowire system. Science 2016, 354, 1557. [Google Scholar] [CrossRef] [Green Version]
- Chen, J.; Yu, P.; Stenger, J.; Hocevar, M.; Car, D.; Plissard, S.R.; Bakkers, E.P.A.M.; Stanescu, T.D.; Frolov, S.M. Experimental phase diagram of zero-bias conductance peaks in superconductor/semiconductor nanowire devices. Sci. Adv. 2017, 3, e1701476. [Google Scholar] [CrossRef] [Green Version]
- Klinovaja, J.; Stano, P.; Loss, D. Transition from Fractional to Majorana Fermions in Rashba Nanowires. Phys. Rev. Lett. 2012, 109, 236801. [Google Scholar] [CrossRef]
- Liu, X.J.; Jiang, L.; Pu, H.; Hu, H. Probing Majorana fermions in spin-orbit-coupled atomic Fermi gases. Phys. Rev. A 2012, 85, 021603. [Google Scholar] [CrossRef] [Green Version]
- Qu, C.; Zheng, Z.; Gong, M.; Xu, Y.; Mao, L.; Zou, X.; Guo, G.; Zhang, C. Topological superfluids with finite-momentum pairing and Majorana fermions. Nat. Commun. 2013, 4, 2710. [Google Scholar] [CrossRef] [Green Version]
- Chen, C. Inhomogeneous Topological Superfluidity in One-Dimensional Spin-Orbit-Coupled Fermi Gases. Phys. Rev. Lett. 2013, 111, 235302. [Google Scholar] [CrossRef] [Green Version]
- Ruhman, J.; Berg, E.; Altman, E. Topological States in a One-Dimensional Fermi Gas with Attractive Interaction. Phys. Rev. Lett. 2015, 114, 100401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nadj-Perge, S.; Drozdov, I.K.; Bernevig, B.A.; Yazdani, A. Proposal for realizing Majorana fermions in chains of magnetic atoms on a superconductor. Phys. Rev. B 2013, 88, 020407. [Google Scholar] [CrossRef] [Green Version]
- Nadj-Perge, S.; Drozdov, I.K.; Li, J.; Chen, H.; Jeon, S.; Seo, J.; MacDonald, A.H.; Bernevig, B.A.; Yazdani, A. Observation of Majorana fermions in ferromagnetic atomic chains on a superconductor. Science 2014, 346, 602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dumitrescu, E.; Roberts, B.; Tewari, S.; Sau, J.D.; Sarma, S.D. Majorana fermions in chiral topological ferromagnetic nanowires. Phys. Rev. B 2015, 91, 094505. [Google Scholar] [CrossRef] [Green Version]
- Jeon, S.; Xie, Y.L.; Wang, Z.J.; Bernevig, B.A.; Yazdani, A. Distinguishing a Majorana zero mode using spin-resolved measurements. Science 2017, 358, 772. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fu, L.; Kane, C.L. Superconducting Proximity Effect and Majorana Fermions at the Surface of a Topological Insulator. Phys. Rev. Lett. 2008, 100, 096407. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cook, A.; Franz, M. Majorana fermions in a topological-insulator nanowire proximity-coupled to an s-wave superconductor. Phys. Rev. B 2011, 84, 201105. [Google Scholar] [CrossRef] [Green Version]
- Tanaka, Y.; Sato, M.; Nagaosa, N. Symmetry and Topology in Superconductors –Odd-Frequency Pairing and Edge States. J. Phys. Soc. Jpn. 2012, 81, 011013. [Google Scholar] [CrossRef] [Green Version]
- Sun, H.H.; Zhang, K.W.; Hu, L.H.; Li, C.; Wang, G.Y.; Ma, H.Y.; Xu, Z.A.; Gao, C.L.; Guan, D.D.; Li, Y.Y.; et al. Majorana Zero Mode Detected with Spin Selective Andreev Reflection in the Vortex of a Topological Superconductor. Phys. Rev. Lett. 2016, 116, 257003. [Google Scholar] [CrossRef] [Green Version]
- Hell, M.; Leijnse, M.; Flensberg, K. Two-Dimensional Platform for Networks of Majorana Bound States. Phys. Rev. Lett. 2017, 118, 107701. [Google Scholar] [CrossRef] [Green Version]
- Pientka, F.; Keselman, A.; Berg, E.; Yacoby, A.; Stern, A.; Halperin, B.I. Topological Superconductivity in a Planar Josephson Junction. Phys. Rev. X 2017, 7, 021032. [Google Scholar] [CrossRef] [Green Version]
- Fornieri, A.; Whiticar, A.M.; Setiawan, F.; Marín, E.P.; Asbjórn, C.C.D.; Keselman, A.; Gronin, S.; Thomas, C.; Wang, T.; Kallaher, R.; et al. Evidence of topological superconductivity in planar Josephson junctions. Nature 2019, 569, 89. [Google Scholar] [CrossRef] [PubMed]
- Takagi, D.; Tamura, S.; Tanaka, Y. Odd-frequency pairing and proximity effect in Kitaev chain systems including a topological critical point. Phys. Rev. B 2020, 101, 024509. [Google Scholar] [CrossRef] [Green Version]
- Nayak, C.; Simon, S.H.; Stern, A.; Freedman, M.; Sarma, S.D. Non-Abelian anyons and topological quantum computation. Rev. Mod. Phys. 2008, 80, 1083. [Google Scholar] [CrossRef] [Green Version]
- Aguado, R.; Kouwenhoven, L.P. Majorana qubits for topological quantum computing. Phys. Today 2020, 73, 44. [Google Scholar] [CrossRef]
- Alicea, J. New directions in the pursuit of Majorana fermions in solid state systems. Rep. Prog. Phys. 2012, 75, 076501. [Google Scholar] [CrossRef] [Green Version]
- Beenakker, C.W.J. Search for Majorana Fermions in Superconductors. Ann. Rev. Condens. Matter Phys. 2013, 4, 113. [Google Scholar] [CrossRef] [Green Version]
- Elliott, S.R.; Franz, M. Colloquium: Majorana fermions in nuclear, particle, and solid-state physics. Rev. Mod. Phys. 2015, 87, 137. [Google Scholar] [CrossRef] [Green Version]
- Ando, Y.; Fu, L. Topological Crystalline Insulators and Topological Superconductors: From Concepts to Materials. Annu. Rev. Condens. Matter Phys. 2015, 6, 361. [Google Scholar] [CrossRef]
- Sato, M.; Ando, Y. Topological superconductors: A review. Rep. Prog. Phys. 2017, 80, 076501. [Google Scholar] [CrossRef] [Green Version]
- Becerra, V.F.; Sardella, E.; Peeters, F.M.; Milošević, M.V. Vortical versus skyrmionic states in mesoscopic p-wave superconductors. Phys. Rev. B 2016, 93, 014518. [Google Scholar] [CrossRef] [Green Version]
- Aguirre, C.; Blas, H.; Barba-Ortega, J. Mesoscale vortex pinning landscapes in a two component superconductor. Phys. C 2018, 554, 8. [Google Scholar] [CrossRef]
- Kitaev, A.Y. Unpaired Majorana fermions in quantum wires. Phys. Usp. 2001, 44, 131. [Google Scholar] [CrossRef]
- Anderson, P.W. Absence of Diffusion in Certain Random Lattices. Phys. Rev. 1958, 109, 1492. [Google Scholar] [CrossRef]
- Brouwer, P.W.; Furusaki, A.; Gruzberg, I.A.; Mudry, C. Localization and Delocalization in Dirty Superconducting Wires. Phys. Rev. Lett. 2000, 85, 1064. [Google Scholar] [CrossRef] [Green Version]
- Motrunich, O.; Damle, K.; Huse, D.A. Griffiths effects and quantum critical points in dirty superconductors without spin-rotation invariance: One-dimensional examples. Phys. Rev. B 2001, 63, 224204. [Google Scholar] [CrossRef] [Green Version]
- Gruzberg, I.A.; Read, N.; Vishveshwara, S. Localization in disordered superconducting wires with broken spin-rotation symmetry. Phys. Rev. B 2005, 71, 245124. [Google Scholar] [CrossRef] [Green Version]
- Brouwer, P.W.; Duckheim, M.; Romita, A.; Oppen, F.V. Probability Distribution of Majorana End-State Energies in Disordered Wires. Phys. Rev. Lett. 2011, 107, 196804. [Google Scholar] [CrossRef] [Green Version]
- Lobos, A.; Lutchyn, R.; Sarma, S.D. Interplay of Disorder and Interaction in Majorana Quantum Wires. Phys. Rev. Lett. 2012, 109, 146403. [Google Scholar] [CrossRef] [Green Version]
- DeGottardi, W.; Sen, D.; Vishveshwara, S. Majorana Fermions in Superconducting 1D Systems Having Periodic, Quasiperiodic, and Disordered Potentials. Phys. Rev. Lett. 2013, 110, 146404. [Google Scholar] [CrossRef] [Green Version]
- DeGottardi, W.; Thakurathi, M.; Vishveshwara, S.; Sen, D. Majorana fermions in superconducting wires: Effects of long-range hopping, broken time-reversal symmetry, and potential landscapes. Phys. Rev. B 2013, 88, 165111. [Google Scholar] [CrossRef] [Green Version]
- Yahyavi, M.; Hetényi, B.; Tanatar, B. Generalized Aubry-André-Harper model with modulated hopping and p-wave pairing. Phys. Rev. B 2019, 100, 064202. [Google Scholar] [CrossRef] [Green Version]
- Cai, X.; Lang, L.J.; Chen, S.; Wang, Y. Topological Superconductor to Anderson Localization Transition in One-Dimensional Incommensurate Lattices. Phys. Rev. Lett. 2013, 110, 176403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zeng, Q.B.; Shu, S.; Lü, R. Quench dynamics in the Aubry–André–Harper model with p-wave superconductivity. New. J. Phys. 2018, 20, 053012. [Google Scholar] [CrossRef]
- Tong, X.; Meng, Y.; Jiang, X.; Lee, C.; de Moraes Neto, G.D.; Xianlong, G. Dynamics of a quantum phase transition in the Aubry-André-Harper model with p-wave superconductivity. Phys. Rev. B 2021, 103, 104202. [Google Scholar] [CrossRef]
- Wang, J.; Liu, X.J.; Gao, X.; Hu, H. Phase diagram of a non-Abelian Aubry-André-Harper model with p-wave superfluidity. Phys. Rev. B 2016, 93, 104504. [Google Scholar] [CrossRef]
- Lang, L.J.; Chen, S. Majorana fermions in density-modulated p-wave superconducting wires. Phys. Rev. B 2012, 126, 205135. [Google Scholar] [CrossRef] [Green Version]
- Liu, T.; Yan, H.Y.; Guo, H. Fate of topological states and mobility edges in one-dimensional slowly varying incommensurate potentials. Phys. Rev. B 2017, 96, 174207. [Google Scholar] [CrossRef] [Green Version]
- Roati, G.; Errico, C.D.; Fallani, L.; Fattori, M.; Fort, C.; Zaccanti, M.; Modugno, G.; Modugno, M.; Inguscio, M. Anderson localization of a non-interacting Bose–Einstein condensate. Nature 2008, 453, 895. [Google Scholar] [CrossRef] [Green Version]
- Alex, A.F.; Padavić, K.; Meier, E.J.; Hegde, S.; Ganeshan, S.; Pixley, J.H.; Vishveshwara, S.; Gadway, B. Interactions and Mobility Edges: Observing the Generalized Aubry-André Model. Phys. Rev. Lett. 2021, 126, 040603. [Google Scholar] [CrossRef]
- Osterloh, K.; Baig, M.; Santos, L.; Zoller, P.; Lewenstein, M. Cold Atoms in Non-Abelian Gauge Potentials: From the Hofstadter “Moth” to Lattice Gauge Theory. Phys. Rev. Lett. 2005, 95, 010403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Goldman, N.; Kubasiak, A.; Gaspard, P.; Lewenstein, M. Ultracold atomic gases in non-Abelian gauge potentials: The case of constant Wilson loop. Phys. Rev. A 2009, 79, 023624. [Google Scholar] [CrossRef] [Green Version]
- Jiang, L.; Kitagawa, T.; Alicea, J.; Akhmerov, A.R.; Pekker, D.; Refael, G.; Cirac, J.I.; Demler, E.; Lukin, M.D.; Zoller, P. Majorana Fermions in Equilibrium and in Driven Cold-Atom Quantum Wires. Phys. Rev. Lett. 2011, 106, 220402. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Goldman, N.; Juzeliūnas, G.; Öhberg, P.; Spielman, I.B. Light-induced gauge fields for ultracold atoms. Rep. Prog. Phys. 2014, 77, 126401. [Google Scholar] [CrossRef]
- Liu, X.J.; Hu, H. Topological superfluid in one-dimensional spin-orbit-coupled atomic Fermi gases. Phys. Rev. A 2012, 85, 033622. [Google Scholar] [CrossRef]
- Aubry, S.; André, G. Analyticity Breaking and Anderson Localization in Incommensurate Lattices. Ann. Isr. Phys. Soc. 1980, 3, 18. Available online: https://www.researchgate.net/publication/265502988_Analyticity_breaking_and_Anderson_localization_in_incommensurate_lattices (accessed on 23 January 2022).
- Paige, C.; Loan, C.V. A Schur decomposition for Hamiltonian matrices. Linear Algeb. Its Appl. 1981, 41, 11. [Google Scholar] [CrossRef] [Green Version]
- Liu, T.; Cheng, S.; Guo, H.; Gao, X. Fate of Majorana zero modes, exact location of critical states, and unconventional real-complex transition in non-Hermitian quasiperiodic lattices. Phys. Rev. B 2021, 103, 104203. [Google Scholar] [CrossRef]
- Cheng, S.; Xianlong, G. Majorana zero modes, unconventional real–complex transition, and mobility edges in a one-dimensional non-Hermitian quasi-periodic lattice. Chin. Phys. B 2022, 31, 017401. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, S.; Zhu, Y.; Gao, X. Topological Superconducting Transition Characterized by a Modified Real-Space-Pfaffian Method and Mobility Edges in a One-Dimensional Quasiperiodic Lattice. Symmetry 2022, 14, 371. https://doi.org/10.3390/sym14020371
Cheng S, Zhu Y, Gao X. Topological Superconducting Transition Characterized by a Modified Real-Space-Pfaffian Method and Mobility Edges in a One-Dimensional Quasiperiodic Lattice. Symmetry. 2022; 14(2):371. https://doi.org/10.3390/sym14020371
Chicago/Turabian StyleCheng, Shujie, Yufei Zhu, and Xianlong Gao. 2022. "Topological Superconducting Transition Characterized by a Modified Real-Space-Pfaffian Method and Mobility Edges in a One-Dimensional Quasiperiodic Lattice" Symmetry 14, no. 2: 371. https://doi.org/10.3390/sym14020371
APA StyleCheng, S., Zhu, Y., & Gao, X. (2022). Topological Superconducting Transition Characterized by a Modified Real-Space-Pfaffian Method and Mobility Edges in a One-Dimensional Quasiperiodic Lattice. Symmetry, 14(2), 371. https://doi.org/10.3390/sym14020371






