A Peek Outside Our Universe
Abstract
:1. Introduction
1.1. deSitter Metric
1.2. The FLRW Metric and
1.3. SW–FLRW Perturbation
2. Outside Our FLRW Universe
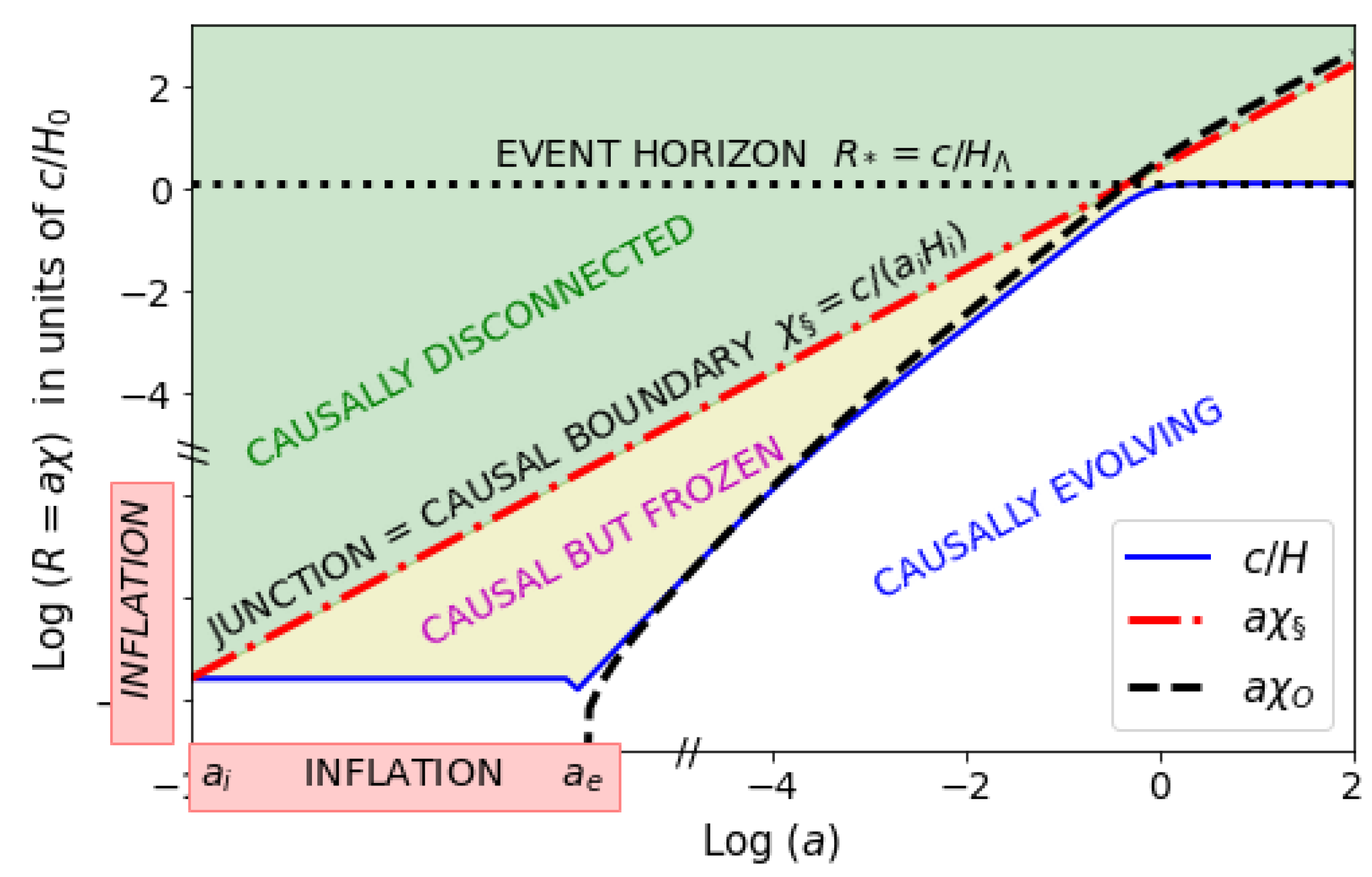
2.1. Causal Structure
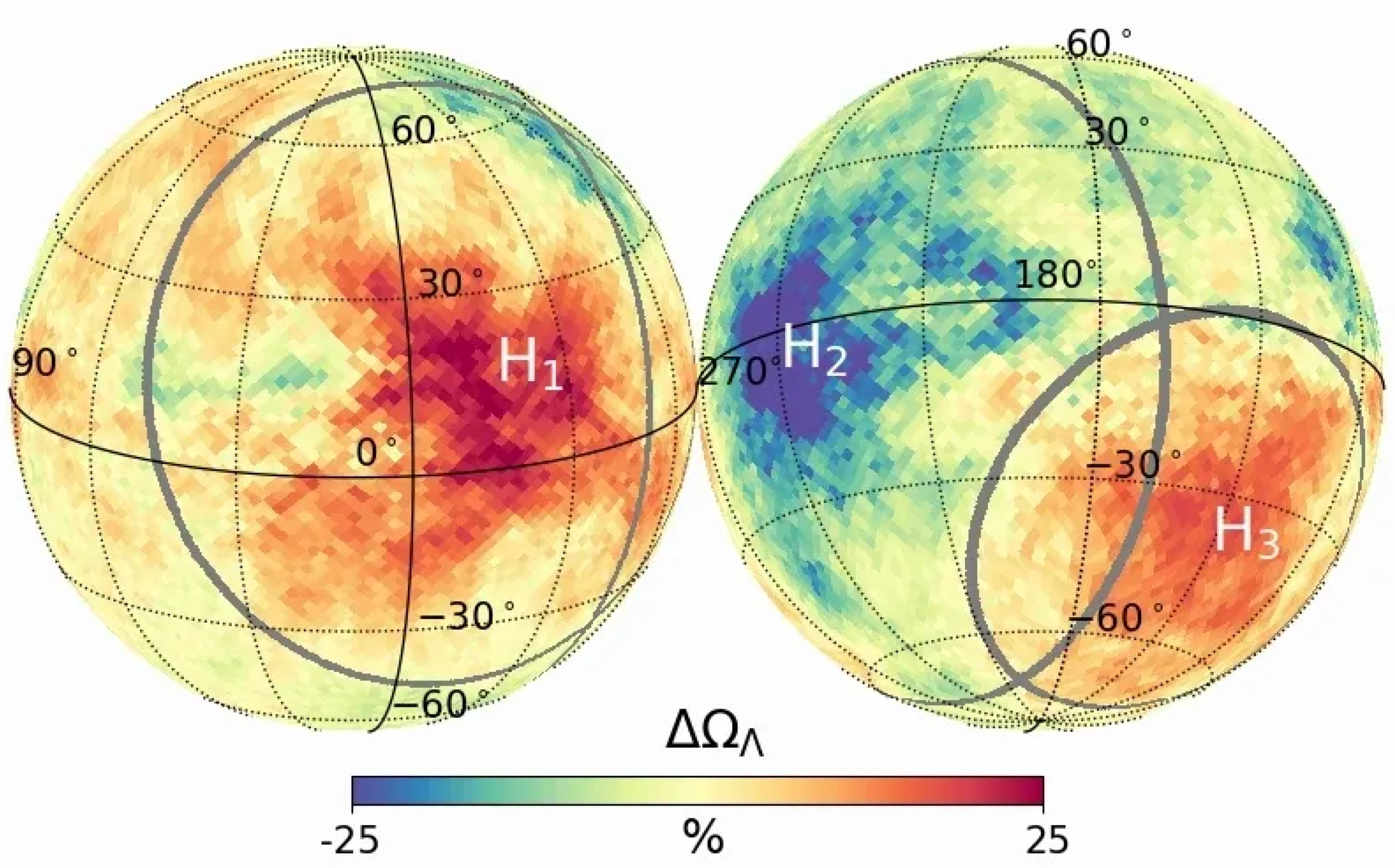
2.2. A Peek Outside
Author Contributions
Funding
Conflicts of Interest
References
- Padmanabhan, T. Gravitation: Foundations and Frontiers; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Penrose, R. Gravitational Collapse: The Role of General Relativity. Nuovo Cimento Rivista Serie 1969, 1, 252. [Google Scholar]
- Mitra, A. Interpretational conflicts between the static and non-static forms of de Sitter metric. Sci. Rep. 2012, 2, 923. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gaztanaga, E.D. The Black Hole Universe (BHU) from a FLRW Cloud. Submitted to Physics of the Dark Universe. Available online: https://hal.archives-ouvertes.fr/hal-03344159 (accessed on 14 September 2021).
- O’Raifeartaigh, C.; Mitton, S. A new perspective on steady-state cosmology. arXiv 2015, arXiv:1506.01651. [Google Scholar]
- Gaztañaga, E. The cosmological constant as a zero action boundary. Mon. Not. R. Astron. Soc. 2021, 502, 436–444. [Google Scholar] [CrossRef]
- Gaztanaga, E. The size of our causal Universe. Mon. Not. R. Astron. Soc. 2020, 494, 2766–2772. [Google Scholar] [CrossRef] [Green Version]
- Kaloper, N.; Kleban, M.; Martin, D. McVittie’s legacy: Black holes in an expanding universe. Phys. Rev. D 2010, 81, 104044. [Google Scholar] [CrossRef] [Green Version]
- Israel, W. Singular hypersurfaces and thin shells in general relativity. Nuovo Cimento B Serie 1967, 48, 463. [Google Scholar] [CrossRef] [Green Version]
- Stuckey, W.M. The observable universe inside a black hole. Am. J. Phys. 1994, 62, 788–795. [Google Scholar] [CrossRef]
- Starobinskiǐ, A.A. Spectrum of relict gravitational radiation and the early state of the universe. Sov. Exp. Theor. Phys. Lett. 1979, 30, 682. [Google Scholar]
- Guth, A.H. Inflationary universe: A possible solution to the horizon and flatness problems. Phys. Rev. D 1981, 23, 347–356. [Google Scholar] [CrossRef] [Green Version]
- Linde, A.D. A new inflationary universe scenario: A possible solution of the horizon, flatness, homogeneity, isotropy and primordial monopole problems. Phys. Lett. B 1982, 108, 389–393. [Google Scholar] [CrossRef]
- Albrecht, A.; Steinhardt, P.J. Cosmology for Grand Unified Theories with Radiatively Induced Symmetry Breaking. Phys. Rev. Lett. 1982, 48, 1220–1223. [Google Scholar] [CrossRef]
- Planck Collaboration. Planck 2018 results. VII. Isotropy and statistics of the CMB. Astron. Astrophys. 2020, 641, A7. [Google Scholar] [CrossRef] [Green Version]
- Schwarz, D.J.; Copi, C.J.; Huterer, D.; Starkman, G.D. CMB anomalies after Planck. Class. Quantum Gravity 2016, 33, 184001. [Google Scholar] [CrossRef]
- Planck Collaboration. Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6. [Google Scholar] [CrossRef] [Green Version]
- Riess, A.G. The expansion of the Universe is faster than expected. Nat. Rev. Phys. 2019, 2, 10–12. [Google Scholar] [CrossRef] [Green Version]
- DES Collaboration. Cosmological Constraints from Multiple Probes in the Dark Energy Survey. Phys. Rev. Lett. 2019, 122, 171301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Di Valentino, E.; Mena, O.; Pan, S.; Visinelli, L.; Yang, W.; Melchiorri, A.; Mota, D.F.; Riess, A.G.; Silk, J. In the realm of the Hubble tension-a review of solutions. Class. Quantum Gravity 2021, 38, 153001. [Google Scholar] [CrossRef]
- Fosalba, P.; Gaztañaga, E. Explaining cosmological anisotropy: Evidence for causal horizons from CMB data. Mon. Not. R. Astron. Soc. 2021, 504, 5840–5862. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gaztanaga, E.; Fosalba, P. A Peek Outside Our Universe. Symmetry 2022, 14, 285. https://doi.org/10.3390/sym14020285
Gaztanaga E, Fosalba P. A Peek Outside Our Universe. Symmetry. 2022; 14(2):285. https://doi.org/10.3390/sym14020285
Chicago/Turabian StyleGaztanaga, Enrique, and Pablo Fosalba. 2022. "A Peek Outside Our Universe" Symmetry 14, no. 2: 285. https://doi.org/10.3390/sym14020285
APA StyleGaztanaga, E., & Fosalba, P. (2022). A Peek Outside Our Universe. Symmetry, 14(2), 285. https://doi.org/10.3390/sym14020285






