Discrete Memristance and Nonlinear Term for Designing Memristive Maps
Abstract
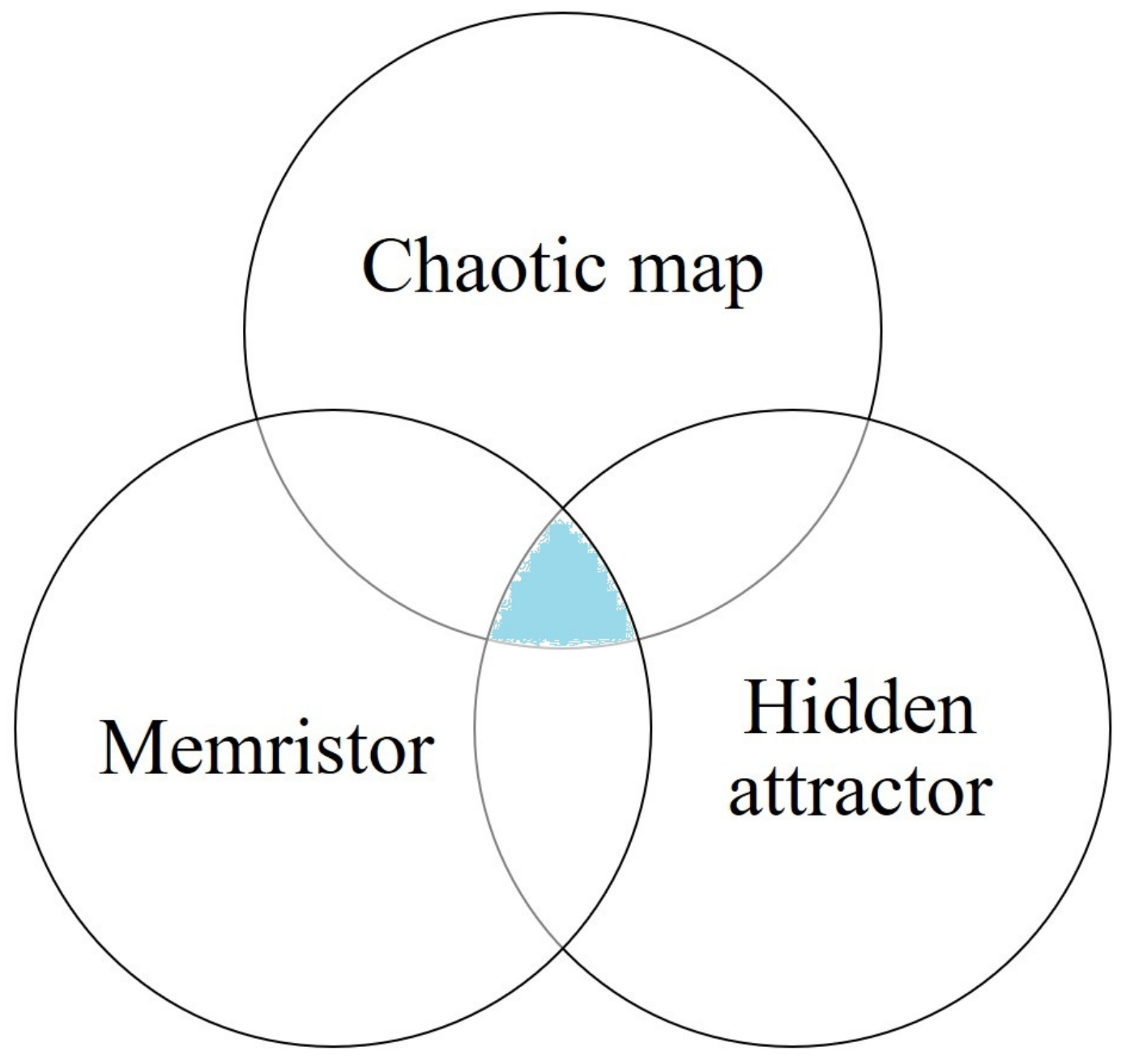
1. Introduction
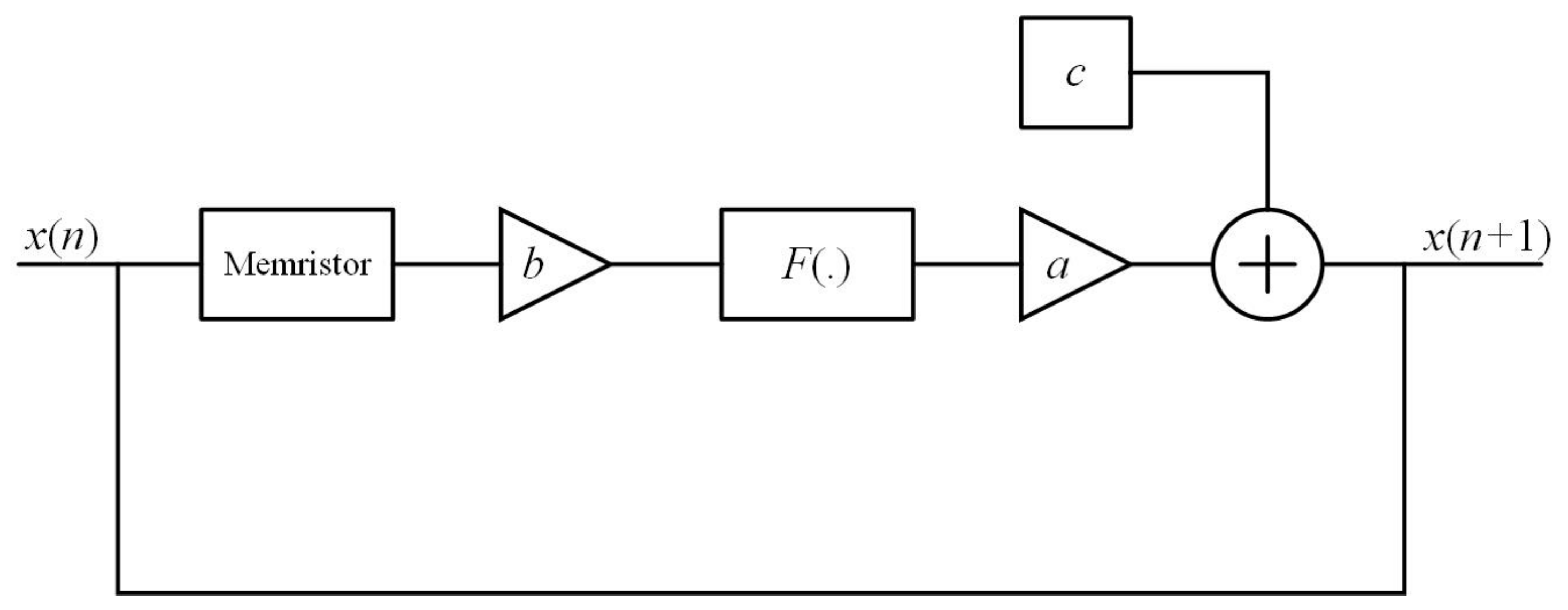
2. General Model
3. MM Map
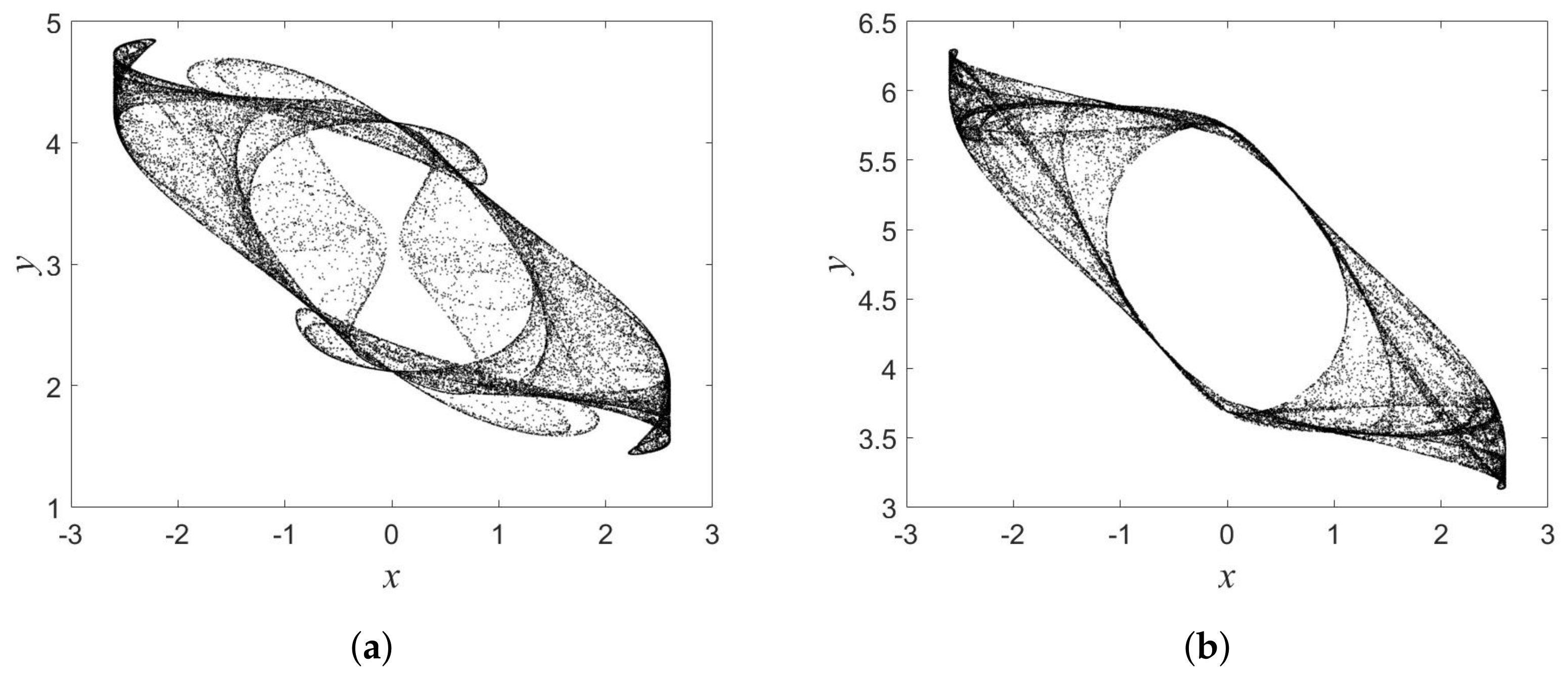
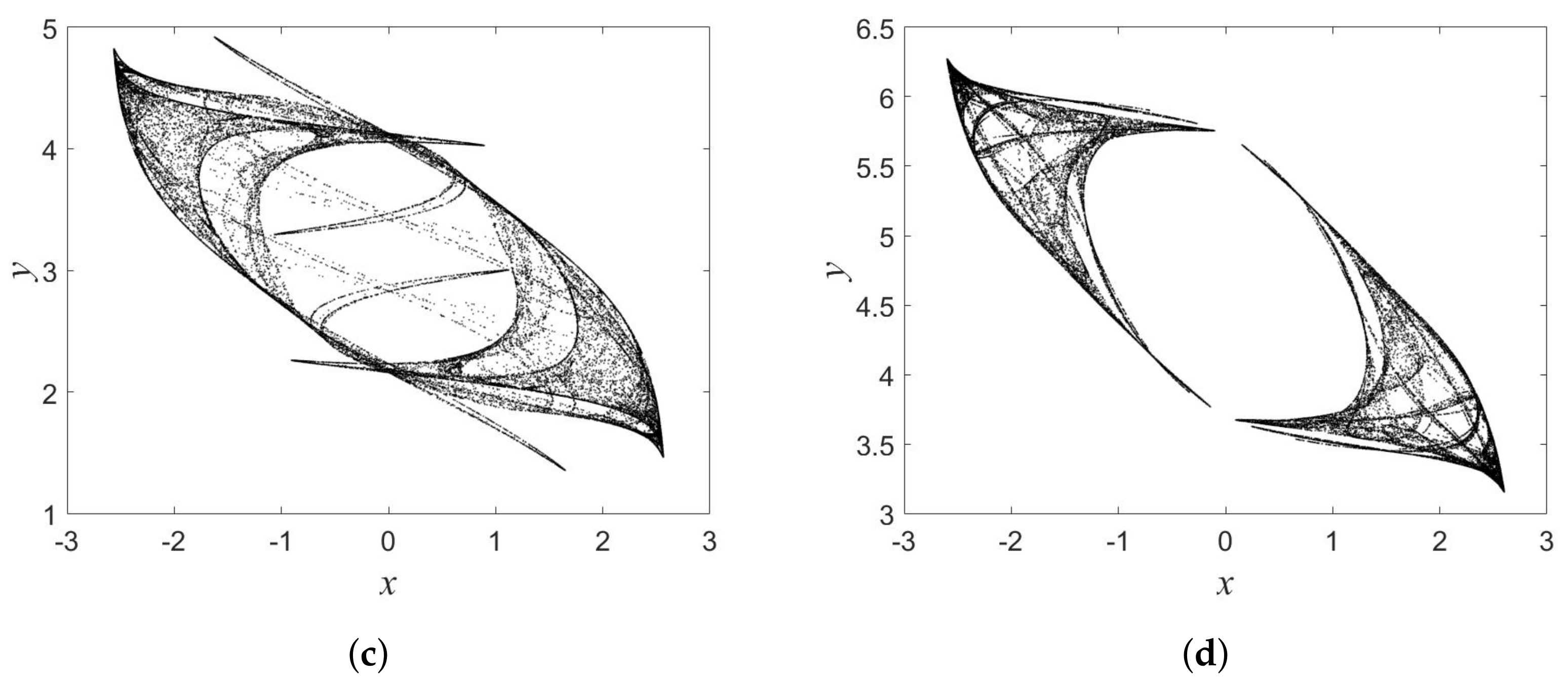
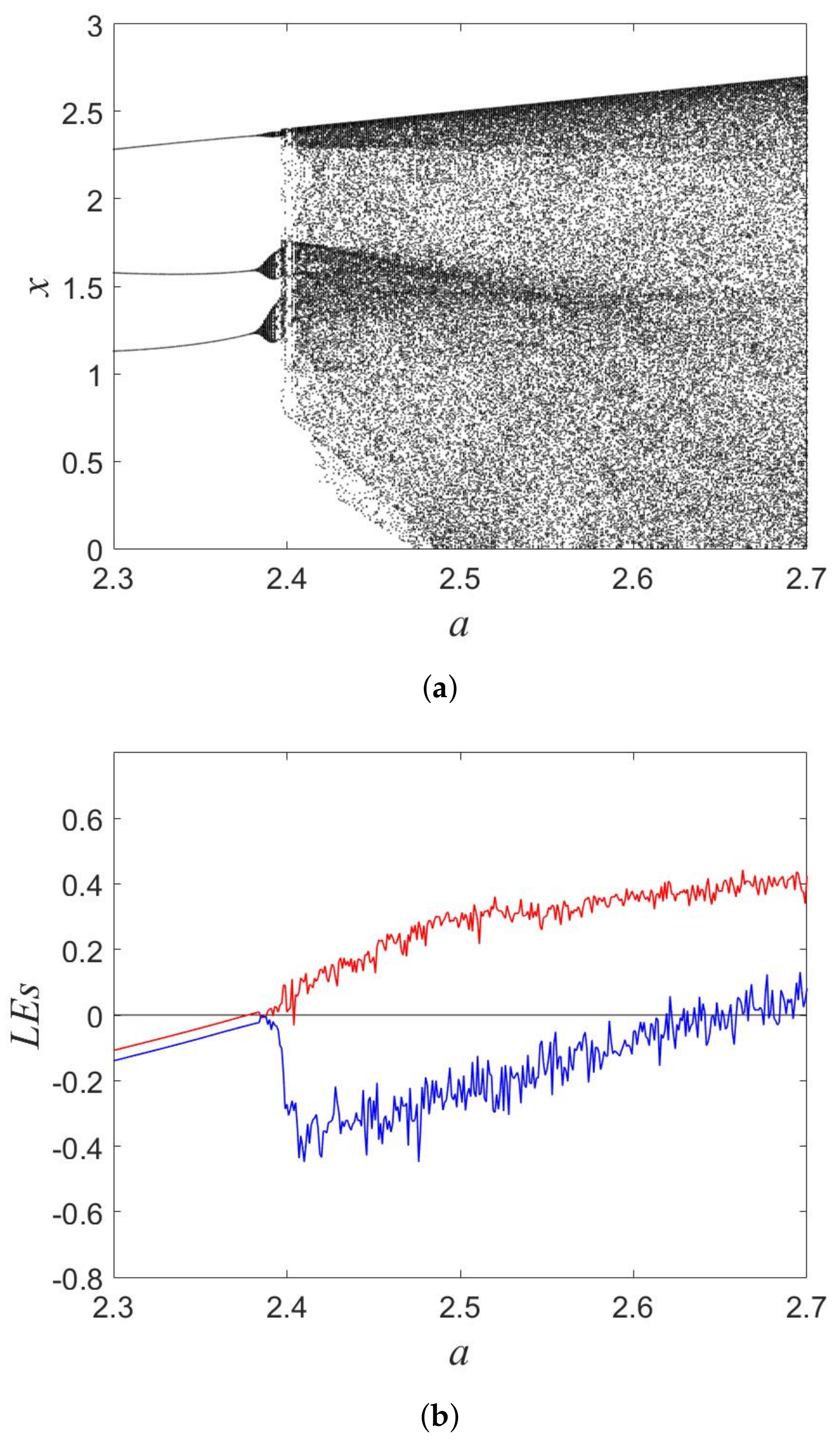
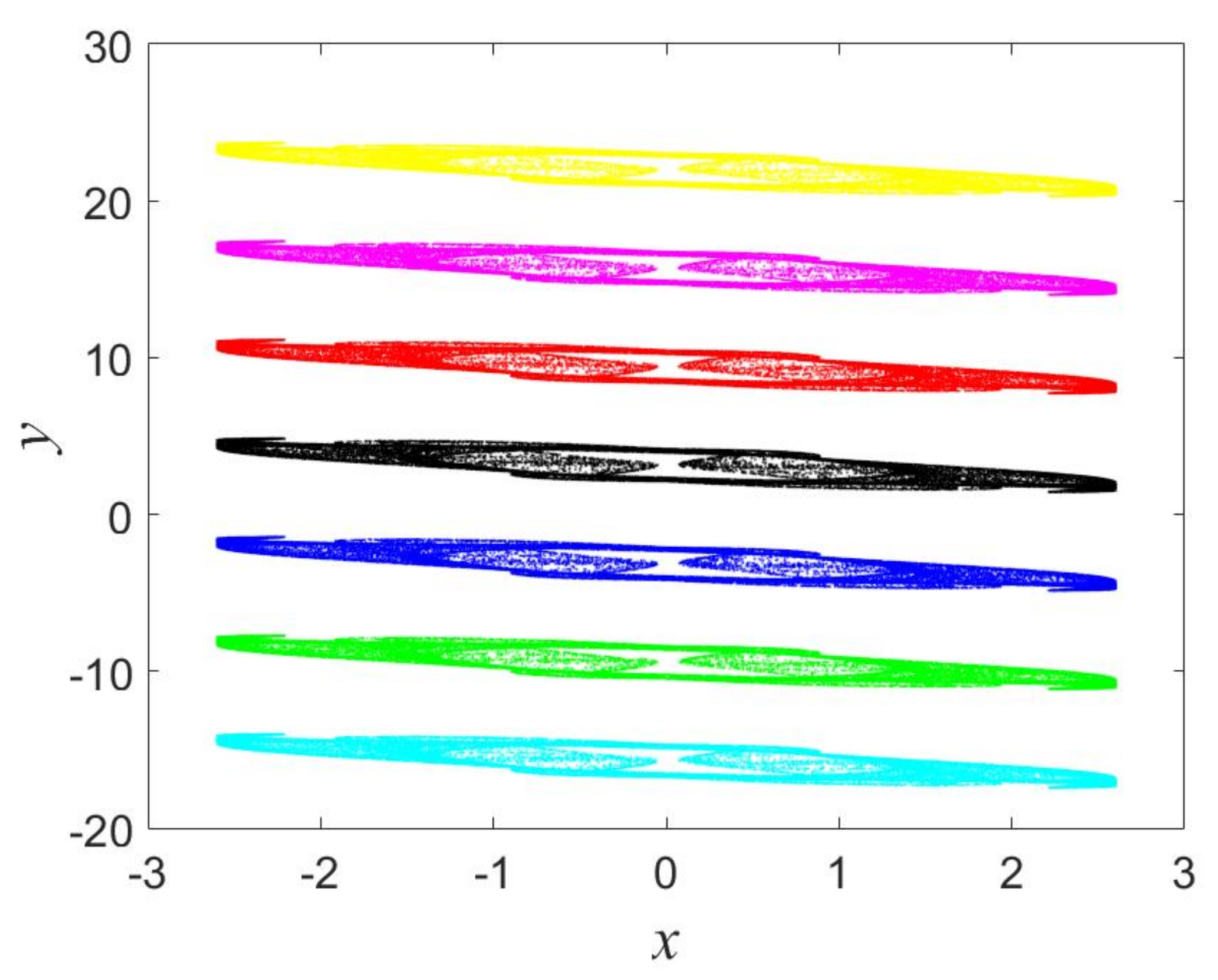
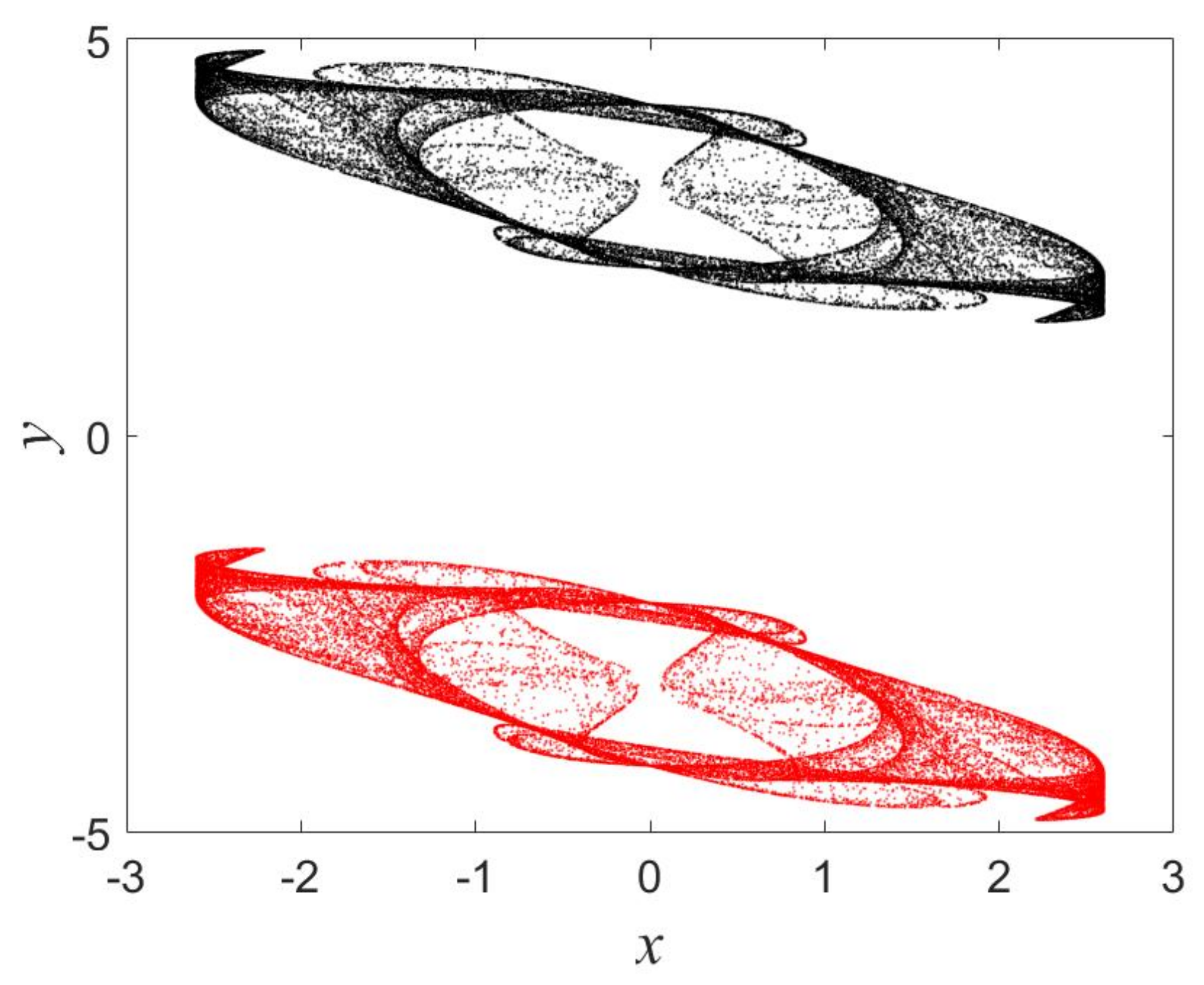
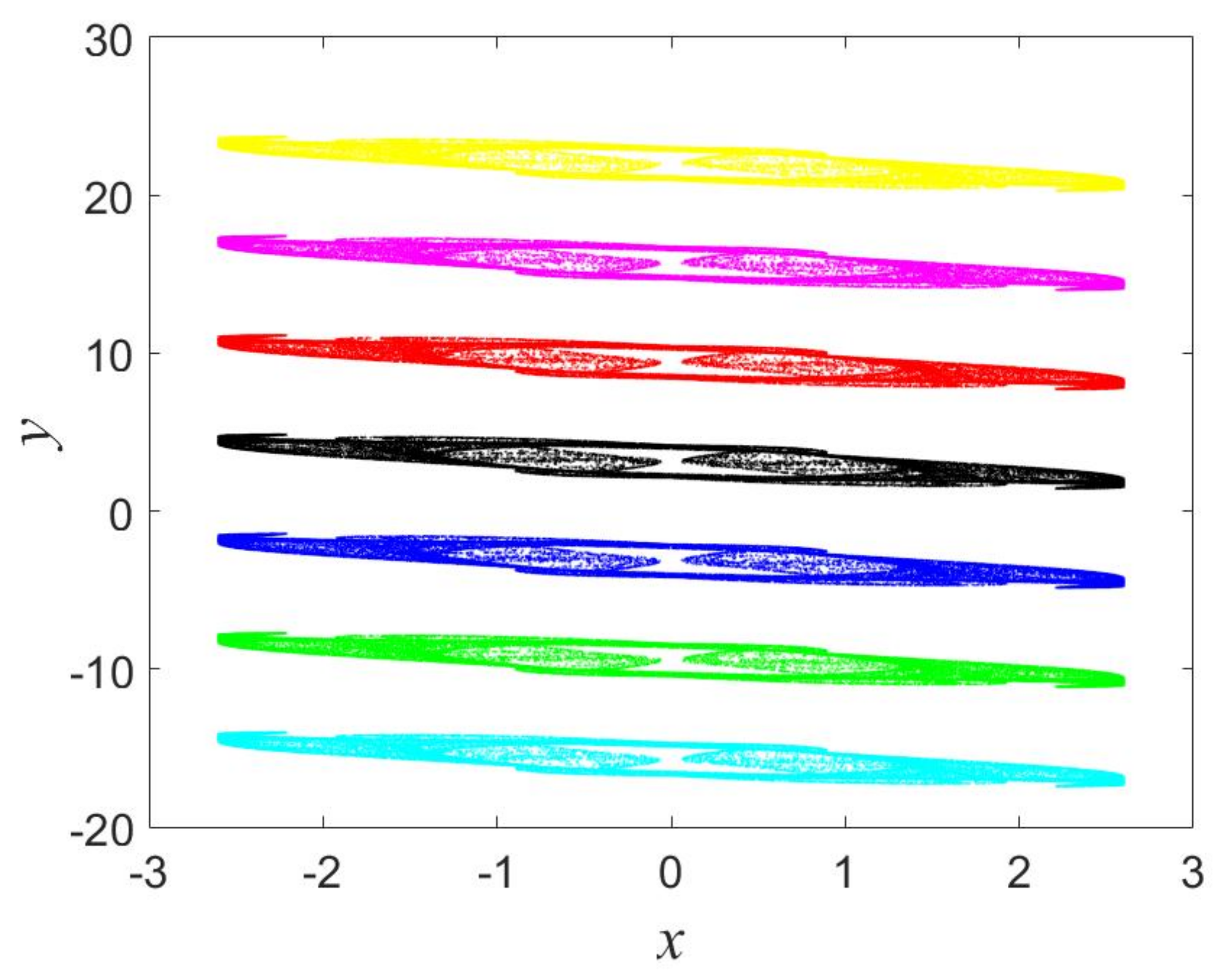
3.1. Case 1 When
3.2. Case 2 When
3.3. Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yan, X.; Wang, X.; Xian, Y. Synchronously scrambled diffuse image encryption method based on a new cosine chaotic map. Chin. Phys. B 2022, 31, 080504. [Google Scholar] [CrossRef]
- Bao, B.C.; Bao, H.; Wang, N.; Chen, M.; Xu, Q. Hidden extreme multistability in memristive hyperchaotic system. Chaos Solitons Fractals 2017, 94, 102–111. [Google Scholar] [CrossRef]
- Wang, Z.; Ma, J.; Cang, S.; Wang, Z.; Chen, Z. Simplified hyper–chaotic systems generating multi–wing non–equilibrium attractors. Neurocomputing 2016, 127, 2424–2431. [Google Scholar] [CrossRef]
- Rajagopal, K.; Karthikeyan, A.; Srinivasan, A.K. FPGA implementation of novel fractional–order chaotic systems with two equilibriums and no equilibrium and its adaptive sliding mode synchronization. Nonlinear Dyn. 2017, 87, 2281–2304. [Google Scholar] [CrossRef]
- Pierre, C.; Jean-Pierre, E. Iterated Map on the Interval as Dynamical Systems; Springer: Berlin/Heidelberg, Germany, 1980. [Google Scholar]
- Elaydi, S.N. Discrete Chaos: With Applications in Science and Engineering, 2nd ed.; Chapman and Hall/CRC: Boca Raton, FL, USA, 2007. [Google Scholar]
- Hénon, M.A. A two-dimensional mapping with a strange attractor. Commun. Math. Phys. 1976, 50, 69–77. [Google Scholar] [CrossRef]
- Gibson, W.T.; Wilson, W.C. Individual-based chaos: Extensions of the discrete logistic model. J. Theory Biol. 2013, 339, 84–92. [Google Scholar] [CrossRef]
- Wei, C.; Li, G.; Xu, X. Design of a new dimension-changeable hyperchaotic model based on discrete memristor. Symmetry 2022, 14, 1019. [Google Scholar] [CrossRef]
- Dai, W.; Xu, X.; Song, X.; Li, G. Audio encryption algorithm based on Chen memristor chaotic system. Symmetry 2022, 14, 17. [Google Scholar] [CrossRef]
- Lambic, D. A novel method of S-box design based on chaotic map and composition method. Chaos Solitons Fractals 2014, 58, 16–21. [Google Scholar] [CrossRef]
- Valtierra, J.L.; Tlelo-Cuautle, E.; Rodriguez-Vazquez, A. A switched-capacitor skew-tent map implementation for random number generation. Int. J. Circ. Theory Appl. 2017, 45, 305–315. [Google Scholar] [CrossRef]
- Acho, L. A discrete-time chaotic oscillator based on the logistic map: A secure communication scheme and a simple experiment using Arduino. J. Frankl. Inst. 2015, 352, 3113–3121. [Google Scholar] [CrossRef]
- de la Fraga, L.G.; Torres-Perez, E.; Tlelo-Cuautle, E.; Mancillas-Lopez, C. Hardware implementation of pseudo-random number generators based on chaotic maps. Nonlinear Dyn. 2017, 90, 1661–1670. [Google Scholar] [CrossRef]
- Borujeni, S.; Ehsani, M. Modified logistic maps for cryptographic application. Appl. Math. 2015, 6, 773–782. [Google Scholar] [CrossRef]
- Lu, Q.; Yu, L.; Zhu, C. Symmetric image encryption algorithm based on a new product trigonometric chaotic map. Symmetry 2022, 14, 373. [Google Scholar] [CrossRef]
- Bao, H.; Hua, Z.; Li, H.; Chen, M.; Bao, B. Memristor-based hyperchaotic maps and application in auxiliary classifier generative adversarial nets. IEEE Trans. Ind. Inform. 2022, 18, 5297–5306. [Google Scholar] [CrossRef]
- Buscarino, A.; Fortuna, L.; Frasca, M.; Gambuzza, L.V. A chaotic circuit based on Hewlett–Packard memristor. Chaos 2012, 22, 023136. [Google Scholar] [CrossRef]
- Li, Q.; Hu, S.; Tang, S.; Zeng, G. Hyperchaos and horseshoe in a 4D memristive system with a line of equilibria and its implementation. Int. J. Cir. Theory Appl. 2014, 42, 1172–1188. [Google Scholar] [CrossRef]
- Bao, H.; Wang, N.; Bao, B.; Chen, M.; Jin, P.; Wang, G. Initial condition-dependent dynamics and transient period in memristor–based hypogenetic jerk system with four line equilibria. Commun. Nonlinear Sci. Numer. Simul. 2018, 57, 264–275. [Google Scholar] [CrossRef]
- Xu, Q.; Ding, S.; Bao, H.; Chen, M.; Bao, B. Piecewise-linear simplification for adaptive synaptic neuron model. IEEE Trans. Circ. Syst. II Express Briefs 2022, 69, 1832–1836. [Google Scholar] [CrossRef]
- Wu, X.; He, S.; Tan, W.; Wang, H. From memristor-modeled jerk system to the nonlinear systems with memristor. Symmetry 2022, 14, 659. [Google Scholar] [CrossRef]
- Yang, B.; Wang, Z.; Tian, H.; Liu, J. Symplectic Dynamics and Simultaneous Resonance Analysis of Memristor Circuit Based on Its van der Pol Oscillator. Symmetry 2022, 14, 1215. [Google Scholar] [CrossRef]
- Xu, Q.; Cheng, S.; Ju, Z.; Chen, M.; Wu, H. Asymmetric coexisting bifurcations and multi-stability in an asymmetric memristive diode-bridge-based jerk circuit. Chin. J. Phys. 2021, 70, 69–81. [Google Scholar] [CrossRef]
- Bao, H.; Li, H.; Hua, Z.; Xu, Q.; Bao, B. Sine-transform-based memristive hyperchaotic model with hardware implementation. IEEE Trans. Ind. Inform. 2022, 1. [Google Scholar] [CrossRef]
- Bao, H.; Gu, Y.; Xu, Q.; Zhang, X.; Bao, B. Parallel bi-memristor hyperchaotic map with extreme multistability. Chaos Solitons Fractals 2022, 160, 112273. [Google Scholar] [CrossRef]
- Sprott, J.C. Elegant Chaos Algebraically Simple Chaotic Flows; World Scientific: Singapore, 2010. [Google Scholar]
- Bouali, S.; Buscarino, A.; Fortuna, L.; Frasca, M.; Gambuzza, L.V. Emulating complex business cycles by using an electronic analogue. Nonlinear Anal. Real World Appl. 2012, 13, 2459–2465. [Google Scholar] [CrossRef]
- Li, C.; Li, H.; Tong, Y. Analysis of a novel three–dimensional chaotic system. Optik 2013, 124, 1516–1522. [Google Scholar] [CrossRef]
- Lai, Q.; Yang, L. Chaos, bifurcation, coexisting attractors and circuit design of a three–dimensional continuous autonomous system. Optik 2016, 127, 5400–5406. [Google Scholar] [CrossRef]
- Yu, F.; Li, P.; Bo, K.; Yin, B. Research progess of multi–scroll chaotic oscillators based on current–mode devices. Optik 2016, 127, 5486–5490. [Google Scholar] [CrossRef]
- Akgul, A.; Moroz, I.; Pehlivan, I.; Vaidyanathan, S. A new four–scroll chaotic attractor and its engineering applications. Optik 2016, 127, 5491–5499. [Google Scholar] [CrossRef]
- Dudkowski, D.; Jafari, S.; Kapitaniak, T.; Kuznetsov, N.; Leonov, G.; Prasad, A. Hidden attractors in dynamical systems. Phys. Rep. 2016, 637, 1–50. [Google Scholar] [CrossRef]
- Dadras, S.; Momeni, H.R.; Qi, G.; Wang, Z. Four–wing hyperchaotic attractor generated from a new 4D system with one equilibrium and its fractional–order form. Nonlinear Dyn. 2012, 67, 1161–1173. [Google Scholar] [CrossRef]
- Wang, Z.; Cang, S.; Ochala, E.; Sun, Y. A hyperchaotic system without equilibrium. Nonlinear Dyn. 2012, 69, 531–537. [Google Scholar] [CrossRef]
- Jafari, S.; Sprott, J.C.; Golpayegani, S.M.R.H. Elementary quadratic chaotic flows with no equilibria. Phys. Lett. A 2013, 377, 699–702. [Google Scholar] [CrossRef]
- Pham, V.T.; Rahma, F.; Frasca, M.; Fortuna, L. Dynamics and synchronization of a novel hyperchaotic system without equilibrium. Int. J. Bifurc. Chaos 2014, 24, 1450087. [Google Scholar] [CrossRef]
- Kapitaniak, T.; Leonov, G.A. Multistability: Uncovering hidden attractors. Eur. Phys. J. Spec. Top. 2015, 224, 1405–1408. [Google Scholar] [CrossRef]
- Zhusubaliyev, Z.T.; Mosekilde, E. Multistability and hidden attractors in a multilevel DC/DC converter. Math. Comput. Simul. 2015, 109, 32–45. [Google Scholar] [CrossRef]
- Jafari, S.; Pham, V.T.; Moghtadaei, M.; Kingni, S.T. The relationship between chaotic maps and some chaotic systems with hidden attractors. Int. J. Bifurc. Chaos 2016, 26, 1650211. [Google Scholar] [CrossRef]
- Castaneda, C.E.; Lopez-Mancilla, D.; Chiu, R.; Villafana-Rauda, E.; Orozco-Lopez, O.; Casillas-Rodriguez, F.; Sevilla-Escoboza, R. Discrete-time neural synchronization between an Arduino microcontroller and a Compact Development System using multiscroll chaotic signals. Chaos Solitons Fractals 2019, 119, 269–275. [Google Scholar] [CrossRef]
| Map | Equations | Parameters | |
|---|---|---|---|
| MM | |||
| MM | |||
| MM | |||
| MM | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ramadoss, J.; Almatroud, O.A.; Momani, S.; Pham, V.-T.; Thoai, V.P. Discrete Memristance and Nonlinear Term for Designing Memristive Maps. Symmetry 2022, 14, 2110. https://doi.org/10.3390/sym14102110
Ramadoss J, Almatroud OA, Momani S, Pham V-T, Thoai VP. Discrete Memristance and Nonlinear Term for Designing Memristive Maps. Symmetry. 2022; 14(10):2110. https://doi.org/10.3390/sym14102110
Chicago/Turabian StyleRamadoss, Janarthanan, Othman Abdullah Almatroud, Shaher Momani, Viet-Thanh Pham, and Vo Phu Thoai. 2022. "Discrete Memristance and Nonlinear Term for Designing Memristive Maps" Symmetry 14, no. 10: 2110. https://doi.org/10.3390/sym14102110
APA StyleRamadoss, J., Almatroud, O. A., Momani, S., Pham, V.-T., & Thoai, V. P. (2022). Discrete Memristance and Nonlinear Term for Designing Memristive Maps. Symmetry, 14(10), 2110. https://doi.org/10.3390/sym14102110







