Asymmetrical Damage Aspects Based Investigations on the Disc Brake of Long-Range UAVs through Verified Computational Coupled Approaches
Abstract
1. Introduction
1.1. Literature Survey
1.2. Related Inferred Terminologies
1.3. Contributions and Organizations
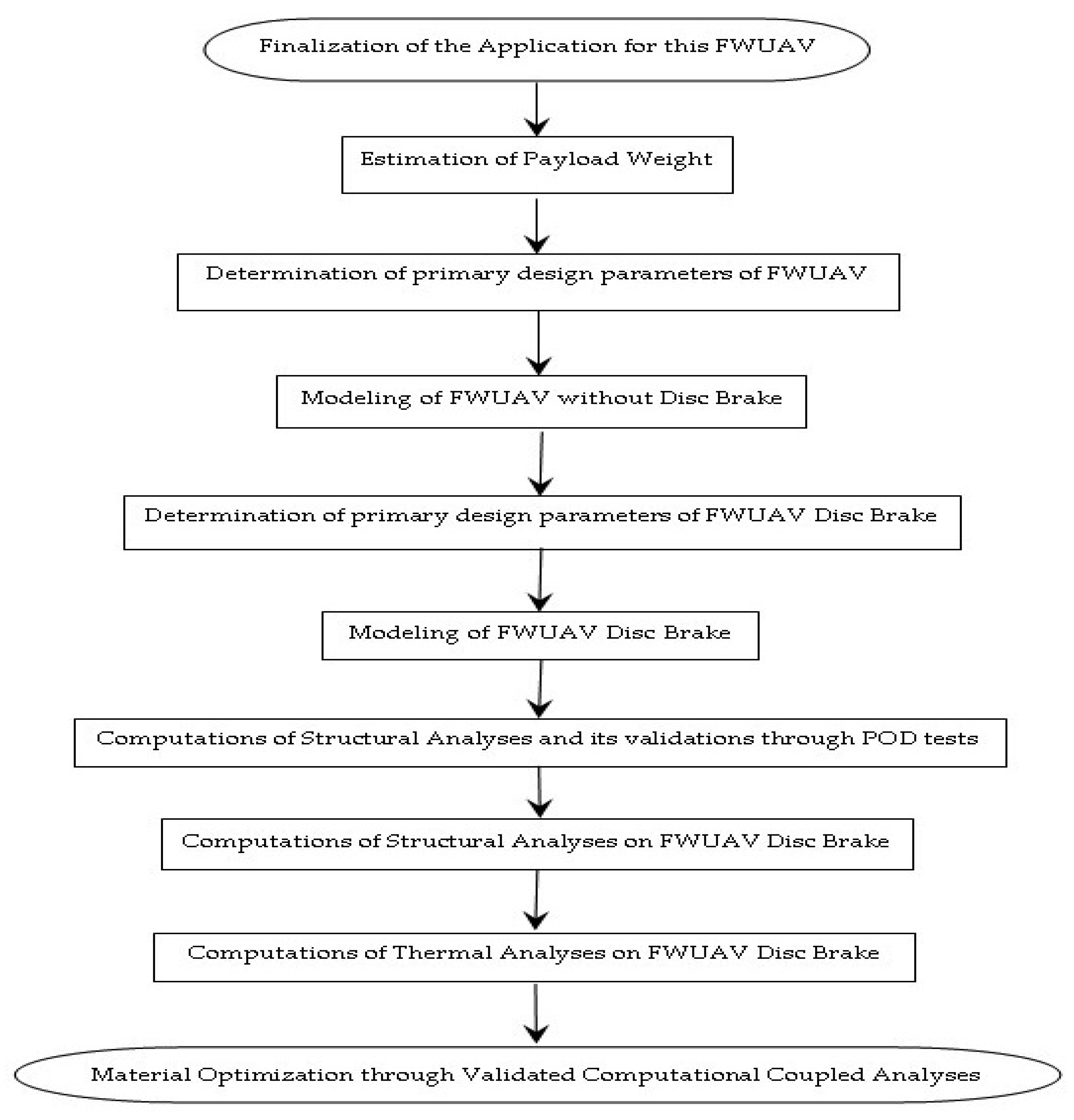
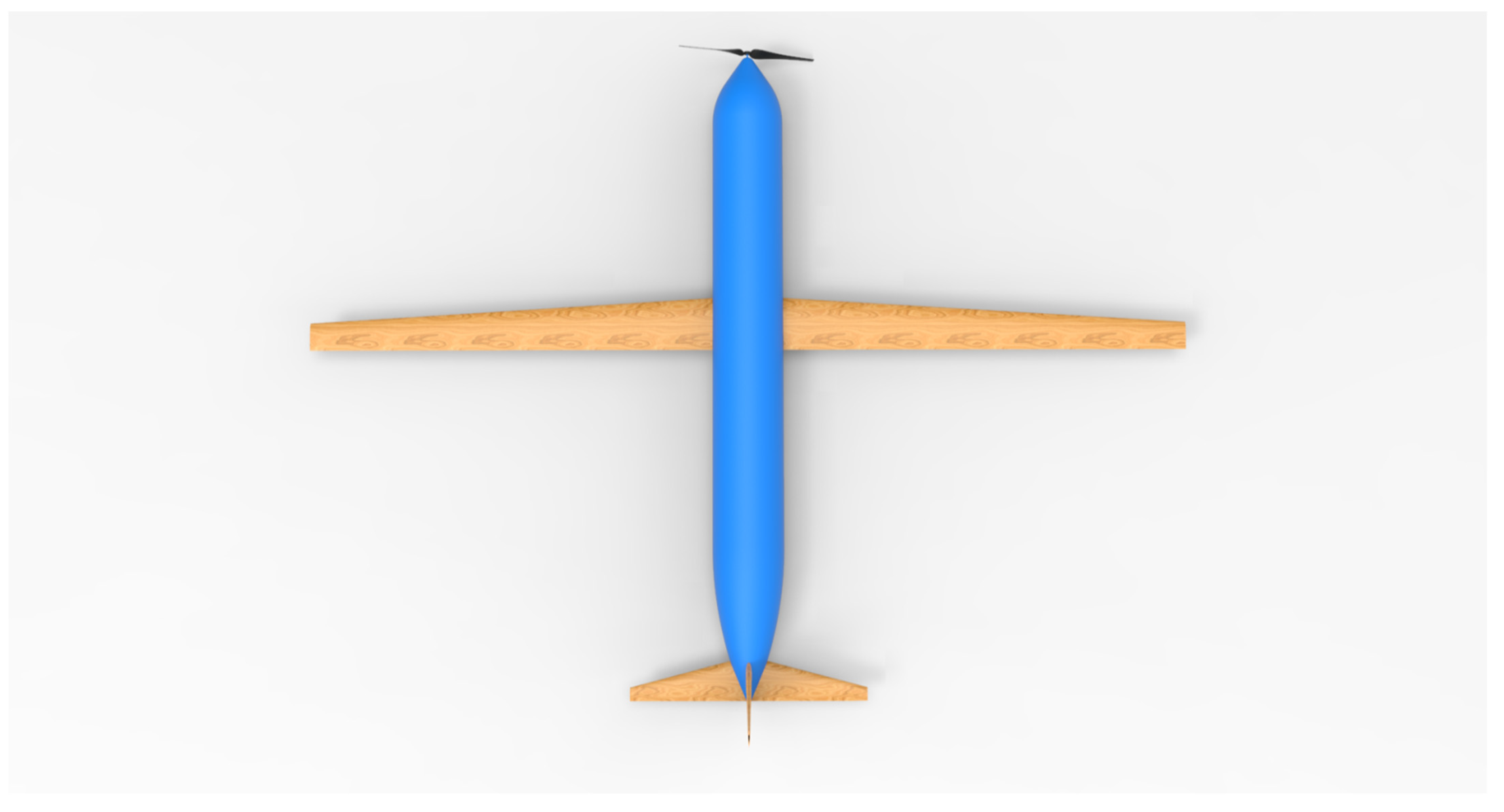
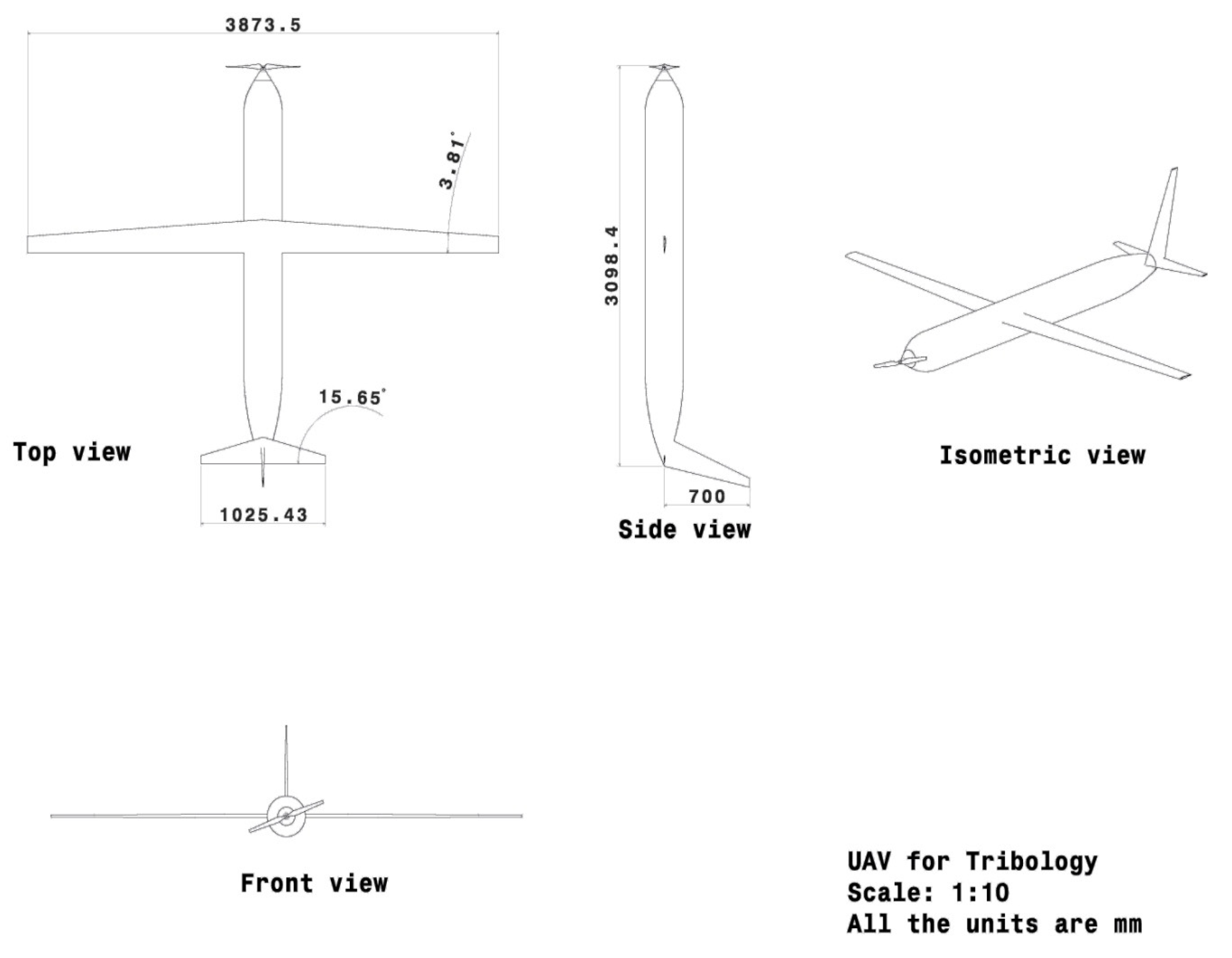
2. Proposed Design of UAV and Its Wheel Disc Brake
2.1. Estimation of Wing Surface Area, Wingspan, Chord Length, and Fuselage Length
2.2. Empennage Design—Horizontal Tail
2.3. Empennage Design—Vertical Tail
2.4. Estimation of Propulsive System and Its Weight
2.4.1. Estimation of Co-Efficient of Lift (CL)
2.4.2. Estimation of Power, Propeller’s Pitch and its RPM
2.4.3. Estimation of Pitch angle and Chord of the Propeller
2.5. Aerofoil Selection
2.6. Weight Estimation
Estimation of Electrical and Electronics System and Its Weight
2.7. Estimation of Stick of Landing Gear and Its Weight (Assymetrical Component)
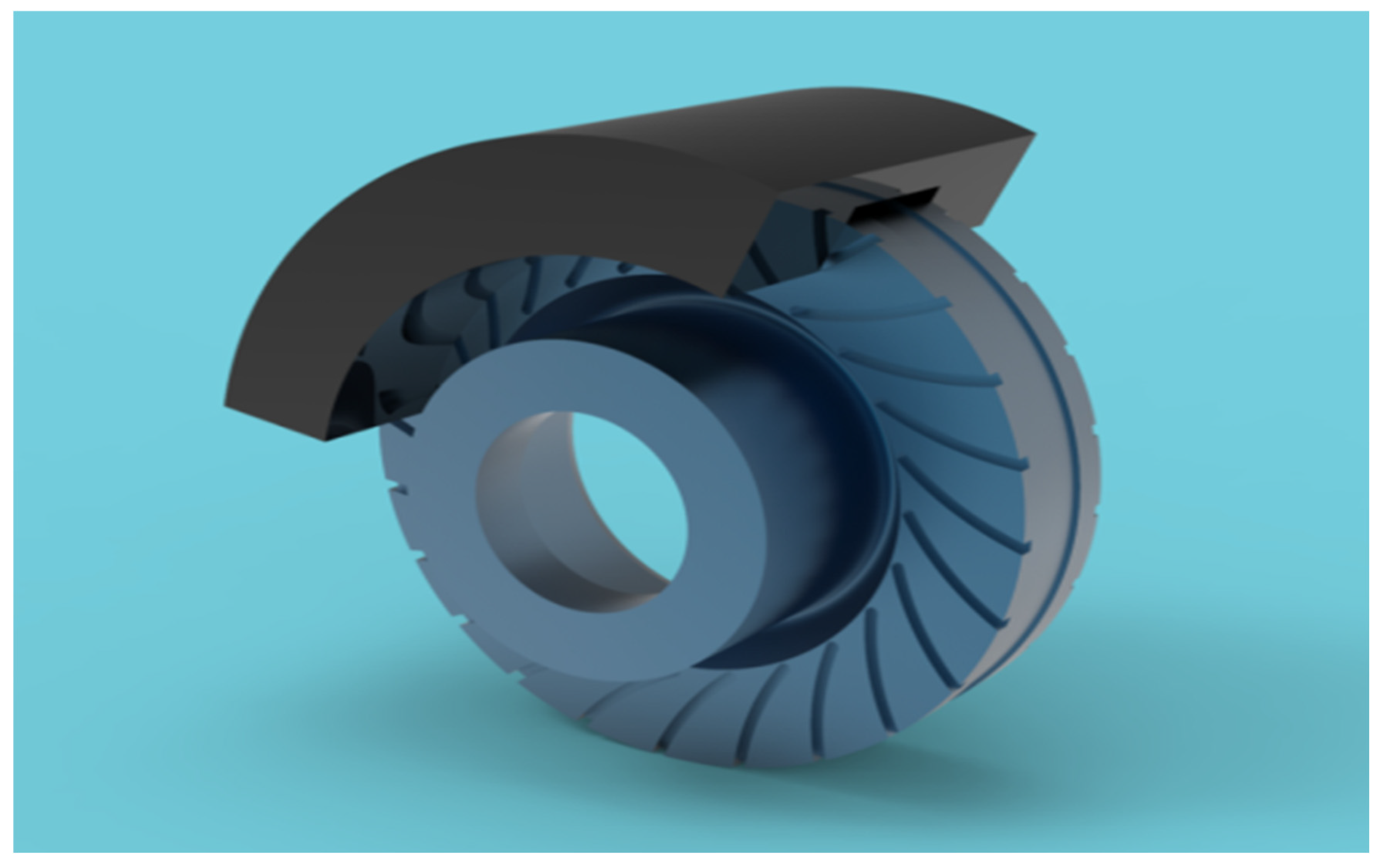
2.8. Conceptual Design UAV’s Disc Brake (Assymetrical Component)
3. Proposed Methodology and Its Validations
3.1. Computational Model (Symmetrical Study)
3.2. Boundary Conditions and Description about Analyses
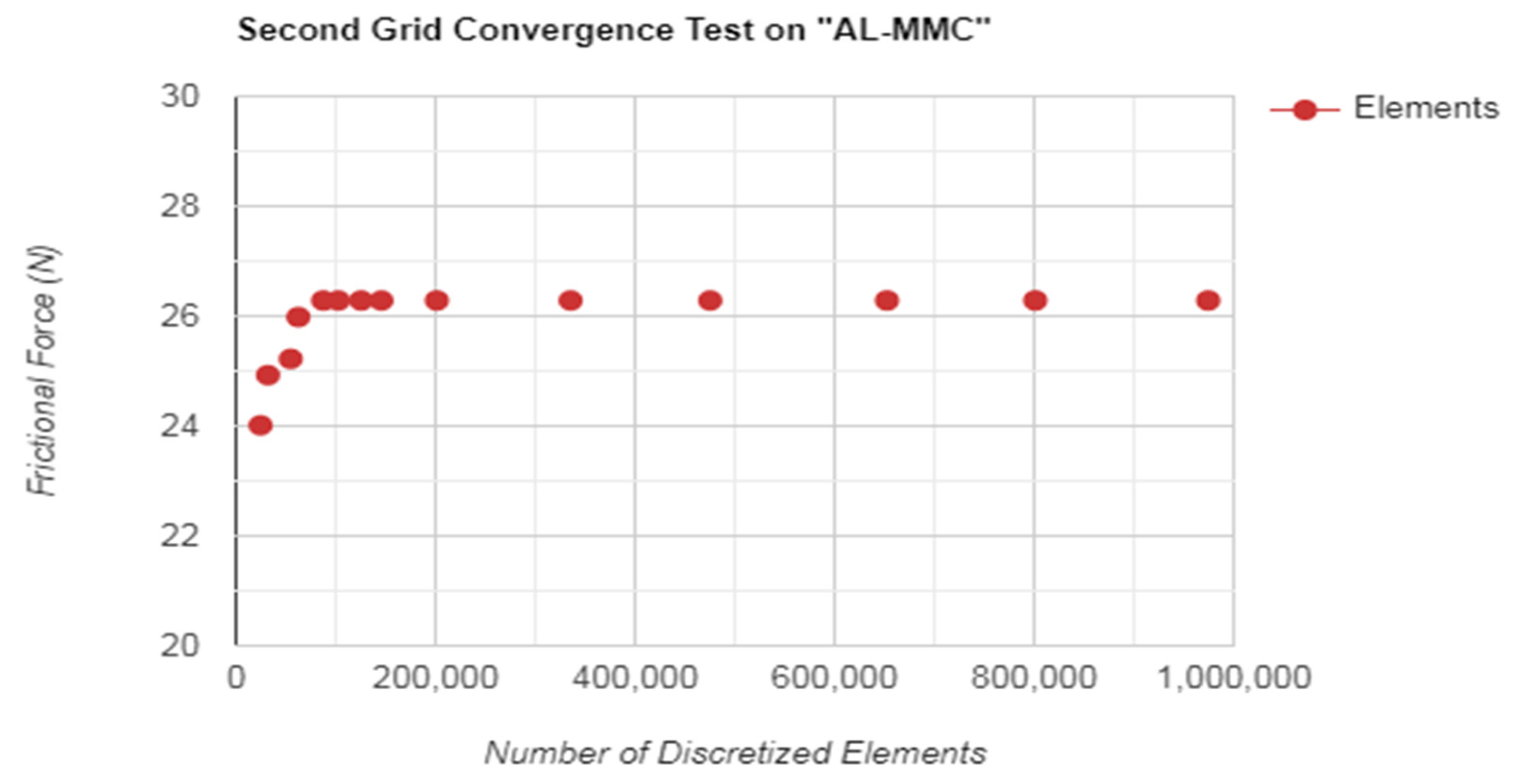
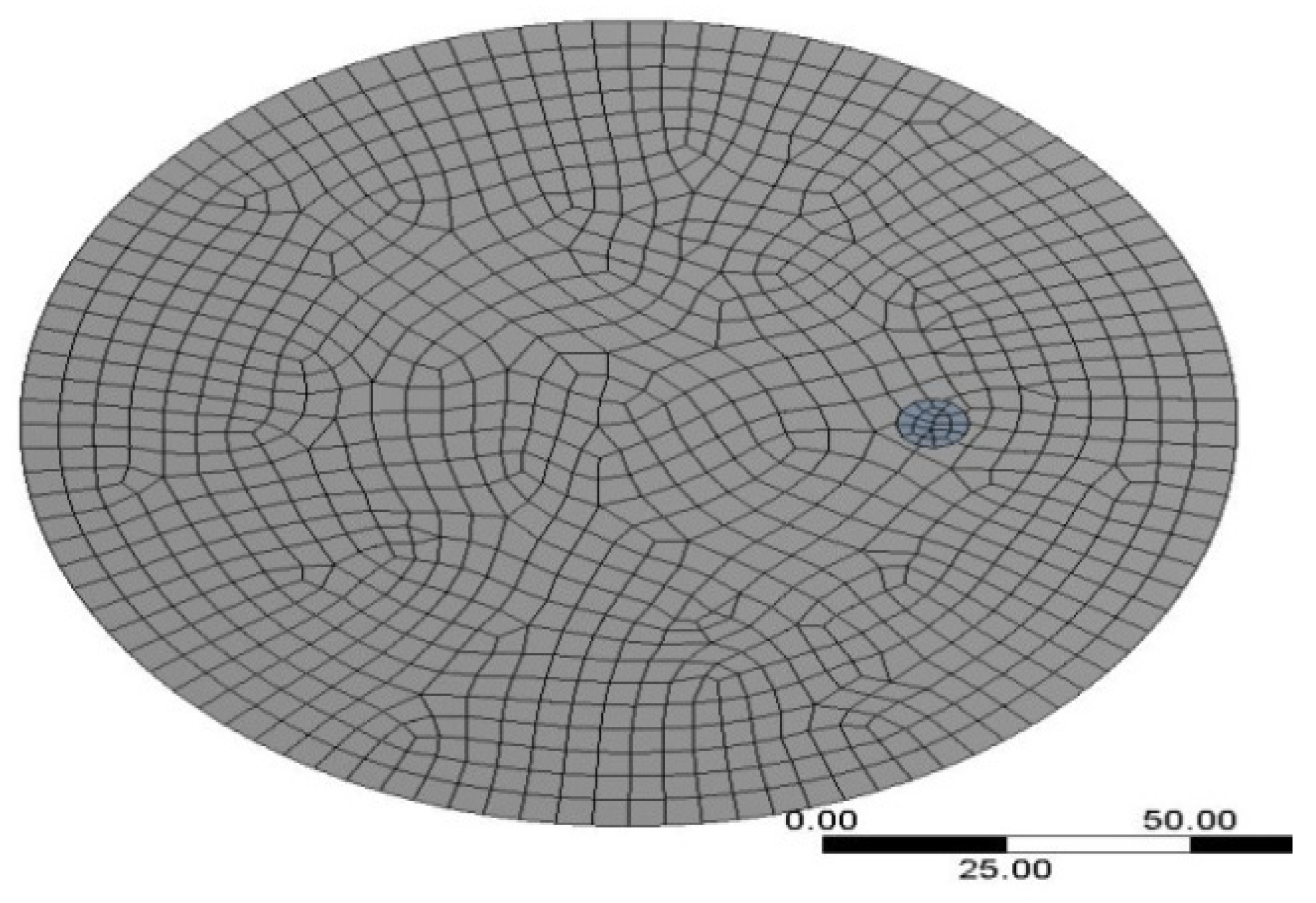
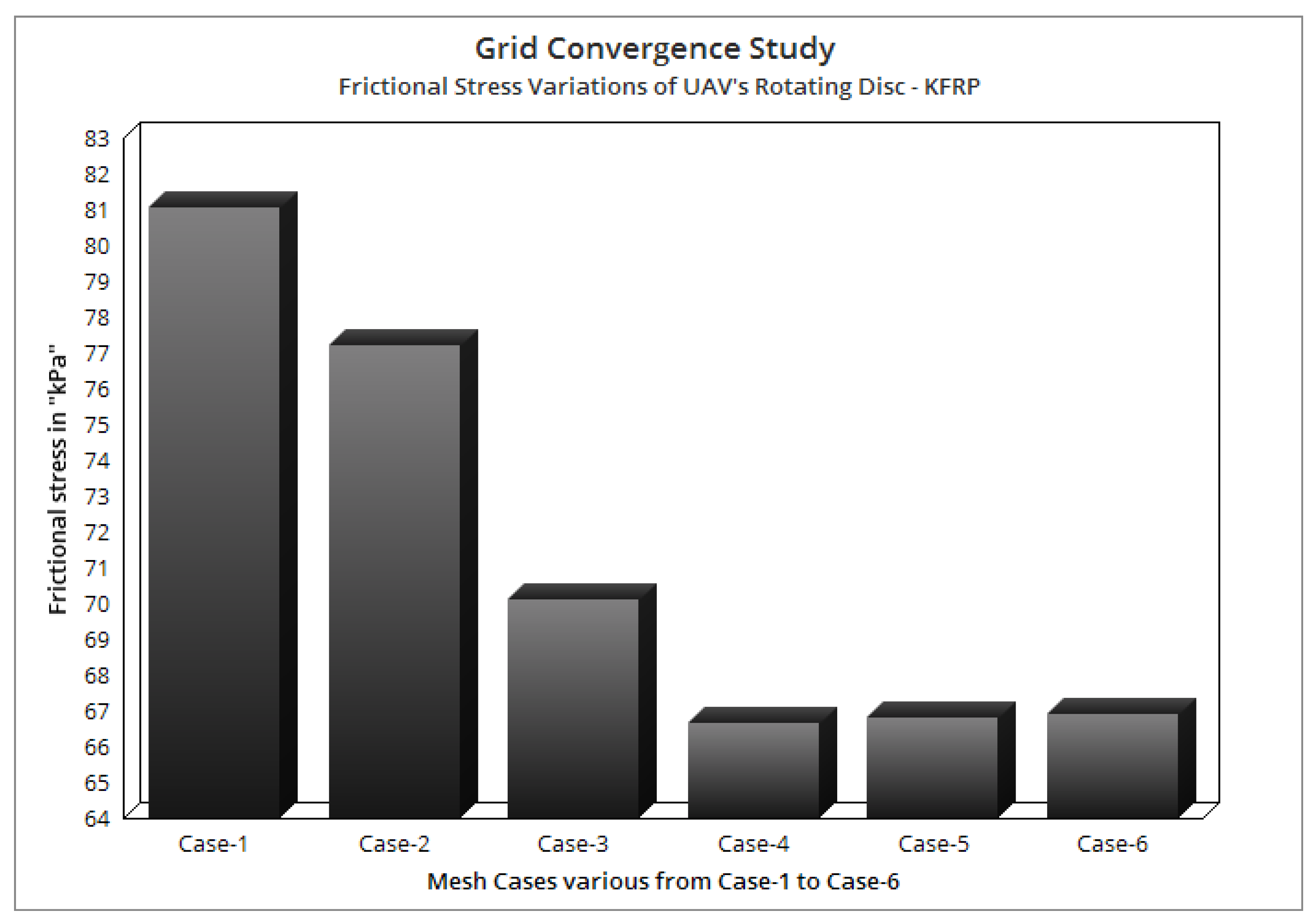
3.3. Validation—I—Grid Convergence Study
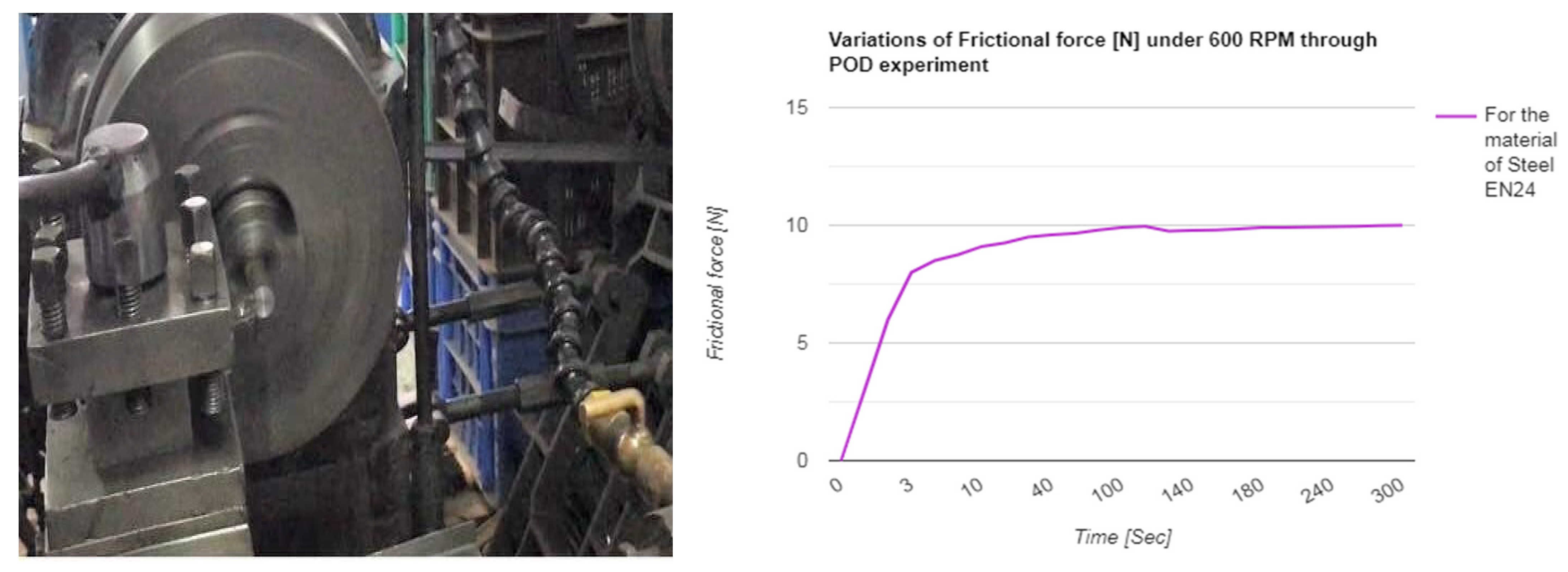
3.4. Validation—II—Pin on Disc Based Validation
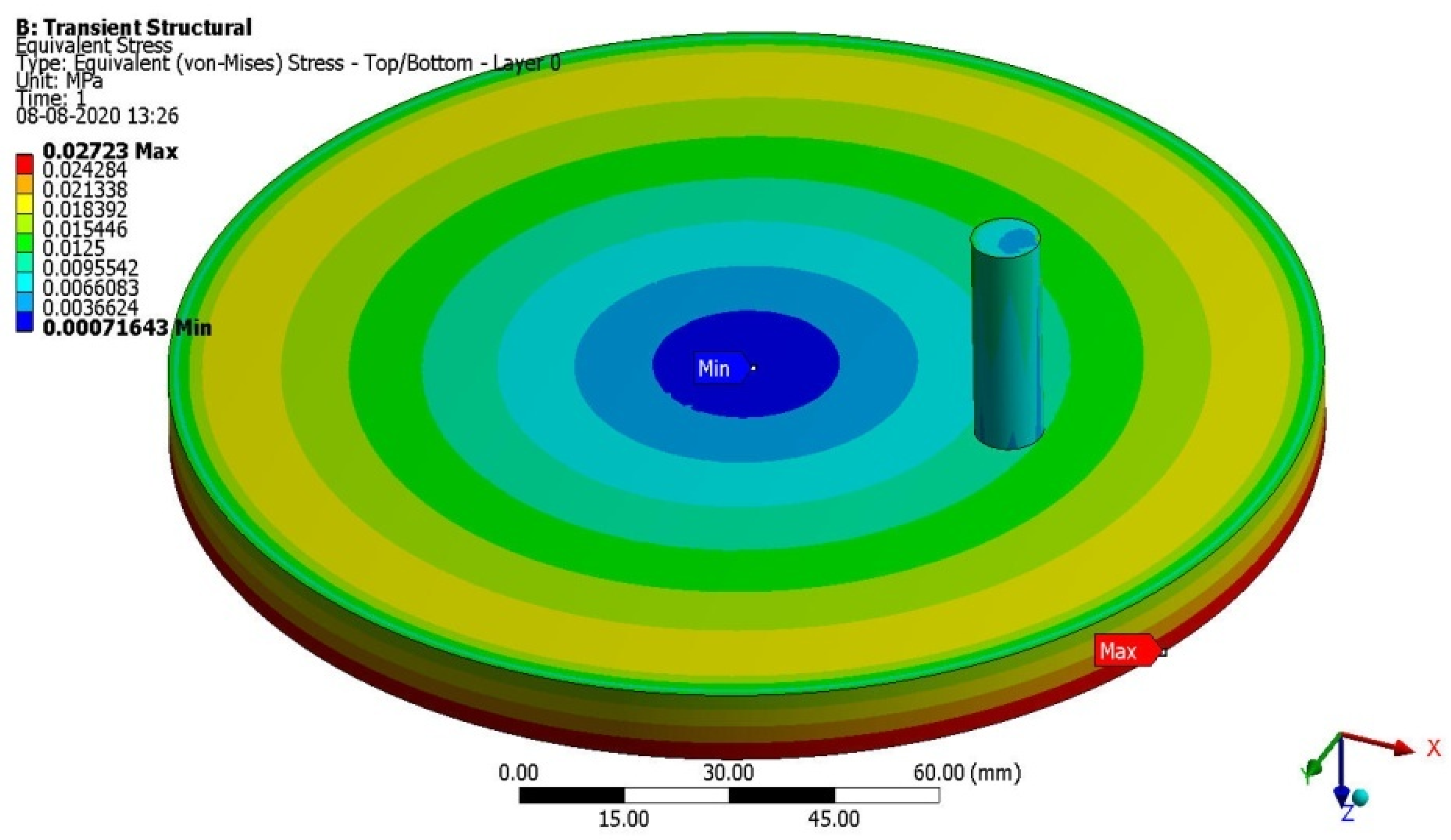
3.4.1. Finite Element Analysis Results
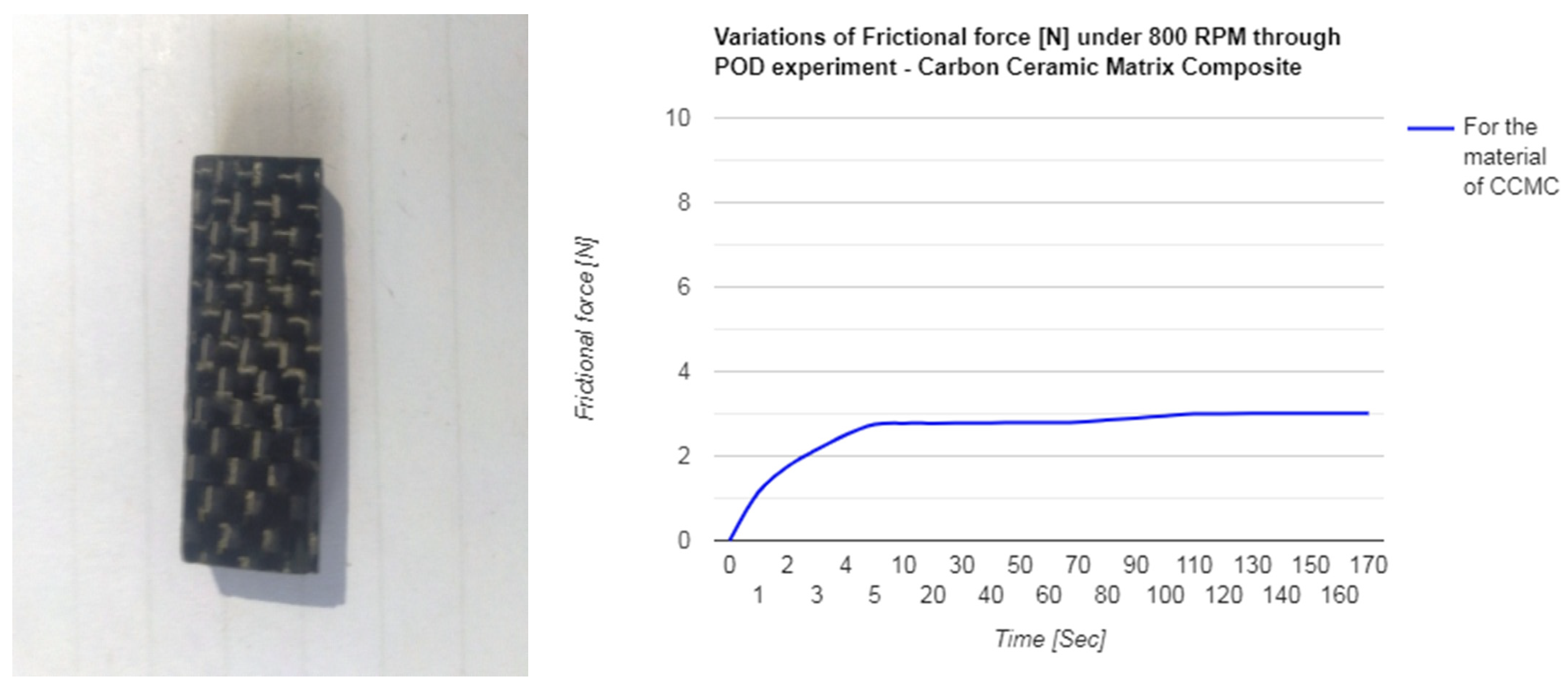
3.4.2. Experimental Results
3.5. Validation—III—Pin on Disc Based Validation
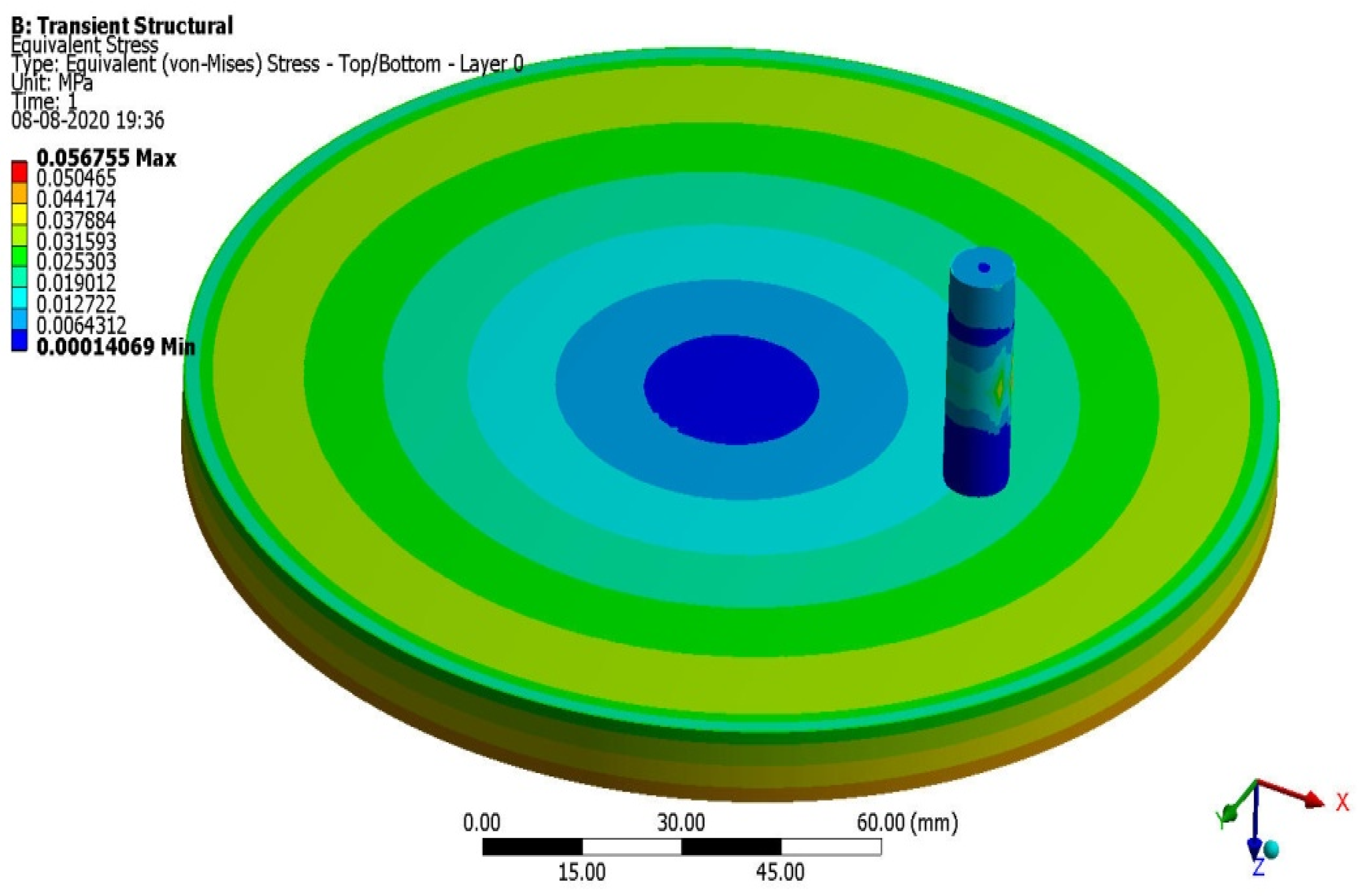
3.6. Validation—IV—Pin on Disc Based Validation
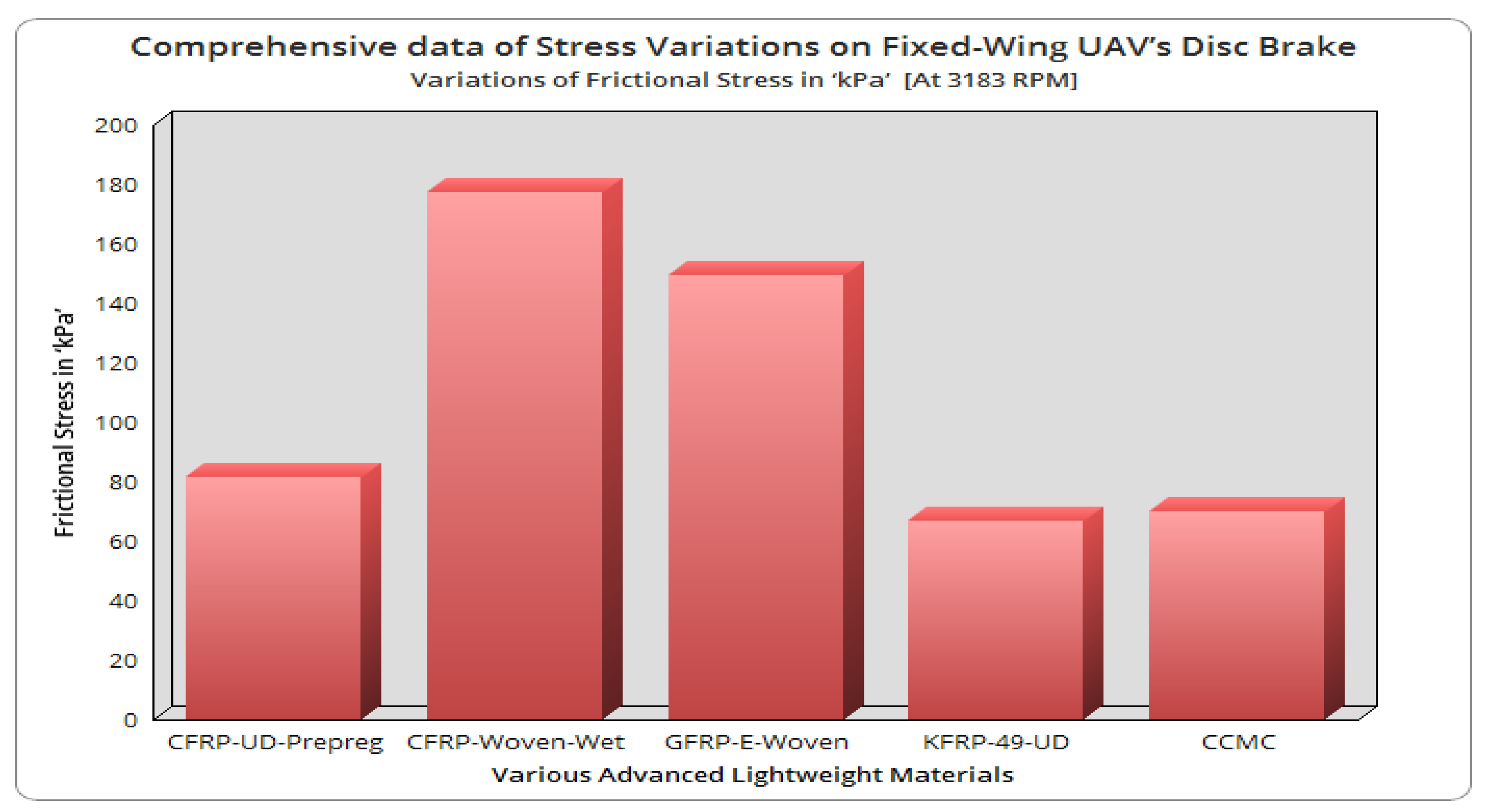
4. Results and Discussions on UAV’S Disc Brake
4.1. Conceptual Design (Asymmterical Case Study)
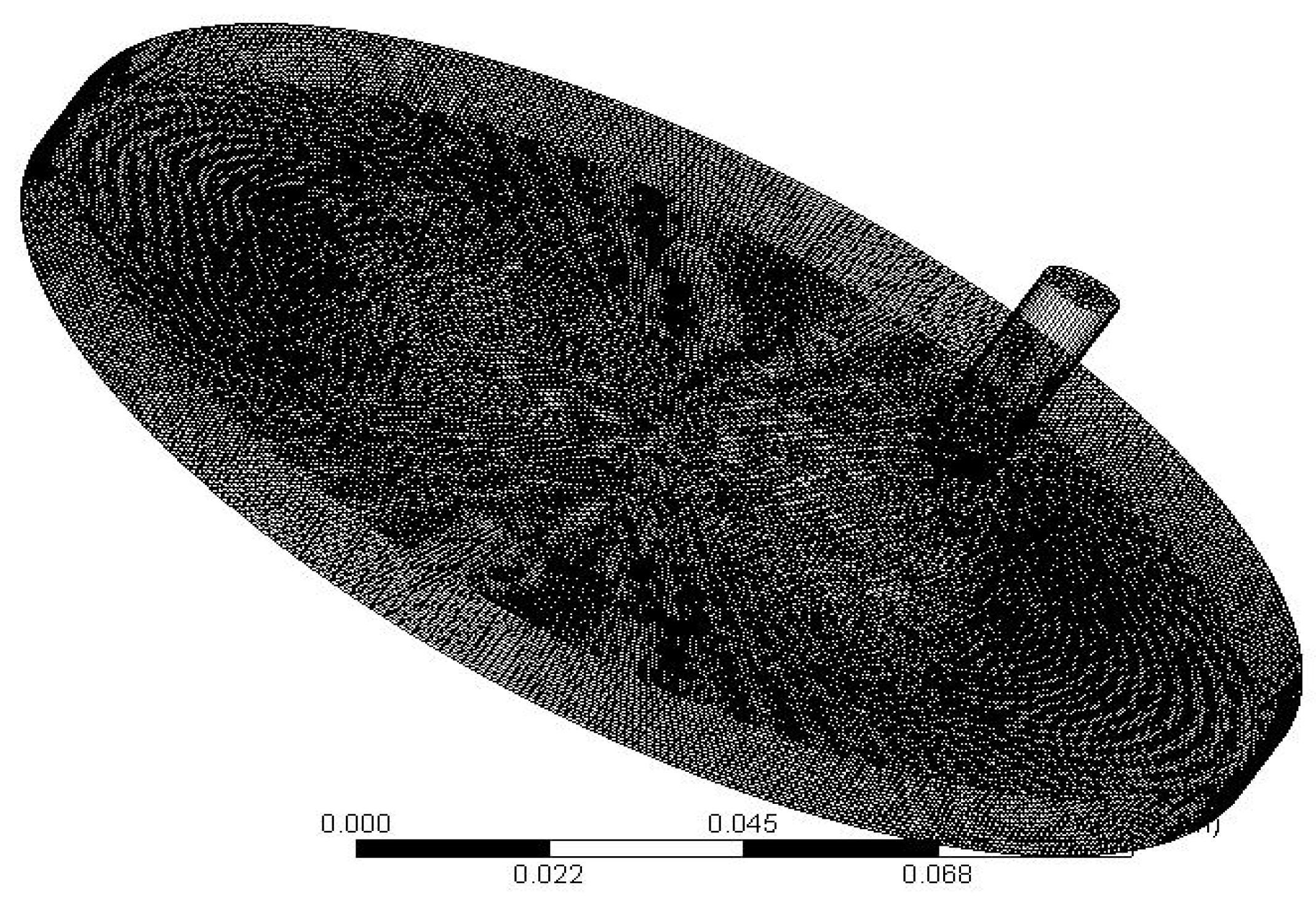
4.2. Discretization and Its Convergence Test
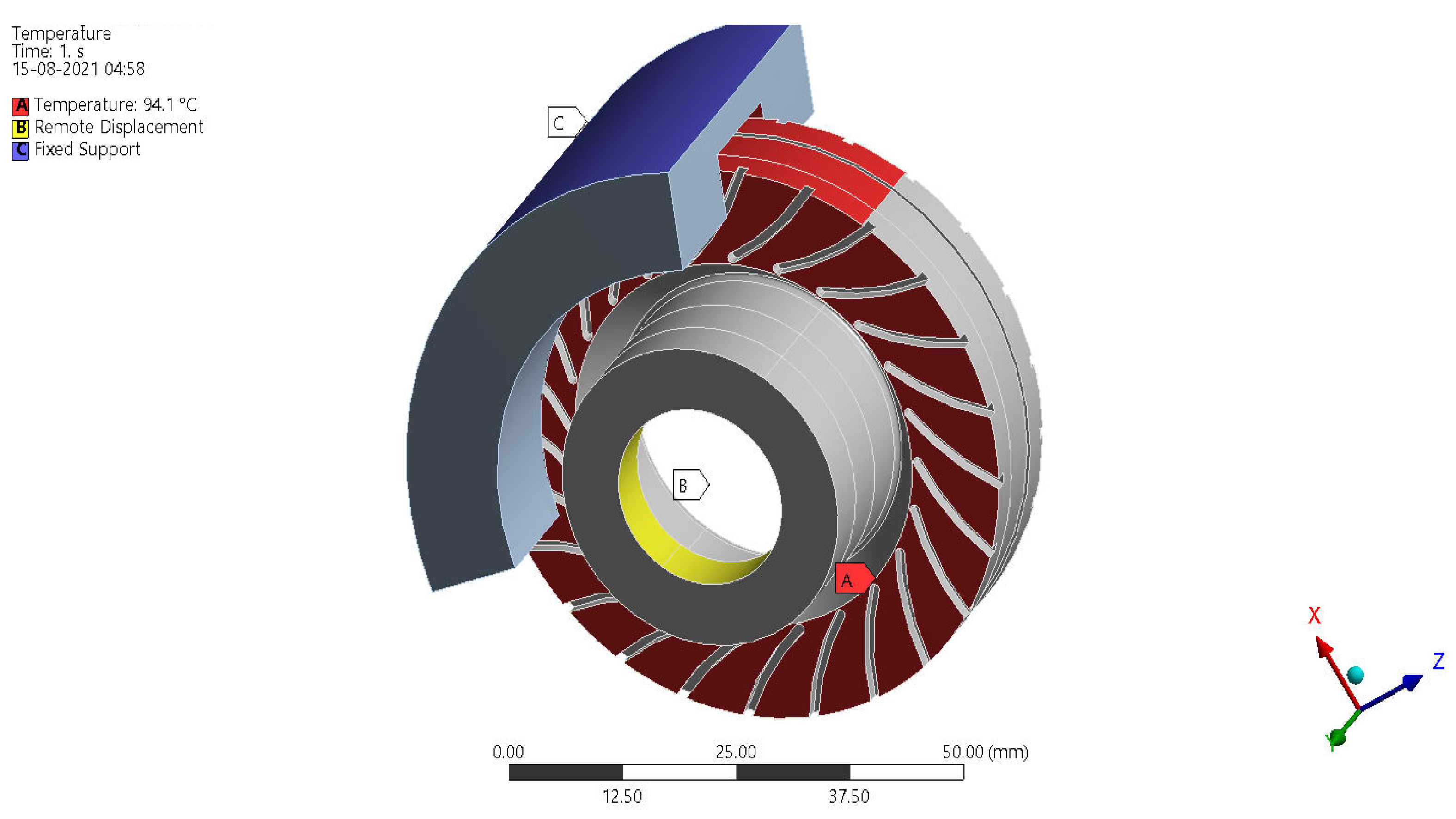
4.3. Boundary Conditions
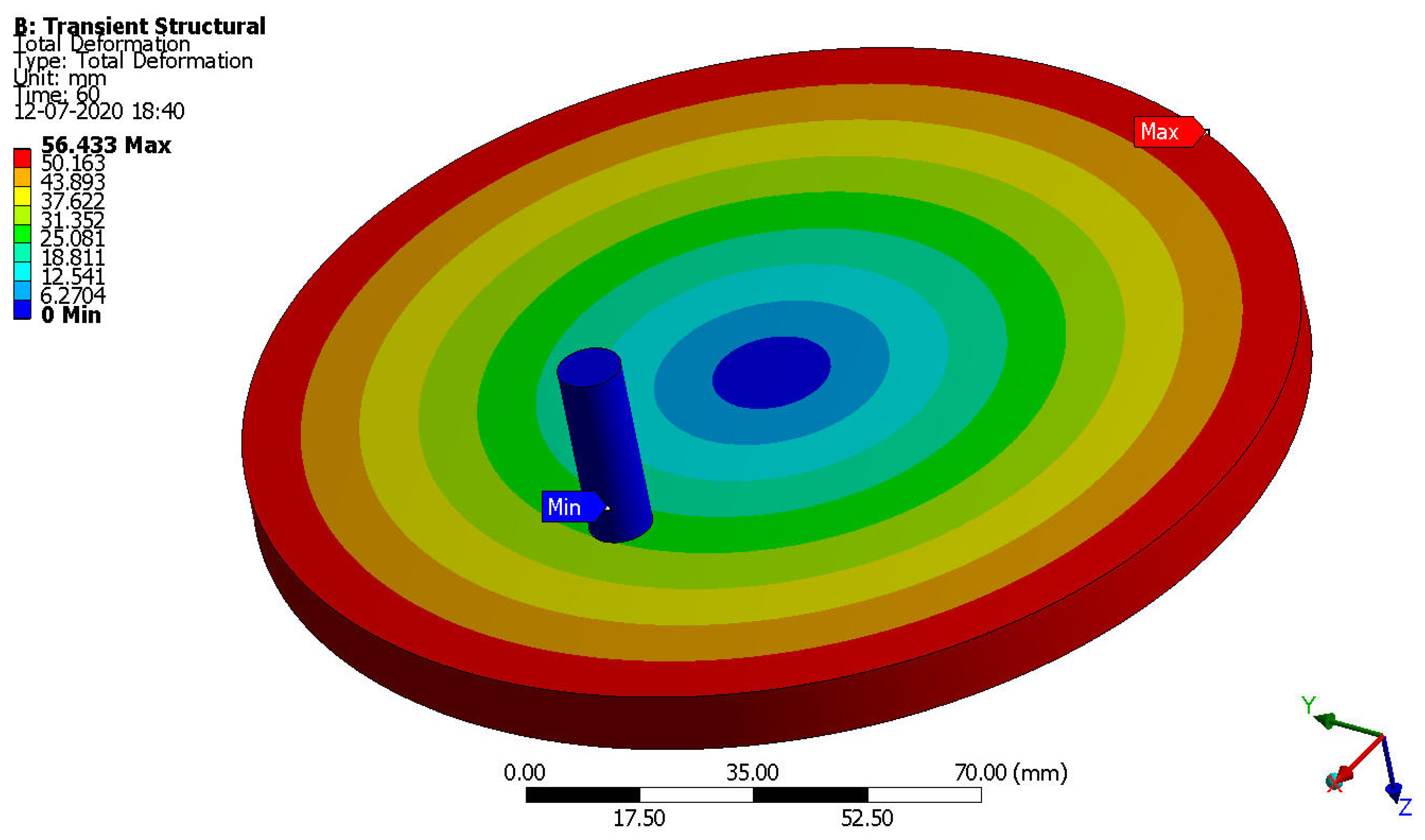
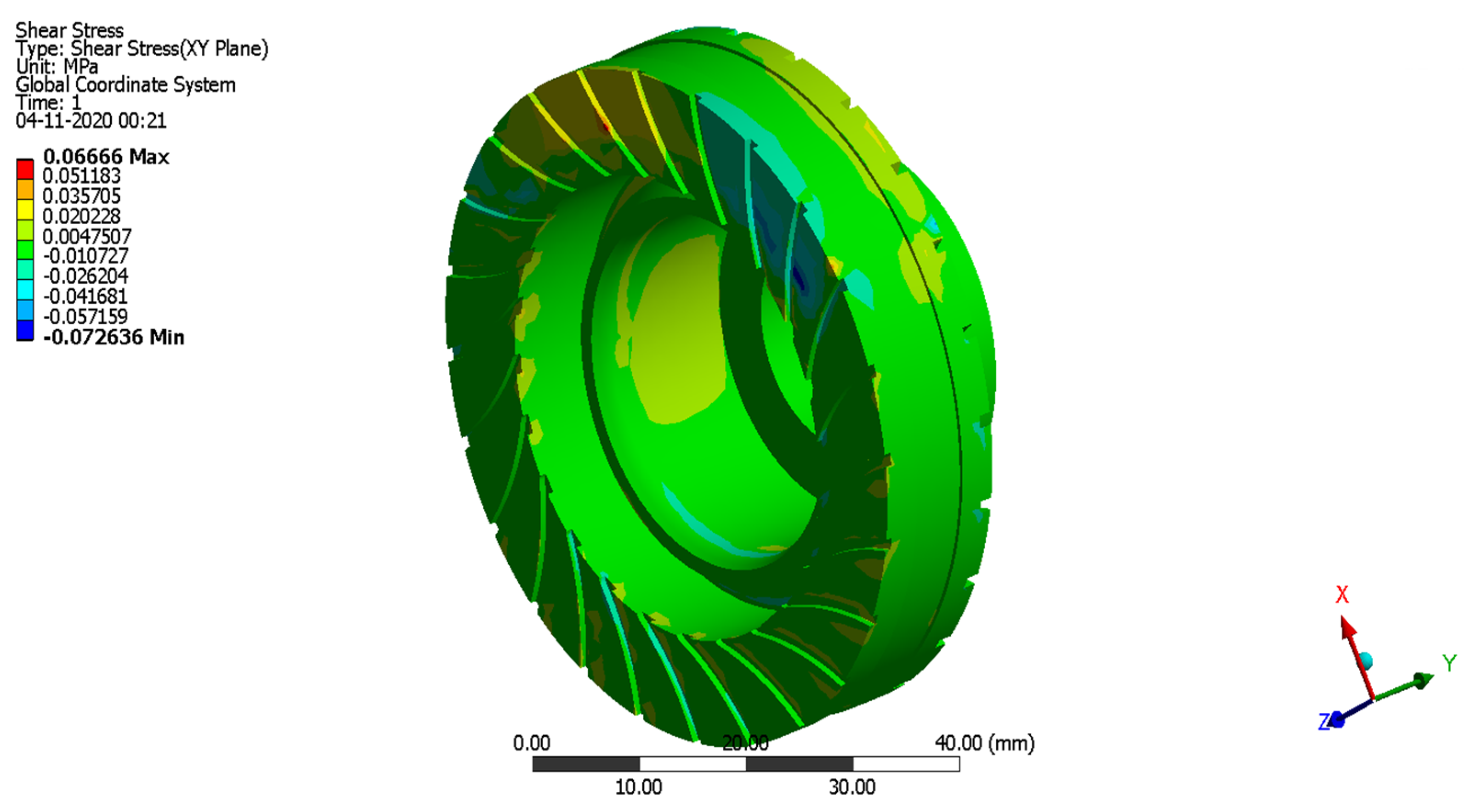
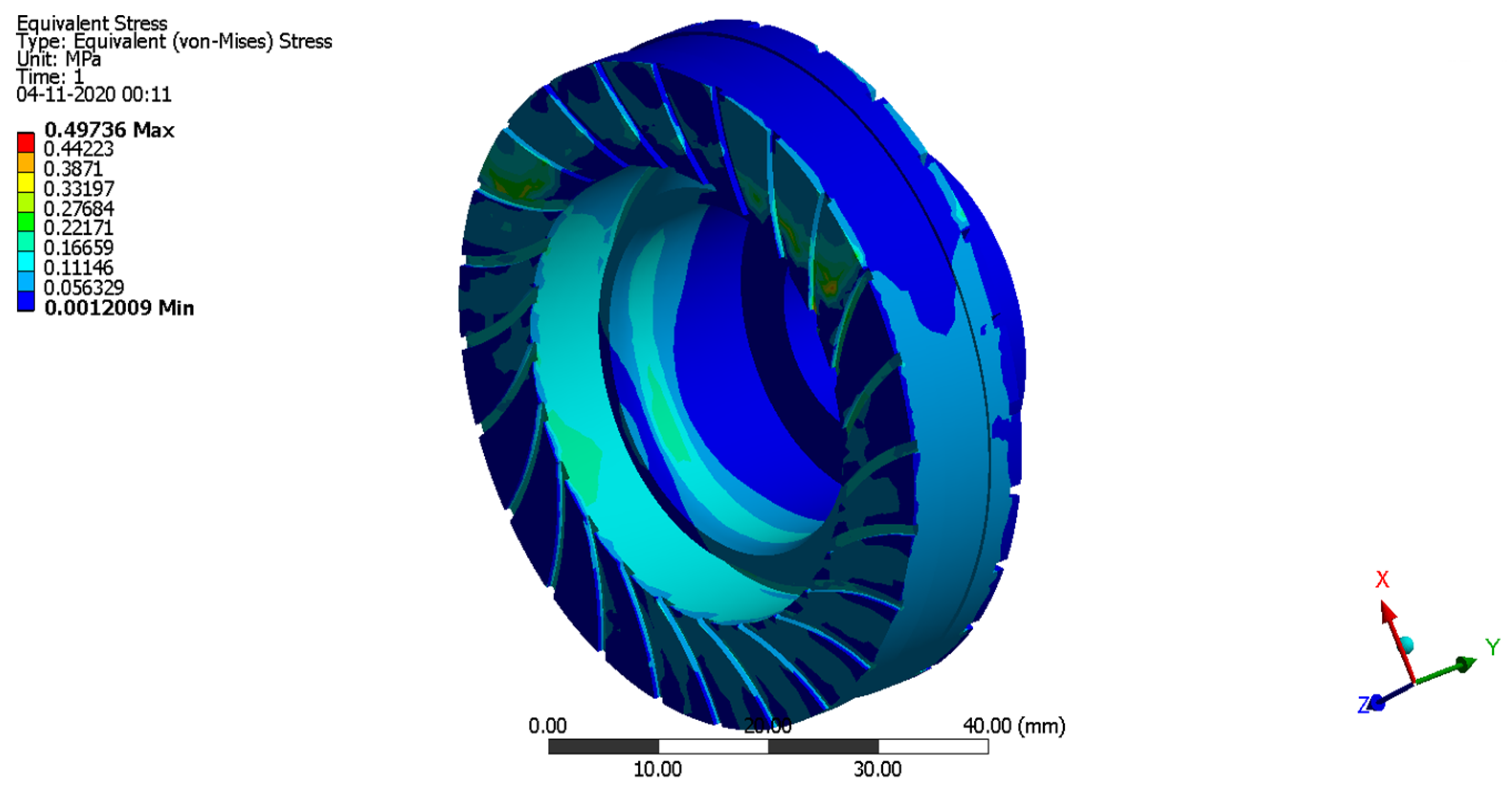
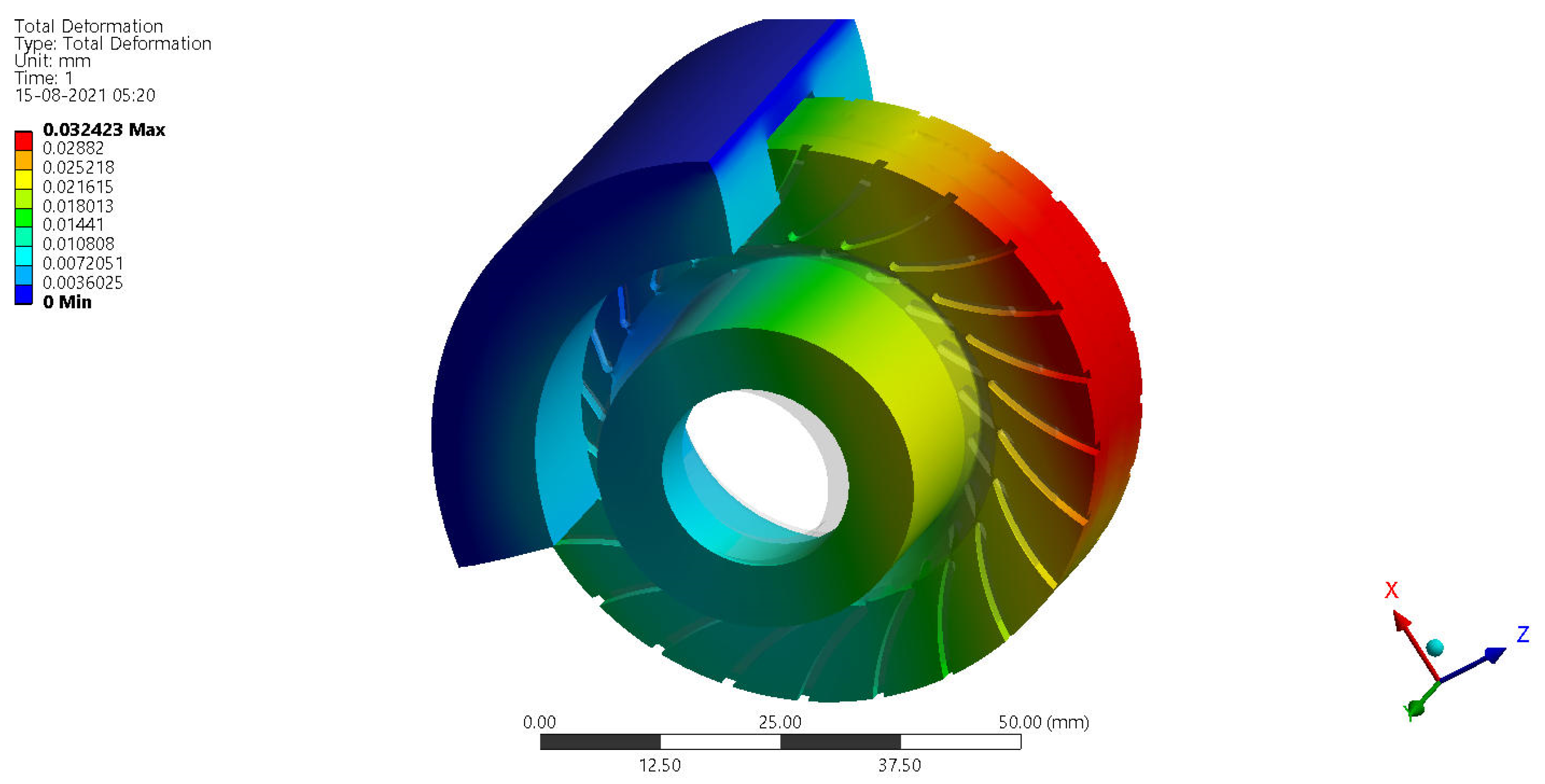
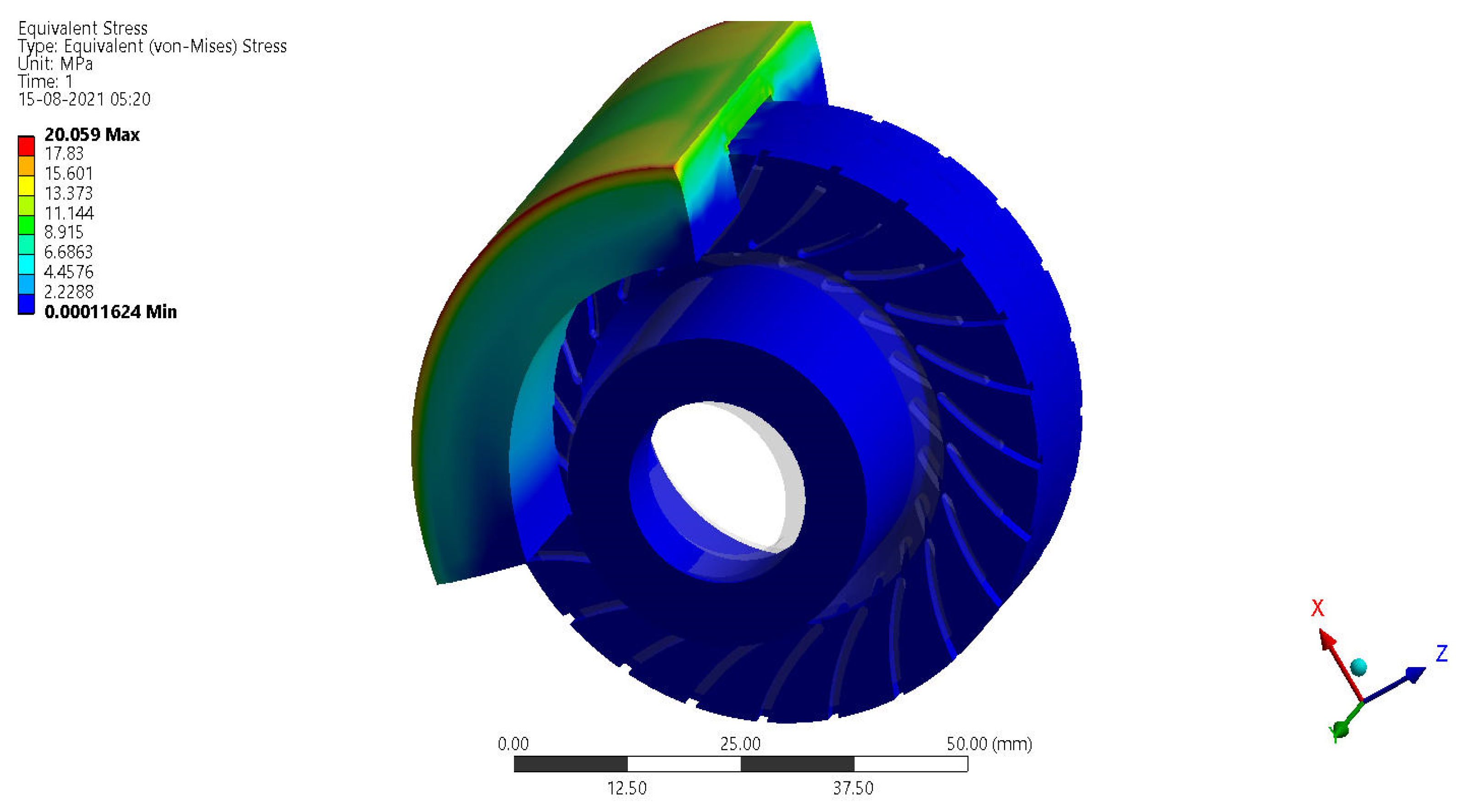
4.4. Structural Results—Assymetrical Failure Factors
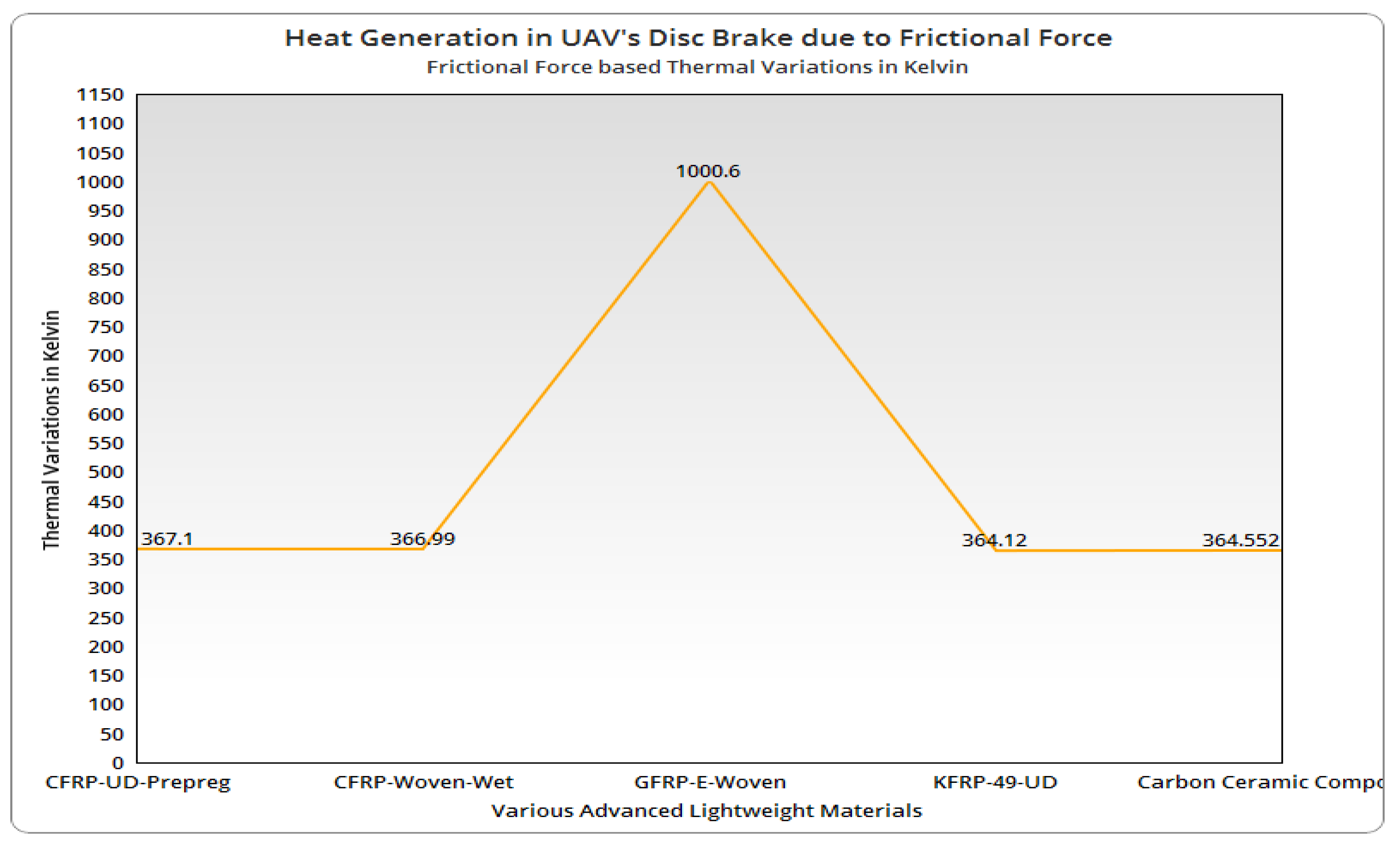
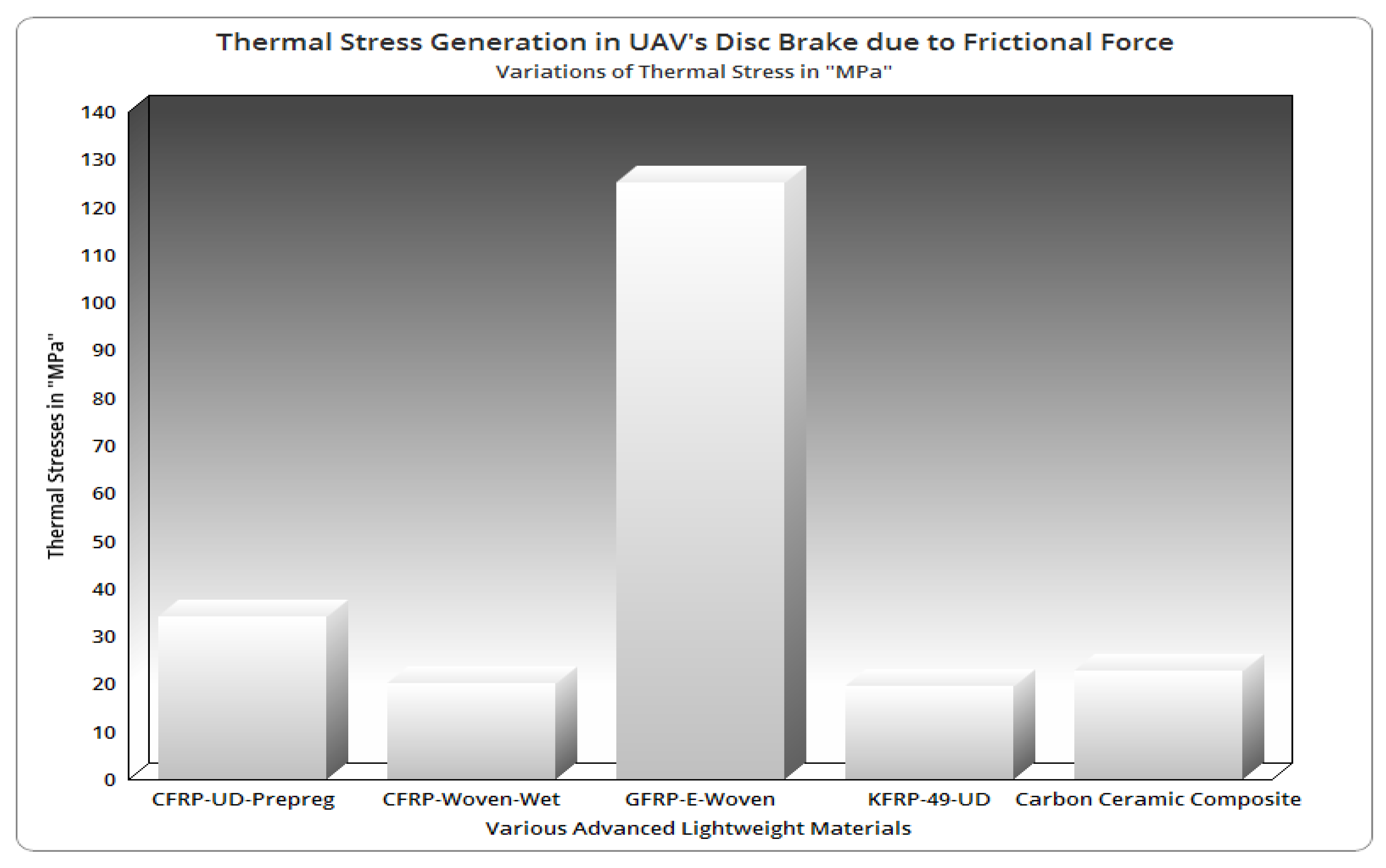
4.5. Thermal Stress Results—Assymetrical Failure Factors
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Symbols and Notations
| Symbol | Meaning |
| Payload Weight for UAV | |
| Overall Take-Off Weight of UAV | |
| Wing Loading of the UAV | |
| Surface area of the Wing | |
| Wingspan | |
| Root chord of the Wing | |
| Length of the UAV | |
| Diameter of the Fuselage | |
| Taper ratio | |
| Tip chord of the Wing | |
| Mean Aerodynamic Chord of the Wing | |
| ‘y’ location of the Mean Aerodynamic Chord | |
| ‘y’ wise position in Wing | |
| Surface Area of the Horizontal Tail | |
| Volume Coefficient of the Horizontal Tail | |
| Distance between MAC of Hori. Tail to MAC of Wing | |
| Horizontal Tail-Span | |
| Aspect Ratio of the Horizontal Tail | |
| Radius at Wing-Fuselage Interaction | |
| Radius at Tail region | |
| Velocity of the Atmospheric fluid | |
| Velocity of the UAV | |
| Thrust | |
| Density of the Atmospheric fluid | |
| Radius of the Propeller | |
| Coefficient of Lift | |
| Disc Area of the Propeller | |
| Velocity at forward manuvering | |
| Pitch angle | |
| Chord of the propeller | |
| Radius of the propeller | |
| n | Number of blades involved in propeller |
| k | Design factor [5.3×10−15] |
| R | Rotational speed of the rotor in RPM |
| D | Diameter of a rotor in inches |
| Pitch of rotor in inches | |
| P | Required power in watts |
| a | Chord at wing root |
| b | Chord at wing tip |
| c | Half of the wingspan |
| Height of the Landing Stick | |
| r1, r2 | Inner and Outer radius of the Landing Stick |
| DW | Nominal diameter of wheel/disc in inch |
| WW | Nominal section Width of wheel/disc in inch |
| dW | Nominal Wheel/Rim Diameter in inch |
References
- Kumar, G.R.; Vijayanandh, R.; Kamaludeen, M.B.; Balasubramanian, S.; Jagadeeshwaran, P.; Ramesh, M. Comparative Structural Characterization of Fiber Reinforced Composite Rotating Disc: A Validated Investigation. Tribol. Ind. 2020, 42, 608–620. [Google Scholar] [CrossRef]
- Vashi, Y. Structural and thermal optimization of rotor of multi-disc brake of aircraft. Int. J. Mech. Prod. Eng. 2018, 6, 16–23. [Google Scholar]
- Kisabo, A.B.; Osheku, C.A.; Samuel, S.O. Conceptual Design, Analysis and Construction of a Fixed-Wing Unmanned Arial Vehicle for Oil and Gas Pipeline Surveillance. J. Aircr. Spacecr. Technol. 2017, 1, 18–29. [Google Scholar] [CrossRef][Green Version]
- Gómez-Rodríguez, A.; Sanchez-Carmona, A.; García-Hernández, L.; Cuerno-Rejado, C. Preliminary Correlations for Remotely Piloted Aircraft Systems Sizing. Aerospace 2018, 5, 5. [Google Scholar] [CrossRef]
- Raymer, D. Aircraft Design A Conceptual Approach, 6th ed.; AIAA: Reston, VA, USA, 2018; ISBN 978-1-62410-490-9. [Google Scholar] [CrossRef]
- Wróbel, G.; Pawlak, S.; Muzia, G. Thermal diffusivity measurements of selected fiber reinforced polymer composites using heat pulse method. Arch. Mater. Sci. Eng. 2011, 48, 25–32. [Google Scholar]
- Scruggs, A.M.; Kirmse, S.; Hsiao, K.-T. Enhancement of Through-Thickness Thermal Transport in Unidirectional Carbon Fiber Reinforced Plastic Laminates due to the Synergetic Role of Carbon Nanofiber Z-Threads. J. Nanomater. 2019, 2019, 1–13. [Google Scholar] [CrossRef]
- Dong, K.; Liu, K.; Zhang, Q.; Gu, B.; Sun, B. Experimental and numerical analyses on the thermal conductive behaviors of carbon fiber/epoxy plain woven composites. Int. J. Heat Mass Transf. 2016, 102, 501–517. [Google Scholar] [CrossRef]
- Alarifi, I.M. Investigation the conductivity of carbon fiber composites focusing on measurement techniques under dynamic and static loads. J. Mater. Res. Technol. 2019, 8, 4863–4893. [Google Scholar] [CrossRef]
- Takizawa, Y.; Chung, D.D.L. Through-thickness thermal conduction in glass fiber polymer–matrix composites and its enhancement by composite modification. J. Mater. Sci. 2015, 51, 3463–3480. [Google Scholar] [CrossRef]
- Brown, I.; Burgoyne, C. The friction and wear of Kevlar 49 sliding against aluminium at low velocity under high contact pressures. Wear 1999, 236, 315–327. [Google Scholar] [CrossRef]
- Ventura, G.; Martelli, V. Thermal conductivity of Kevlar 49 between 7 and 290 K. Cryogenics 2009, 49, 735–737. [Google Scholar] [CrossRef]
- Zhao, J.; Cai, R.; Ma, Z.; Zhang, K.; Liang, H.; Qiu, H.; Liu, S.; Xie, W. Preparation and properties of C/SiC composites reinforced by high thermal conductivity graphite films. Diam. Relat. Mater. 2021, 116, 108376. [Google Scholar] [CrossRef]
- Raja, V.; Gnanasekaran, R.K.; Kaladgi, A.R.; Rajendran, P.; Khan, S.A.; Asif, M. Multi-Disciplinary Computational Investigations on Asymmetrical Failure Factors of Disc Brakes for Various CFRP Materials: A Validated Approach. Symmetry 2022, 14, 1616. [Google Scholar] [CrossRef]
- Vijayakumar, M.; Vijayanandh, R.; Ramesh, M.; Senthil Kumar, M.; Raj Kumar, G.; Sivaranjani, S.; Jung, D.W. Conceptual Design and Numerical analysis of an Unmanned Amphibious Vehicle. In Proceedings of the AIAA Scitech 2021 Forum, Reston, VA, USA, 4 January 2021. [Google Scholar]
- Yeong, S.P.; Dol, S.S. Aerodynamic Optimization of Micro Aerial. Veh. J. Appl. Fluid Mech. 2016, 9, 2111–2121. [Google Scholar]
- Vijayanandh, R.; Senthilkumar, S.; Rajkumar, R.; Kumar, A.; Kumar, J.D.; Kumar, K.K.; Prakash, R.A. Conceptual design and computational investigations of fixed wing unmanned aerial vehicle for medium-range applications. Autonomous Connect. Heavy Veh. Technol. 2022, 353–374. [Google Scholar] [CrossRef]
- Ong, W.; Srigrarom, S.; Hesse, H. Design methodology for heavy-lift unmanned aerial vehicles with coaxial rotors. In Proceedings of the AIAA SciTech Forum, San Diego, CA, USA, 7–11 January 2019. [Google Scholar] [CrossRef]
- Perdiou, A.S.; Eldin, R.A.; Hajaj, K.R.; Rominu, M.; Sinescu, C.; Negrutiu, M.; Hajaj, T.A. Comparative Evaluation of Stress Resistance Between Nano-hybrid Composite and Ormocer Restorations on Posterior Teeth-in vitro Study. Mater. Plast. 2020, 57, 8–12. [Google Scholar] [CrossRef]
- Lei, Y.; Huang, Y.; Wang, H. Aerodynamic Performance of an Octorotor SUAV with Different Rotor Spacing in Hover. Processes 2020, 8, 1364. [Google Scholar] [CrossRef]
- Raja, V.; Solaiappan, S.K.; Kumar, L.; Marimuthu, A.; Gnanasekaran, R.K.; Choi, Y. Design and Computational Analyses of Nature Inspired Unmanned Amphibious Vehicle for Deep Sea Mining. Minerals 2022, 12, 342. [Google Scholar] [CrossRef]
- Lichota, P. Multi-Axis Inputs for Identification of a Reconfigurable Fixed-Wing UAV. Aerospace 2020, 7, 113. [Google Scholar] [CrossRef]
- Venkatesan, K.; Geetha, S.; Vijayanandh, R.; Kumar, G.R.; Jagadeeshwaran, P. Advanced structural analysis of various composite materials with carbon nano-tubes for property enhancement. AIP Conf. Proc. 2020, 2270, 030005. [Google Scholar] [CrossRef]
- Chu, T.; Starek, M.; Berryhill, J.; Quiroga, C.; Pashaei, M. Simulation and Characterization of Wind Impacts on sUAS Flight Performance for Crash Scene Reconstruction. Drones 2021, 5, 67. [Google Scholar] [CrossRef]
- García-León, R.; Afanador-García, N.; Gómez-Camperos, J. Numerical Study of Heat Transfer and Speed Air Flow on Performance of an Auto-Ventilated Disc Brake. Fluids 2021, 6, 160. [Google Scholar] [CrossRef]
- Kumar, G.R.; Vijayanandh, R.; Venkatesan, K.; Ramesh, M.; Kumar, M.S.; Balaji, S. Comparative Investigations onthe Main Elements of Carbon Fiber Based Composites Using Computational Structural Simulations. J. Phys. Conf. Ser. 2020, 1504, 012003. [Google Scholar] [CrossRef]
- Venkatesan, K.; Ramanathan, K.; Vijayanandh, R.; Selvaraj, S.; Kumar, G.R.; Kumar, M.S. Comparative structural analysis of advanced multi-layer composite materials. Mater. Today Proc. 2019, 27, 2673–2687. [Google Scholar] [CrossRef]
- Mirrudula, P.; Priya, P.K.; Malavika, M.; Kumar, G.R.; Vijayanandh, R.; Kumar, M.S. Comparative structural analysis of the sandwich composite using advanced numerical simulation. AIP Conf. Proc. 2020, 2270, 040005. [Google Scholar] [CrossRef]
- Raja, V.; Venkatesan, K.; Ramesh, M.; Kumar, G.R.; Kumar, M.S. Optimization of orientation of carbon fiber reinforced polymer based on structural analysis. Int. J. Sci. Technol. Res. 2019, 8, 3020–3029. [Google Scholar]
- Arul, P.R.; Vijayanandh, R.; Ramesh, G.; Hariaran, S.; Janardhanan, Y.; Senthil, K.M.; Jagadeeshwaran, P. Investigation of Automotive Disc Brake′s Material Based on Tribological Parameters by Using Computational Structural Analysis. Tribol. Charact. Surf. Coat. 2022, 10, 211–238. [Google Scholar] [CrossRef]
- Vinsiya, A.M.; Janaki, B.R.; Kings, R.M.; Vijayanandh, R.; Prakash, R.A.; Kumar, G.R.; Balasubramanian, S. Comparative structural and frictional analyses on various lightweight materials for aircraft disc brake. Mater. Today Proc. 2022, 59, A22–A35. [Google Scholar] [CrossRef]
- León, R.A.G.; Rojas, E.P. Analisis de la cantidad de flujo de calorcircundante entre los canales de refrigeraciónentres discos de frenoautoventilados. Ing. Univ. 2016, 21, 71–96. [Google Scholar] [CrossRef]
- Kesavan, K.; Kiran, P.; Sivaguru, M.; Prasanth, S.I.; Sudharsan, R.; Kumar, G.R.; Vijayanandh, R. Multi-objective Structural Analysis of Kevlar Fiber Reinforced Polymer Composite. Recent Adv. Smart Manuf. Mater. 2021, 11, 137–151. [Google Scholar] [CrossRef]
- Pisciotta, A.; Di Lorenzo, R.; Novara, A.; Laudicina, V.A.; Barone, E.; Santoro, A.; Gristina, L.; Barbagallo, M.G. Cover crop and pruning residue management to reduce nitrogen mineral fertilization in mediterranean vineyards. Agronomy 2021, 11, 164. [Google Scholar] [CrossRef]
- Prasanth, S.I.; Kesavan, K.; Kiran, P.; Sivaguru, M.; Sudharsan, R.; Kumar, G.R.; Vijayanandh, R. Fiber Oriental Optimization on Glass Fiber Reinforced Polymer Composite in Multi Objective Perspective based on Computational Structural Analysis. J. Phys. Conf. Ser. 2021, 1849, 012005. [Google Scholar] [CrossRef]
- Sharath, B.; Venkatesh, C.; Afzal, A.; Aslfattahi, N.; Aabid, A.; Baig, M.; Saleh, B. Multi Ceramic Particles Inclusion in the Aluminium Matrix and Wear Characterization through Experimental and Response Surface-Artificial Neural Networks. Materials 2021, 14, 2895. [Google Scholar] [CrossRef] [PubMed]
- Rethnam, G.S.N.; Manivel, S.; Sharma, V.K.; Srinivas, C.; Afzal, A.; Razak, R.K.A.; Alamri, S.; Saleel, C.A. Parameter Study on Friction Surfacing of AISI316Ti Stainless Steel over EN8 Carbon Steel and Its Effect on Coating Dimensions and Bond Strength. Materials 2021, 14, 4967. [Google Scholar] [CrossRef] [PubMed]
- Prasanth, S.I.; Kesavan, K.; Kiran, P.; Sivaguru, M.; Sudharsan, R.; Vijayanandh, R. Advanced structural analysis on e-glass fiber reinforced with polymer for enhancing the mechanical properties by optimizing the orientation of fiber. AIP Conf. Proc. 2020, 2270, 040006. [Google Scholar] [CrossRef]
- Afzal, A.; Mujeebu, M.A. Thermo-Mechanical and Structural Performances of Automobile Disc Brakes: A Review of Numerical and Experimental Studies. Arch. Comput. Methods Eng. 2018, 26, 1489–1513. [Google Scholar] [CrossRef]
- Bhagavathiyappan, S.; Balamurugan, M.; Rajamanickam, M.; Vijayanandh, R.; Kumar, G.R.; Kumar, M.S. Comparative computational impact analysis of multi-layer composite materials. AIP Conf. Proc. 2020, 2270, 040007. [Google Scholar] [CrossRef]
- Belhocine, A.; Afzal, A. Computational finite element analysis of brake disc rotors employing different materials. Aust. J. Mech. Eng. 2020, 20, 637–650. [Google Scholar] [CrossRef]










| Location (Inch) | Pitch Angle (°) | Chord Length (Inch) | Location (Inch) | Pitch Angle (°) | Chord Length (Inch) |
|---|---|---|---|---|---|
| 1.274 | 62.37 | 1.79 | 7.644 | 17.66 | 1.254 |
| 2.548 | 43.68 | 2.011 | 8.918 | 15.26 | 1.122 |
| 3.822 | 32.49 | 1.835 | 10.192 | 13.43 | 1.013 |
| 5.096 | 25.53 | 1.62 | 11.466 | 11.98 | 0.923 |
| 6.37 | 20.91 | 1.42 | 12.74 | 10.82 | 0.85 |
| Aerofoil | CL | CD | Aerofoil | CL | CD |
|---|---|---|---|---|---|
| NACA 2412 | 0.9744 | 0.035 | NACA 23015 | 0.9744 | 0.04 |
| NACA 1412 | 0.05 | NACA 23112 | 0.04 | ||
| S8052 | 0.036 | NACA 24112 | 0.039 | ||
| NACA 2415 | 0.04 | NACA 63(1)-412 | 0.0425 | ||
| NACA 4415 | 0.04 | NACA 64(1)-212 MOD B | 0.0475 | ||
| NACA 4418 | 0.06 | NACA 66(4)-021 | 0.055 | ||
| NACA 22112 | 0.05 | NACA 63(2)-615 | 0.0475 | ||
| NACA 23012 | 0.04 |
| Sl. No. | Components Name | Weight (Grams) | Sl. No. | Components Name | Weight (Grams) |
|---|---|---|---|---|---|
| 1 | Payload | 5000 | 6 | Other electronic items | 100 |
| 2 | Propeller | 125.1783 | 7 | Fuselage | 10250 |
| 3 | Motor | 232 | 8 | Horizontal tail | 184.32 |
| 4 | Battery | 2240 | 9 | Main wing | 900 |
| 5 | ESC | 79 | 10 | Vertical tail | 145.08 |
| Total weight | 19,255.5783 | ||||
| Landing gear weight ] | 25,000 − 19,255.5783 = 5744.4217 | ||||
| Mesh Case | Nodes | Elements | Mesh Case | Nodes | Elements |
|---|---|---|---|---|---|
| Case—I | 32478 | 24567 | Case—IV | 399554 | 475256 |
| Case—II | 55142 | 54783 | Case—V | 912220 | 874590 |
| Case—III | 154545 | 145789 | Case—VI | 962451 | 974562 |
| Sl. No. | Methodology Used | Frictional Force (N) | Error (%) |
|---|---|---|---|
| 1 | FEA results | 26.80 | 0.75 |
| 2 | Pin-on-disc results | 27.01 |
| Sl. No. | Methodology Used | Frictional Force (N) | Error (%) |
|---|---|---|---|
| 1 | FEA results | 9.7949 | 0.46 |
| 2 | Pin-on-disc results | 9.75 |
| Sl. No. | Methodology Used | Frictional Force (N) | Error (%) |
|---|---|---|---|
| 1 | FEA results | 2.5265 | 0.9215 |
| 2 | Pin-on-disc results | 2.55 |
| Mesh Case | Nodes | Elements | Mesh Case | Nodes | Elements |
|---|---|---|---|---|---|
| Case—I | 50254 | 87459 | Case—III | 124550 | 245709 |
| Case—II | 80451 | 99874 | Case—IV | 321001 | 547120 |
| Case—V | 91420 | 112457 | Case—VI | 664512 | 978451 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Raja, V.; Gnanasekaran, R.K.; Rajendran, P.; Mohd Ali, A.; Rasheed, R.; AL-bonsrulah, H.A.Z.; Al-Bahrani, M. Asymmetrical Damage Aspects Based Investigations on the Disc Brake of Long-Range UAVs through Verified Computational Coupled Approaches. Symmetry 2022, 14, 2035. https://doi.org/10.3390/sym14102035
Raja V, Gnanasekaran RK, Rajendran P, Mohd Ali A, Rasheed R, AL-bonsrulah HAZ, Al-Bahrani M. Asymmetrical Damage Aspects Based Investigations on the Disc Brake of Long-Range UAVs through Verified Computational Coupled Approaches. Symmetry. 2022; 14(10):2035. https://doi.org/10.3390/sym14102035
Chicago/Turabian StyleRaja, Vijayanandh, Raj Kumar Gnanasekaran, Parvathy Rajendran, Aiffah Mohd Ali, Raffik Rasheed, Hussein A. Z. AL-bonsrulah, and Mohammed Al-Bahrani. 2022. "Asymmetrical Damage Aspects Based Investigations on the Disc Brake of Long-Range UAVs through Verified Computational Coupled Approaches" Symmetry 14, no. 10: 2035. https://doi.org/10.3390/sym14102035
APA StyleRaja, V., Gnanasekaran, R. K., Rajendran, P., Mohd Ali, A., Rasheed, R., AL-bonsrulah, H. A. Z., & Al-Bahrani, M. (2022). Asymmetrical Damage Aspects Based Investigations on the Disc Brake of Long-Range UAVs through Verified Computational Coupled Approaches. Symmetry, 14(10), 2035. https://doi.org/10.3390/sym14102035














