Electronic Origin of Tc in Bulk and Monolayer FeSe
Abstract
1. Introduction
- Present the mathematical formulation and implementation of different susceptibilities in spin, charge and superconducting channels;
- List the structural and Hubbard parameters used for different materials, and present ab initio results bulk FeSe;
- By treating J and the Se height as free parameters, show how they affect correlations and superconductivity in bulk FeSe;
- Show results for a standing monolayer of FeSe (M-FeSe);
- Show results for a monolayer of FeSe on SrTiO (M-FeSe/STO);
- Interpret these results to explain what controls T.
2. Methodology
3. Results and Discussion
3.1. Structural Parameters and c-RPA Estimates for U and J
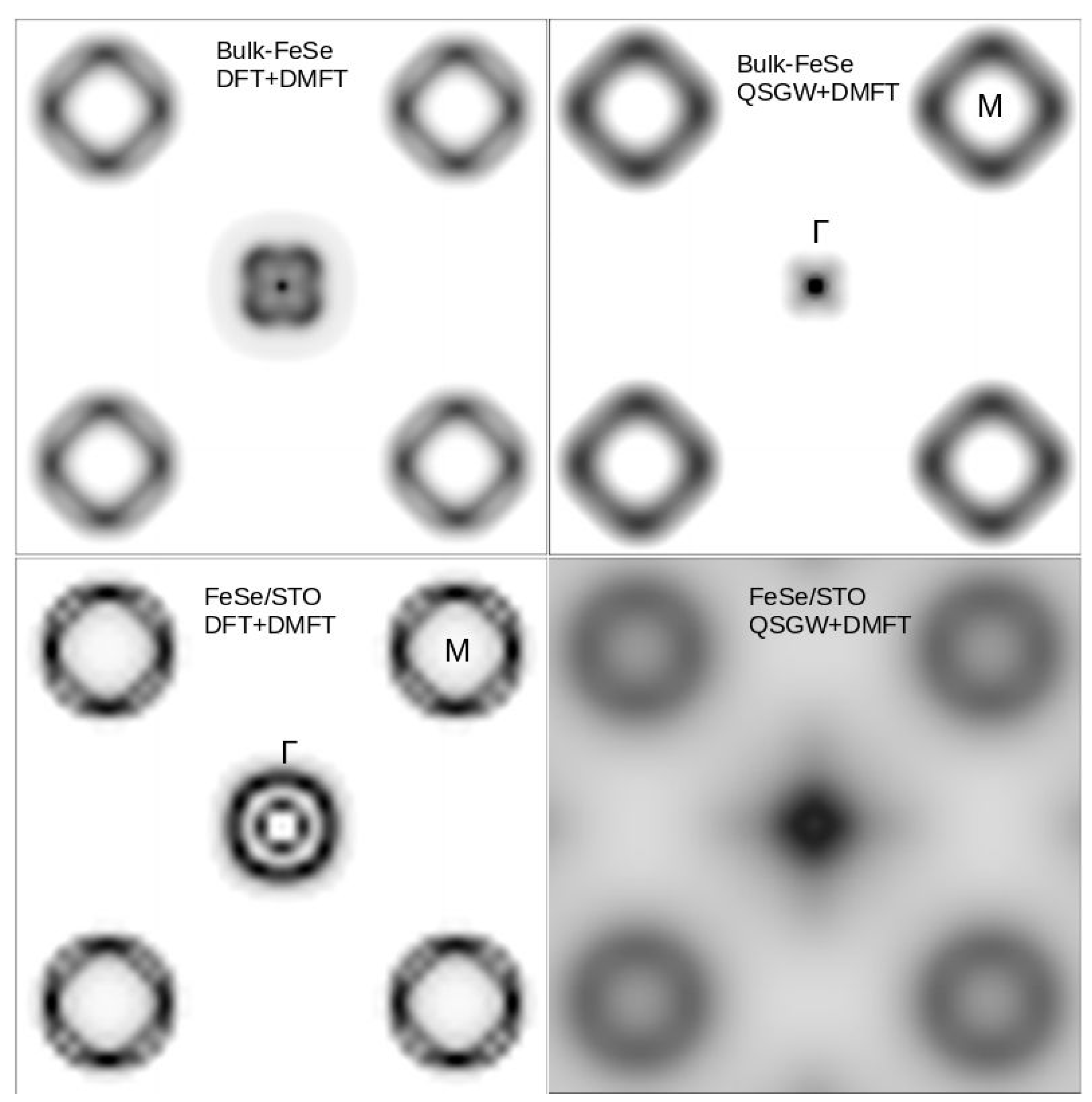
3.2. Fermi Surfaces and Spectral Functions
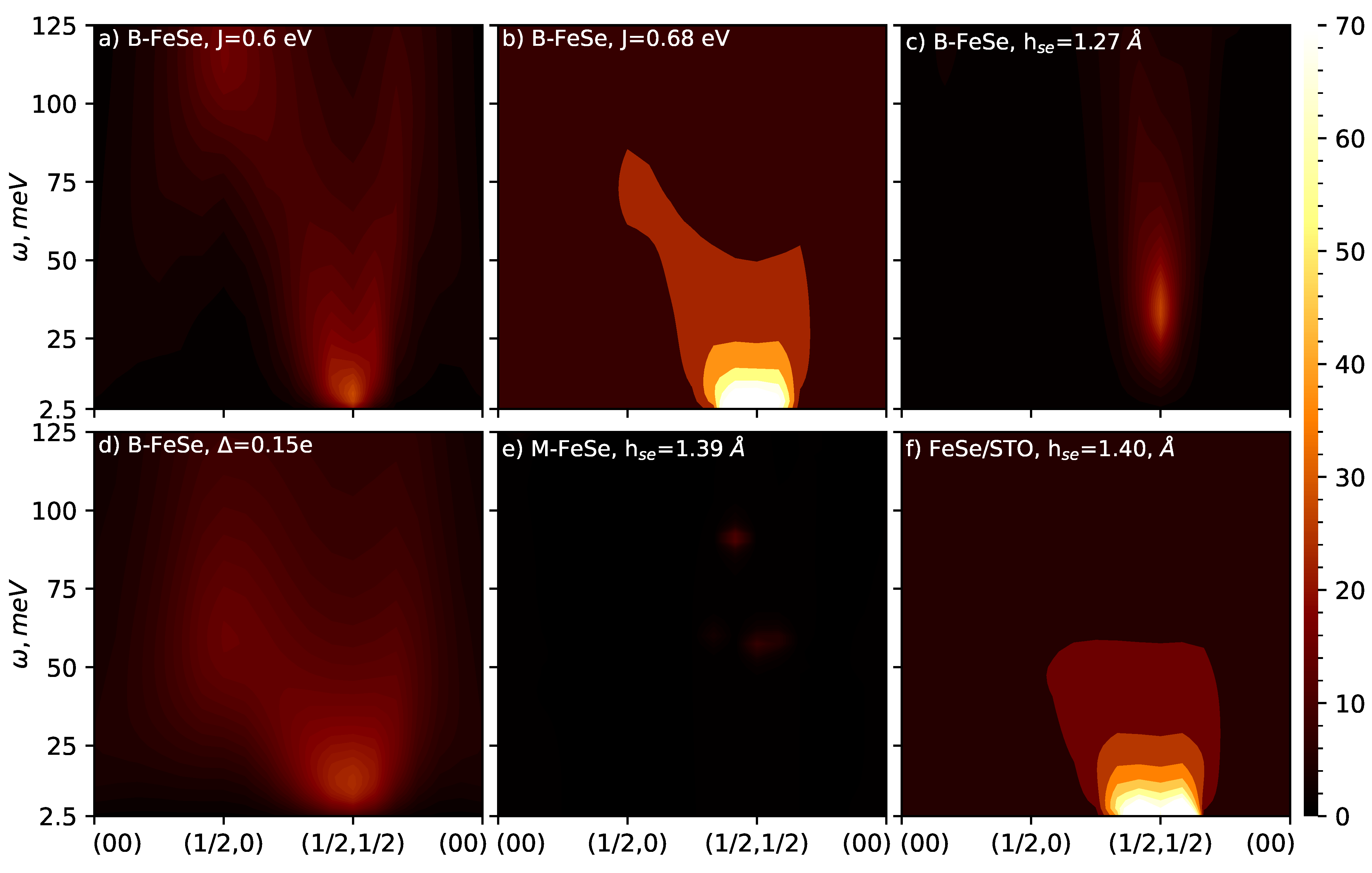
3.3. Bulk FeSe
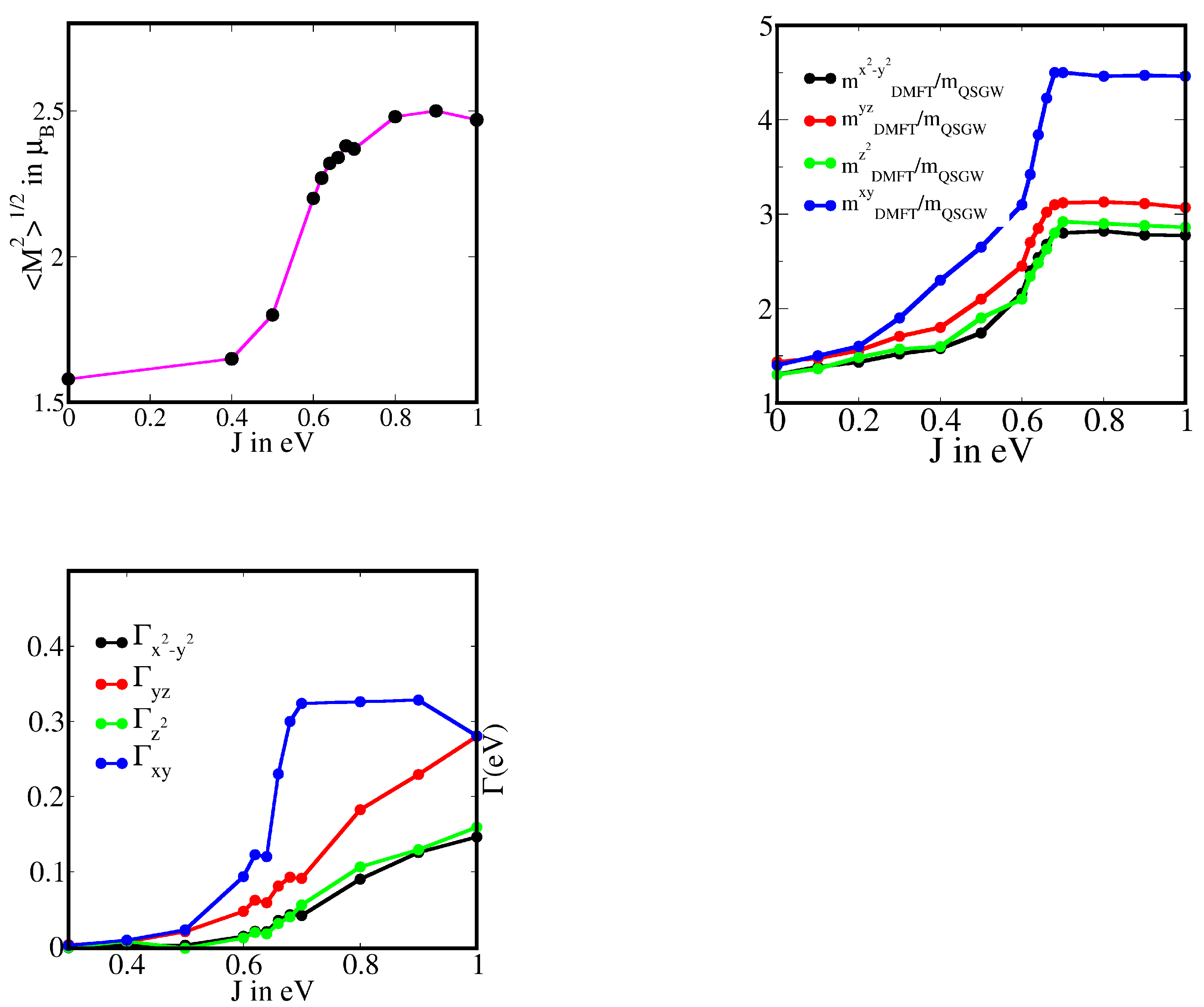
3.4. Excursion in Fe-Se bond length in Bulk FeSe
3.5. Free Standing Monolayer of FeSe
3.6. Monolayer of FeSe/SrTiO
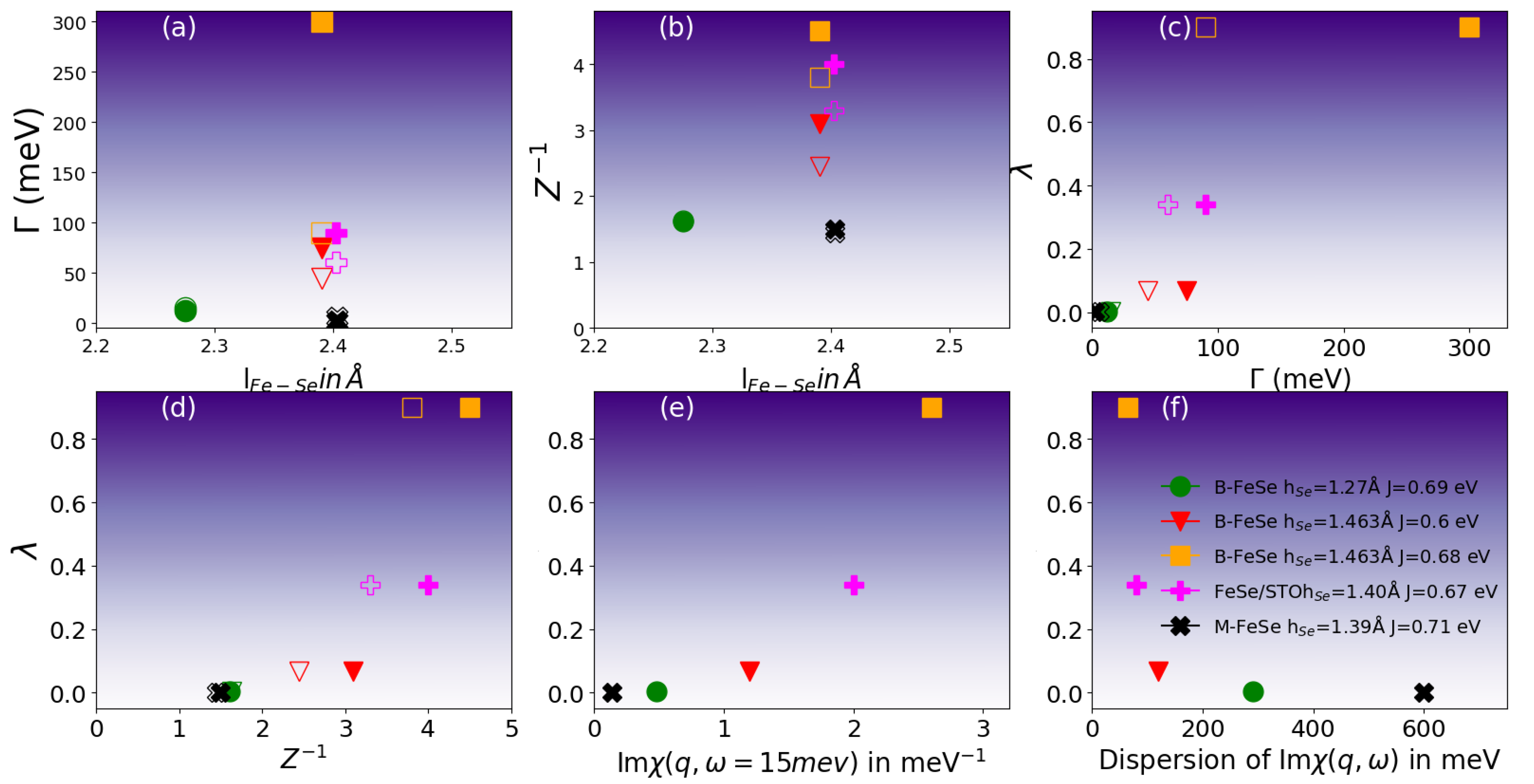
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Georges, A.; Medici, L.d.; Mravlje, J. Strong Correlations from Hund’s Coupling. Ann. Rev. Cond. Matt Phys. 2013, 4, 137–178. [Google Scholar] [CrossRef]
- Yin, Z.P.; Haule, K.; Kotliar, G. Fractional power-law behavior and its origin in iron-chalcogenide and ruthenate superconductors: Insights from first-principles calculations. Phys. Rev. B 2012, 86, 195141. [Google Scholar] [CrossRef]
- Lanata, N.; Strand, H.U.; Giovannetti, G.; Hellsing, B.; de’Medici, L.; Capone, M. Orbital selectivity in Hund’s metals: The iron chalcogenides. Phys. Rev. B 2013, 87, 045122. [Google Scholar] [CrossRef]
- Yin, Z.; Haule, K.; Kotliar, G. Kinetic frustration and the nature of the magnetic and paramagnetic states in iron pnictides and iron chalcogenides. Nat. Mater. 2011, 10, 932. [Google Scholar] [CrossRef] [PubMed]
- Kostin, A.; Sprau, P.O.; Kreisel, A.; Chong, Y.X.; Böhmer, A.E.; Canfield, P.C.; Hirschfeld, P.J.; Andersen, B.M.; Davis, J.S. Imaging orbital-selective quasiparticles in the Hund’s metal state of FeSe. Nat. Mater. 2018, 17, 869. [Google Scholar] [CrossRef] [PubMed]
- Yi, M.; Zhang, Y.; Shen, Z.X.; Lu, D. Role of the orbital degree of freedom in iron-based superconductors. Npj Quantum Mater. 2017, 2, 57. [Google Scholar] [CrossRef]
- Zwicknagl, G.; Yaresko, A.; Fulde, P. Microscopic description of origin of heavy quasiparticles in UPt 3. Phys. Rev. B 2002, 65, 081103. [Google Scholar] [CrossRef]
- Kądzielawa-Major, E.; Fidrysiak, M.; Kubiczek, P.; Spałek, J. Spin-triplet paired phases inside a ferromagnet induced by Hund’s rule coupling and electronic correlations: Application to UGe2. Phys. Rev. B 2018, 97, 224519. [Google Scholar] [CrossRef]
- Wang, Q.-Y.; Li, Z.; Zhang, W.-H.; Zhang, Z.-C.; Zhang, J.-S.; Li, W.; Ding, H.; Ou, Y.-B.; Deng, P.; Chang, K.; et al. Interface-induced high-temperature superconductivity in single unit-cell FeSe films on SrTiO3. Chin. Phys. Lett. 2012, 29, 037402. [Google Scholar] [CrossRef]
- Ge, J.-F.; Liu, Z.-L.; Liu, C.; Gao, C.-L.; Qian, D.; Xue, Q.-K.; Liu, Y.; Jia, J.-F. Superconductivity above 100 K in single-layer FeSe films on doped SrTiO3. Nat. Mater. 2015, 14, 285. [Google Scholar] [CrossRef]
- Sponza, L.; Pisanti, P.; Vishina, A.; Pashov, D.; Weber, C.; van Schilfgaarde, M.; Acharya, S.; Vidal, J.; Kotliar, G. Self-energies in Itinerant Magnets: A Focus on Fe and Ni. Phys. Rev. B 2017, 95, 041112. [Google Scholar] [CrossRef]
- Pashov, D.; Acharya, S.; Lambrecht, W.R.L.; Jackson, J.; Belashchenko, K.D.; Chantis, A.; Jamet, F.; van Schilfgaarde, M. Questaal: A package of electronic structure methods based on the linear muffin-tin orbital technique. Comput. Phys. Commun. 2019. [Google Scholar] [CrossRef]
- Kotani, T.; van Schilfgaarde, M.; Faleev, S.V. Quasiparticle self-consistent GW method: A basis for the independent-particle approximation. Phys. Rev. B 2007, 76, 165106. [Google Scholar] [CrossRef]
- Haule, K. Quantum Monte Carlo impurity solver for cluster dynamical mean-field theory and electronic structure calculations with adjustable cluster base. Phys. Rev. B 2007, 75, 155113. [Google Scholar] [CrossRef]
- Gull, E.; Millis, A.J.; Lichtenstein, A.I.; Rubtsov, A.N.; Troyer, M.; Werner, P. Continuous-time Monte Carlo methods for quantum impurity models. Rev. Mod. Phys. 2011, 83, 349. [Google Scholar] [CrossRef]
- Georges, A.; Kotliar, G.; Krauth, W.; Rozenberg, M.J. Dynamical mean-field theory of strongly correlated fermion systems and the limit of infinite dimensions. Rev. Mod. Phys. 1996, 68, 13. [Google Scholar] [CrossRef]
- Acharya, S.; Weber, C.; Plekhanov, E.; Pashov, D.; Taraphder, A.; van Schilfgaarde, M. Metal-Insulator Transition in Copper Oxides Induced by Apex Displacements. Phys. Rev. X 2018, 8, 021038. [Google Scholar] [CrossRef]
- Baldini, E.; Sentef, M.A.; Acharya, S.; Brumme, T.; Sheveleva, E.; Lyzwa, F.; Pomjakushina, E.; Bernhard, C.; van Schilfgaarde, M.; Carbone, F.; et al. Electron–phonon-driven three-dimensional metallicity in an insulating cuprate. Proc. Natl. Acad. Sci. USA 2020, 117, 6409–6416. [Google Scholar] [CrossRef]
- Park, H. The Study of Two-Particle Response Functions in Strongly Correlated Electron Systems within the Dynamical Mean Field Theory. Ph.D. Thesis, Rutgers University-Graduate School, New Brunswick, NJ, USA, 2011. [Google Scholar]
- Yin, Z.; Haule, K.; Kotliar, G. Spin dynamics and orbital-antiphase pairing symmetry in iron-based superconductors. Nat. Phys. 2014, 10, 845. [Google Scholar] [CrossRef]
- Acharya, S.; Pashov, D.; Weber, C.; Park, H.; Sponza, L.; van Schilfgaarde, M. Maximization of Tc via Conspired Even-Parity Spin and Charge Collective Excitations in Strained Sr2RuO4. Commun. Phys. 2019, 2. [Google Scholar] [CrossRef]
- Acharya, S.; Pashov, D.; Jamet, F.; van Schilfgaarde, M. Controlling Tc through Band Structure and Correlation Engineering in Collapsed and Uncollapsed Phases of Iron Arsenides. Phys. Rev. Lett. 2020, 124, 237001. [Google Scholar] [CrossRef] [PubMed]
- Boehnke, L.; Hafermann, H.; Ferrero, M.; Lechermann, F.; Parcollet, O. Orthogonal polynomial representation of imaginary-time Green’s functions. Phys. Rev. B 2011, 84, 075145. [Google Scholar] [CrossRef]
- Tomczak, J.M.; Liu, P.; Toschi, A.; Kresse, G.; Held, K. Merging GW with DMFT and non-local correlations beyond. Eur. Phys. J. Spec. Top. 2017, 226, 2565–2590. [Google Scholar] [CrossRef]
- Aryasetiawan, F.; Imada, M.; Georges, A.; Kotliar, G.; Biermann, S.; Lichtenstein, A. Frequency-dependent local interactions and low-energy effective models from electronic structure calculations. Phys. Rev. B 2004, 70, 195104. [Google Scholar] [CrossRef]
- Şaşıoğlu, E.; Friedrich, C.; Blügel, S. Effective Coulomb interaction in transition metals from constrained random-phase approximation. Phys. Rev. B 2011, 83, 121101. [Google Scholar] [CrossRef]
- Acharya, S.; Pashov, D.; van Schilfgaarde, M. Role of nematicity in controlling spin fluctuations and superconducting Tc in bulk FeSe. arXiv 2020, arXiv:2005.07729. [Google Scholar]
- Wang, Q.; Shen, Y.; Pan, B.; Zhang, X.; Ikeuchi, K.; Iida, K.; Christianson, A.D.; Walker, H.C.; Adroja, D.T.; Abdel-Hafiez, M.; et al. Magnetic ground state of FeSe. Nat. Commun. 2016, 7, 12182. [Google Scholar] [CrossRef]
- Rahn, M.C.; Ewings, R.A.; Sedlmaier, S.J.; Clarke, S.J.; Boothroyd, A.T. Strong (π,0) spin fluctuations in β-FeSe observed by neutron spectroscopy. Phys. Rev. B 2015, 91, 180501. [Google Scholar] [CrossRef]
- Deng, X.; Haule, K.; Kotliar, G. Transport Properties of Metallic Ruthenates: A DFT+DMFT Investigation. Phys. Rev. Lett. 2016, 116. [Google Scholar] [CrossRef]
- Acharya, S.; Pashov, D.; Chachkarova, E.; van Schilfgaarde, M.; Weber, C. Electronic structure correspondence of singlet-triplet scale separation in strained Sr2RuO4. Appl. Sci. 2021, 11, 508. [Google Scholar] [CrossRef]
- Hirsch, J. BCS theory of superconductivity: It is time to question its validity. Phys. Scr. 2009, 80, 035702. [Google Scholar] [CrossRef]
- Hirsch, J. Inconsistency of the conventional theory of superconductivity. EPL Europhys. Lett. 2020, 130, 17006. [Google Scholar] [CrossRef]
- Koizumi, H. Reversible superconducting-normal phase transition in a magnetic field and the existence of topologically protected loop currents that appear and disappear without Joule heating. EPL Europhys. Lett. 2020, 131, 37001. [Google Scholar] [CrossRef]
- Koizumi, H.; Ishikawa, A. Theory of supercurrent in superconductors. Int. J. Mod. Phys. B 2020, 34, 2030001. [Google Scholar] [CrossRef]
- Koizumi, H. Explanation of Superfluidity Using the Berry Connection for Many-Body Wave Functions. J. Supercond. Nov. Magn. 2020, 33, 1697–1707. [Google Scholar] [CrossRef]
- Kumar, R.S.; Zhang, Y.; Sinogeikin, S.; Xiao, Y.; Kumar, S.; Chow, P.; Cornelius, A.L.; Chen, C. Crystal and electronic structure of FeSe at high pressure and low temperature. J. Phys. Chem. B 2010, 114, 12597–12606. [Google Scholar] [CrossRef]
- Mandal, S.; Zhang, P.; Ismail-Beigi, S.; Haule, K. How Correlated is the FeSe/SrTiO3 System? Phys. Rev. Lett. 2017, 119, 067004. [Google Scholar] [CrossRef]
- Tomczak, J.M.; van Schilfgaarde, M.; Kotliar, G. Many-body effects in iron pnictides and chalcogenides – non-local vs dynamic origin of effective masses. Phys. Rev. Lett. 2012, 109, 237010. [Google Scholar] [CrossRef]
- Watson, M.D.; Kim, T.K.; Rhodes, L.C.; Eschrig, M.; Hoesch, M.; Haghighirad, A.A.; Coldea, A.I. Evidence for unidirectional nematic bond ordering in FeSe. Phys. Rev. B 2016, 94, 201107. [Google Scholar] [CrossRef]
- Liu, X.; Zhao, L.; He, S.; He, J.; Liu, D.; Mou, D.; Shen, B.; Hu, Y.; Huang, J.; Zhou, X. Electronic structure and superconductivity of FeSe-related superconductors. J. Physics: Condens. Matter 2015, 27, 183201. [Google Scholar] [CrossRef]
- Rhodes, L.; Watson, M.; Haghighirad, A.; Eschrig, M.; Kim, T. Strongly enhanced temperature dependence of the chemical potential in FeSe. Phys. Rev. B 2017, 95, 195111. [Google Scholar] [CrossRef]
- Han, Q.; Dang, H.T.; Millis, A. Ferromagnetism and correlation strength in cubic barium ruthenate in comparison to strontium and calcium ruthenate: A dynamical mean-field study. Phys. Rev. B 2016, 93, 155103. [Google Scholar] [CrossRef]
- Gretarsson, H.; Lupascu, A.; Kim, J.; Casa, D.; Gog, T.; Wu, W.; Julian, S.; Xu, Z.; Wen, J.; Gu, G.; et al. Revealing the dual nature of magnetism in iron pnictides and iron chalcogenides using x-ray emission spectroscopy. Phys. Rev. B 2011, 84, 100509. [Google Scholar] [CrossRef]
- Dasari, N.; Yamijala, S.R.K.C.S.; Jain, M.; Dasgupta, T.S.; Moreno, J.; Jarrell, M.; Vidhyadhiraja, N.S. First-principles investigation of cubic BaRuO3: A Hund’s metal. Phys. Rev. B 2016, 94, 085143. [Google Scholar] [CrossRef]
- Nica, E.M.; Yu, R.; Si, Q. Orbital-selective pairing and superconductivity in iron selenides. Npj Quantum Mater. 2017, 2, 24. [Google Scholar] [CrossRef]
- Lynn, J.W.; Dai, P. Neutron studies of the iron-based family of high Tc magnetic superconductors. Phys. C Supercond. 2009, 469, 469–476. [Google Scholar] [CrossRef][Green Version]
- Kreisel, A.; Hirschfeld, P.J.; Andersen, B.M. On the Remarkable Superconductivity of FeSe and Its Close Cousins. Symmetry 2020, 12, 1402. [Google Scholar] [CrossRef]
- Iyo, A.; Ishida, S.; Fujihisa, H.; Gotoh, Y.; Hase, I.; Yoshida, Y.; Eisaki, H.; Kawashima, K. Superconductivity in Uncollapsed Tetragonal LaFe2As2. J. Phys. Chem. Lett. 2019, 10, 1018–1023. [Google Scholar] [CrossRef]
- Haule, K.; Pascut, G.L. Forces for structural optimizations in correlated materials within a DFT+embedded DMFT functional approach. Phys. Rev. B 2016, 94, 195146. [Google Scholar] [CrossRef]
- Xiang, Y.Y.; Wang, F.; Wang, D.; Wang, Q.H.; Lee, D.H. High-temperature superconductivity at the FeSe/SrTiO3 interface. Phys. Rev. B 2012, 86, 134508. [Google Scholar] [CrossRef]
- Li, B.; Xing, Z.W.; Huang, G.Q.; Xing, D.Y. Electron-phonon coupling enhanced by the FeSe/SrTiO3 interface. J. Appl. Phys. 2014, 115, 193907. [Google Scholar] [CrossRef]
- Lee, J.J.; Schmitt, F.T.; Moore, R.G.; Johnston, S.; Cui, Y.T.; Li, W.; Yi, M.; Liu, Z.K.; Hashimoto, M.; Zhang, Y.; et al. Interfacial mode coupling as the origin of the enhancement of Tc in FeSe films on SrTiO3. Nat. Vol. 2014, 515, 245. [Google Scholar] [CrossRef] [PubMed]
- Li, F.; Sawatzky, G.A. Electron Phonon Coupling versus Photoelectron Energy Loss at the Origin of Replica Bands in Photoemission of FeSe on SrTiO3. Phys. Rev. Lett. 2018, 120, 237001. [Google Scholar] [CrossRef] [PubMed]
- Liu, D.; Zhang, W.; Mou, D.; He, J.; Ou, Y.-B.; Wang, Q.-Y.; Li, Z.; Wang, L.; Zhao, L.; He, S.K.; et al. Electronic origin of high-temperature superconductivity in single-layer FeSe superconductor. Nat. Commun. 2012, 3, 1–6. [Google Scholar] [CrossRef]
- Zeng, B.; Mu, G.; Luo, H.; Xiang, T.; Mazin, I.; Yang, H.; Shan, L.; Ren, C.; Dai, P.; Wen, H.H. Anisotropic structure of the order parameter in FeSe 0.45 Te 0.55 revealed by angle-resolved specific heat. Nat. Commun. 2010, 1, 112. [Google Scholar] [CrossRef]
- Hanaguri, T.; Niitaka, S.; Kuroki, K.; Takagi, H. Unconventional s-wave superconductivity in Fe (Se, Te). Science 2010, 328, 474–476. [Google Scholar] [CrossRef]
- Nourafkan, R.; Kotliar, G.; Tremblay, A.M.S. Correlation-Enhanced Odd-Parity Interorbital Singlet Pairing in the Iron-Pnictide Superconductor LiFeAs. Phys. Rev. Lett. 2016, 117, 137001. [Google Scholar] [CrossRef]
- Zhang, Y.; Lee, J.; Moore, R.; Li, W.; Yi, M.; Hashimoto, M.; Lu, D.; Devereaux, T.; Lee, D.H.; Shen, Z.-X. Superconducting Gap Anisotropy in Monolayer FeSe Thin Film. Phys. Rev. Lett. 2016, 117, 117001. [Google Scholar] [CrossRef]
- Ye, Z.R.; Zhang, C.F.; Ning, H.L.; Li, W.; Chen, L.; Jia, T.; Hashimoto, M.; Lu, D.-H.; Shen, Z.-X.; Zhang, Y. Simultaneous emergence of superconductivity, inter-pocket scattering and nematic fluctuation in potassium-coated FeSe superconductor. arXiv 2015, arXiv:1512.02526. [Google Scholar]
- Schrodi, F.; Aperis, A.; Oppeneer, P.M. Eliashberg theory for spin fluctuation mediated superconductivity: Application to bulk and monolayer FeSe. Phys. Rev. B 2020, 102, 014502. [Google Scholar] [CrossRef]

| Variants | a (Å) | c (Å) | (A) | U (eV) | J (eV) | d (eV) | d (eV) |
|---|---|---|---|---|---|---|---|
| B-FeSe [37] | 3.779 | 5.5111 | 1.463 | 3.4 | 0.60 | 2.5 | 3.3 |
| B-FeSe (reduced h) | 3.779 | 5.511 | 1.27 | 3.9 | 0.69 | 3.1 | 4.3 |
| M-FeSe [38] | 3.905 | 1.39 | 4.3 | 0.71 | 2.4 | 3.2 | |
| M-FeSe/STO [38] | 3.905 | 1.40 | 3.8 | 0.67 | 2.35 | 2.95 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Acharya, S.; Pashov, D.; Jamet, F.; van Schilfgaarde, M. Electronic Origin of Tc in Bulk and Monolayer FeSe. Symmetry 2021, 13, 169. https://doi.org/10.3390/sym13020169
Acharya S, Pashov D, Jamet F, van Schilfgaarde M. Electronic Origin of Tc in Bulk and Monolayer FeSe. Symmetry. 2021; 13(2):169. https://doi.org/10.3390/sym13020169
Chicago/Turabian StyleAcharya, Swagata, Dimitar Pashov, Francois Jamet, and Mark van Schilfgaarde. 2021. "Electronic Origin of Tc in Bulk and Monolayer FeSe" Symmetry 13, no. 2: 169. https://doi.org/10.3390/sym13020169
APA StyleAcharya, S., Pashov, D., Jamet, F., & van Schilfgaarde, M. (2021). Electronic Origin of Tc in Bulk and Monolayer FeSe. Symmetry, 13(2), 169. https://doi.org/10.3390/sym13020169







