Symmetry Energy and the Pauli Exclusion Principle
Abstract
1. Introduction
1.1. Antecedents
1.2. Quantum Caveats
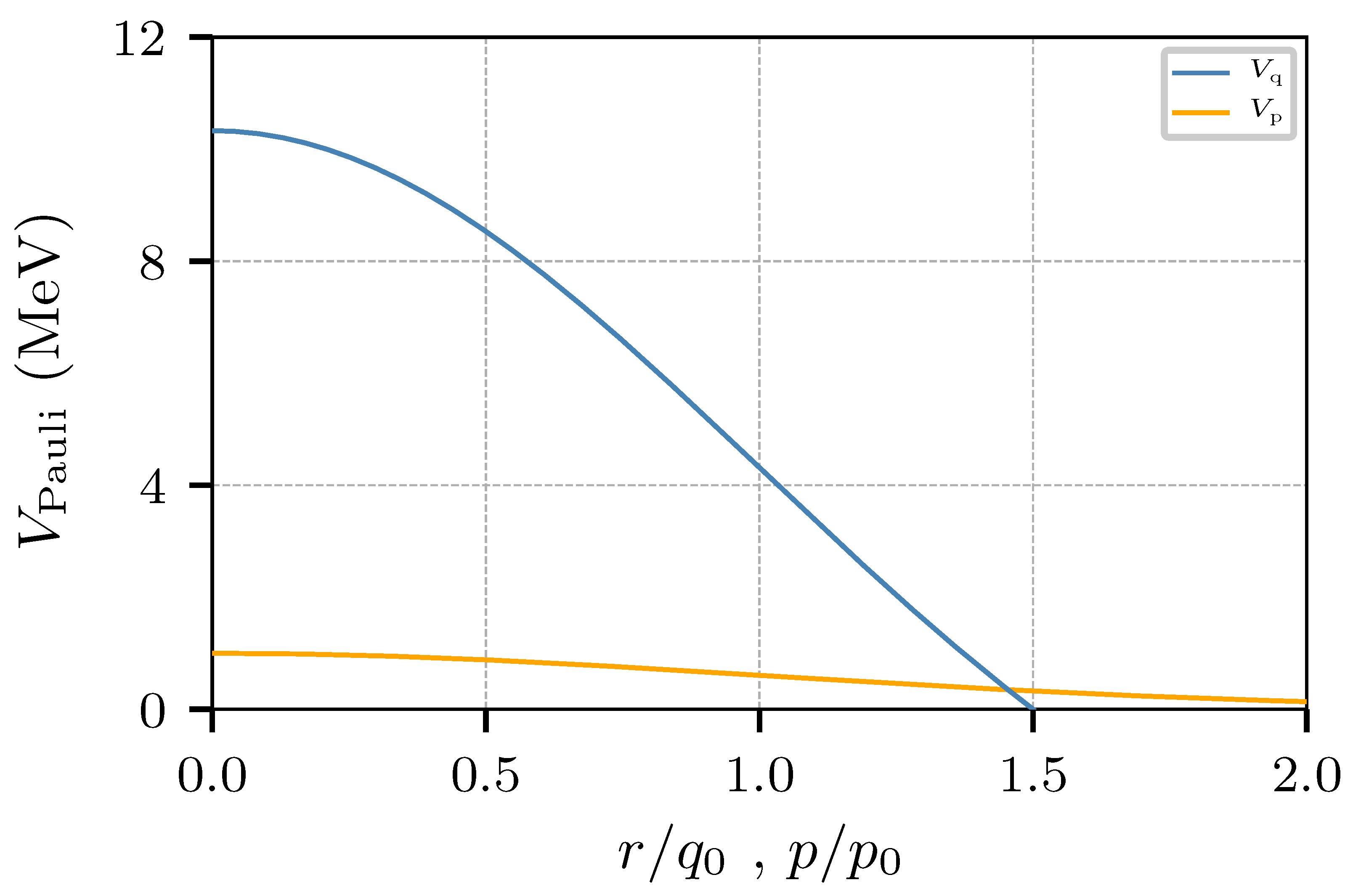
1.3. Pauli Blocking
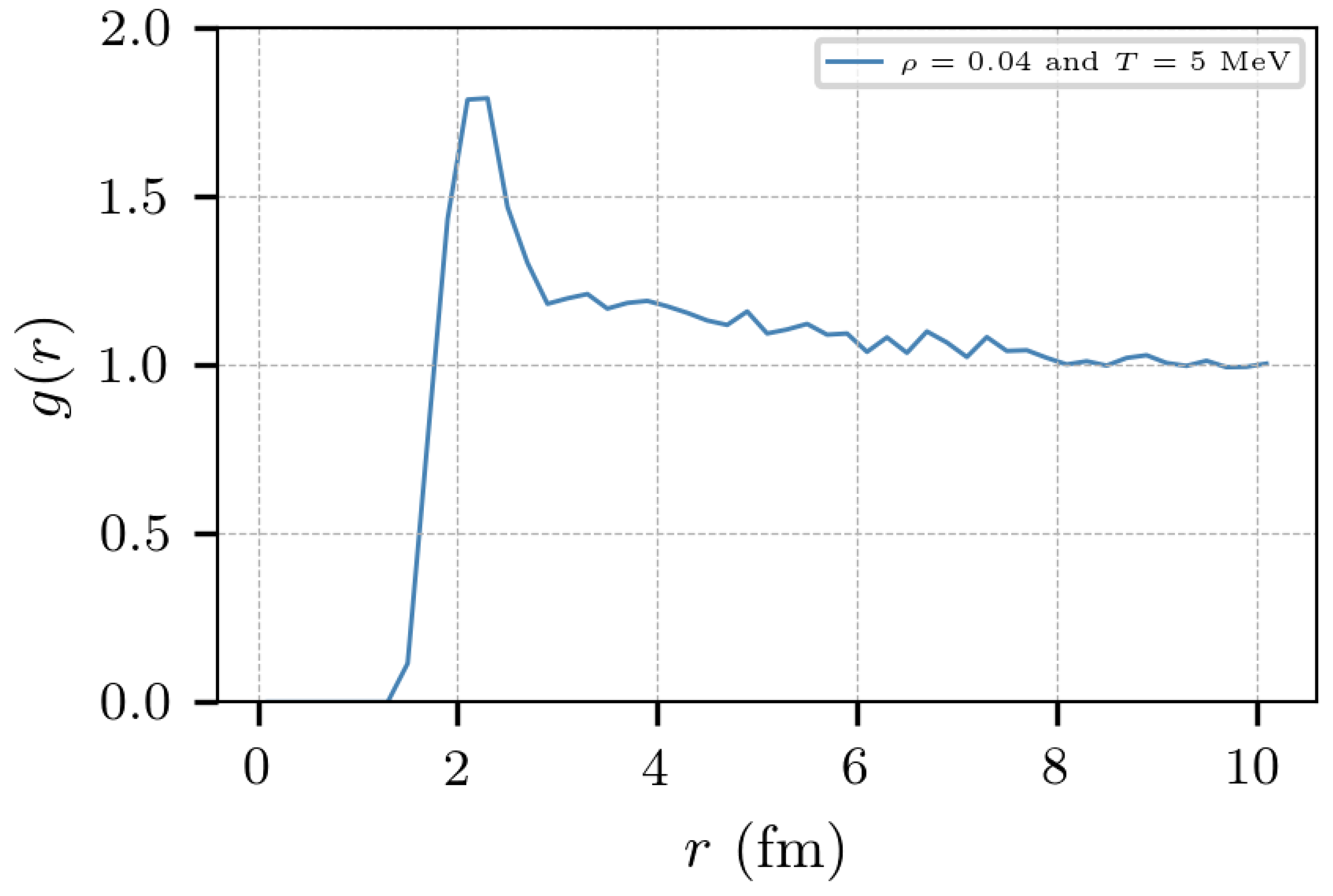
2. The Model
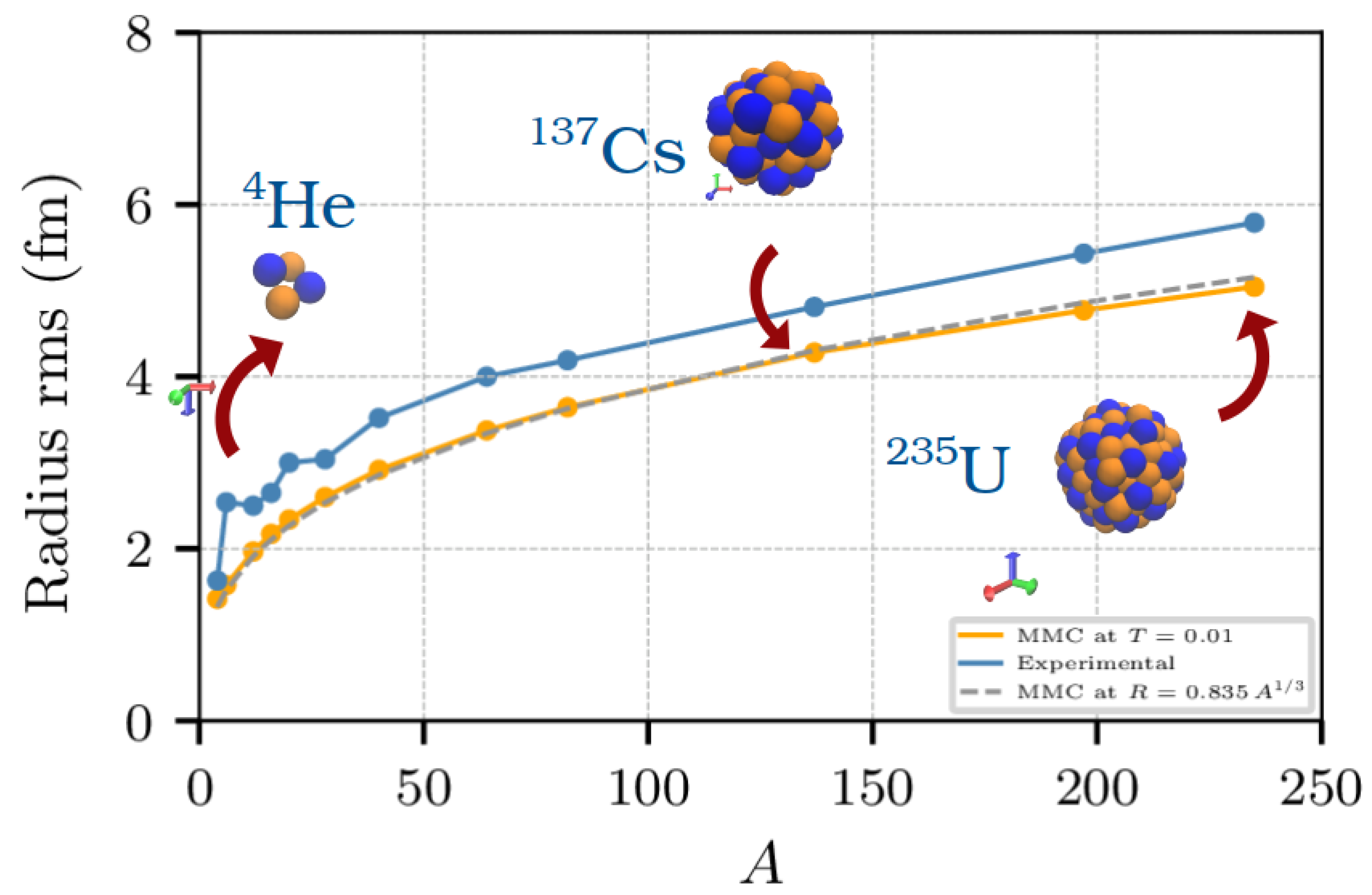
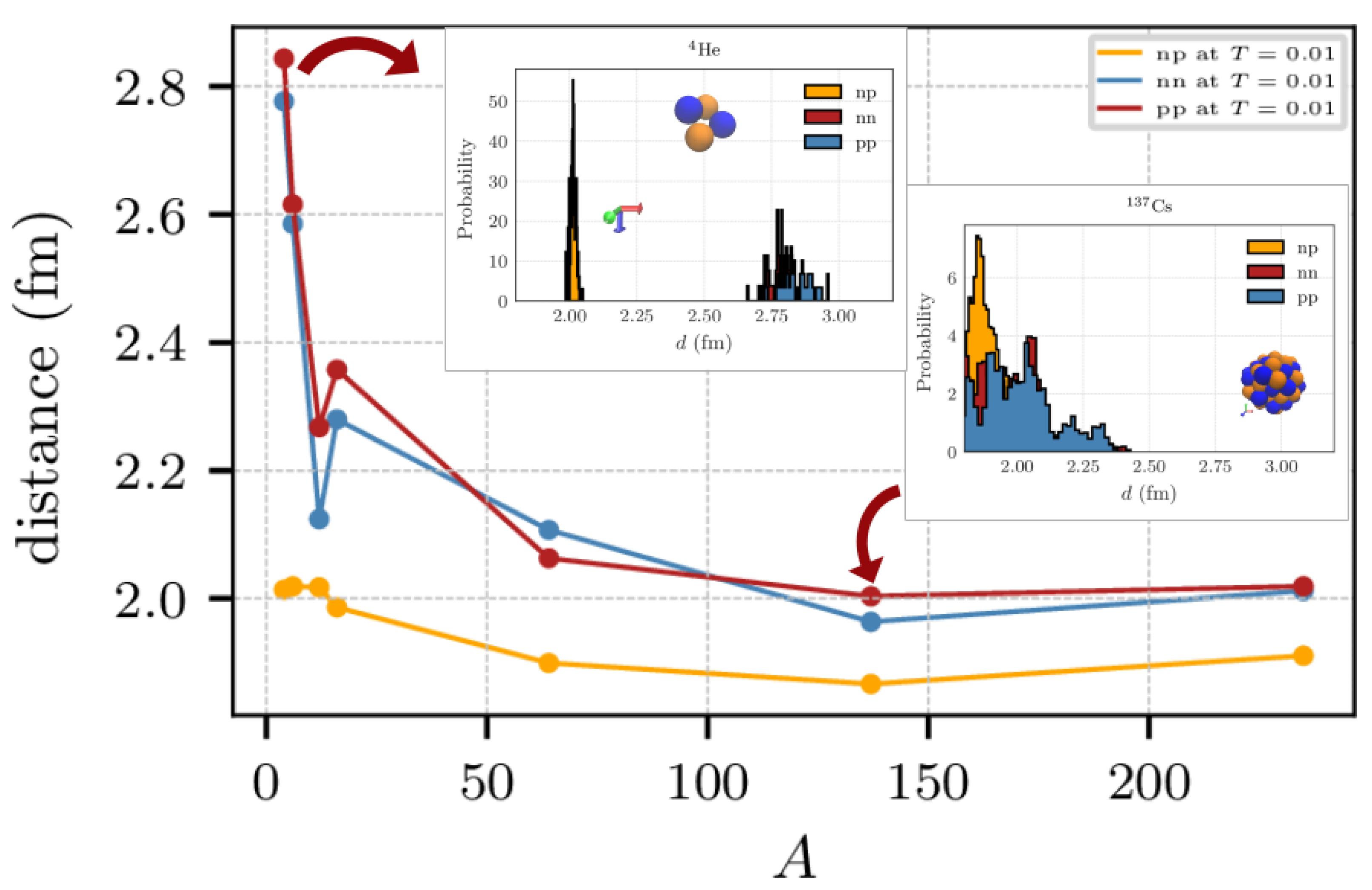
3. Finite Nuclei
4. Infinite Nuclear Matter
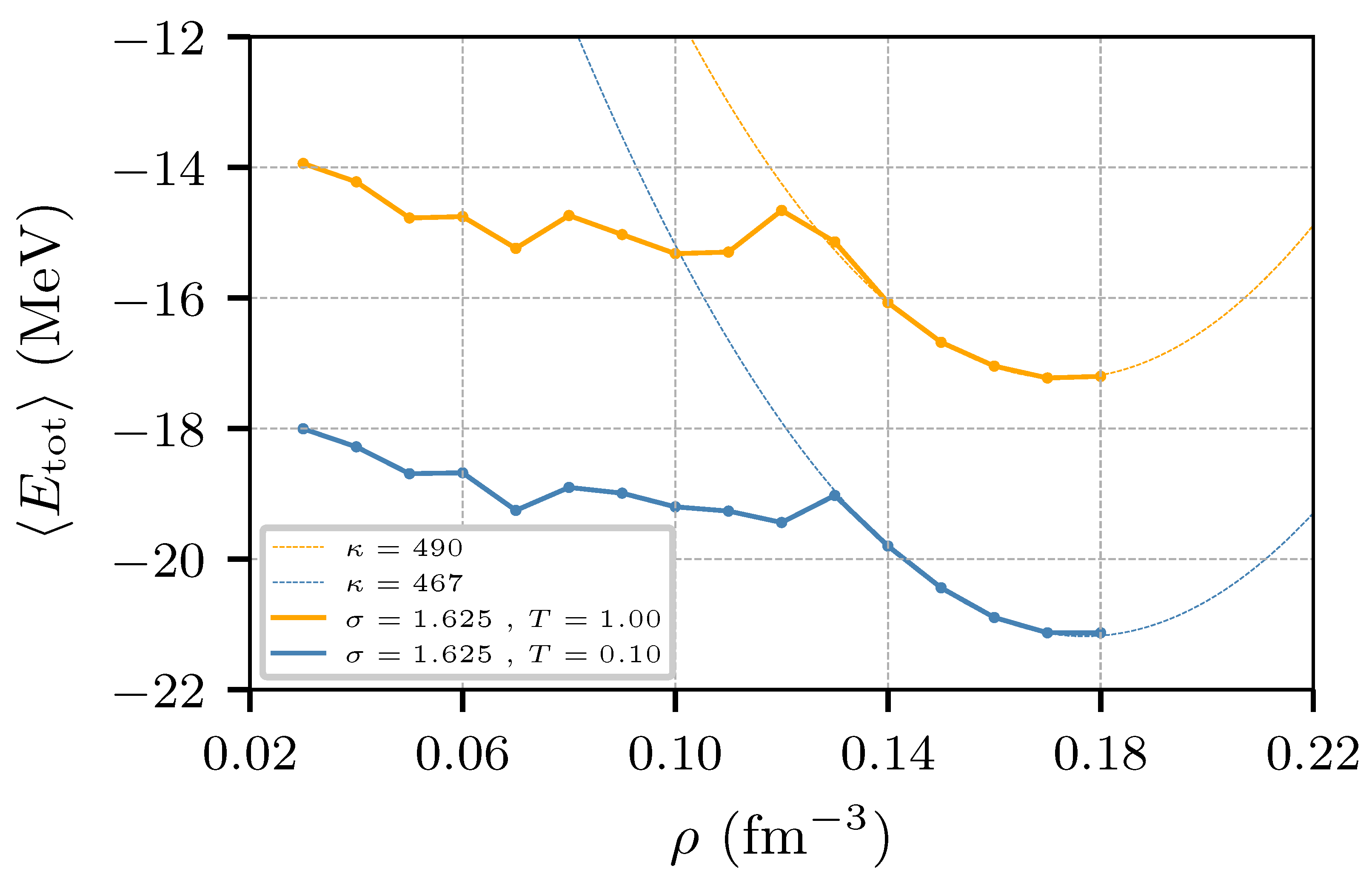
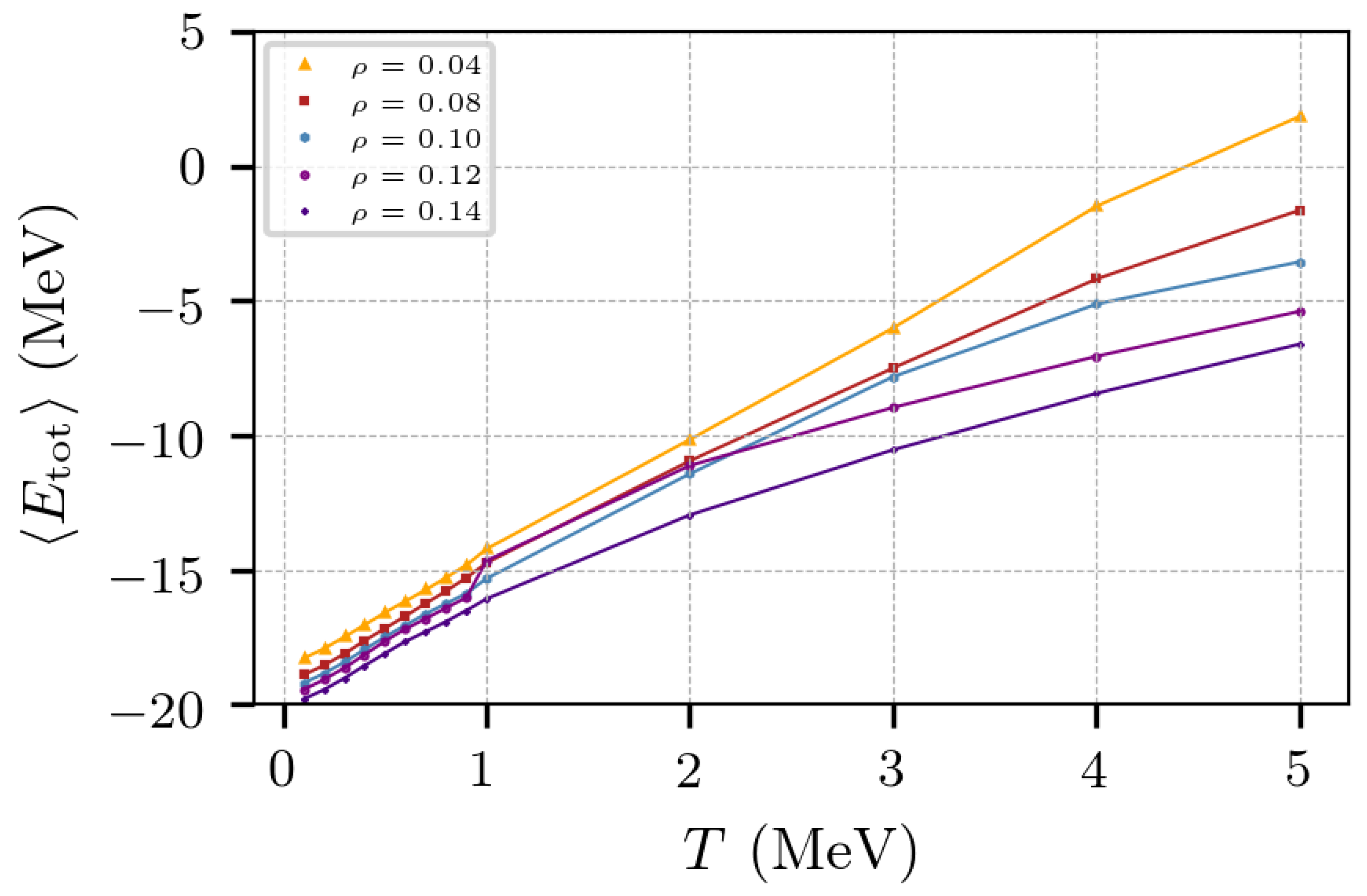
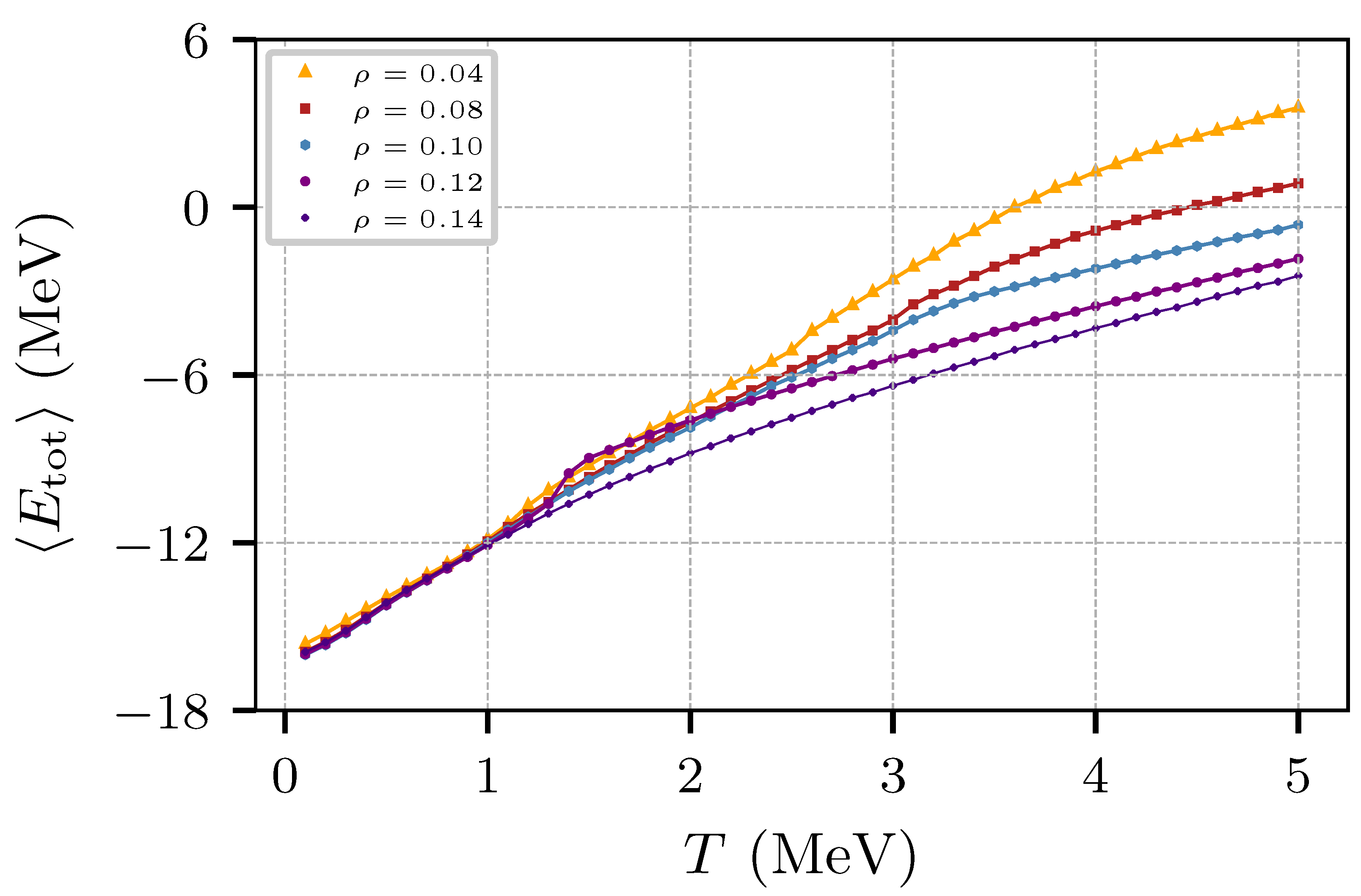
4.1. Nuclear Matter
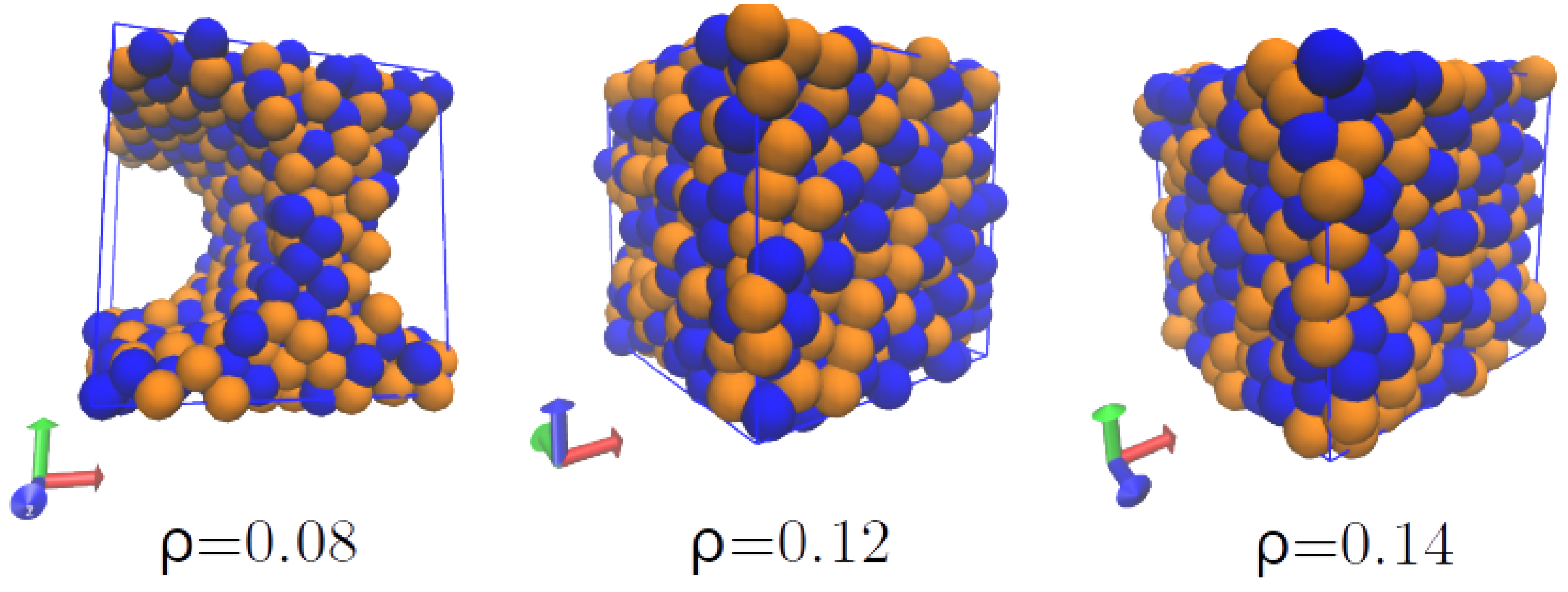
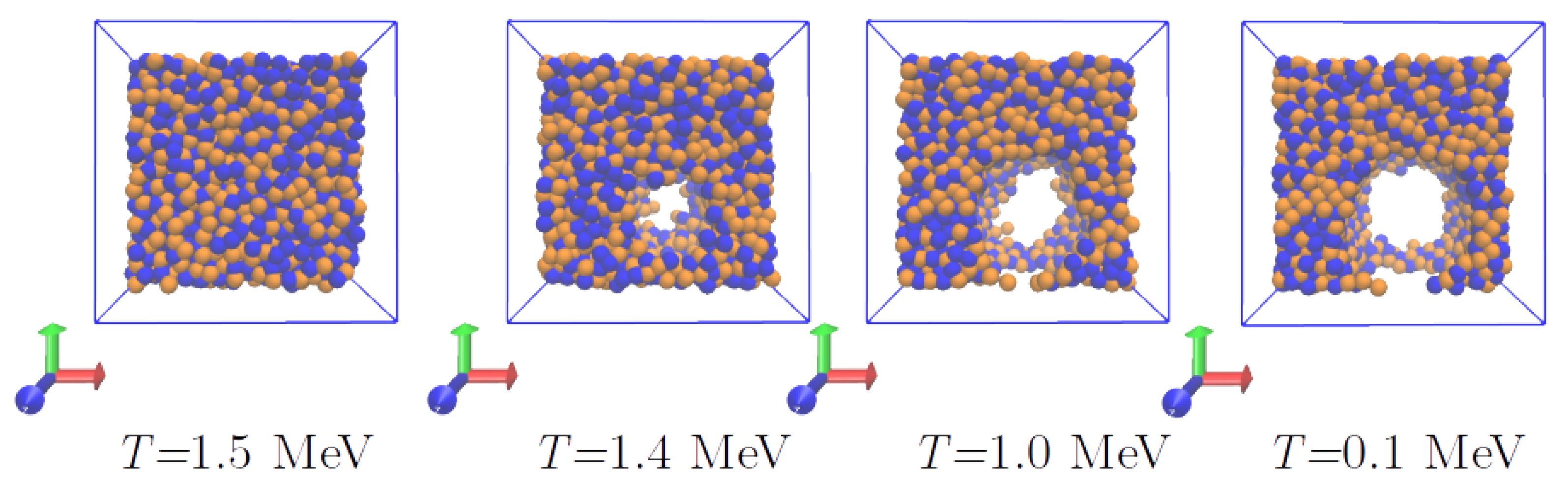
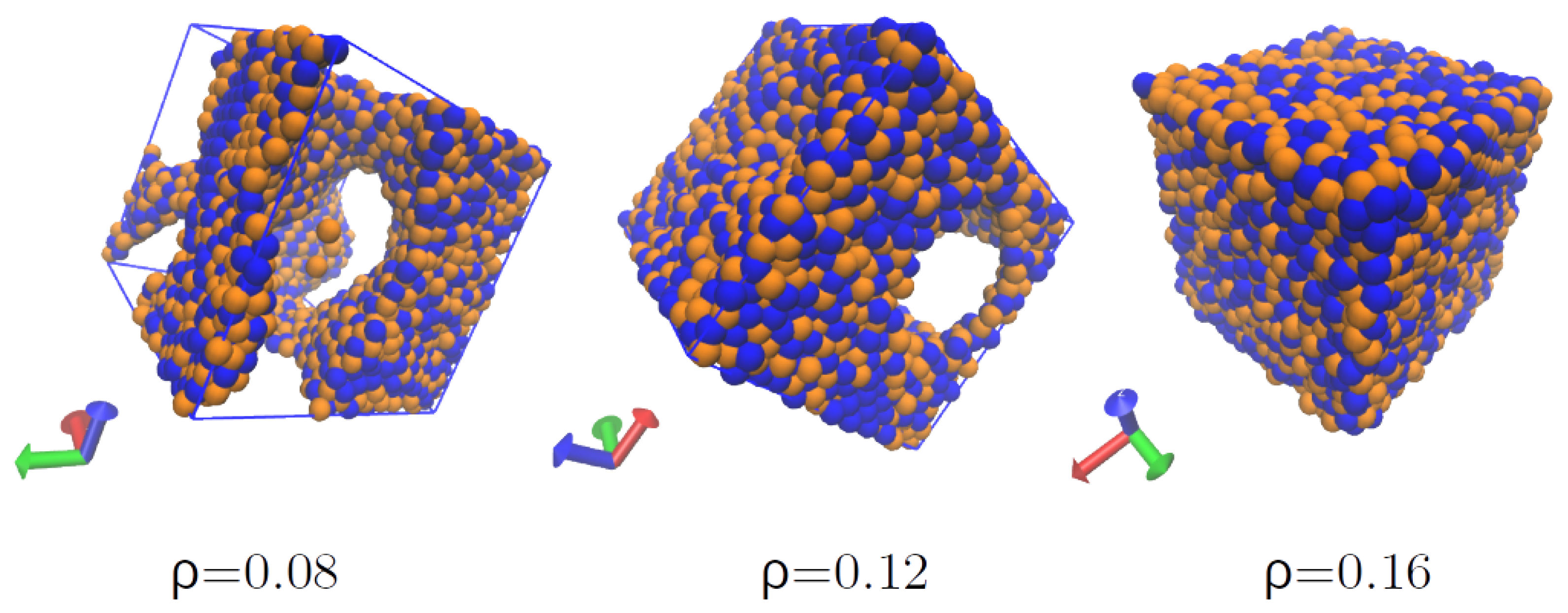
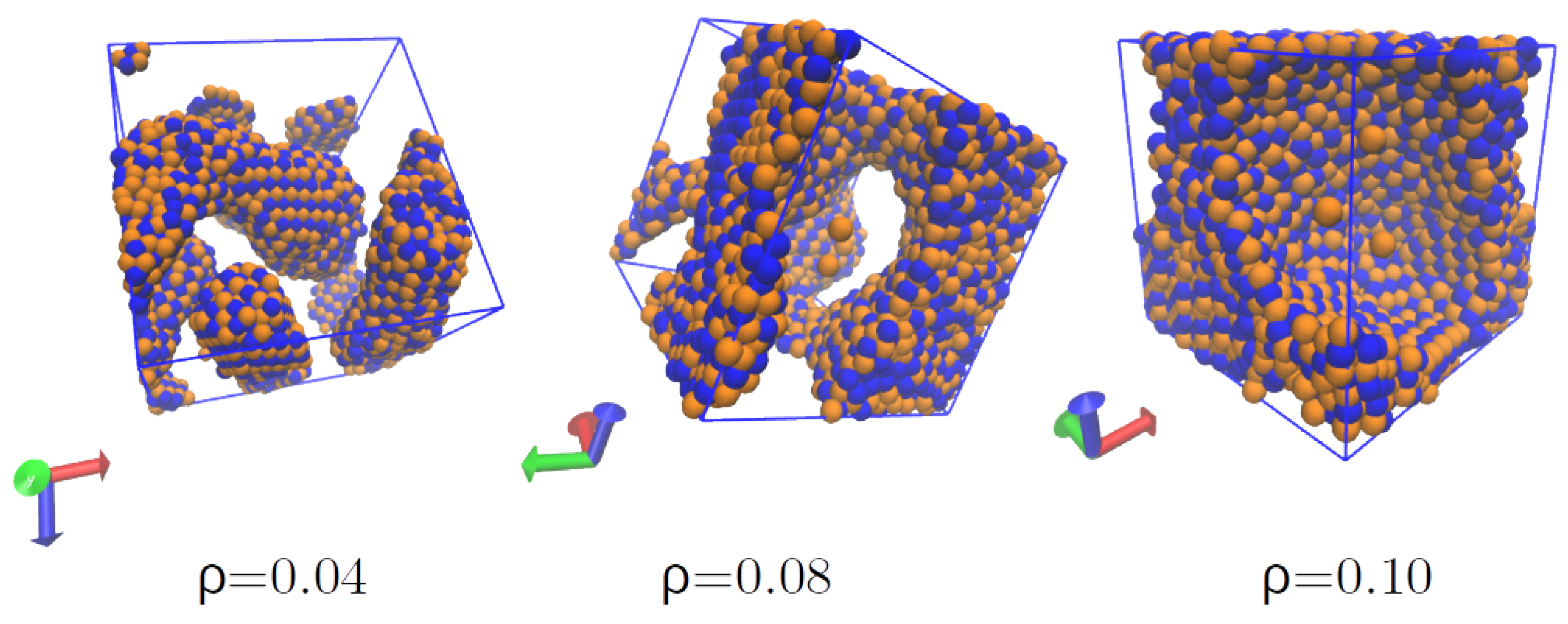
Pastas of Nuclear Matter
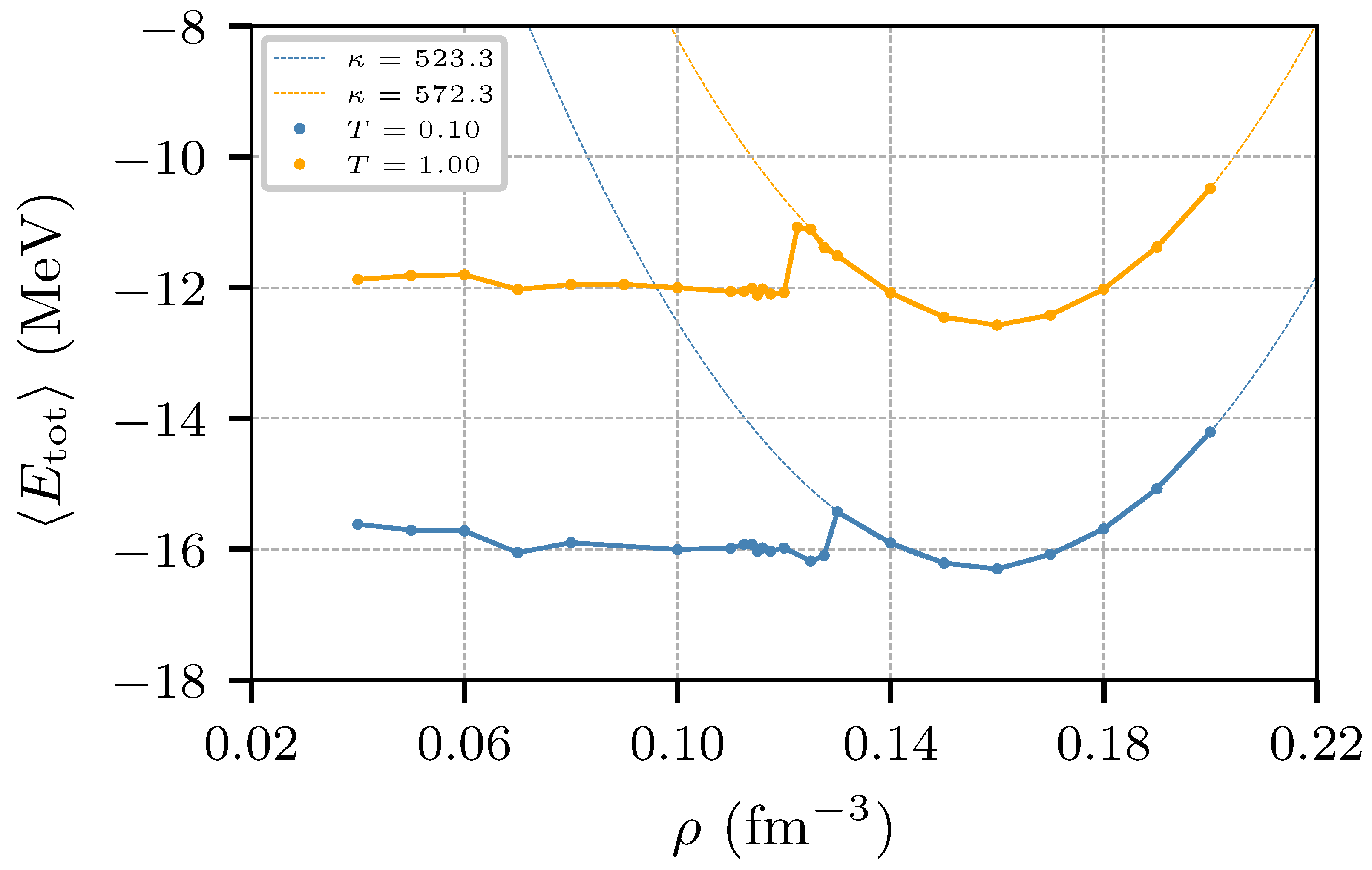
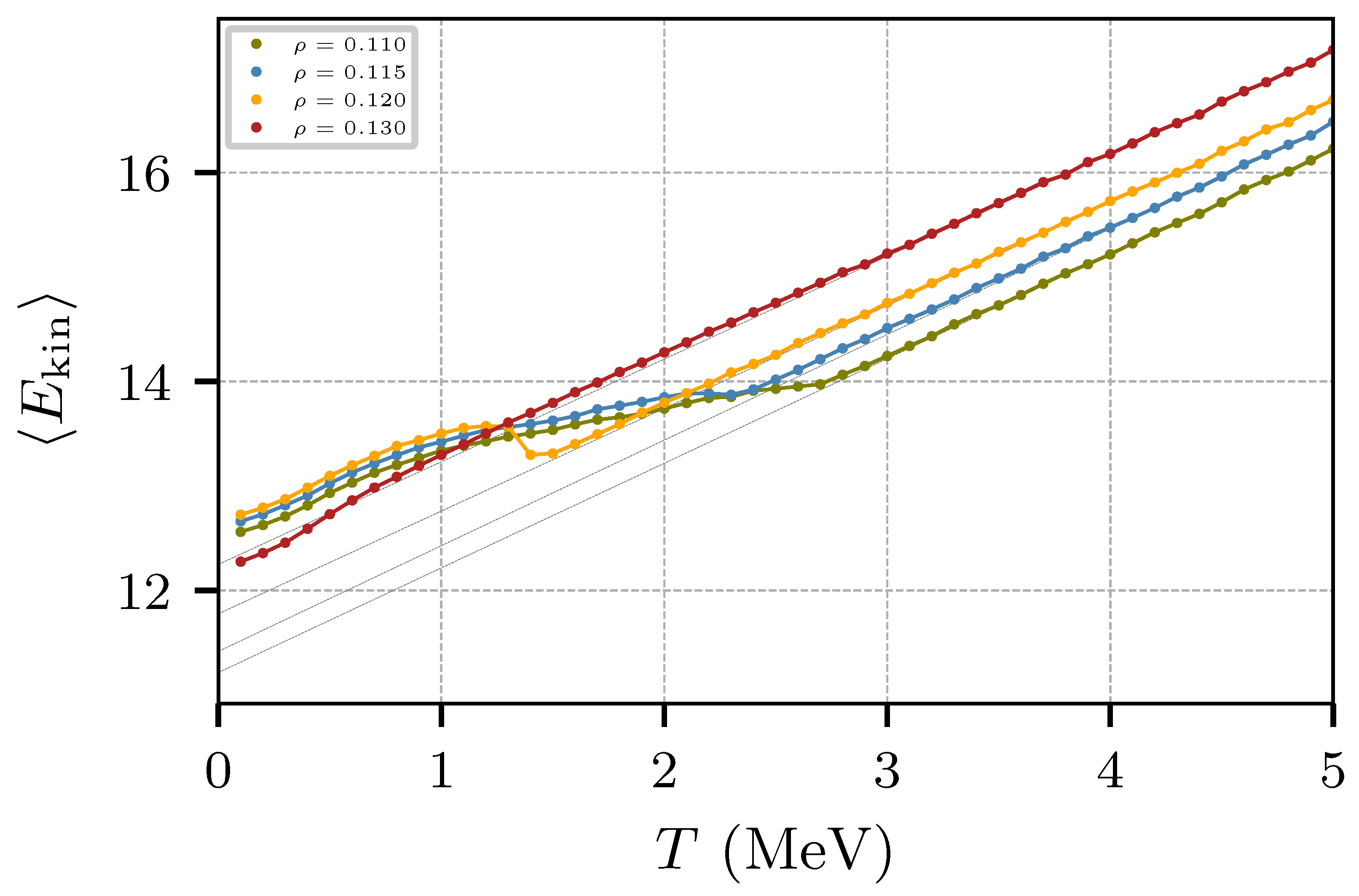
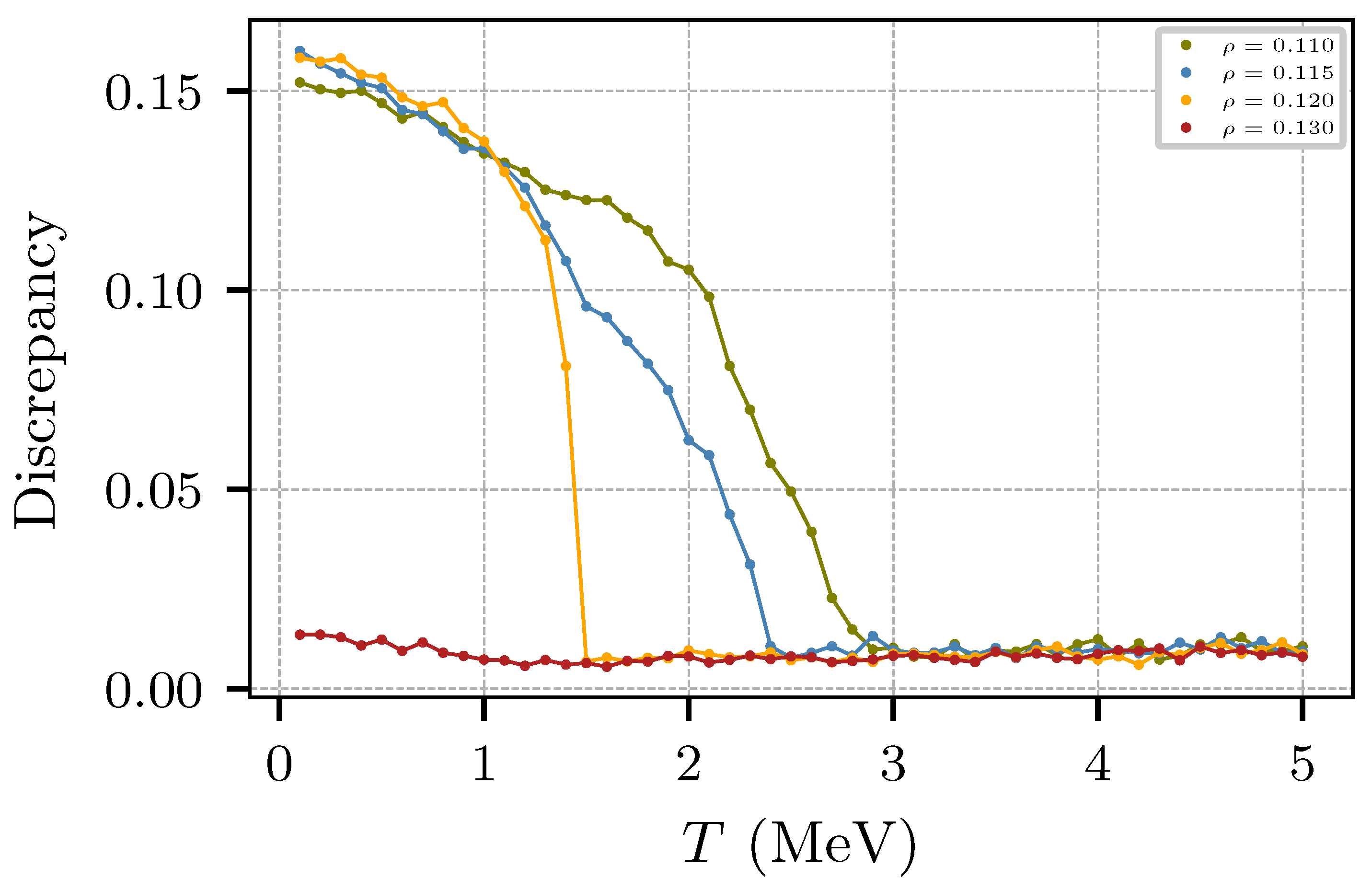
4.2. Neutron Star Matter
Pastas of Neutron Star Matter
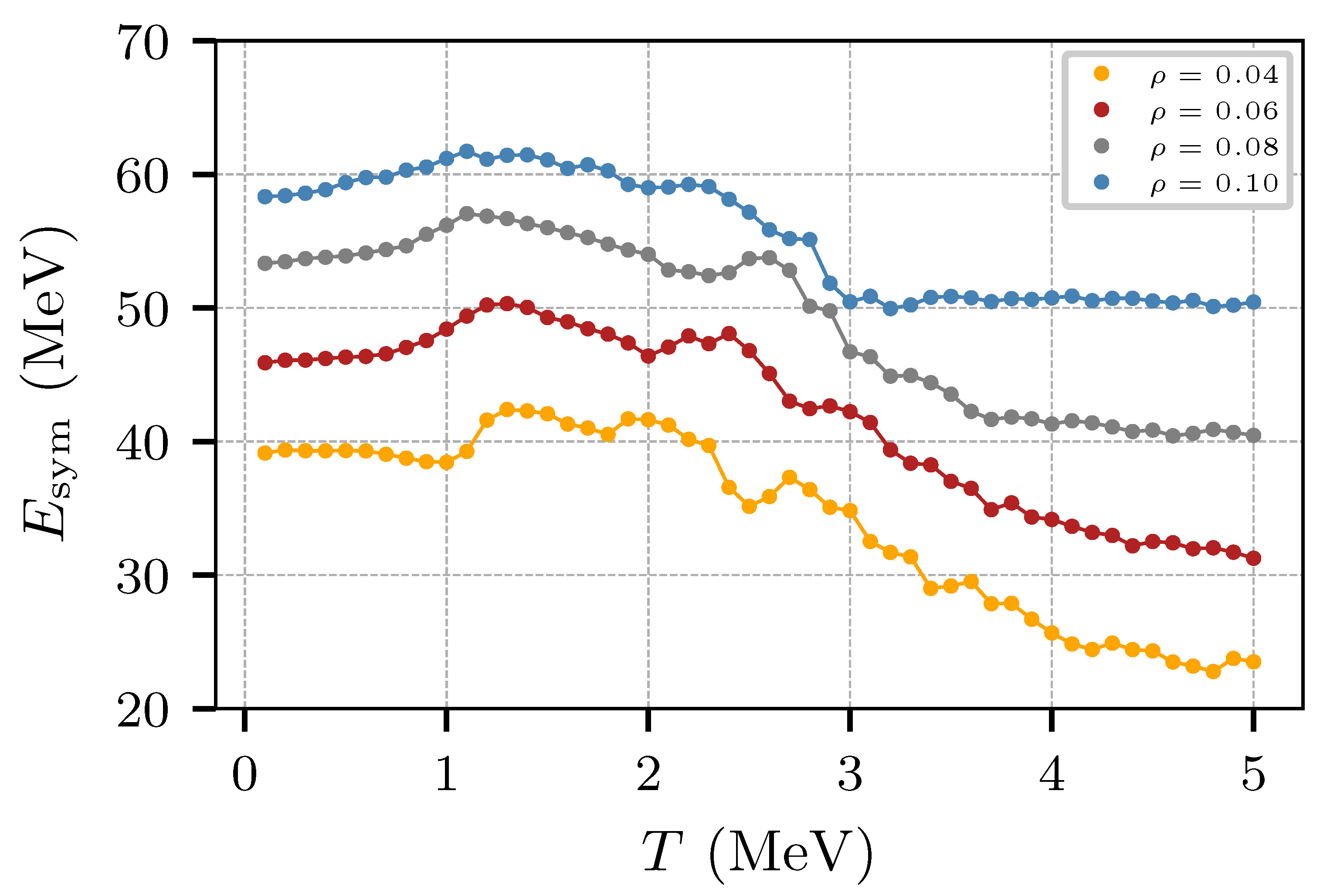
5. Symmetry Energy
6. Discussion
Author Contributions
Funding
Conflicts of Interest
Appendix A. Metropolis Monte Carlo

Appendix B. Symmetry Energy
References
- Bethe, H.A. What holds the nucleus together? Sci. Am. 1953, 189, 58–63. [Google Scholar] [CrossRef]
- Koonin, S.E. Time-dependent Hartree-Fock calculations for/sup 16/O+/sup 16/O reactions. Phys. Lett. B 1976, 61, 165. [Google Scholar] [CrossRef]
- Ulrych, S.; Mauther, H. Relativistic structure of the nucleon self-energy in asymmetric nuclei. Phys. Rev. C 1997, 56, 1788–1794. [Google Scholar] [CrossRef]
- van Dalen, E.N.E.; Fuchs, C.; Faessler, A. The relativistic Dirac–Brueckner approach to asymmetric nuclear matter. Nucl. Phys. A 2004, 741, 227–248. [Google Scholar] [CrossRef]
- Ma, Z.Y.; Rong, J.; Chen, B.Q.; Zhu, Z.Y.; Song, H.Q. Isospin dependence of nucleon effective mass in Dirac Brueckner–Hartree–Fock approach. Phys. Lett. B 2004, 604, 170–174. [Google Scholar] [CrossRef][Green Version]
- Sammarruca, F.; Barredo, W.; Krastev, P. Predicting the single-proton and single-neutron potentials in asymmetric nuclear matter. Phys. Rev. C 2005, 71, 064306. [Google Scholar] [CrossRef]
- van Dalen, E.N.E.; Fuchs, C.; Faessler, A. Effective nucleon masses in symmetric and asymmetric nuclear matter. Phys. Rev. Lett. 2005, 95, 022302. [Google Scholar] [CrossRef] [PubMed]
- van Dalen, E.N.E.; Fuchs, C.; Faessler, A. Momentum, density, and isospin dependence of symmetric and asymmetric nuclear matter properties. Phys. Rev. C 2005, 72, 065803. [Google Scholar] [CrossRef]
- Rong, J.; Ma, Z.Y.; Giai, N.V. Isospin-dependent optical potentials in Dirac-Brueckner-Hartree-Fock approach. Phys. Rev. C 2006, 73, 014614. [Google Scholar] [CrossRef]
- Bombaci, I.; Lombardo, U. Asymmetric nuclear matter equation of state. Phys. Rev. C 1991, 44, 1892–1900. [Google Scholar] [CrossRef] [PubMed]
- Zuo, W.; Cao, L.G.; Li, B.A.; Lombardo, U.; Shen, C.W. Isospin splitting of the nucleon mean field. Phys. Rev. C 2005, 72, 014005. [Google Scholar] [CrossRef]
- Davies, K.T.R.; Sierk, A.J.; Nix, J.R. Effect of viscosity on the dynamics of fission. Phys. Rev. C 1976, 13, 2385–2403. [Google Scholar] [CrossRef]
- Baran, V.; Colonna, M.; Greco, V.; Toro, M.D. Reaction dynamics with exotic nuclei. Phys. Rep. 2005, 410, 335–466. [Google Scholar] [CrossRef]
- Das, C.B.; Gupta, S.D.; Gale, C.; Li, B.A. Momentum dependence of symmetry potential in asymmetric nuclear matter for transport model calculations. Phys. Rev. C 2003, 67, 034611. [Google Scholar] [CrossRef]
- Li, B.A.; Das, C.B.; Gupta, S.D.; Gale, C. Momentum dependence of the symmetry potential and nuclear reactions induced by neutron-rich nuclei at RIA. Phys. Rev. C 2004, 69, 011603. [Google Scholar] [CrossRef]
- Li, B.A.; Das, C.B.; Gupta, S.D.; Gale, C. Effects of momentum-dependent symmetry potential on heavy-ion collisions induced by neutron-rich nuclei. Nucl. Phys. A 2004, 735, 563. [Google Scholar] [CrossRef]
- Li, B.A. Constraining the neutron-proton effective mass splitting in neutron-rich matter. Phys. Rev. C 2004, 69, 064602. [Google Scholar] [CrossRef]
- Chen, L.W.; Ko, C.M.; Li, B.A. Effects of momentum-dependent nuclear potential on two-nucleon correlation functions and light cluster production in intermediate energy heavy-ion collisions. Phys. Rev. C 2004, 69, 054606. [Google Scholar] [CrossRef]
- Rizzo, J.; Colonna, M.; Toro, M.D.; Greco, V. Transport properties of isospin effective mass splitting. Nucl. Phys. A 2004, 732, 202–217. [Google Scholar] [CrossRef]
- Behera, B.; Routray, T.R.; Pradhan, A.; Patra, S.K.; Sahu, P.K. Momentum and density dependence of the isospin part of nuclear mean field and equation of state of asymmetric nuclear matter. Nucl. Phys. A 2005, 753, 367–386. [Google Scholar] [CrossRef]
- Rizzo, J.; Colonna, M.; Toro, M.D. Fast nucleon emission as a probe of the momentum dependence of the symmetry potential. Phys. Rev. C 2005, 72, 064609. [Google Scholar] [CrossRef]
- Li, B.A.; Chen, L.W.; Ko, C.M. Recent progress and new challenges in isospin physics with heavy-ion reactions. Phys. Rep. 2008, 464, 113–281. [Google Scholar] [CrossRef]
- Chen, L.W.; Ko, C.M.; Li, B.A. Isospin-dependent properties of asymmetric nuclear matter in relativistic mean field models. Phys. Rev. C 2007, 76, 054316. [Google Scholar] [CrossRef]
- Aichelin, J. “Quantum” molecular dynamics—A dynamical microscopic n-body approach to investigate fragment formation and the nuclear equation of state in heavy ion collisions. Phys. Rept. 1991, 202, 233–360. [Google Scholar] [CrossRef]
- Ono, A. Antisymmetrized molecular dynamics with quantum branching processes for collisions of heavy nuclei. Phys. Rev. C 1999, 59, 853. [Google Scholar] [CrossRef]
- Ono, A.; Horiuchi, H. Antisymmetrized molecular dynamics for heavy ion collisions. Prog. Part. Nucl. Phys. A 2004, 53, 501–581. [Google Scholar] [CrossRef]
- López, J.A.; Lübeck, G. Nuclear spinodal decomposition. Phys. Lett. B 1989, 219, 215–221. [Google Scholar] [CrossRef]
- Wilets, L.; Henley, E.M.; Kraft, M.; Mackellar, A.D. Classical many-body model for heavy-ion collisions incorporating the Pauli principle. Nucl. Phys. A 1977, 282, 341–350. [Google Scholar] [CrossRef]
- Wilets, L.; Yariv, Y.; Chestnut, R. Classical many-body model for heavy-ion collisions (II). Nucl. Phys. A 1978, 301, 359–364. [Google Scholar] [CrossRef]
- Dorso, C.; Randrup, J. Classical simulation of nuclear systems. Phys. Lett. B 1988, 215, 611–616. [Google Scholar] [CrossRef][Green Version]
- López, J.; Dorso, C.O.; Frank, G. Properties of nuclear pastas. Front. Phys. 2021, 16, 24301. [Google Scholar] [CrossRef]
- Horowitz, C.J.; Pérez-García, M.A.; Piekarewicz, J. Neutrino-“pasta” scattering: The opacity of nonuniform neutron-rich matter. Phys. Rev. C 2004, 69, 045804. [Google Scholar] [CrossRef]
- Horowitz, C.J.; Pérez-García, M.A.; Carriere, J.; Berry, D.K.; Piekarewicz, J. Nonuniform neutron-rich matter and coherent neutrino scattering. Phys. Rev. C 2004, 70, 065806. [Google Scholar] [CrossRef]
- Horowitz, C.J.; Pérez-García, M.A.; Berry, D.K.; Piekarewicz, J. Dynamical response of the nuclear “pasta” in neutron star crusts. Phys. Rev. C 2005, 72, 035801. [Google Scholar] [CrossRef]
- Piekarewicz, J.; Sánchez, G.T. Proton fraction in the inner neutron-star crust. Phys. Rev. C 2012, 85, 015807. [Google Scholar] [CrossRef]
- Nordheim, L.W. Transport phenomena in Einstein-Bose and fermi-dirac gases. Proc. R. Soc. 1928, 119, 689. [Google Scholar]
- Lenk, R.J.; Schlagel, T.J.; Pandharipande, V.R. Accuracy of the Vlasov-Nordheim approximation in the classical limit. Phys. Rev. C 1990, 42, 372. [Google Scholar] [CrossRef]
- Dorso, C.O.; Frank, G.; López, J.A. Phase transitions and symmetry energy in nuclear pasta. Nucl. Phys. A 2018, 978, 35–64. [Google Scholar] [CrossRef]
- Dorso, C.O.; Molinelli, P.A.G.; López, J.A. From nuclei to nuclear pasta. In Neutron Star Crust; Bertulani, C.A., Piekarewicz, J., Eds.; Nova Science Publishers: Hauppauge, NY, USA, 2012; pp. 151–169. [Google Scholar]
- Dorso, C.O.; Molinelli, P.A.G.; López, J.A. Topological characterization of neutron star crusts. Phys. Rev. C 2012, 86, 055805. [Google Scholar] [CrossRef]
- López, J.A.; Ramírez-Homs, E. Effect of an electron gas on a neutron-rich nuclear pasta. Nuc. Sci. Tech. 2015, 26, S20502. [Google Scholar]
- Dorso, C.O.; Frank, G.; López, J.A. Symmetry energy in neutron star matter. Nucl. Phys. A 2019, 984, 77–98. [Google Scholar] [CrossRef]
- Molinelli, P.A.G.; Nichols, J.I.; López, J.A.; Dorso, C.O. Simulations of cold nuclear matter at sub-saturation densities. Nucl. Phys. A 2014, 923, 31–50. [Google Scholar] [CrossRef]
- López, J.A.; Ramírez-Homs, E.; González, R.; Ravelo, R. Isospin-asymmetric nuclear matter. Phys. Rev. C 2014, 89, 024611. [Google Scholar] [CrossRef]
- Dorso, C.O.; Molinelli, P.A.; López, J.A. Isoscaling and the nuclear EoS. J. Phys. G Nucl. Part. Phys. 2011, 38, 115101. [Google Scholar] [CrossRef]
- López, J.A.; Terrazas, A.G.; Porras, S.T. Isospin-dependent phase diagram of nuclear matter. Nucl. Phys. A 2020, 994, 121664. [Google Scholar] [CrossRef]
- Boal, D.H.; Glosli, J.N. From binary breakup to multifragmentation: Computer simulation. Phys. Rev. C 1988, 37, 91. [Google Scholar] [CrossRef] [PubMed]
- Boal, D.H.; Glosli, J.N. Quasiparticle model for nuclear dynamics studies: Ground-state properties. Phys. Rev. C 1988, 38, 1870. [Google Scholar] [CrossRef]
- Dorso, C.O.; Duarte, S.; Randrup, J. Classical simulation of the Fermi gas. Phys. Lett. B 1987, 188, 287. [Google Scholar] [CrossRef][Green Version]
- Dorso, C.O.; Randrup, J. Early recognition of clusters in molecular dynamics. Phys. Lett. B 1993, 301, 328. [Google Scholar] [CrossRef]
- Metropolis, N.; Rosenbluth, A.W.; Rosenbluth, M.N.; Teller, A.H.; Teller, E. Equation of state calculations by fast computing machines. J. Chem. Phys. 1953, 21, 1087–1092. [Google Scholar] [CrossRef]
- Alcain, P.N.; Dorso, C.O. Dynamics of fragment formation in neutron-rich matter. Phys. Rev. C 2018, 97, 015803. [Google Scholar] [CrossRef]
- Ravenhall, D.G.; Pethick, C.J.; Wilson, J.R. Structure of matter below nuclear saturation density. Phys. Rev. Lett. 1983, 50, 2066. [Google Scholar] [CrossRef]
- Hashimoto, M.; Seki, H.; Yamada, M. Shape of nuclei in the crust of neutron star. Prog. Theor. Phys. 1984, 71, 320. [Google Scholar] [CrossRef]
- Page, D.; Lattimer, J.M.; Prakash, M.; Steiner, A.W. Minimal cooling of neutron stars: A new paradigm. Strophys. J. Supp. 2004, 155, 623. [Google Scholar] [CrossRef]
- Williams, R.D.; Koonin, S.E. Sub-saturation phases of nuclear matter. Nucl. Phys. A 1985, 435, 844–858. [Google Scholar] [CrossRef]
- Maruyama, T.; Niita, K.; Oyamatsu, K.; Maruyama, T.; Chiba, S.; Iwamoto, A. Quantum molecular dynamics approach to the nuclear matter below the saturation density. Phys. Rev. C 1998, 57, 655. [Google Scholar] [CrossRef]
- Kido, T.; Maruyama, T.; Niita, K.; Chiba, S. MD simulation study for nuclear matter. Nucl. Phys. A 2000, 663–664, 877c–880c. [Google Scholar] [CrossRef]
- Watanabe, G.; Sato, K.; Yasuoka, K.; Ebisuzaki, T. Microscopic study of slablike and rodlike nuclei: Quantum molecular dynamics approach. Phys. Rev. C 2002, 66, 012801. [Google Scholar] [CrossRef]
- Alcain, P.N.; Molinelli, P.A.G.; Dorso, C.O. Beyond nuclear “pasta”: Phase transitions and neutrino opacity of new “pasta” phases. Phys. Rev. C 2014, 90, 065803. [Google Scholar] [CrossRef]
- Alcain, P.N.; Molinelli, P.A.G.; Nichols, J.I.; Dorso, C.O. Effect of Coulomb screening length on nuclear “pasta” simulations. Phys. Rev. C 2014, 89, 055801. [Google Scholar] [CrossRef]
- von Weizsäcker, C.F. Zur theorie der kernmassen. Z. Physik 1935, 96, 431–458. [Google Scholar] [CrossRef]
- Tsang, M.B.; Zhang, Y.; Danielewicz, P.; Famiano, M.; Li, Z.; Lynch, W.G.; Steiner, A.W. Constraints on the density dependence of the symmetry energy. Phys. Rev. Lett. 2009, 102, 122701. [Google Scholar] [CrossRef] [PubMed]
- López, J.A.; Porras, S.T. Symmetry energy in the liquid–gas mixture. Nucl. Phys. A 2017, 957, 312–320. [Google Scholar] [CrossRef]
- Horowitz, C.J.; Schwenk, A. Cluster formation and the virial equation of state of low-density nuclear matter. Nucl. Phys. A 2006, 776, 55. [Google Scholar] [CrossRef]
- Xu, J.; Chen, L.W.; Li, B.A.; Ma, H.R. Temperature effects on the nuclear symmetry energy and symmetry free energy with an isospin and momentum dependent interaction. Phys. Rev. C 2007, 75, 014607. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dorso, C.O.; Frank, G.; López, J.A. Symmetry Energy and the Pauli Exclusion Principle. Symmetry 2021, 13, 2116. https://doi.org/10.3390/sym13112116
Dorso CO, Frank G, López JA. Symmetry Energy and the Pauli Exclusion Principle. Symmetry. 2021; 13(11):2116. https://doi.org/10.3390/sym13112116
Chicago/Turabian StyleDorso, Claudio O., Guillermo Frank, and Jorge A. López. 2021. "Symmetry Energy and the Pauli Exclusion Principle" Symmetry 13, no. 11: 2116. https://doi.org/10.3390/sym13112116
APA StyleDorso, C. O., Frank, G., & López, J. A. (2021). Symmetry Energy and the Pauli Exclusion Principle. Symmetry, 13(11), 2116. https://doi.org/10.3390/sym13112116





