New Sufficient Conditions to Ulam Stabilities for a Class of Higher Order Integro-Differential Equations

Abstract

In this work, we present sufficient conditions in order to establish different types of Ulam stabilities for a class of higher order integro-differential equations. In particular, we consider a new kind of stability, the $\sigma$-semi-Hyers-Ulam stability, which is in some sense between the Hyers–Ulam and the Hyers–Ulam–Rassias stabilities. These new sufficient conditions result from the application of the Banach Fixed Point Theorem, and by applying a specific generalization of the Bielecki metric.

1. Introduction

In 1940, S. M. Ulam [1] proposed the well-known Ulam stability problem. The difficulty of this problem lies in the conditions to be imposed to guarantee the existence of a linear mapping near an approximately linear mapping. It is known that most of the time it is not possible to obtain exact solutions for some integro-differential equations. Therefore, special techniques are applied, allowing us to obtain approximate solutions. In this case, it is crucial to find error bounds to the approximations when replacing the exact solutions in practical problems.

In 1941, D. H. Hyers [2] gave a partial answer to the problem under the assumption that the groups are Banach spaces, considering the additive Cauchy equation $f(x+y)=f\left(x\right)+f\left(y\right)$. This contribution originated the naming Hyers–Ulam stability. Meanwhile some other approaches came to light, and later in 1978, new directions were introduced by Th. M. Rassias [3] aiming to solve the Ulam stability problem, which gave origin to the concept of Hyers–Ulam–Rassias stability. Furthermore, new developments were carried out involving different norms and other types of equations. We refer in particular to the works presented by T. Aoki [4], Z. Gajda [5] and Th. M. Rassias [6]. For more details on the subject, we refer to [7,8] and the references therein.

The work initiated by S. M. Ulam in the 1940s had relevant consequences in the field of applications as, for example, in chemical reactions, elasticity, fluid flows, semiconductors and population dynamics (see [9,10,11,12]). The study of problems involving differential, functional, integro-differential and integral equations, in particular their stability issues, has suffered greatly from the growing engagement over the years with a spread of interest among researchers, for example, see [2,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27]. Their applicability in mathematical models for which we cannot easily find exact solutions, namely those involving symmetry issues, the study of the stability of the approximate solutions is an open field of research. In particular, this work may be relevant in the study of the stability of the symmetrical flow of Newtonian and non-Newtonian fluids related to one-dimensional models obtained by Cosserat Theory associated with fluid dynamics (see [28,29]).

2. Notations and Preliminaries

Let us consider the higher order integro-differential equation defined by

$$F\left(x,\phi \left(x\right),{\int }_a^{x}G\left(x,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)={\phi }^{\left(n\right)}\left(x\right),$$

(1)

with initial conditions

$${\phi }^{\left(j\right)}\left(a\right)=0,j=0,\dots ,n-1,$$

(2)

where $n\in \mathbb{N}$, $\phi \in C^n\left([a,b]\right)$ and $x\in [a,b]$, with fixed real numbers a and b. Moreover, we consider two continuous functions $F:[a,b]\times \mathbb{C}\times \mathbb{C}\to \mathbb{C}$ and $G:[a,b]\times [a,b]\times {\mathbb{C}}^n\to \mathbb{C}$.

Next, we introduce several stability concepts related to problem (1) and (2).

Definition 1

(Hyers–Ulam stability). If for each continuously differentiable function φ satisfying

$$\left|F\left(x,\phi \left(x\right),{\int }_a^{x}G\left(x,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)-{\phi }^{\left(n\right)}\left(x\right)\right|\le \beta ,$$

(3)

with $x\in [a,b]$ and $\beta \ge 0$, the higher order integro-differential equation has a solution ${\phi }_0$ and there is a constant $C>0$, independent of ${\phi }_0$ and φ, such that

$$\left|{\phi }_0\left(x\right)-\phi \left(x\right)\right|\le C\beta ,$$

(4)

for all $x\in [a,b]$, then we say that the given problem (1) and (2) has the Hyers–Ulam stability.

Definition 2

(Hyers–Ulam–Rassias stability). Let θ be a non-decreasing continuous function defined in the interval $[a,b]$. If for each continuously differentiable function φ satisfying

$$\left|F\left(x,\phi \left(x\right),{\int }_a^{x}G\left(x,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)-{\phi }^{\left(n\right)}\left(x\right)\right|\le \theta \left(x\right),$$

(5)

with $x\in [a,b]$, the higher order integro-differential equation has a solution ${\phi }_0$ and there is a constant $C>0$, independent of ${\phi }_0$ and φ, such that

$$\left|{\phi }_0\left(x\right)-\phi \left(x\right)\right|\le C\theta \left(x\right),$$

(6)

for all $x\in [a,b]$, then we say that the given problem (1) and (2) has the Hyers–Ulam–Rassias stability.

Now, we will introduce a new kind of stability which was presented in [30].

Definition 3

($\theta$-semi-Hyers–Ulam stability). Let θ be a non-decreasing continuous function defined in the interval $[a,b]$. If for each continuously differentiable function φ satisfying

$$\left|F\left(x,\phi \left(x\right),{\int }_a^{x}G\left(x,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)-{\phi }^{\left(n\right)}\left(x\right)\right|\le \beta ,$$

(7)

where $x\in [a,b]$ and $\beta \ge 0$, the higher order integro-differential equation has a solution ${\phi }_0$ and there is a constant $C>0$, independent of ${\phi }_0$ and φ, such that

$$\left|{\phi }_0\left(x\right)-\phi \left(x\right)\right|\le C\theta \left(x\right),$$

(8)

for all $x\in [a,b]$, then we say that the given problem (1) and (2) has the θ-semi-Hyers–Ulam stability.

In general, to study the stability of functional, integral and integro-differential equations, it is usual to consider fixed point arguments, for example, see [18,31,32,33,34,35,36,37,38]. Here, we consider the Banach fixed point theorem, which we recall next.

Theorem 1

(Banach fixed point theorem). Let $(X,d)$ be a generalized complete metric space and let $T:X\to X$ a strictly contractive operator with a Lipschitz constant $L<1$. If there exists a non-negative integer k such that $d(T^{k+1}x,T^{k}x)<\infty$ for some $x\in X$, then the following three propositions hold true:

1. 

the sequence ${\left(T^{n}x\right)}_{n\in \mathbb{N}}$ converges to a fixed point $x^{\ast }$ of T;

2. 

$x^{\ast }$ is the unique fixed point of T in $X^{\ast }=\{y\in X:d(T^{k}x,y)<\infty \}$;

3. 

if $y\in X^{\ast }$, then

$$d(y,x^{\ast })\le \frac{1}{1-L}d(Ty,y).$$

(9)

In the following, we consider the space of continuously differentiable functions in the interval $[a,b]$, $C^n\left([a,b]\right)$, endowed with a generalization of the Bielecki metric, given by

$$d(\phi ,\psi )=\underset{x\in [a,b]}{sup}\frac{\left|\phi \right(x)-\psi (x\left)\right|}{\theta \left(x\right)},$$

(10)

with $\theta$ a non-decreasing continuous function $\theta :[a,b]\to (0,\infty )$ and $\left(C^n\left([a,b]\right),d\right)$ is a complete metric space (see [39,40]).

3. Hyers-Ulam-Rassias Stability

In the following theorem we will present sufficient conditions for the Hyers–Ulam–Rassias stability relating to problem (1) and (2).

Theorem 2.

Let $\theta :[a,b]\to (0,\infty )$ be a non-decreasing continuous function which fulfills the inequality

$${\int }_a^x\theta \left(t\right)dt\le \alpha \theta \left(x\right),$$

(11)

for all $x\in [a,b]$, where $\alpha \in \mathbb{R}$. Suppose also that the continuous function $F:[a,b]\times \mathbb{C}\times \mathbb{C}\to \mathbb{C}$ satisfies the condition

$$\left|F(x,u(x),g(x\left)\right)-F(x,v(x),h(x\left)\right)\right|\le M\left(\left|u\right(x)-v(x\left)\right|+\left|g\right(x)-h(x\left)\right|\right)$$

(12)

where $M>0$ is a Lipschitz constant, and the continuous kernel $G:[a,b]\times [a,b]\times {\mathbb{C}}^n\to \mathbb{C}$ satisfies the condition

$$\begin{array}{c}\hfill \left|G\left(x,t,u\left(t\right),u^{\prime }\left(t\right),\dots ,u^{(n-1)}\left(t\right)\right)-G\left(x,t,v\left(t\right),v^{\prime }\left(t\right),\dots ,v^{(n-1)}\left(t\right)\right)\right|\le L|u\left(t\right)-v\left(t\right)|\end{array}$$

(13)

where $L>0$ is a Lipschitz constant. If $\phi \in C^n\left([a,b]\right)$ is such that

$$\left|F\left(x,\phi \left(x\right),{\int }_a^{x}G\left(x,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)-{\phi }^{\left(n\right)}\left(x\right)\right|\le \theta \left(x\right),$$

(14)

where $x\in [a,b]$, and $M\left({\alpha }^n+L{\alpha }^{n+1}\right)<1$, then there is a unique function ${\phi }_0\in C^n\left([a,b]\right)$, solution of problem (1) and (2), such that

$$|{\phi }_0\left(x\right)-\phi \left(x\right)|\le \frac{{\alpha }^n}{1-M\left({\alpha }^n+L{\alpha }^{n+1}\right)}\theta \left(x\right),$$

(15)

for all $x\in [a,b]$.

Proof. 

Considering the initial conditions (2), i.e., ${\phi }^{\left(j\right)}\left(a\right)=0$, $j=0,\dots ,n-1$ and using integration properties, Equation (1) is equivalent to

$$\begin{array}{ccc}& & {\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F\left(\right(r_1,\phi \left(r_1\right),\hfill \\ & & \left.{\int }_a^{r_1}G\left(r_1,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n=\phi \left(x\right).\hfill \end{array}$$

Let us define the continuous operator $T:C^n\left([a,b]\right)\to C^n\left([a,b]\right)$ by

$$\begin{array}{ccc}\hfill & & \left(T\phi \right)\left(x\right)={\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F(r_1,\phi \left(r_1\right),\hfill \\ & & {\int }_a^{r_1}G\left(r_1,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n,\hfill \end{array}$$

(16)

for all $x\in [a,b]$ and $\phi \in C^n\left([a,b]\right)$. Indeed, for any continuous function $\phi$, we have

$$\begin{array}{ccc}\hfill & & \left|\left(T\phi \right)\left(x\right)-\left(T\phi \right)\left(x_0\right)\right|=\left|{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F\left(r_1,\phi \left(r_1\right),\right.\right.\hfill \\ & & \left.{\int }_a^{r_1}G\left(r_1,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & -{\int }_a^{x_0}{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F\left(r_1,\phi \left(r_1\right),\right.\hfill \\ & & \left.\left.{\int }_a^{r_1}G\left(r_1,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\right|\hfill \\ & & =\left|{\int }_x^{x_0}{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F\left(r_1,\phi \left(r_1\right),\right.\right.\hfill \\ & & \left.\left.{\int }_a^{r_1}G\left(r_1,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\right|⟶0\hfill \end{array}$$

(17)

when $x\to x_0$. Therefore, using condition (11), we have

$$\begin{array}{ccc}\hfill & & {\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}{\int }_a^{r_1}\theta \left(t\right)dtd{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & \le {\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\alpha \theta \left(r_1\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & \le {\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_3}{\alpha }^2\theta \left(r_2\right)d{r}_2\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & ⋮\hfill \\ & & \le {\int }_a^x{\alpha }^n\theta \left(r_n\right)d{r}_n\hfill \\ & & \le {\alpha }^{n+1}\theta \left(x\right).\hfill \end{array}$$

(18)

Consequently, using conditions (17) and (18), we will prove that the operator T is strictly contractive to the Bielecki metric (10). In order to prove that, we have for all $\phi ,\psi \in C^n\left([a,b]\right)$:

$$\begin{array}{ccc}& & d\left(T\phi ,T\psi \right)=\underset{x\in [a,b]}{sup}\frac{\left|\left(T\phi \right)\left(x\right)-\left(T\psi \right)\left(x\right)\right|}{\theta \left(x\right)}\hfill \\ & & =\underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}\left|{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F\left(r_1,\phi \left(r_1\right),{\int }_a^{r_1}G\left(r_1,\right.\right.\right.\hfill \\ & & \left.\left.t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & -{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F\left(r_1,\psi \left(r_1\right),{\int }_a^{r_1}G\left(r_1,\right.\right.\hfill \\ & & \left.\left.\left.t,\psi \left(t\right),{\psi }^{\prime }\left(t\right),\dots ,{\psi }^{(n-1)}\left(t\right)\right)dt\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\right|\hfill \\ & & \le \underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\left|F\left(r_1,\right.\right.\hfill \\ & & \left.\phi \left(r_1\right),{\int }_a^{r_1}G\left(r_1,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)\hfill \\ & & -F\left(r_1,\psi \left(r_1\right),{\int }_a^{r_1}G\left(r_1,t,\psi \left(t\right),\right.\right.\hfill \\ & & \left.\left.\left.{\psi }^{\prime }\left(t\right),\dots ,{\psi }^{(n-1)}\left(t\right)\right)dt\right)\right|d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & \le M\underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\left|\phi \left(r_1\right)-\psi \left(r_1\right)\right|d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & +M\underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}{\int }_a^{r_1}\left|G\left(r_1,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)\right.\hfill \\ & & \left.-G\left(r_1,t,\psi \left(t\right),{\psi }^{\prime }\left(t\right),\dots ,{\psi }^{(n-1)}\left(t\right)\right)\right|dtd{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & \le M\underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\left|\phi \left(r_1\right)-\psi \left(r_1\right)\right|d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & +ML\underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\hfill \\ & & {\int }_a^{r_1}\left|\phi \left(t\right)-\psi \left(t\right)\right|dtd{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & =M\underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\theta \left(r_1\right)\frac{\left|\phi \left(r_1\right)-\psi \left(r_1\right)\right|}{\theta \left(r_1\right)}d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & +ML\underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\hfill \\ & & {\int }_a^{r_1}\theta \left(t\right)\frac{\left|\phi \left(t\right)-\psi \left(t\right)\right|}{\theta \left(t\right)}dtd{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & \le M\underset{r_1\in [a,b]}{sup}\frac{\left|\phi \left(r_1\right)-\psi \left(r_1\right)\right|}{\theta \left(r_1\right)}\underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}{\int }_a^x{\int }_a^{r_n}\hfill \\ & & {\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\theta \left(r_1\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & +ML\underset{t\in [a,b]}{sup}\frac{\left|\phi \left(t\right)-\psi \left(t\right)\right|}{\theta \left(t\right)}\underset{x\in [a,b]}{sup}\frac{1}{\theta \left(x\right)}{\int }_a^x{\int }_a^{r_n}\hfill \\ & & {\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}{\int }_a^{r_1}\theta \left(t\right)dtd{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & \le Md(\phi ,\psi ){\alpha }^n+MLd(\phi ,\psi )\underset{x\in [a,b]}{sup}\frac{{\alpha }^{n+1}\theta \left(x\right)}{\theta \left(x\right)}\hfill \\ & & =M\left({\alpha }^n+L{\alpha }^{n+1}\right)d(\phi ,\psi ).\hfill \end{array}$$

Therefore, by the fact that

$$M\left({\alpha }^n+L{\alpha }^{n+1}\right)<1$$

we have that the operator T is strictly contractive. Thus, we can apply Theorem 1, which ensures that we have the Hyers–Ulam–Rassias stability for problem (1) and (2). Additionally, from (14), we have

$$\begin{array}{c}\hfill -\theta \left(x\right)\le F\left(x,\phi \left(x\right),{\int }_a^{x}G\left(x,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)-{\phi }^{\left(n\right)}\left(x\right)\le \theta \left(x\right),\end{array}$$

(19)

where $x\in [a,b]$. Therefore, using integration, we obtain

$$\begin{array}{ccc}\hfill & & \left|{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F\left(r_1,\phi \left(r_1\right),{\int }_a^{r_1}G\left(r_1,\right.\right.\right.\hfill \\ & & \left.\left.\left.t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n-\phi \left(x\right)\right|\hfill \\ & & \le {\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\theta \left(r_1\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & \le {\alpha }^n\theta \left(x\right).\hfill \end{array}$$

(20)

Now, using conditions (16) and (20), we have

$$\begin{array}{c}\hfill \left|\left(T\phi \right)\left(x\right)-\phi \left(x\right)\right|\le {\alpha }^n\theta \left(x\right),x\in [a,b].\end{array}$$

(21)

Moreover, from (9) follows

$$\begin{array}{c}\hfill d\left({\phi }_0,\phi \right)\le \frac{1}{1-M\left({\alpha }^n+L{\alpha }^{n+1}\right)}d\left(T\phi ,\phi \right).\end{array}$$

(22)

Finally, from the definition of the metric d and (21), we obtain

$$\begin{array}{c}\hfill \underset{x\in [a,b]}{sup}\frac{\left|{\phi }_0\left(x\right)-\phi \left(x\right)\right|}{\theta \left(x\right)}\le \frac{{\alpha }^n}{1-M\left({\alpha }^n+L{\alpha }^{n+1}\right)}\end{array}$$

(23)

and consequently condition (15) holds. □

4. $\mathbf{\theta }$-Semi-Hyers–Ulam and Hyers–Ulam Stabilities

Next theorems presents sufficient conditions for the $\theta$-semi-Hyers–Ulam stability and for the Hyers–Ulam stability regarding problem (1) and (2).

Theorem 3.

Let $\theta :[a,b]\to (0,\infty )$ be a non-decreasing continuous function which fulfills the inequality

$$\begin{array}{c}\hfill {\int }_a^x\theta \left(t\right)dt\le \alpha \theta \left(x\right),\end{array}$$

(24)

for all $x\in [a,b]$, where $\alpha \in \mathbb{R}$. Suppose also that the continuous function $F:[a,b]\times \mathbb{C}\times \mathbb{C}\to \mathbb{C}$ satisfies the condition

$$\begin{array}{c}\hfill \left|F(x,u(x),g(x\left)\right)-F(x,v(x),h(x\left)\right)\right|\le M\left(\left|u\right(x)-v(x\left)\right|+\left|g\right(x)-h(x\left)\right|\right)\end{array}$$

(25)

where $M>0$ is a Lipschitz constant, and the continuous kernel $G:[a,b]\times [a,b]\times {\mathbb{C}}^n\to \mathbb{C}$ satisfies the condition

$$\begin{array}{c}\hfill \left|G\left(x,t,u\left(t\right),u^{\prime }\left(t\right),\dots ,u^{(n-1)}\left(t\right)\right)-G\left(x,t,v\left(t\right),v^{\prime }\left(t\right),\dots ,v^{(n-1)}\left(t\right)\right)\right|\le L|u\left(t\right)-v\left(t\right)|\end{array}$$

(26)

where $L>0$ is a Lipschitz constant. If $\phi \in C^n\left([a,b]\right)$ is such that

$$\begin{array}{c}\hfill \left|F\left(x,\phi \left(x\right),{\int }_a^{x}G\left(x,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)-{\phi }^{\left(n\right)}\left(x\right)\right|\le \beta ,\end{array}$$

(27)

where $x\in [a,b]$, $\beta \ge 0$, and $M\left({\alpha }^n+L{\alpha }^{n+1}\right)<1$, then there exists a unique function ${\phi }_0\in C^n\left([a,b]\right)$, solution of the problem (1) and (2), such that

$$\begin{array}{c}\hfill |{\phi }_0\left(x\right)-\phi \left(x\right)|\le \frac{{(b-a)}^n\beta }{\left(1-M\left({\alpha }^n+L{\alpha }^{n+1}\right)\right)\theta \left(a\right)}\theta \left(x\right),\end{array}$$

(28)

for all $x\in [a,b]$.

Proof. 

Let us consider the operator $T:C^n\left([a,b]\right)\to C^n\left([a,b]\right)$, defined by

$$\begin{array}{ccc}\hfill & & \left(T\phi \right)\left(x\right)={\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F\left(r_1,\phi \left(r_1\right),\right.\hfill \\ & & \left.{\int }_a^{r_1}G\left(r_1,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n,\hfill \end{array}$$

(29)

for all $x\in [a,b]$ and $\phi \in C^n\left([a,b]\right)$.

Following the same ideas as in the proof of Theorem 2, we can prove that T is strictly contractive to the metric (10) due to the fact that

$$M\left({\alpha }^n+L{\alpha }^{n+1}\right)<1.$$

Therefore, we can apply Theorem 1, which ensures that the $\theta$-semi-Hyers–Ulam stability condition is valid for problem (1) and (2). Moreover, using condition (27) and integration, we obtain

$$\begin{array}{ccc}\hfill & & \left|{\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}F\left(r_1,\phi \left(r_1\right),{\int }_a^{r_1}G\left(r_1,t,\right.\right.\right.\hfill \\ & & \left.\left.\left.\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n-\phi \left(x\right)\right|\hfill \\ & & \le {\int }_a^x{\int }_a^{r_n}{\int }_a^{r_{n-1}}\dots {\int }_a^{r_2}\beta d{r}_1\dots d{r}_{n-2}d{r}_{n-1}d{r}_n\hfill \\ & & \le \beta {(b-a)}^n.\hfill \end{array}$$

(30)

Next, using conditions (29) and (30), we obtain

$$\begin{array}{c}\hfill \left|\left(T\phi \right)\left(x\right)-\phi \left(x\right)\right|\le \beta {(b-a)}^n,\end{array}$$

(31)

for all $x\in [a,b]$. From (9), Bielecki metric d, and (31), follows

$$\begin{array}{c}\hfill \underset{x\in [a,b]}{sup}\frac{\left|{\phi }_0\left(x\right)-\phi \left(x\right)\right|}{\theta \left(x\right)}\le \frac{1}{1-M\left({\alpha }^n+L{\alpha }^{n+1}\right)}\underset{x\in [a,b]}{sup}\frac{\beta {(b-a)}^n}{\theta \left(x\right)}.\end{array}$$

(32)

Consequently, considering the definition of $\theta$, the condition (28) holds. □

By using Theorem 3, we can deduce the Hyers–Ulam stability to problem (1) and (2).

Theorem 4.

Let $\theta :[a,b]\to (0,\infty )$ be a non-decreasing continuous function, which fulfills the inequality

$$\begin{array}{c}\hfill {\int }_a^x\theta \left(t\right)dt\le \alpha \theta \left(x\right),\end{array}$$

(33)

for all $x\in [a,b]$, where $\alpha \in \mathbb{R}$. Suppose also that the continuous function $F:[a,b]\times \mathbb{C}\times \mathbb{C}\to \mathbb{C}$ satisfies the condition

$$\begin{array}{c}\hfill \left|F(x,u(x),g(x\left)\right)-F(x,v(x),h(x\left)\right)\right|\le M\left(\left|u\right(x)-v(x\left)\right|+\left|g\right(x)-h(x\left)\right|\right)\end{array}$$

(34)

where $M>0$ is a Lipschitz constant, and the continuous kernel $G:[a,b]\times [a,b]\times {\mathbb{C}}^n\to \mathbb{C}$ satisfies the condition

$$\begin{array}{c}\hfill \left|G\left(x,t,u\left(t\right),u^{\prime }\left(t\right),\dots ,u^{(n-1)}\left(t\right)\right)-G\left(x,t,v\left(t\right),v^{\prime }\left(t\right),\dots ,v^{(n-1)}\left(t\right)\right)\right|\le L|u\left(t\right)-v\left(t\right)|\end{array}$$

(35)

where $L>0$ is a Lipschitz constant. If $\phi \in C^n\left([a,b]\right)$ is such that

$$\begin{array}{c}\hfill \left|F\left(x,\phi \left(x\right),{\int }_a^{x}G\left(x,t,\phi \left(t\right),{\phi }^{\prime }\left(t\right),\dots ,{\phi }^{(n-1)}\left(t\right)\right)dt\right)-{\phi }^{\left(n\right)}\left(x\right)\right|\le \beta ,\end{array}$$

(36)

where $x\in [a,b]$, $\beta \ge 0$, and $M\left({\alpha }^n+L{\alpha }^{n+1}\right)<1$, then there exists a unique function ${\phi }_0\in C^n\left([a,b]\right)$, solution of the problem (1)–(2), such that

$$\begin{array}{c}\hfill |{\phi }_0\left(x\right)-\phi \left(x\right)|\le \frac{{(b-a)}^n\theta \left(b\right)}{\left(1-M\left({\alpha }^n+L{\alpha }^{n+1}\right)\right)\theta \left(a\right)}\beta ,\end{array}$$

(37)

for all $x\in [a,b]$.

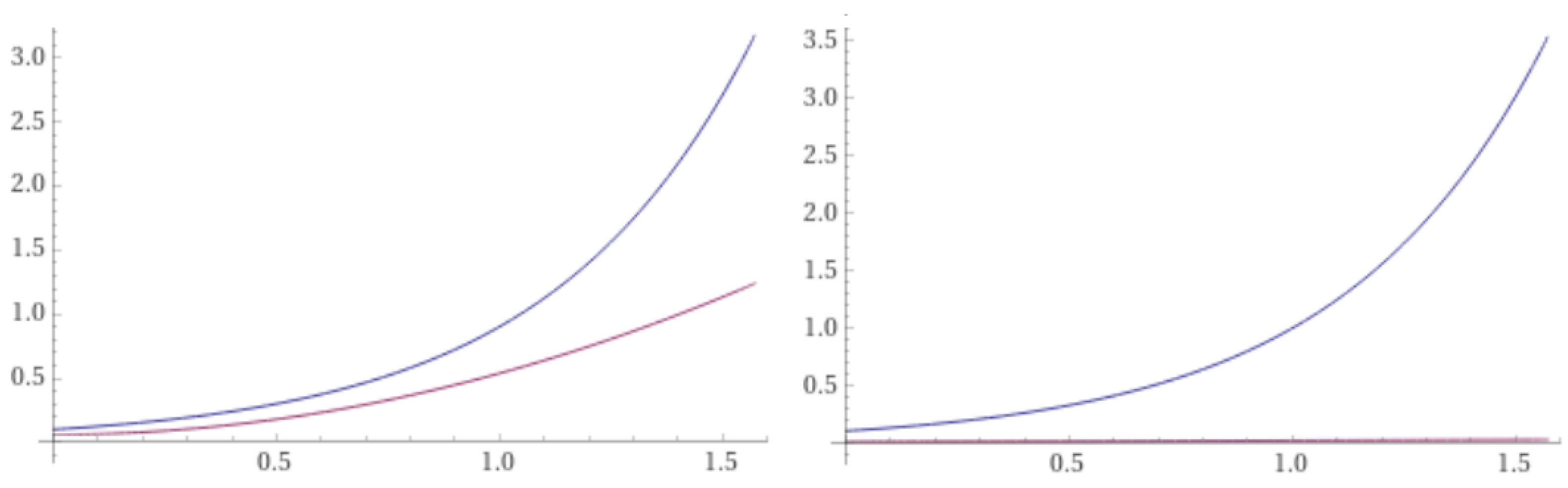
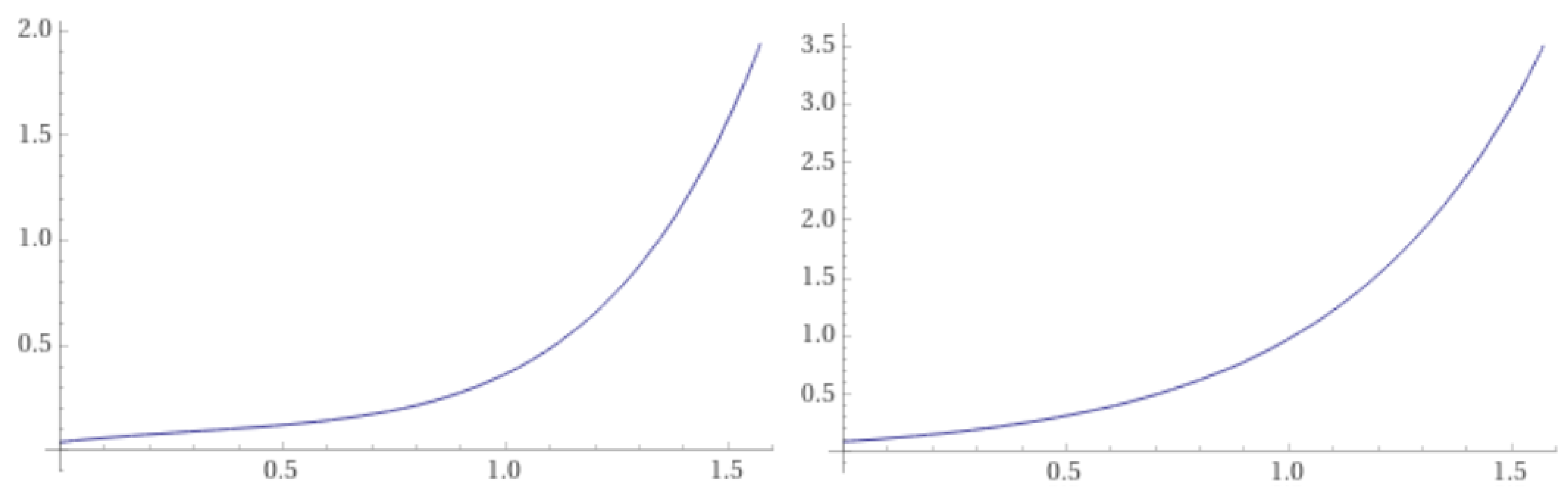
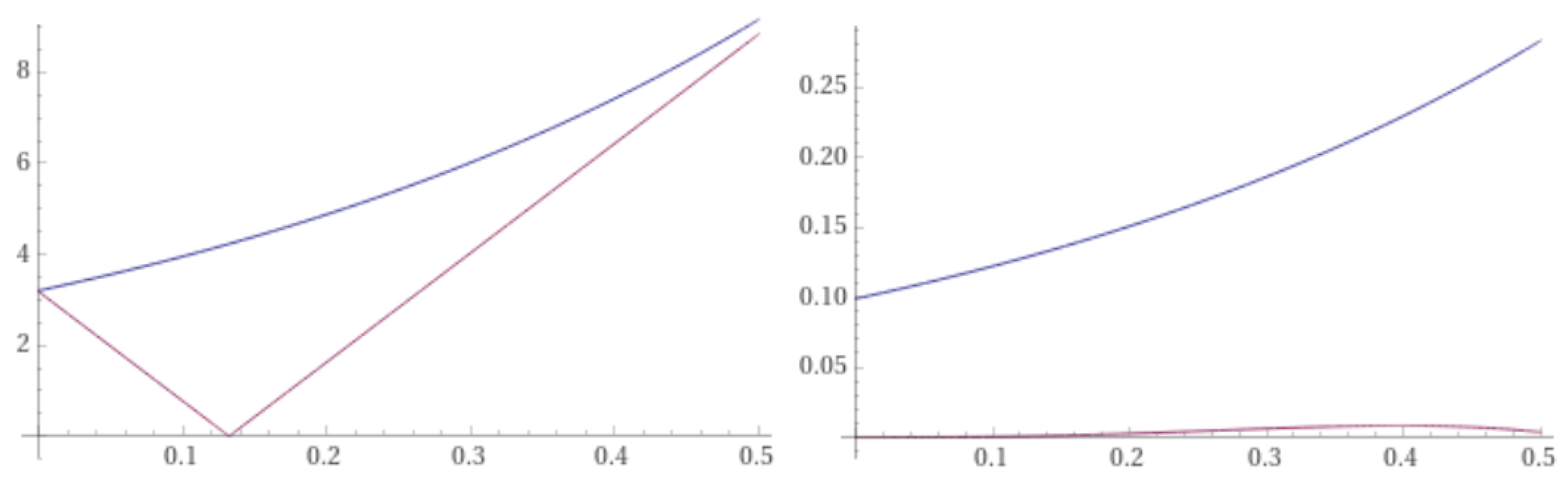
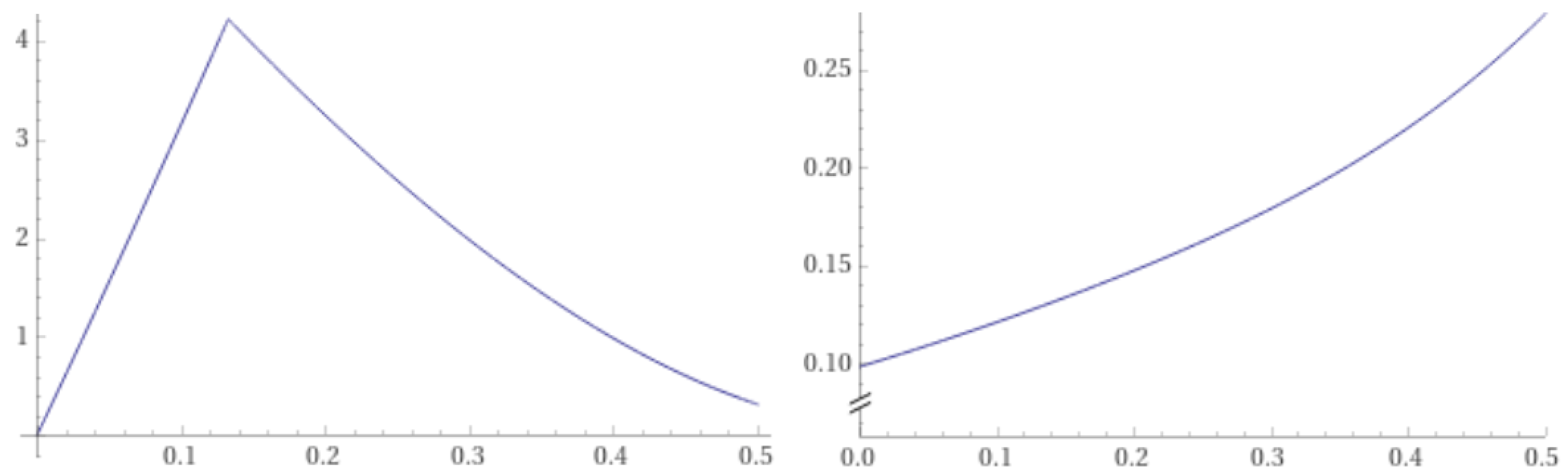
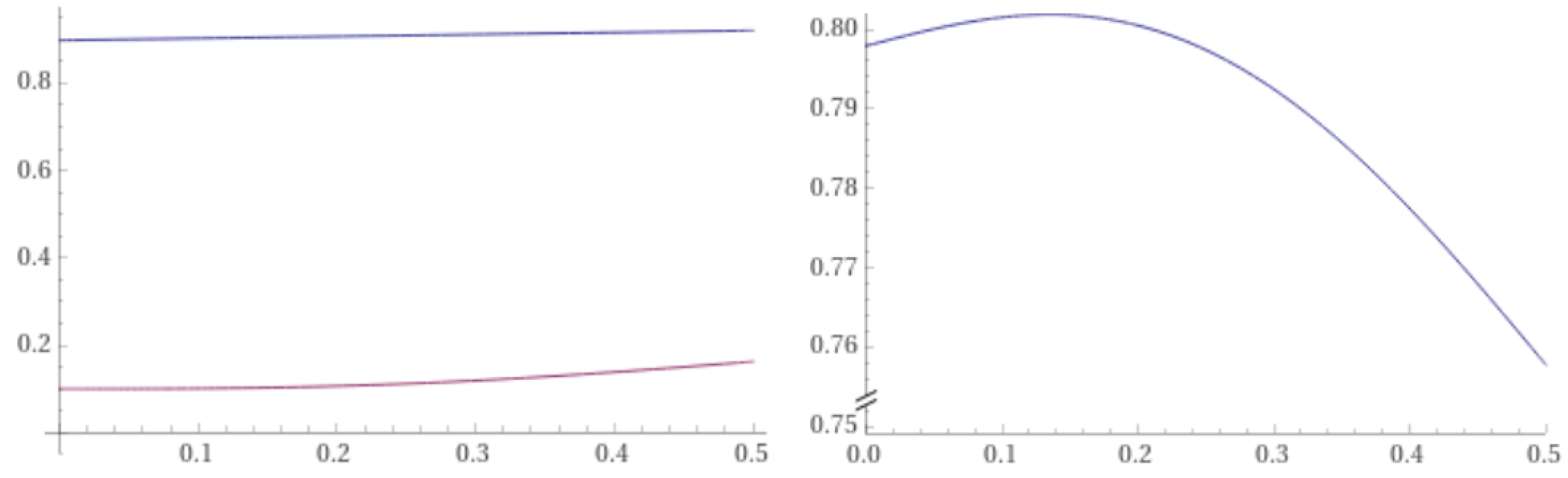
5. Examples

In this section, we present some examples in order to illustrate the results obtained throughout the work.

5.1. First Example: 2-Differentiable Function

Let us define the space

$$\mathbb{D}=\left\{u,v\in C^n\left(\left[0,\frac{\pi }{2}\right]\right):|u^{\prime }\left(x\right)-v^{\prime }\left(x\right)|\le |u\left(x\right)-v\left(x\right)|\right\}.$$

We consider the 2-differentiable functions $\phi :\left[0,\frac{\pi }{2}\right]\to \mathbb{R}$ on the space $\mathbb{D}$, and the integro-differential equation given by

$$\begin{array}{c}\hfill -2\phi \left(x\right)-xsin\left(x\right)-cos\left(x\right)+\frac{x^2}{2}+{\int }_0^{x}t\phi \left(t\right)+{\phi }^{\prime }\left(t\right)dt={\phi }^{″}\left(x\right),\end{array}$$

(38)

with $x\in \left[0,\frac{\pi }{2}\right]$.

We also consider the continuous function $\theta :\left[0,\frac{\pi }{2}\right]\to (0,\infty )$ defined by

$$\theta \left(x\right)=0.1e^{2.2x},$$

which fulfills the inequality

$$\begin{array}{c}\hfill {\int }_0^{x}0.1e^{2.2t}dt\le \alpha 0.1e^{2.2x}=\alpha \theta \left(x\right),\end{array}$$

(39)

with $\alpha \in \left[\frac{10}{22}\left(1-e^{-\frac{22}{20}\pi }\right),\infty \right)$.

Thus, considering these assumptions all the conditions of Theorem 2 are satisfied.

Now, considering the continuous function $F:\left[0,\frac{\pi }{2}\right]\times \mathbb{C}\times \mathbb{C}\to \mathbb{C}$ defined by

$$F(x,u\left(x\right),g\left(x\right))=-2u\left(x\right)-xsin\left(x\right)-cos\left(x\right)+\frac{x^2}{2}+g\left(x\right),$$

we have

$$\begin{array}{c}\hfill \left|F(x,u(x),g(x\left)\right)-F(x,v(x),h(x\left)\right)\right|\le 2\left(\left|u\right(x)-v(x\left)\right|+\left|g\right(x)-h(x\left)\right|\right)\end{array}$$

(40)

with $M=2$.

Moreover, the kernel $G:\left[0,\frac{\pi }{2}\right]\times \left[0,\frac{\pi }{2}\right]\times \mathbb{C}\to \mathbb{C}$ defined by

$$G(x,t,u\left(t\right),u^{\prime }\left(t\right))=tu\left(t\right)+u^{\prime }\left(t\right)$$

is a continuous function satisfying

$$\begin{array}{c}\hfill \left|G(x,t,u\left(t\right),u^{\prime }\left(t\right))-G(x,t,v\left(t\right),v^{\prime }\left(t\right))\right|\le \left(\frac{\pi }{2}+1\right)\left|u\left(t\right)-v\left(t\right)\right|\end{array}$$

(41)

with $L=\frac{\pi }{2}+1$.

Consequently, by choosing $\phi \left(x\right)=cos\left(\frac{101}{100}x\right)-\frac{102}{100}$, it follows

$$\begin{array}{ccc}\hfill & & \left|{\phi }^{″}\left(x\right)+2\phi \left(x\right)+xsin\left(x\right)+cos\left(x\right)-\frac{x^2}{2}-{\int }_0^{x}t\phi \left(t\right)+{\phi }^{\prime }\left(t\right)dt\right|\hfill \\ & & =\left|-\frac{102,050,401}{102,010,000}cos\left(\frac{101}{100}x\right)-\frac{15,226}{255,025}+xsin\left(x\right)+cos\left(x\right)\right.\hfill \\ & & \left.-\frac{49}{100}{x}^2-\frac{100}{101}xsin\left(\frac{101}{100}x\right)\right|\le \theta \left(x\right),\hfill \end{array}$$

(42)

for all $x\in \left[0,\frac{\pi }{2}\right]$. Then, for any

$$\alpha \in \left[\frac{10}{22}\left(1-e^{-\frac{22}{20}\pi }\right),0.474575\right),$$

we have $M({\alpha }^2+L{\alpha }^3)<1$.

Therefore, we have the Hyers–Ulam–Rassias stability of the integro-differential Equation (38).

Moreover, if we consider the exact solution ${\phi }_0\left(x\right)=cos\left(x\right)-1$, we obtain

$$\begin{array}{c}\hfill |{\phi }_0\left(x\right)-\phi \left(x\right)|=\left|cos\left(x\right)-cos\left(\frac{101}{100}x\right)+\frac{1}{50}\right|\le \frac{{\alpha }^2}{1-2\left({\alpha }^2+\left(\frac{\pi }{2}+1\right){\alpha }^3\right)}0.1e^{2.2x}\end{array}$$

(43)

for all $x\in \left[0,\frac{\pi }{2}\right]$.

Both inequalities (42) and (43) are illustrated in Figure 1 and Figure 2.

5.2. Second Example: 3-Differentiable Function

In this example, we consider the 3-differentiable functions $\phi :\left[0,\frac{1}{2}\right]\to \mathbb{R}$, and the integro-differential equation given by

$$\begin{array}{c}\hfill -\frac{1}{6}{x}^3\phi \left(x\right)-\frac{1}{5}x\phi \left(x\right)+24x+{\int }_0^{x}t\phi \left(t\right)+\phi \left(t\right)dt={\phi }^{‴}\left(x\right),\end{array}$$

(44)

with $x\in \left[0,\frac{1}{2}\right]$. Moreover, we consider the continuous function $\theta :\left[0,\frac{1}{2}\right]\to (0,\infty )$ defined by

$$\theta \left(x\right)=3.2e^{2.1x},$$

which fulfills the inequality

$$\begin{array}{c}\hfill {\int }_0^{x}3.2e^{2.1t}dt\le \alpha 3.2e^{2.1x}=\alpha \theta \left(x\right),\end{array}$$

(45)

with $\alpha \in \left[\frac{10}{21}\left(1-e^{-\frac{21}{20}}\right),\infty \right)$. Therefore, all conditions of Theorem 2 are satisfied.

Let us consider the continuous function $F:\left[0,\frac{1}{2}\right]\times \mathbb{C}\times \mathbb{C}\to \mathbb{C}$ defined by

$$F(x,u\left(x\right),g\left(x\right))=-\frac{1}{6}{x}^{3}u\left(x\right)-\frac{1}{5}xu\left(x\right)+24x+g\left(x\right),$$

which verifies

$$\begin{array}{c}\hfill \left|F(x,u(x),g(x\left)\right)-F(x,v(x),h(x\left)\right)\right|\le |u\left(x\right)-v\left(x\right)|+|g\left(x\right)-h\left(x\right)|\end{array}$$

(46)

with $M=1$. Furthermore, the kernel $G:\left[0,\frac{1}{2}\right]\times \left[0,\frac{1}{2}\right]\times \mathbb{C}\to \mathbb{C}$ defined by

$$G(x,t,u\left(t\right),u^{\prime }\left(t\right))=tu\left(t\right)+u\left(t\right)$$

is a continuous function and verifies

$$\begin{array}{c}\hfill \left|G(x,t,u\left(t\right),u^{\prime }\left(t\right))-G(x,t,v\left(t\right),v^{\prime }\left(t\right))\right|\le \frac{5}{4}\left|u\left(t\right)-v\left(t\right)\right|\end{array}$$

(47)

with $L=\frac{5}{4}$. Now, if we choose $\phi \left(x\right)=0.53x^3$, it follows

$$\begin{array}{c}\hfill \left|{\phi }^{‴}\left(x\right)+\frac{1}{6}{x}^3\phi \left(x\right)+\frac{1}{5}x\phi \left(x\right)-24x-{\int }_0^{x}t\phi \left(t\right)+\phi \left(t\right)dt\right|=\left|\frac{318}{100}-24x\right|\le \theta \left(x\right),\end{array}$$

(48)

for all $x\in \left[0,\frac{1}{2}\right]$. Additionally, for any $\alpha \in \left[\frac{10}{21}\left(1-e^{-\frac{21}{20}}\right),0.794596\right)$ we have $M({\alpha }^3+L{\alpha }^4)<1$. Consequently, we have the Hyers–Ulam–Rassias stability of the integro-differential Equation (44). If we consider the exact solution ${\phi }_0\left(x\right)=x^4$, we obtain

$$\begin{array}{c}\hfill |{\phi }_0\left(x\right)-\phi \left(x\right)|=\left|0.53x^3-x^4\right|\le \frac{{\alpha }^3}{1-\left({\alpha }^3+\frac{5}{4}{\alpha }^4\right)}3.2e^{2.1x}\end{array}$$

(49)

for all $x\in \left[0,\frac{1}{2}\right]$, which is in accordance with the presented results.

Both inequalities (48) and (49) are illustrated in Figure 3 and Figure 4.

5.3. Third Example: 3-Differentiable Function and a Bigger Perturbation

We will consider the integro-differential Equation (44) but with another function $\theta$ and a bigger perturbation of the solution. Considering the continuous function $\theta :\left[0,\frac{1}{2}\right]\to [0,\infty )$ defined by

$$\theta \left(x\right)=6+0.3x,$$

we have

$$\begin{array}{c}\hfill {\int }_0^{x}6+0.3tdt\le \alpha (0.6+0.3x)=\alpha \theta \left(x\right),\end{array}$$

(50)

with $\alpha \in \left[\frac{81}{164},\infty \right)$.

Choosing $\phi \left(x\right)=x^3+0.1$, it follows

$$\begin{array}{ccc}\hfill & & \left|{\phi }^{‴}\left(x\right)+\frac{1}{6}{x}^3\phi \left(x\right)+\frac{1}{5}x\phi \left(x\right)-24x-{\int }_0^{x}t\phi \left(t\right)+\phi \left(t\right)dt\right|\hfill \\ & & =\left|-\frac{1}{30}{x}^6-\frac{1}{20}{x}^4-\frac{1}{30}{x}^3-\frac{602}{25}x+6\right|\le \theta \left(x\right),\hfill \end{array}$$

(51)

for all $x\in \left[0,\frac{1}{2}\right]$.

To illustrate the inequality (51), we have Figure 5.

Therefore, this result exhibits the Hyers–Ulam–Rassias stability of the integro-differential Equation (44).

Moreover, by using the exact solution ${\phi }_0\left(x\right)=x^4$ with

$$\begin{array}{c}\hfill \alpha \in \left[\frac{81}{164},0.794596\right]\end{array}$$

(52)

we obtain $M({\alpha }^3+L{\alpha }^4)<1$, and

$$\begin{array}{c}\hfill |{\phi }_0\left(x\right)-\phi \left(x\right)|=\left|x^3+0.1-x^4\right|\le \frac{{\alpha }^3}{1-\left({\alpha }^3+\frac{5}{4}{\alpha }^4\right)}(6+0.3x)\end{array}$$

(53)

for all $x\in \left[0,\frac{1}{2}\right]$.

To illustrate the inequality (53), we have Figure 6.

6. Conclusions

In this work, we presented new sufficient conditions for the Hyers–Ulam–Rassias, the Hyers–Ulam and the $\sigma$-semi-Hyers–Ulam stabilities for a general higher order integro-differential equation by using the Banach fixed point theorem and a generalization of the Bielecki metric thus enabling the study of the stability of an expanding number of particular equations. Some examples were presented to illustrate the theoretical results.