Dynamic Response of the Newton Voigt–Kelvin Modelled Linear Viscoelastic Systems at Harmonic Actions
Abstract
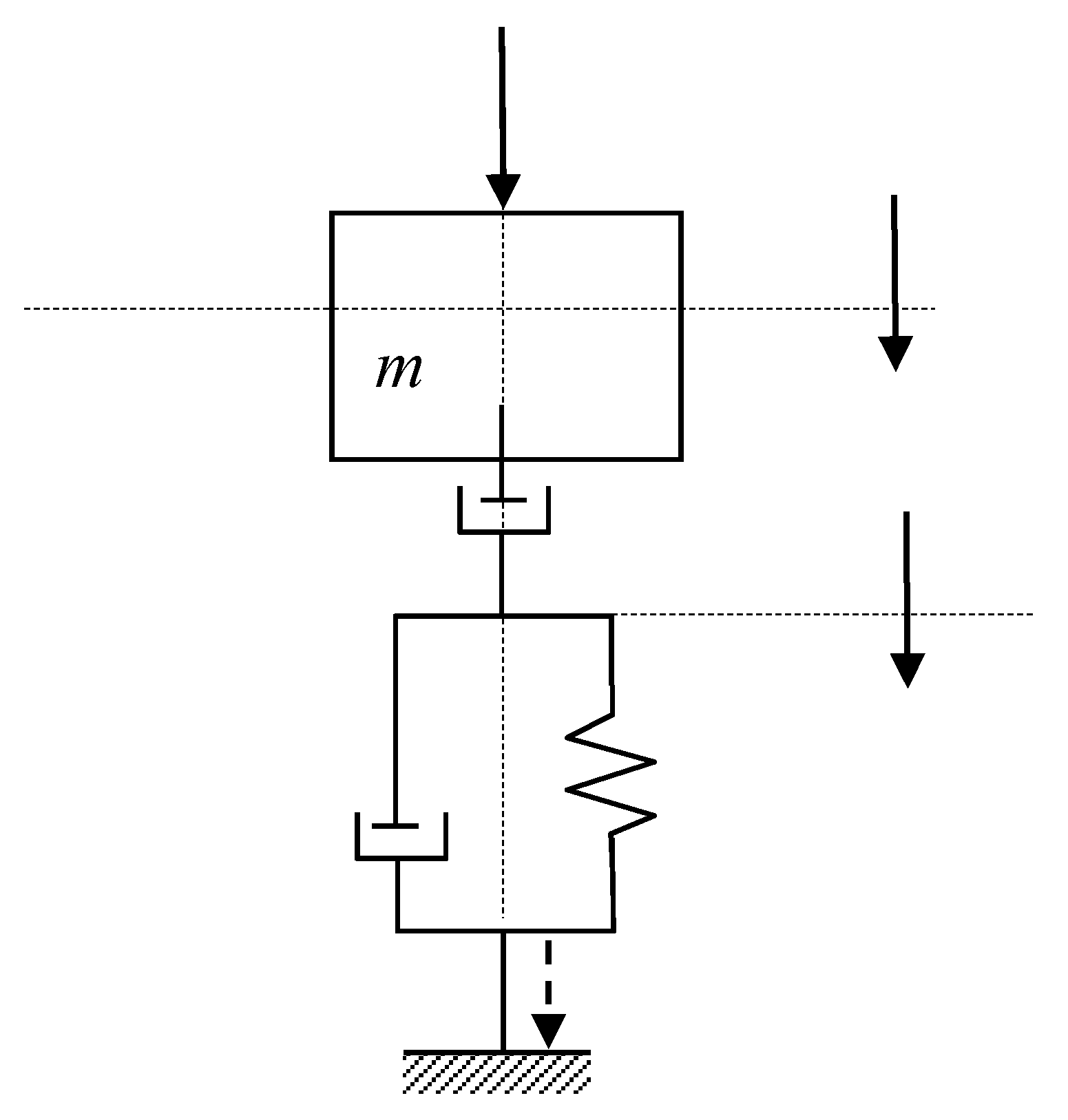
1. Introduction
2. Dynamic Response at Displacements
- with and
- with
- for we have
- for we have
3. Transmitted Dynamic Force
4. Dynamic Insulation Capacity
5. Deformation of Damper Systems
6. Dissipated Energy
7. Conclusions
Funding
Conflicts of Interest
References
- Bratu, P. Evaluation of the Dissipated Energy for Viscoelastic and Hysteretic Soil Systems for Road Works; Test Report for Bechtel company—“Transylvania Highway”; ICECON SA: Bucharest, Romania, 2012. [Google Scholar]
- Bratu, P. Dynamic Stress Dissipated Energy Rating of Materials with Maxwell Rheological Behaviour. Appl. Mech. Mater. 2015, 801, 115–121. [Google Scholar] [CrossRef]
- Bratu, P. The Behaviour of Nonlinear Viscoelastic Systems Subjected to Harmonic Dynamic Excitation. In Proceedings of the 9th International Congress on Sound and Vibration, University of Central Florida Orlando, Orlando, FL, USA, 8–11 July 2002. [Google Scholar]
- Bratu, P. Dynamic response of nonlinear systems under stationary harmonic excitation. In Proceedings of the Non-Linear Acoustics and Vibration, 11th International Congress on Sound and Vibration, St. Petersburg, Russia, 5–8 July 2004; pp. 2767–2770. [Google Scholar]
- Bratu, P. Dynamic analysis in case of compaction vibrating rollers intended for road works. In Proceedings of the 17th International Congress on Sound& Vibration, ICSV, Cairo, Egypt, 18–22 July 2010. [Google Scholar]
- Bratu, P.; Stuparu, A.; Popa, S.; Iacob, N.; Voicu, O. The Assessment of the Dynamic Response to Seismic Excitation for Constructions Equipped with Base Isolation Systems According to the Newton-Voigt-Kelvin Model. Acta Tech. Napoc. Ser. Appl. Math. Eng. 2017, 60, 459–464. [Google Scholar]
- Bratu, P.; Stuparu, A.; Leopa, A.; Popa, S. The Dynamic Analyses of a Construction with the Base Insulation Consisting in Anti-Seismic Devices Modelled as a Hooke-Voigt-Kelvin Linear Rheological System. Acta Tech. Napoc. Ser. Appl. Math. Eng. 2018, 60, 465–472. [Google Scholar]
- Bratu, P.; Stuparu, A.; Popa, S.; Iacob, N.; Voicu, O.; Iacob, N.; Spanu, G. The Dynamic Isolation Performances Analysis of the Vibrating Equipment with Elastic Links to a Fixed Base. Acta Tech. Napoc. Ser. Appl. Math. Eng. 2018, 61, 23–28. [Google Scholar]
- Bratu, P. Hysteretic Loops in Correlation with the Maximum Dissipated Energy, for Linear Dynamic Systems. Symmetry 2019, 11, 315. [Google Scholar] [CrossRef]
- Bratu, P.; Dobrescu, C.F. Dynamic Response of Zener-Modelled Linearly Viscoelastic Systems under Harmonic Excitation. Symmetry 2019, 11, 1050. [Google Scholar] [CrossRef]
- Bratu, P.; Buruga, A.; Chilari, O.; Ciocodeiu, A.; Oprea, I. Evaluation of the Linear Viscoelastic Force for a Dynamic System (m, c, k) Excited with a Rotating Force. RJAV 2019, 16, 39–46. [Google Scholar]
- Dobrescu, C.F. Analysis of the dynamic regime of forced vibrations in the dynamic compacting process with vibrating roller. Acta Tech. Napoc. Appl. Math. Mech. Eng. 2019, 62, 71–76. [Google Scholar]
- Dobrescu, C.F. Highlighting the Change of the Dynamic Response to Discrete Variation of Soil Stiffness in the Process of Dynamic Compaction with Roller Compactors Based on Linear Rheological Modelling. In Acoustics & Vibration of Mechanical Structures II; Herisanu, N., Marinca, V., Eds.; [ISI Web of Science (WoS)]; Trans Tech Publications Ltd.: Freienbach, Switzerland, 2015; Volume 801, pp. 242–248. ISBN 978-3-03835-628-8. [Google Scholar] [CrossRef]
- Dobrescu, C.F.; Brăguţă, E. Optimization of Vibro-Compaction Technological Process. Considering Rheological Properties. In Proceedings of the 14th AVMS Conference, Timisoara, Romania, 25–26 May 2017; Nicolae, H., Vasile, M., Eds.; Springer: Berlin/Heidelberg, Germany; pp. 287–293. [Google Scholar] [CrossRef]
- Morariu-Gligor, R.M.; Crisan, A.V.; Şerdean, F.M. Optimal Design of a One-Way Plate Compactor. Acta Tech. Napoc. Ser. Appl. Math. Mech. Eng. 2017, 60, 557–564. [Google Scholar]
- Mooney, M.A.; Rinehart, R.V. Field Monitoring of Roller Vibration during Compaction of Subgrade Soil. J. Geotech. Geoenviron. Eng. ASCE 2007, 133, 257–265. [Google Scholar] [CrossRef]
- Mooney, M.A.; Rinehart, R.V. In-Situ Soil Response to Vibratory Loading and Its Relationship to Roller-Measured Soil Stiffness. J. Geotech. Geoenviron. Eng. ASCE 2009, 135, 1022–1031. [Google Scholar] [CrossRef]
- Vucetic, M.; Dobry, R. Effect of Soil Elasticity on Cyclic Response. J. Geotech. Eng. 1991, 117, 89–107. [Google Scholar] [CrossRef]
- Wersäll, C.; Larsson, S.; Bodare, A. Dynamic Response of Vertically Oscillating Foundation At. large Strain. In Proceedings of the 14th International Conference of the International Association for Computer Methods and Advances in Geomechanics, Kyoto, Japan, 22–25 September 2014; pp. 643–647. [Google Scholar]
- Wersäll, C.; Larsson, S.; Ryden, N.; Nordfeld, I. Frequency Variable Surface Compaction of Sand Using Rotating Mass Oscillators. Geotech. Test. J. 2015, 38, 1–10. [Google Scholar] [CrossRef]
- Wersäll, C.; Nordfeld, I.; Larsson, S. Soil Compaction by Vibratory Roller with Variable Frequency; Submitted to Geotechnics for Sustainable Infrastructure Development—Geotech: Hanoi, Vietnam; Available online: https://geotechn.vn/geotec-hanoi-rewind/geotec-hanoi-2016/ (accessed on 24 November 2016).
- Yoo, T.-S.; Selig, E.T. Dynamics of Vibratory Roller Compaction. J. Geotech. Eng. Div. 1979, 105, 1211–1231. [Google Scholar]
- ICECON. Test. Report for Laboratory and “In Situ” Tests Performed on the Road Layers of the Bucureşti-Constanța Highway; ICECON: Bucharest, Romania, 2004. [Google Scholar]
- Lucas, E.F.; Soares, B.G.; Monteiro, E. Caracterização de Polimeros; e-Papers: Rio de Janeiro, Brazil, 2001. [Google Scholar]
- Gedde, U.W. Polymer Physics; Kluver: Dordrecht, The Netherlands; Boston, MA, USA; London, UK, 2001. [Google Scholar]
- ICECON. Test. Report for Laboratory and “In Situ” Tests for the Lugoj-Deva Highway; ICECON: Bucharest, Romania, 2015. [Google Scholar]
- ICECON. Test. Report for Laboratory and “In Situ” Tests for the Timisoara-Lugoj Highway; ICECON: Bucharest, Romania, 2016. [Google Scholar]
- ICECON. Test. Report for Laboratory and “In Situ” Tests. Transylvania Highway, Bechtel Romania; ICECON: Bucharest, Romania, 2008. [Google Scholar]
- Menard, K.P. Dynamic Mechanical Analysis: An. Introduction, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2008. [Google Scholar]
- Brostow, W.; Hagg Lobland, H.E. Materials: Introduction and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]





© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dobrescu, C. Dynamic Response of the Newton Voigt–Kelvin Modelled Linear Viscoelastic Systems at Harmonic Actions. Symmetry 2020, 12, 1571. https://doi.org/10.3390/sym12091571
Dobrescu C. Dynamic Response of the Newton Voigt–Kelvin Modelled Linear Viscoelastic Systems at Harmonic Actions. Symmetry. 2020; 12(9):1571. https://doi.org/10.3390/sym12091571
Chicago/Turabian StyleDobrescu, Cornelia. 2020. "Dynamic Response of the Newton Voigt–Kelvin Modelled Linear Viscoelastic Systems at Harmonic Actions" Symmetry 12, no. 9: 1571. https://doi.org/10.3390/sym12091571
APA StyleDobrescu, C. (2020). Dynamic Response of the Newton Voigt–Kelvin Modelled Linear Viscoelastic Systems at Harmonic Actions. Symmetry, 12(9), 1571. https://doi.org/10.3390/sym12091571




