Impacts of Viscous Dissipation and Brownian motion on Jeffrey Nanofluid Flow over an Unsteady Stretching Surface with Thermophoresis
Abstract
1. Introduction
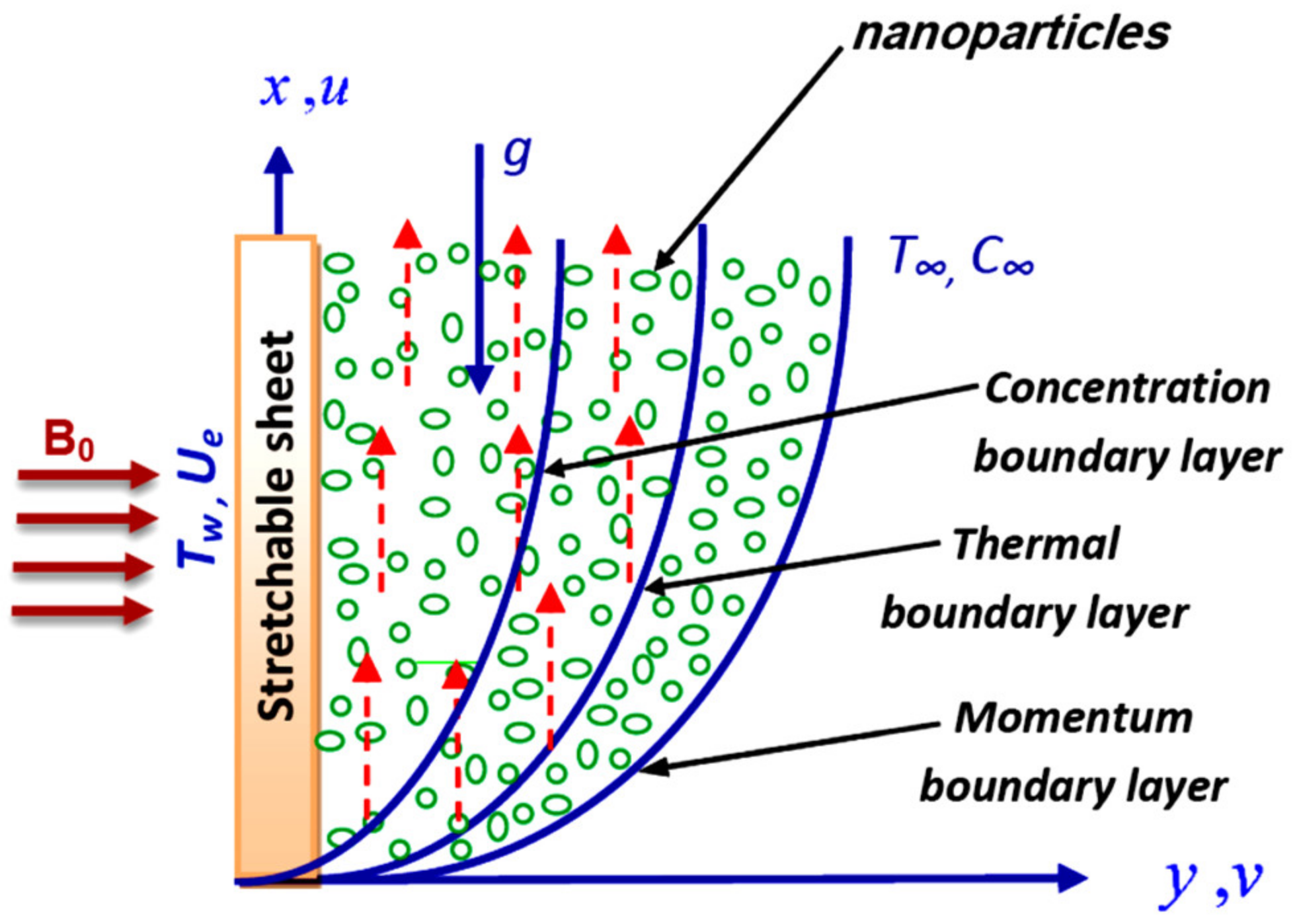
2. Modeling
3. Fourth-Order Finite Difference Continuation Method (FFDCM)
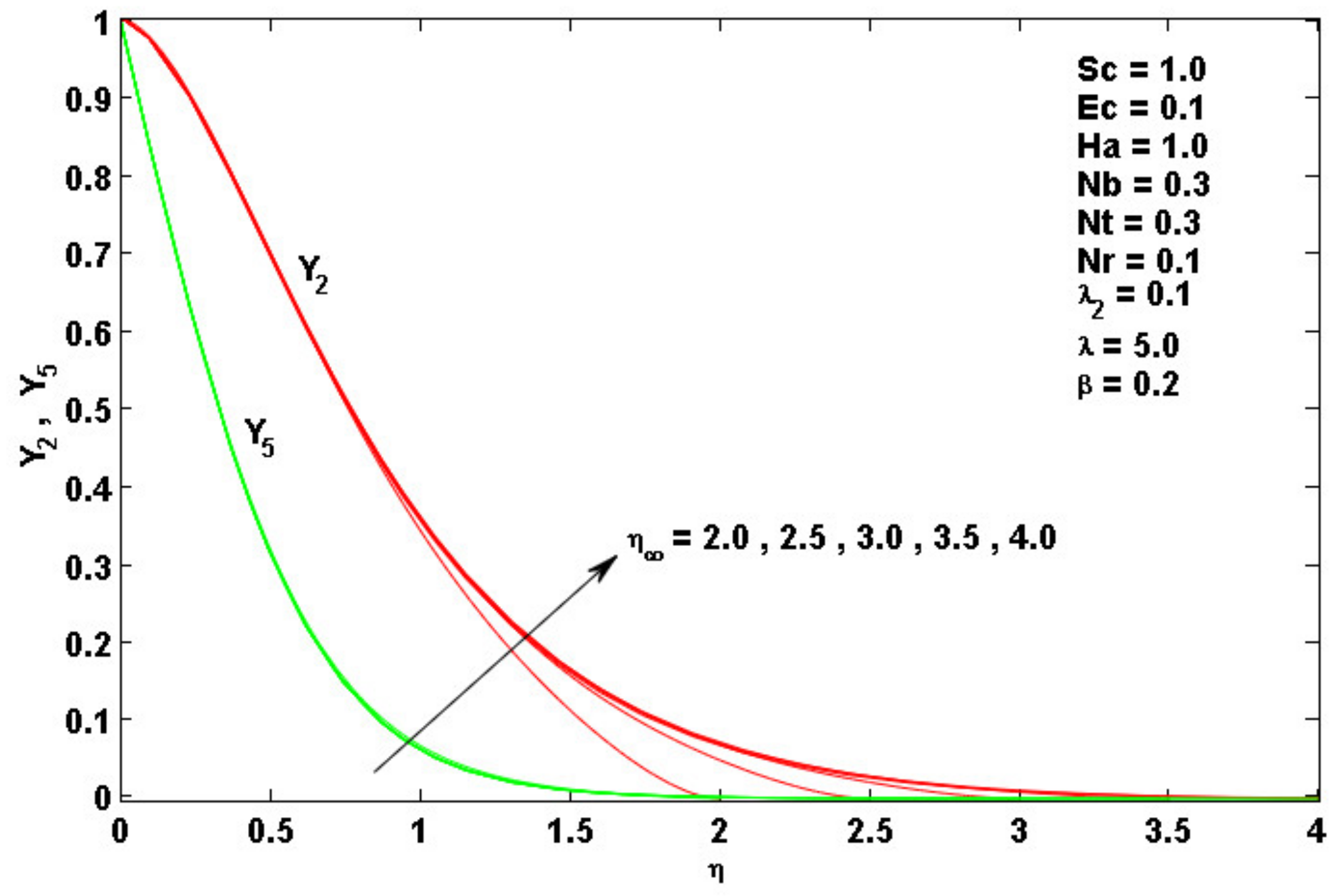
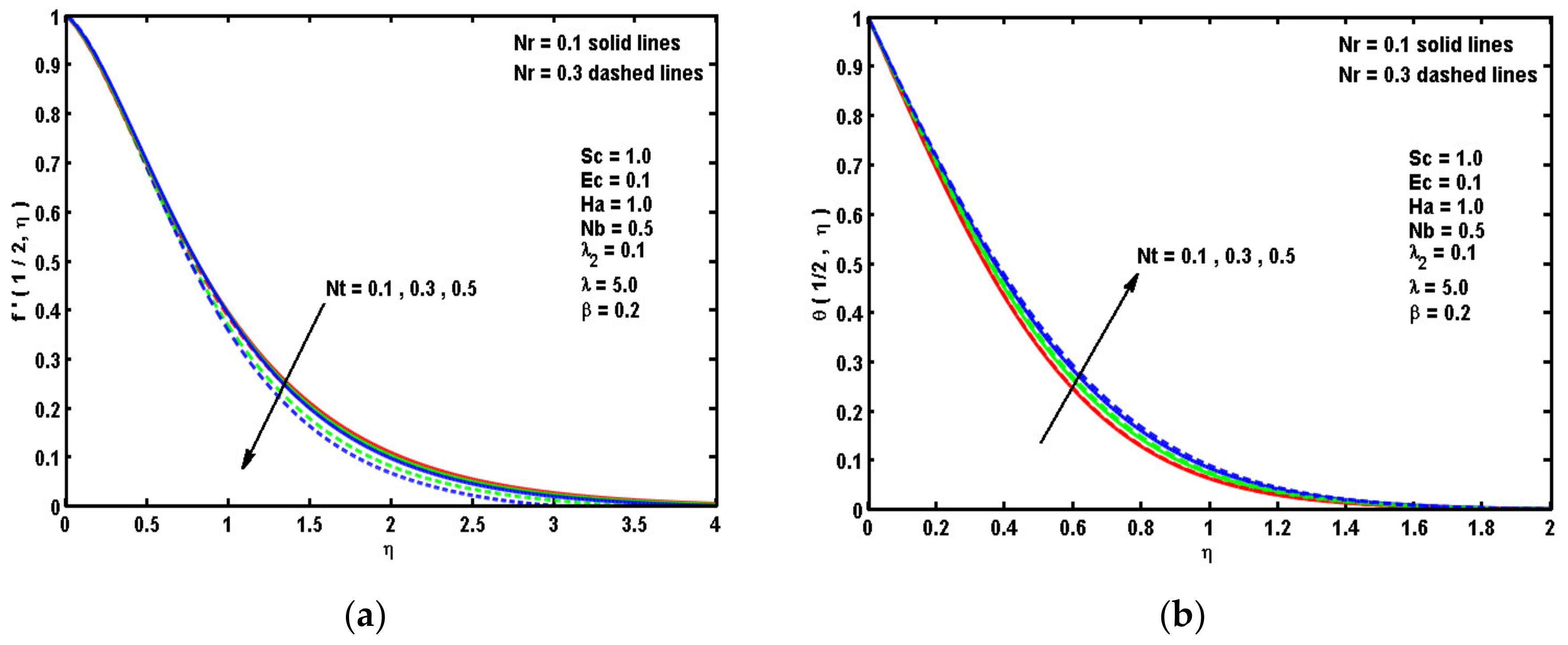
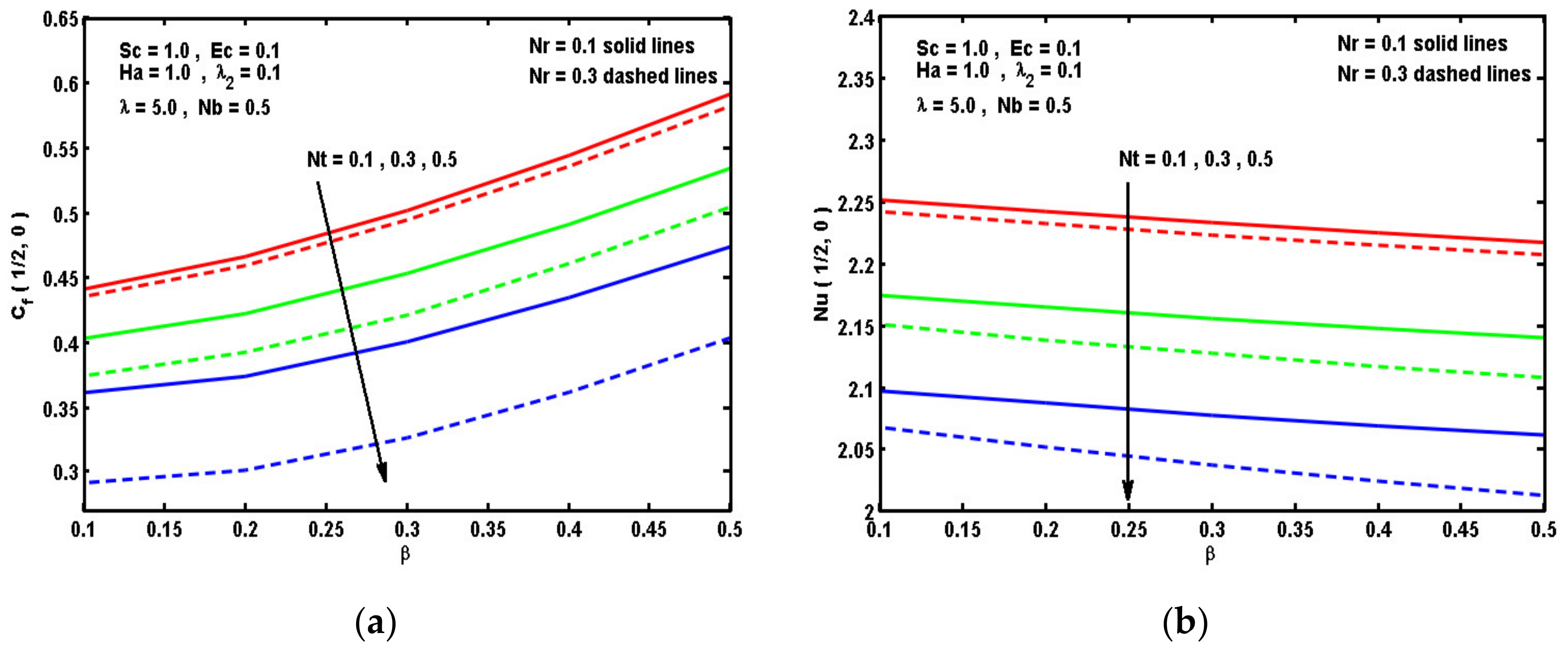
4. Discussion
5. Conclusions
- A comparative investigation among the current outcomes and the former cited investigation are explored to trust our outcomes and a notable agreement is observed. The following observations are structured for the current investigation:
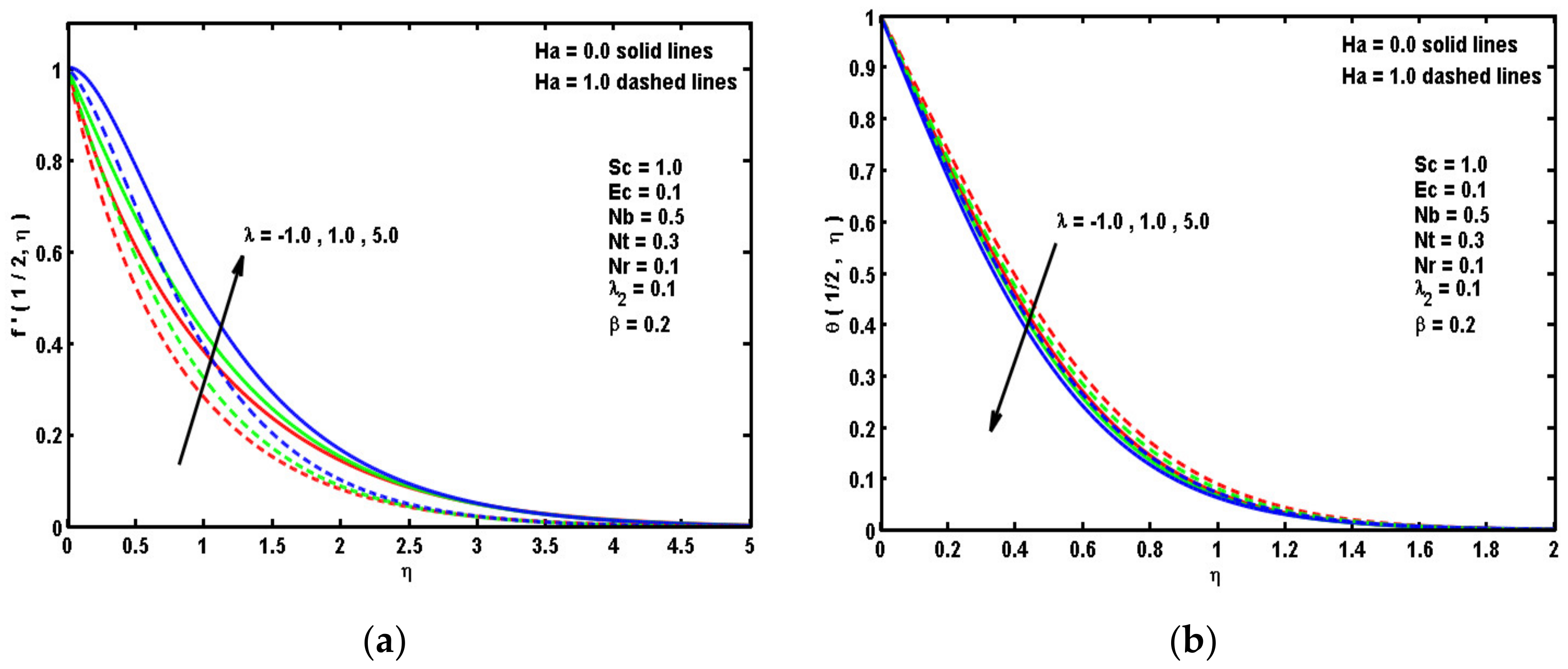
- Augmentation in buoyance ratio and thermophoretic parameters leads to diminish the velocity curves and increase the temperature curves ability that boosts the thermal boundary.
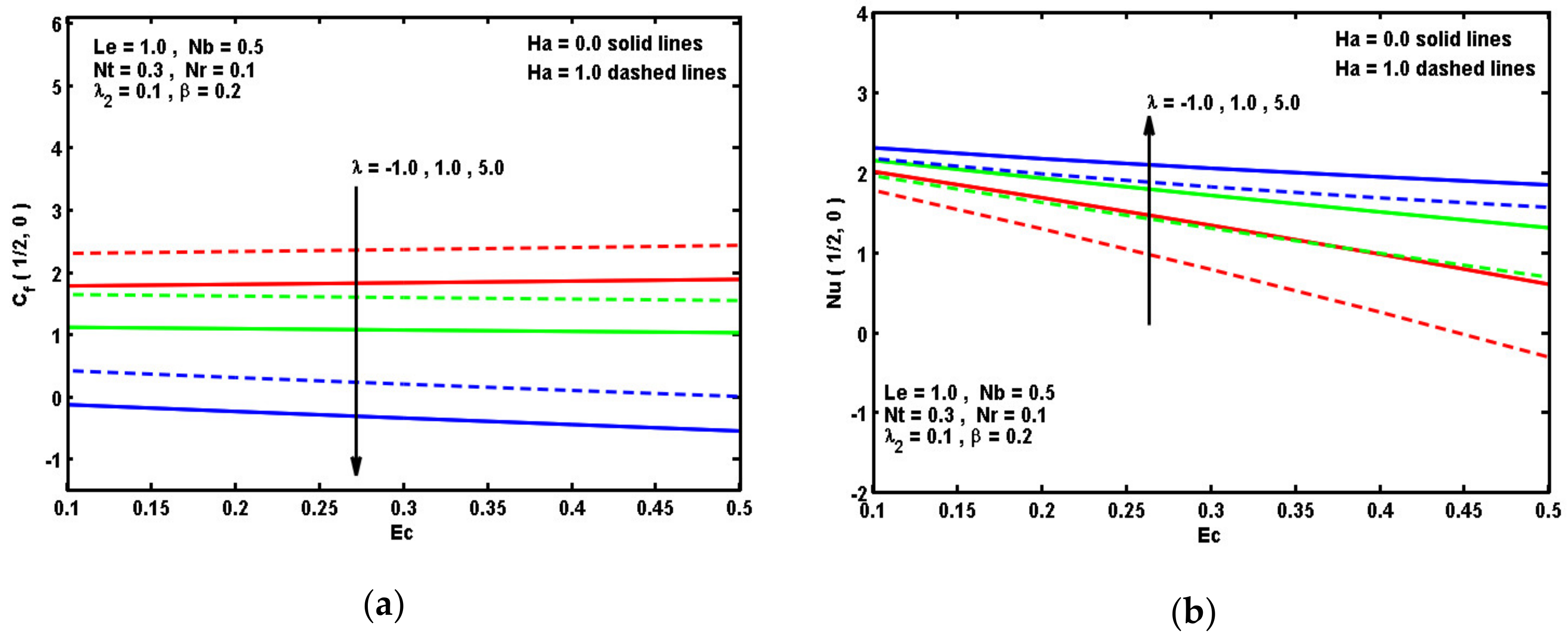
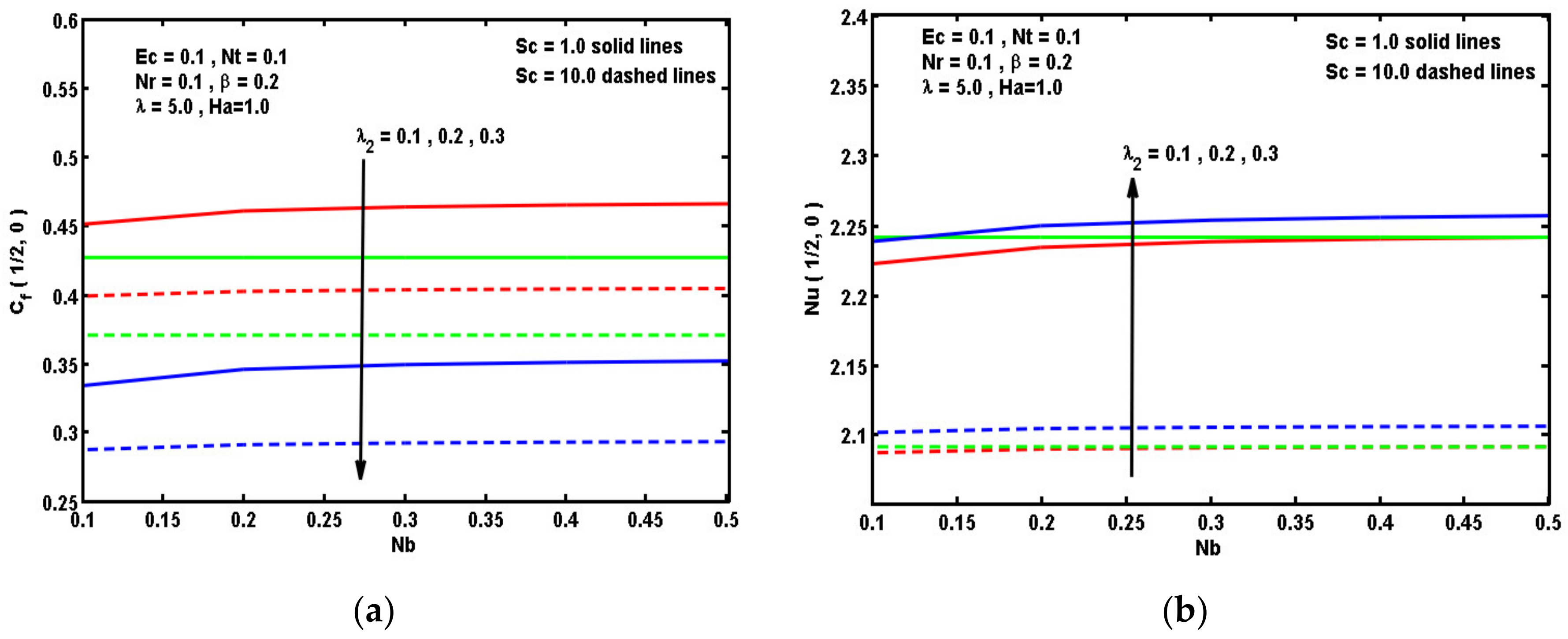
- A greater Deborah number exhibits increasing skin friction and salient decreasing heat transmission.
- The Nusselt number enhances and skin friction reduces for the improved magnetic strength, while an opposite reaction is found with mixed convection aspects.
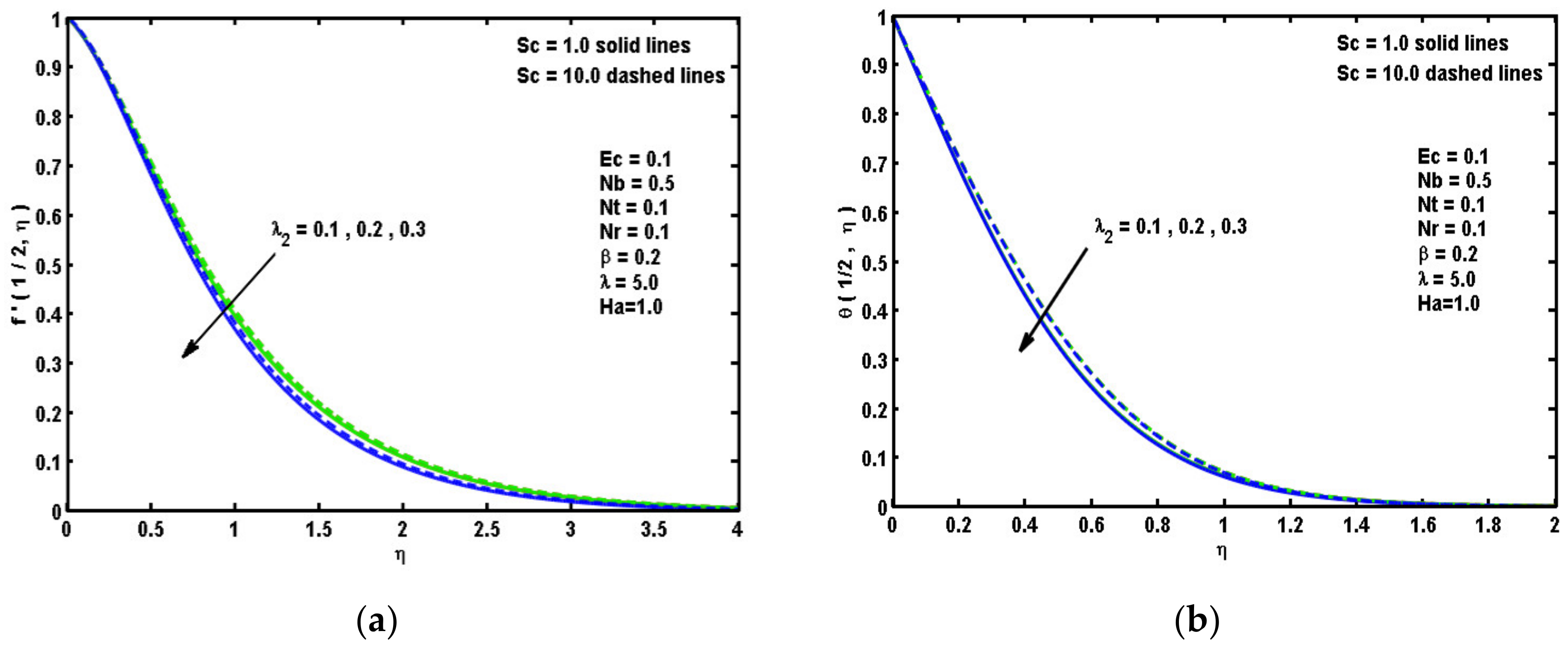
- Both velocity and temperature curves decline with the enhancement in the ratio of relaxation to retardation times while opposite results are obtained for the intensity of Schmidt number.
Author Contributions
Funding
Conflicts of Interest
References
- Choi, S.U.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles; Argonne National Lab.: Lemont, IL, USA, 1995.
- Buongiorno, J. Convective transport in nanofluids. J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Kumar, K.G.; Ramesh, G.K.; Gireesha, B.J.; Rashad, A.M. On Stretched Magnetic Flow of Carreau Nanofluid with Slip Effects and Nonlinear Thermal Radiation. Nonlinear Eng. 2019, 8, 340–349. [Google Scholar] [CrossRef]
- EL-Zahar, E.R.; Rashad, A.M.; Seddek, L.F. The Impact of Sinusoidal Surface Temperature on the Natural Convective Flow of a Ferrofluid along a Vertical Plate. Mathematics 2019, 7, 1014. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Rashad, A.M. MHD Forced Convection Flow of a Nanofluid Adjacent to a Non-isothermal Wedge. Comput. Therm. Sci. 2014, 6, 27–39. [Google Scholar] [CrossRef]
- Murthy, P.V.S.N.; RamReddy, C.; Chamkha, A.J.; Rashad, A.M. Significance of Viscous Dissipation and Chemical Reaction on Convective Transport In A Boundary Layer Stagnation Point Flow Past A Stretching/Shrinking Sheet In A Nanofluid. J. Nanofluids 2015, 4, 214–222. [Google Scholar] [CrossRef]
- EL-Zahar, E.R.; Rashad, A.M.; Gelany, A.M. Studying High Suction Effect on Boundary-Layer Flow of a Nanofluid on Permeable Surface via Singular Perturbation Technique. J. Comput. Theor. Nanosci. 2015, 12, 4828–4836. [Google Scholar] [CrossRef]
- Reddy, P.B.A. Magnetohydrodynamic flow of a Casson fluid over an exponentially inclined permeable stretching surface with thermal radiation and chemical reaction. Ain Shams Eng. J. 2016, 7, 593–602. [Google Scholar] [CrossRef]
- Rashad, A.M.; Mallikarjuna, B.; Chamkha, A.J.; Raju, S.H. Thermophoresis Effect on Heat and Mass Transfer from A Rotating Cone In A Porous Medium With Thermal Radiation. Afr. Mat. 2016, 27, 1409–1424. [Google Scholar] [CrossRef]
- Sheikholeslami, L.Z.M.; Chamkha, A.J.; Raizah, Z.A.; Saleem, S. Control volume finite element method for nanofluid MHD natural convective flow inside a sinusoidal annulus under the impact of thermal radiation. Comput. Methods Appl. Mech. Eng. 2018, 338, 618–633. [Google Scholar]
- Nasir, S.; Islam, S.; Gul, T.; Khan, Z.S.; Khan, M.A.; Khan, W.; Khan, S. Three-dimensional rotating flow of MHD single wall carbon nanotubes over a stretching sheet in presence of thermal radiation. Appl. Nanosci. 2018, 8, 1361–1378. [Google Scholar] [CrossRef]
- Alwawi, F.A.; Alkasasbeh, H.T.; Rashad, A.M.; Idris, R. MHD Natural Convection of Sodium Alginate Casson Nanofluid Over A Solid Sphere. Results Phys. 2020, 16, 102818. [Google Scholar] [CrossRef]
- Nabwey, H.A.; Khan, W.A.; Rashad, A.M. Lie Group Analysis of Unsteady Flow of Kerosene/Cobalt Ferrofluid Past A Radiated Stretching Surface with Navier Slip and Convective Heating. Mathematics 2020, 8, 826. [Google Scholar] [CrossRef]
- Sandeep, N.; Sulochana, C.; Isaac Lare, A. Stagnation-point flow of a Jeffrey nanofluid over a stretching surface with induced magnetic field and chemical reaction. Int. J. Eng. Res. Afr. 2015, 20, 93–111. [Google Scholar] [CrossRef]
- Dalir, N.; Dehsara, M.; Salman Nourazar, S. Entropy analysis for magnetohydrodynamic flow and heat transfer of a jeffrey nanofluid over a stretching sheet. Energy 2015, 79, 351–362. [Google Scholar] [CrossRef]
- Hayat, T.; Imtiaz, M.; Alsaedi, A. Magnetohydrodynamic stagnation point flow of a Jeffrey nanofluid with newtonian heating. J. Aerosp. Eng. 2016, 29, 04015063. [Google Scholar] [CrossRef]
- Shehzad, S.A.; Abdullah, Z.; Alsaedi, A.; Abbasi, F.M.; Hayat, T. Thermally radiative three-dimensional flow of jeffrey nanofluid with internal heat generation and magnetic field. J. Magn. Magn. Mater. 2016, 397, 108–114. [Google Scholar] [CrossRef]
- Raju, C.S.K.; Jayachandra Babu, M.; Sandeep, N. Chemically reacting radiative MHD Jeffrey nanofluid flow over a cone in porous medium. Int. J. Eng. Res. Afr. 2015, 19, 75–90. [Google Scholar] [CrossRef]
- Hayat, T.; Asad, S.; Alsaedi, A. Analysis for flow of Jeffrey fluid with nanoparticles. Chin. Phys. B 2015, 24, 044702. [Google Scholar] [CrossRef]
- Nadeem, S.; Saleem, S. An optimized study of mixed convection flow of a rotating Jeffrey nanofluid on a rotating vertical cone. J. Thermophys. Heat Transf. 2015, 12, 3028–3035. [Google Scholar] [CrossRef]
- Zin, N.A.M.; Khan, I.; Shafie, S.; Alshomrani, A.S. Analysis of heat transfer for unsteady MHD free convection flow of rotating Jeffrey nanofluid saturated in a porous medium. Results Phys. 2017, 7, 288–309. [Google Scholar]
- Hsiao, K.L. To promote radiation electrical MHD activation energy thermal extrusion manufacturing system efficiency by using Carreau-Nanofluid with parameters control method. Energy 2017, 130, 486–499. [Google Scholar] [CrossRef]
- Hsiao, K.L. Combined electrical MHD heat transfer thermal extrusion system using Maxwell fluid with radiative and viscous dissipation effects. Appl. Therm. Eng. 2017, 112, 1281–1288. [Google Scholar] [CrossRef]
- Hsiao, K.L. Micropolar nanofluid flow with MHD and viscous dissipation effects towards a stretching sheet with multimedia feature. Int. J. Heat Mass Transf. 2017, 112, 983–990. [Google Scholar] [CrossRef]
- Hsiao, K.L. Stagnation electrical MHD nanofluid mixed convection with slip boundary on a stretching sheet. Appl. Therm. Eng. 2016, 98, 850–861. [Google Scholar] [CrossRef]
- Habib, H.M.; El-Zahar, E.R. An algorithm for solving singular perturbation problems with mechanization. Appl. Math. Comput. 2007, 188, 286–302. [Google Scholar] [CrossRef]
- Burden, L.R.; Faires, J.D.; Reynolds, A.C. Numerical Analysis: Teachers Manual to Accompany; Prindle Weber Schmidt: Boston, MA, USA, 1981. [Google Scholar]
- Roberts, S.M.; Shipman, J.S. Continuation in shooting methods for two-point boundary value problems. J. Math. Anal. Appl. 1967, 18, 45–58. [Google Scholar] [CrossRef]
- Kotamraju, G.R.; Akella, M.R. Stabilized continuation methods for boundary value problems. Appl. Math. Comput. 2000, 112, 317–332. [Google Scholar] [CrossRef]
- EL-Zahar, E.R.; AL-Gelany, A.M.; Rashad, A.M. Sinusoidal Natural Convective Flow of Non-Newtonian Nanoliquid over a Radiative Vertical Plate in a Saturated Porous Medium. IEEE Access 2020, 8, 136131–136140. [Google Scholar]
- EL-Zahar, E.R.; Rashad, A.M.; Saad, W.; Seddek, L.F. Magneto-Hybrid Nanofluids Flow via Mixed Convection past a Radiative Circular Cylinder. Sci. Rep. 2020, 10, 10494. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Unsteady Mixed Convection Boundary Layer Flow Due To A Stretching Vertical Surface. Arab. J. Sci. Eng. (Springer Sci. Bus. Media BV) 2006, 31, 2B. [Google Scholar]
| Pr | λ | Ishak et al. [33] | FFDCM Results | ||
|---|---|---|---|---|---|
| 0.7 | 0 | −1.0000 | 0.7937 | −1.00000 | 0.79371 |
| 1.0 | −0.5076 | 0.8961 | −0.50763 | 0.89613 | |
| 10 | 2.5777 | 1.1724 | 2.57772 | 1.17244 | |
| 100 | 21.8052 | 1.8075 | 21.80527 | 1.80756 | |
| 1.0 | 0 | −1.0000 | 1.0000 | −1.00000 | 1.00000 |
| 1.0 | −0.5608 | 1.0873 | −0.56081 | 1.08733 | |
| 10 | 2.3041 | 1.3716 | 2.30414 | 1.37164 | |
| 100 | 20.3786 | 2.0667 | 20.37862 | 2.06673 | |
| 3.0 | 0 | −1.0000 | 1.9237 | −1.00000 | 1.92374 |
| 1.0 | −0.7092 | 1.9743 | −0.70924 | 1.97433 | |
| 10 | 1.4567 | 2.2442 | 1.45673 | 2.24424 | |
| 100 | 15.9716 | 3.1042 | 15.97168 | 3.10427 | |
| 7.0 | 0.0 | −1.0000 | 3.0722 | −1.00000 | 3.07221 |
| 1.0 | −0.7962 | 3.1055 | −0.79621 | 3.10553 | |
| 10 | 0.8505 | 3.3318 | 0.85054 | 3.33185 | |
| 100 | 12.7216 | 4.2663 | 12.72167 | 4.26636 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
El-Zahar, E.R.; Rashad, A.M.; Seddek, L.F. Impacts of Viscous Dissipation and Brownian motion on Jeffrey Nanofluid Flow over an Unsteady Stretching Surface with Thermophoresis. Symmetry 2020, 12, 1450. https://doi.org/10.3390/sym12091450
El-Zahar ER, Rashad AM, Seddek LF. Impacts of Viscous Dissipation and Brownian motion on Jeffrey Nanofluid Flow over an Unsteady Stretching Surface with Thermophoresis. Symmetry. 2020; 12(9):1450. https://doi.org/10.3390/sym12091450
Chicago/Turabian StyleEl-Zahar, Essam R., Ahmed M. Rashad, and Laila F. Seddek. 2020. "Impacts of Viscous Dissipation and Brownian motion on Jeffrey Nanofluid Flow over an Unsteady Stretching Surface with Thermophoresis" Symmetry 12, no. 9: 1450. https://doi.org/10.3390/sym12091450
APA StyleEl-Zahar, E. R., Rashad, A. M., & Seddek, L. F. (2020). Impacts of Viscous Dissipation and Brownian motion on Jeffrey Nanofluid Flow over an Unsteady Stretching Surface with Thermophoresis. Symmetry, 12(9), 1450. https://doi.org/10.3390/sym12091450






