RETRACTED: Hybrid Chemical Enhanced Oil Recovery Techniques: A Simulation Study
Abstract
1. Introduction
2. Methodology
2.1. Experimental Investigation
2.2. Interaction Between PPG and Surfactant
2.3. Governing Equations for Polymer and Surfactant Flooding
2.4. Governing Equations for PPG
3. Results and Discussion
3.1. Water Cut
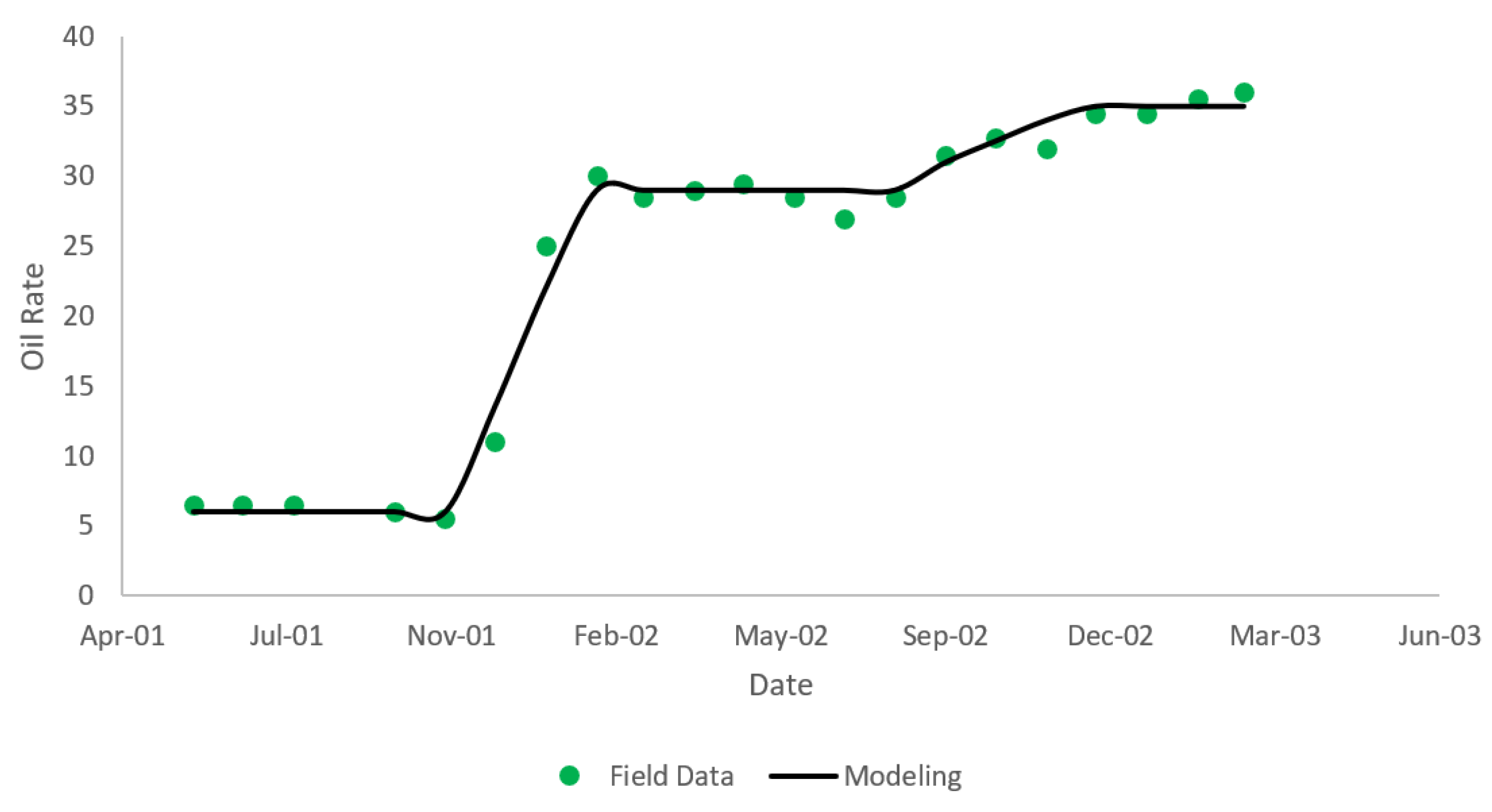
3.2. Oil Rate
3.3. Injected Pressure
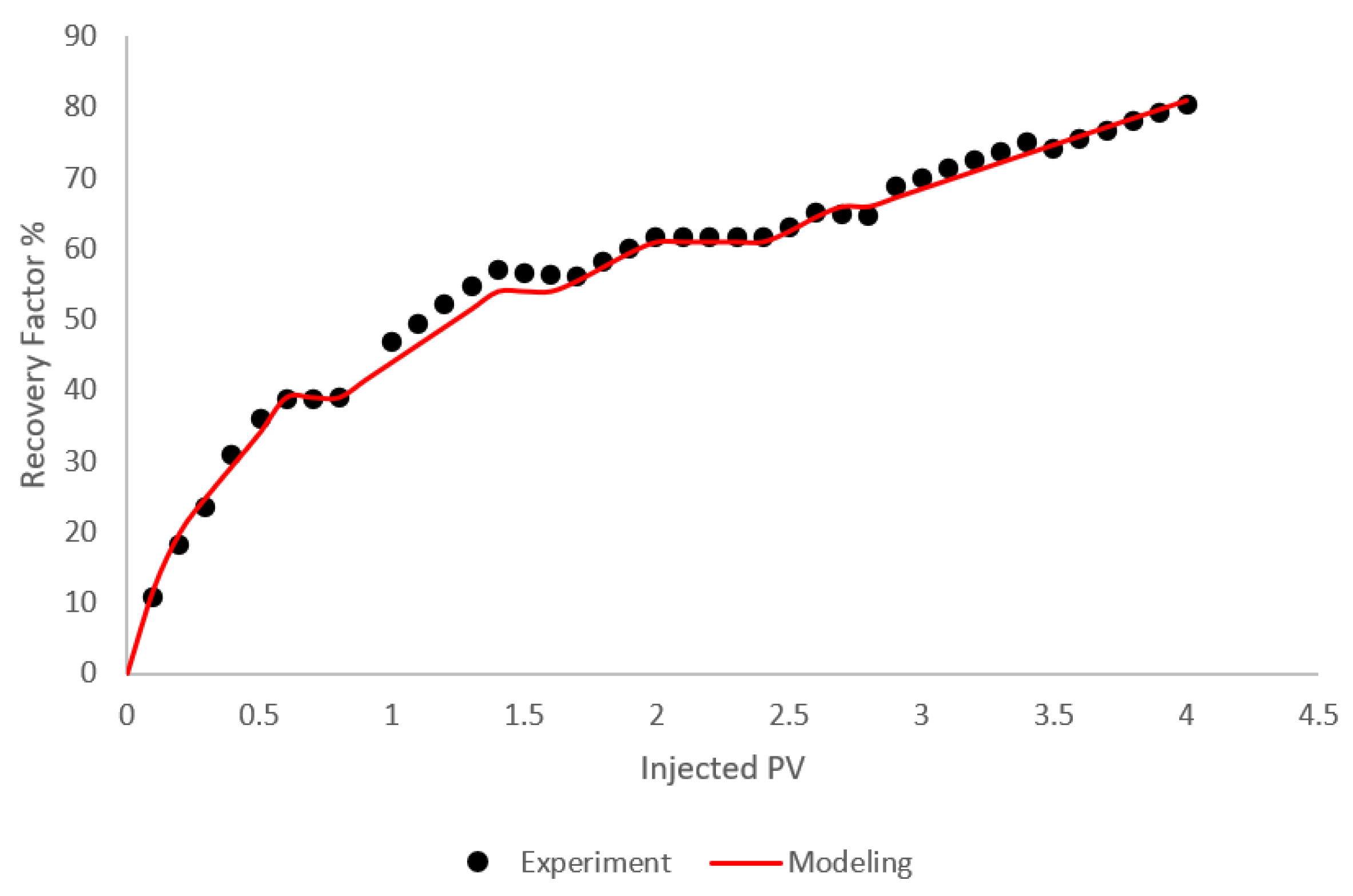
3.4. Recovery Factor
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| Parameter | Description |
| Polymer concentration, ppm | |
| Polymer adsorption concentration, ppm | |
| Inaccessible pore volume, dimensionless | |
| Porosity, fraction | |
| Water saturation, dimensionless | |
| Rock density, | |
| PPG retention in the porous media, fraction | |
| Original porosity, fraction | |
| Filtration coefficient, 1/m | |
| Initial filtration coefficient, 1/m | |
| y | Experimental indices, dimensionless |
| z | Experimental indices, dimensionless |
| x | Experimental indices, dimensionless |
| m | Geometrical constant parameter which is related to the grain sizes of the filler in the clean medium, dimensionless |
| Experimental coefficient; dimensionless | |
| SWR | the swelling ratio in the polymer solution |
| Porosity reduction, dimensionless | |
| Value of specific deposit saturation, dimensionless | |
| Desorption coefficient, dimensionless | |
| Filtration rate, m/min | |
| C | Gelled particles mass concentration which is in the suspension mode, percent |
References
- Davarpanah, A. A feasible visual investigation for associative foam>\polymer injectivity performances in the oil recovery enhancement. Eur. Polym. J. 2018, 105, 405–411. [Google Scholar] [CrossRef]
- Afsharpoor, A.; Lee, G.; Kam, S. Mechanistic simulation of continuous gas injection period during surfactant-alternating-gas (SAG) processes using foam catastrophe theory. Chem. Eng. Sci. 2010, 65, 3615–3631. [Google Scholar] [CrossRef]
- Davarpanah, A.; Mirshekari, B. Numerical simulation and laboratory evaluation of alkali–surfactant–polymer and foam flooding. Int. J. Environ. Sci. Technol. 2020, 17, 1123–1136. [Google Scholar] [CrossRef]
- Davarpanah, A.; Mirshekari, B. A mathematical model to evaluate the polymer flooding performances. Energy Rep. 2019, 5, 1651–1657. [Google Scholar]
- Alhuraishawy, A.K.; Bai, B.; Imqam, A.; Wei, M. Experimental study of combining low salinity water flooding and preformed particle gel to enhance oil recovery for fractured carbonate reservoirs. Fuel 2018, 214, 342–350. [Google Scholar] [CrossRef]
- Hu, X.; Li, M.; Peng, C.; Davarpanah, A. Hybrid Thermal-Chemical Enhanced Oil Recovery Methods; An Experimental Study for Tight Reservoirs. Symmetry 2020, 12, 947. [Google Scholar] [CrossRef]
- Davarpanah, A.; Mirshekari, B. Sensitivity analysis of reservoir and rock properties during low salinity water injection. Energy Rep. 2019, 5, 1001–1009. [Google Scholar] [CrossRef]
- Davarpanah, A.; Mirshekari, B.; Behbahani, T.J.; Hemmati, M. Integrated production logging tools approach for convenient experimental individual layer permeability measurements in a multi-layered fractured reservoir. J. Pet. Explor. Prod. Technol. 2018, 8, 743–751. [Google Scholar] [CrossRef]
- Davarpanah, A. Feasible analysis of reusing flowback produced water in the operational performances of oil reservoirs. Environ. Sci. Pollut. Res. 2018, 25, 35387–35395. [Google Scholar] [CrossRef]
- Bai, B.; Zhang, H. Preformed-particle-gel transport through open fractures and its effect on water flow. SPE J. 2011, 16, 388–400. [Google Scholar] [CrossRef]
- Davarpanah, A.; Mirshekari, B. Experimental Investigation and Mathematical Modeling of Gas Diffusivity by Carbon Dioxide and Methane Kinetic Adsorption; Industrial & Engineering Chemistry Research: New York, NY, USA, 2019; Volume 58, pp. 12392–12400. [Google Scholar]
- Cohen, Y.; Christ, F. Polymer retention and adsorption in the flow of polymer solutions through porous media. SPE Reserv. Eng. 1986, 1, 113–118. [Google Scholar] [CrossRef]
- Lu, W.; Davarpanah, A. An experimental investigation to consider thermal methods efficiency on oil recovery enhancement. Heat Transfer. 2020, 49, 3066–3074. [Google Scholar] [CrossRef]
- Coste, J.P.; Liu, Y.; Bai, B.; Li, Y.; Shen, P.; Wang, Z.; Zhu, G. In-Depth Fluid Diversion by Pre-Gelled Particles. Laboratory Study and Pilot Testing. In SPE/DOE Improved Oil Recovery Symposium; Society of Petroleum Engineers: Tulsa, OK, USA, 2000. [Google Scholar]
- Cui, X.; Li, Z.; Cao, X.; Song, X.; Chen, X.; Zhang, X. A novel PPG enhanced surfactant-polymer system for EOR. In Proceedings of the SPE Enhanced Oil Recovery Conference, Kuala Lumpur, Malaysia, 19–21 July 2011; Society of Petroleum Engineers: Kuala Lumpur, Malaysia, 2011. [Google Scholar]
- Ebadati, A.; Davarpanah, A.; Mirshekari, B. Stimulated-based characterization recovery enhancement feedback of oil-rim reservoirs. Energy Sources Part A Recovery Util. Environ. Eff. 2018, 40, 2528–2541. [Google Scholar] [CrossRef]
- Feng, Q.; Chen, X.; Zhang, G. Experimental and Numerical Study of Gel Particles Movement and Deposition in Porous Media after Polymer Flooding. Transp. Porous Media 2013, 97, 67–85. [Google Scholar] [CrossRef]
- Gao, H.W.; Chang, M.M.; Burchfield, T.E.; Tham, M.K. Permeability modification simulator studies of polymer-gel-treatment initiation time and crossflow effects on waterflood oil recovery. SPE Reserv. Eng. 1993, 8, 221–227. [Google Scholar] [CrossRef]
- Liu, X.; Civan, F. Formation damage and filter cake buildup in laboratory core tests: Modeling and model-assisted analysis. SPE Form. Eval. 1996, 11, 26–30. [Google Scholar] [CrossRef]
- Liu, Y.; Bai, B.; Wang, Y. Applied technologies and prospects of conformance control treatments in China. Oil Gas Sci. Technol.–Rev. d’IFP Energ. Nouv. 2010, 65, 859–878. [Google Scholar] [CrossRef]
- Liu, Y.; Hou, J.; Wang, Q.; Liu, J.; Guo, L.; Yuan, F.; Zhou, K. Flow of preformed particle gel through porous media: A numerical simulation study based on the size exclusion theory. Ind. Eng. Chem. Res. 2017, 56, 2840–2850. [Google Scholar] [CrossRef]
- Luo, H.; Ma, K.; Mateen, K.; Ren, G.; Bourdarot, G.; Morel, D.; Romero, C. Modeling polymer enhanced foam flow in porous media using an improved population-balance foam model. In SPE Improved Oil Recovery Conference; Society of Petroleum Engineers: Tulsa, OK, USA, 2018. [Google Scholar]
- Lyu, Y.; Gu, C.; Fan, X.; Tao, J.; Yao, X.; Dai, C.; Zhao, G. Interfacial rheology of a novel dispersed particle gel soft heterogeneous combination flooding system at the oil-water interface. Colloids Surf. A Physicochem. Eng. Asp. 2018, 559, 23–34. [Google Scholar] [CrossRef]
- Mehrabian, S.; Acosta, E.; Bussmann, M. Oil-particle separation in a falling sphere configuration: Effect of viscosity ratio & interfacial tension. Int. J. Multiph. Flow 2018, 98, 120–127. [Google Scholar]
- Mohammadi, H.; Delshad, M.; Pope, G.A. Mechanistic modeling of alkaline/surfactant/polymer floods. SPE Reserv. Eval. Eng. 2009, 12, 518–527. [Google Scholar] [CrossRef]
- Muhammed, F.A.; Bai, B.; Imqam, A.; Almansour, A.O. Preformed particle gel-enhanced surfactant imbibition for improving oil recovery in fractured carbonate reservoirs. In SPE Heavy Oil Conference-Canada; Society of Petroleum Engineers: Calgary, AB, Canada, 2014. [Google Scholar]
- Salama, D.; Kantzas, A. Monitoring of diffusion of heavy oils with hydrocarbon solvents in the presence of sand. In SPE International Thermal Operations and Heavy Oil Symposium; Society of Petroleum Engineers: Calgary, AB, Canada, 2005. [Google Scholar]
- Sang, Q.; Li, Y.; Yu, L.; Li, Z.; Dong, M. Enhanced oil recovery by branched-preformed particle gel injection in parallel-sandpack models. Fuel 2014, 136, 295–306. [Google Scholar] [CrossRef]
- Shapiro, A.A.; Bedrikovetsky, P.G.; Santos, A.; Medvedev, O.O. A stochastic model for filtration of particulate suspensions with incomplete pore plugging. Transp. Porous Media 2007, 67, 135–164. [Google Scholar] [CrossRef]
- Sharma, A.; Delshad, M.; Huh, C.; Pope, G.A. A practical method to calculate polymer viscosity accurately in numerical reservoir simulators. In SPE Annual Technical Conference and Exhibition; Society of Petroleum Engineers: Denver, CO, USA, 2011. [Google Scholar]
- Sun, H. Application of pilot test for well pattern adjusting heterogeneous combination flooding after polymer flooding case of Zhongyiqu Ng3 block, Gudao oilfield. Pet. Geol Recovery Effic. 2014, 21, 1–4. [Google Scholar]
- Taksaudom, P. Simulation Study of Preformed Particle Gel for Conformance Control. 2014. Available online: https://repositories.lib.utexas.edu/handle/2152/26459 (accessed on 30 June 2020).
- Feng, Q.; Zhang, G.; Yin, X.; Luan, Z. Numerical simulation of the blocking process of gelled particles in porous media with remaining polymers. Pet. Sci. 2009, 6, 284–288. [Google Scholar] [CrossRef]
- Telmadarreie, A.; Trivedi, J.J. New insight on carbonate-heavy-oil recovery: Pore-scale mechanisms of post-solvent carbon dioxide foam/polymer-enhanced-foam flooding. SPE J. 2016, 21, 1655–1668. [Google Scholar] [CrossRef]
- Tran, D.; Nghiem, L.; Buchanan, L. An overview of iterative coupling between geomechanical deformation and reservoir flow. In SPE International Thermal Operations and Heavy Oil Symposium; Society of Petroleum Engineers: Calgary, AB, Canada, 2005. [Google Scholar]
- Wang, J.; Liu, H.Q.; Wang, Z.L.; Hou, P.C. Experimental investigation on the filtering flow law of pre-gelled particle in porous media. Transp. Porous Media 2012, 94, 69–86. [Google Scholar] [CrossRef]
- Wang, J.; Liu, H.; Wang, Z.; Xu, J.; Yuan, D. Numerical simulation of preformed particle gel flooding for enhancing oil recovery. J. Pet. Sci. Eng. 2013, 112, 248–257. [Google Scholar] [CrossRef]
- Wu, Y.-S.; Bai, B. Modeling particle gel propagation in porous media. In SPE Annual Technical Conference and Exhibition; Society of Petroleum Engineers: Denver, CO, USA, 2008. [Google Scholar]
- Xiang’an, Y.; Jirui, H.; Maojun, Q.; Yue, X. Research Center of Enhanced Oil Recovery, China University of Petroleum (Beijing), Beijing, China; Evaluation on character of profile control by polymer gel particle. Pet. Geol. Recovery Effic. 2006, 2, 102249. [Google Scholar]
- Gong, H.; Zhang, H.; Xu, L.; Li, K.; Yu, L.; San, Q.; Li, Y.; Dong, M. The synergistic effect of branched-preformed particle gel and hydrolyzed polyacrylamide on further-enhanced oil recovery after polymer flooding. Energy Fuels 2017, 31, 7904–7910. [Google Scholar] [CrossRef]
- He, H.; Fu, J.; Hou, B.; Yuan, F.; Guo, L.; Li, Z.; You, Q. Investigation of Injection Strategy of Branched-Preformed Particle Gel/Polymer/Surfactant for Enhanced Oil Recovery after Polymer Flooding in Heterogeneous Reservoirs. Energies 2018, 11, 1950. [Google Scholar] [CrossRef]
- Zhang, H.; Bai, B. Preformed particle gel transport through open fractures and its effect on water flow. In SPE Improved Oil Recovery Symposium; Society of Petroleum Engineers: Tulsa, OK, USA, 2010. [Google Scholar]
- Xu, L.; Qiu, Z.; Gong, H.; Zhu, C.; Sang, Q.; Li, Y.; Dong, M. Synergy of microbial polysaccharides and branched-preformed particle gel on thickening and enhanced oil recovery. Chem. Eng. Sci. 2019, 208, 115138. [Google Scholar] [CrossRef]
- Zhu, W.; Li, B.; Liu, Y.; Song, H.; Yue, M.; Wu, N. A New Calculating Method for Relative Permeability of Branched Preformed Particle Gel Flooding in Multiphase Composite System. Spec. Top. Rev. Porous Media Int. J. 2019, 10, 89–98. [Google Scholar] [CrossRef]
- Zhang, Y.; Yue, X.; Dong, J.; Yu, L. New and effective foam flooding to recover oil in heterogeneous reservoir. In SPE/DOE Improved Oil Recovery Symposium; Society of Petroleum Engineers: Tulsa, OK, USA, 2000. [Google Scholar]


| Layers | Sand Thickness (m) | Water Saturation (%) | |
|---|---|---|---|
| 1 | 2.72 | 47.31 | 541.47 |
| 2 | 1.13 | 45.37 | 546.28 |
| 3 | 1.30 | 48.14 | 581.67 |
| 4 | 0.72 | 48.55 | 602.54 |
| 5 | 1.12 | 49.63 | 658.32 |
| 6 | 1.90 | 49.89 | 664.22 |
| Layer | Porosity | Oil Saturation (%) | ||
|---|---|---|---|---|
| 1 | 200 | 20 | 0.15 | 0.8 |
| 2 | 400 | 40 | 0.16 | 0.8 |
| 3 | 600 | 60 | 0.17 | 0.8 |
| 4 | 800 | 80 | 0.18 | 0.8 |
| 5 | 1000 | 100 | 0.19 | 0.8 |
| 6 | 1200 | 120 | 0.20 | 0.8 |
| 7 | 1400 | 140 | 0.21 | 0.8 |
| 8 | 1600 | 160 | 0.22 | 0.8 |
| 9 | 1800 | 180 | 0.23 | 0.8 |
| 10 | 2000 | 200 | 0.24 | 0.8 |
| Depth (m) | 1173–1230 |
| Net Thickness (m) | 14.2 |
| Porosity (%) | 33 |
| 1.5–2.5 | |
| Oil Viscosity (mPa.s) | 16.3 |
| Temperature (°C) | 65.9 |
| Salinity (mg/L) | 5923 |
| Stage | Procedure |
|---|---|
| 1 | Water injected into the core sample to reach the water cut up to 98%. |
| 2 | 0.7 PV of polymer with the concentration of 1000 mg/L is injected. |
| 3 | 0.3 PV of water is injected again. |
| 4 | 0.3 PV of PPG with the concentration of 2000 mg/L is injected. |
| 5 | 0.2 PV of cross-linking agent with a concentration of 100 mg/L is injected. |
| 6 | 0.3 PV of surfactant is injected. |
| 7 | Water injected into the core sample to reach the water cut up to 98%. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, H.; Davarpanah, A. RETRACTED: Hybrid Chemical Enhanced Oil Recovery Techniques: A Simulation Study. Symmetry 2020, 12, 1086. https://doi.org/10.3390/sym12071086
Zhou H, Davarpanah A. RETRACTED: Hybrid Chemical Enhanced Oil Recovery Techniques: A Simulation Study. Symmetry. 2020; 12(7):1086. https://doi.org/10.3390/sym12071086
Chicago/Turabian StyleZhou, Haiyan, and Afshin Davarpanah. 2020. "RETRACTED: Hybrid Chemical Enhanced Oil Recovery Techniques: A Simulation Study" Symmetry 12, no. 7: 1086. https://doi.org/10.3390/sym12071086
APA StyleZhou, H., & Davarpanah, A. (2020). RETRACTED: Hybrid Chemical Enhanced Oil Recovery Techniques: A Simulation Study. Symmetry, 12(7), 1086. https://doi.org/10.3390/sym12071086






