Hydromagnetic Flow of Micropolar Nanofluid
Abstract
1. Introduction
2. Problem Formulation
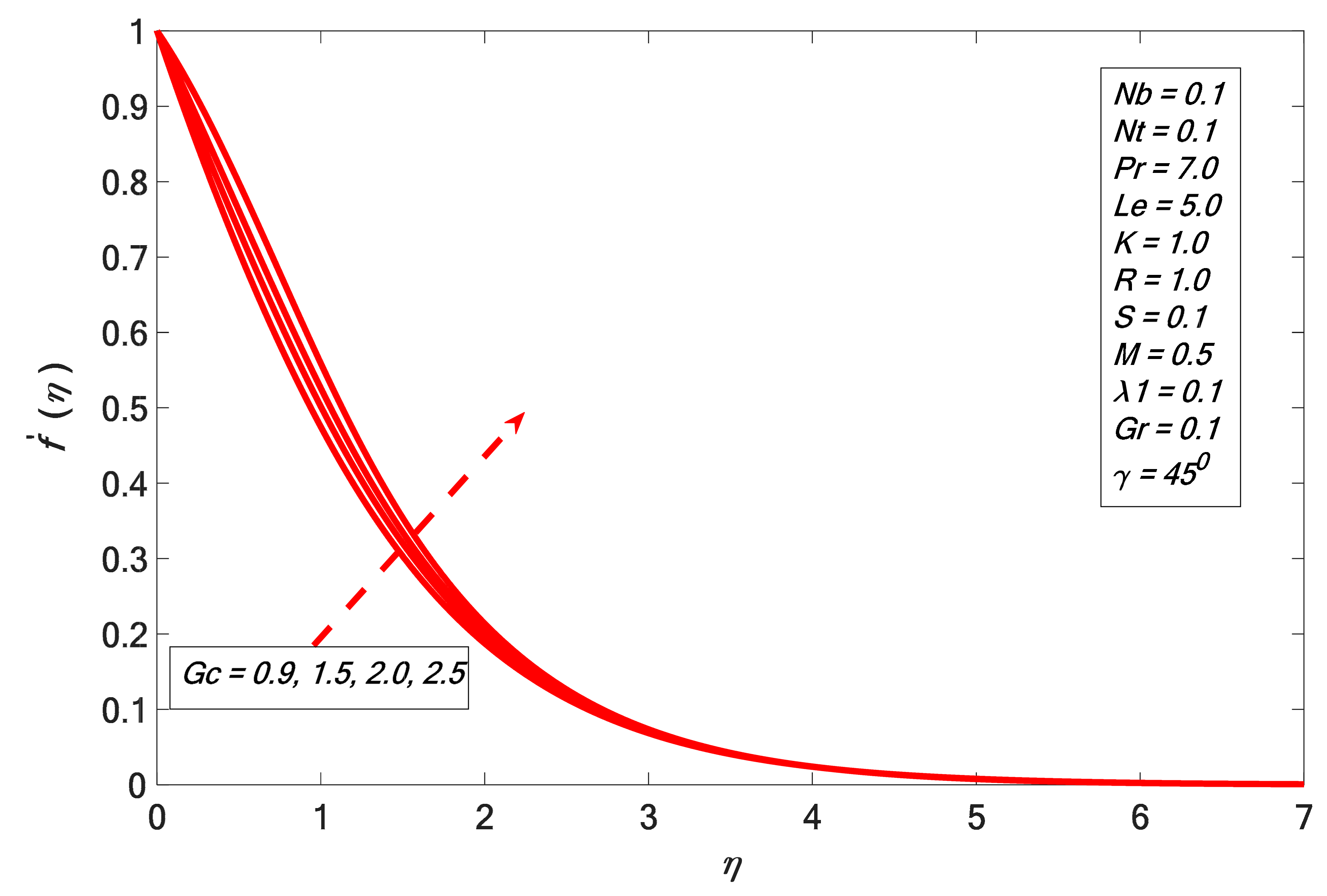
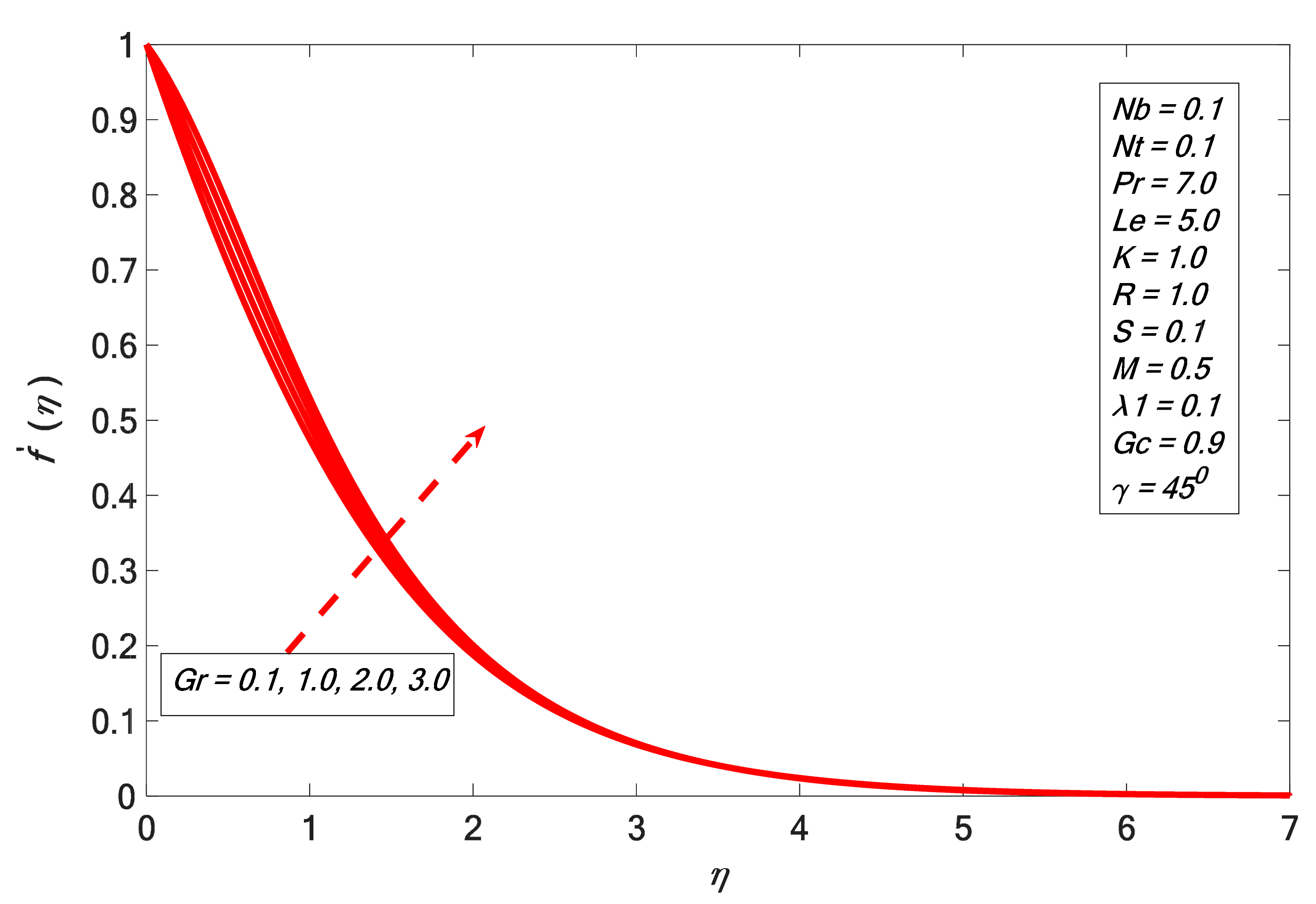
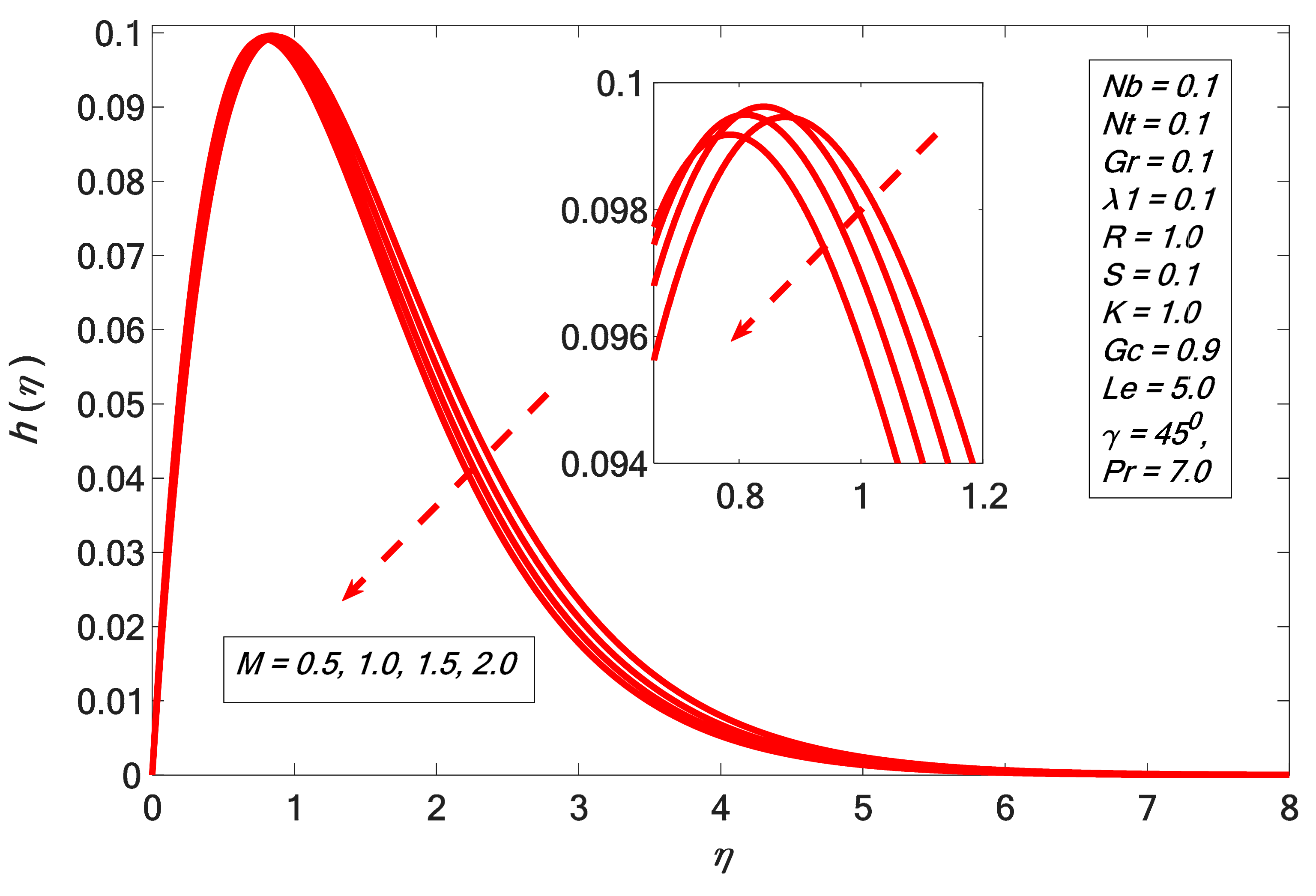
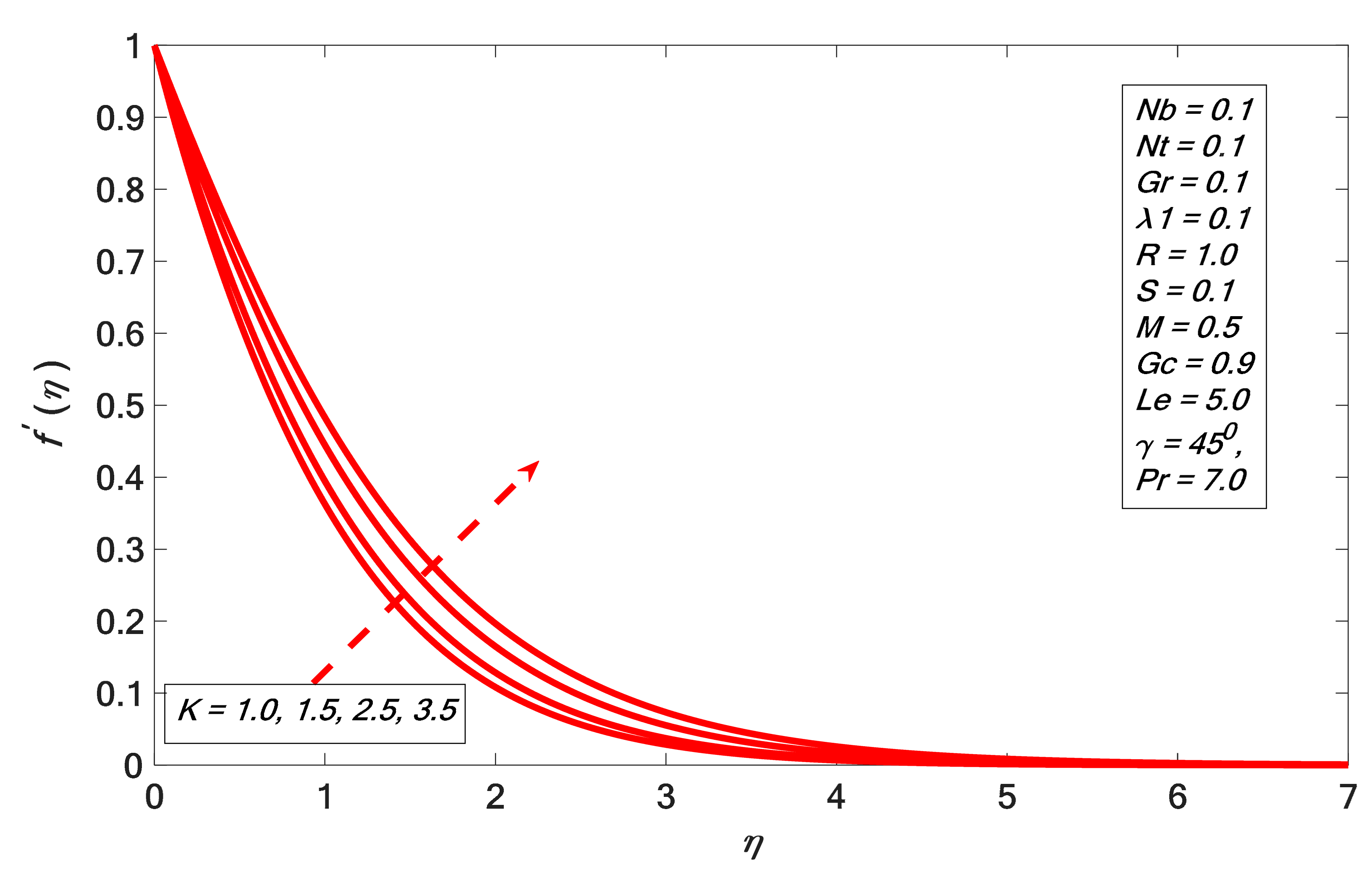
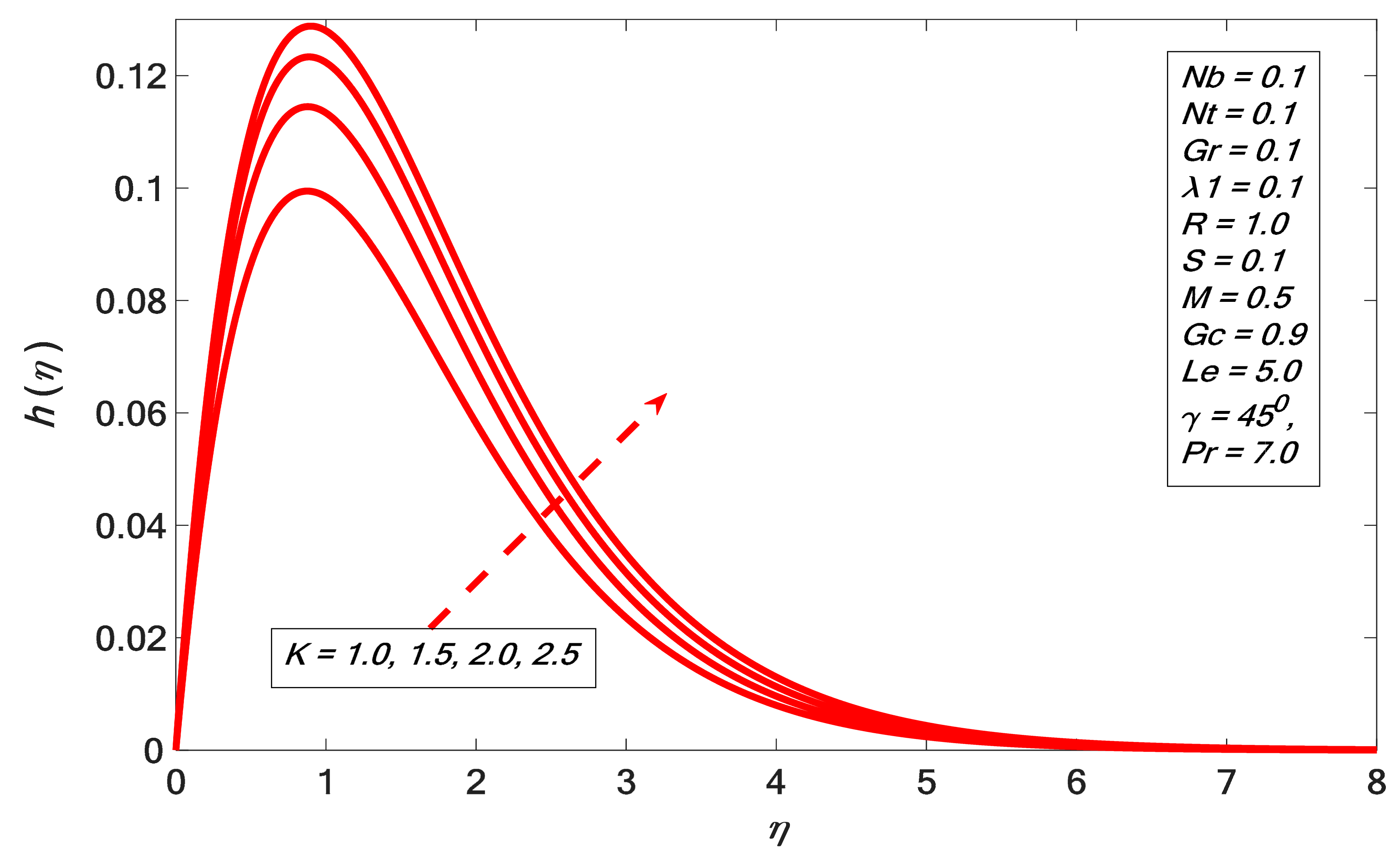
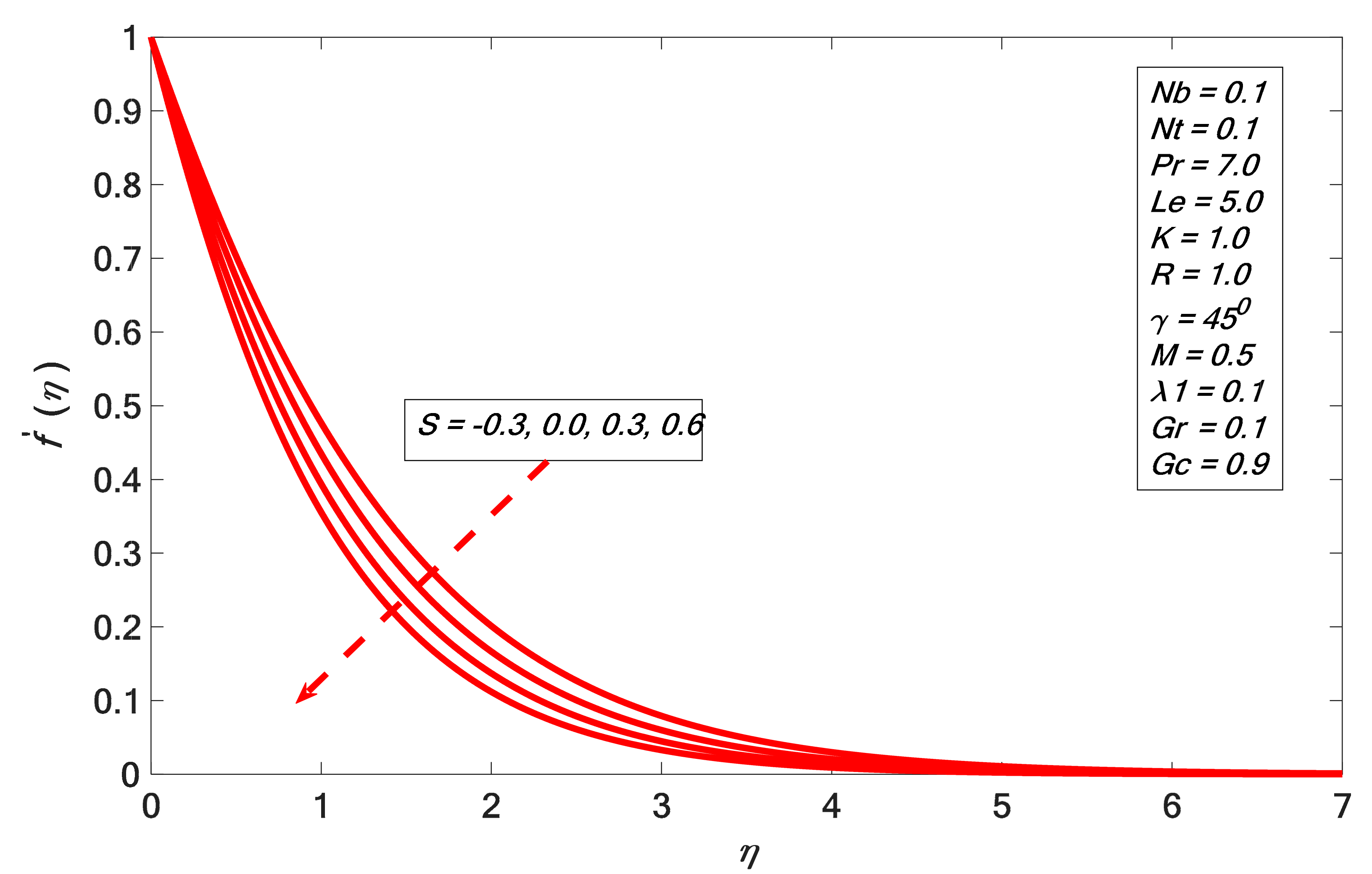
3. Results and Discussion
4. Conclusions
- ⮚
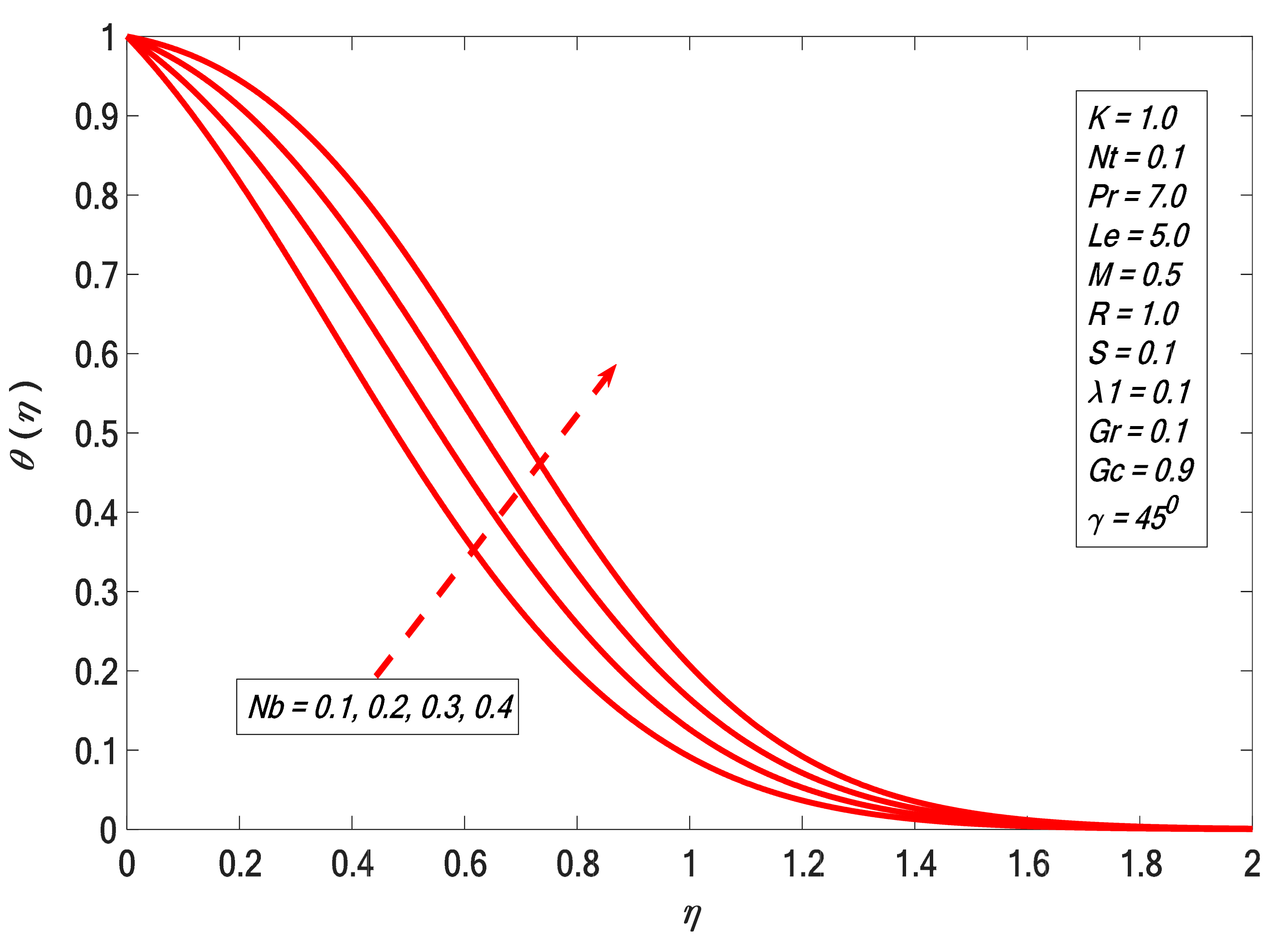
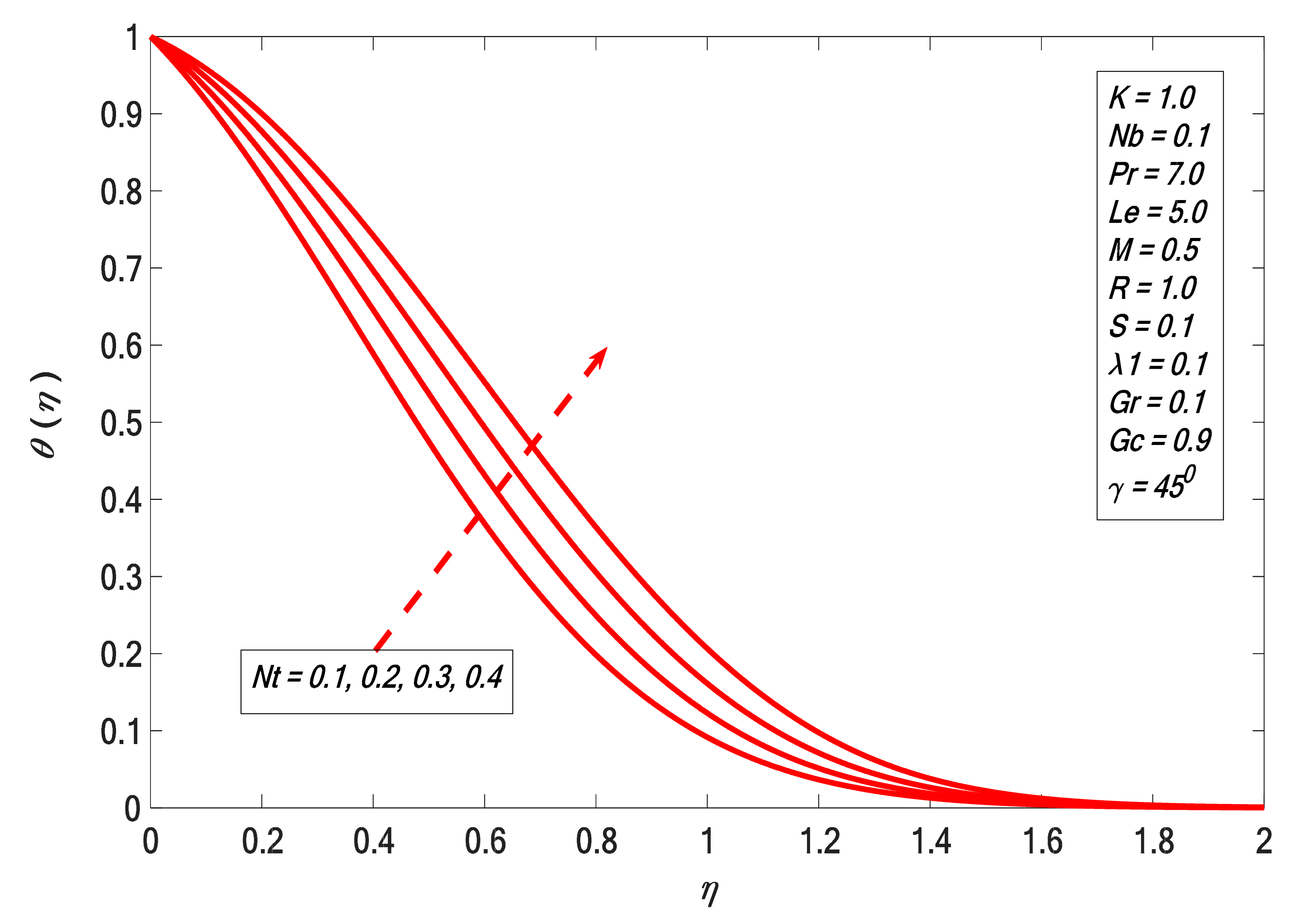
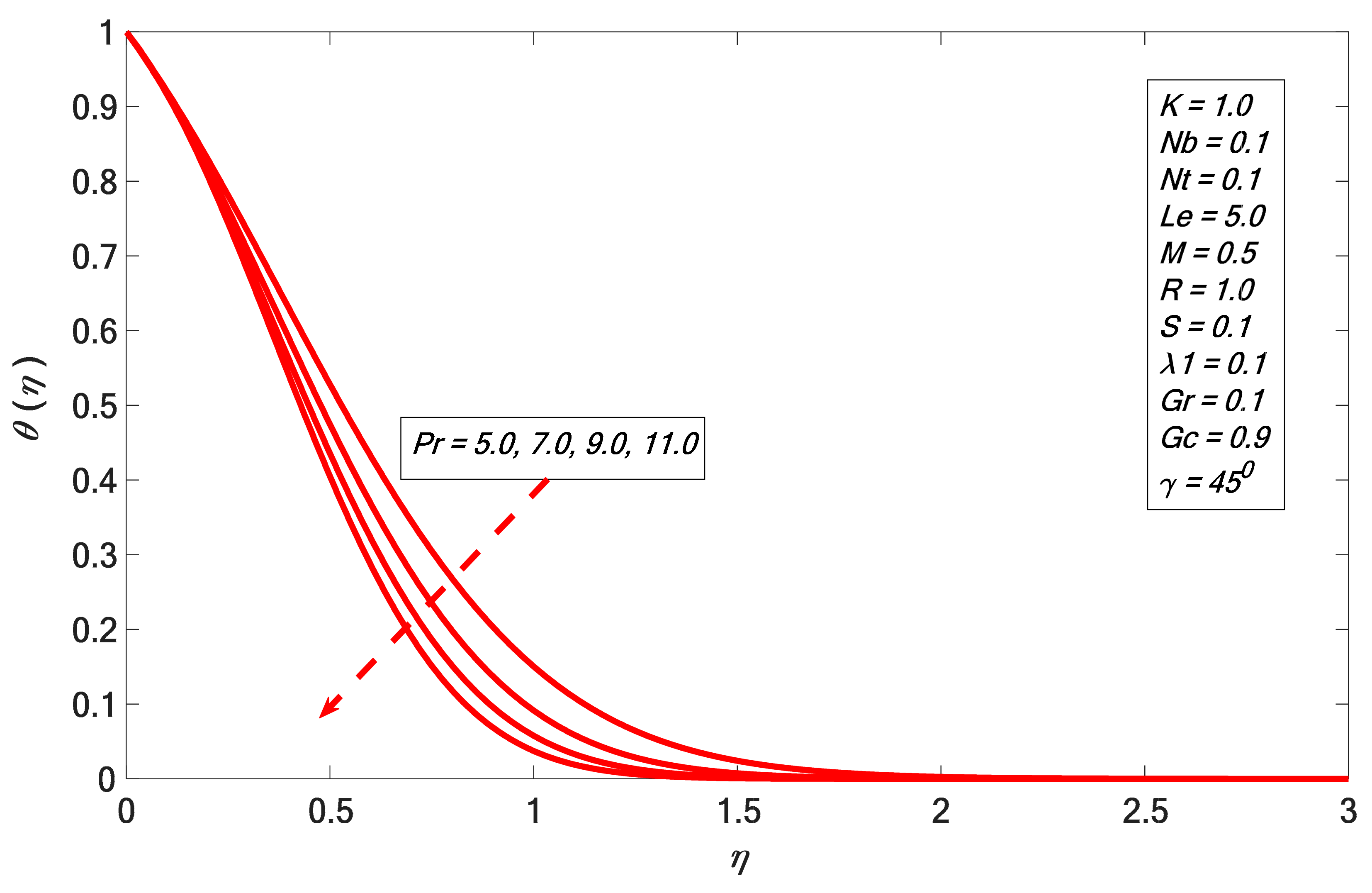
- The temperature profile falls by improving .
- ⮚
- The Nusselt number enhances with the increase of the chemical reaction effect.
- ⮚
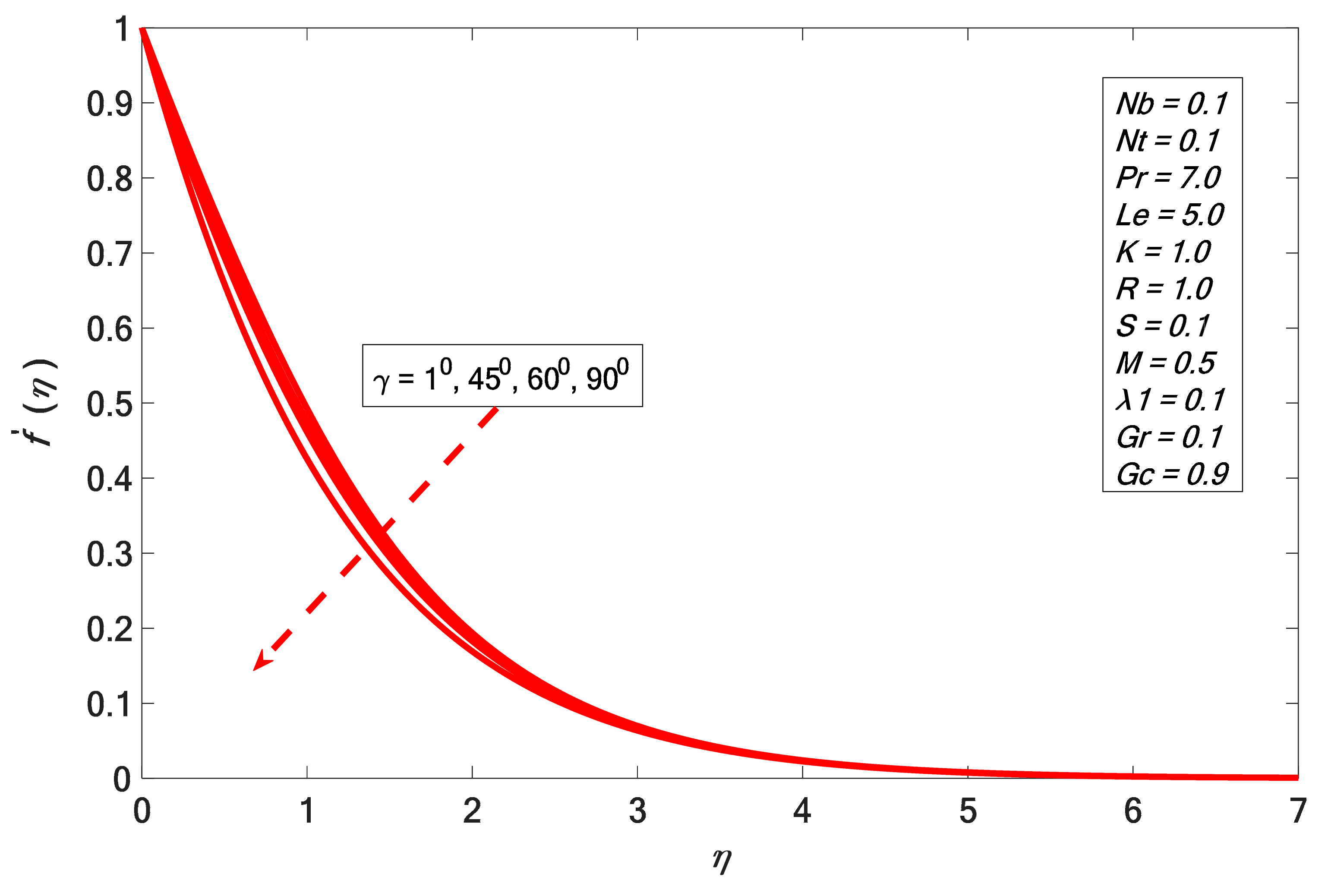
- The Nusselt number improves the higher the magnitude of inclination.
- ⮚
- The Sherwood number upturns by improving the inclination parameter.
- ⮚
- The Sherwood number decreases by improving the chemical reaction impact.
- ⮚
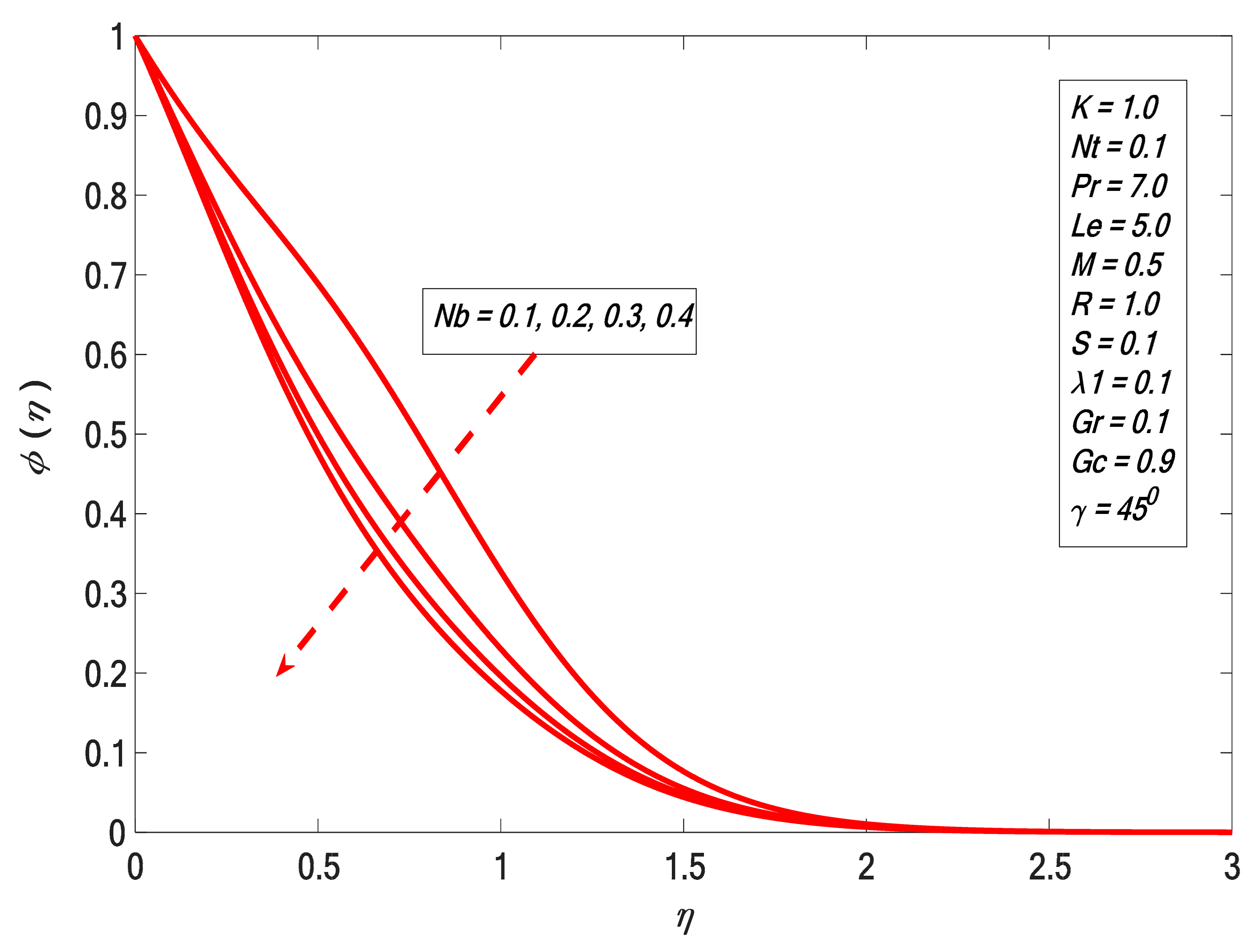
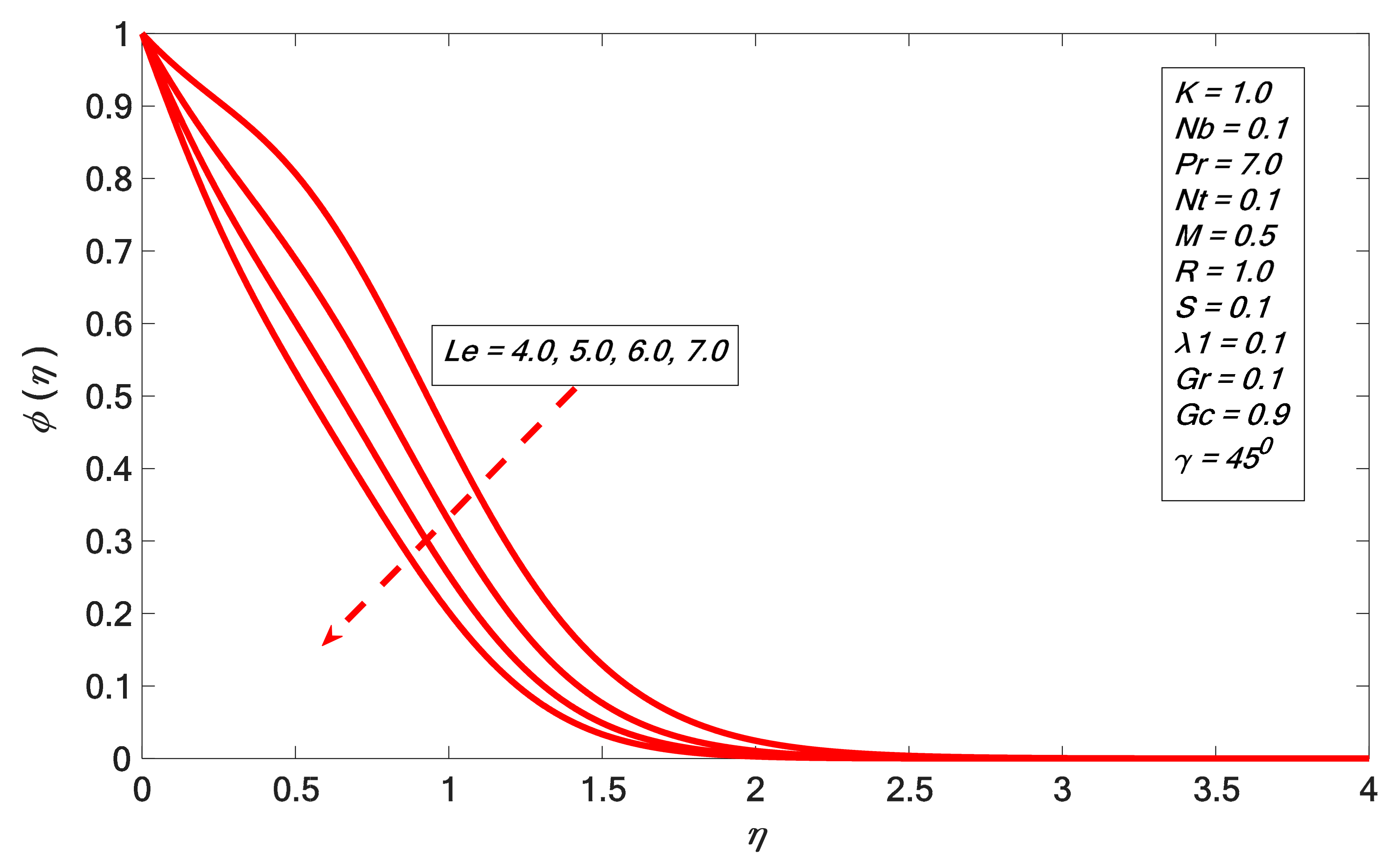
- The Sherwood number reduces the larger the value of .
- ⮚
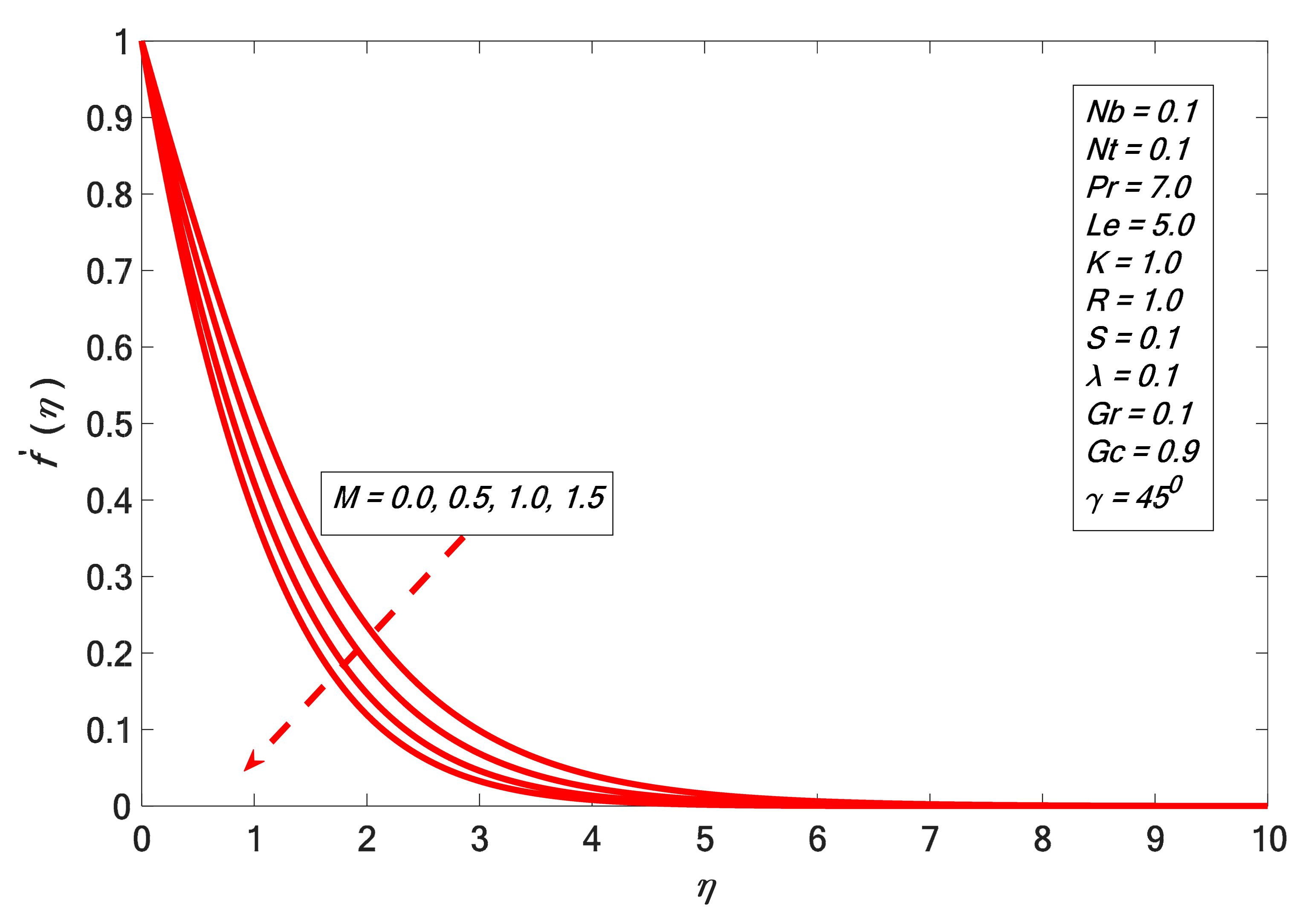
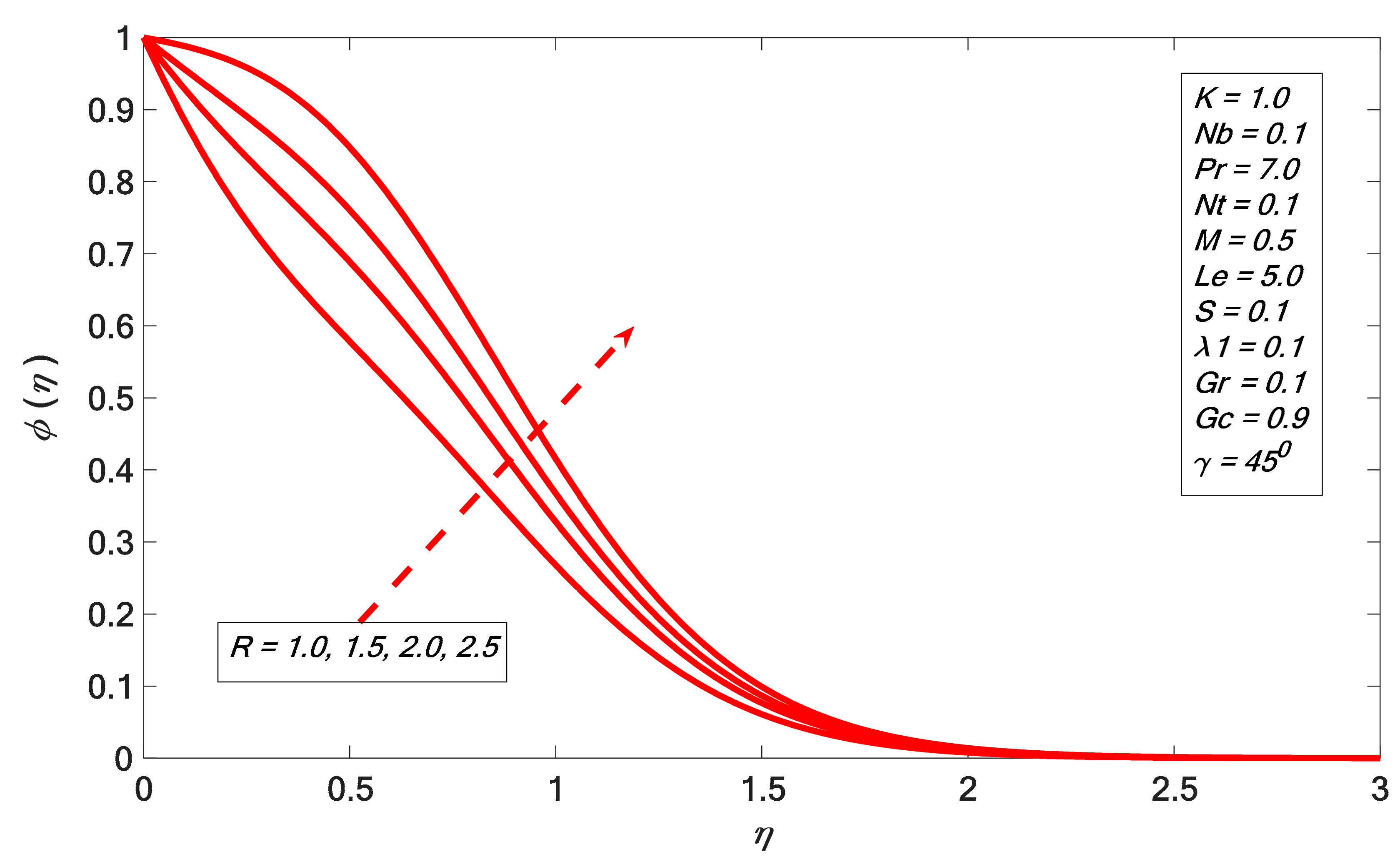
- The skin friction decreases for higher values of .
- ⮚
- Skin friction decreases with the enhancement of the chemical reaction parameter.
Author Contributions
Acknowledgments
Conflicts of Interest
Nomenclature
| Fluid concentration | Heat generation or absorption | Chemical reaction | |||
| Skin friction coefficient | Thermal diffusivity | Reynolds number | |||
| Ambient nanoparticle volume fraction | Lewis number | Sherwood number | |||
| Surface volume fraction | Brownian motion parameter | Fluid temperature | |||
| Specific heat at constant pressure | Thermophoretic parameter | Wall temperature | |||
| Brownian diffusion coefficient | Nusselt number | Ambient temperature | |||
| Thermophoretic diffusion coefficient | Prandtle number | wall velocity | |||
| Similarity function for velocity | Gravitational acceleration | ambient velocity | |||
| Volume heat capacity | Kinematic viscosity | Dynamic viscosity | |||
| Dimensionless solid volume fraction | Condition at the wall | Ambient condition | |||
| Local Grashof number | Thermal expansion coefficient | Concentration expansion coefficient | |||
| Electric conductivity | Spin gradient viscosity | Vertex viscosity | |||
| Micro inertia per unit mass | Inclination parameter | Differentiation with respect to | |||
| Velocity in direction | Velocity in direction | Cartesian coordinate | |||
| Dimensionless temperature | Suction or injection parameter | Thermal conductivity | |||
| Fluid density | Bouncy parameter | Uniform magnetic field strength | |||
| Material parameter | Similarity independent variable | ||||
| Non-dimensional angular velocity | Stretching rate |
References
- Choi, S.U.S.; Singer, D.A.; Wang, H.P. Developments and applications of non-Newtonian flows. ASME FED 1995, 66, 99–105. [Google Scholar]
- Eastman, J.A.; Choi, U.S.; Li, S.; Thompson, L.J.; Lee, S. Enhanced thermal conductivity through the development of nanofluids. MRS Online Proc. Libr. Arch. 1996, 457. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective transport in nanofluids. J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Sivashanmugam, P. Application of nanofluids in heat transfer. In An Overview of Heat Transfer Phenomena; InTech: Rijeka, Croatia, 2012; Volume 16. [Google Scholar]
- Zaimi, K.; Ishak, A.; Pop, I. Boundary layer flow and heat transfer over a nonlinearly permeable stretching/shrinking sheet in a nanofluid. Sci. Rep. 2014, 4, 4404. [Google Scholar] [CrossRef]
- Sandeep, N.; Kumar, M.S. Heat and Mass Transfer in Nanofluid Flow over an Inclined Stretching Sheet with Volume Fraction of Dust and Nanoparticles. J. Appl. Fluid Mech. 2014, 9, 2205–2215. [Google Scholar] [CrossRef]
- Chakraborty, T.; Das, K.; Kundu, P.K. Ag-water nanofluid flow over an inclined porous plate embedded in a non-Darcy porous medium due to solar radiation. J. Mech. Sci. Technol. 2017, 31, 2443–2449. [Google Scholar] [CrossRef]
- Rashad, A. Unsteady nanofluid flow over an inclined stretching surface with convective boundary condition and anisotropic slip impact. Int. J. Heat Technol. 2017, 35, 82–90. [Google Scholar] [CrossRef]
- Mitra, A. Computational Modelling of Boundary-Layer Flow of a Nano fluid Over a Convective Heated Inclined Plate. J. Mech. Contin. Math. Sci. 2018, 13, 88–94. [Google Scholar] [CrossRef]
- Shit, G.C.; Majee, S. Hydromagnetic Flow over an Inclined Non-Linear Stretching Sheet with Variable Viscosity in the Presence of Thermal Radiation and Chemical Reaction. J. Appl. Fluid Mech. 2014, 7, 239–247. [Google Scholar]
- Eid, M.R. Chemical reaction effect on MHD boundary-layer flow of two-phase nano fluid model over an exponentially stretching sheet with a heat generation. J. Mol. Liq. 2016, 220, 718–725. [Google Scholar] [CrossRef]
- Jain, S.; Bohra, S. Heat and mass transfer over a three-dimensional inclined non-linear stretching sheet with convective boundary conditions. Indian J. Pure Appl. Phys. 2017, 55, 847–856. [Google Scholar]
- Sheri, S.R.; Modugula, P. Thermal-diffusion and diffusion-thermo effects on MHD flow through porous medium past an exponentially accelerated inclined plate with variable temperature. ARPN J. Eng. Appl. Sci. 2017, 12, 5518–5526. [Google Scholar] [CrossRef]
- Rafique, K.; Anwar, M.I.; Misiran, M. Keller-box Study on Casson Nano Fluid Flow over a Slanted Permeable Surface with Chemical Reaction. Asian Res. J. Math. 2019, 14, 1–17. [Google Scholar] [CrossRef][Green Version]
- Bhatti, M.M.; Mishra, S.R.; Abbas, T.; Rashidi, M.M. A mathematical model of MHD nanofluid flow having gyrotactic microorganisms with thermal radiation and chemical reaction effects. Neural Comput. Appl. 2018, 30, 1237–1249. [Google Scholar] [CrossRef]
- Abbas, T.; Bhatti, M.M.; Ayub, M. Aiding and opposing of mixed convection Casson nanofluid flow with chemical reactions through a porous Riga plate. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2018, 232, 519–527. [Google Scholar] [CrossRef]
- Qing, J.; Bhatti, M.; Abbas, M.; Rashidi, M.; Ali, M. Entropy generation on MHD Casson nanofluid flow over a porous stretching/shrinking surface. Entropy 2016, 18, 123. [Google Scholar] [CrossRef]
- Mishra, S.R.; Baag, S.; Bhatti, M.M. Study of heat and mass transfer on MHD Walters B′ nanofluid flow induced by a stretching porous surface. Alex. Eng. J. 2018, 57, 2435–2443. [Google Scholar] [CrossRef]
- Eringen, A.C. Simple microfluids. Int. J. Eng. Sci. 1964, 2, 205–217. [Google Scholar] [CrossRef]
- Rahman, M.M.; Aziz, A.; Al-Lawatia, M.A. International Journal of Thermal Sciences Heat transfer in micropolar fluid along an inclined permeable plate with variable fluid properties. Int. J. Therm. Sci. 2010, 49, 993–1002. [Google Scholar] [CrossRef]
- Das, K. Slip effects on heat and mass transfer in MHD micropolar fluid flow over an inclined plate with thermal radiation and chemical reaction. Int. J. Numer. Methods Fluids 2012, 70, 96–113. [Google Scholar] [CrossRef]
- Kasim AR, M.; Mohammad, N.F.; Shafie, S. Unsteady MHD mixed convection flow of a micropolar fluid along an inclined stretching plate. Heat Transf. Asian Res. 2013, 42, 89–99. [Google Scholar]
- Srinivasacharya, D.; Bindu, K.H. Entropy generation in a micropolar fluid flow through an inclined channel. Alex. Eng. J. 2016, 55, 973–982. [Google Scholar] [CrossRef]
- Anwar, M.I.; Shafie, S.; Hayat, T.; Shehzad, S.A.; Salleh, M.Z. Numerical study for MHD stagnation-point flow of a micropolar nanofluid towards a stretching sheet. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 89–100. [Google Scholar] [CrossRef]
- Hazbavi, A.; Sharhani, S. IMECE2017-72528 Micropolar Fluid Flow between Two Inclined Parallel Plates. In Proceedings of the ASME 2017 International Mechanical Engineering Congress and Exposition, Tampa, FL, USA, 3–9 November 2017; pp. 1–6. [Google Scholar]
- Srinivasacharya, D.; RamReddy, C.; Naveen, P. Double dispersion effect on nonlinear convective flow over an inclined plate in a micropolar fluid saturated non-Darcy porous medium. Eng. Sci. Technol. Int. J. 2018, 21, 984–995. [Google Scholar] [CrossRef]
- Mishra, S.R.; Baag, S.; Mohapatra, D.K. Engineering Science and Technology, an International Journal Chemical reaction and Soret effects on hydromagnetic micropolar fluid along a stretching sheet. Eng. Sci. Technol. Int. J. 2016, 19, 1919–1928. [Google Scholar] [CrossRef]
- Rafique, K.; Anwar, M.I.; Misiran, M.; Khan, I.; Sherif, E.S.M. The Implicit Keller Box Scheme for Combined Heat and Mass Transfer of Brinkman-Type Micropolar Nanofluid with Brownian Motion and Thermophoretic Effect Over an Inclined Surface. Appl. Sci. 2020, 10, 280. [Google Scholar] [CrossRef]
- Prakash, J.; Tripathi, D.; Tiwari, A.K.; Sait, S.M.; Ellahi, R. Peristaltic Pumping of Nanofluids through a Tapered Channel in a Porous Environment: Applications in Blood Flow. Symmetry 2019, 11, 868. [Google Scholar] [CrossRef]
- Rafique, K.; Anwar, M.I.; Misiran, M.; Khan, I.; Seikh, A.H.; Sherif, E.S.M.; Nisar, K.S. Brownian Motion and Thermophoretic Diffusion Effects on Micropolar Type Nanofluid Flow with Soret and Dufour Impacts over an Inclined Sheet: Keller-Box Simulations. Energies 2019, 12, 4191. [Google Scholar] [CrossRef]
- Rafique, K.; Anwar, M.I.; Misiran, M. Numerical Study on Micropolar Nanofluid Flow over an Inclined Surface by Means of Keller-Box. Asian J. Probab. Stat. 2019, 4, 1–21. [Google Scholar] [CrossRef]
- Ellahi, R.; Sait, S.M.; Shehzad, N.; Mobin, N. Numerical simulation and mathematical modeling of electro-osmotic Couette–Poiseuille flow of MHD power-law nanofluid with entropy generation. Symmetry 2019, 11, 1038. [Google Scholar] [CrossRef]
- Khan, U.; Abbasi, A.; Ahmed, N.; Alharbi, S.O.; Noor, S.; Khan, I.; Mohyud-Din, S.T.; Khan, W.A. Modified MHD Radiative Mixed Convective Nanofluid Flow Model with Consideration of the Impact of Freezing Temperature and Molecular Diameter. Symmetry 2019, 11, 833. [Google Scholar] [CrossRef]
- Makinde, O.D.; Olanrewaju, P.O. Buoyancy effects on thermal boundary layer over a vertical plate with a convective surface boundary condition. J. Fluids Eng. 2010, 132, 044502. [Google Scholar] [CrossRef]
- Ilias, M.R.; Rawi, N.A.; Shafie, S. MHD Free Convection Flow and Heat Transfer of Ferrofluids over a Vertical Flat Plate with Aligned and Transverse Magnetic Field. Indian J. Sci. Technol. 2016, 9, 7. [Google Scholar] [CrossRef][Green Version]
- Olanrewaju, P.O.; Hayat, T. The effects of buoyancy and transpiration on the flow and heat transfer over a moving permeable surface in a parallel stream in the presence of radiation. Int. J. Comput. Methods Eng. Sci. Mech. 2014, 15, 330–336. [Google Scholar] [CrossRef]
- Rafique, K.; Anwar, M.I.; Misiran, M.; Khan, I.; Seikh, A.H.; Sherif, E.S.M.; Sooppy Nisar, K. Keller-Box Simulation for the Buongiorno Mathematical Model of Micropolar Nanofluid Flow over a Nonlinear Inclined Surface. Processes 2019, 7, 926. [Google Scholar] [CrossRef]
- Khan, W.A.; Pop, I. Boundary-layer flow of a nanofluid past a stretching sheet. Int. J. Heat Mass Transf. 2010, 53, 2477–2483. [Google Scholar] [CrossRef]


| Khan and Pop [38] | Present Results | ||||
|---|---|---|---|---|---|
| 0.1 | 0.1 | 0.9524 | 2.1294 | 0.9524 | 2.1294 |
| 0.2 | 0.2 | 0.3654 | 2.5152 | 0.3654 | 2.5152 |
| 0.3 | 0.3 | 0.1355 | 2.6088 | 0.1355 | 2.6088 |
| 0.4 | 0.4 | 0.0495 | 2.6038 | 0.0495 | 2.6038 |
| 0.5 | 0.5 | 0.0179 | 2.5731 | 0.0179 | 2.5731 |
| Nb | Nt | Pr | Le | M | K | R | λ1 | S | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 0.9 | 0.1 | 45° | 1.1123 | 2.9146 | 1.5227 |
| 0.5 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 0.9 | 0.1 | 45° | 0.0978 | 3.1368 | 1.5550 |
| 0.1 | 0.5 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 0.9 | 0.1 | 45° | 0.3667 | 4.5619 | 1.4355 |
| 0.1 | 0.1 | 10.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 0.9 | 0.1 | 45° | 1.1212 | 2.9771 | 1.5206 |
| 0.1 | 0.1 | 7.0 | 10.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 0.9 | 0.1 | 45° | 0.9984 | 4.5817 | 1.5818 |
| 0.1 | 0.1 | 7.0 | 5.0 | 1.5 | 1.0 | 1.0 | 0.1 | 0.1 | 0.9 | 0.1 | 45° | 1.0760 | 2.9014 | 2.0284 |
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 3.0 | 1.0 | 0.1 | 0.1 | 0.9 | 0.1 | 45° | 1.1457 | 2.9288 | 2.0779 |
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 2.0 | 0.1 | 0.1 | 0.9 | 0.1 | 45° | 1.0466 | 3.8926 | 1.5556 |
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.5 | 0.1 | 0.9 | 0.1 | 45° | 0.3832 | 3.4477 | 1.5301 |
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 1.0 | 0.9 | 0.1 | 45° | 1.1218 | 2.9175 | 1.3303 |
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 2.0 | 0.1 | 45° | 1.1237 | 2.9185 | 1.2989 |
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 0.9 | 0.5 | 45° | 2.4776 | 3.0066 | 1.7661 |
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 1.0 | 0.0 | 45° | 0.8317 | 2.8251 | 1.4666 |
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 1.0 | −0.5 | 45° | −0.0228 | 1.8648 | 1.2083 |
| 0.1 | 0.1 | 7.0 | 5.0 | 0.5 | 1.0 | 1.0 | 0.1 | 0.1 | 1.0 | 0.1 | 60° | 1.1092 | 2.9136 | 1.5830 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rafique, K.; Anwar, M.I.; Misiran, M.; Khan, I.; Baleanu, D.; Nisar, K.S.; Sherif, E.-S.M.; Seikh, A.H. Hydromagnetic Flow of Micropolar Nanofluid. Symmetry 2020, 12, 251. https://doi.org/10.3390/sym12020251
Rafique K, Anwar MI, Misiran M, Khan I, Baleanu D, Nisar KS, Sherif E-SM, Seikh AH. Hydromagnetic Flow of Micropolar Nanofluid. Symmetry. 2020; 12(2):251. https://doi.org/10.3390/sym12020251
Chicago/Turabian StyleRafique, Khuram, Muhammad Imran Anwar, Masnita Misiran, Ilyas Khan, Dumitru Baleanu, Kottakkaran Sooppy Nisar, El-Sayed M. Sherif, and Asiful H. Seikh. 2020. "Hydromagnetic Flow of Micropolar Nanofluid" Symmetry 12, no. 2: 251. https://doi.org/10.3390/sym12020251
APA StyleRafique, K., Anwar, M. I., Misiran, M., Khan, I., Baleanu, D., Nisar, K. S., Sherif, E.-S. M., & Seikh, A. H. (2020). Hydromagnetic Flow of Micropolar Nanofluid. Symmetry, 12(2), 251. https://doi.org/10.3390/sym12020251








