Utilizing Macro Fiber Composite to Control Rotating Blade Vibrations
Abstract
:1. Introduction
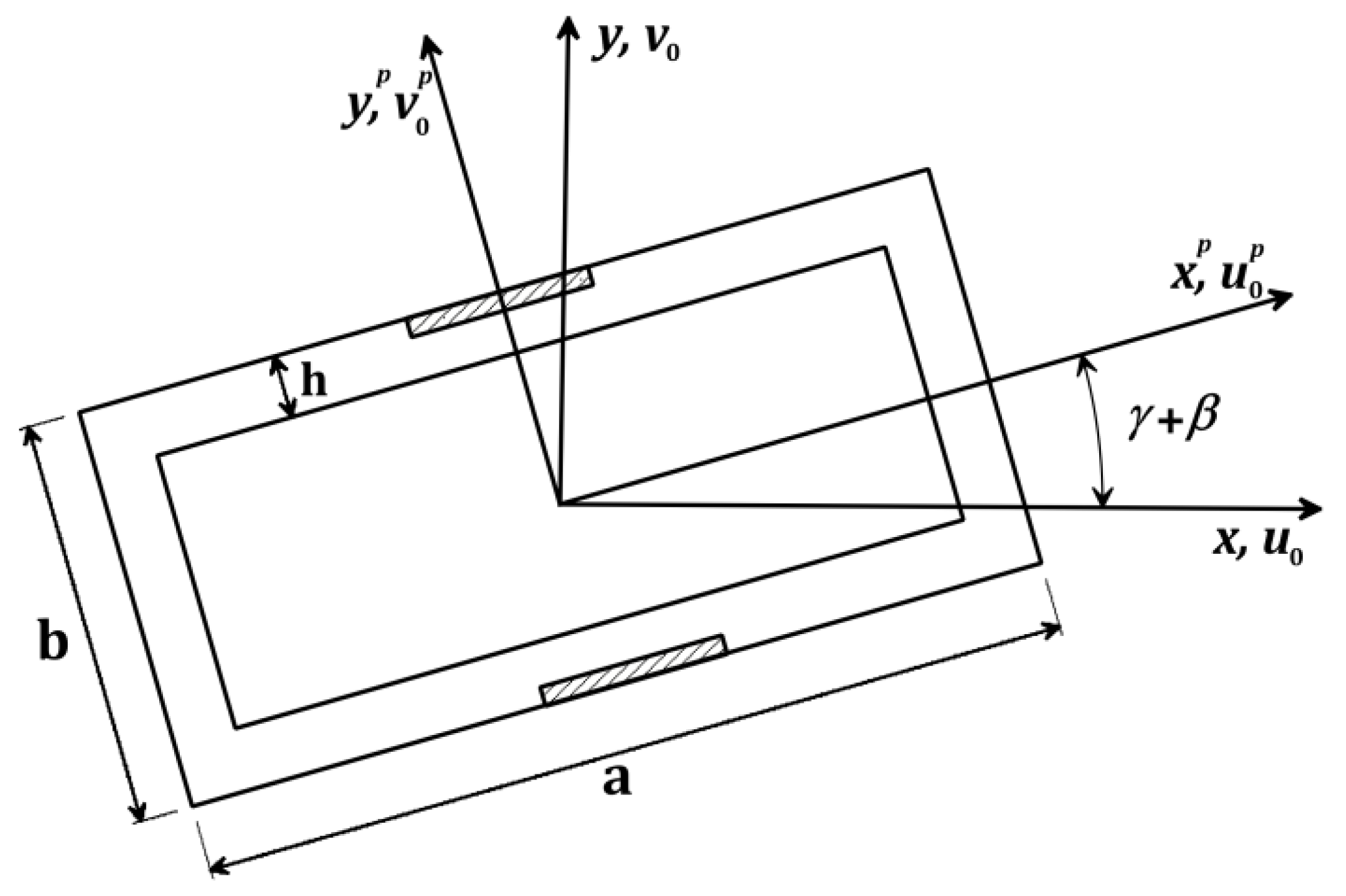
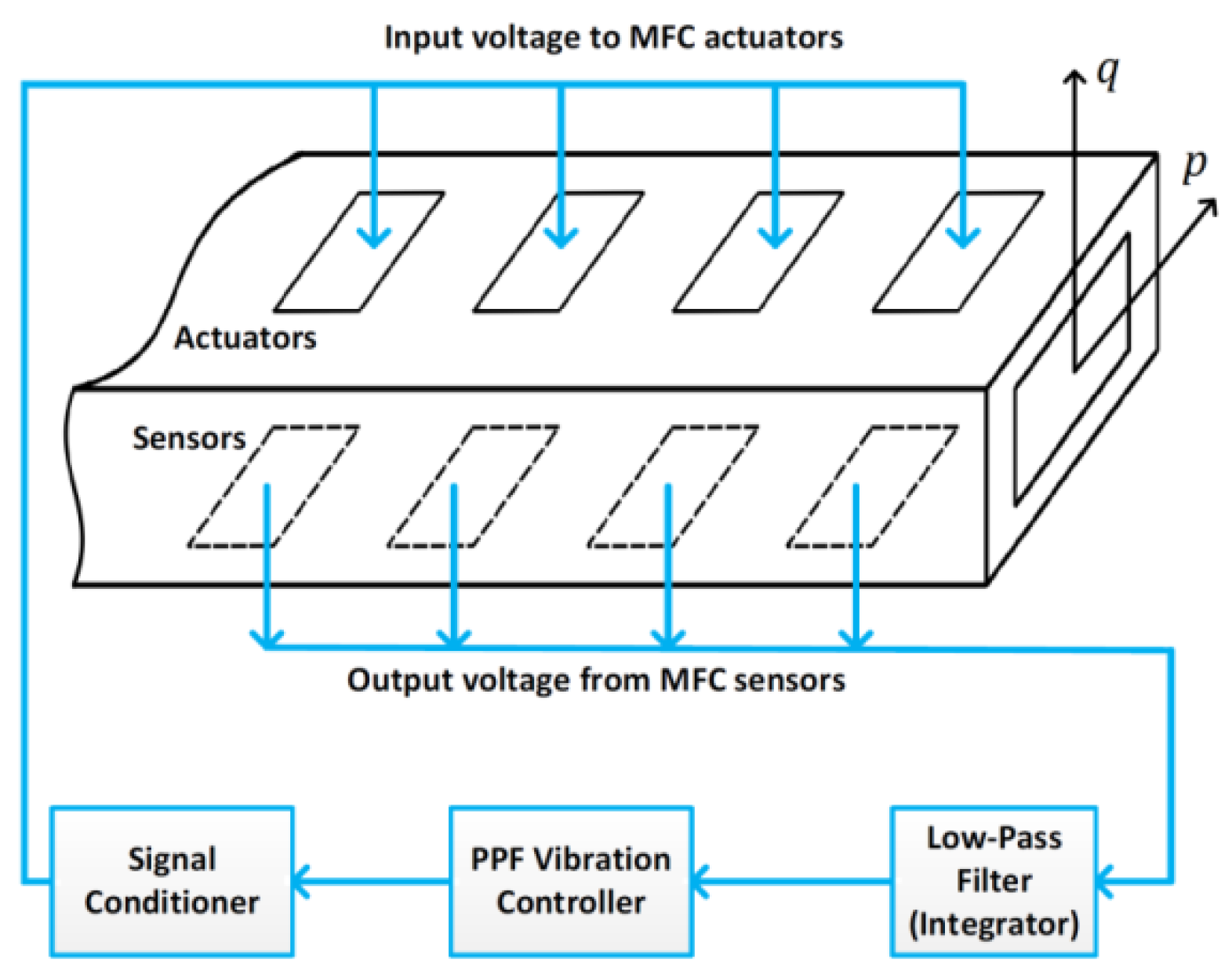
2. Equations of Motion and Their Approximate Solutions
3. Results and Discussion
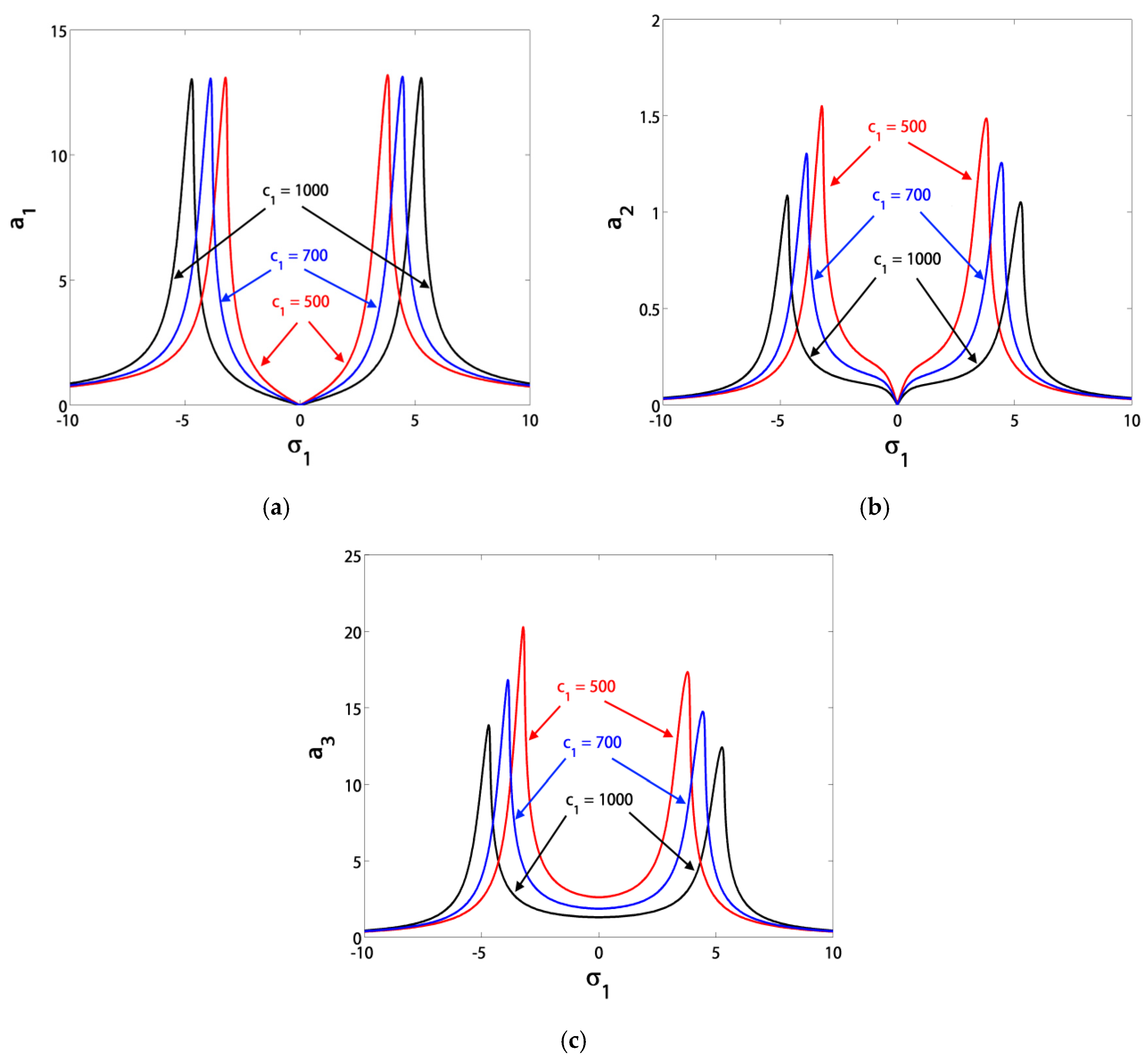
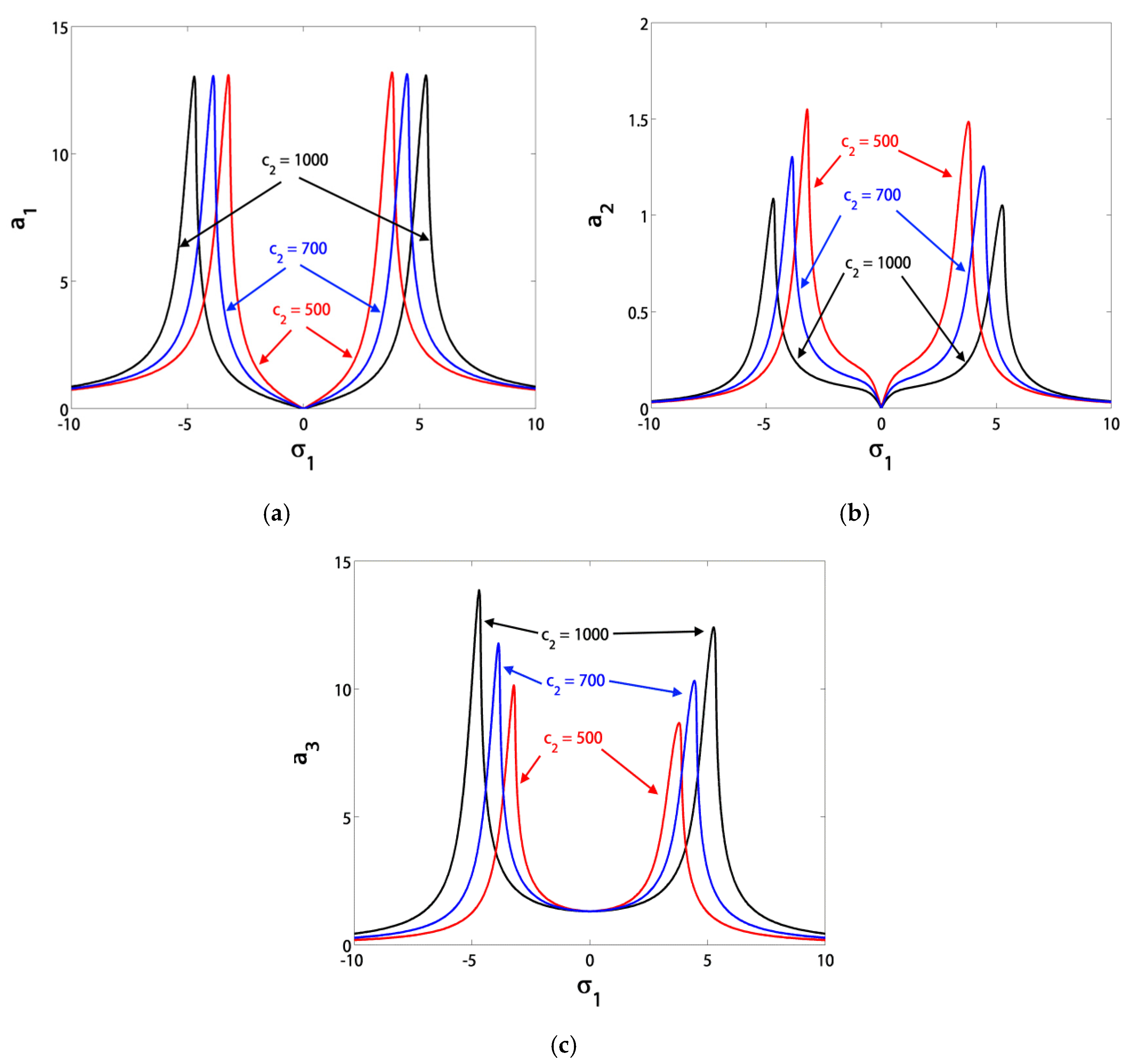
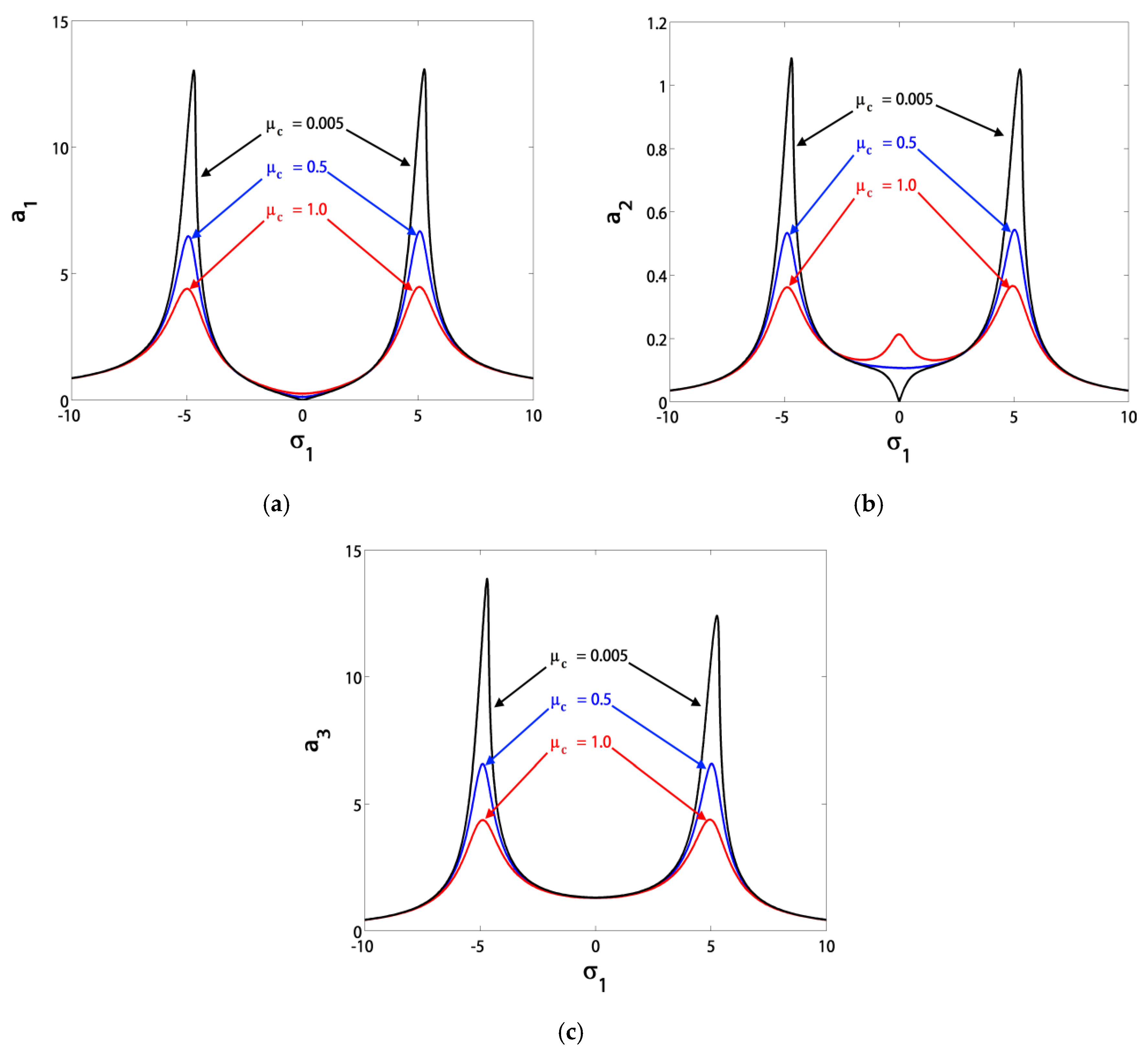
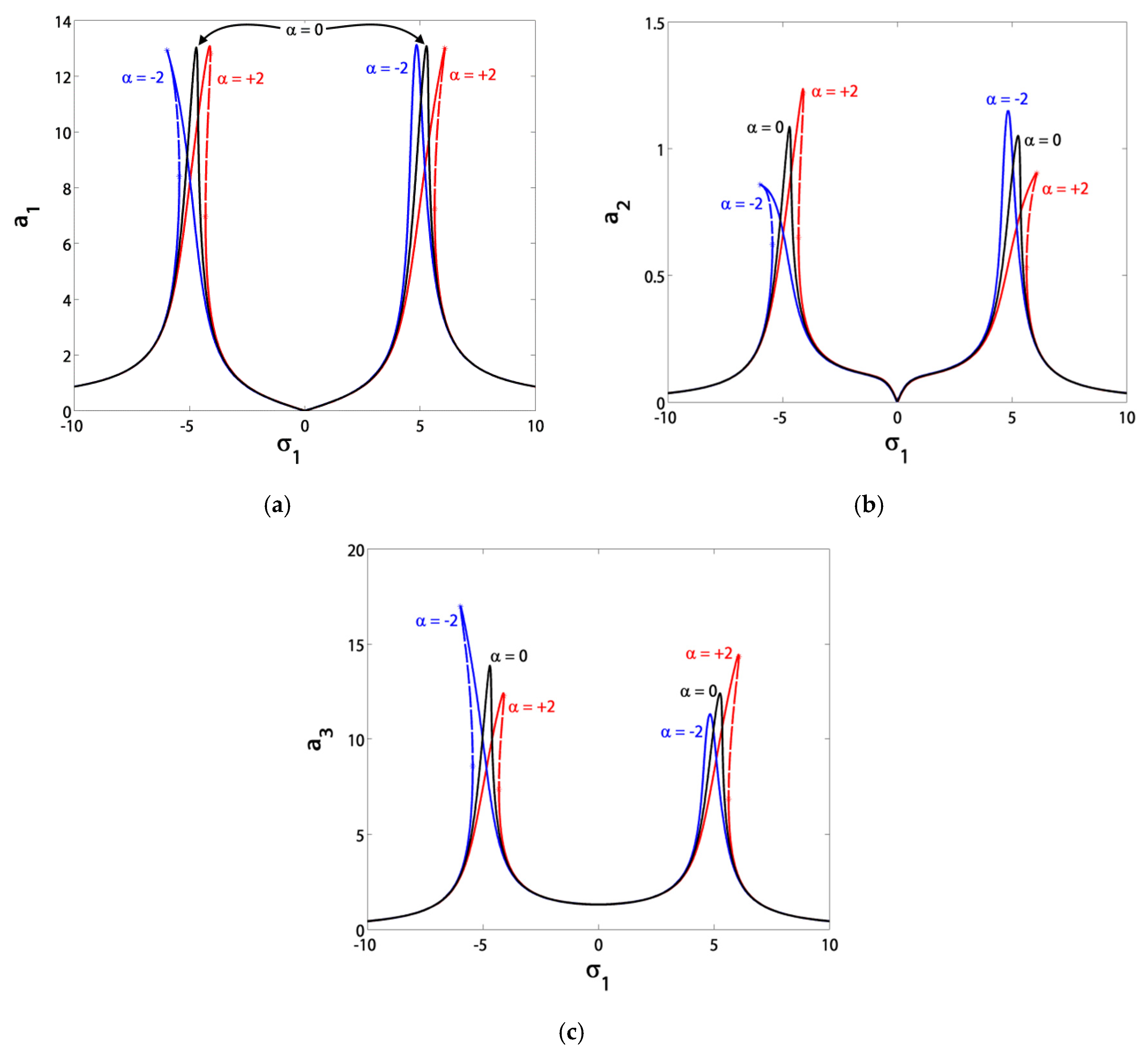
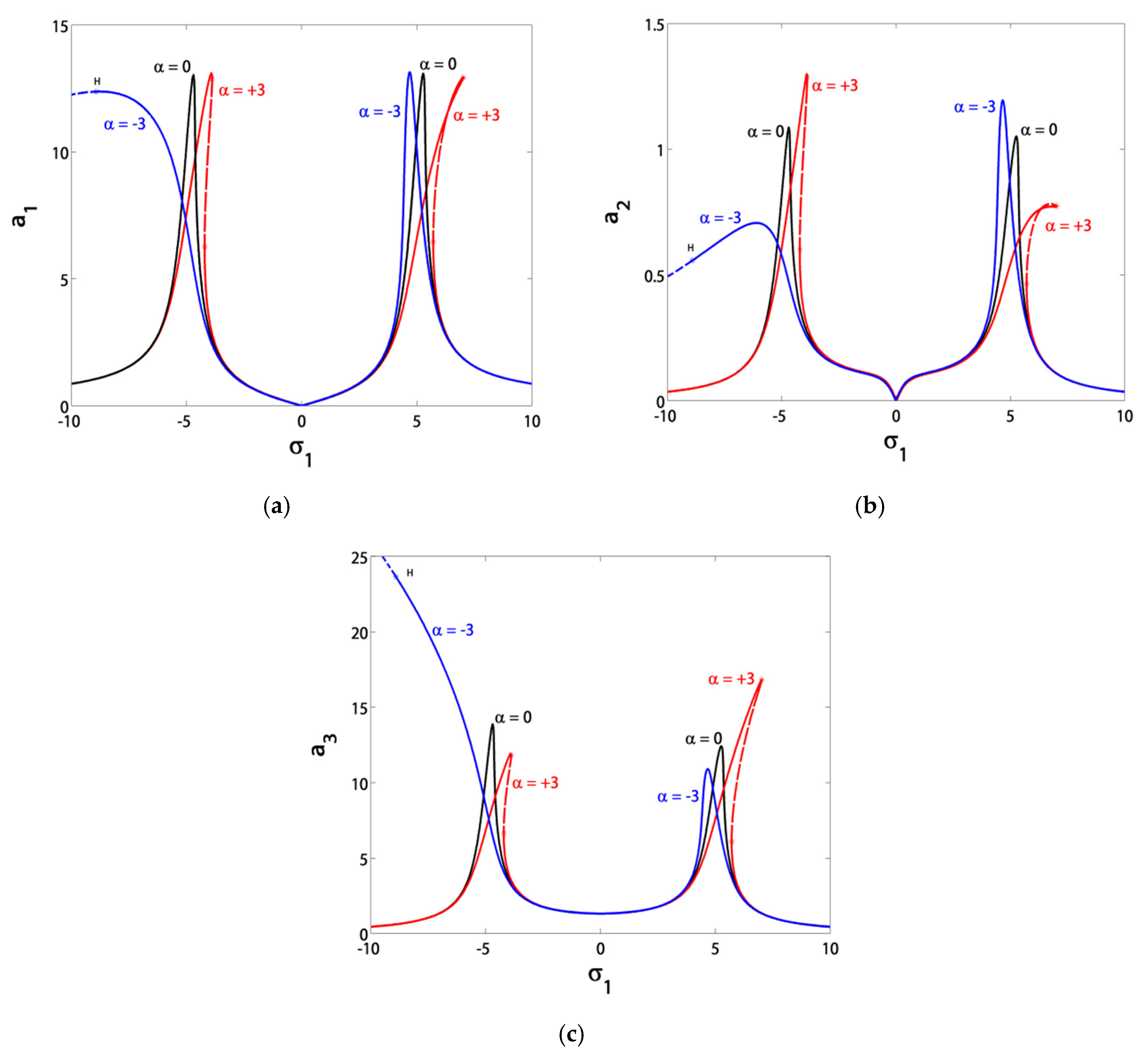
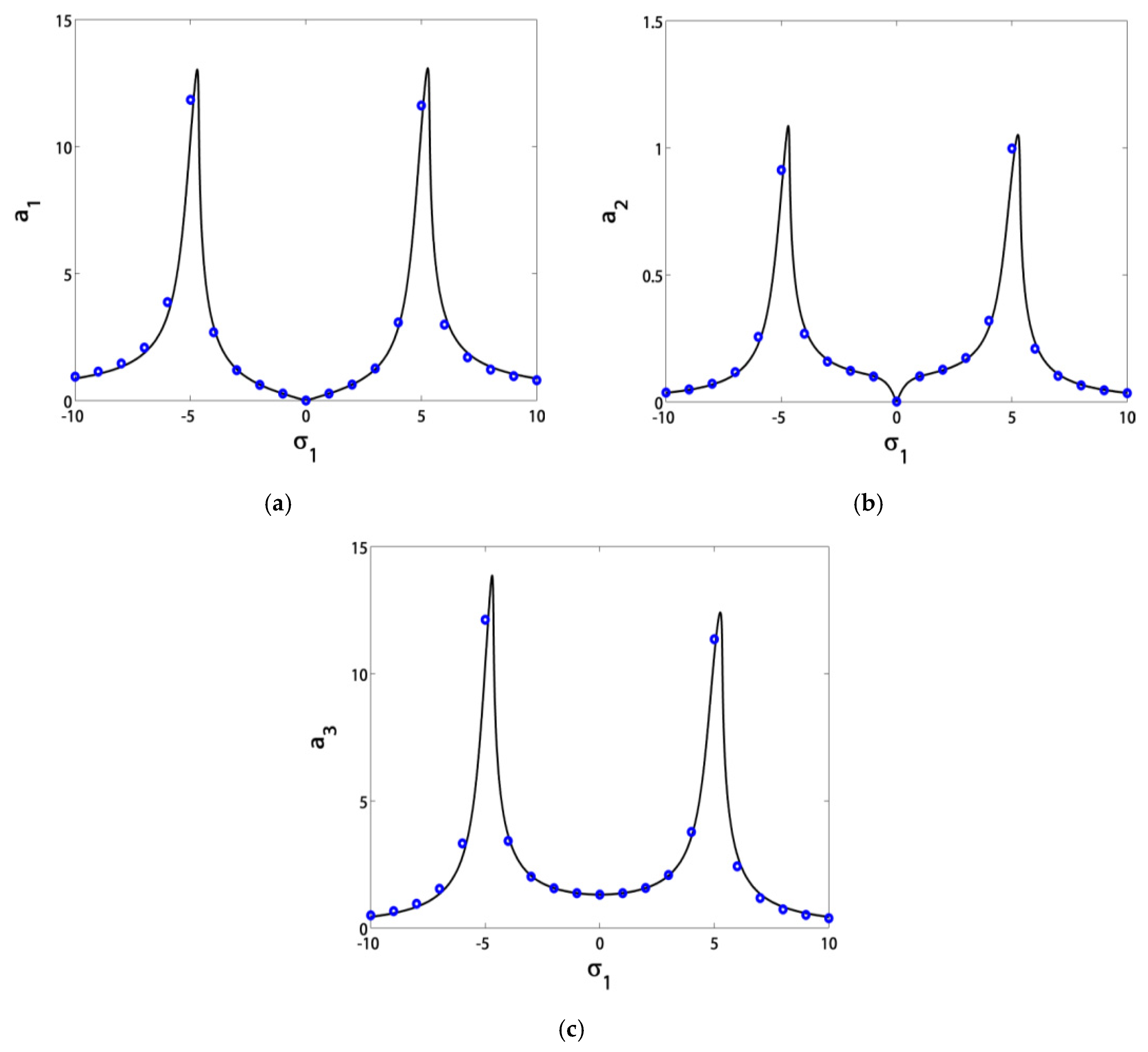
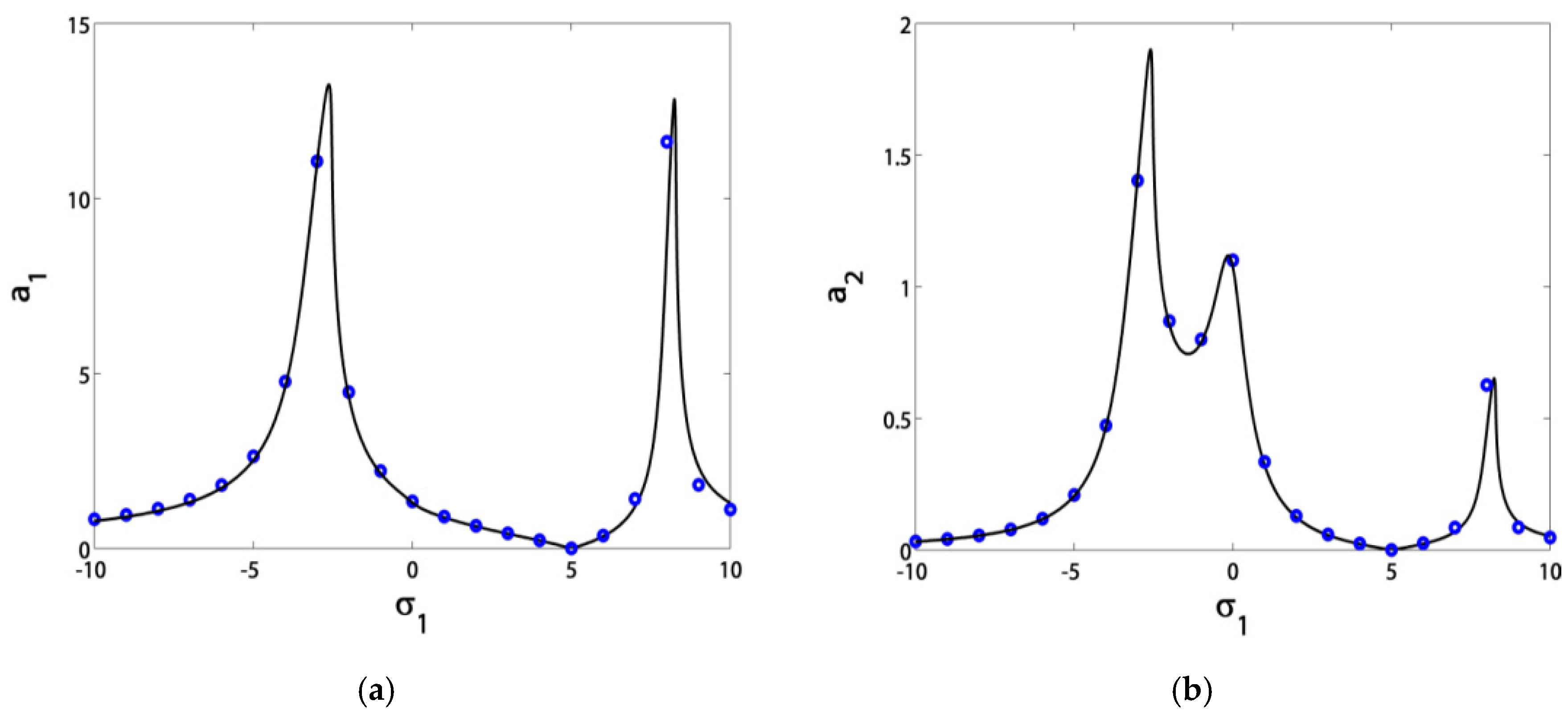
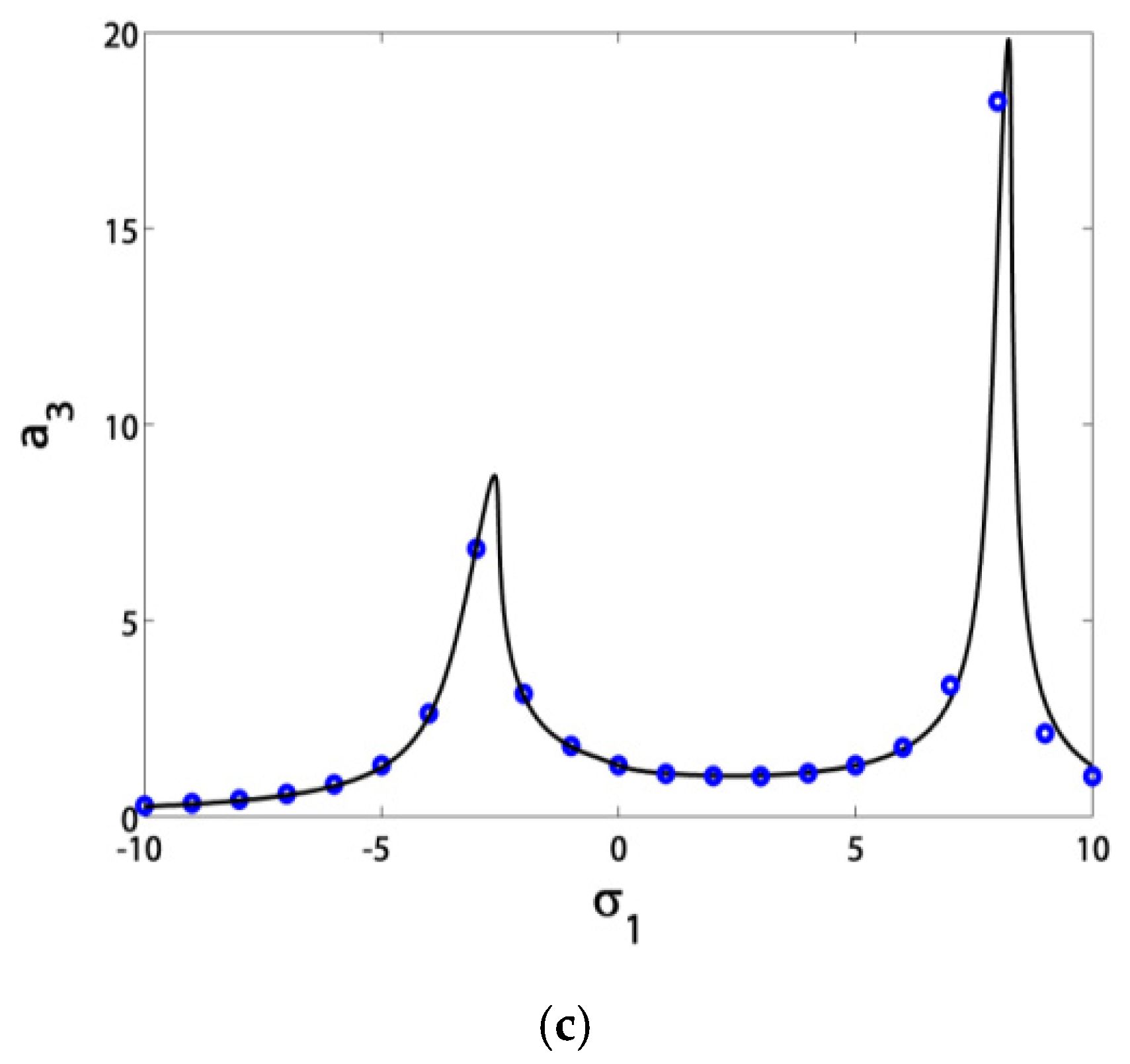
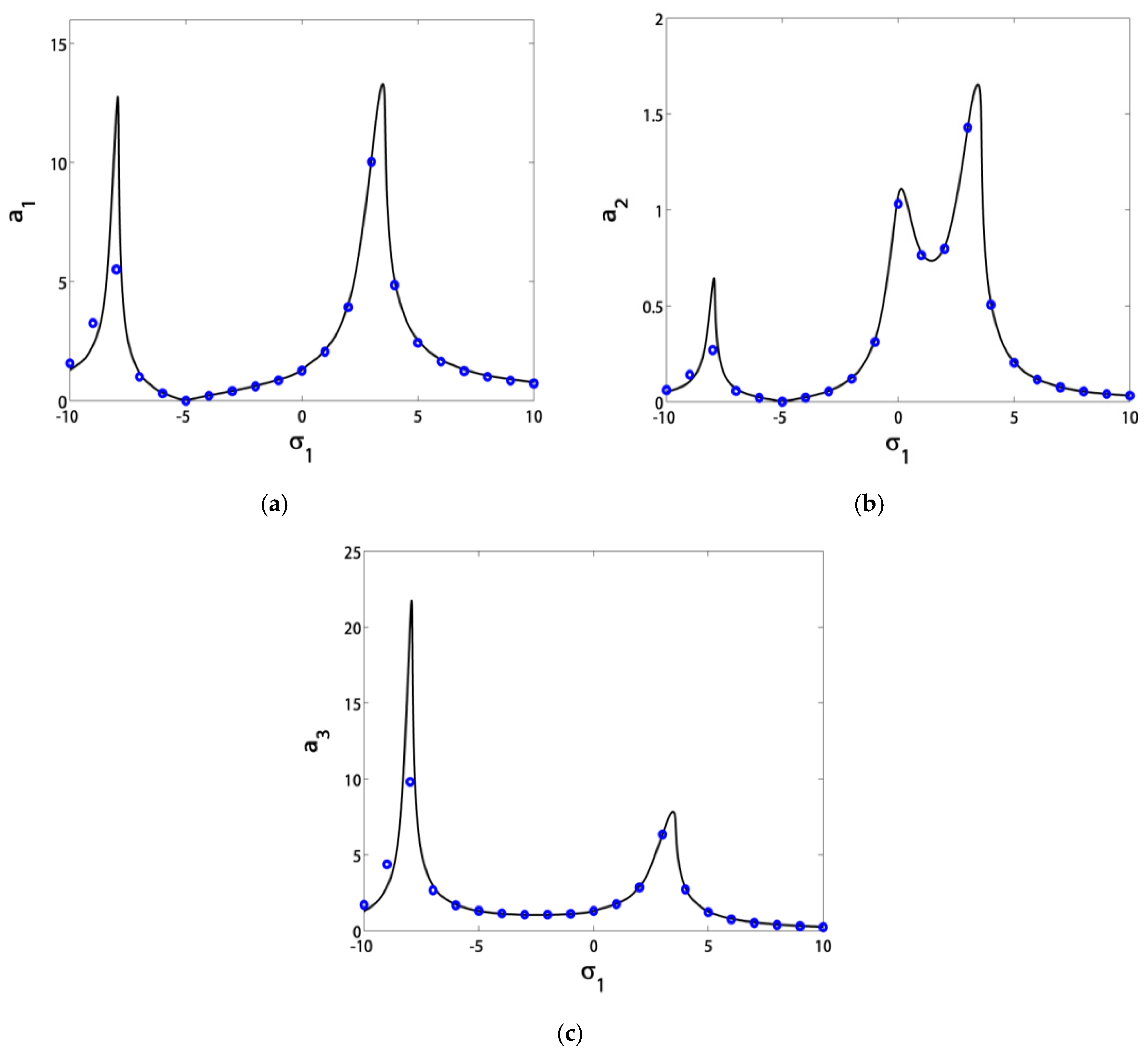
3.1. Effects of the Parameter Variation on the Blade Steady-State Vibrational Amplitudes
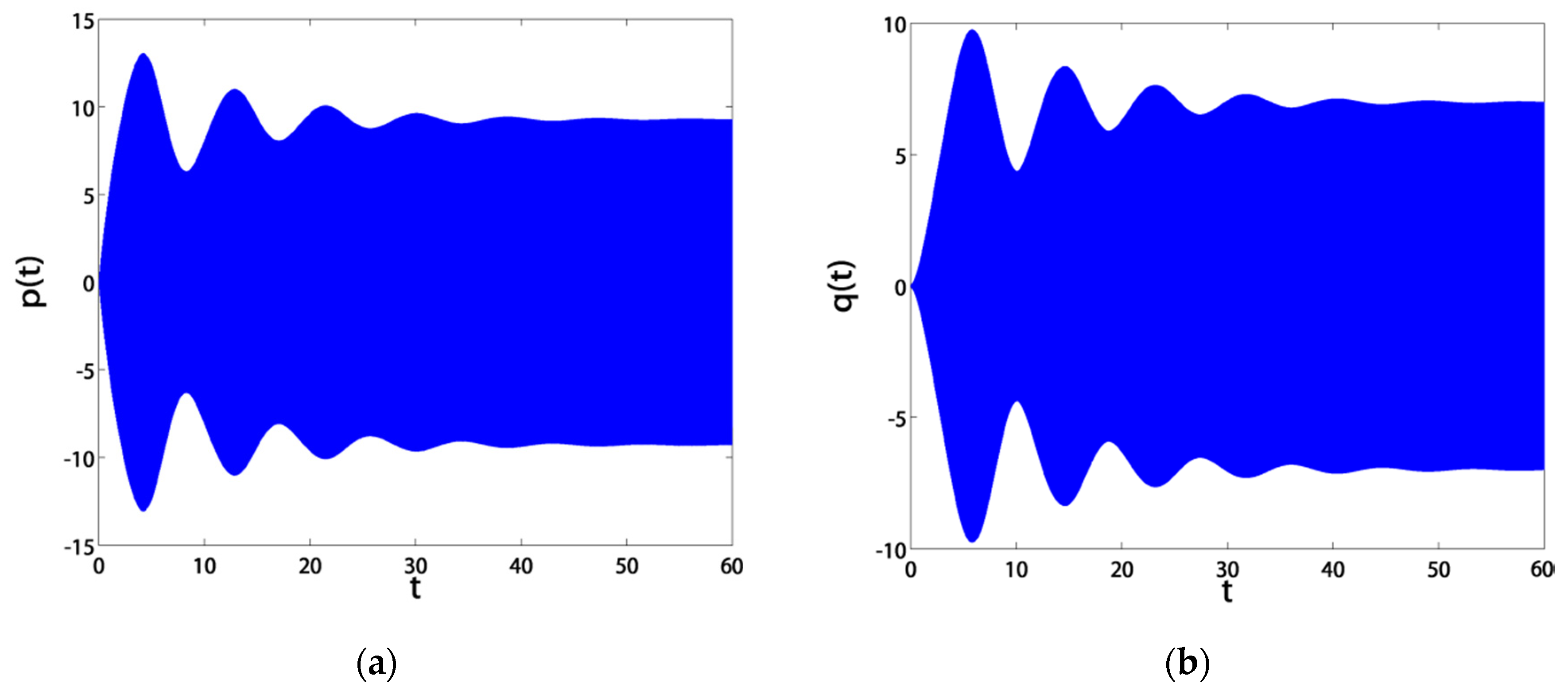
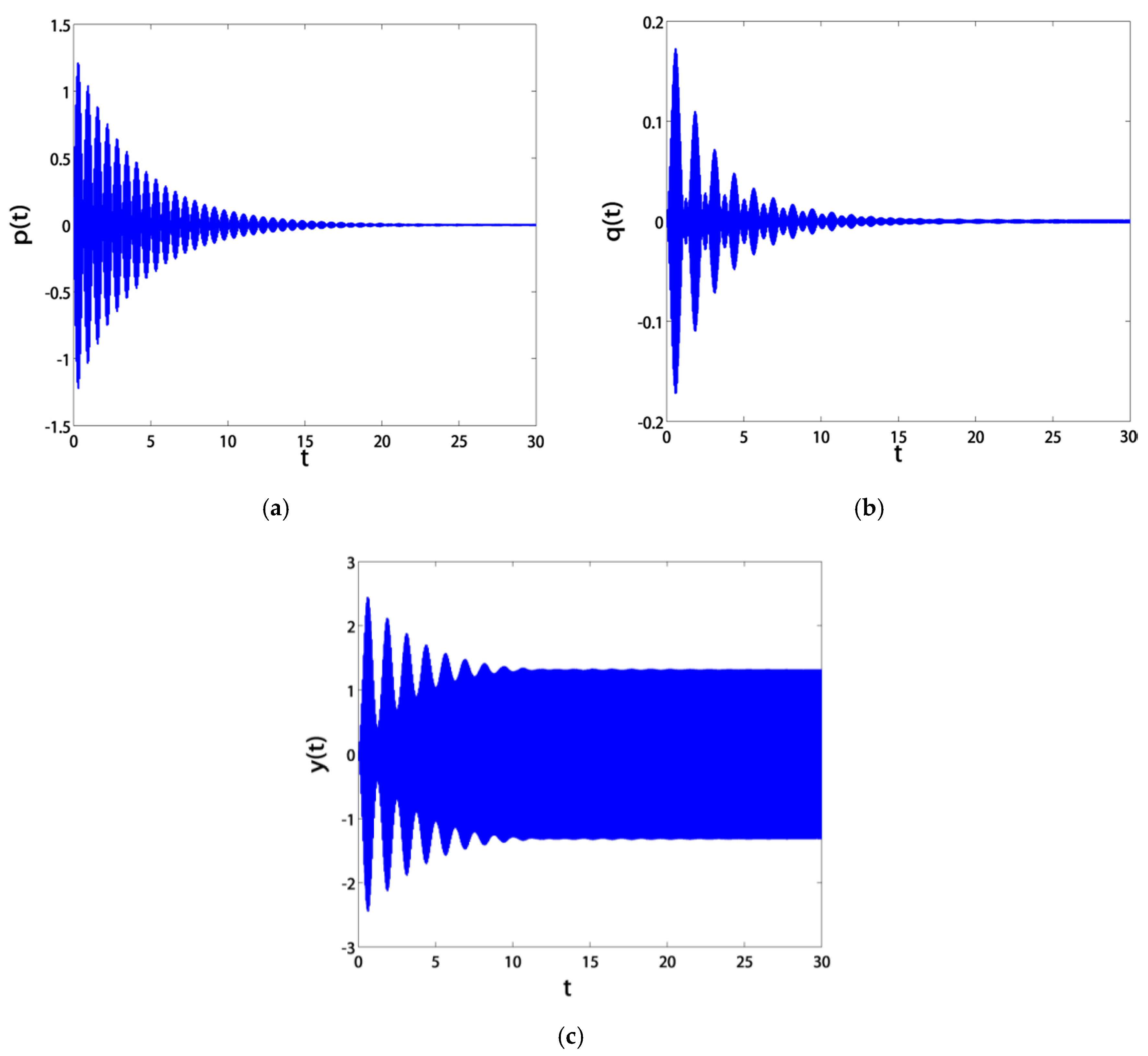
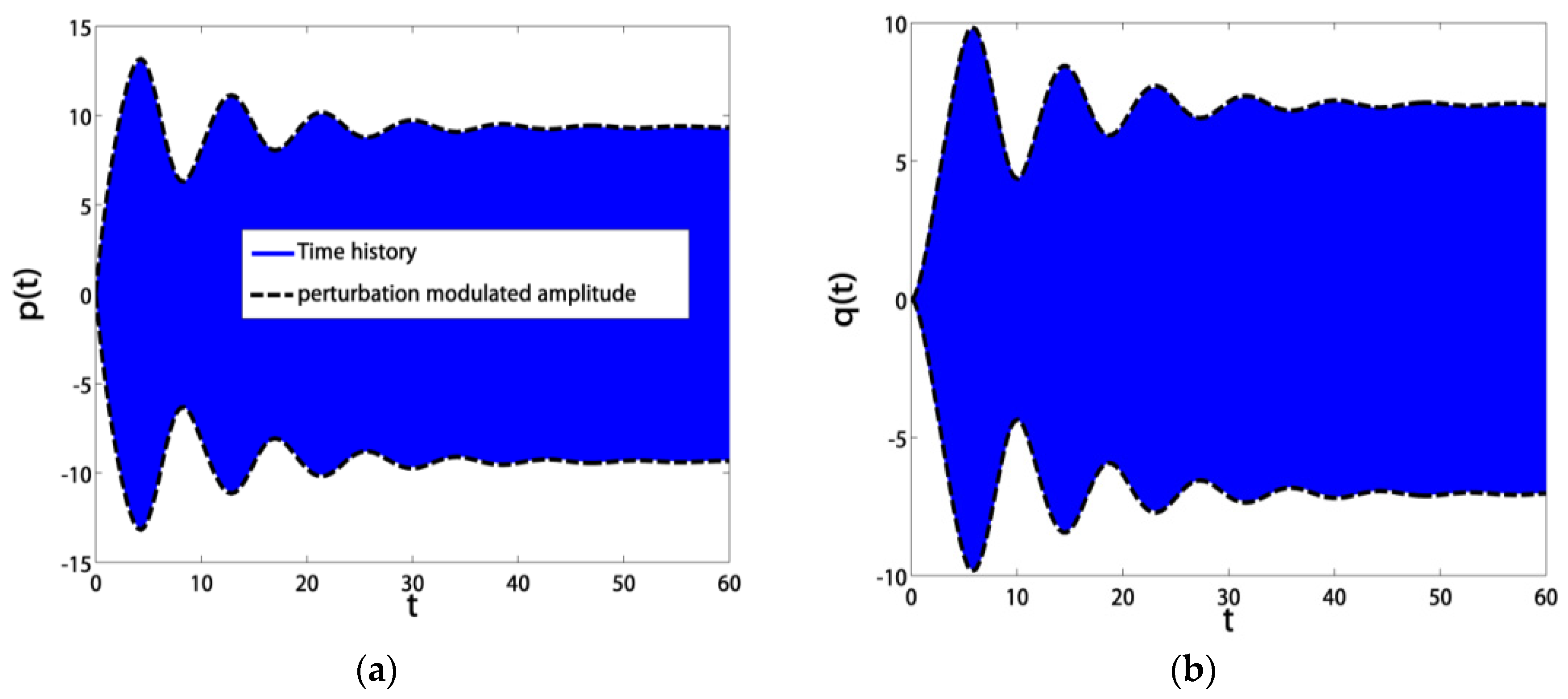
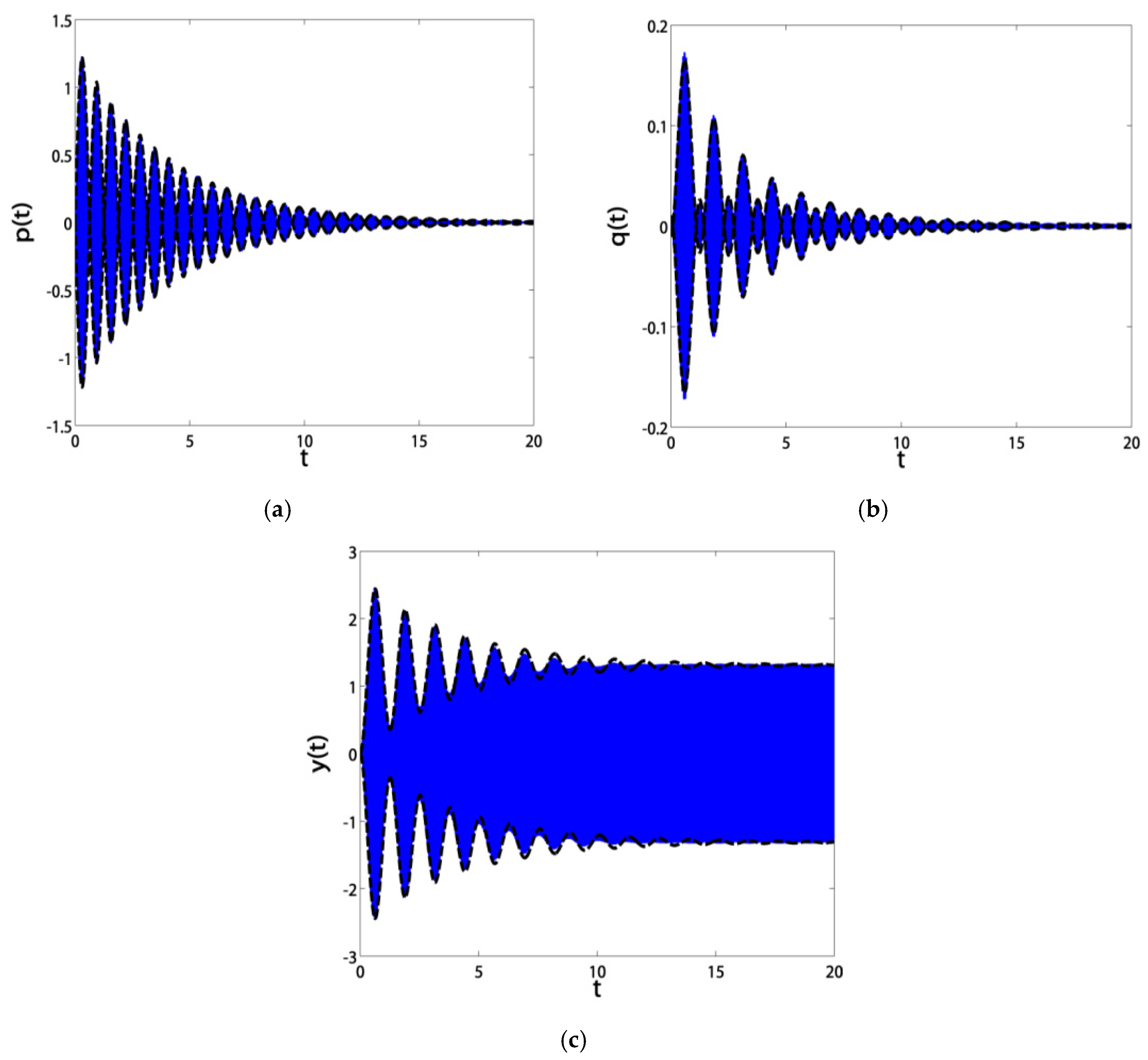
3.2. Time Responses
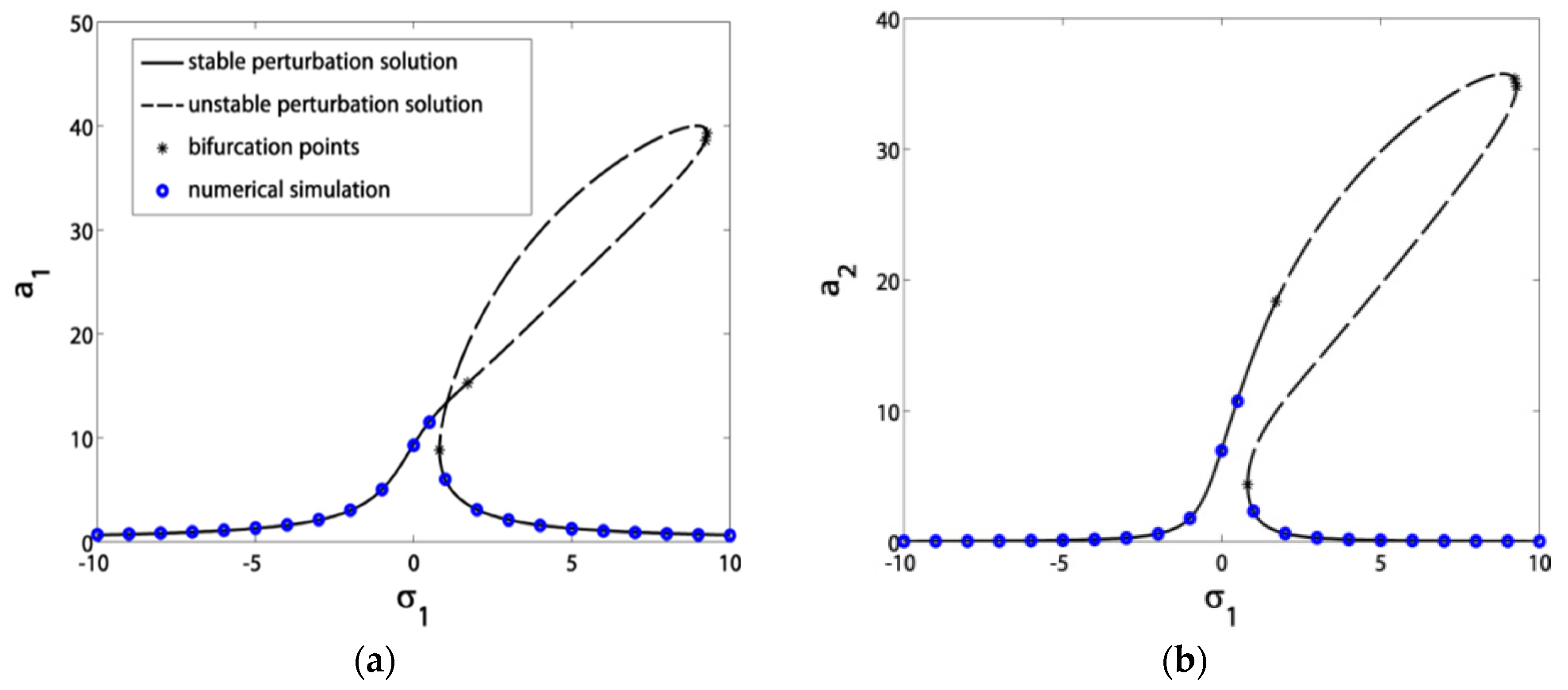
3.3. Validation Curves
4. Conclusions
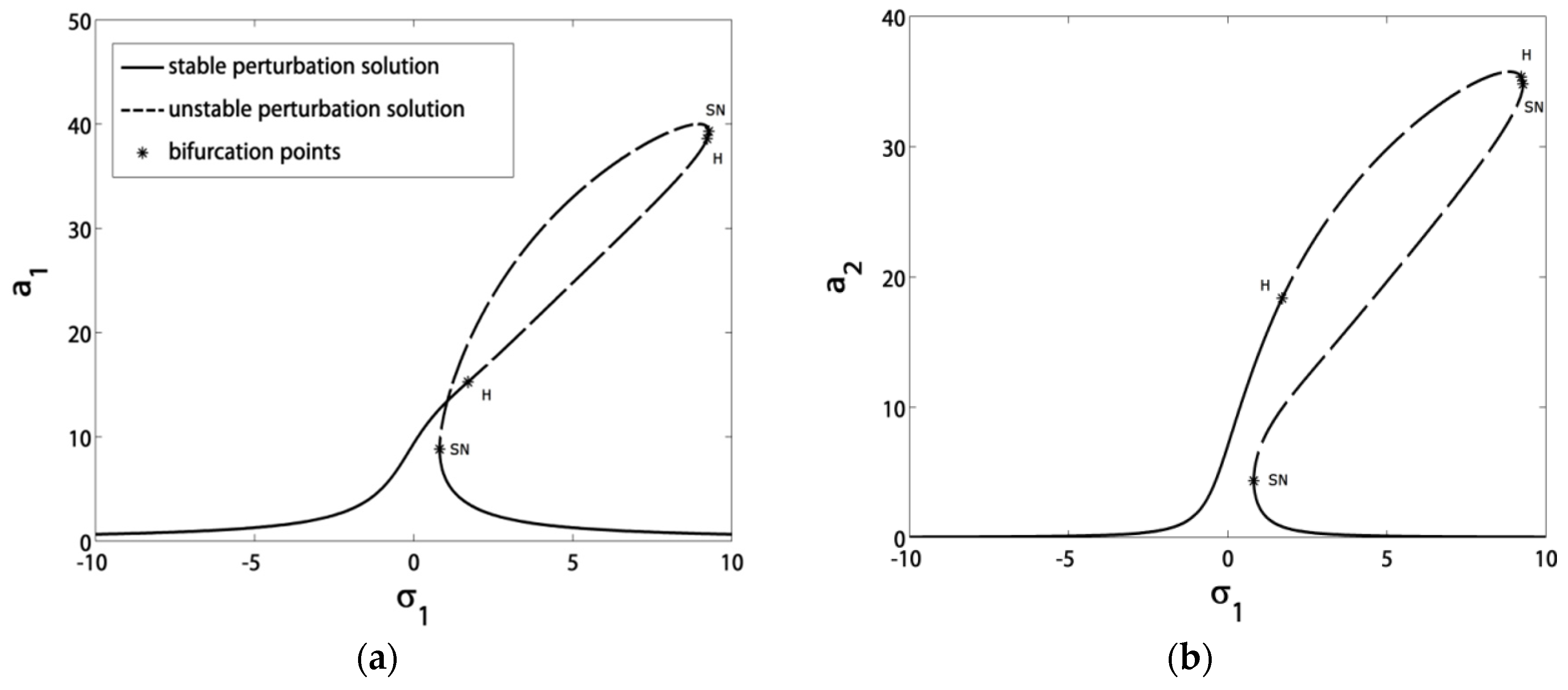
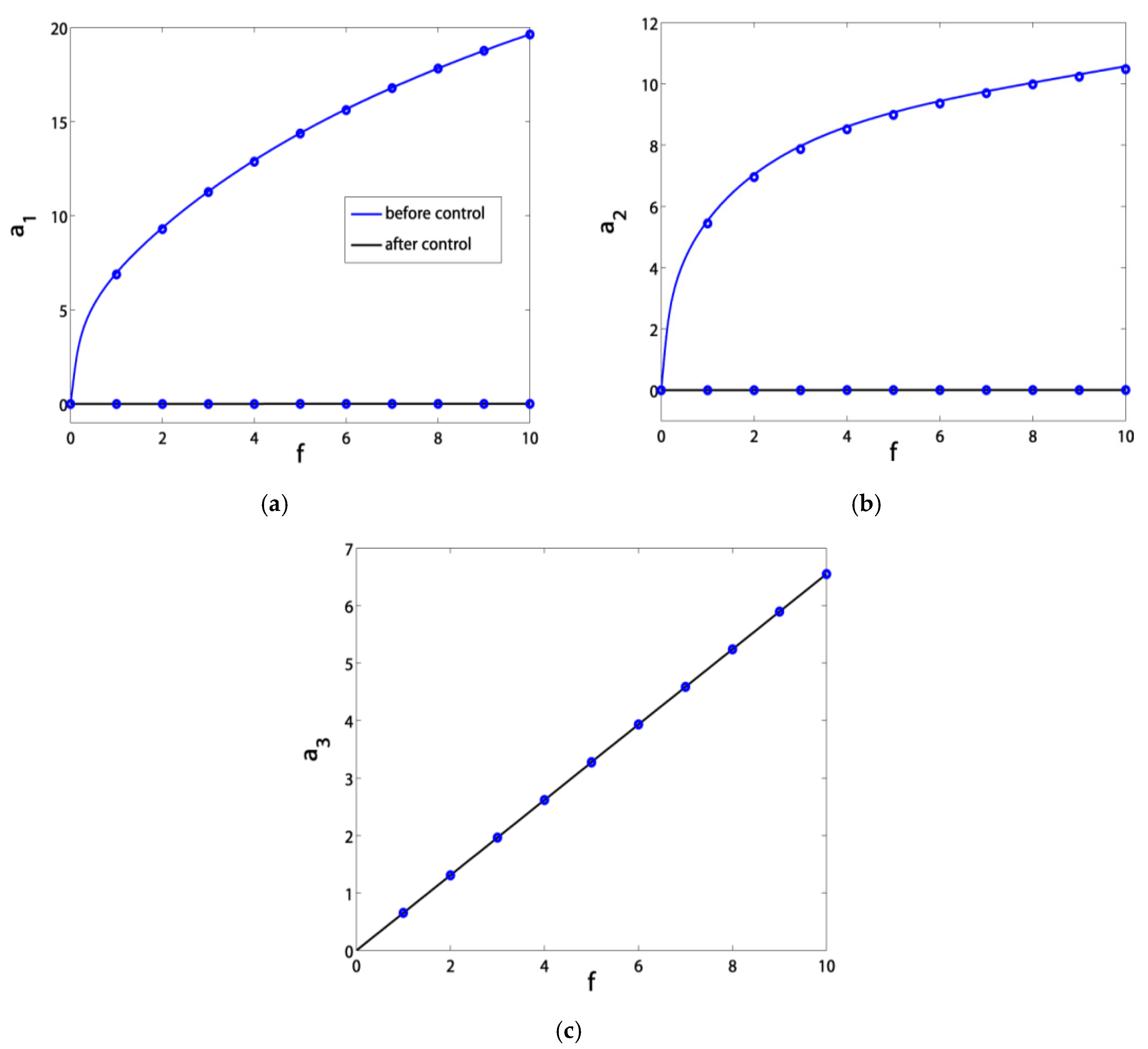
- Before control, the blade suffered from severe vibrations and jumps due to the existence of bifurcation points. After control, the blade exhibited stable solutions without jumps due to the absence of bifurcation points;
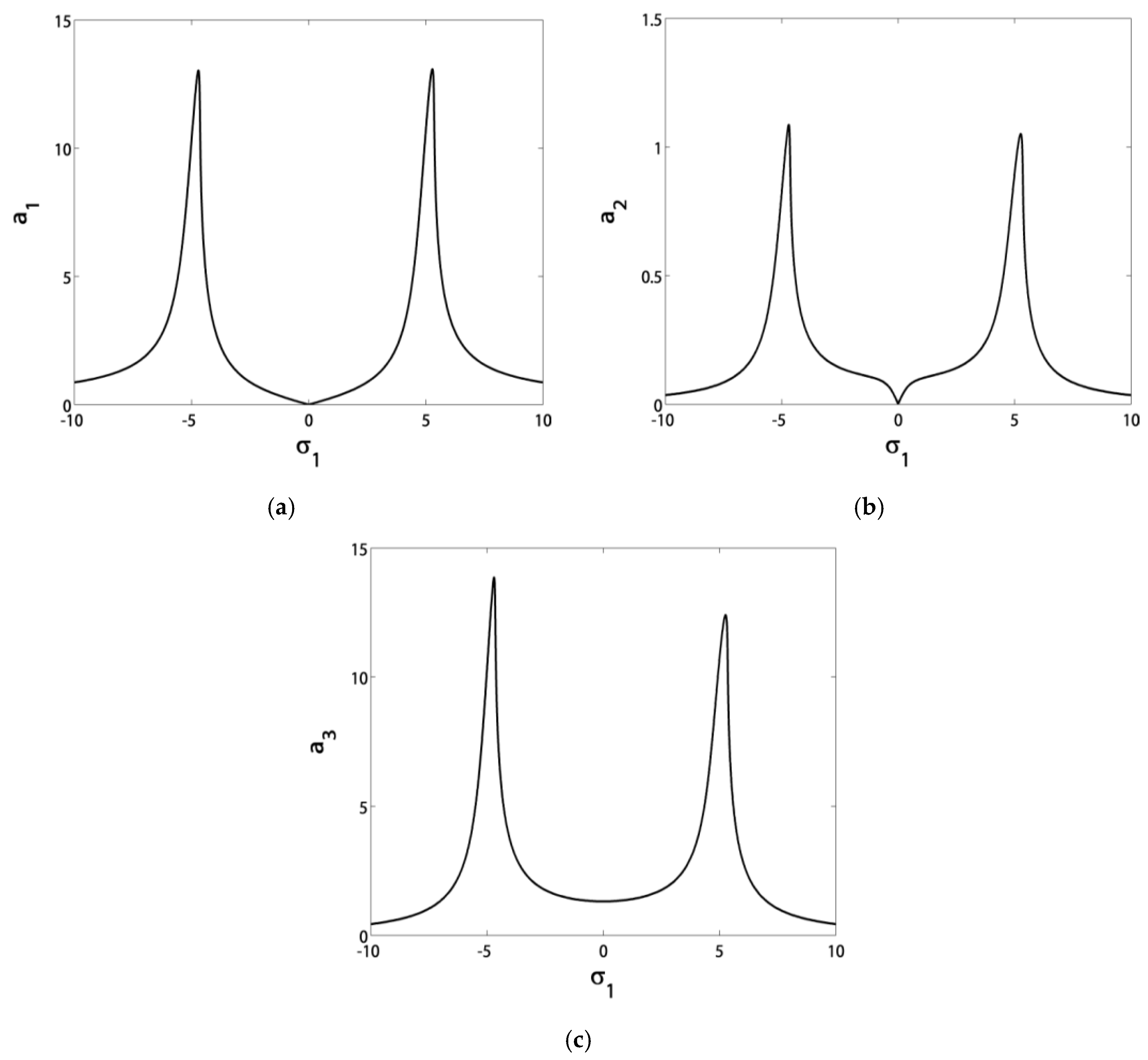
- The blade vibrations reached minimum levels in the range of σ1 ∈ [−3, 3], especially at σ1 = 0;
- The minimum amplitude bandwidth could be adjusted via the control signal gain c1 or the feedback signal gain c2;
- If we guaranteed that σ1 = σ2, then the blade operated safely in the range of σ1 ∈ [−5, 5];
- The controller damping μc was inversely proportional with the minimum vibratory level reached at σ1 = σ2;
- The controller nonlinearity parameter α should stay in the range of α ∈ (−3, 3), either for hardening or softening effects in the response curves without creating new jumps;
- The blade vibration amplitudes were very sensitive to small rises in the excitation amplitude f before control. However, after control, they became saturated at a level of almost zero thanks to channeling most of the vibration energy to the controller.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| p, q, p, q, p, q | Horizontal and vertical displacements, velocities, and accelerations of the blade’s cross-section. |
| , , | Acceleration, velocity, and displacement of the PPF controller. |
| , | Damping coefficients of the blade and controller. |
| , | Linear natural frequencies of the blade and controller. |
| , , , , | Coupling factors between the blade’s vibrational directions. |
| , | Cubic nonlinearity coefficients of the blade and controller. |
| , | Parametric excitation coefficients. |
| , | Amplitudes of the excitation force. |
| , , | Excitation frequency and detuning parameters. |
| , | Gains of the control and feedback signals. |
Appendix A
- There will be no deformation in the cross-section for the long-term operation;
- The blade’s thickness is very small, compared with its radius of gyration;
- The blade can be considered an Euler–Bernoulli beam to neglect the shear force transversally;
- In addition, we can neglect the elongation axially compared to the shown deflections.
Appendix B
References
- Yoo, H.H.; Kwak, J.Y.; Chung, J. Vibration analysis of rotating pre-twisted blades with a concentrated mass. J. Sound Vib. 2001, 240, 891–908. [Google Scholar] [CrossRef]
- Lin, C.Y.; Chen, L.W. Dynamic stability of rotating pre-twisted blades with a constrained damping layer. Compos. Struct. 2003, 61, 235–245. [Google Scholar] [CrossRef]
- Oh, S.Y.; Song, O.; Librescu, L. Effects of pretwist and presetting on coupled bending vibrations of rotating thin-walled composite beams. Int. J. Solids Struct. 2003, 40, 1203–1224. [Google Scholar] [CrossRef]
- Librescu, L.; Oh, S.Y.; Song, O. Spinning thin-walled beams made of functionally graded materials: Modeling, vibration and instability. Eur. J. Mech. A Solids 2004, 23, 499–515. [Google Scholar] [CrossRef]
- Sinha, S.K. Dynamic characteristics of a flexible bladed-rotor with Coulomb damping due to tip-rub. J. Sound Vib. 2004, 273, 875–919. [Google Scholar] [CrossRef]
- Hamdan, M.N.; El-Sinawi, A.H. On the non-linear vibrations of an inextensible rotating arm with setting angle and flexible hub. J. Sound Vib. 2005, 281, 375–398. [Google Scholar] [CrossRef]
- Choi, S.C.; Park, J.S.; Kim, J.H. Active damping of rotating composite thin-walled beams using MFC actuators and PVDF sensors. Compos. Struct. 2006, 76, 362–374. [Google Scholar] [CrossRef]
- Choi, S.C.; Park, J.S.; Kim, J.H. Vibration control of pre-twisted rotating composite thin-walled beams with piezoelectric fiber composites. J. Sound Vib. 2007, 300, 176–196. [Google Scholar] [CrossRef]
- Fazelzadeh, S.A.; Malekzadeh, P.; Zahedinejad, P.; Hosseini, M. Vibration analysis of functionally graded thin-walled rotating blades under high temperature supersonic flow using the differential quadrature method. J. Sound Vib. 2007, 306, 333–348. [Google Scholar] [CrossRef]
- Vadiraja, D.N.; Sahasrabudhe, A.D. Vibration analysis and optimal control of rotating pre-twisted thin-walled beams using MFC actuators and sensors. Thin-Walled Struct. 2009, 47, 555–567. [Google Scholar] [CrossRef]
- Younesian, D.; Esmailzadeh, E. Vibration suppression of rotating beams using time-varying internal tensile force. J. Sound Vib. 2011, 330, 308–320. [Google Scholar] [CrossRef]
- Yao, M.H.; Chen, Y.P.; Zhang, W. Nonlinear vibrations of blade with varying rotating speed. Nonlinear Dyn. 2012, 68, 487–504. [Google Scholar] [CrossRef]
- Yao, M.H.; Zhang, W.; Chen, Y.P. Analysis on nonlinear oscillations and resonant responses of a compressor blade. Acta Mech. 2014, 225, 3483–3510. [Google Scholar] [CrossRef]
- Latalski, J.; Warminski, J.; Rega, G. Bending-twisting vibrations of a rotating hub-thin-walled composite beam system. Math. Mech. Solids 2017, 22, 1303–1325. [Google Scholar] [CrossRef]
- Rafiee, M.; Nitzsche, F.; Labrosse, M. Dynamics, vibration and control of rotating composite beams and blades: A critical review. Thin-Walled Struct. 2017, 119, 795–819. [Google Scholar] [CrossRef]
- Chen, J.; Li, Q.S. Vibration characteristics of a rotating pre-twisted composite laminated blade. Compos. Struct. 2019, 208, 78–90. [Google Scholar] [CrossRef]
- Liu, T.R.; Gong, A.L. Theoretical modeling and vibration control for pre-twisted composite blade based on LLI controller. Trans. Inst. Meas. Control. 2020, 42, 1255–1270. [Google Scholar] [CrossRef]
- Zhang, Y.F.; Niu, Y.; Zhang, W. Nonlinear vibrations and internal resonance of pretwisted rotating cantilever rectangular plate with varying cross-section and aerodynamic force. Eng. Struct. 2020, 225, 111259. [Google Scholar] [CrossRef]
- Zhang, W.; Liu, G.; Siriguleng, B. Saturation phenomena and nonlinear resonances of rotating pretwisted laminated composite blade under subsonic air flow excitation. J. Sound Vib. 2020, 478, 115353. [Google Scholar] [CrossRef]
- Zhang, W.; Niu, Y.; Behdinan, K. Vibration characteristics of rotating pretwisted composite tapered blade with graphene coating layers. Aerosp. Sci. Technol. 2020, 98, 105644. [Google Scholar] [CrossRef]
- Wang, Z.; Almeida, J.H.S.; St-Pierre, L.; Wang, Z.; Castro, S.G.P. Reliability-based buckling optimization with an accelerated Kriging metamodel for filament-wound variable angle tow composite cylinders. Compos. Struct. 2020, 254. [Google Scholar] [CrossRef]
- Almeida, J.H.S.; Bittrich, L.; Spickenheuer, A. Improving the open-hole tension characteristics with variable-axial composite laminates: Optimization, progressive damage modeling and experimental observations. Compos. Sci. Technol. 2020, 185. [Google Scholar] [CrossRef]
- Monticeli, F.M.; Almeida, J.H.S.; Neves, R.M.; Ornaghi, F.G.; Ornaghi, H.L. On the 3D void formation of hybrid carbon/glass fiber composite laminates: A statistical approach. Compos. Part A Appl. Sci. Manuf. 2020, 137, 106036. [Google Scholar] [CrossRef]
- Shin, C.; Hong, C.; Jeong, W.B. Active vibration control of clamped beams using positive position feedback controllers with moment pair. J. Mech. Sci. Technol. 2012, 26, 731–740. [Google Scholar] [CrossRef]
- El-Ganaini, W.A.; Saeed, N.A.; Eissa, M. Positive position feedback (PPF) controller for suppression of nonlinear system vibration. Nonlinear Dyn. 2013, 72, 517–537. [Google Scholar] [CrossRef]
- El-Ganaini, W.A.; Kandil, A.; Eissa, M.; Kamel, M. Effects of delayed time active controller on the vibration of a nonlinear magnetic levitation system to multi excitations. J. Vib. Control. 2016, 22, 1257–1275. [Google Scholar] [CrossRef]
- Hamed, Y.S.; El Shehry, A.; Sayed, M. Nonlinear modified positive position feedback control of cantilever beam system carrying an intermediate lumped mass. Alex. Eng. J. 2020, 59, 3847–3862. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Mook, D.T. Nonlinear Oscillations; Wiley: New York, NY, USA, 1995. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hamed, Y.S.; Kandil, A.; Machado, J.T. Utilizing Macro Fiber Composite to Control Rotating Blade Vibrations. Symmetry 2020, 12, 1984. https://doi.org/10.3390/sym12121984
Hamed YS, Kandil A, Machado JT. Utilizing Macro Fiber Composite to Control Rotating Blade Vibrations. Symmetry. 2020; 12(12):1984. https://doi.org/10.3390/sym12121984
Chicago/Turabian StyleHamed, Y. S., Ali Kandil, and José Tenreiro Machado. 2020. "Utilizing Macro Fiber Composite to Control Rotating Blade Vibrations" Symmetry 12, no. 12: 1984. https://doi.org/10.3390/sym12121984
APA StyleHamed, Y. S., Kandil, A., & Machado, J. T. (2020). Utilizing Macro Fiber Composite to Control Rotating Blade Vibrations. Symmetry, 12(12), 1984. https://doi.org/10.3390/sym12121984






