Influence of the Replacement of the Actual Plastic Orthotropy with Various Approximations of Normal Anisotropy on Residual Stresses and Strains in a Thin Disk Subjected to External Pressure
Abstract
1. Introduction
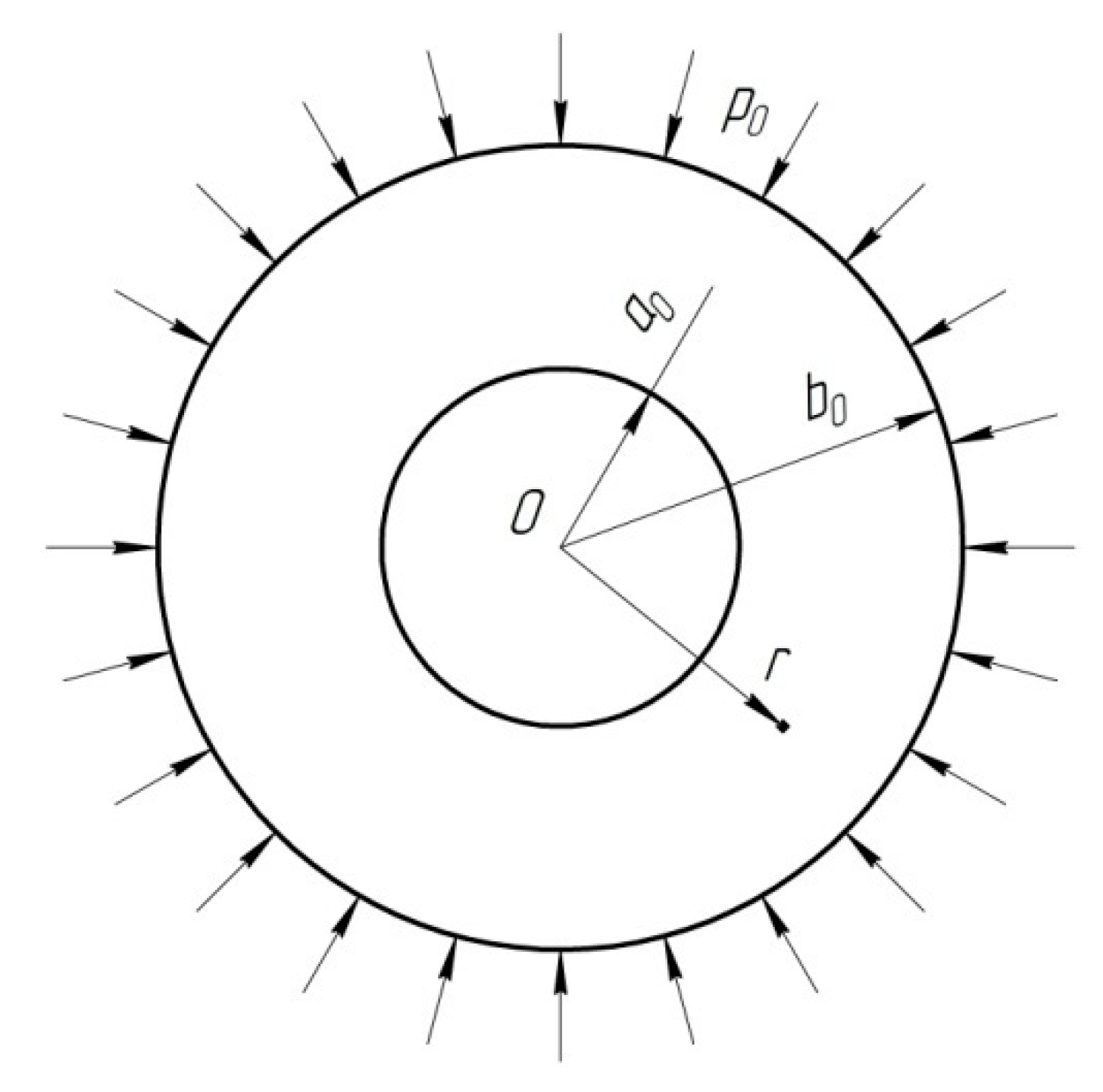
2. Statement of the Problem
3. Stress Solution
4. Strain Solution
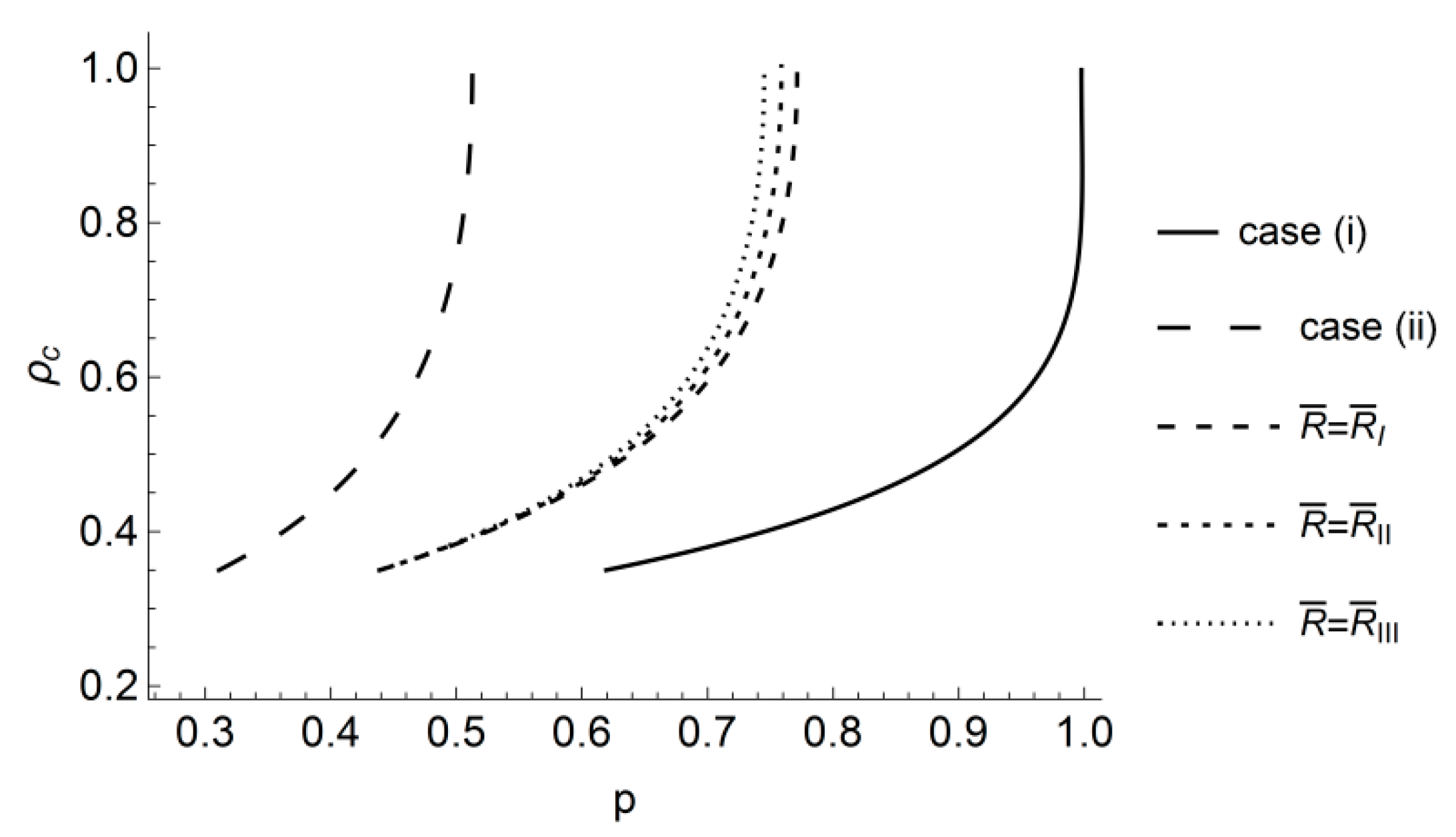
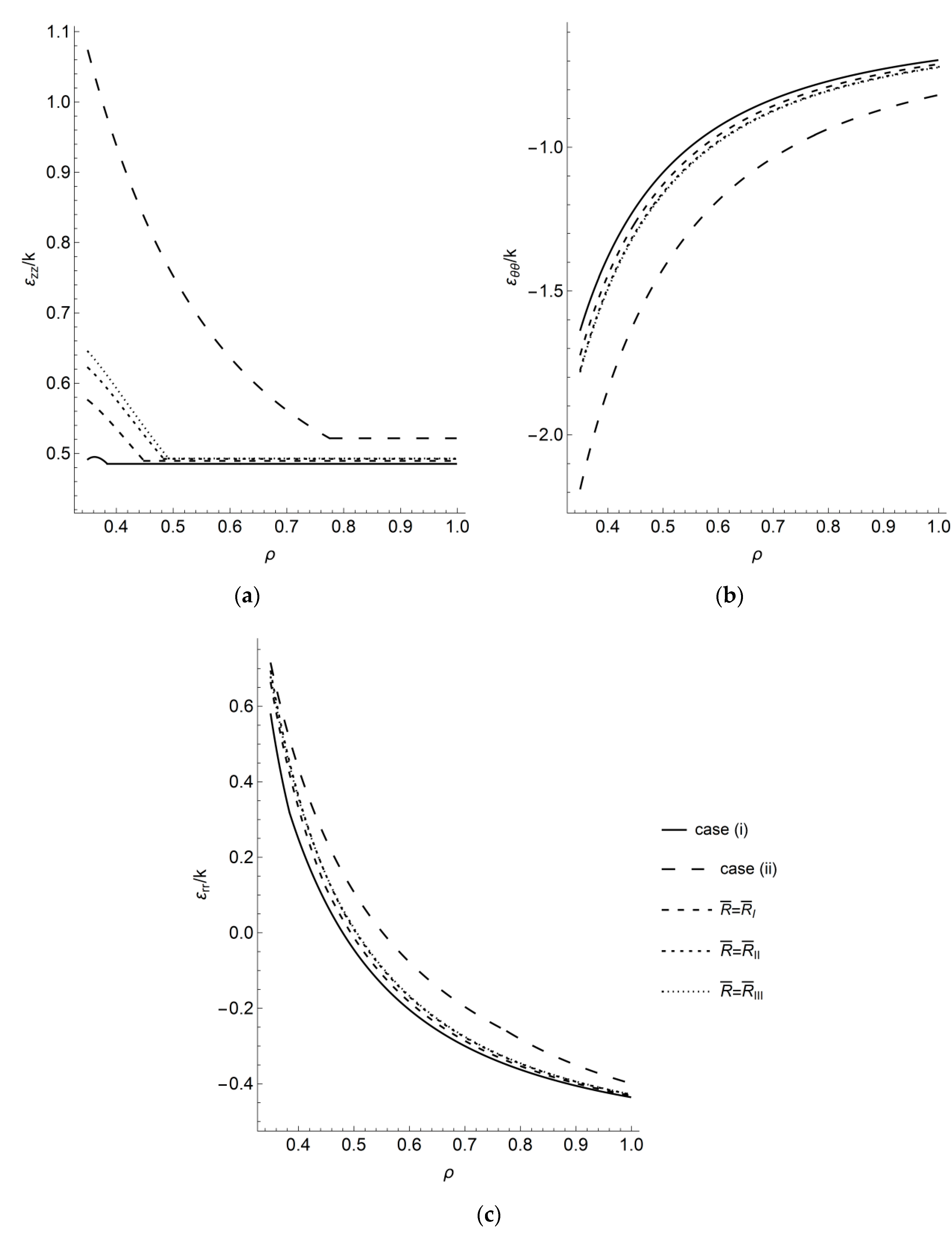
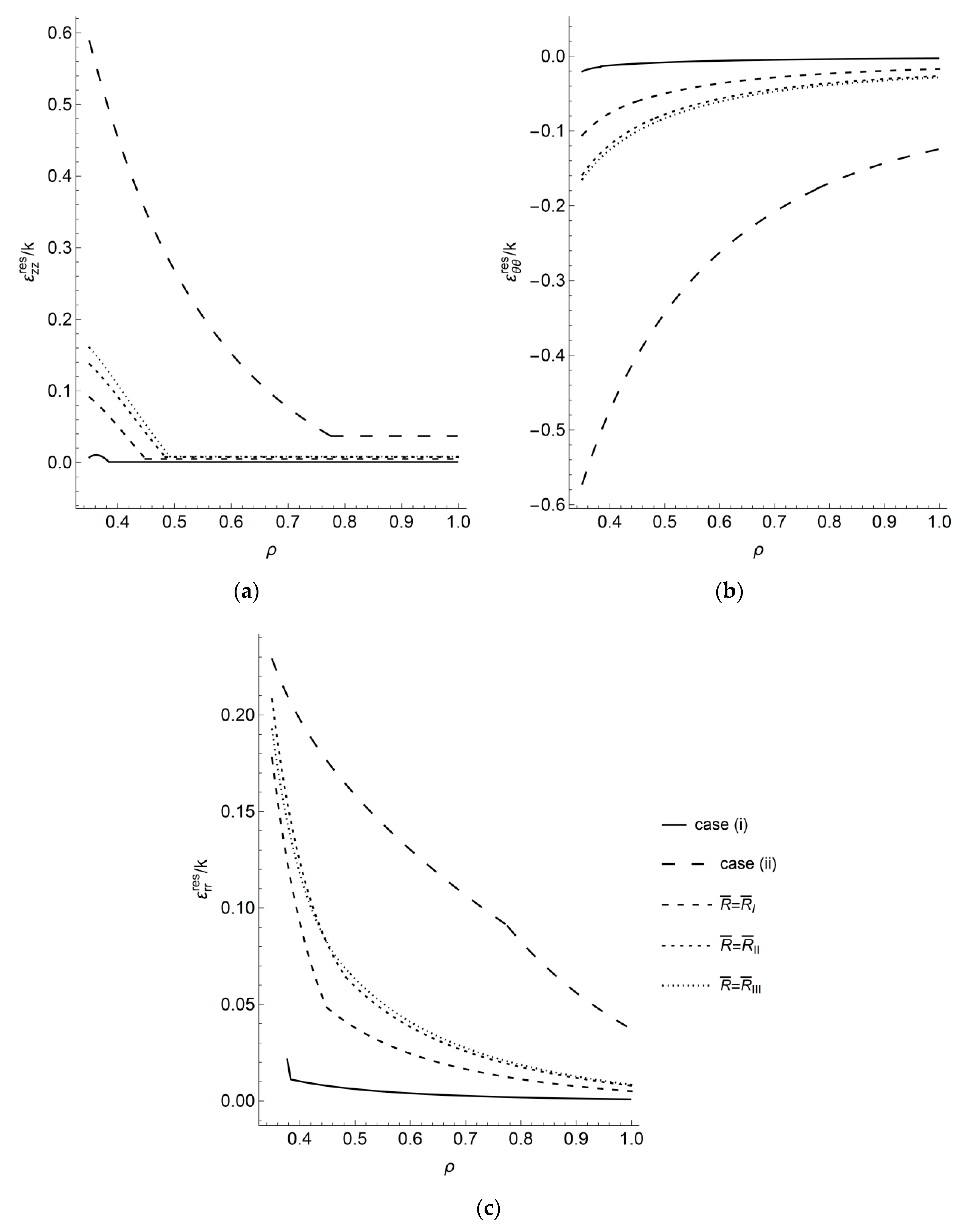
5. Numerical Example and Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| a0, b0 | inner and outer radius of hollow disk |
| external pressure | |
| cylindrical coordinate system | |
| , , | normal stresses |
| X, Y, Z | yield stresses in tension in the radial, circumferential and axial directions |
| F, G, H | Hill’s coefficients |
| , | Lankford’s coefficients in the radial and circumferential directions |
| , | average yield stress in tension and Lankford’s coefficient |
| , | Lankford’s coefficient and yield stress in tension in the direction |
| n | number of directions in which the Lankford’s coefficients and yield stresses are measured |
| , , , , , , | normal strains (the superscript e denotes the elastic portion of the strain components, the superscript p denotes the plastic portion of the strain components) |
| , , | normal plastic strain rates |
| E | Young’s modulus |
| Poisson’s ratio | |
| , | non-negative multipliers in Equations (10) and (11) |
| , , , , | dimensionless quantities introduced in Equation (13) |
| , | parameters introduced in Equation (14) |
| , | auxiliary variables |
| , | radius of the elastic/plastic boundary and its dimensionless representation |
| , | values of and at |
| parameter introduced in Equation (15) | |
| , | parameters introduced in Equation (17) |
| , | parameters introduced in Equation (18) |
| , | stress increments at the end of unloading |
| , | stresses at the end of loading |
| , | residual stresses |
| , | integration variables |
| , , | strain increments at the end of unloading |
| , , | strains at the end of loading |
| , , | residual strains |
References
- Rees, D. Basic Engineering Plasticity; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar] [CrossRef]
- Banabic, D. Sheet Metal Forming Processes: Constitutive Modeling and Numerical Simulation; Springer: Heidelberg, Germany, 2010. [Google Scholar] [CrossRef]
- Wagoner, R.; Li, M. Simulation of springback: Through-thickness integration. Int. J. Plast. 2007, 23, 345–360. [Google Scholar] [CrossRef]
- Prime, M.B. Amplified effect of mild plastic anisotropy on residual stress and strain anisotropy. Int. J. Solids Struct. 2017, 70–77. [Google Scholar] [CrossRef]
- Essa, S.; Argeso, H. Elastic analysis of variable profile and polar orthotropic FGM rotating disks for a variation function with three parameters. Acta Mech. 2017, 228, 3877–3899. [Google Scholar] [CrossRef]
- Yıldırım, V. Numerical/analytical solutions to the elastic response of arbitrarily functionally graded polar orthotropic rotating discs. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 320. [Google Scholar] [CrossRef]
- Jeong, W.; Alexandrov, S.; Lang, L. Effect of plastic anisotropy on the distribution of residual stresses and strains in rotating annular disks. Symmetry 2018, 10, 420. [Google Scholar] [CrossRef]
- Wang, C.; Kinzel, G.; Altan, T. Mathematical modeling of plane-strain bending of sheet and plate. J. Mater. Process. Technol. 1993, 39, 279–304. [Google Scholar] [CrossRef]
- Pourboghrat, F.; Chung, K.; Richmond, O. A hybrid membrane/shell method for rapid estimation of Springback in anisotropic sheet metals. J. Appl. Mech. 1998, 65, 671–684. [Google Scholar] [CrossRef]
- Chakrabarty, J.; Bin Lee, R.; Chan, K. An exact solution for the elastic/plastic bending of anisotropic sheet metal under conditions of plane strain. Int. J. Mech. Sci. 2001, 43, 1871–1880. [Google Scholar] [CrossRef]
- Leu, D.-K. Relationship between mechanical properties and geometric parameters to limitation condition of springback based on springback–radius concept in V-die bending process. Int. J. Adv. Manuf. Technol. 2018, 101, 913–926. [Google Scholar] [CrossRef]
- Salimi, M.; Jamshidian, M.; Beheshti, A.; Sadeghi Dolatabadi, A. Bending-unbending analysis of anisotropic sheet under plane strain condition. J. Adv. Mat. Eng. 2008, 26, 77–86. [Google Scholar]
- Li, D.; Luo, Y.; Peng, Y.; Hu, P. The numerical and analytical study on stretch flanging of V-shaped sheet metal. J. Mater. Process. Technol. 2007, 189, 262–267. [Google Scholar] [CrossRef]
- Paul, S.K. Non-linear correlation between uniaxial tensile properties and shear-edge hole expansion ratio. J. Mater. Eng. Perform. 2014, 23, 3610–3619. [Google Scholar] [CrossRef]
- Kim, J.H.; Kwon, Y.J.; Lee, T.; Lee, K.-A.; Kim, H.S.; Lee, C.S. Prediction of hole expansion ratio for various steel sheets based on uniaxial tensile properties. Met. Mater. Int. 2018, 24, 187–194. [Google Scholar] [CrossRef]
- Marciniak, Z.; Kuczyński, K. Limit strains in the processes of stretch-forming sheet metal. Int. J. Mech. Sci. 1967, 9, 609–620. [Google Scholar] [CrossRef]
- Bressan, J.; Williams, J. The use of a shear instability criterion to predict local necking in sheet metal deformation. Int. J. Mech. Sci. 1983, 25, 155–168. [Google Scholar] [CrossRef]
- Bressan, J.D.; Wang, Q.; Simonetto, E.; Ghiotti, A.; Bruschi, S. Formability prediction of Ti6Al4V titanium alloy sheet deformed at room temperature and 600 °C. Int. J. Mater. Form. 2020, 1–15. [Google Scholar] [CrossRef]
- Liao, K.-C.; Pan, J.; Tang, S. Approximate yield criteria for anisotropic porous ductile sheet metals. Mech. Mater. 1997, 26, 213–226. [Google Scholar] [CrossRef]
- Huang, H.-M.; Pan, J.; Tang, S. Failure prediction in anisotropic sheet metals under forming operations with consideration of rotating principal stretch directions. Int. J. Plast. 2000, 16, 611–633. [Google Scholar] [CrossRef]
- Chien, W.Y.; Pan, J.; Tang, S.C. Modified anisotropic Gurson yield criterion for porous ductile sheet metals. J. Eng. Mater. Technol. 2000, 123, 409–416. [Google Scholar] [CrossRef]
- Harikrishna, C.; Davidson, M.J.; Nagaraju, C.; RatnaPrasad, A.V. Effect of lubrication and anisotropy on hardness in the upsetting test. Trans. Indian Inst. Met. 2015, 69, 1449–1457. [Google Scholar] [CrossRef]
- Yang, X.-Y.; Yang, C.; Liang, F.; Zhang, W.-X.; Niu, Y. Influence of normal anisotropy coefficient on no-rivet connection quality. Suxing Gongcheng Xuebao/J. Plast. Eng. 2019, 26, 245–250. [Google Scholar] [CrossRef]
- Uscinowicz, R. Characterization of directional Elastoplastic properties of Al/Cu bimetallic sheet. J. Mater. Eng. Perform. 2019, 28, 1350–1359. [Google Scholar] [CrossRef]
- Ning, B.; Zhao, Z.; Mo, Z.; Wu, H.; Peng, C.; Gong, H. Influence of continuous annealing temperature on mechanical properties and texture of battery shell steel. Metals 2019, 10, 52. [Google Scholar] [CrossRef]
- Moura, A.N.; Ferreira, J.L.; Martins, J.B.R.; De Souza, M.V.; Castro, N.A.; Orlando, M.T.D. Microstructure, crystallographic texture, and stretch-Flangeability of hot-rolled multiphase steel. Steel Res. Int. 2020, 91. [Google Scholar] [CrossRef]
- Lequeu, P.; Jonas, J.J. Modeling of the plastic anisotropy of textured sheet. Met. Mater. Trans. A 1988, 19, 105–120. [Google Scholar] [CrossRef]
- Wang, X.; Cao, L.; Peng, X.; Zhang, J.; Zhuang, L.; Guo, M. Relationship among mechanical properties anisotropy, microstructure and texture in AA 6111 alloy sheets. J. Wuhan Univ. Technol. Sci. Ed. 2016, 31, 648–653. [Google Scholar] [CrossRef]
- Inoue, H.; Takasugi, T. Texture control for improving deep Drawability in rolled and annealed aluminum alloy sheets. Mater. Trans. 2007, 48, 2014–2022. [Google Scholar] [CrossRef]
- Serebryany, V.N.; Pozdnyakova, N.N. Evaluation of the normal anisotropy coefficient in AZ31 alloy sheets. Russ. Met. (Metally) 2009, 2009, 58–64. [Google Scholar] [CrossRef]
- Grechnikov, F.V.; Erisov, Y.A.; Alexandrov, S.E. Effect of the anisotropic yield condition on the predicted distribution of residual stresses in a thin disk. Dokl. Phys. 2019, 64, 233–237. [Google Scholar] [CrossRef]
- Cohen, T.; Masri, R.; Durban, D. Analysis of circular hole expansion with generalized yield criteria. Int. J. Solids Struct. 2009, 46, 3643–3650. [Google Scholar] [CrossRef]
- Hill, R. The Mathematical Theory of Plasticity; Oxford University Press: New York, NY, USA, 1950. [Google Scholar]
- Watson, M.; Dick, R.; Huang, Y.H.; Lockley, A.; Cardoso, R.; Santos, A. Benchmark 1—Failure prediction after cup drawing, reverse redrawing and expansion part A: Benchmark description. J. Phys. Conf. Ser. 2016, 734, 022001. [Google Scholar] [CrossRef]
- Dixit, U.S.; Kamal, S.M.; Shufen, R. Autofrettage Processes: Technology and Modelling; CRC Press: Boka Raton, FL, USA, 2019. [Google Scholar]
| Hill’s Yield Criterion (Equation (3)) | Normal Anisotropy (Equation (6)) | ||||
|---|---|---|---|---|---|
| Case (i) | Case (ii) | n = 2 | n = 3 | n = 7 | |
| X, MPa (R0) | 198 (0.53) | 279 (2.27) | 238 (1.40) | 226 (1.25) | 225 (1.11) |
| Y, MPa (R90) | 279 (2.27) | 198 (0.53) | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Erisov, Y.; Surudin, S.; Alexandrov, S.; Lang, L. Influence of the Replacement of the Actual Plastic Orthotropy with Various Approximations of Normal Anisotropy on Residual Stresses and Strains in a Thin Disk Subjected to External Pressure. Symmetry 2020, 12, 1834. https://doi.org/10.3390/sym12111834
Erisov Y, Surudin S, Alexandrov S, Lang L. Influence of the Replacement of the Actual Plastic Orthotropy with Various Approximations of Normal Anisotropy on Residual Stresses and Strains in a Thin Disk Subjected to External Pressure. Symmetry. 2020; 12(11):1834. https://doi.org/10.3390/sym12111834
Chicago/Turabian StyleErisov, Yaroslav, Sergei Surudin, Sergei Alexandrov, and Lihui Lang. 2020. "Influence of the Replacement of the Actual Plastic Orthotropy with Various Approximations of Normal Anisotropy on Residual Stresses and Strains in a Thin Disk Subjected to External Pressure" Symmetry 12, no. 11: 1834. https://doi.org/10.3390/sym12111834
APA StyleErisov, Y., Surudin, S., Alexandrov, S., & Lang, L. (2020). Influence of the Replacement of the Actual Plastic Orthotropy with Various Approximations of Normal Anisotropy on Residual Stresses and Strains in a Thin Disk Subjected to External Pressure. Symmetry, 12(11), 1834. https://doi.org/10.3390/sym12111834







