Thermo-Mechanical Performance of a Phase Change Energy Pile in Saturated Sand
Abstract
Featured Application
Abstract
1. Introduction
2. Brief Description of Experiments
2.1. PC Pile Development and Experiment Conditions
2.2. Loading Condition
3. Numerical Models
3.1. Basic Assumptions
- Since the vertical static load imposed on the pile head was small and the rigidity of the pile itself was relatively large, the load on pile head would not cause plastic damage, so an ideal linear elastic material was used to approximately model the pile.
- As the temperature change range was not large, the change of material parameters, such as the thermal conductivity, specific heat, and elastic modulus of the pile and soil, were not considered during the thermal cycles and loading.
- During the heat transfer process of the energy pile, the temperature change in the longitudinal direction was much smaller than the temperature change in the radial direction. Therefore, the heat transfer of the energy pile in the longitudinal direction was negligible.
3.2. Governing Equations
3.2.1. The Governing Equation of Heat Conduction
3.2.2. The Governing Equation of Heat Convection
3.3. Constitutive Models
3.3.1. Thermo-Elastic Model of the Pile
3.3.2. Mohr–Coulomb Model of Soil
3.4. Finite Element Model
3.5. Mechanical Model
- (1)
- The elastic modulus of the pile (E = 3.25 × 104 MPa) and Poisson’s ratio of soil (), and the friction angle of the soil were input into the material properties module. In addition, the permeability coefficient of saturated soil was input as cm/s, whereas the specific gravity of the interstitial fluid was set to 10 kN/m3.
- (2)
- The heat exchange tube model and the pile body model were set as binding constraints, the interface between the energy pile and soil were simulated as a frictional contact in the tangential direction. The coefficient of friction (μ) between soil and concrete pile was considered as tanϕ, where ϕ is the internal friction angle of the sand used in the experiments.
- (3)
- The pile and soil were first subject to gravity force, and then a static load of 6.5 kN was applied to the pile head. The surrounding and bottom of the soil were set as a completely fixed boundary, and the top surface was a free boundary with a pore pressure set to 0 in the predefined field, which means that the top surface was the drainage boundary. Finally, the calculation results of the temperature model were substituted into the stress model for the thermal and mechanical coupling.
4. Results and Discussions
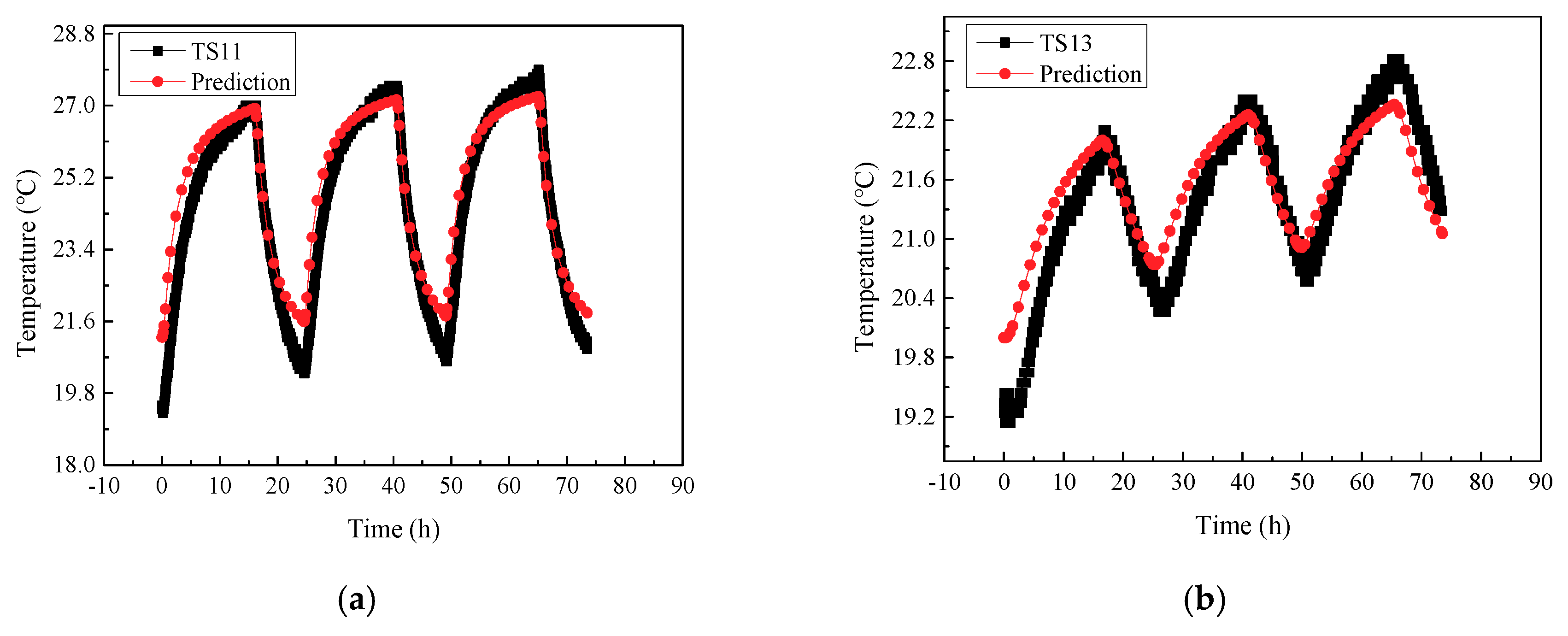
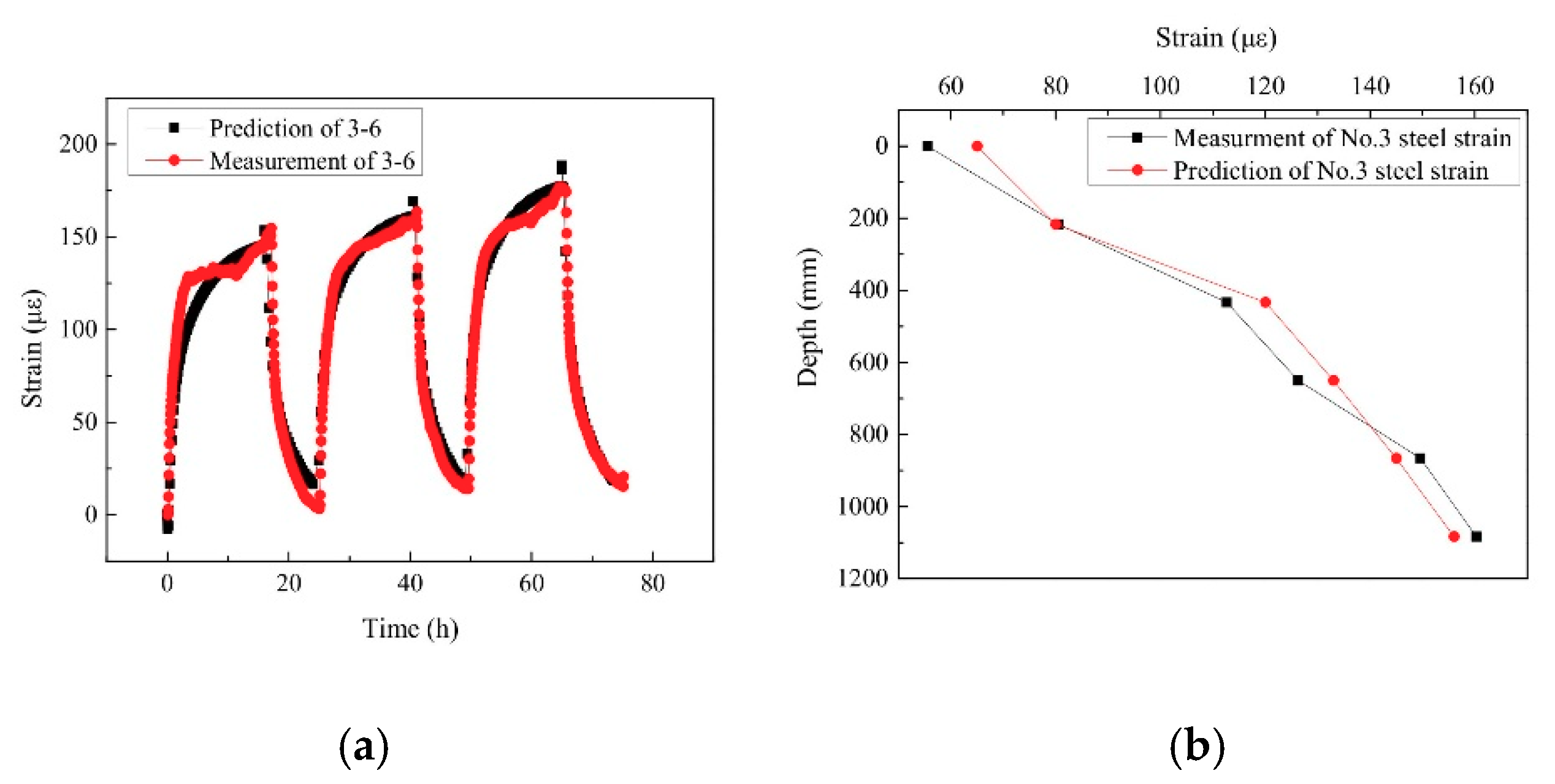
4.1. Validation of Numerical Model
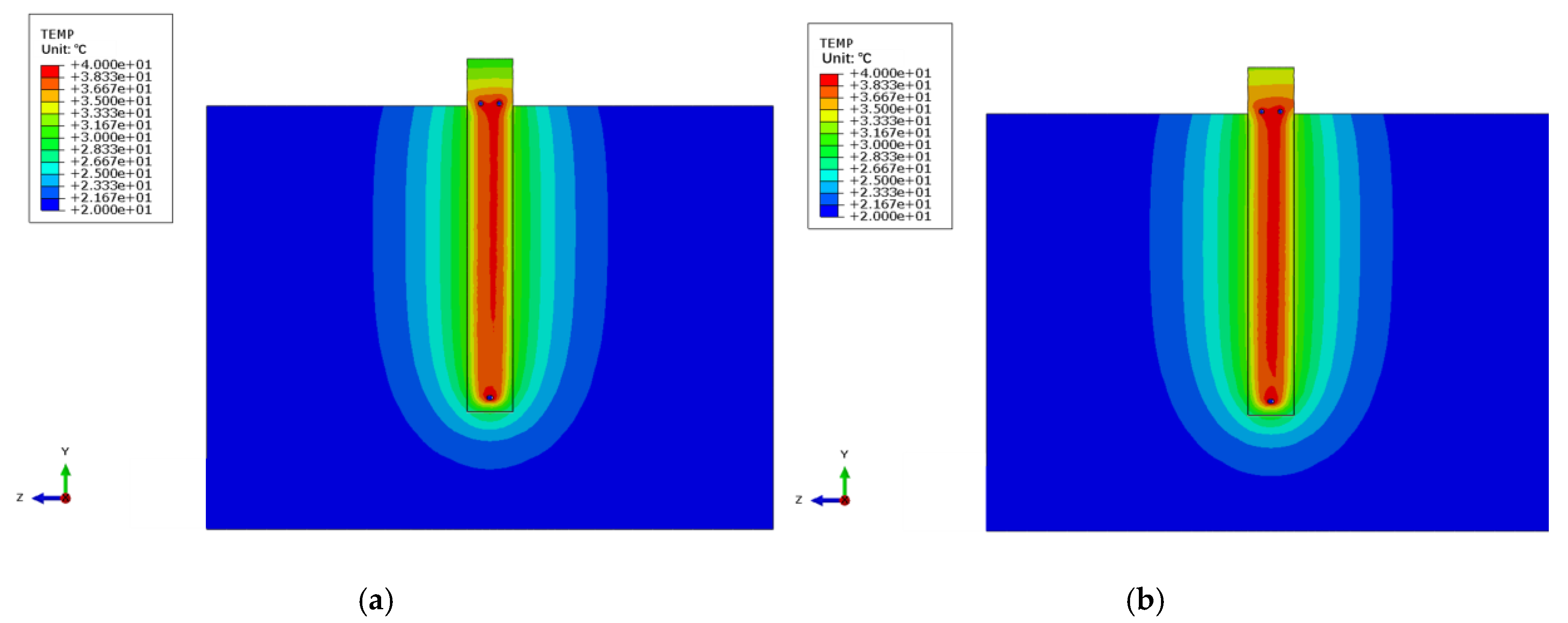
4.1.1. Temperature Change in Soil
4.1.2. Strain Change in the Pile
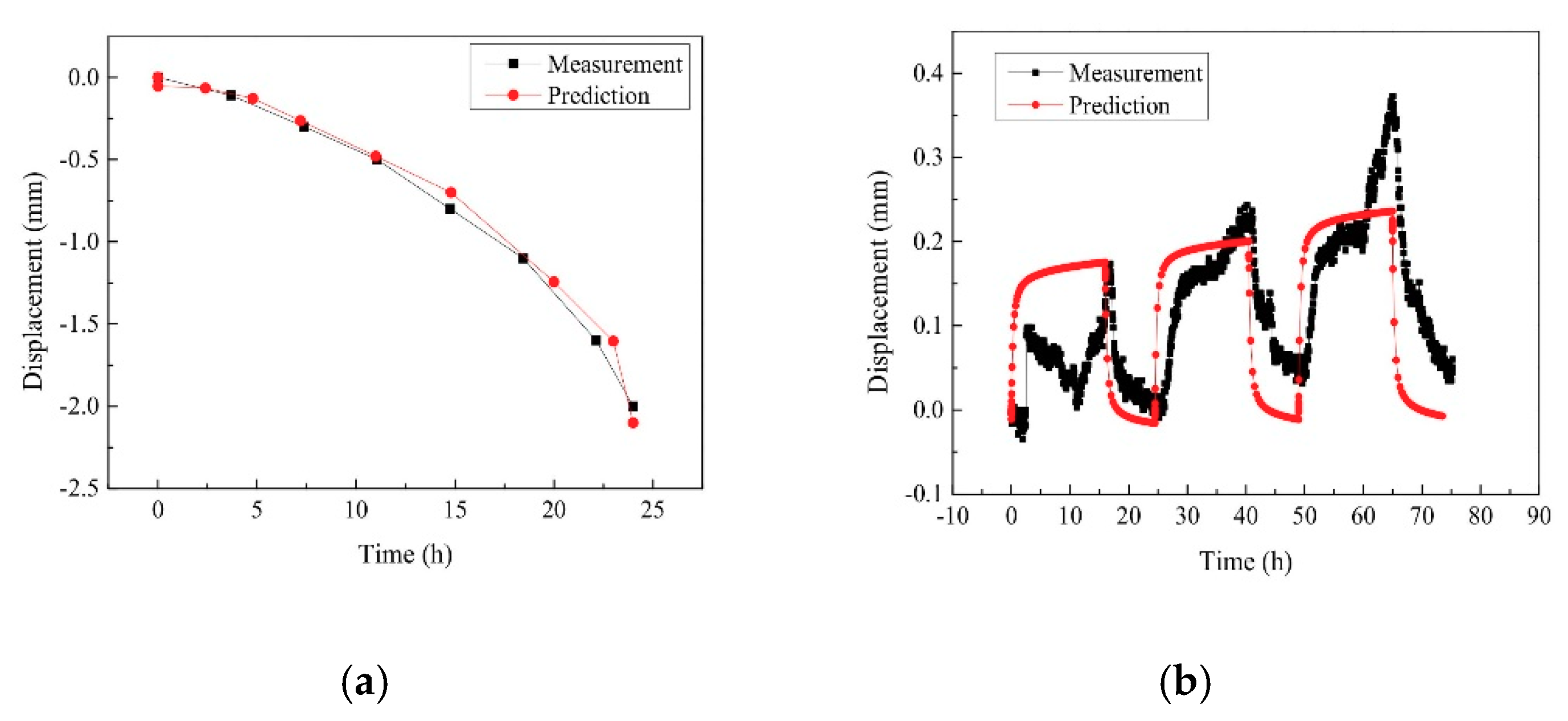
4.1.3. Pile Displacement
4.1.4. Soil Pressure around Pile
4.2. Comparison between PC pile and Ordinary Pile
4.2.1. Temperature Change of Pile
4.2.2. Strain in Pile
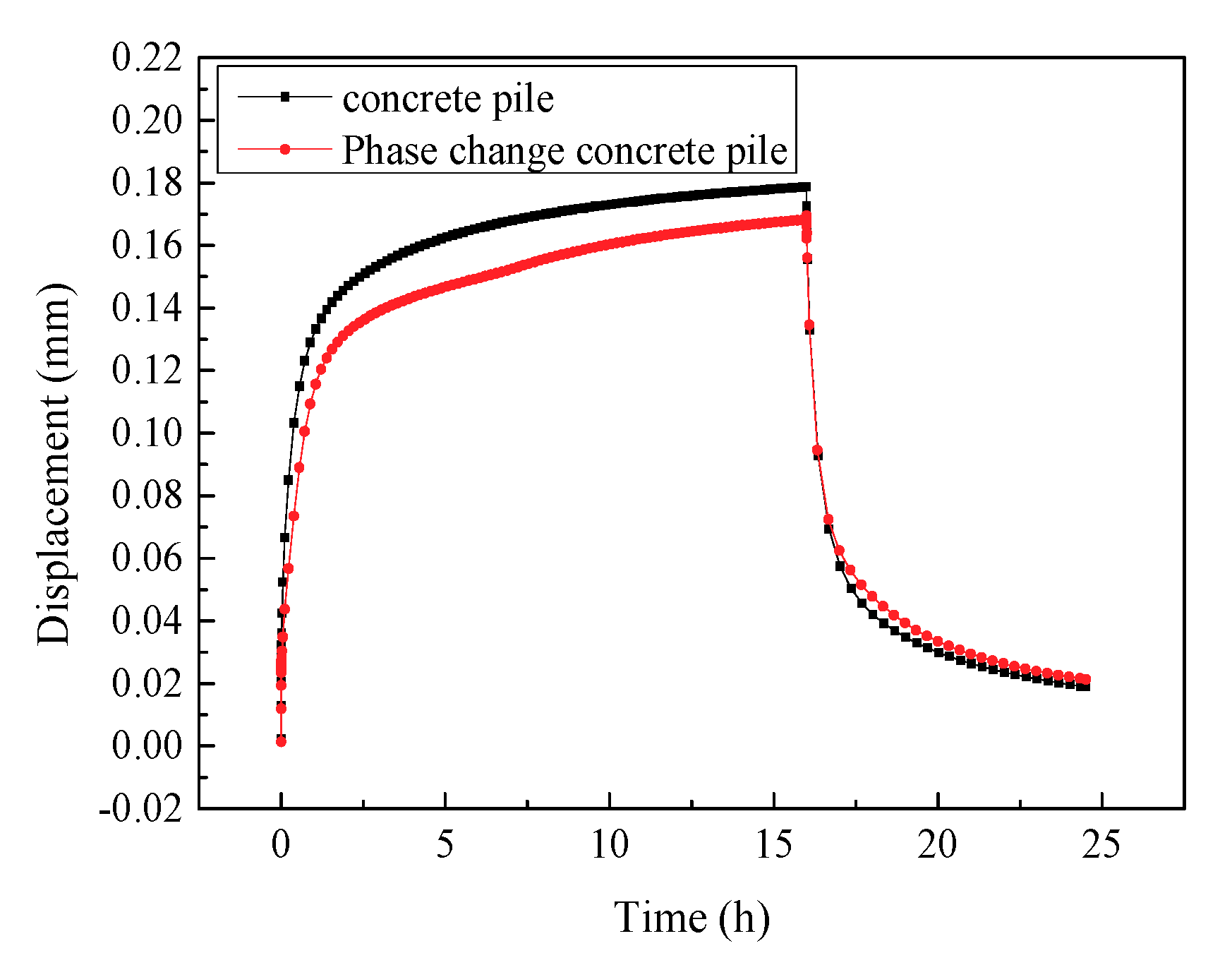
4.2.3. The Displacement of Pile
4.2.4. Soil Pressure around Pile
4.3. Influence of Thermal Loads on PC Piles
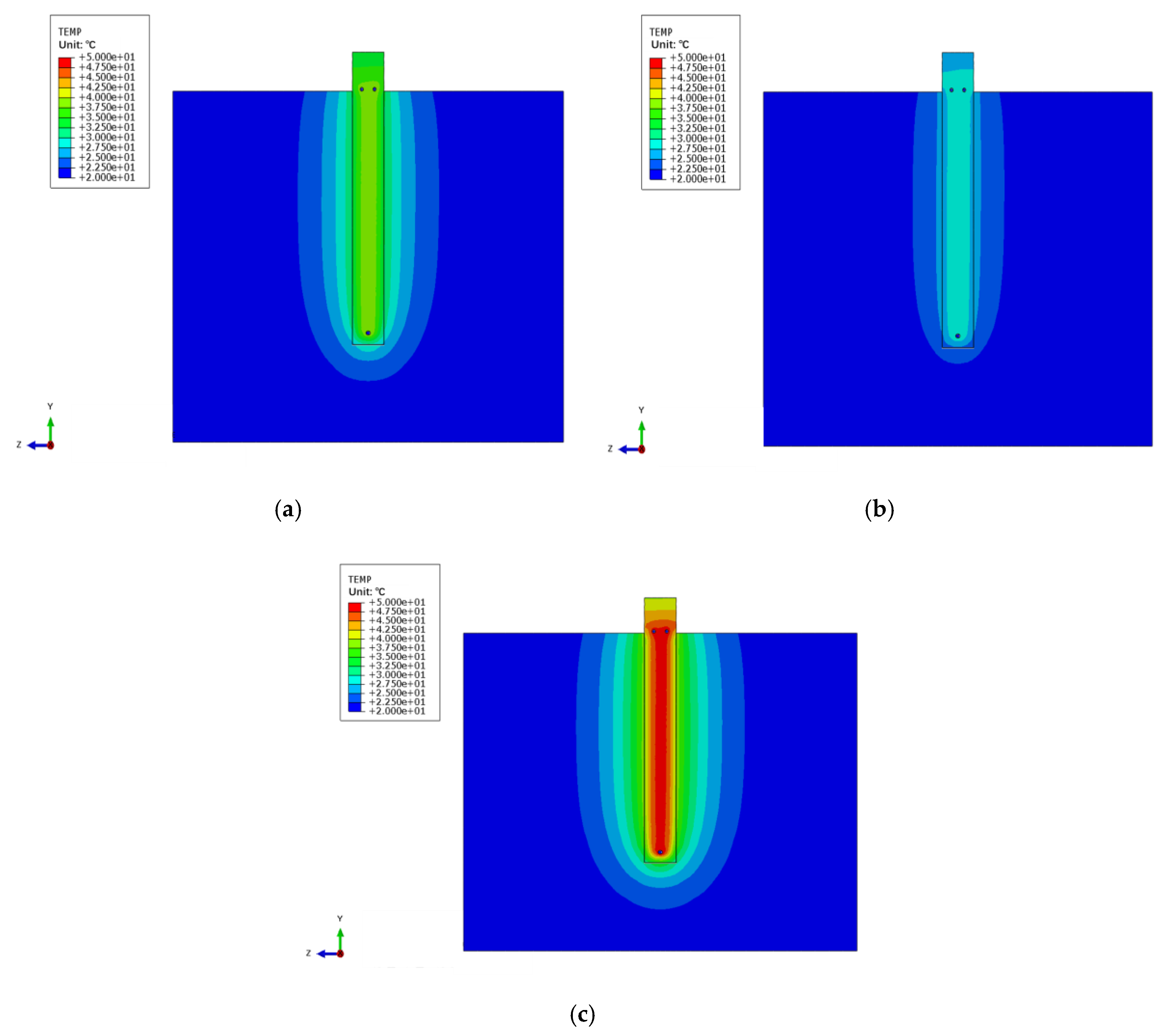
4.3.1. Temperature Change
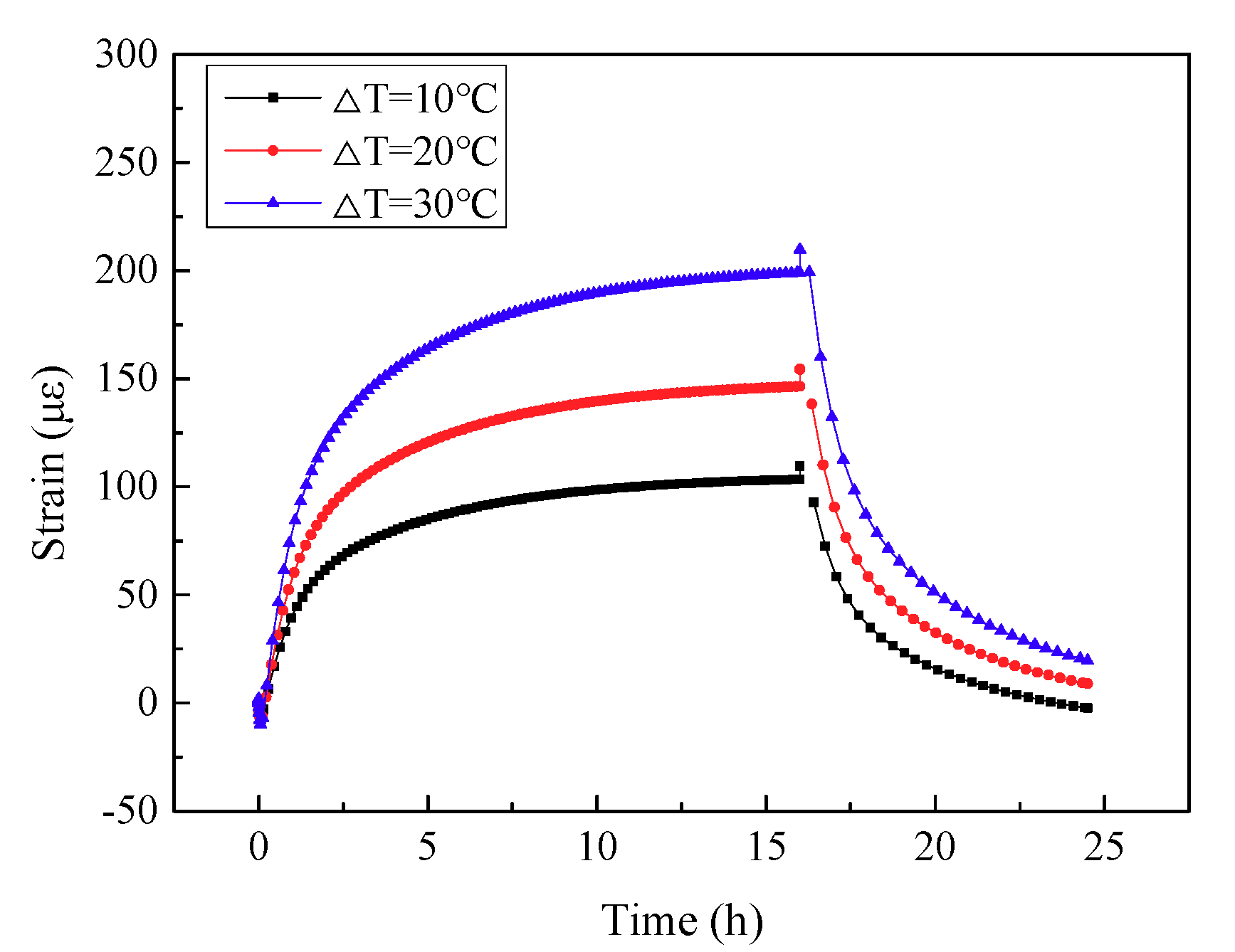
4.3.2. Strain Change in PC Pile
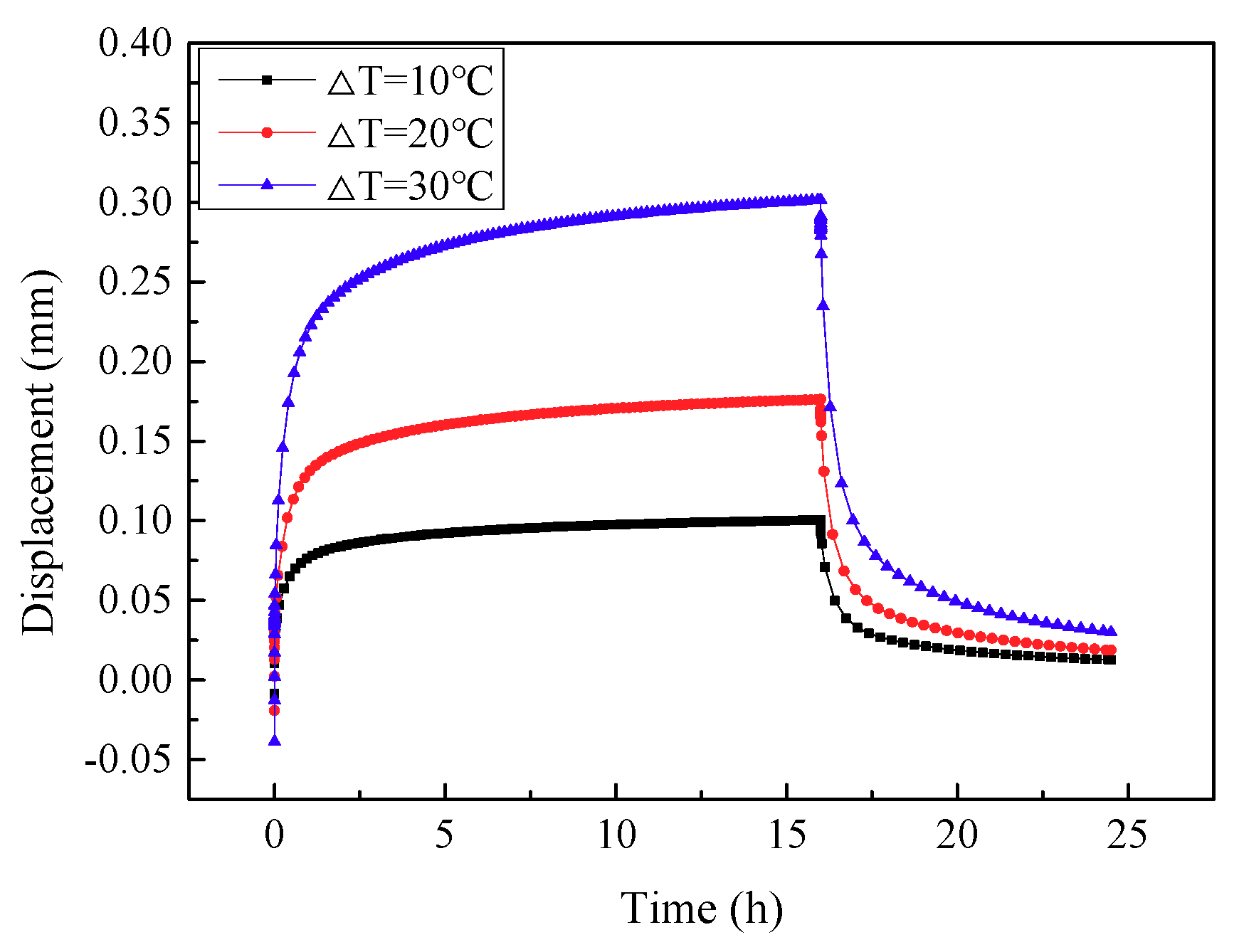
4.3.3. Displacement on Pile Head
5. Conclusions
- (1)
- Phase change materials can be used in energy piles to reduce the temperature change of the pile to a certain extent. At the end of heating, the temperature of the PC pile was 0.6 °C lower than that of ordinary energy pile without phase change materials. When the temperature dropped below 22.5 °C, the temperature decreased more slowly than the ordinary pile due to the exothermic phase change of paraffin, and the range of soil temperature change around the pile reduced by 0.03 m compared to the ordinary pile.
- (2)
- At the end of heating, the strain at the measuring point of the phase change energy pile was 2% smaller than that of the ordinary energy pile, and the displacement was reduced by 6%, which shows that the addition of phase change materials can reduce the thermal response of the energy pile compared with ordinary pile. Moreover, the stress distribution in the pile can be reduced and consequently the durability of the pile can be improved. After the thermal cycles, the residual deformation was basically the same.
- (3)
- The difference in the soil pressure change around the PC pile at the end of heating was small, indicating that the addition of phase change materials has little effect on the soil pressure around the pile.
- (4)
- When the thermal load increased from 10 °C to 30 °C, the exchange range between pile and soil increased from 0.2 m to 0.45 m, and the growth rate decreased with the increase in thermal loading. The pile strain showed a nonlinear increasing trend with the increase of thermal loading. Meanwhile, the observed strain and axial stress of the pile increased with the increase of the depth.
- (5)
- In the heating stage, the increment of the displacement at the head of the PC pile increased with the increase of the thermal loading. After the cooling stage, the residual strain and plastic displacement also increased with the increase of the thermal loading. At the loading condition of = 10 °C, the phase change material had the greatest influence on the displacement and residual strain of the pile. With the increase of the thermal loading, its influence became less and less obvious. Therefore, in the design of phase change energy piles, full consideration should be given to the compatibility of the thermal loads and the phase-change temperature of the material. While phase-change material is of great potential for use in controlling the thermal response of energy piles, more attention should be paid to balance the heat transfer rate and the thermal response of the pile.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Adinolfi, M.; Loria, A.F.R.; Laloui, L.; Aversa, S. Experimental and numerical investigation of the thermo-mechanical behaviour of an energy sheet pile wall. Geomech. Energy Environ. 2020, 25, 100208. [Google Scholar]
- Brandl, H. Energy foundations and other thermo-active ground structures. Géotechnique 2006, 56, 81–122. [Google Scholar] [CrossRef]
- You, S.; Cheng, X.; Guo, H.; Yao, Z. In-situ experimental study of heat exchange capacity of CFG pile geothermal exchangers. Energy Build. 2014, 79, 23–31. [Google Scholar] [CrossRef]
- Hamada, Y.; Saitoh, H.; Nakamura, M.; Kubota, H.; Ochifuji, K. Field performance of an energy pile system for space heating. Energy Build. 2007, 39, 517–524. [Google Scholar] [CrossRef]
- Kramer, C.A.; Ghasemi-Fare, O.; Basu, P. Laboratory Thermal Performance Tests on a Model Heat Exchanger Pile in Sand. Geotech. Geol. Eng. 2014, 33, 253–271. [Google Scholar] [CrossRef]
- Cui, P.; Li, X.; Man, Y.; Fang, Z. Heat transfer analysis of pile geothermal heat exchangers with spiral coils. Appl. Energy 2011, 88, 4113–4119. [Google Scholar] [CrossRef]
- Cui, Y.; Zhu, J. 3D transient heat transfer numerical analysis of multiple energy piles. Energy Build. 2017, 134, 129–142. [Google Scholar] [CrossRef]
- Luo, J.; Zhao, H.; Gui, S.; Xiang, W.; Rohn, J. Study of thermal migration and induced mechanical effects in double U-tube energy piles. Comput. Geotech. 2017, 91, 1–11. [Google Scholar] [CrossRef]
- Laloui, L.; Nuth, M.; Vulliet, L. Experimental and numerical investigations of the behaviour of a heat exchanger pile. Int. J. Numer. Anal. Methods Geomech. 2006, 30, 763–781. [Google Scholar] [CrossRef]
- Bourne-Webb, P.; Freitas, T.B. Thermally-activated piles and pile groups under monotonic and cyclic thermal loading—A review. Renew. Energy 2020, 147, 2572–2581. [Google Scholar] [CrossRef]
- Marto, A.; Amaludin, A. Response of shallow geothermal energy pile from laboratory model tests. IOP Conf. Ser. Earth Environ. Sci. 2015, 26, 012038. [Google Scholar] [CrossRef]
- Ng, C.W.W.; Gunawan, A.; Shi, C.; Ma, Q.; Liu, H.L. Centrifuge modelling of displacement and replacement energy piles constructed in saturated sand: A comparative study. Géotech. Lett. 2016, 6, 34–38. [Google Scholar] [CrossRef]
- Ng, C.W.W.; Shi, C.; Gunawan, A.; Laloui, L. Centrifuge modelling of energy piles subjected to heating and cooling cycles in clay. Géotech. Lett. 2014, 4, 310–316. [Google Scholar] [CrossRef]
- Kalantidou, A.; Tang, A.M.; Pereira, J.M.; Hassen, G. Preliminary study on the mechanical behaviour of heat exchanger pile in physical model. Géotechnique 2012, 62, 1047–1051. [Google Scholar] [CrossRef]
- Yavari, N.; Tang, A.M.; Pereira, J.M.; Hassen, G. Mechanical behaviour of a small-scale energy pile in saturated clay. Géotechnique 2016, 66, 878–887. [Google Scholar] [CrossRef]
- Yavari, N.; Tang, A.M.; Pereira, J.-M.; Hassen, G. Experimental study on the mechanical behaviour of a heat exchanger pile using physical modelling. Acta Geotech. 2014, 9, 385–398. [Google Scholar] [CrossRef]
- Nguyen, V.T.; Tang, A.M.; Pereira, J.M. Long-term thermo-mechanical behavior of energy pile in dry sand. Acta Geotech. 2017, 12, 729–737. [Google Scholar] [CrossRef]
- You, S.; Cheng, X.; Guo, H.; Yao, Z. Experimental study on structural response of CFG energy piles. Appl. Eng. 2016, 96, 640–651. [Google Scholar] [CrossRef]
- Man, Y.; Yang, H.; Diao, N.; Cui, P.; Lu, L.; Fang, Z.J.H. Development of spiral heat source model for novel pile ground heat exchangers. Hvac R Res. 2011, 17, 1075–1088. [Google Scholar]
- Man, Y.; Yang, H.; Diao, N.; Liu, J.; Fang, Z. A new model and analytical solutions for borehole and pile ground heat exchangers. Int. J. Heat Mass Transf. 2010, 53, 2593–2601. [Google Scholar] [CrossRef]
- Ghasemi-Fare, O.; Basu, P. A practical heat transfer model for geothermal piles. Energy Build. 2013, 66, 470–479. [Google Scholar] [CrossRef]
- Hu, P.; Zha, J.; Lei, F.; Zhu, N.; Wu, T. A composite cylindrical model and its application in analysis of thermal response and performance for energy pile. Energy Build. 2014, 84, 324–332. [Google Scholar] [CrossRef]
- Wang, D.; Lu, L.; Cui, P. A novel composite-medium solution for pile geothermal heat exchangers with spiral coils. Int. J. Heat Mass Transf. 2016, 93, 760–769. [Google Scholar] [CrossRef]
- Dubey, A.A.; Kumar, S. Assessment of stress-strain behavior of energy piles installed in sand. Int. J. Geomate 2017, 12, 112–120. [Google Scholar] [CrossRef]
- Yang, W.; Lu, P.; Chen, Y. Laboratory investigations of the thermal performance of an energy pile with spiral coil ground heat exchanger. Energy Build. 2016, 128, 491–502. [Google Scholar] [CrossRef]
- Wang, B.; Bouazza, A.; Haberfield, C. Preliminary Observations from Laboratory Scale Model Geothermal Pile Subjected to Thermal-Mechanical Loading. Geo-Frontiers 2011, 2011, 430–439. [Google Scholar]
- Bao, X.; Li, Y.; Feng, T.; Cui, H.; Chen, X. Investigation on thermo-mechanical behavior of reinforced concrete energy pile with large cross-section in saturated sandy soil by model experiments. Undergr. Space 2019, 5, 229–241. [Google Scholar] [CrossRef]

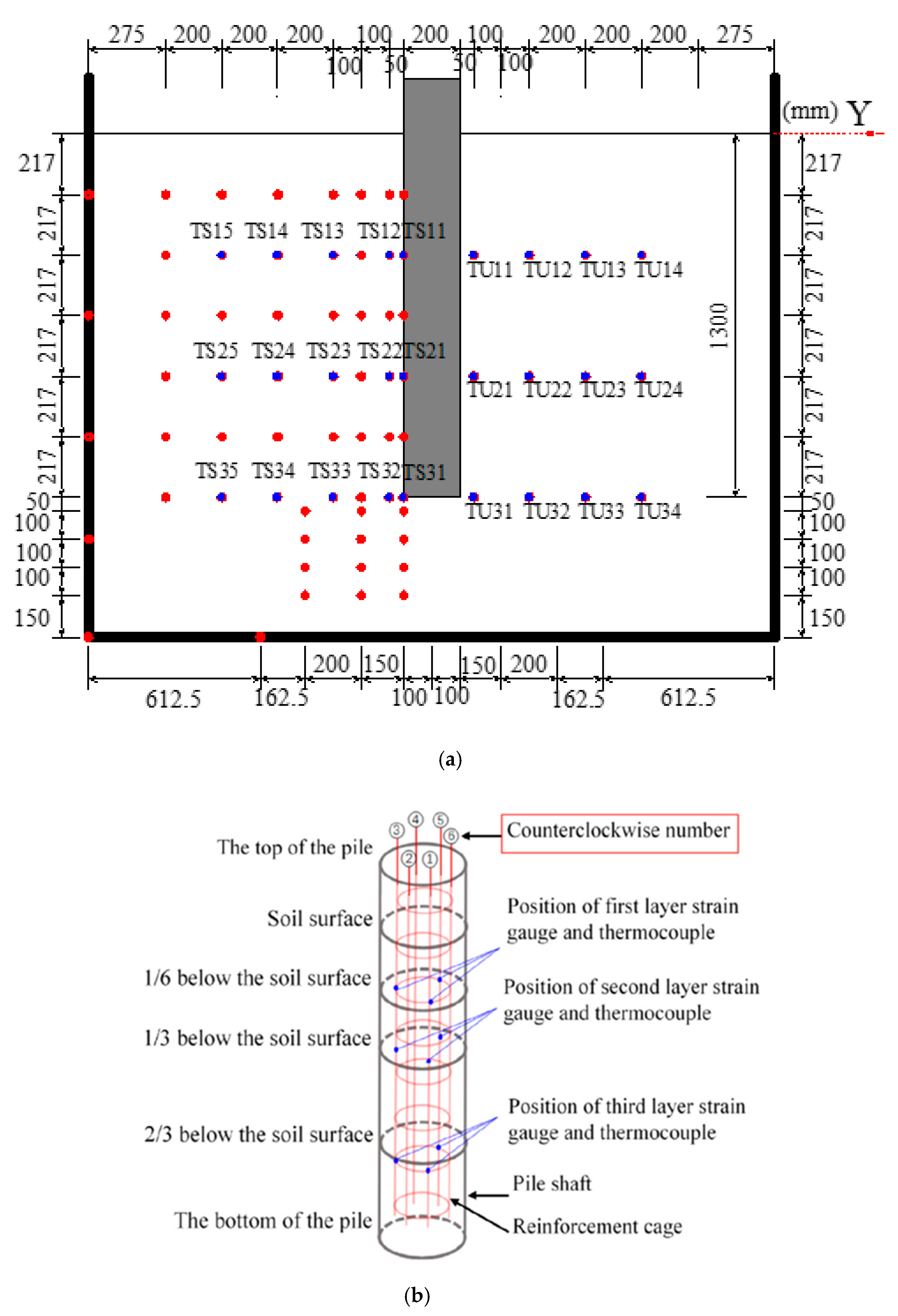
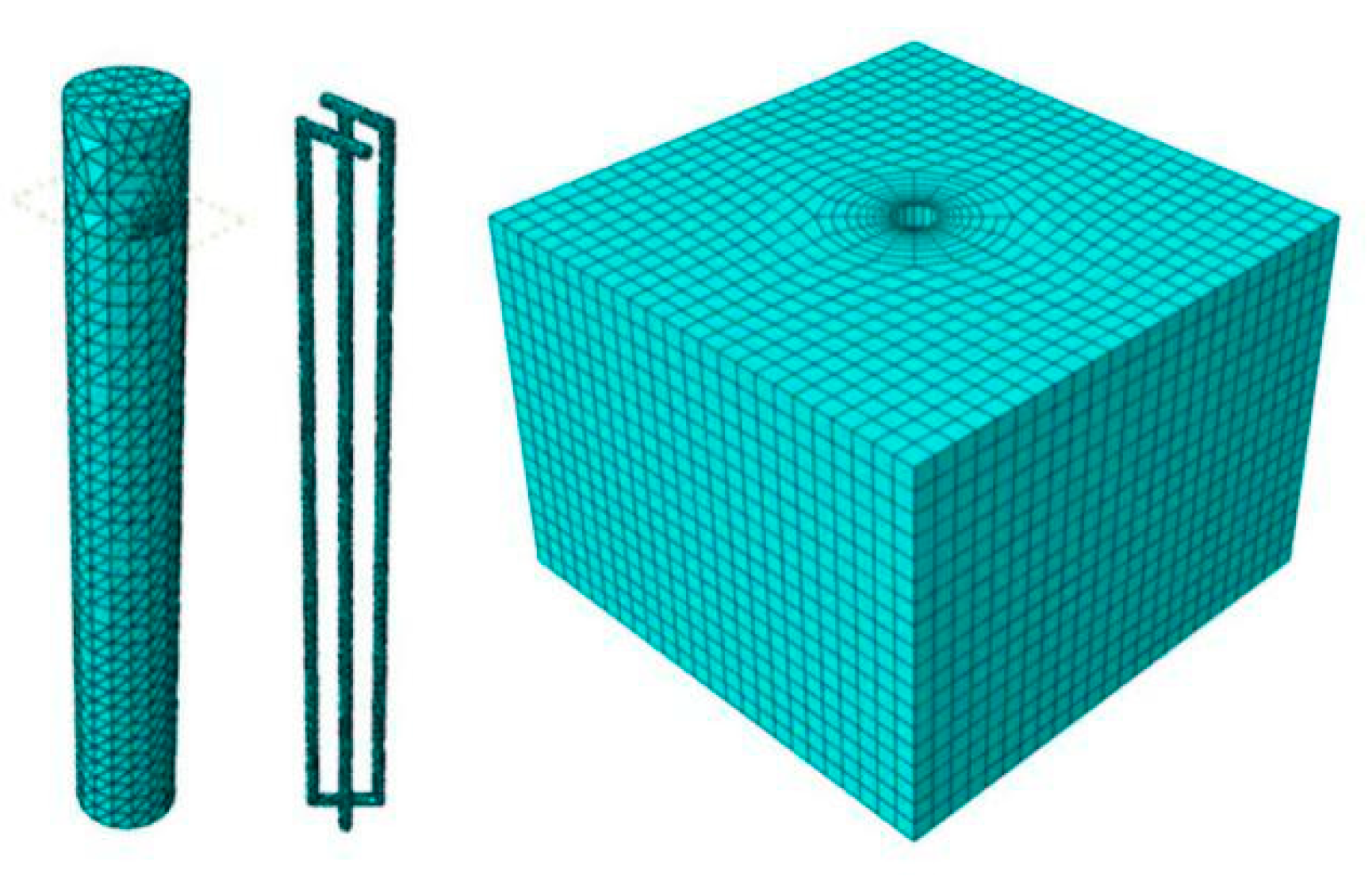





| Sample Material | Thermal Conductivity W/(m·K) | Specific Heat Capacity J/(kg °C) | Coefficient of Thermal Expansion (/°C) | Relative Density |
|---|---|---|---|---|
| saturated sand | 2.7 | 1155 | 1.46 × 10−6 | 42% |
| ordinary concrete | 1.89 | 963 | 0.98 × 10−5 | -- |
| phase change concrete | 1.65 | 1080 | 0.9 × 10−5 | -- |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, T.; Li, Y.; Bao, X.; Tang, W.; Cui, H. Thermo-Mechanical Performance of a Phase Change Energy Pile in Saturated Sand. Symmetry 2020, 12, 1781. https://doi.org/10.3390/sym12111781
Du T, Li Y, Bao X, Tang W, Cui H. Thermo-Mechanical Performance of a Phase Change Energy Pile in Saturated Sand. Symmetry. 2020; 12(11):1781. https://doi.org/10.3390/sym12111781
Chicago/Turabian StyleDu, Ting, Yubo Li, Xiaohua Bao, Waiching Tang, and Hongzhi Cui. 2020. "Thermo-Mechanical Performance of a Phase Change Energy Pile in Saturated Sand" Symmetry 12, no. 11: 1781. https://doi.org/10.3390/sym12111781
APA StyleDu, T., Li, Y., Bao, X., Tang, W., & Cui, H. (2020). Thermo-Mechanical Performance of a Phase Change Energy Pile in Saturated Sand. Symmetry, 12(11), 1781. https://doi.org/10.3390/sym12111781








