Research of RBF-PID Control in Maglev System
Abstract
1. Introduction
2. Maglev Levitation System Modeling
- (1)
- Ignore the leakage magnetic flux of the winding ;
- (2)
- Ignore the magnetic resistance in the magnet core and the rail, that is, the magnetic potential is evenly reduced on the air gap ;
- (3)
- Ignore the magnetic saturation characteristics of the materials of the track and the iron core;
- (4)
- The track is regarded as a rigid track; ignore elastic vibration and dynamic deformation;
- (5)
- The mass distribution of the entire system is uniform, and the module center coincides with the geometric center.
3. RBF-PID Controller
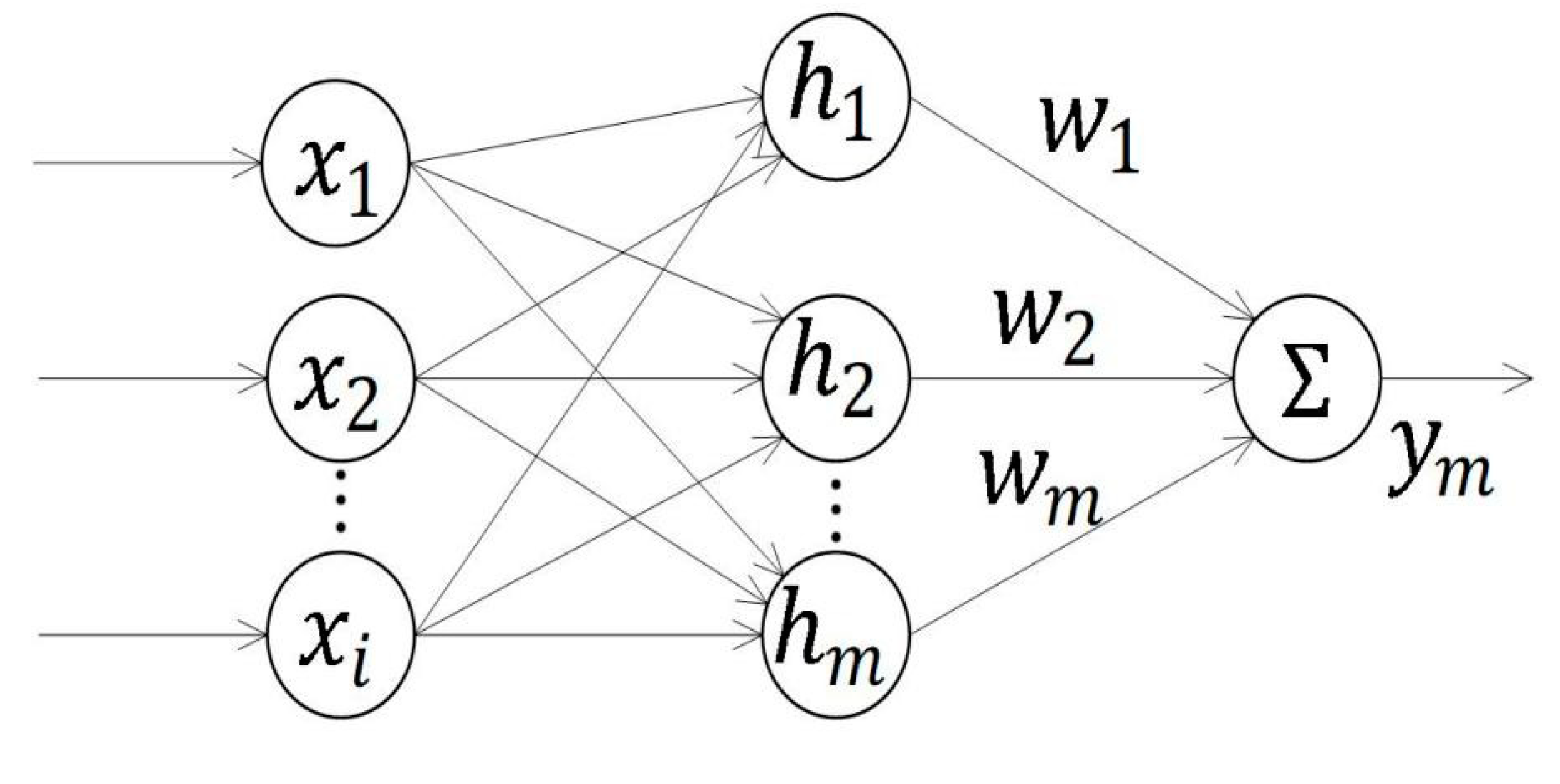
3.1. The Structure of the RBF Network
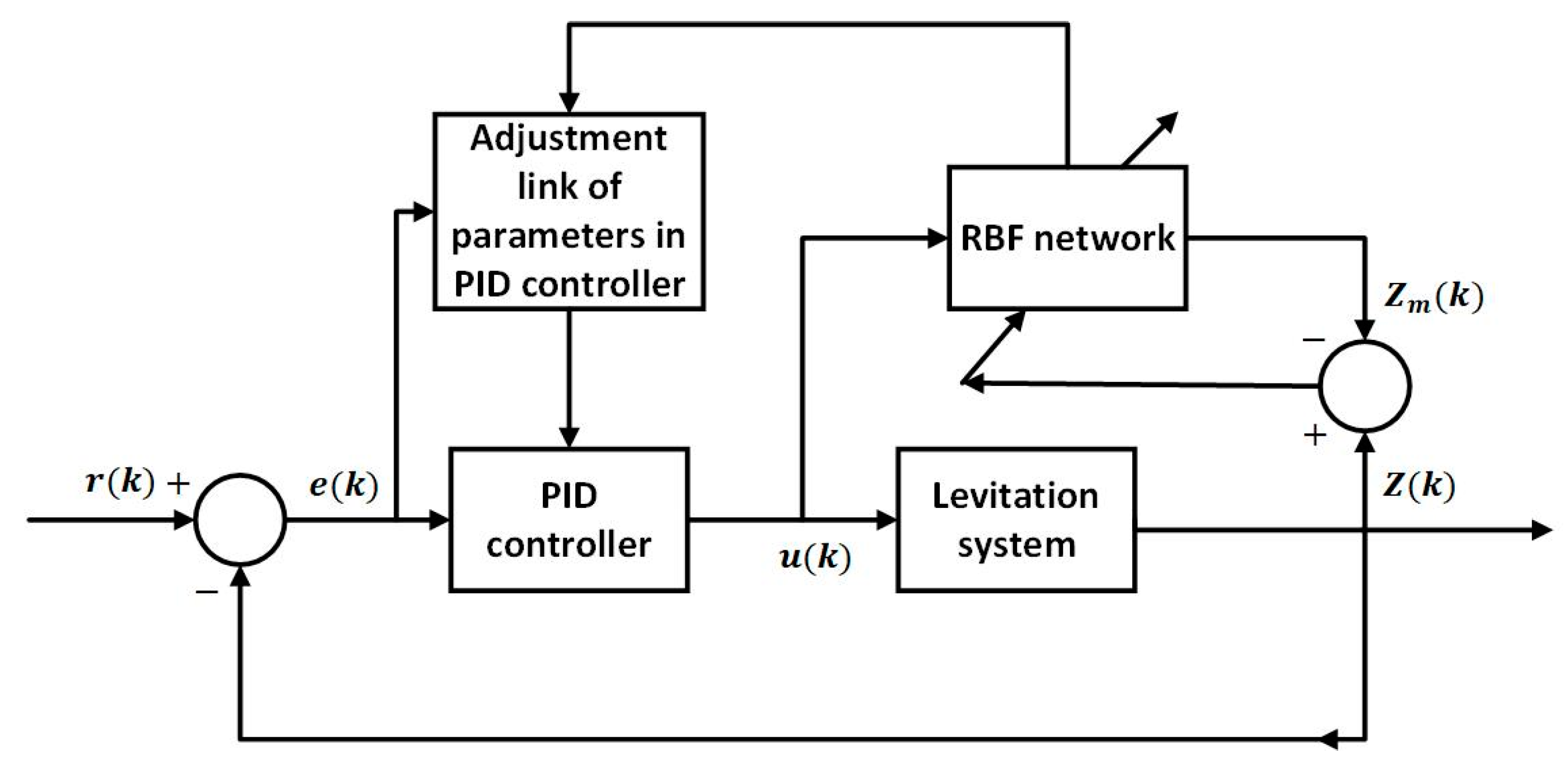
3.2. The Design of the RBF-PID Controller
4. Simulation
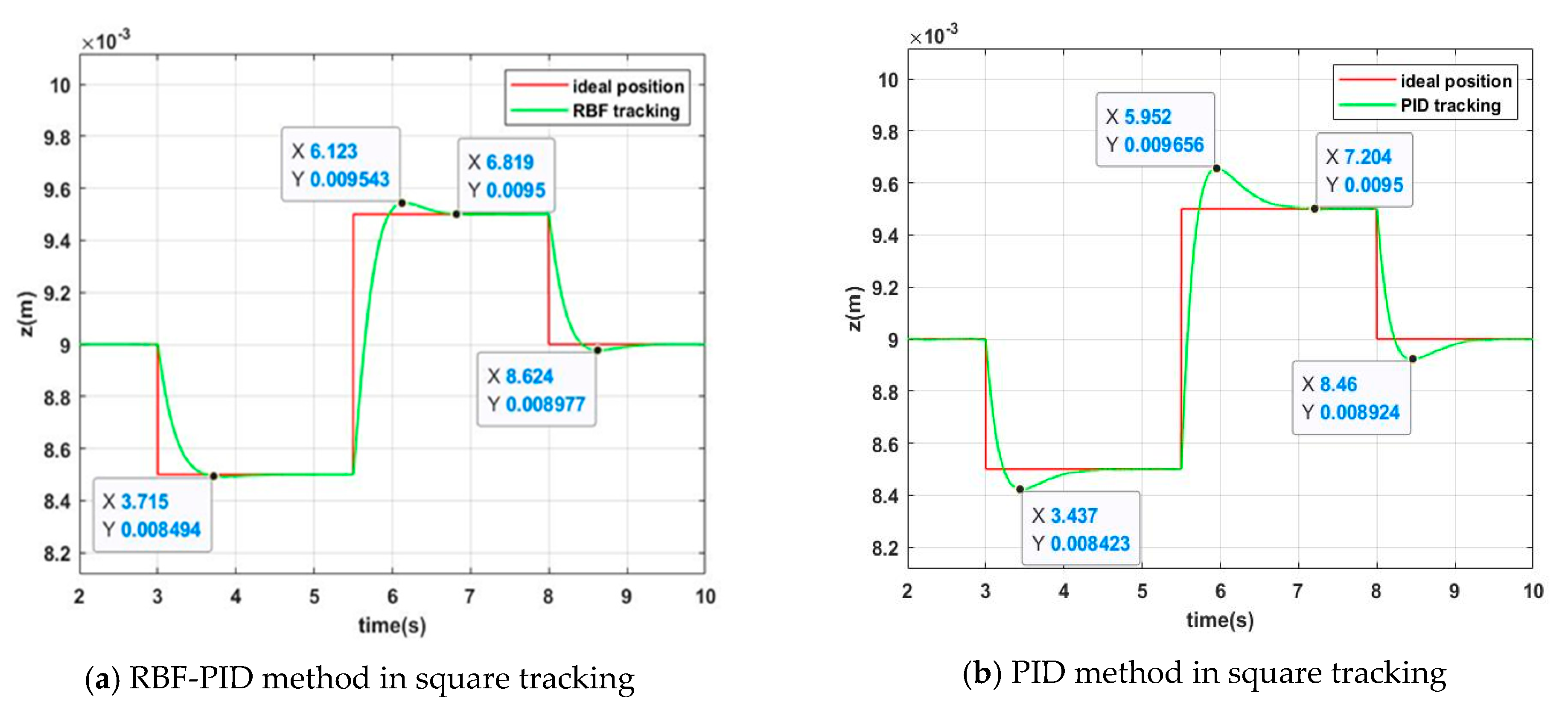
4.1. Simulation of Square Wave Tracking
4.2. Simulation of Load Quality Changing
5. Experiments
5.1. Introduction of the Small Levitation Platform
5.2. Hardware Implementation of RBF Network
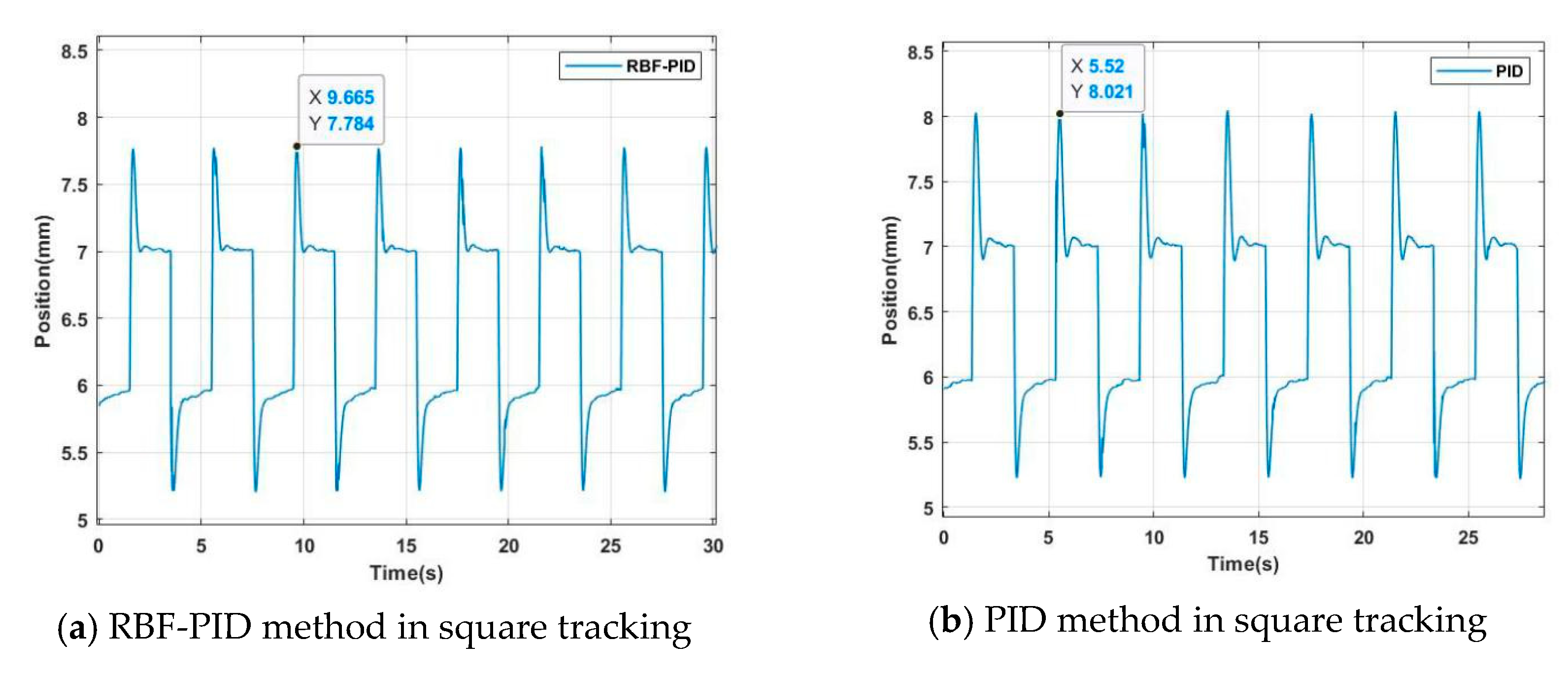
5.3. Experiment of Square Wave Tracking
5.4. Experiment of Load Quality Changing
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Lee, H.W.; Kim, K.C.; Lee, J. Review of maglev train technologies. IEEE Trans. Magn. 2006, 42, 1917–1925. [Google Scholar]
- Hypiusova, M.; Osusky, J. Pid controller design for magnetic levitation model. In Proceedings of the Internation Conference, Cybernetics and Information, Vyšná Boca, Slovakia, 10–13 February 2010. [Google Scholar]
- Ahmad, I.; Shahzad, M.; Palensky, P. Optimal PID control of magnetic levitation system using genetic algorithm. In Proceedings of the 2014 IEEE International Energy Conference (ENERGYCON), Cavtat, Croatia, 13–16 May 2014. [Google Scholar]
- Long, Z.Q.; Hao, A.M.; Chang, W.S. Suspension controller design of maglev train considering the rail track periodical irregularity. J. Natl. Univ. Def. Technol. 2003, 25, 84–89. [Google Scholar]
- Goodall, R.M. Dynamics and control requirements for EMS Maglev suspensions. In Proceedings of the International Conference on Maglev, Shanghai, China, 26–28 October 2004; pp. 926–993. [Google Scholar]
- Liu, J.; Yu, L.U. Adaptive RBF neural network control of robot with actuator nonlinearities. J. Control Theory Appl. 2010, 8, 129–136. [Google Scholar] [CrossRef]
- Junyi, L.; Xiaoming, X. Intelligent control based on neural network. Chem. Ind. Autom. Instrum. 1995, 22, 53–59. [Google Scholar]
- Antić, D.; Milovanović, M.; Nikolić, S.; Milojković, M.; Perić, S. Simulation model of magnetic levitation based on NARX neural networks. Int. J. Intell. Syst. Appl. 2013, 5, 25–32. [Google Scholar] [CrossRef][Green Version]
- Suebsomran, A. Adaptive neural network control of electromagnetic suspension system. Int. J. Robot. Autom. 2014, 29, 144–154. [Google Scholar] [CrossRef]
- Jing, Y.; Xiao, J.; Zhang, K. Compensation of gap sensor for high-speed maglev train with RBF neural network. Trans. Inst. Meas. Control 2013, 35, 933–939. [Google Scholar] [CrossRef]
- Sun, Y.; Xu, J. Adaptive sliding mode control of maglev system based on RBF neural network minimum parameter learning method. Measurement 2018, 141, 217–226. [Google Scholar] [CrossRef]
- Yungang, L.; Chaoxiong, K.; Hu, C. Current loop analysis and optimization design of maglev train suspension controller. J. Natl. Univ. Def. Technol. 2006, 28, 94–97. [Google Scholar]
- Xudong, W.; Huihe, S. RBF neural network theory and its application in control. Inf. Control 1997, 26, 272–284. [Google Scholar]
- Sun, Q.; Li, J.; Wang, H. Optimization of levitation control parameters of maglev train based on genetic algorithm. Comput. Simul. 2006, 8, 229–231. [Google Scholar]







| Symbol | Quantity | Value |
|---|---|---|
| Cross-sectional area of iron core | ||
| Coil resistance | ||
| Permeability of air | ||
| Number of turns of coil | ||
| Quality of electromagnet system and load |
| Symbol | Quantity | Value |
|---|---|---|
| Cross-sectional area of iron core | ||
| Coil resistance | ||
| Stable levitation gap | ||
| Number of turns of coil | ||
| Mass of electromagnet system and load |
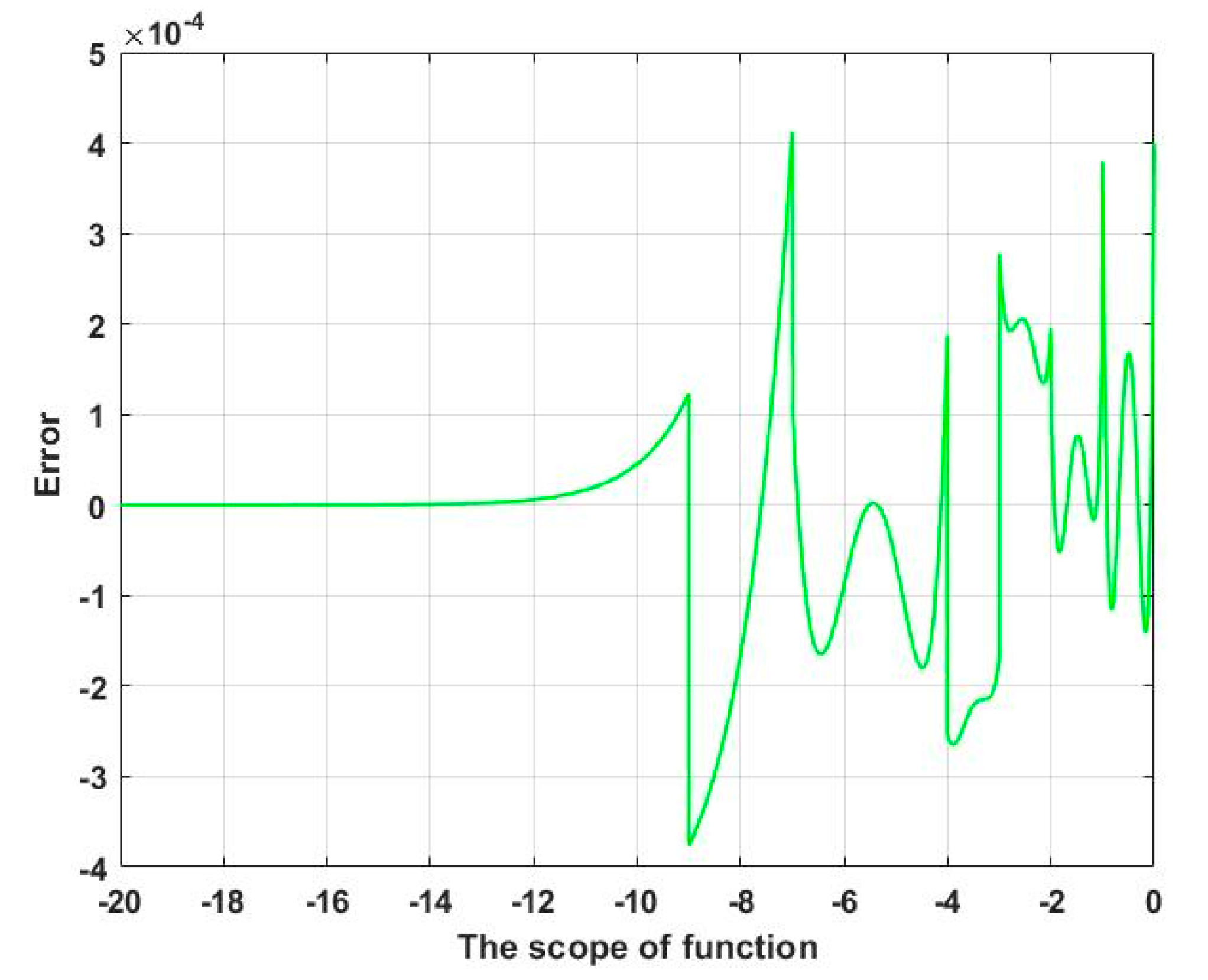
| Range | Polynomial Function | MSE |
|---|---|---|
| / | ||
| / |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, D.; Song, M.; Yu, P.; Li, J. Research of RBF-PID Control in Maglev System. Symmetry 2020, 12, 1780. https://doi.org/10.3390/sym12111780
Ma D, Song M, Yu P, Li J. Research of RBF-PID Control in Maglev System. Symmetry. 2020; 12(11):1780. https://doi.org/10.3390/sym12111780
Chicago/Turabian StyleMa, Danrui, Mengxiao Song, Peichang Yu, and Jie Li. 2020. "Research of RBF-PID Control in Maglev System" Symmetry 12, no. 11: 1780. https://doi.org/10.3390/sym12111780
APA StyleMa, D., Song, M., Yu, P., & Li, J. (2020). Research of RBF-PID Control in Maglev System. Symmetry, 12(11), 1780. https://doi.org/10.3390/sym12111780