A New Approach in Analytical Dynamics of Mechanical Systems
Abstract
1. Introduction
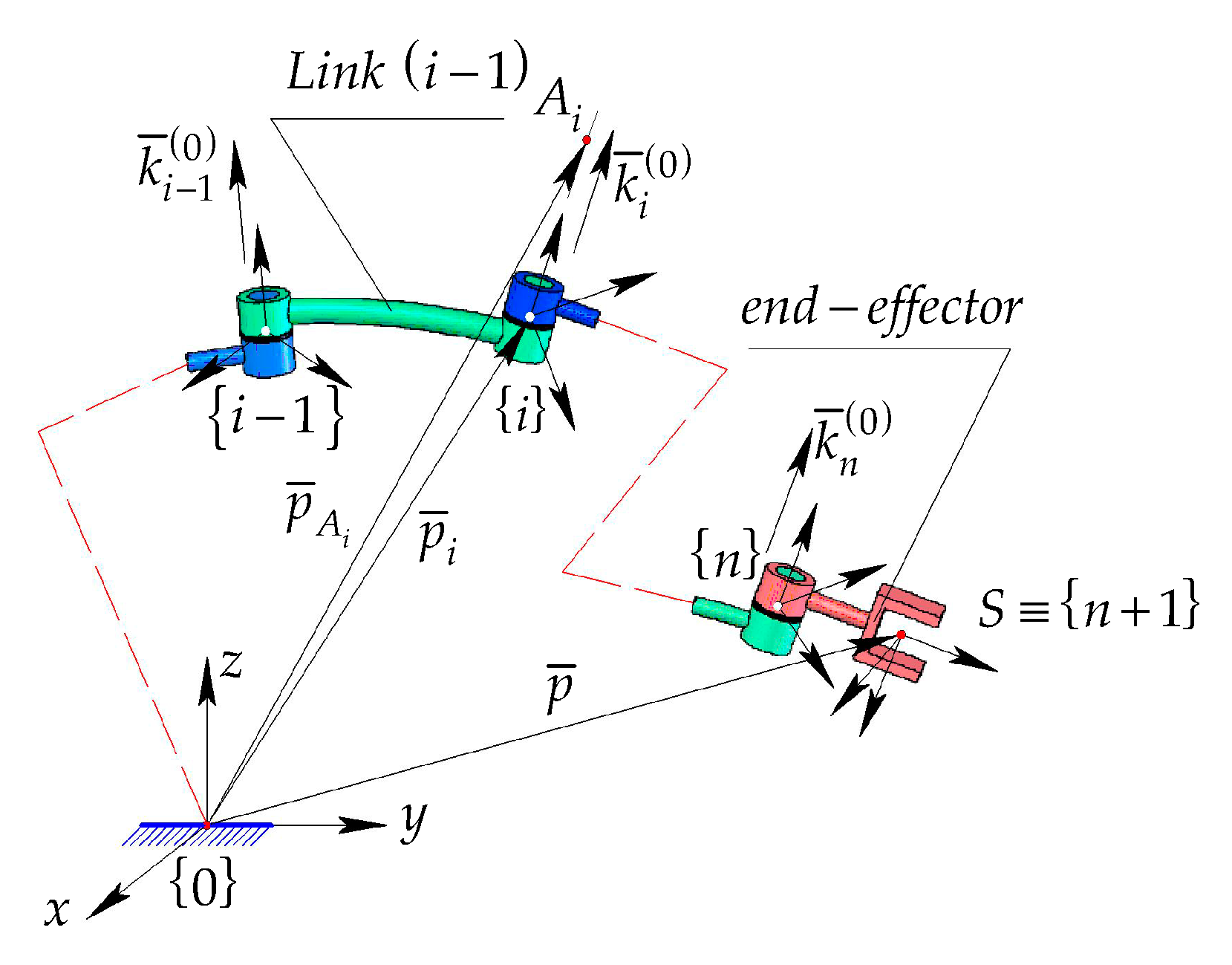
2. Matrices of the Homogenous Transformations
- is the homogenous transformation between the system and the fixed system ;
- is the homogenous transformation between the mobile system and fixed system ;
- is the orientation matrix between the adjoining mobile systems and ;
- defines the orientation matrix between the mobile system and fixed system ;
- defines the relative position between systems and the absolute position of the systems .
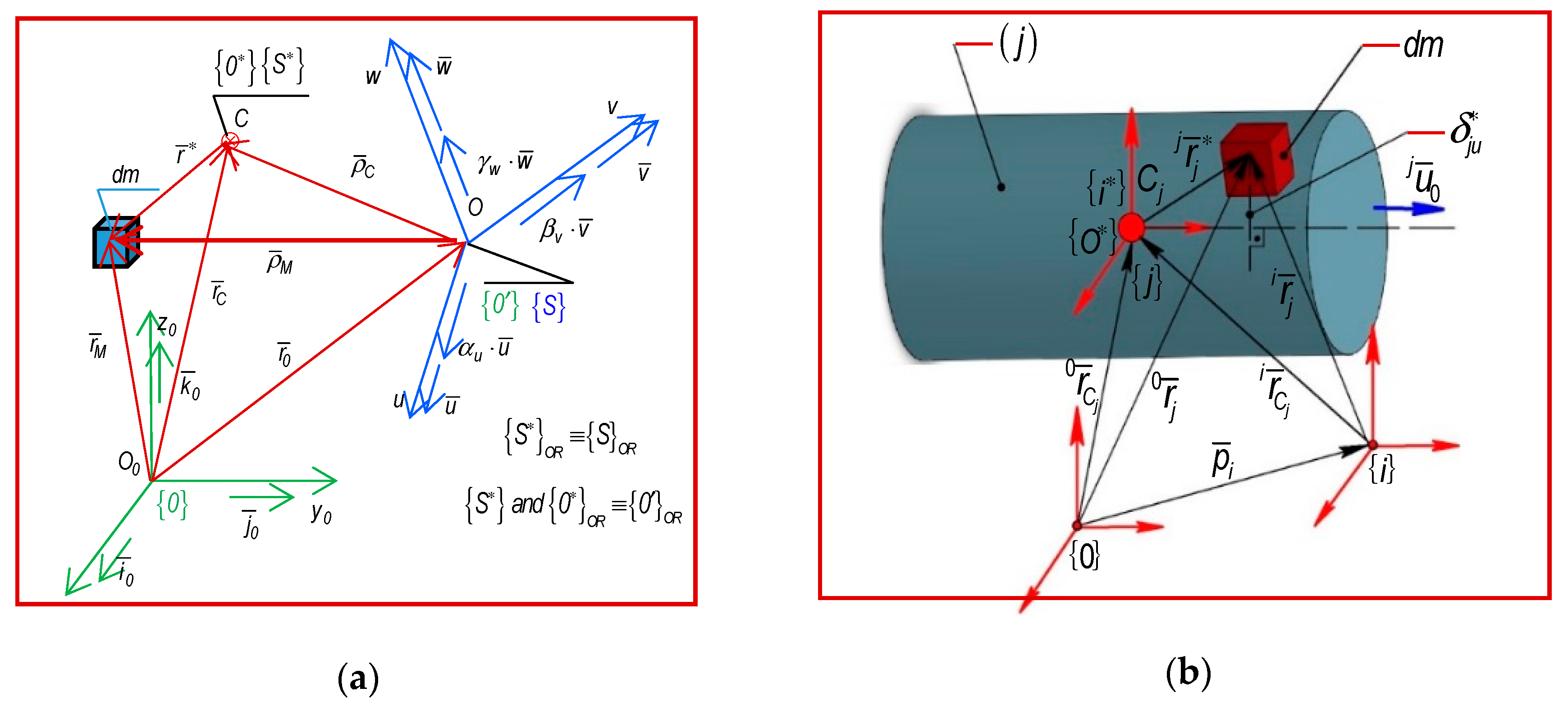
3. Advanced Kinematics Notions
3.1. Matrix Exponentials in Advanced Kinematics
- is the unit vector, in the initial configuration of the driving axis corresponding to the generalized coordinate ;
- when corresponds to an angular coordinate, otherwise .
3.2. The Differential Matrices in Advanced Kinematics
- and defines the position vector in the initial configuration and the orientation matrix of the system relative to , respectively;
- , the sign of generalized coordinate relative to the unit vector of the driving axis;
- represents the derivative matrix operator (Uicker operator).
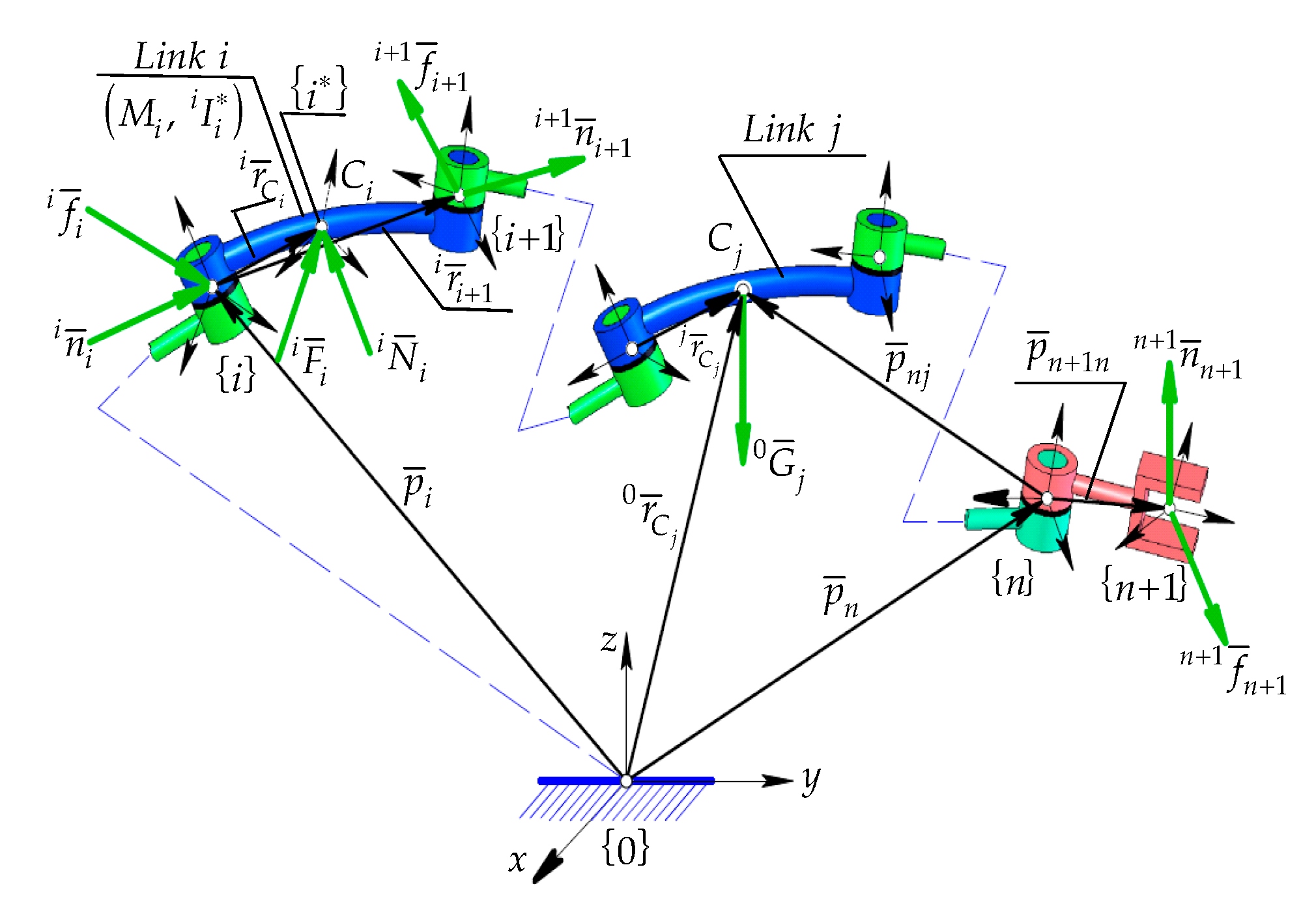
4. The Matrix Expressions of the Acceleration Energies
4.1. The Acceleration Energy of the First Order
4.2. The Acceleration Energy of Second-Order
4.3. The Acceleration Energy of Third-Order
5. The Advanced Dynamics Equations
5.1. The Generalized Forces of Higher-Order
5.2. The Generalized Inertia Forces of Higher-Order
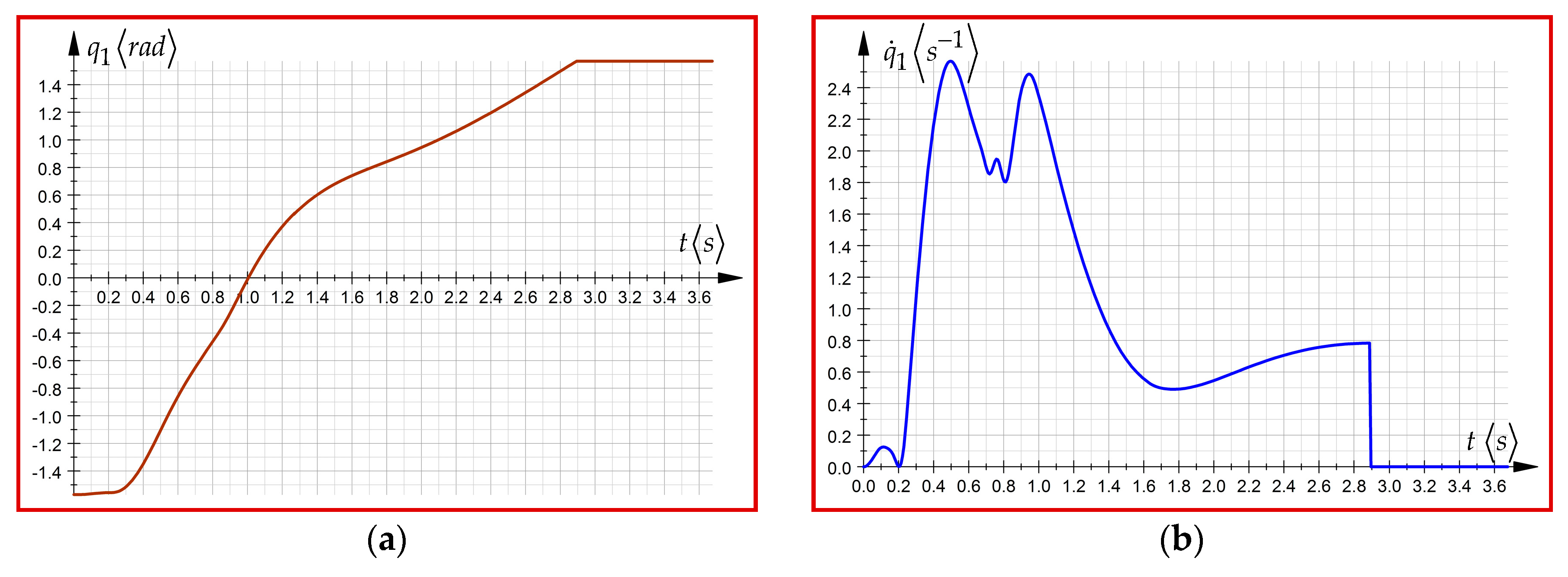
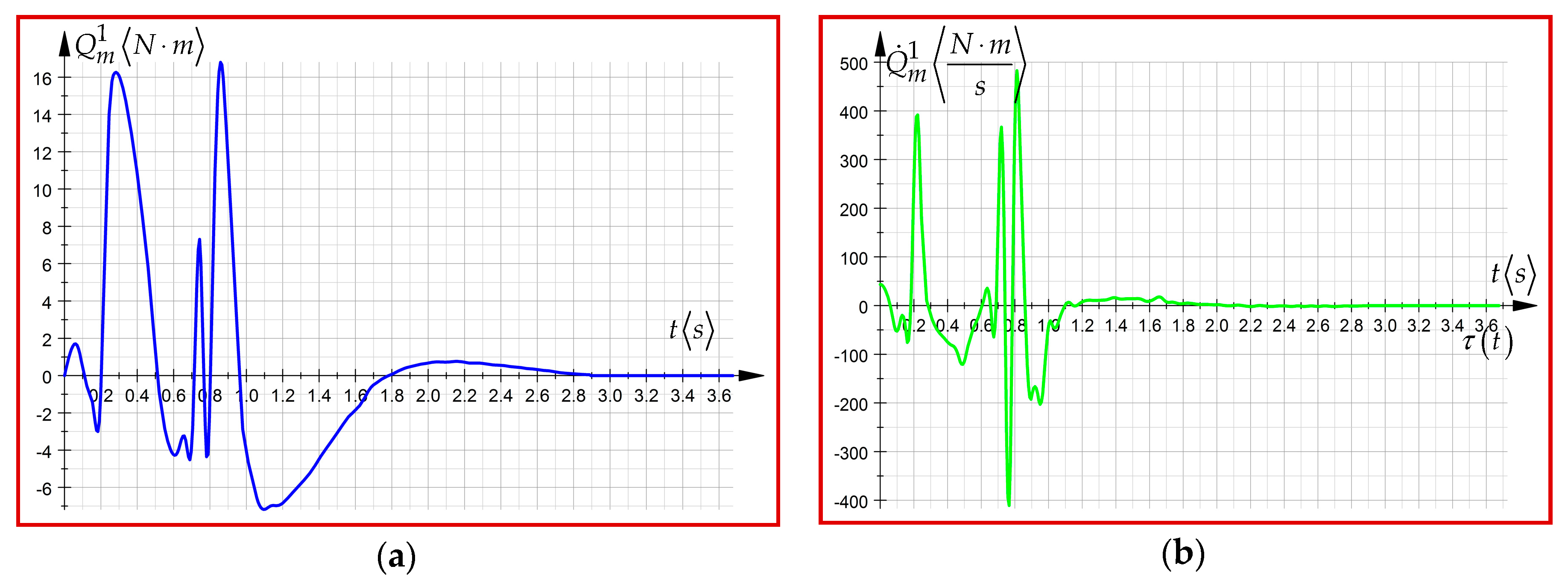
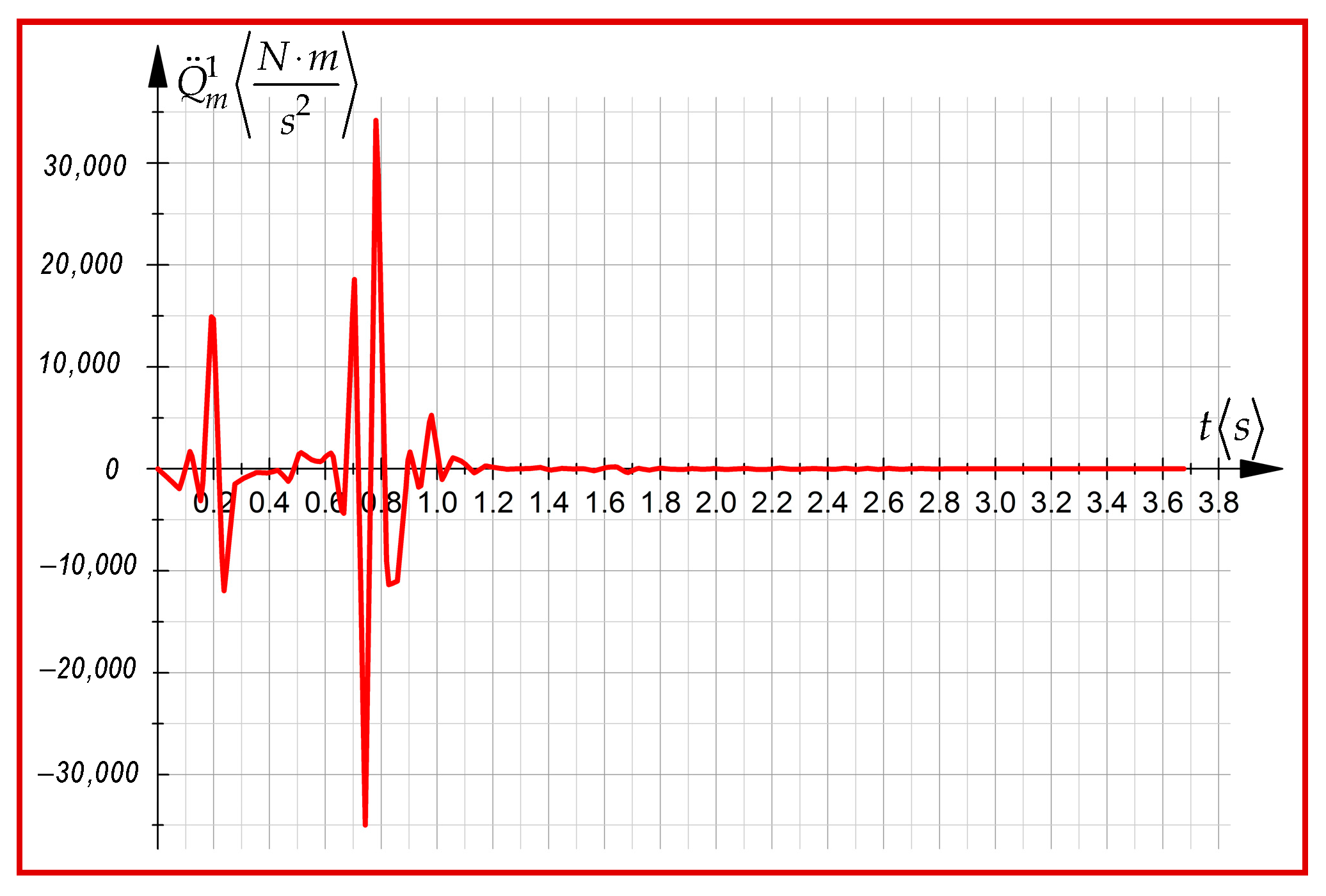
6. Application
7. Conclusions
8. Contributions of the Authors
- (1)
- 2. Matrices of the homogenous transformations. The new expressions are (1)–(7);
- (2)
- 3.1. Matrix exponentials in advanced kinematics. New Equations: (18), (23), (25)–(36), (38), (39);
- (3)
- 3.2. The differential matrices in advanced kinematics. New Equations: (41), (42), (44), (46)–(55);
- (4)
- 4. The matrix expressions of the acceleration energies. New expressions: (56)–(61), (64)–(75);
- (5)
- 5. The advanced dynamics equations. New Equations: (76), (80), (83), (85)–(87), (93)–(98).
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Eager, D.; Pendrill, A.M.; Reinstad, N. Beyond velocity and acceleration: Jerk, snap and higher derivatives. Eur. J. Phys. 2016, 37, 065008. [Google Scholar] [CrossRef]
- Visser, M. Jerk, Snap and the Cosmological Equation of State. Class. Quantum. Grav. 2004, 21, 2603–2616. [Google Scholar] [CrossRef]
- Negrean, I.; Negrean, D.C. The Acceleration Energy to Robot Dynamics. In Proceedings of the A&QT-R International Conference on Automation, Quality and Testing, Robotics, Cluj-Napoca, Romania, 23–25 May 2002; pp. 59–64. [Google Scholar]
- Negrean, I.; Crișan, A.-V. Synthesis on the Acceleration Energies in the Advanced Mechanics of the Multibody Systems. Symmetry 2019, 11, 1077. [Google Scholar] [CrossRef]
- Thompson, P. Snap, Crackle, and Pop; Systems Technology: Hawthorne, CA, USA, 2011. [Google Scholar]
- Shojaee, S.; Rostami, S.; Abbasi, A. An unconditionally stable implicit time integration algorithm: Modified quartic B-spline method. Comput. Struct. 2015, 153, 98–111. [Google Scholar] [CrossRef]
- Bruner, A.M.; Belvin, W.K.; Horta, L.G.; Juang, J.N. Active Vibration Absorber for the CSI Evolutionary Model–Design and Experimental Results. J. Guid. Control Dyn. 1992, 15, 1253–1257. [Google Scholar] [CrossRef]
- Kerkhoven, T.; Saad, Y. On acceleration methods for coupled nonlinear elliptic systems. Numer. Math. 1991, 60, 525–548. [Google Scholar] [CrossRef]
- Aghasi, M.; Dodson, C.T.J.; Galanis, G.N.; Suri, A. Infinite-dimensional second order ordinary differential equations via (TM)-M-2. Nonlinear Anal. Theor. 2007, 67, 2829–2838. [Google Scholar] [CrossRef]
- Thornbury, A.; Drury, L.O. Power requirements for cosmic ray propagation models involving re-acceleration and comment on second-order Fermi acceleration theory. Mon. Not. R. Astron. Soc. 2012, 442, 3010–3012. [Google Scholar] [CrossRef]
- Gao, C.J. Generalized modified gravity with the second-order acceleration equation. Phys. Rev. D 2012, 86, 103512. [Google Scholar] [CrossRef]
- Allan, W. The Ponderomotive Force of Standing Alfven Waves in a Dipolar Magnetoshere. J. Geophys. Res. Space Phys. 1993, 98, 1409–1417. [Google Scholar] [CrossRef]
- Hartquist, T.W.; Morfill, G.E. Cosmic-Ray Diffusion at Energies of 1-MEV to 10(5) GEV. Astrophys. Space Sci. 1994, 216, 223–234. [Google Scholar] [CrossRef]
- Wheaton, B.J.; Maybeck, P.S. 2nd-Order Acceleration Models for an MMAE Target Tracker. IEEE Trans. Aerosp. Electron. Syst. 1995, 31, 151–167. [Google Scholar] [CrossRef]
- Zhang, J.J.; Rajabhandharaks, D.; Xuan, J.R.; Chia, R.W.J.; Hasenberg, T. Calculus migration characterization during Ho:YAG laser lithotripsy by high-speed camera using suspended pendulum method. Laser Med. Sci. 2017, 32, 1017–1021. [Google Scholar] [CrossRef]
- Voltairas, P.A.; Fotiadis, D.I.; Michalis, L.K. An-Harmonic Analysis and the Arterial Pulse. Adv. Top. Scatt. Biomed. Eng. 2009, 30, 125–131. [Google Scholar]
- Appell, P. Sur Une Forme Générale des Equations de la Dynamique, 1st ed.; Gauthier-Villars: Paris, France, 1899. [Google Scholar]
- Appell, P. Traité de Mécanique Rationnelle, 1st ed.; Garnier Frères: Paris, France, 1903. [Google Scholar]
- Kitamura, Y.; Ishioka, K. Equatorial jets in decaying shallow-water turbulence on a rotating sphere. J. Atmos. Sci. 2007, 64, 3340–3353. [Google Scholar] [CrossRef]
- Mirtaheri, S.M.; Zohoor, H. The Explicit Gibbs-Appell Equations of Motion for Rigid-Body Constrained Mechanical System; Book Series: RSI International Conference on Robotics and Mechatronics ICRoM; IEEE: Piscataway, NJ, USA, 2018; pp. 304–309. [Google Scholar]
- Mehrjooee, O.; Dehkordi, S.F.; Korayem, M.H. Dynamic modeling and extended bifurcation analysis of flexible-link manipulator. Mech. Based Des. Struc. 2019, 6631650. [Google Scholar] [CrossRef]
- Shafei, A.M.; Shafei, H.R. A systematic method for the hybrid dynamic modeling of open kinematic chains confined in a closed environment. Multibody Syst. Dyn. 2017, 38, 21–42. [Google Scholar] [CrossRef]
- Amini, S.; Dehkordi, S.F.; Fahraji, S.H. Motion equation derivation and tip-over evaluations for K mobile manipulators with the consideration of drivings mass by the use of Gibbs-Appell formulation. In Proceedings of the 5th RSI International Conference on Robotics and Mechatronics (IcRoM), Tehran, Iran, 25–27 October 2017. [Google Scholar]
- Korayem, M.H.; Dehkordi, S.F. Derivation of dynamic equation of viscoelastic manipulator with revolute-prismatic joint using recursive Gibbs-Appell formulation. Nonlinear Dynam. 2017, 89, 2041–2064. [Google Scholar] [CrossRef]
- Shafei, A.M.; Korayem, M.H. Theoretical and experimental study of dynamic load-carrying capacity for flexible robotic arms in point-to-point motion. Optim. Contr. Appl. Met. 2017, 38, 963–972. [Google Scholar] [CrossRef]
- Shafei, A.M.; Shafei, H.R. Oblique Impact of Multi-Flexible-Link Systems. J. Vib. Control 2018, 24, 904–923. [Google Scholar] [CrossRef]
- Bachau, O.A. Flexible Multibody Dynamics, 1st ed.; Series: Solid Mechanics and its Applications; Springer: Dordrecht, The Netherlands, 2011; Volume 176, p. 730. [Google Scholar]
- Fu, K.S.; Gonzalez, R.C.; Lee, C.G. Control, Sensing, Vision and Intelligence; International Edition; McGraw-Hill Book Co.: Singapore, 1987. [Google Scholar]
- Marin, M.; Vlase, S.; Ellahi, R.; Bhatti, M. On the Partition of Energies for the Backward in Time Problem of Thermoelastic Materials with a Dipolar Structure. Symmetry 2019, 11, 863. [Google Scholar] [CrossRef]
- Negrean, I.; Negrean, D.C. Matrix Exponentials to Robot Kinematics. In Proceedings of the 17th International Conference on CAD/CAM, Robotics and Factories of the Future, CARS&FOF, Durban, South Africa, 10–12 July 2001; pp. 1250–1257. [Google Scholar]
- Park, F.C. Computational Aspects of the Product-of-Exponentials Formula for Robot Kinematics. IEEE Trans. Autom. Control 1994, 39, 643–647. [Google Scholar] [CrossRef]
- Jazar, R.N. Theory of Applied Robotics: Kinematics, Dynamics, and Control, 2nd ed.; Springer: Cham/Basel, Switzerland, 2010. [Google Scholar]
- Schiehlen, W.; Eberhard, P. Applied Dynamics, 1st ed.; Springer: Cham, Switzerland, 2014; p. 215. [Google Scholar]
- Gattringer, H.; Gerstmayr, J. Multibody System Dynamics, Robotics and Control, 1st ed.; Springer: Wien, Austria, 2013; p. 314. [Google Scholar]
- Negrean, I. Advanced Equations in Analytical Dynamics of Systems. Acta Tech. Napoc. Ser. Appl. Math. Mech. Eng. 2017, 60, 503–514. [Google Scholar]
- Pars, L.A. A Treatise on Analytical Dynamics; Heinemann: London, UK, 2007; Volume 1. [Google Scholar]
- Cassel, K. Variational Methods with Applications in Science and Engineering; Cambridge University Press: Cambridge, NY, USA, 2013; p. 413. [Google Scholar]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Negrean, I.; Crișan, A.-V.; Vlase, S. A New Approach in Analytical Dynamics of Mechanical Systems. Symmetry 2020, 12, 95. https://doi.org/10.3390/sym12010095
Negrean I, Crișan A-V, Vlase S. A New Approach in Analytical Dynamics of Mechanical Systems. Symmetry. 2020; 12(1):95. https://doi.org/10.3390/sym12010095
Chicago/Turabian StyleNegrean, Iuliu, Adina-Veronica Crișan, and Sorin Vlase. 2020. "A New Approach in Analytical Dynamics of Mechanical Systems" Symmetry 12, no. 1: 95. https://doi.org/10.3390/sym12010095
APA StyleNegrean, I., Crișan, A.-V., & Vlase, S. (2020). A New Approach in Analytical Dynamics of Mechanical Systems. Symmetry, 12(1), 95. https://doi.org/10.3390/sym12010095





