Small-Angle Scattering from Fractals: Differentiating between Various Types of Structures
Abstract
1. Introduction
- The fractal dimension , from the generalized power-law decay:
- The fractal scaling factor , from the period of the scattering curve on a double logarithmic scale,
- The number of fractal iterations m, equal to the number of the main minima,
- The inner and outer fractal cutoffs from the beginning and the end of periodicity region, i.e., from fractal regime,
- The total number of basic objects composing the fractal, from the relation .
2. Theoretical Background
2.1. Fractals
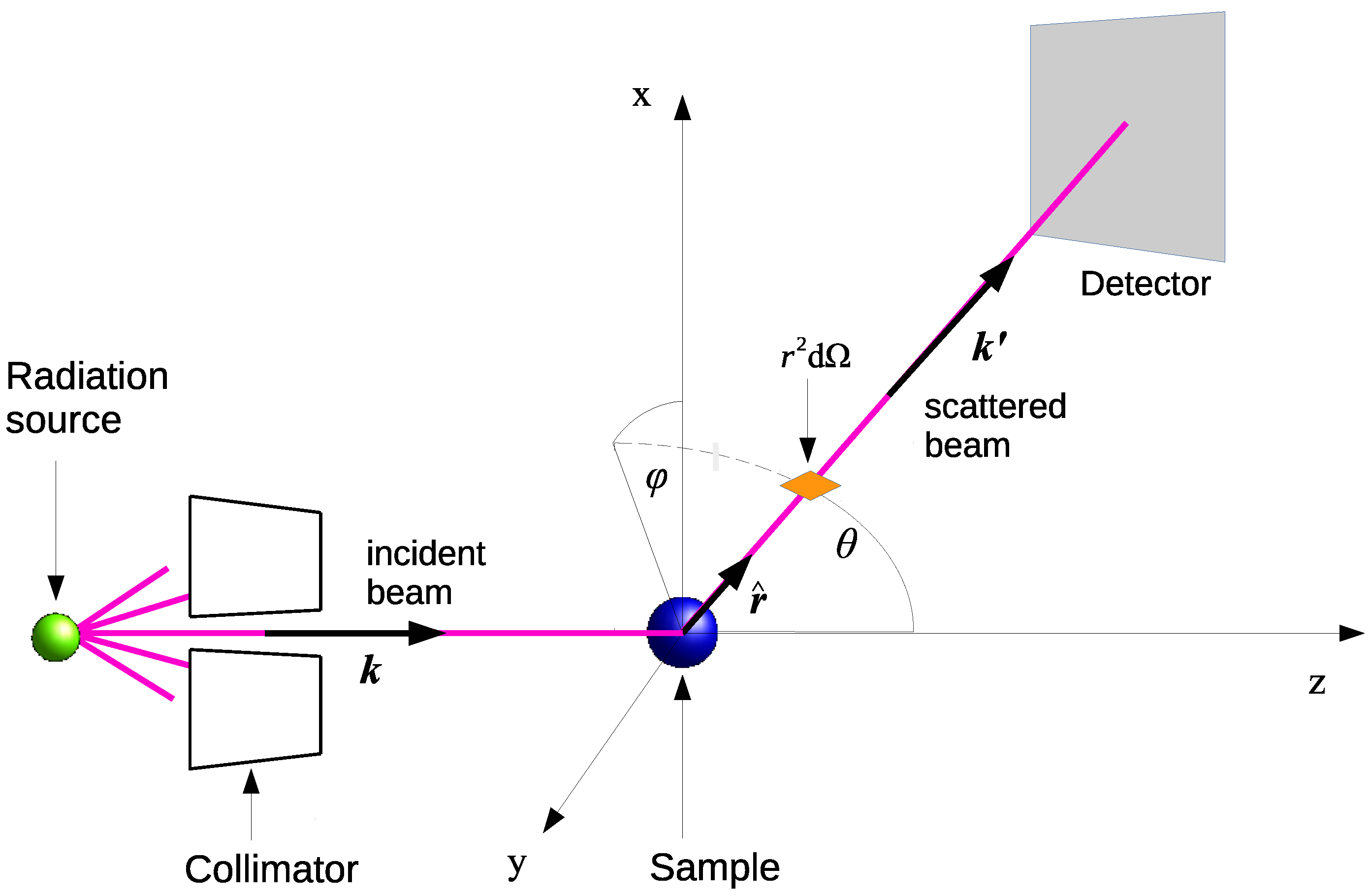
2.2. Small-Angle Scattering
2.2.1. General Background
2.2.2. Two-Phase Fractal Systems
2.2.3. Form Factor
2.2.4. Structure Factor
2.2.5. Polydispersity
- when the length is scaled as ,
- when the particle is translated ,
- , when the particle consists of two non-overlapping subsets I and .
2.3. Monte Carlo Simulations
3. Small-Angle Scattering from Fractals
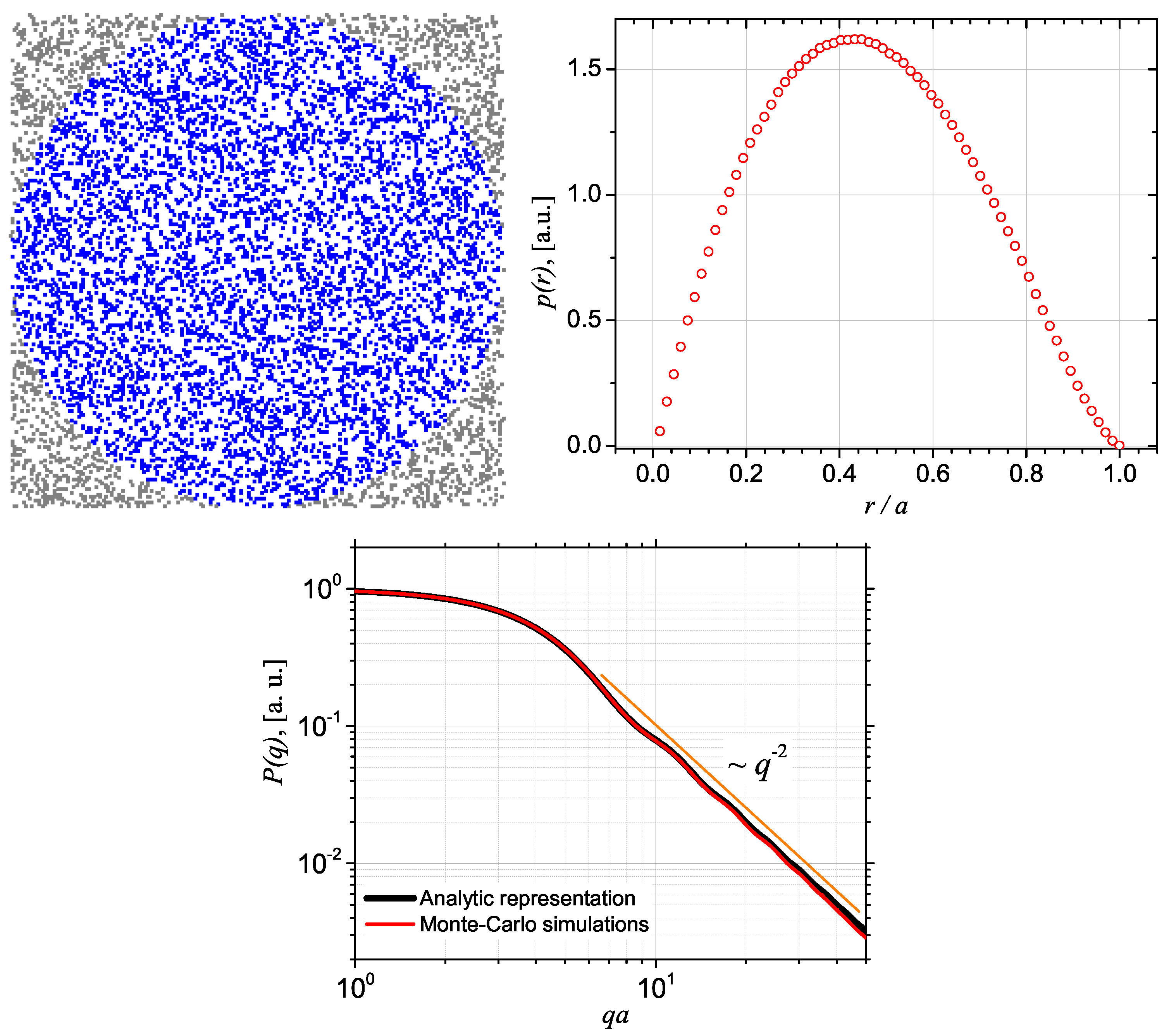
3.1. Random Mass Fractals
- When ,and therefore still dominates in Equation (34) since yet . The last equation shows that the scattering intensity of a mass fractal is a straight line on a double logarithmic scale. Therefore, deviations from this line at low and values of the scattering vector q provide information about the fractal overall size , and respectively about the radius a of fractal basic unit.
- When ,and thus, for , , while dominates in the scattering intensity [73]. Therefore, scattering at large q values provides information about the fractal basic particles.
3.2. Random Surface Fractals
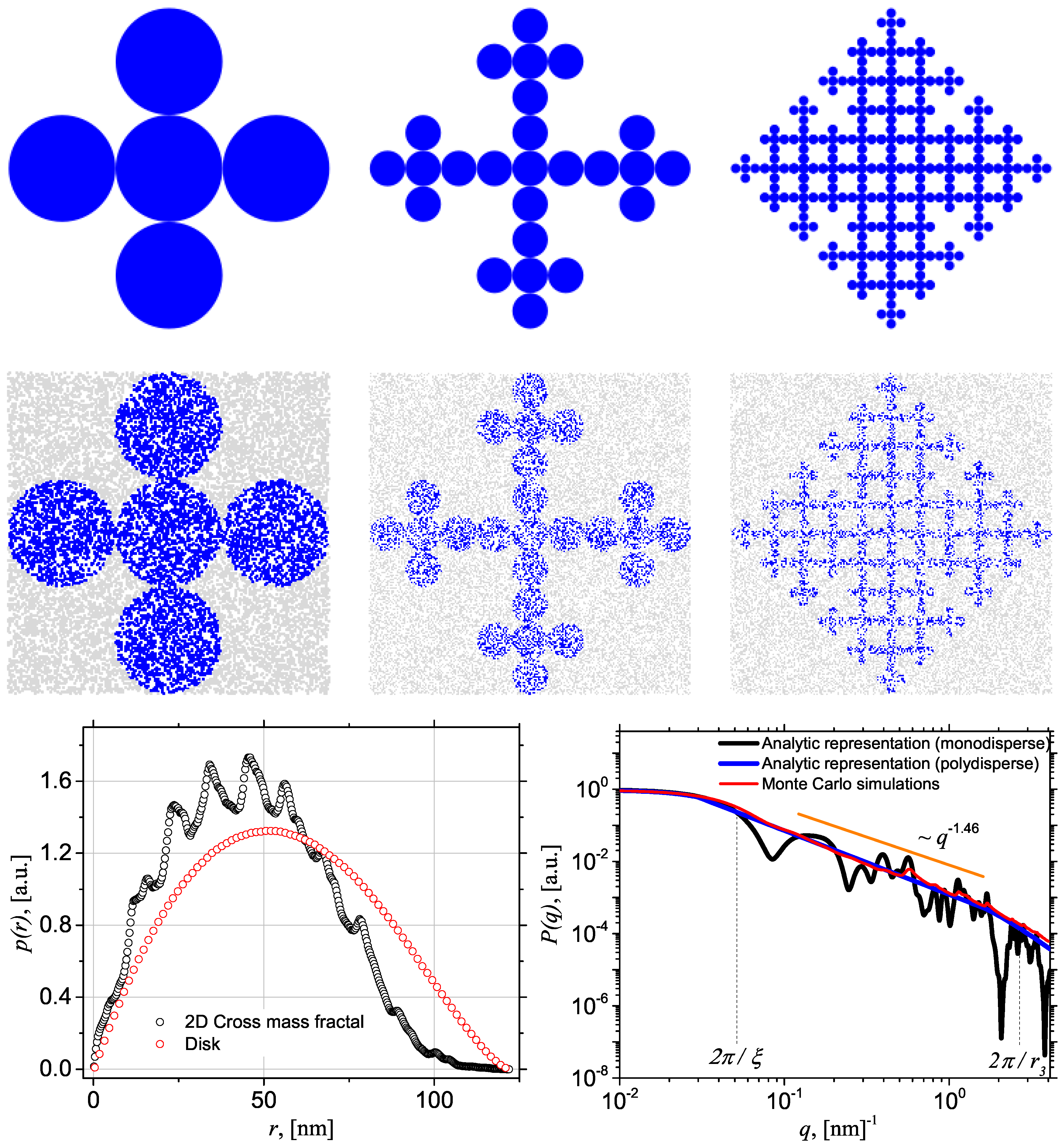
3.3. Deterministic Mass Factals
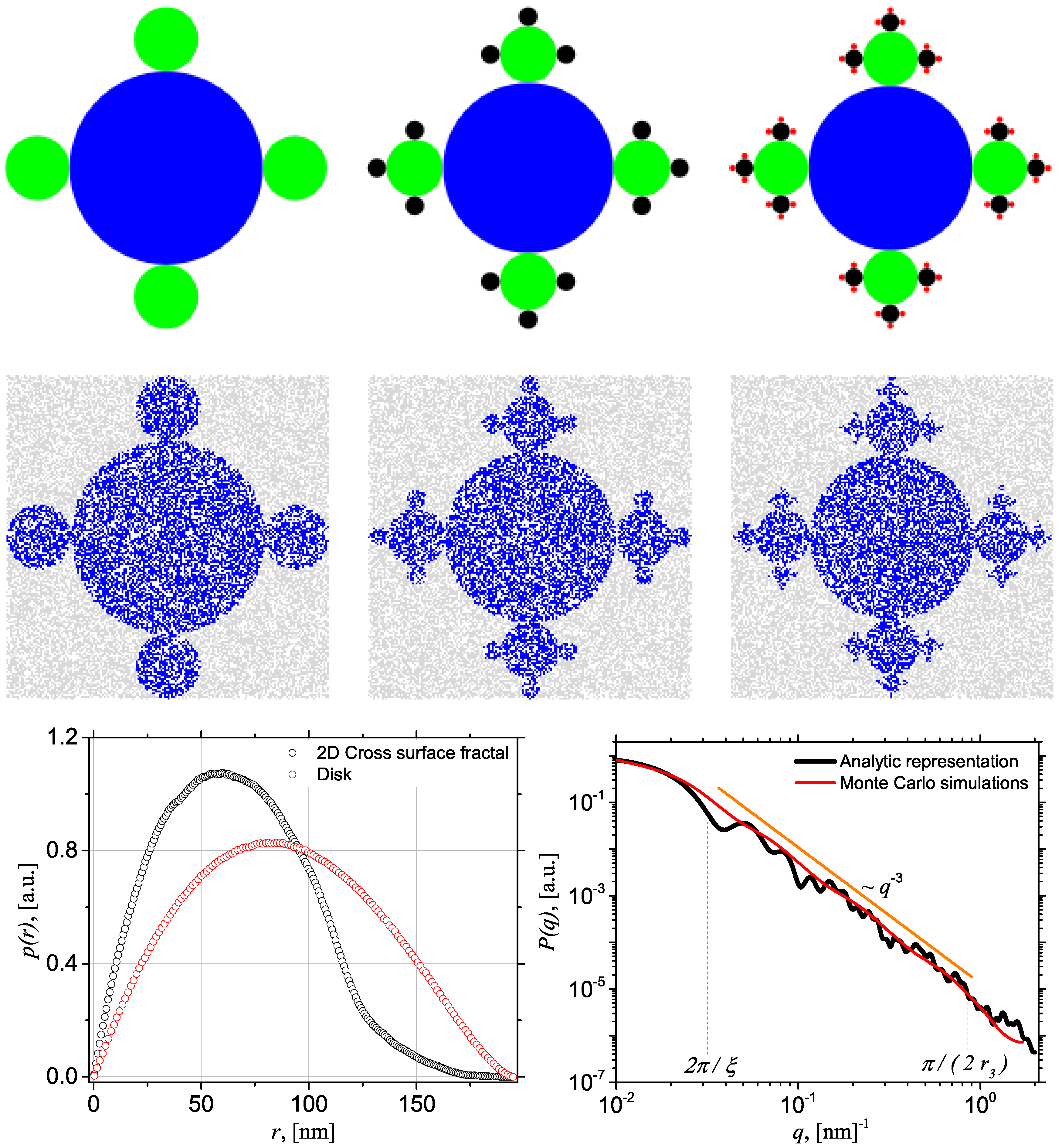
3.4. Deterministic Surface Fractals
3.5. Deterministic Multifractals
4. Conclusions
- Mass and surface fractals (Figure 9 upper row, left). The differentiation is made through the value of the scattering exponent in the fractal region that is for random mass fractals, and for surface fractals. Here, d is the Euclidean dimension of the space in which the fractal is embedded.
- Random and deterministic fractals (Figure 9 upper row, right). The differentiation is made based on the type of power-law decay, i.e., a simple power-law decay for random fractals, and a generalized power-law decay (a complex superposition of minima and maxima on a simple power-law decay) for deterministic fractals.
- Mono and multifractals (Figure 9 middle row, left). The differentiation is made through the presence of one or more power-decays, either simple or generalized. For monofractals, there is a single power-law decay, while for two-scale multifractals, there is a succession of a mass fractal followed by a surface fractal. When the two scaling factors have similar values, the length of the surface fractal region is very short, and vice-versa.
- Thin and fat fractals (Figure 9 middle row, right). The differentiation is made in a similar way as in the previous case. However, the main difference is that the surface fractal region is replaced by another mass fractal region with the exponent smaller than the one of the first mass fractal region.
- and fractals (Figure 9 lower row). The differentiation is made through the presence of an additional region of constant intensity between the fractal and Porod regions. For fractal in which the ratio of the size of basic units to the minimal characteristic distances between them is about unity, the length of this constant region is very short. However, for fractals with , the length of the constant region is much bigger.
Funding
Conflicts of Interest
References
- Chaudhuri, B.; Muñoz, I.G.; Qian, S.; Urban, V.S. (Eds.) Biological Small Angle Scattering: Techniques, Strategies and Tips, 1st ed.; Springer: Singapore, 2017; Volume 1009, p. 268. [Google Scholar] [CrossRef]
- Glatter, O. Scattering Methods and Their Application in Colloid And Interface Science, 1st ed.; Elsevier: Amsterdam, The Netherlands, 2018; p. 404. [Google Scholar]
- Jaksch, S.; Gutberlet, T.; Müller-Buschbaum, P. Grazing-incidence scattering—Status and perspectives in soft matter and biophysics. Curr. Opin. Colloid Interface Sci. 2019, 42, 73–86. [Google Scholar] [CrossRef]
- Allen, A.J. Scattering methods for disordered heterogeneous materials. In International Tables for Crystallography Volume H: Powder Diffraction; Gilmore, C.J., Kaduk, J.A., Schnek, H., Eds.; Wiley: Hoboken, NJ, USA, 2019; Chapter 5.8; pp. 673–696. [Google Scholar] [CrossRef]
- Wittmeier, A.; Cassini, C.; Hemonnot, C.; Weinhausen, B.; Bernhardt, M.; Salditt, T.; Koster, S. Scanning Small-Angle-X-Ray Scattering for Imaging Biological Cells. Microsc. Microanal. 2018, 24, 336–339. [Google Scholar] [CrossRef][Green Version]
- Mühlbauer, S.; Honecker, D.; Périgo, E.A.; Bergner, F.; Disch, S.; Heinemann, A.; Erokhin, S.; Berkov, D.; Leighton, C.; Eskildsen, M.R.; et al. Magnetic small-angle neutron scattering. Rev. Mod. Phys. 2019, 91, 015004. [Google Scholar] [CrossRef]
- Mahieu, E.; Gabel, F.; IUCr. Biological small-angle neutron scattering: Recent results and development. Acta Cryst. D 2018, 74, 715–726. [Google Scholar] [CrossRef]
- Oliver, R.C.; Rolband, L.A.; Hutchinson-Lundy, A.M.; Afonin, K.A.; Krueger, J.K. Small-Angle Scattering as a Structural Probe for Nucleic Acid Nanoparticles (NANPs) in a Dynamic Solution Environment. Nanomaterials 2019, 9, 681. [Google Scholar] [CrossRef]
- Kryukova, A.E.; Shpichka, A.I.; Konarev, P.V.; Volkov, V.V.; Timashev, P.S.; Asadchikov, V.E. Shape Determination of Bovine Fibrinogen in Solution Using Small-Angle Scattering Data. Cryst. Rep. 2018, 63, 871–873. [Google Scholar] [CrossRef]
- Palit, S.; He, L.; Hamilton, W.A.; Yethiraj, A.; Yethiraj, A. Combining Diffusion NMR and Small-Angle Neutron Scattering Enables Precise Measurements of Polymer Chain Compression in a Crowded Environment. Phys. Rev. Lett. 2017, 118, 097801. [Google Scholar] [CrossRef]
- Sakurai, S. Recent developments in polymer applications of synchrotron small-angle X-ray scattering. Polym. Int. 2017, 66, 237–249. [Google Scholar] [CrossRef]
- Horkay, F.; Basser, P.J.; Hecht, A.M.; Geissler, E. Ionic effects in semi-dilute biopolymer solutions: A small angle scattering study. J. Chem. Phys. 2018, 149, 163312. [Google Scholar] [CrossRef]
- Mortensen, K.; Annaka, M. Stretching PEO–PPO Type of Star Block Copolymer Gels: Rheology and Small- Angle Scattering. ACS Macro Lett. 2018, 7, 1438–1442. [Google Scholar] [CrossRef]
- Bocz, K.; Decsov, K.E.; Farkas, A.; Vadas, D.; Bárány, T.; Wacha, A.; Bóta, A.; Marosi, G. Non-destructive characterisation of all-polypropylene composites using small angle X-ray scattering and polarized Raman spectroscopy. Compos. Part A 2018, 114, 250–257. [Google Scholar] [CrossRef]
- Wolff, M.; Saini, A.; Simonne, D.; Adlmann, F.; Nelson, A. Time Resolved Polarised Grazing Incidence Neutron Scattering from Composite Materials. Polymers 2019, 11, 445. [Google Scholar] [CrossRef] [PubMed]
- Bhaway, S.M.; Qiang, Z.; Xia, Y.; Xia, X.; Lee, B.; Yager, K.G.; Zhang, L.; Kisslinger, K.; Chen, Y.M.; Liu, K.; et al. Operando Grazing Incidence Small-Angle X-ray Scattering/X-ray Diffraction of Model Ordered Mesoporous Lithium-Ion Battery Anodes. ACS Nano 2017, 11, 1443–1454. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.M.; Petracic, O.; Kentzinger, E.; Rücker, U.; Schmitz, M.; Wei, X.K.; Heggen, M.; Brückel, T. Strain and electric-field control of magnetism in supercrystalline iron oxide nanoparticle–BaTiO3 composites. Nanoscale 2017, 9, 12957–12962. [Google Scholar] [CrossRef] [PubMed]
- Choi, Y.; Paik, D.; Bogdanov, S.; Valiev, E.; Borisova, P.; Murashev, M.; Em, V.; Pirogov, A. Neutron scattering on humane compact bone. Phys. B 2018, 551, 218–221. [Google Scholar] [CrossRef]
- Busch, A.; Kampman, N.; Bertier, P.; Pipich, V.; Feoktystov, A.; Rother, G.; Harrington, J.; Leu, L.; Aertens, M.; Jacops, E. Predicting Effective Diffusion Coefficients in Mudrocks Using a Fractal Model and Small-Angle Neutron Scattering Measurements. Water Resour. Res. 2018, 54, 7076–7091. [Google Scholar] [CrossRef]
- Penttilä, P.A.; Rautkari, L.; Österberg, M.; Schweins, R. Small-angle scattering model for efficient characterization of wood nanostructure and moisture behaviour. J. Appl. Cryst. 2019, 52, 369–377. [Google Scholar] [CrossRef]
- Li, A.; He, R.; Bian, Z.; Song, H.; Chen, X.; Zhou, J. Enhanced lithium storage performance of hierarchical CuO nanomaterials with surface fractal characteristics. App. Surf. Sci. 2018, 443, 382–388. [Google Scholar] [CrossRef]
- Wang, Y.; Maurel, G.; Couty, M.; Detcheverry, F.; Merabia, S. Implicit Medium Model for Fractal Aggregate Polymer Nanocomposites: Linear Viscoelastic Properties. Macromolecules 2019, 52, 2021–2032. [Google Scholar] [CrossRef]
- Wei, R.; Wang, X.; Zhang, X.; Wang, S.; Zhu, S.; Du, S. A Novel Method for Manufacturing High-Performance Layered Silicate/Epoxy Nanocomposites Using an Epoxy-Diamine Adduct to Enhance Compatibility and Interfacial Reactivity. Macromol. Mater. Eng. 2018, 303, 1800065. [Google Scholar] [CrossRef]
- Yue, H.; Reguero, V.; Senokos, E.; Monreal-Bernal, A.; Mas, B.; Fernández-Blázquez, J.; Marcilla, R.; Vilatela, J. Fractal carbon nanotube fibers with mesoporous crystalline structure. Carbon 2017, 122, 47–53. [Google Scholar] [CrossRef]
- Zhang, J.; Li, H.; Ye, J.; Cao, Z.; Chen, J.; Kuang, Q.; Zheng, J.; Xie, Z. Sierpinski gasket-like Pt–Ag octahedral alloy nanocrystals with enhanced electrocatalytic activity and stability. Nano Energy 2019, 61, 397–403. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. The Fractal Geometry of Nature; W.H. Freeman: New York, NY, USA, 1982; p. 460. [Google Scholar]
- Zhu, W.; Esteban, R.; Borisov, A.G.; Baumberg, J.J.; Nordlander, P.; Lezec, H.J.; Aizpurua, J.; Crozier, K.B. Quantum mechanical effects in plasmonic structures with subnanometre gaps. Nat. Commun. 2016, 7, 11495. [Google Scholar] [CrossRef] [PubMed]
- Sichert, J.A.; Tong, Y.; Mutz, N.; Vollmer, M.; Fischer, S.; Milowska, K.Z.; García Cortadella, R.; Nickel, B.; Cardenas-Daw, C.; Stolarczyk, J.K.; et al. Quantum Size Effect in Organometal Halide Perovskite Nanoplatelets. Nano Lett. 2015, 15, 6521–6527. [Google Scholar] [CrossRef]
- Nazmitdinov, R.G. From Chaos to Order in Mesoscopic Systems. Phys. Part. Nucl. Lett. 2019, 16, 159–169. [Google Scholar] [CrossRef]
- Schaefer, D.W.; Justice, R.S. How Nano Are Nanocomposites? Macromolecules 2007, 40, 8501–8517. [Google Scholar] [CrossRef]
- Yurekli, K.; Mitchell, C.A.; Krishnamoorti, R. Small-angle neutron scattering from surfactant-assisted aqueous dispersions of carbon nanotubes. J. Am. Chem. Soc. 2004, 126, 9902–9903. [Google Scholar] [CrossRef]
- Yin, W.; Dadmun, M. A New Model for the Morphology of P3HT/PCBM Organic Photovoltaics from Small-Angle Neutron Scattering: Rivers and Streams. ACS Nano 2011, 5, 4756–4768. [Google Scholar] [CrossRef]
- Stevens, D.A.; Dahn, J.R. An In Situ Small-Angle X-Ray Scattering Study of Sodium Insertion into a Nanoporous Carbon Anode Material within an Operating Electrochemical Cell. J. Electrochem. Soc. 2000, 147, 4428–4431. [Google Scholar] [CrossRef]
- Zabar, S.; Lesmes, U.; Katz, I.; Shimoni, E.; Bianco-Peled, H. Studying different dimensions of amylose-long chain fatty acid complexes: Molecular, nano and micro level characteristics. Food Hydrocoll. 2009, 23, 1918–1925. [Google Scholar] [CrossRef]
- Jacques, D.A.; Trewhella, J. Small-angle scattering for structural biology-Expanding the frontier while avoiding the pitfalls. Prot. Sci. 2010, 19, 642–657. [Google Scholar] [CrossRef] [PubMed]
- Li, T.; Senesi, A.J.; Lee, B. Small Angle X-Ray Scattering for Nanoparticle Research. Chem. Rev. 2016, 116, 11128–11180. [Google Scholar] [CrossRef] [PubMed]
- Pfeifer, P.; Ehrburger-Dolle, F.; Rieker, T.P.; González, M.T.; Hoffman, W.P.; Molina-Sabio, M.; Rodríguez-Reinoso, F.; Schmidt, P.W.; Voss, D.J. Nearly Space-Filling Fractal Networks of Carbon Nanopores. Phys. Rev. Lett. 2002. [Google Scholar] [CrossRef] [PubMed]
- Bale, H.D.; Schmidt, P.W. Small-Angle X-Ray-Scattering Investigation of Submicroscopic Porosity with Fractal Properties. Phys. Rev. Lett. 1984, 53, 596–599. [Google Scholar] [CrossRef]
- Teixeira, J. Small-angle scattering by fractal systems. J. Appl. Cryst. 1988, 21, 781–785. [Google Scholar] [CrossRef]
- Pfeifer, P. Simple Models for the Formation of Random Rough Surfaces. In Multiple Scattering of Waves in Random Media and Random Rough Surfaces, Proceedings of an International Symposium, University Park, PA, USA, 29 July–2 August 1985; Varadan, V.V., Varadan, V.K., Eds.; Pennsylvania State University State College: State College, PA, USA, 1987; p. 964. [Google Scholar]
- Martin, J.E.; Hurd, A.J. Scattering from fractals. J. Appl. Cryst. 1987, 20, 61–78. [Google Scholar] [CrossRef]
- Schmidt, P.W. Small-angle scattering studies of disordered, porous and fractal systems. J. Appl. Cryst. 1991, 24, 414–435. [Google Scholar] [CrossRef]
- Beaucage, G. Approximations Leading to a Unified Exponential/Power-Law Approach to Small-Angle Scattering. J. Appl. Cryst. 1995, 28, 717–728. [Google Scholar] [CrossRef]
- Beaucage, G. Small-Angle Scattering from Polymeric Mass Fractals of Arbitrary Mass-Fractal Dimension. J. Appl. Cryst. 1996, 29, 134–146. [Google Scholar] [CrossRef]
- Hammouda, B. A new Guinier–Porod model. J. Appl. Cryst. 2010, 43, 716–719. [Google Scholar] [CrossRef]
- Papagiannopoulos, A.; Meristoudi, A.; Pispas, S.; Keiderling, U. Thermal response of self-organization in an amphiphilic triblock polyelectrolyte and the influence of the globular protein lysozyme. Eur. Polym. J. 2018, 99, 49–57. [Google Scholar] [CrossRef]
- Anovitz, L.M.; Cole, D.R. Characterization and Analysis of Porosity and Pore Structures. Rev. Miner. Geochem. 2015, 80, 61–164. [Google Scholar] [CrossRef]
- Qiao, D.; Liu, H.; Yu, L.; Bao, X.; Simon, G.P.; Petinakis, E.; Chen, L. Preparation and characterization of slow- release fertilizer encapsulated by starch-based superabsorbent polymer. Carbohydr. Polym. 2016, 147, 146–154. [Google Scholar] [CrossRef] [PubMed]
- Liu, D.; Song, L.; Song, H.; Chen, J.; Tian, Q.; Chen, L.; Sun, L.; Lu, A.; Huang, C.; Sun, G. Correlation between mechanical properties and microscopic structures of an optimized silica fraction in silicone rubber. Compos. Sci. Technol. 2018, 165, 373–379. [Google Scholar] [CrossRef]
- Bauer, P.; Amenitsch, H.; Baumgartner, B.; Koberl, G.; Rentenberger, C.; Winkler, P.M. In-situ aerosol nanoparticle characterization by small angle X-ray scattering at ultra-low volume fraction. Nat. Commun. 2019, 10, 1122. [Google Scholar] [CrossRef] [PubMed]
- Song, P.; Tu, X.; Bai, L.; Sun, G.; Tian, Q.; Gong, J.; Zeng, G.; Chen, L.; Qiu, L. Contrast Variation Small Angle Neutron Scattering Investigation of Micro- and Nano-Sized TATB. Materials 2019, 12, 2606. [Google Scholar] [CrossRef] [PubMed]
- Shang, J.; Wang, Y.; Chen, M.; Dai, J.; Zhou, X.; Kuttner, J.; Hilt, G.; Shao, X.; Gottfried, J.M.; Wu, K. Assembling molecular Sierpiński triangle fractals. Nat. Chem. 2015, 7, 389–393. [Google Scholar] [CrossRef]
- Wang, L.; Liu, R.; Gu, J.; Song, B.; Wang, H.; Jiang, X.; Zhang, K.; Han, X.; Hao, X.Q.; Bai, S.; et al. Self-Assembly of Supramolecular Fractals from Generation 1 to 5. J. Am. Chem. Soc. 2018, 140, 14087–14096. [Google Scholar] [CrossRef]
- Wang, Y.; Xue, N.; Li, R.; Wu, T.; Li, N.; Hou, S.; Wang, Y. Construction and Properties of Sierpiński Triangular Fractals on Surfaces. Chem. Phys. Chem. 2019, 20, 2262–2270. [Google Scholar] [CrossRef]
- Newkome, G.R.; Moorefield, C.N. From 1 → 3 dendritic designs to fractal supramacromolecular constructs: Understanding the pathway to the Sierpiński gasket. Chem. Soc. Rev. 2015, 44, 3954–3967. [Google Scholar] [CrossRef]
- Malankowska, M.; Schlautmann, S.; Berenschot, E.; Tiggelaar, R.; Pina, M.; Mallada, R.; Tas, N.; Gardeniers, H. Three-Dimensional Fractal Geometry for Gas Permeation in Microchannels. Micromachines 2018, 9, 45. [Google Scholar] [CrossRef] [PubMed]
- Wu, Z.; Chen, X.; Zheng, Y.; Wang, T. Fabrication of Poly(methyl methacrylate) (PMMA) Microfluidic Chip with Cantor Fractal Structure Using a CO2 Laser System. Laser Eng. 2019, 43, 121–129. [Google Scholar]
- Cherny, A.Y.; Anitas, E.M.; Osipov, V.A.; Kuklin, A.I. Deterministic fractals: Extracting additional information from small-angle scattering data. Phys. Rev. E 2011, 84, 036203. [Google Scholar] [CrossRef]
- Anitas, E.M. Small-angle scattering from fat fractals. Eur. Phys. J. B 2014, 87, 139. [Google Scholar] [CrossRef][Green Version]
- Cherny, A.Y.; Anitas, E.M.; Osipov, V.A.; Kuklin, A.I.; IUCr. Scattering from surface fractals in terms of composing mass fractals. J. Appl. Cryst. 2017, 50, 919–931. [Google Scholar] [CrossRef]
- Anitas, E.M.; Slyamov, A.; Todoran, R.; Szakacs, Z. Small-Angle Scattering from Nanoscale Fat Fractals. Nanoscale Res. Lett. 2017, 12, 389. [Google Scholar] [CrossRef] [PubMed]
- Anitas, E.M.; Marcelli, G.; Szakacs, Z.; Todoran, R.; Todoran, D. Structural Properties of Vicsek-like Deterministic Multifractals. Symmetry 2019, 11, 806. [Google Scholar] [CrossRef]
- Hausdorff, F. Dimension und äußeres Maß. Math. Ann. 1918, 79, 157–179. [Google Scholar] [CrossRef]
- Rogers, C.A. Hausdorff Measures; Cambridge University Press: Cambridge, UK, 1970; p. 179. [Google Scholar]
- Gouyet, J.F. Physics and Fractal Structures; Masson: Paris, France, 1996; p. 234. [Google Scholar]
- Roe, R.J. Methods of X-ray and Neutron Scattering in Polymer Science; Oxford University Press: New York, NY, USA, 2000; p. 352. [Google Scholar]
- Arneodo, A.; Decoster, N.; Roux, S. A wavelet-based method for multifractal image analysis. I. Methodology and test applications on isotropic and anisotropic random rough surfaces. Eur. Phys. J. B 2000, 15, 567–600. [Google Scholar] [CrossRef]
- Decoster, N.; Roux, S.; Arnéodo, A. A wavelet-based method for multifractal image analysis. II. Applications to synthetic multifractal rough surfaces. Eur. Phys. J. B 2000, 15, 739–764. [Google Scholar] [CrossRef]
- Chhabra, A.; Jensen, R.V. Direct Determination of the f (alpha) Singularity Spectrum. Phys. Rev. Lett. 1989, 62, 1327. [Google Scholar] [CrossRef] [PubMed]
- Muzy, J.F.; Bacry, E.; Arneodo, A. Multifractal formalism for fractal signals: The structure-function approach versus the wavelet-transform modulus-maxima method. Phys. Rev. E 1993, 47, 875–884. [Google Scholar] [CrossRef] [PubMed]
- Feigin, L.A.; Svergun, D.I. Structure Analysis by Small-Angle X-ray and Neutron Scattering; Springer: Boston, MA, USA, 1987; p. 335. [Google Scholar] [CrossRef]
- Anitas, E.M. Small-Angle Scattering (Neutrons, X-rays, Light) from Complex Systems; SpringerBriefs in Physics; Springer International Publishing: Cham, Switzerland, 2019. [Google Scholar] [CrossRef]
- Melnichenko, Y.B. Small-Angle Scattering from Confined and Interfacial Fluids; Springer International Publishing: Cham, Switzerland, 2016; p. 329. [Google Scholar] [CrossRef]
- Debye, P.; Bueche, A.M. Scattering by an Inhomogeneous Solid. J. Appl. Phys. 1949, 20, 518–525. [Google Scholar] [CrossRef]
- Kaya, H. Scattering behaviour of Janus particles. Appl. Phys. A 2002, 74. [Google Scholar] [CrossRef]
- Kratky, O.; Porod, G. Diffuse small-angle scattering of X-rays in colloid systems. J. Colloid Sci. 1949, 4, 35–70. [Google Scholar] [CrossRef]
- Sorensen, C.M. Light Scattering by Fractal Aggregates: A Review. Aerosol Sci. Technol. 2001, 35, 648–687. [Google Scholar] [CrossRef]
- Witten, T.A.; Sander, L.M. Diffusion-Limited Aggregation, a Kinetic Critical Phenomenon. Phys. Rev. Lett. 1981, 47, 1400–1403. [Google Scholar] [CrossRef]
- Glatter, O.; May, R. Small-angle techniques. In International Tables for Crystallography; International Union of Crystallography: Chester, UK, 2006; pp. 89–112. [Google Scholar] [CrossRef]
- Muthukumar, M. Mean-Field Theory for Diffusion-Limited Cluster Formation. Phys. Rev. Lett. 1983, 50, 839–842. [Google Scholar] [CrossRef]
- Meakin, P. The structure of two-dimensional Witten-Sander aggregates. J. Phys. A 1985, 18, L661–L666. [Google Scholar] [CrossRef]
- Cherny, A.Y.; Anitas, E.M.; Osipov, V.A.; Kuklin, A.I. The structure of deterministic mass and surface fractals: Theory and methods of analyzing small-angle scattering data. Phys. Chem. Chem. Phys. 2019, 21, 12748–12762. [Google Scholar] [CrossRef]






| Fractal Type | Parameters | Source | Fractal Power-Law Decays |
|---|---|---|---|
| Random mass fractals | Exponent of power-law decay | A single simple power-law decay with exponent . | |
| Random surface fractals | Exponent of power-law decay | A single simple power-law decay with exponent . | |
| Deterministic mass fractals | Exponent of power-law decay | A single generalized power-law decay with exponent . | |
| Period on the logarithmic scale | |||
| m | Number of periods in logarithmic scale | ||
| Deterministic surface fractals | Exponent of power-law decay | A single generalized power-law decay with exponent . | |
| Period on the logarithmic scale | |||
| m | Number of periods in logarithmic scale | ||
| Deterministic fat fractals | Exponents of power-law decays at each structural level | A succession of generalized power-law decay with exponents | |
| Periods on the logarithmic scale at each structural level | |||
| Number of periods in logarithmic scale at each structural level | |||
| As for deterministic mass fractals, but at each structural level | |||
| Deterministic multifractals with two scaling factors | Exponents of power-law decays in each fractal region | A succession of mass-to-surface fractal generalized power-law decays, with exponents , and respectively . | |
| Periods on the logarithmic scale from mass, and surface fractal regions | |||
| m | Number of periods in logarithmic scale from mass or surface fractal regions | ||
| Deterministic mass fractals with | Exponents of the power-law decay | A region with constant intensity occurs after the fractal region. | |
| Periods on the logarithmic scale from mass fractal region | |||
| m | Number of periods in logarithmic scale from mass fractal regions | ||
| h | End of the constant region | ||
| As for deterministic mass fractals |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anitas, E.M. Small-Angle Scattering from Fractals: Differentiating between Various Types of Structures. Symmetry 2020, 12, 65. https://doi.org/10.3390/sym12010065
Anitas EM. Small-Angle Scattering from Fractals: Differentiating between Various Types of Structures. Symmetry. 2020; 12(1):65. https://doi.org/10.3390/sym12010065
Chicago/Turabian StyleAnitas, Eugen Mircea. 2020. "Small-Angle Scattering from Fractals: Differentiating between Various Types of Structures" Symmetry 12, no. 1: 65. https://doi.org/10.3390/sym12010065
APA StyleAnitas, E. M. (2020). Small-Angle Scattering from Fractals: Differentiating between Various Types of Structures. Symmetry, 12(1), 65. https://doi.org/10.3390/sym12010065





