Dynamic Behavior Analysis of a High-Rise Traction System with Tensioned Pulley Acting on Compensating Rope
Abstract
1. Introduction
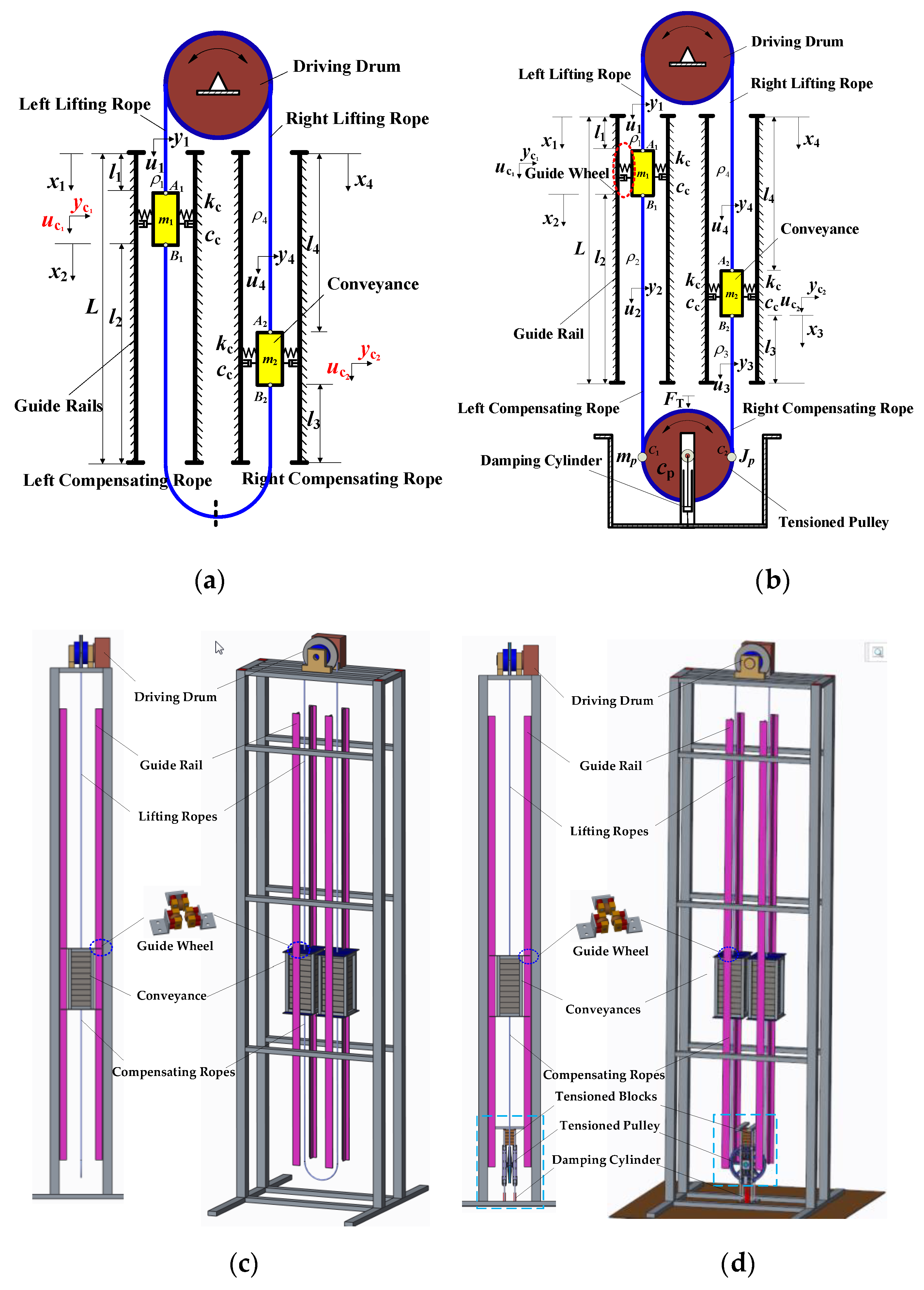
2. Modeling Description
2.1. Description of the System
2.2. Derivation of the Accurate Mathematical Model
3. Study Case
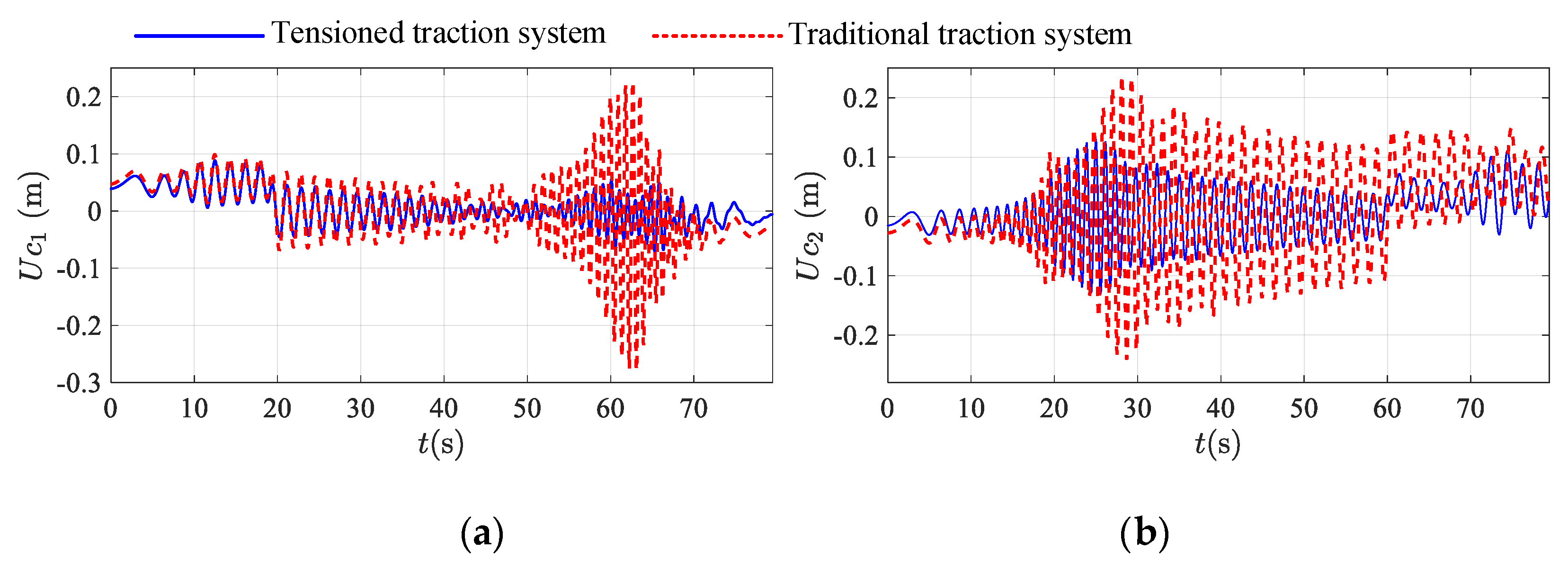
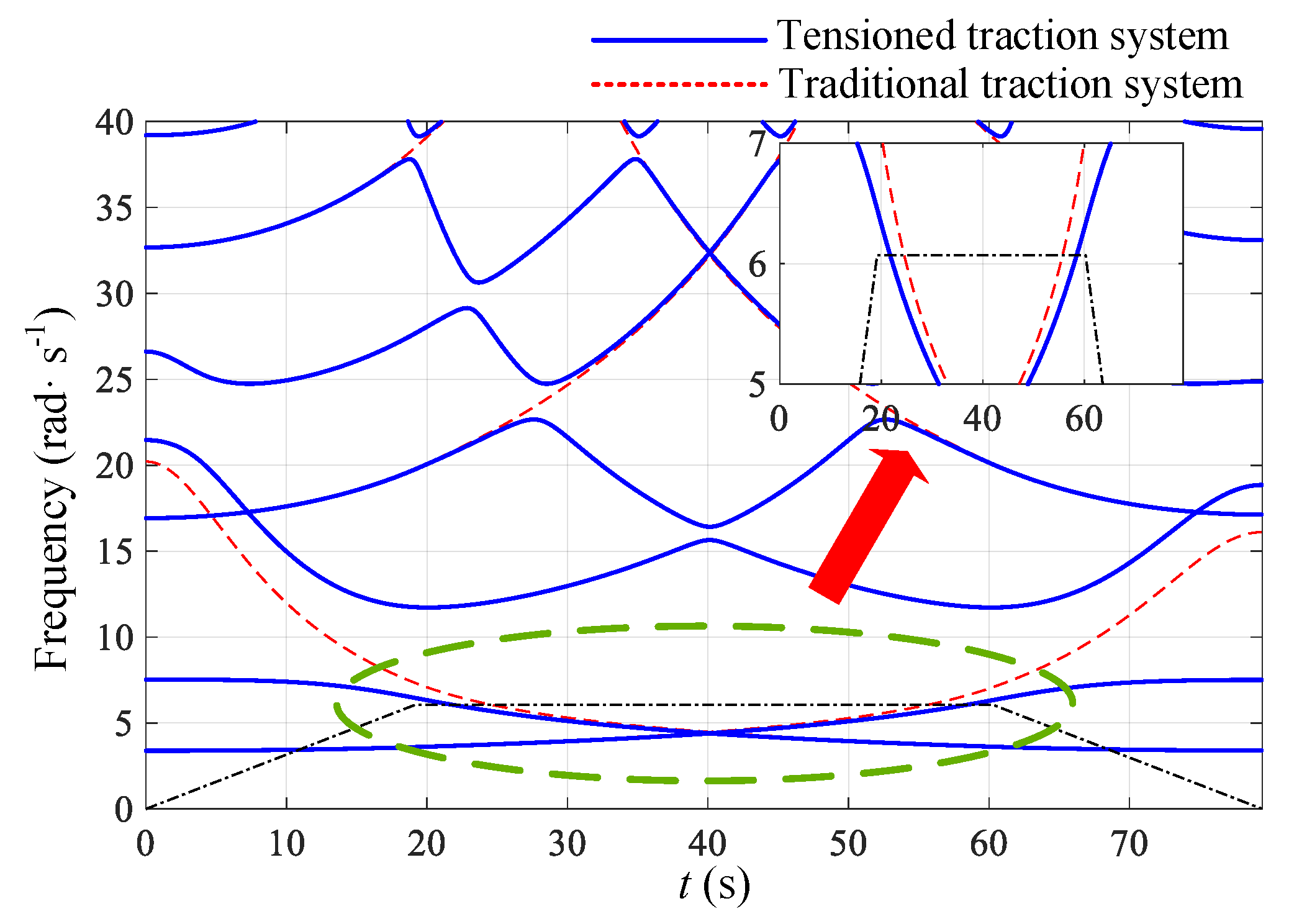
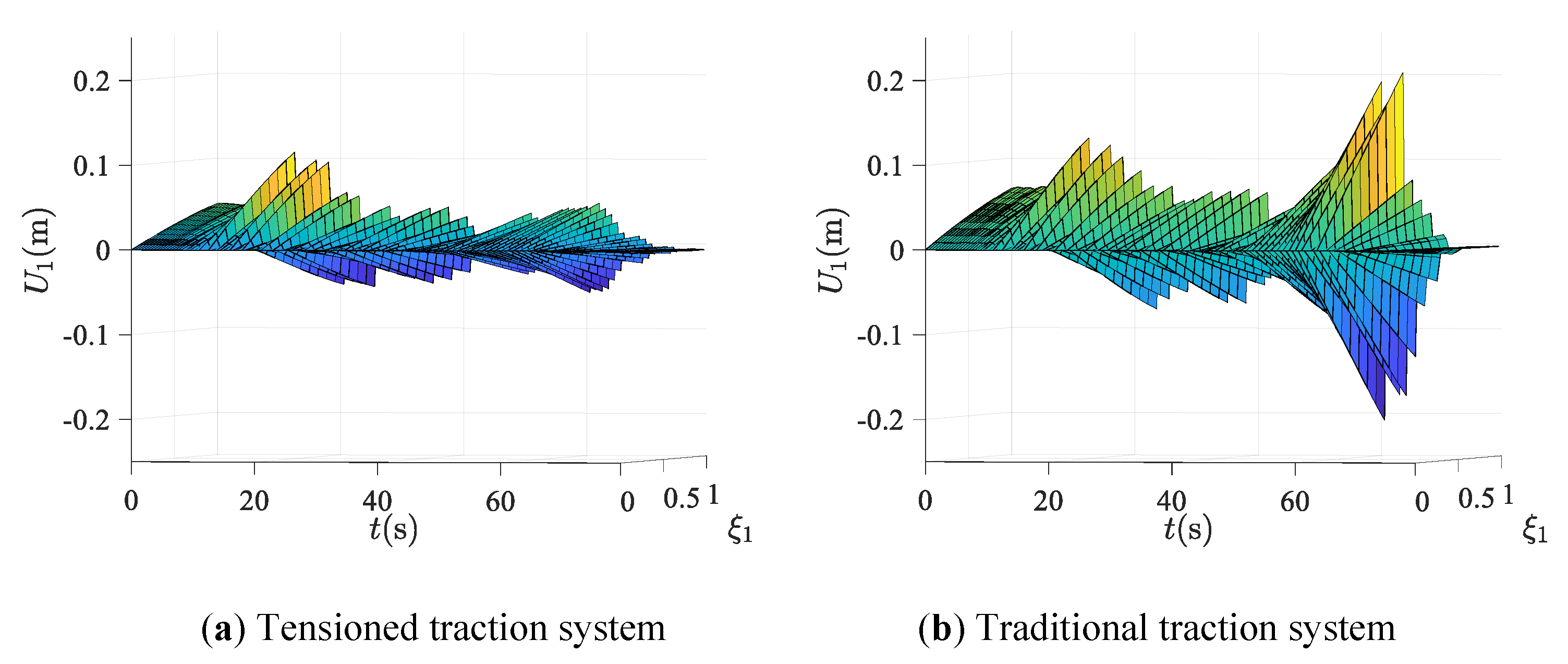
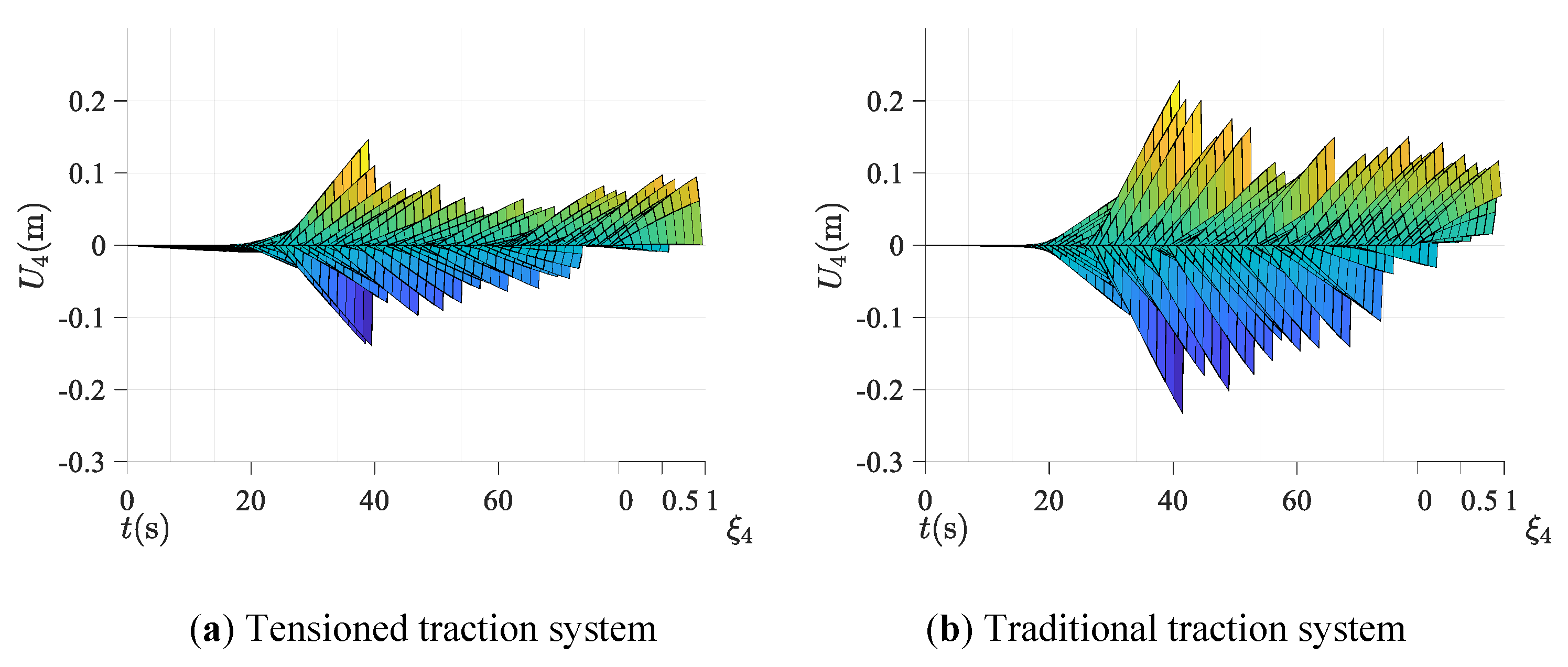
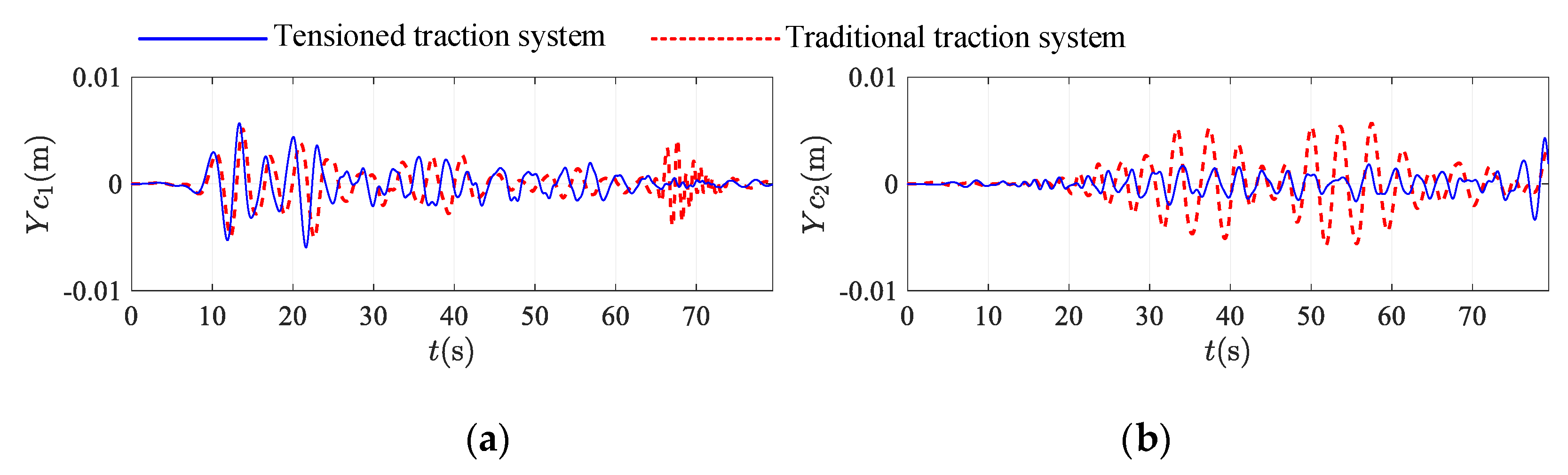
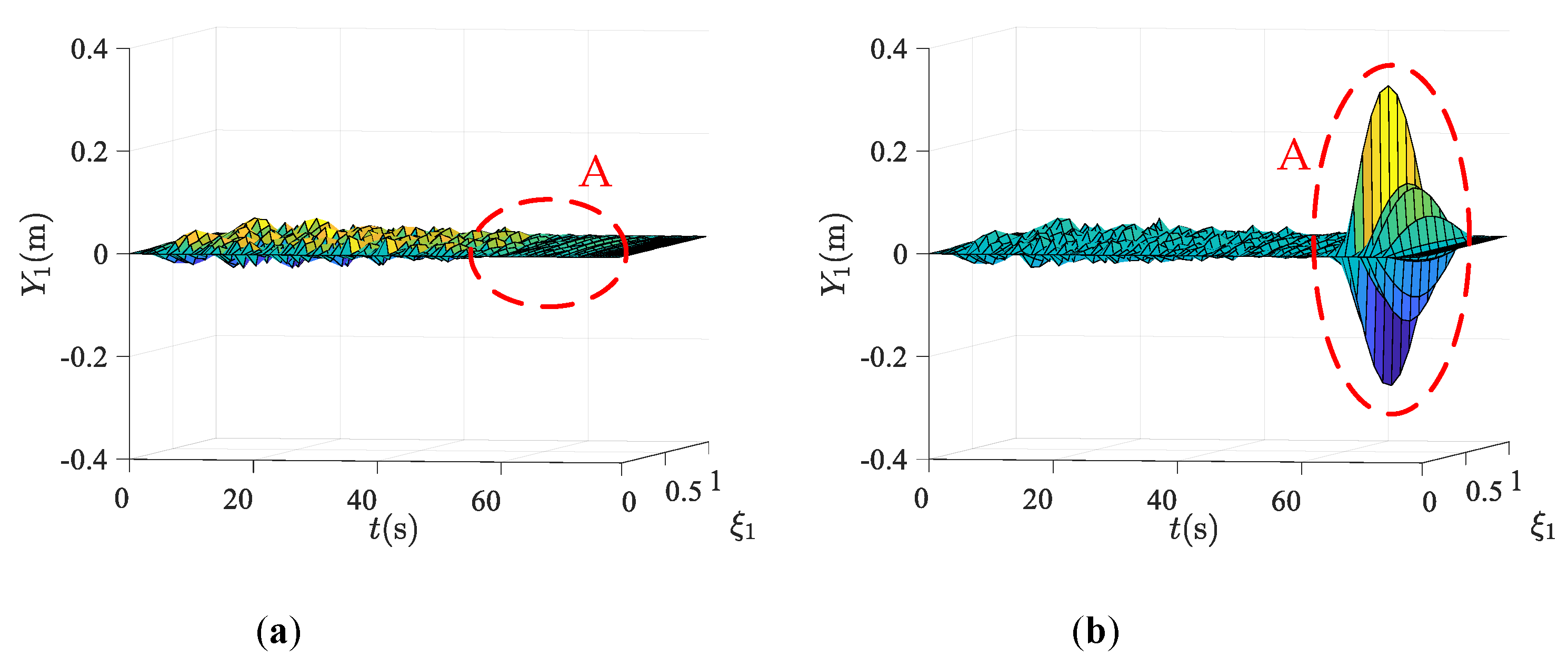
3.1. Comparison between Traditional Traction System and Tensioned Traction System
3.2. Discussion on Tensioned Pattern
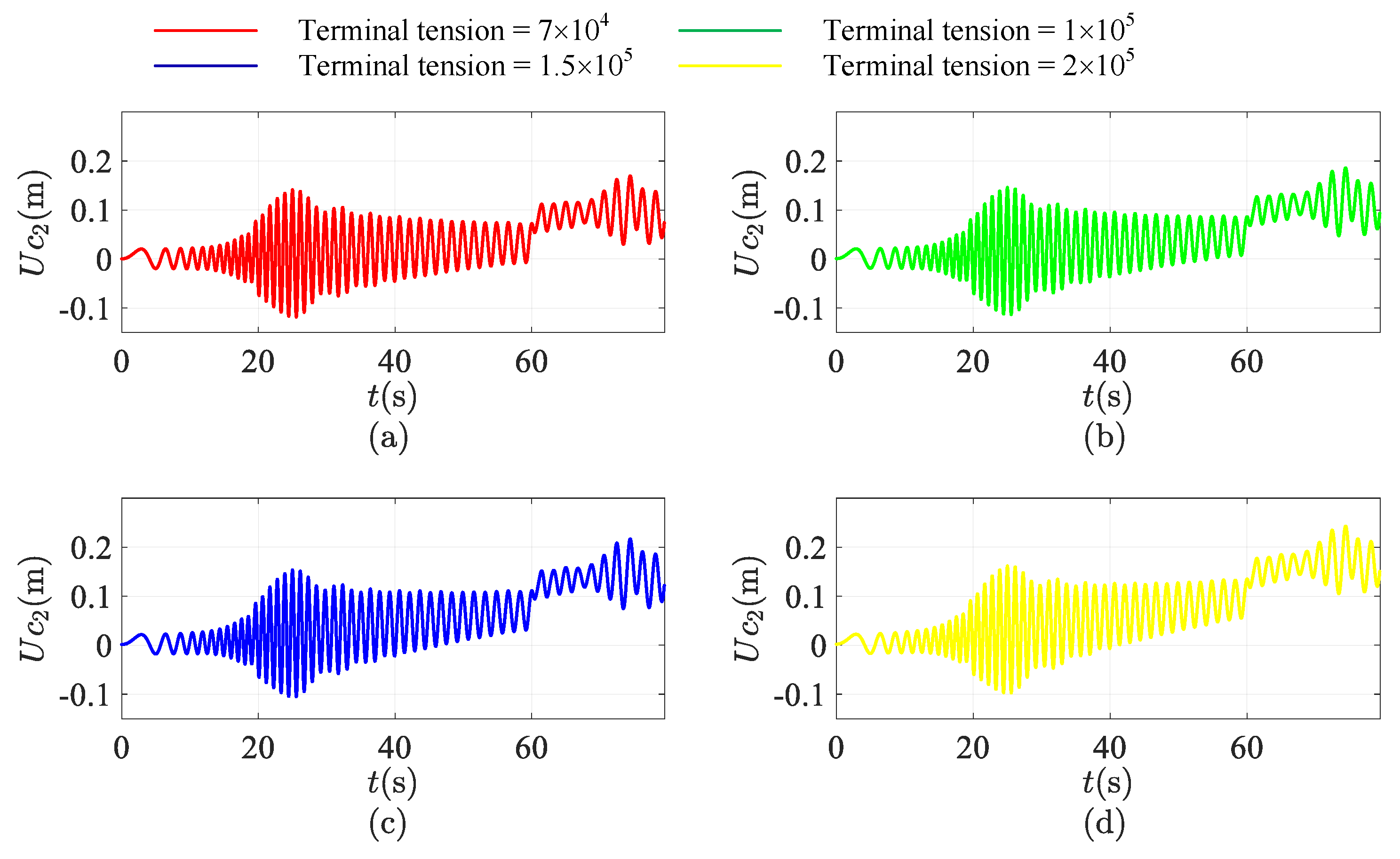
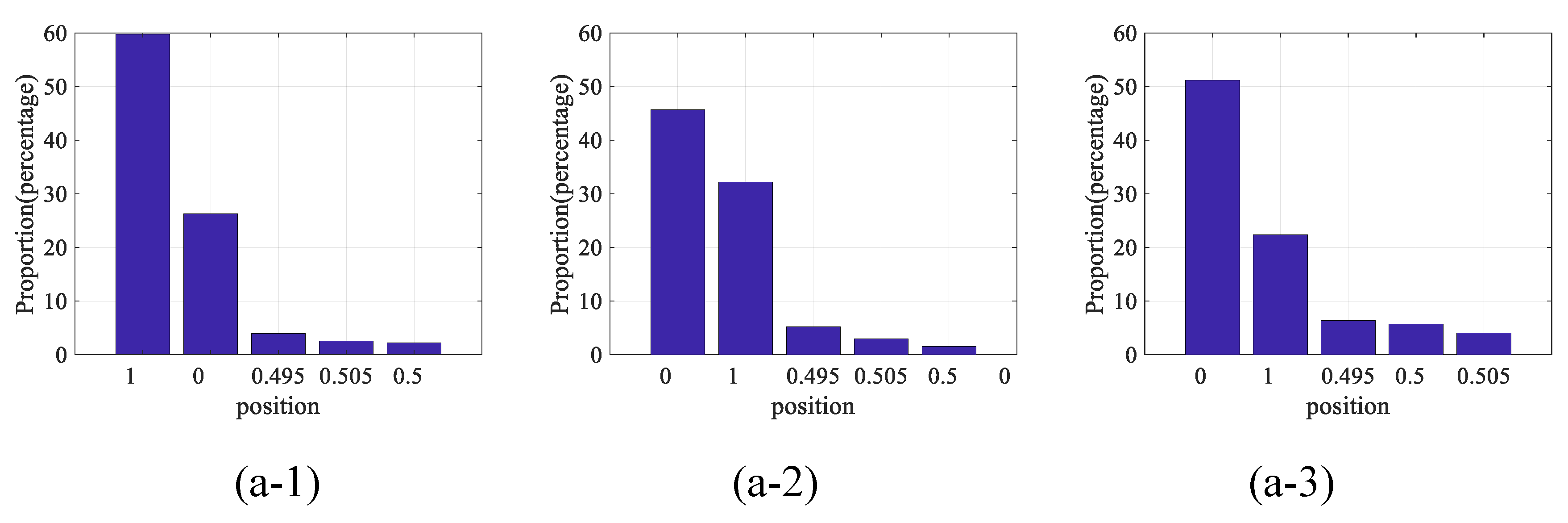
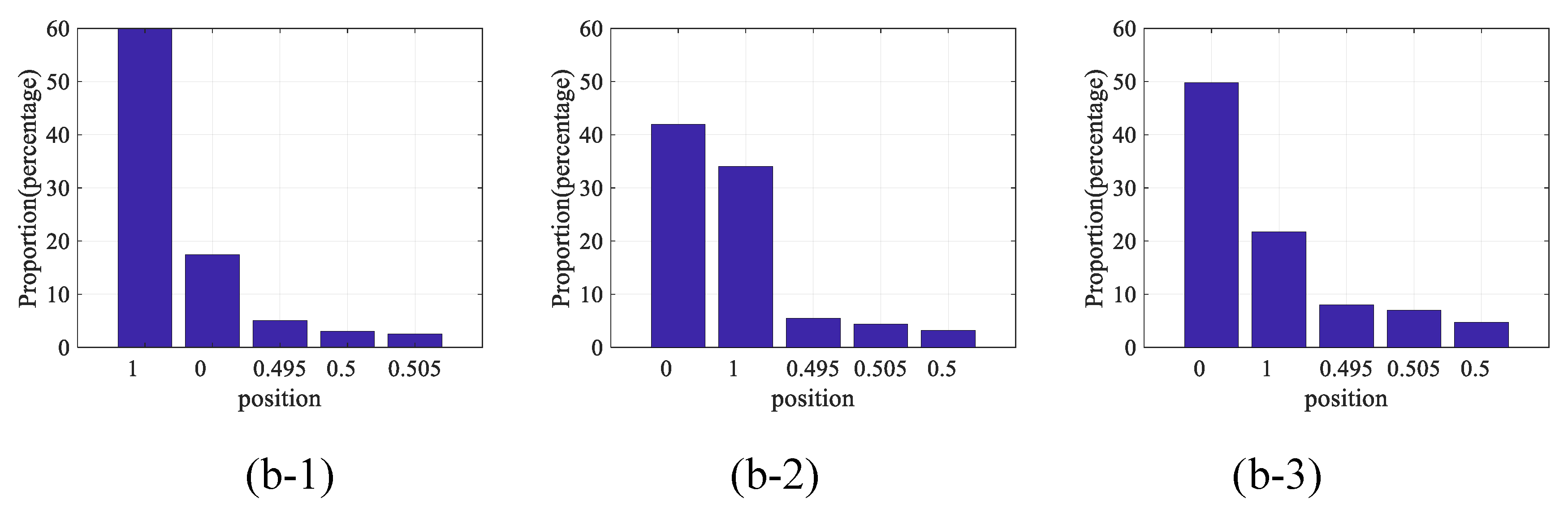
3.2.1. Different Tension Acting on Tensioned Pulley
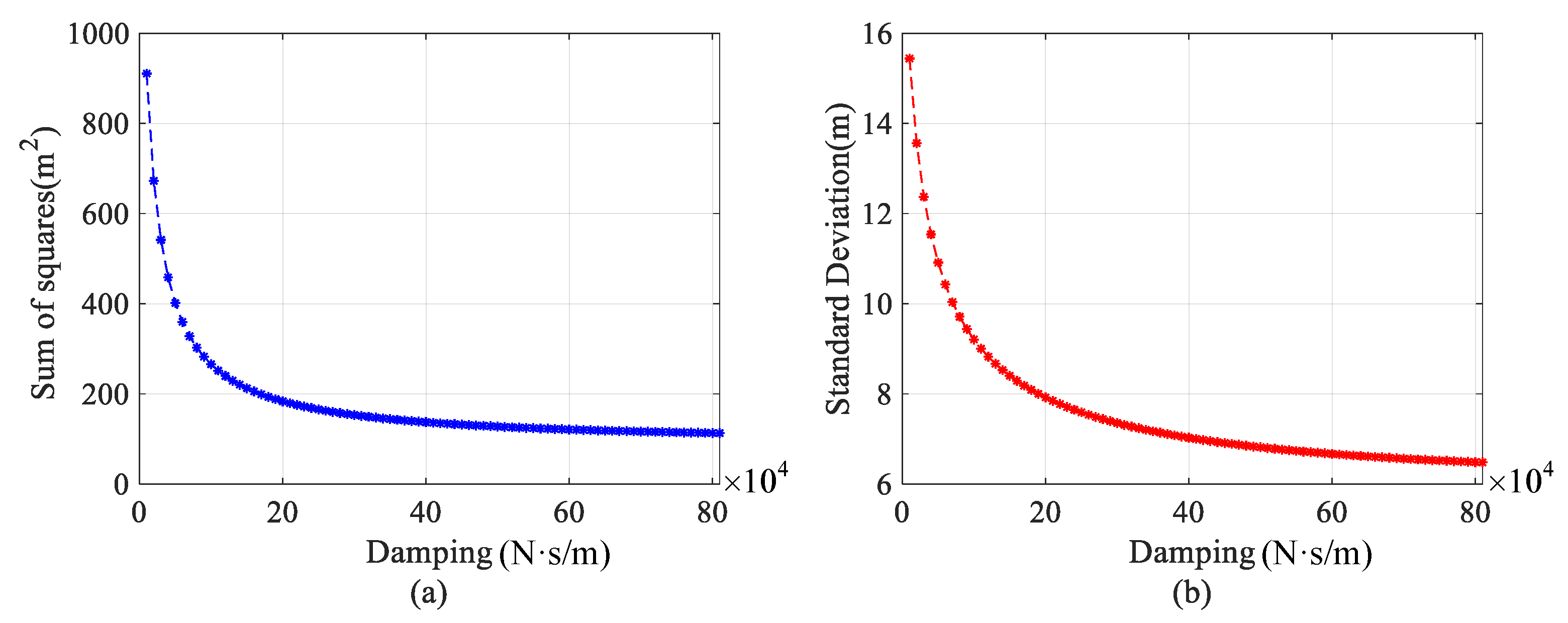
3.2.2. Different Damping Acting on Tensioned Pulley
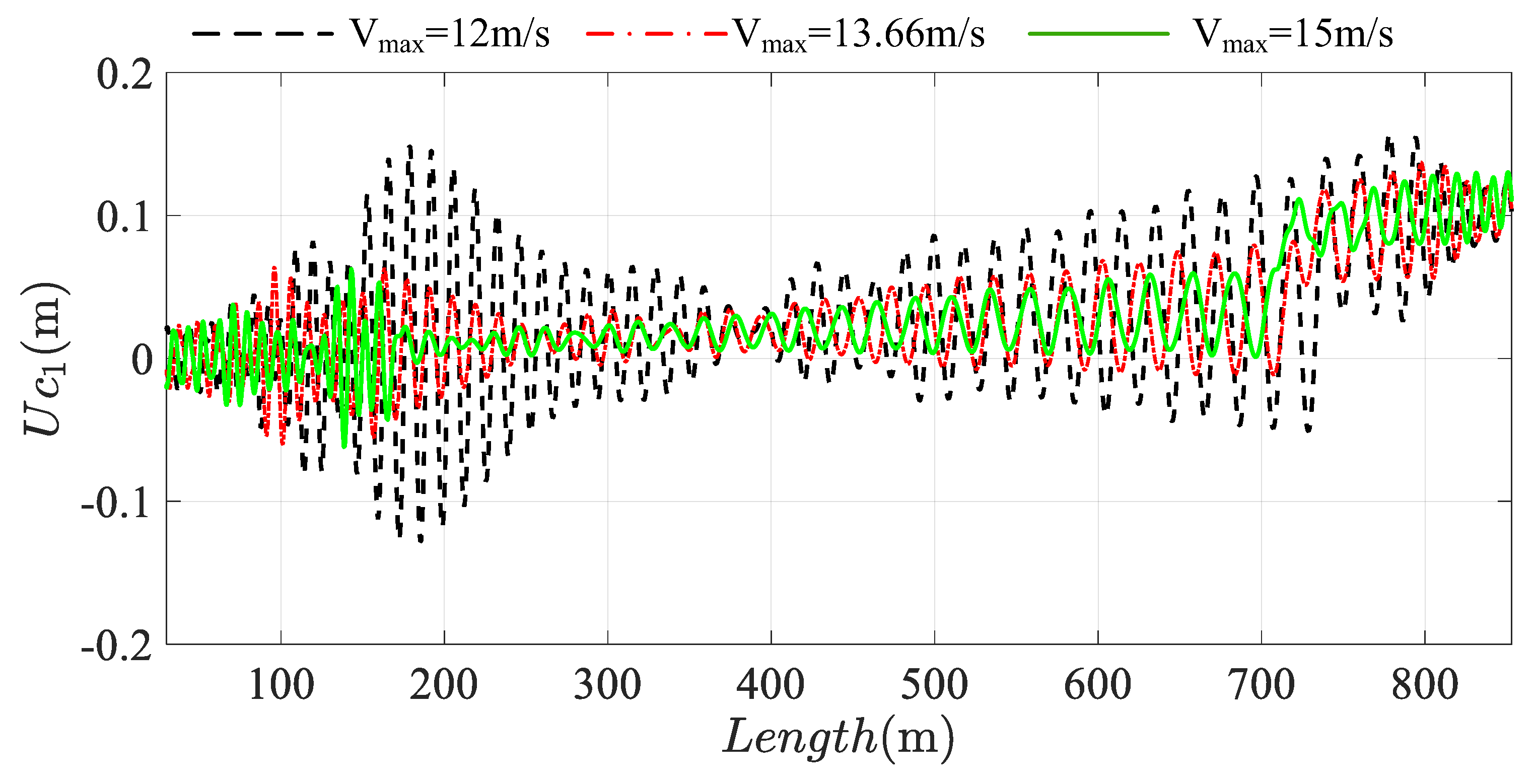
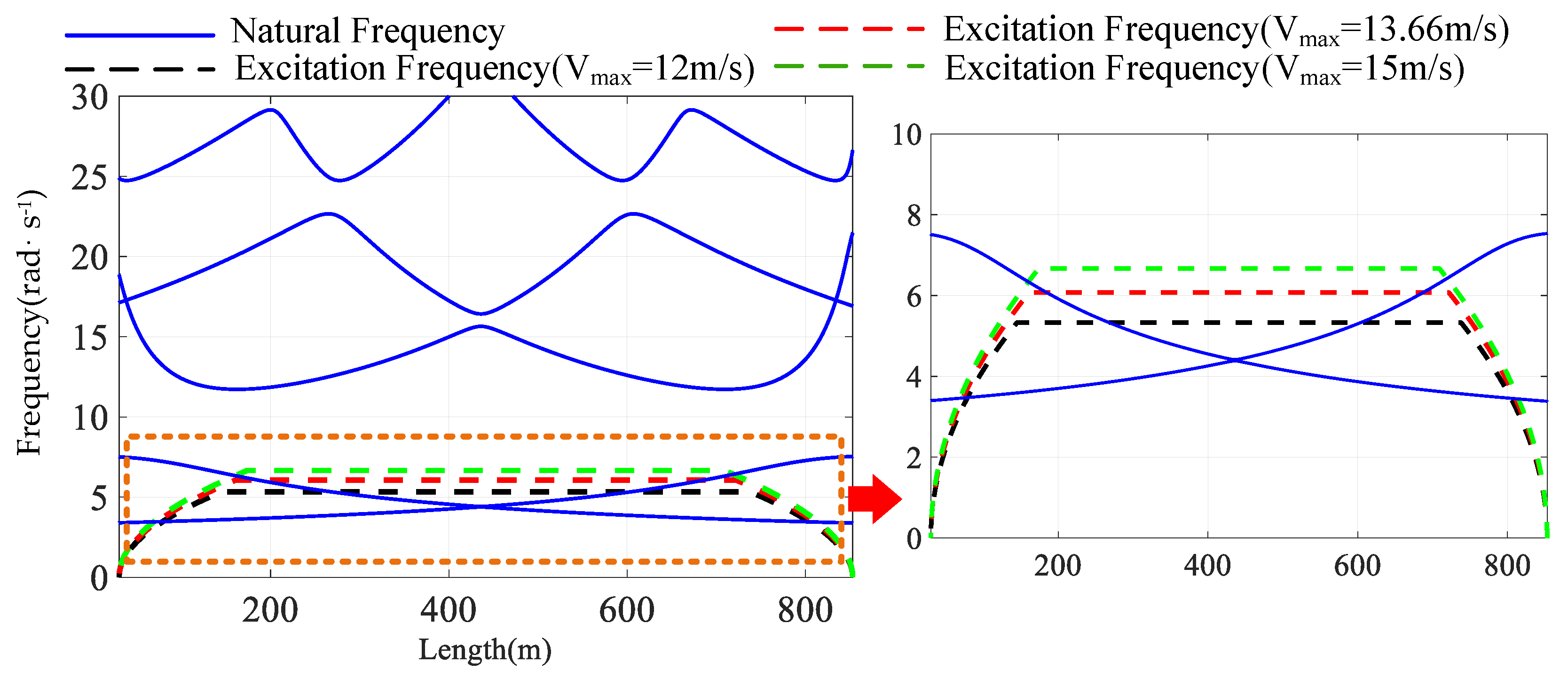
3.3. Discussion on Different Running Speed of Traction System
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Length of lifting and compensating ropes | Damping between tensioned pulley and ground | ||
| Velocity of lifting and compensating ropes | Preload working on tensioned pulley | ||
| Acceleration of lifting and compensating ropes | Connecting point between the lifting(compensating) ropes and conveyances | ||
| Total lifting height | Stiffness between conveyances and guide rails | ||
| Coordinate measurement of the lifting rope and compensating ropes | Damping between conveyances and guide rails | ||
| Tangent points between the compensating ropes and tensioned pulley | Longitudinal interaction forces between the conveyances and lifting(compensating) ropes | ||
| () | Mass and moment of inertia of tensioned pulley | Transverse interaction forces between the conveyances and lifting(compensating) ropes | |
| Radius of the tensioned pulley | Interaction forces between the tensioned pulley and compensating ropes | ||
| Longitudinal vibration of conveyances | Transverse vibration of conveyances | ||
| Longitudinal vibration of ropes | Transverse vibration of ropes | ||
| Longitudinal vibration of tensioned pulley | Rotation vibration of tensioned pulley |
Appendix A
Appendix B
Appendix C
Appendix D
Appendix E
References
- Li, J. Dynamics of Electric Pulley Conveying Cableway System in Banana Plantation. Nongye Jixie Xuebao/Trans. Chin. Soc. Agric. Mach. 2013, 44, 211–216. [Google Scholar]
- Feng, H.; Zhou, C.; Zhou, X.; Zhilong, W.U. Bouncing vibration response analysis of the cargo unhooking of single span aerial cableway. Mech. Eng. 2014, 36, 190–194. [Google Scholar]
- Pi, Y.; Ouyang, H. Vibration control of beams subjected to a moving mass using a successively combined control method. Appl. Math. Model. 2016, 40, 4002–4015. [Google Scholar] [CrossRef]
- Liu, Y.; Fang, G.; Xiuyu, H.; Qing, H. Boundary Control for an Axially Moving System with Input Restriction Based on Disturbance Observers. IEEE Trans. Syst. Man Cybern. Syst. 2018, 49, 1–12. [Google Scholar] [CrossRef]
- Nguyen, Q.C.; Hong, K.-S. Transverse Vibration Control of Axially Moving Membranes by Regulation of Axial Velocity. IEEE Trans. Control Syst. Technol. 2011, 20, 1124–1131. [Google Scholar] [CrossRef]
- Kaczmarczyk, S.; Ostachowicz, W. Transient vibration phenomena in deep mine hoisting cables. Part 1: Mathematical model. J. Sound Vib. 2003, 262, 219–244. [Google Scholar] [CrossRef]
- Kaczmarczyk, S.; Ostachowicz, W. Transient vibration phenomena in deep mine hoisting cables. Part 2: Numerical simulation of the dynamic response. J. Sound Vib. 2003, 262, 245–289. [Google Scholar] [CrossRef]
- Cao, G.H.; Wang, J.J.; Zhu, Z.C. Coupled vibrations of rope-guided hoisting system with tension difference between two guiding ropes, ARCHIVE Proceedings of the Institution of Mechanical Engineers Part C. J. Mech. Eng. Sci. 2018, 232, 231–244. [Google Scholar] [CrossRef]
- Wang, J.J.; Cao, G.H.; Zhu, Z.C. Lateral and torsional vibrations of cable-guided hoisting system with eccentric load. J. Vibroengineering 2016, 18, 3524–3538. [Google Scholar]
- Bao, J.H. Dynamics Modeling and Vibration Control of High-Speed Elevator Hoisting System; Shanghai Jiao Tong University: Shanghai, China, 2014. [Google Scholar]
- Bao, J.H.; Zhang, P.; Zhu, C.M. Modeling and control of longitudinal vibration on flexible hoisting systems with time-varying length. Procedia Eng. 2011, 15, 4521–4526. [Google Scholar]
- Bao, J.H.; Zhang, P.; Zhu, C.M.; Zhu, M.; Jin, L.Q.; Xie, H.X. Vibration control of high-speed elevator hoisting systems based on tensioning devices. J. Vib. Shock 2017, 36, 221–226. [Google Scholar]
- Zhang, P. Theoretic and Test Research on Dynamic Behaviors of High-Speed Elevator Suspended System; Shanghai Jiao Tong University: Shanghai, China, 2007. [Google Scholar]
- Arrasate, X.; Kaczmarczyk, S.; Almandoz, G.; Abete, J.M.; Isasa, I. The modelling, simulation and experimental testing of the dynamic responses of an elevator system. Mech. Syst. Signal Process. 2014, 42, 258–282. [Google Scholar] [CrossRef]
- Zhu, W.D.; Ren, H. An Accurate Spatial Discretization and Substructure Method with Application to Moving Elevator Cable-Car Systems—Part I: Methodology. J. Vib. Acoust. 2013, 135, 051036. [Google Scholar] [CrossRef]
- Arnold, M.; Brüls, O. Convergence of the generalized- α scheme for constrained mechanical systems. Multibody Syst. Dyn. 2007, 18, 185–202. [Google Scholar] [CrossRef]
- Brüls, O.; Arnold, M. The Generalized-α Scheme as a Linear Multistep Integrator: Toward a General Mechatronic Simulator. J. Comput. Nonlinear Dyn. 2018, 3, 041007. [Google Scholar] [CrossRef]
- Brüls, O.; Golinval, J.C. On the numerical damping of time integrators for coupled mechatronic systems. Comput. Methods Appl. Mech. Eng. 2008, 197, 577–588. [Google Scholar] [CrossRef]
- Brüls, O.; Golinval, J.C. The generalized-α method in mechatronic applications, ZAMM. J. Appl. Math. Mech. 2010, 86, 748–758. [Google Scholar]
- Otsuki, M.; Ushijima, Y.; Yoshida, K.; Kimura, H.; Nakagawa, T. Application of Nonstationary Sliding Mode Control to Suppression of Transverse Vibration of Elevator Rope Using Input Device with Gaps. JSME 2006, 49, 385–394. [Google Scholar] [CrossRef][Green Version]
- Wang, N.; Cao, G.; Yan, L.; Wang, L. Modeling and Control for a Multi-Rope Parallel Suspension Lifting System under Spatial Distributed Tensions and Multiple Constraints. Symmetry 2018, 10, 412. [Google Scholar] [CrossRef]
- Wang, L.; Cao, G.; Wang, N.; Yan, L. Modeling and Dynamic Behavior Analysis of Rope-Guided Traction System with Terminal Tension Acting on Compensating Rope. Shock Vib. 2019, 2019, 24. [Google Scholar] [CrossRef]
- Crespo, R.S.; Kaczmarczyk, S.; Picton, P.; Su, H. Modelling and simulation of a stationary high-rise elevator system to predict the dynamic interactions between its components. Int. J. Mech. Sci. 2018, 137, 24–45. [Google Scholar] [CrossRef]
- Watanabe, S.; Okawa, T.; Nakazawa, D.; Fukui, D. Vertical vibration analysis for elevator compensating sheave. J. Phys. Conf. Ser. 2013, 148, 012007. [Google Scholar] [CrossRef]
- Zhu, W.D.; Ni, J. Energetics and Stability of Translating Media with an Arbitrarily Varying Length. J. Vib. Acoust. 2000, 122, 295–304. [Google Scholar] [CrossRef]
- Wu, J.; Kou, Z.-M.; Liang, M. Transverse dynamics analysis of rope in multi-rope friction hoisting system. J. Vib. Shock 2016, 35, 184–188. [Google Scholar]
- Chang, Y. Study on Health Status Monitoring System of Multi Rope Friction Hoisting System; China University of Mining and Technology: Xuzhou, China, 2017. [Google Scholar]
- Ren, H. Accurate Simulation of the Dynamics of Elevator Systems; University of Maryland, Baltimore County: Baltimore, MD, USA, 2011. [Google Scholar]



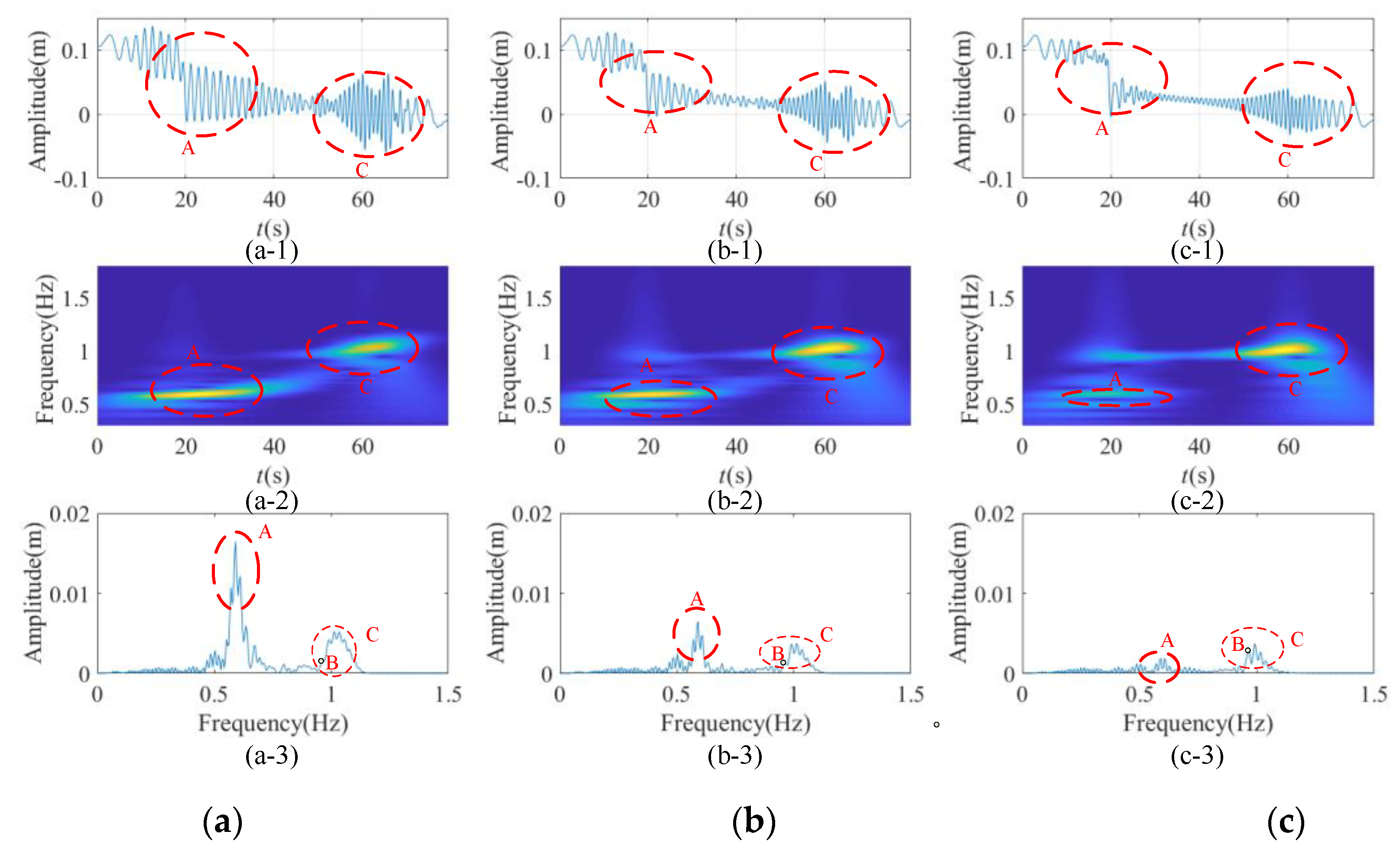

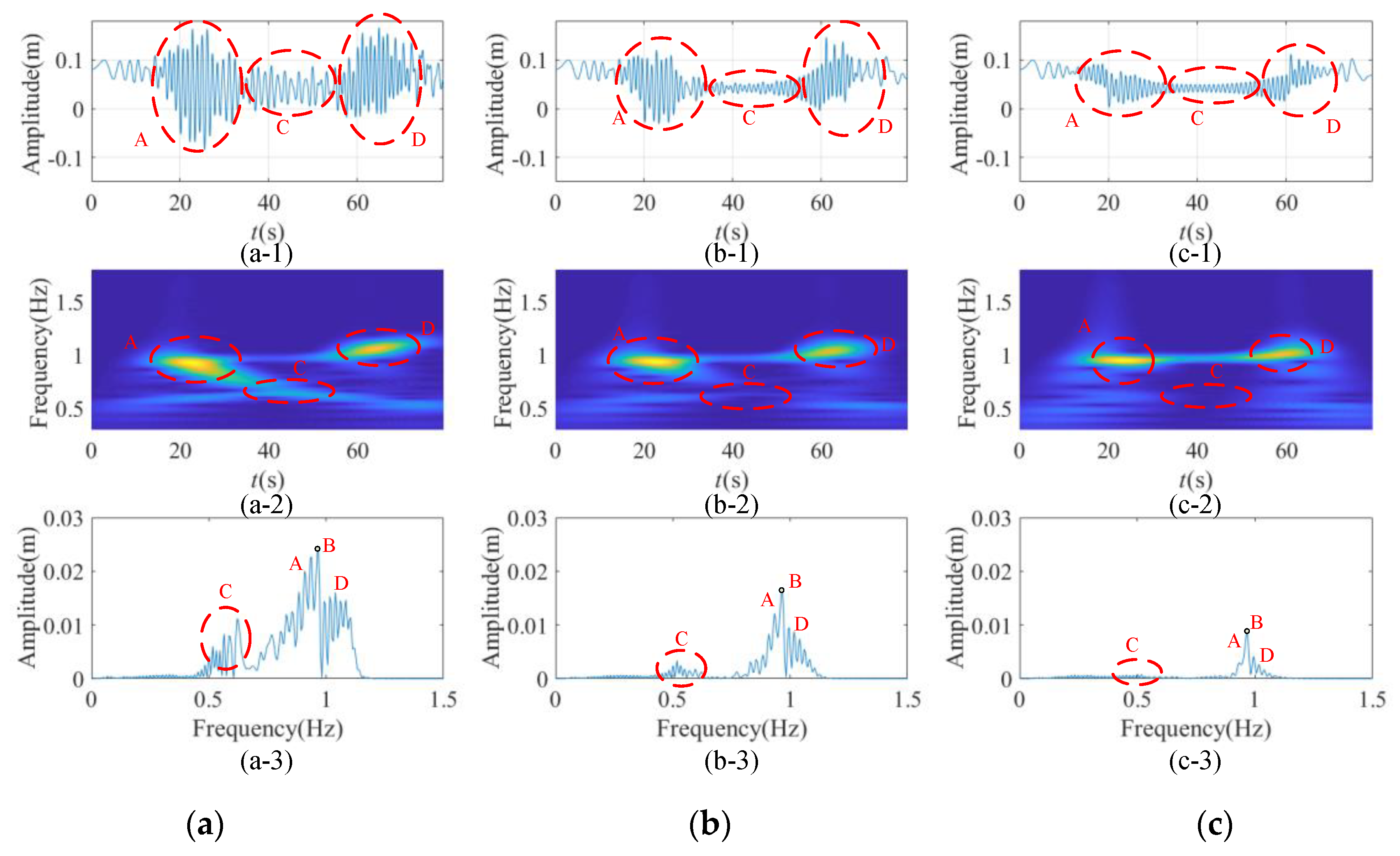
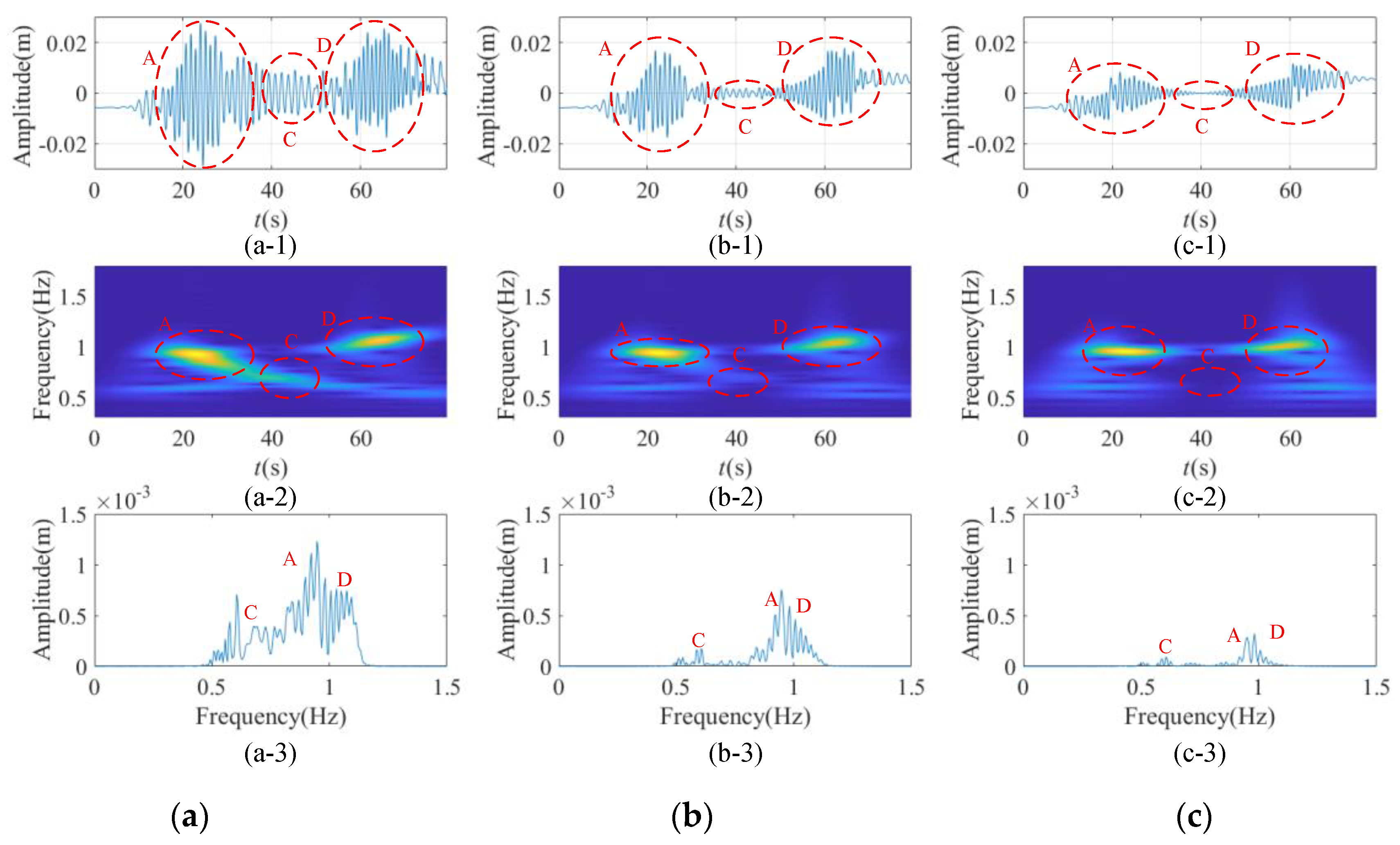
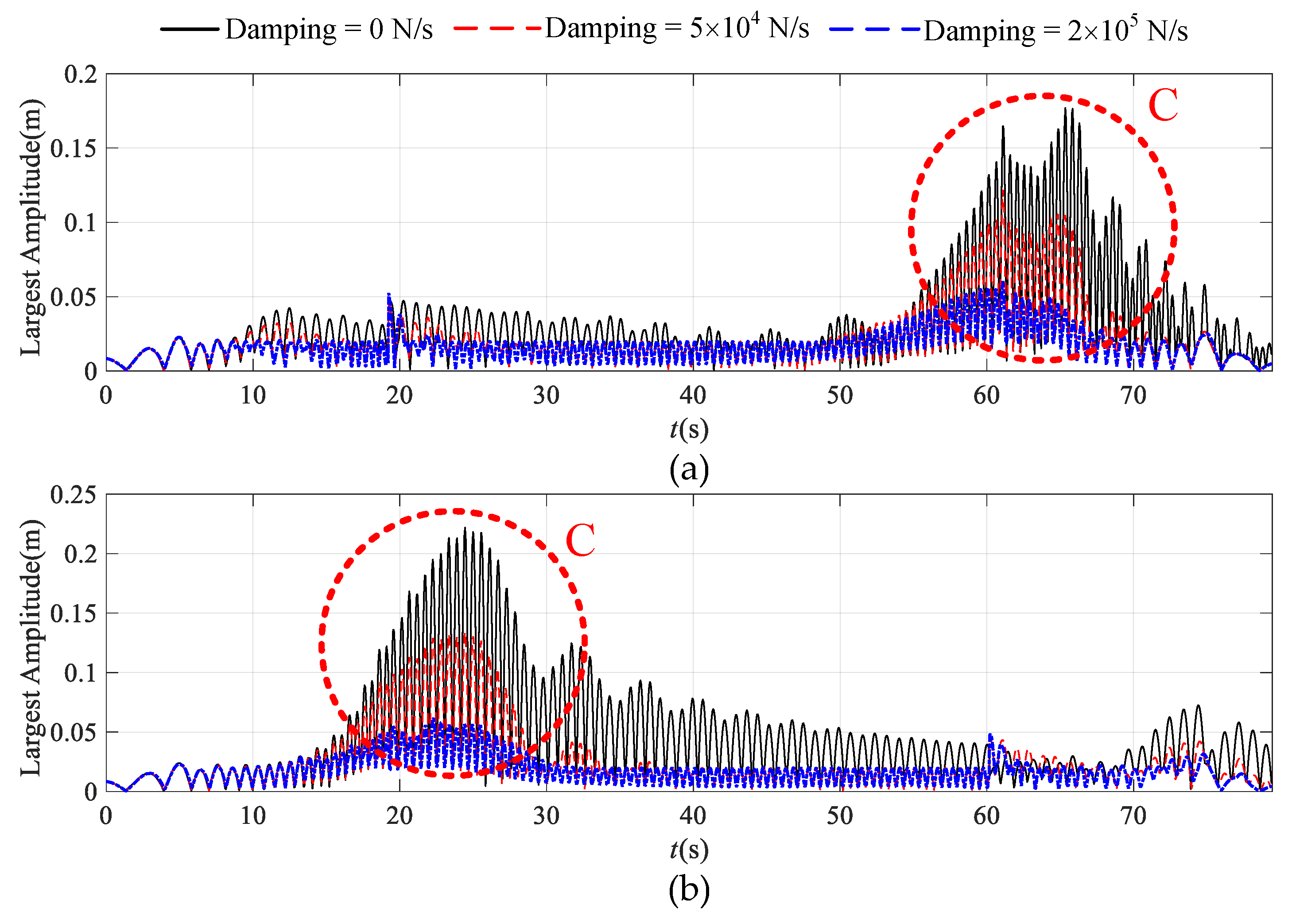





| Parameter. | Description | Value |
|---|---|---|
| () | The linear density of lifting(compensating) rope | |
| N | The number of ropes | 4 |
| () | The mass of bilateral conveyances | |
| The elastic modulus of ropes | ||
| The diameter of ropes | ||
| The mass of tensioned pulley | 4 t | |
| The radius of tensioned pulley | 2.25 m | |
| The stiffness between conveyances and guide | ||
| The damping between conveyances and guide | ||
| The initial lengths of the left lifting ropes | 853 m | |
| The final lengths of the left lifting ropes | 30 m |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Cao, G.; Wang, N.; Zhang, Y. Dynamic Behavior Analysis of a High-Rise Traction System with Tensioned Pulley Acting on Compensating Rope. Symmetry 2020, 12, 129. https://doi.org/10.3390/sym12010129
Wang L, Cao G, Wang N, Zhang Y. Dynamic Behavior Analysis of a High-Rise Traction System with Tensioned Pulley Acting on Compensating Rope. Symmetry. 2020; 12(1):129. https://doi.org/10.3390/sym12010129
Chicago/Turabian StyleWang, Lei, Guohua Cao, Naige Wang, and Yunchang Zhang. 2020. "Dynamic Behavior Analysis of a High-Rise Traction System with Tensioned Pulley Acting on Compensating Rope" Symmetry 12, no. 1: 129. https://doi.org/10.3390/sym12010129
APA StyleWang, L., Cao, G., Wang, N., & Zhang, Y. (2020). Dynamic Behavior Analysis of a High-Rise Traction System with Tensioned Pulley Acting on Compensating Rope. Symmetry, 12(1), 129. https://doi.org/10.3390/sym12010129





