Lie Symmetry Analysis of Burgers Equation and the Euler Equation on a Time Scale
Abstract
1. Introduction
2. Preliminaries
- 1.
- The forward jump operator is defined by
- 2.
- The backward jump operator is defined by
- 3.
- The graininess function is defined by
- 4.
- If , then t is right-scattered. If , then t is left-scattered. Points that are both right scattered and left-scattered are called isolated.
- 5.
- If and , then t is right-dense. If and , then t is left-dense. Points that are both right-dense and left-dense are called dense.
- 6.
- We set if has a maximum t, and if has a minimum t.
3. Main Results
3.1. Symmetry Analysis on a Time Scale
3.2. Lie Symmetry Analysis of the Burgers Equation on a Time Scale
- , satisfying .
- , is an arbitrary constant.
- , is an arbitrary constant.
- , satisfying .
- , is an arbitrary constant.
- .
- .
- .
- .
- ,
- .
- .
- .
- .
- ,
- Case1. .From the seed solution obtained with the help of Maple, we can obtain various solutions to Equation (3)
- (a)
- .
- (b)
- .
- (c)
- .
- (d)
- .
The respective solutions to the Burgers Equation (2) are- (a)
- .
- (b)
- .
- (c)
- .
- (d)
- ,
where is arbitrary constant. satisfying . satisfying . - Case2. .From the seed solution obtained with the help of Maple, we can obtain various solutions to Equation (3)
- (a)
- .
- (b)
- .
- (c)
- .
- (d)
and the respective solutions to the Burgers Equation (2)- (a)
- .
- (b)
- .
- (c)
- .
- (d)
- ,
where is arbitrary constant. satisfying . satisfying .
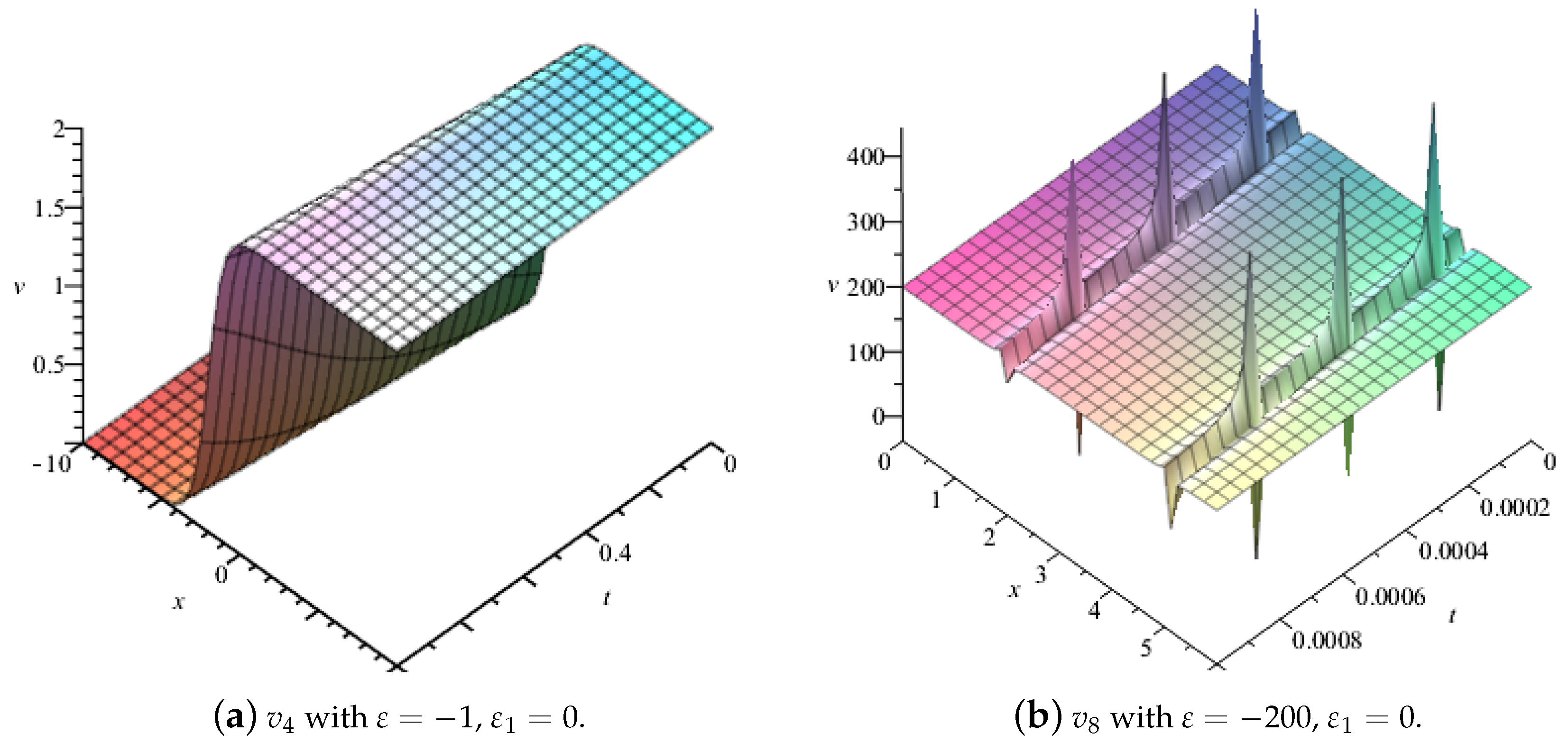
- 1.
- As , the -type smooth kink solution of the Burgers equation is obtained.
- 2.
- As , the singular kink solution of the Burgers equation is obtained, and the shock wave appears, which corresponds to the local worst traffic jam.
- 3.
- By data fitting and changing model parameters, the models for specific practical problems can be built, which can provide a theoretical basis for the prediction of traffic congestion.
3.3. Lie Symmetry Analysis of a Euler Equation with a Coriolis Force on a Time Scale
3.3.1. Lie Symmetry of Euler Equation with Coriolis Force on a Time Scale
- , satisfying .
- , satisfying .
- , is an arbitrary constant.
- , is an arbitrary constant.
- , is an arbitrary constant.
- , is an arbitrary constant.
- , is an arbitrary constant.
3.3.2. Exact Solutions to the Euler Equation with Coriolis Force on a Time Scale
- , is an arbitrary constant.
- , is an arbitrary constant.
- , is an arbitrary constant.
- , is an arbitrary constant.
- , is an arbitrary constant.
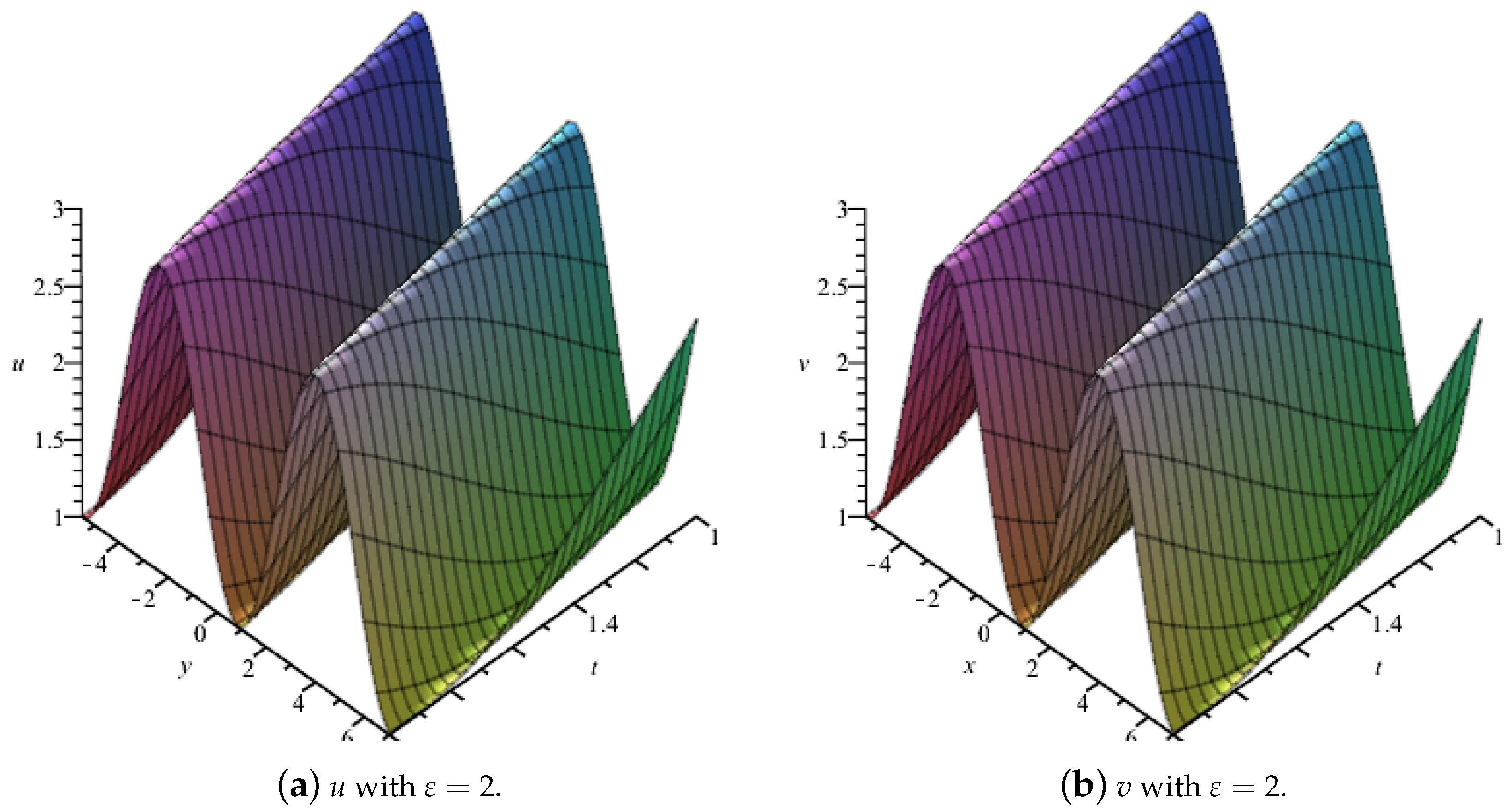
- We obtain the bell-shape single soliton solution to the Euler equation with Coriolis force. The vorticity (i.e., curl of the velocity) of the Euler flow iswhich shows the Euler flow with Coriolis force is a rotational flow.
- Using Equation (24) as a seed solution, various invariant solutions can be given with obtained in Section 3.2.
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bohner, M.; Peterson, A. Advances in Dynamic Equations on a Time Scale; Birkhauser: Boston, MA, USA, 2002. [Google Scholar]
- Bohner, M.; Peterson, A. Dynamic Equations on a Time Scale: An Introduction with Applications; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Hilger, S. Analysis on measure chains—A unified approach to continuous and discrete calculus. Results Math. 1990, 18, 18–56. [Google Scholar] [CrossRef]
- Agarwal, R.P.; O’Regan, D. Triple solutions to boundary value problems on a time scale. Appl. Math. Lett. 2000, 13, 7–11. [Google Scholar] [CrossRef][Green Version]
- Chyan, C.J. Uniqueness Implies Existence on Time Scales. J. Math. Anal. Appl. 2001, 258, 359–365. [Google Scholar] [CrossRef][Green Version]
- Peterson, A.C.; Tisdell, B.C.C. Boundedness and Uniqueness of Solutions to Dynamic Equations on Time Scales. J. Differ. Equ. Appl. 2004, 10, 1295–1306. [Google Scholar] [CrossRef]
- Hoffacker, J.; Tisdell, C.C. Stability and instability for dynamic equations on a time scale. Comput. Math. Appl. 2005, 49, 1327–1334. [Google Scholar] [CrossRef]
- Amster, P.; Rogers, C.; Tisdell, C.C. Existence of solutions to boundary value problems for dynamic systems on a time scale. J. Math. Anal. Appl. 2005, 308, 565–577. [Google Scholar] [CrossRef]
- Sun, H.; Li, W. Existence theory for positive solutions to one-dimensional p-Laplacian boundary value problems on a time scale. J. Differ. Equ. 2007, 240, 217–248. [Google Scholar] [CrossRef]
- Chen, H.; Wang, H.; Zhang, Q.; Zhou, T. Double positive solutions of boundary value problems for p-Laplacian impulsive functional dynamic equations on a time scale. Comput. Math. Appl. 2007, 53, 1473–1480. [Google Scholar] [CrossRef][Green Version]
- Zhang, J.; Fan, M.; Zhu, H. Existence and roughness of exponential dichotomies of linear dynamic equations on a time scale. Comput. Math. Appl. 2010, 59, 2658–2675. [Google Scholar] [CrossRef][Green Version]
- Federson, M.; Grau, R.; Mesquita, J.G.; Toon, E. Boundedness of solutions of measure differential equations and dynamic equations on a time scale. J. Differ. Equ. 2017, 263, 26–56. [Google Scholar] [CrossRef]
- Lou, S. Symmetry analysis and exact solutions of the (2+1)-dimensional sine-Gordon system. J. Math. Phys. 2000, 41, 6509–6524. [Google Scholar] [CrossRef]
- Tian, C. Lie Group and Its Application in Differential Equations; Science Press: Beijing, China, 2005. [Google Scholar]
- Liu, H.; Geng, Y. Symmetry reductions and exact solutions to the systems of carbon nanotubes conveying fluid. J. Differ. Equ. 2013, 254, 2289–2303. [Google Scholar] [CrossRef]
- Liu, H.; Li, J. Symmetry reductions, dynamical behavior and exact explicit solutions to the Gordon types of equations. J. Comput. Appl. Math. 2014, 257, 144–156. [Google Scholar] [CrossRef]
- Tiwari, A.K.; Pandey, S.N.; Senthilvelan, M.; Lakshmanan, M. Lie point symmetries classification of the mixed Liénard-type equation. Nonlinear Dynam. 2015, 82, 1953–1968. [Google Scholar] [CrossRef]
- Baleanu, D.; Inc, M.; Yusuf, A.; Aliyu, I.A. Lie symmetry analysis, exact solutions and conservation laws for the time fractional Caudrey-Dodd-Gibbon-Sawada-Kotera equation. Commun. Nonlinear Sci. 2018, 59, 222–234. [Google Scholar] [CrossRef]
- Burgers, J. The Nonlinear Diffusion Equation; Dordrecht: Amsterdam, The Netherlands, 1974. [Google Scholar]
- Smoller, J. Shock Waves and Reaction Diffusion Equations, 2nd ed.; Springer: Berlin, Germany, 1994. [Google Scholar]
- Biler, P.; Funaki, T.; Woyczynski, W.A. Fractal Burgers equations. J. Differ. Equ. 1998, 148, 9–46. [Google Scholar] [CrossRef]
- Pettersson, P.; Iaccarino, G.; Nordström, J. Numerical analysis of the Burgers’ equation in the presence of uncertainty. J. Comput. Phys. 2009, 228, 8394–8412. [Google Scholar] [CrossRef]
- Neate, A.; Truman, A. The stochastic Burgers equation with vorticity: Semiclassical asymptotic series solutions with applications. J. Math. Phys. 2011, 52, 185–383. [Google Scholar] [CrossRef]
- Heinz, S. Comments on a priori and a posteriori evaluations of sub-grid scale models for the Burgers’ equation. Comput. Fluids 2016, 138, 35–37. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Fluid Mechanics; Pergamon Press: New York, NY, USA, 1987. [Google Scholar]
- Majda, A.J.; Majda, A.J.; Bertozzi, A.L. Vorticity and Incompressible Flow; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Jia, M.; Chen, L.; Lou, S.Y. Relation between the induced flow and the position of typhoon: Chanchu 2006. Chin. Phys. Lett. 2006, 23, 2878. [Google Scholar]
- Koh, Y.; Lee, S.; Takada, R. Strichartz estimates for the Euler equations in the rotational framework. J. Differ. Equ. 2014, 256, 707–744. [Google Scholar] [CrossRef]
- Olver, P.J. Applications of Lie Groups to Differential Equations, 2nd ed.; Graduate Tests in Mathematics; Springer: Berlin, Germany, 1993; Volume 107. [Google Scholar]

| 0 | 0 | 0 | |||
| 0 | 0 | 0 | − | ||
| 0 | 0 | 0 | 0 | 0 | |
| 0 | 0 | ||||
| 0 | 0 |
| Ad | |||||
|---|---|---|---|---|---|
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, M.; Dong, H.; Fang, Y.; Zhang, Y. Lie Symmetry Analysis of Burgers Equation and the Euler Equation on a Time Scale. Symmetry 2020, 12, 10. https://doi.org/10.3390/sym12010010
Liu M, Dong H, Fang Y, Zhang Y. Lie Symmetry Analysis of Burgers Equation and the Euler Equation on a Time Scale. Symmetry. 2020; 12(1):10. https://doi.org/10.3390/sym12010010
Chicago/Turabian StyleLiu, Mingshuo, Huanhe Dong, Yong Fang, and Yong Zhang. 2020. "Lie Symmetry Analysis of Burgers Equation and the Euler Equation on a Time Scale" Symmetry 12, no. 1: 10. https://doi.org/10.3390/sym12010010
APA StyleLiu, M., Dong, H., Fang, Y., & Zhang, Y. (2020). Lie Symmetry Analysis of Burgers Equation and the Euler Equation on a Time Scale. Symmetry, 12(1), 10. https://doi.org/10.3390/sym12010010




