The Perceived Beauty of Regular Polygon Tessellations
Abstract
1. Introduction
2. Experiment 1
2.1. Participants
2.2. Stimuli
2.3. Procedure
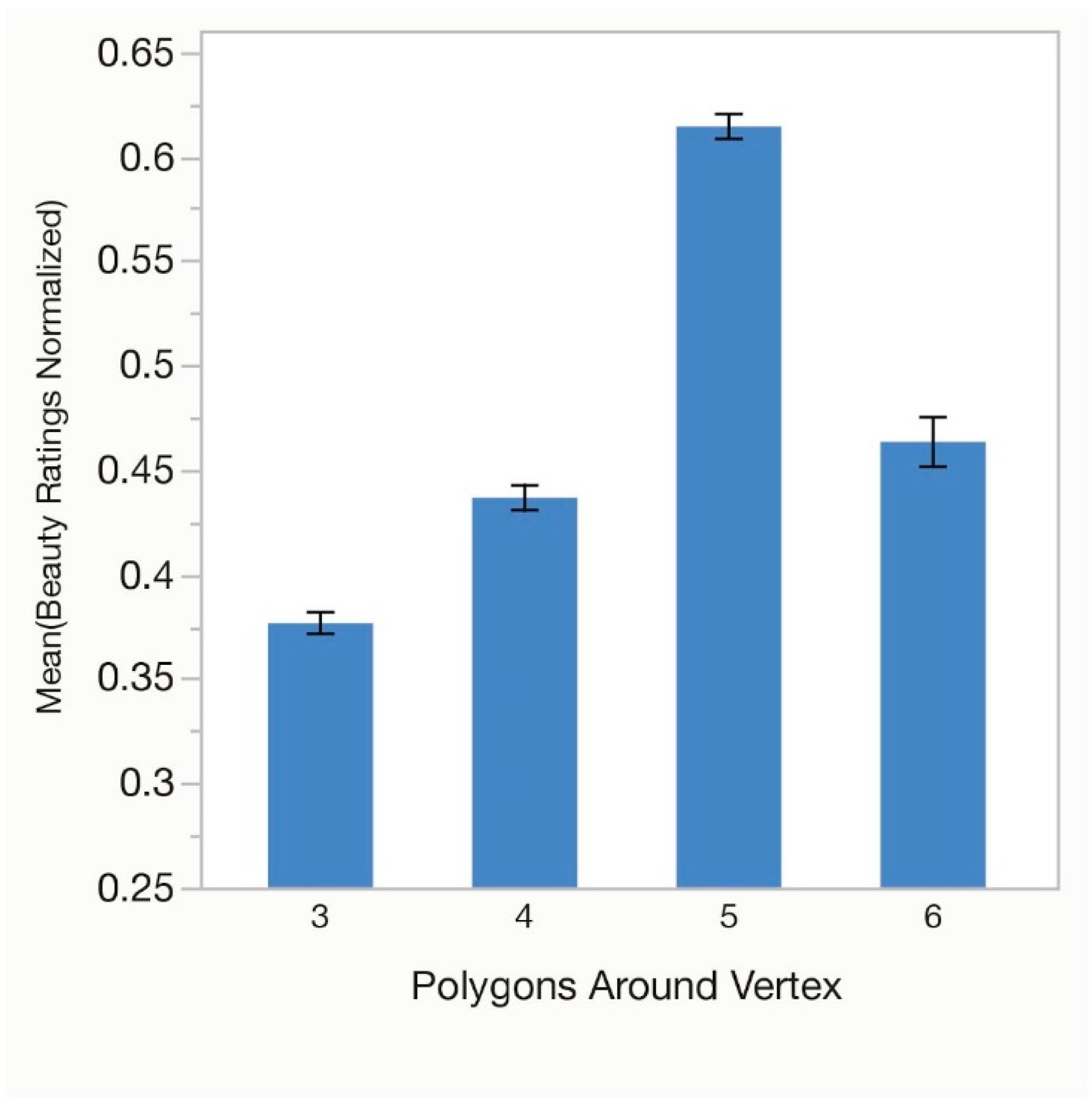
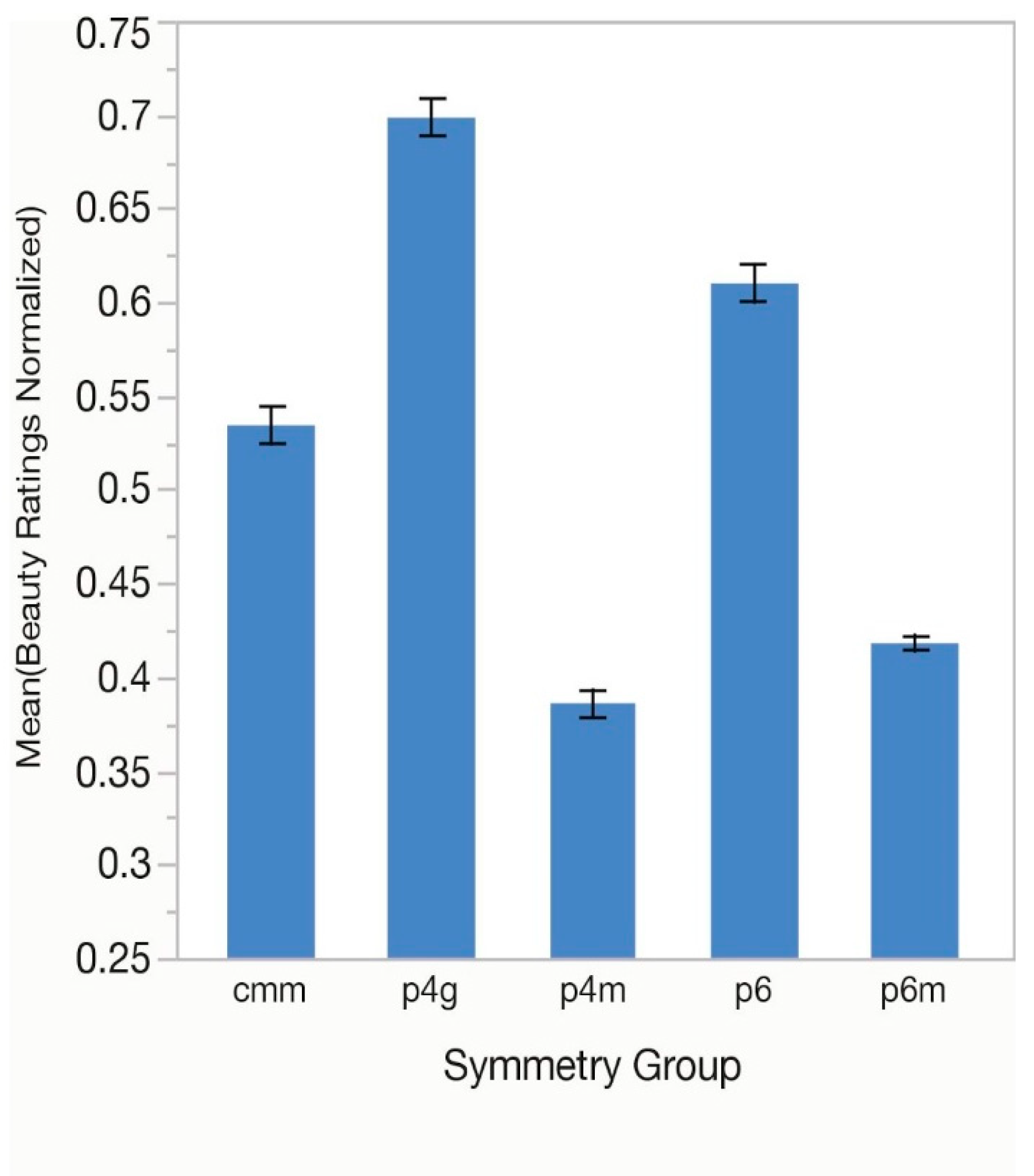
2.4. Results and Discussion
3. Experiment 2
3.1. Participants
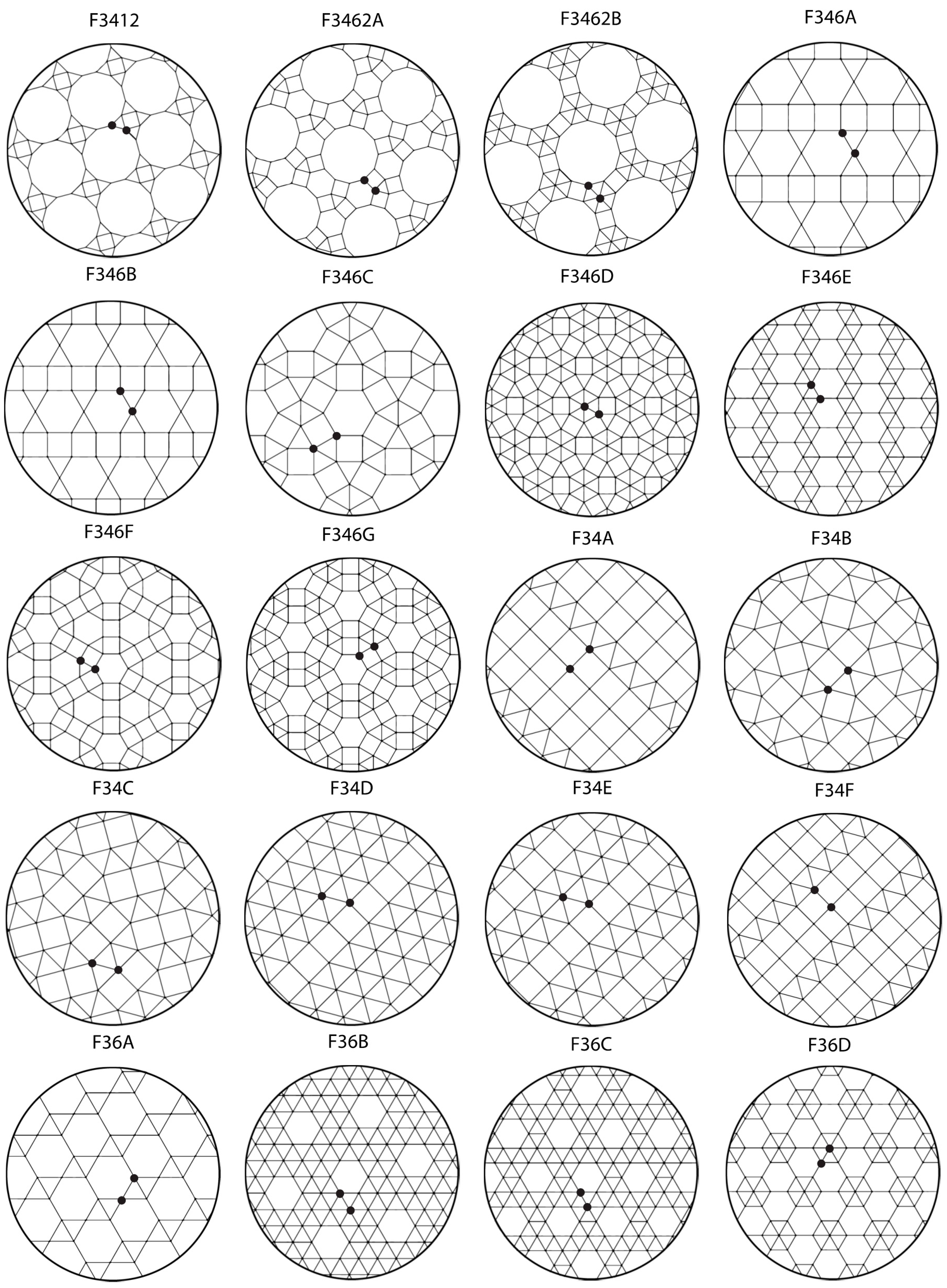
3.2. Stimuli
3.3. Procedure
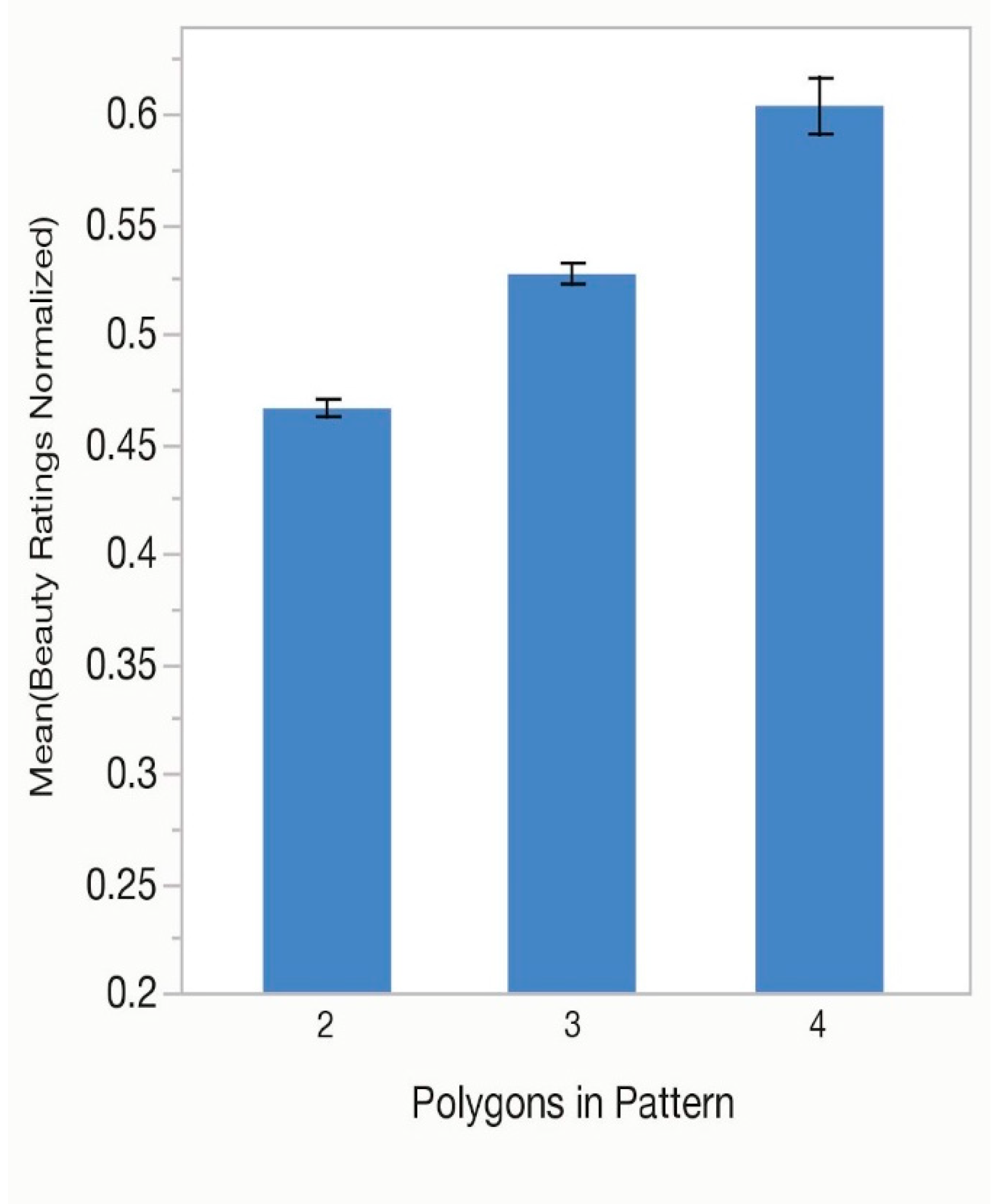
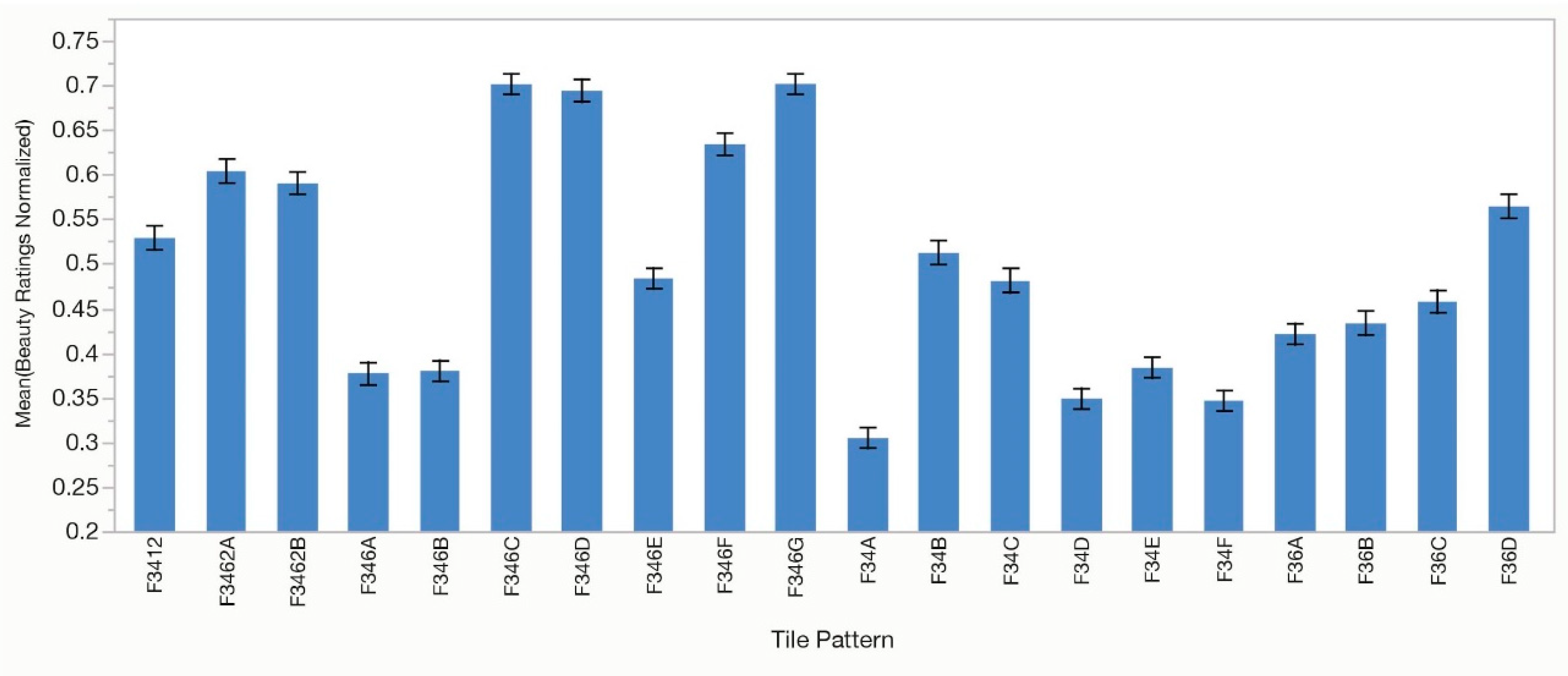
3.4. Results and Discussion
4. Conclusions
Funding
Conflicts of Interest
References
- Weil, C.; Weil, T. Geometric Ornament in Architecture, Art, and Design; Schiffer Publishing: Atglen, PA, USA, 2009. [Google Scholar]
- Washburn, D.K.; Crowe, D.W. Symmetries of Culture. Theory and Practice of Plane Pattern Analysis; University of Washington Press: Seattle, WA, USA, 1988. [Google Scholar]
- Washburn, D.K.; Crowe, D.W. Symmetry Comes of Age: The Role of Pattern in Culture; University of Washington Press: Seattle, WA, USA, 2004. [Google Scholar]
- Beyer, J. The Secrets of Interlocking Patterns: Designing Tessellations; Contemporary Books: Chicago, IL, USA, 1999. [Google Scholar]
- Seymour, D.; Britton, J. Introduction to Tessellations; Dale Seymour Publications: Palo Alto, CA, USA, 1989. [Google Scholar]
- Kinsey, L.C.; Moore, T.E. Symmetry, Shape, and Space: An Introduction to Mathematics Through Geometry; Springer/Key College Publishing: New York, NY, USA, 2002. [Google Scholar]
- Stevens, P.S. Handbook of Regular Patterns: An Introduction to Symmetry in Two Dimensions; MIT Press: Cambridge, MA, USA, 1981. [Google Scholar]
- Arnheim, R. Art and Visual Perception. A Psychology of the Creative Eye; University of California Press: Berkeley, CA, USA, 1974. [Google Scholar]
- Berlyne, D.E. Studies in the New Experimental Aesthetics: Steps Toward an Objective Psychology of Aesthetic Appreciation; Wiley: New York, NY, USA, 1974. [Google Scholar]
- Birkhoff, G.D. Aesthetic Measure; Harvard University Press: Cambridge, MA, USA, 1932. [Google Scholar]
- Day, H. Evaluation of subjective complexity, pleasingness and interestingness for a series of random polygons varying in complexity. Percept. Psychophys. 1967, 2, 281–286. [Google Scholar] [CrossRef]
- Fechner, G. Vorschule der Aesthetic; Breitkopf und Hartel: Leipzig, Germany, 1876. [Google Scholar]
- Valentine, C.W. An Introduction to the Experimental Psychology of Beauty; Nelson & Sons: Edinburgh, UK, 1919. [Google Scholar]
- Friedenberg, J. Geometric regularity, symmetry and the perceived beauty of simple shapes. Empir. Stud. Arts 2018, 36, 71–89. [Google Scholar] [CrossRef]
- Jacobsen, T.; Höfel, L. Aesthetic judgments of novel graphic patterns: Analyses of individual judgments. Percept. Mot. Ski. 2002, 95, 755–766. [Google Scholar] [CrossRef] [PubMed]
- McManus, I.C.; Cook, R.; Hunt, A. Beyond the Golden Section and normative aesthetics: Why do individuals differ so much in their aesthetic preferences for rectangles? Psychol. Aesthet. 2010, 4, 113–126. [Google Scholar] [CrossRef]
- Westphal-Fitch, G.; Fitch, W.T. Beauty for the eye of the beholder: Plane pattern perception and production. Psychol. Aesthet. 2017, 11, 451–456. [Google Scholar] [CrossRef]
- Westphal-Fitch, G.; Fitch, W.T. Spatial analysis of “crazy quilts,” a class of potentially random aesthetic artefacts. PLoS ONE 2013, 8, e74005. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Gombrich, E.H. The Sense of Order: A Study in the Psychology of Decorative Art; Phaidon Press: London, UK, 1984. [Google Scholar]
- Eberle, R.S. Children’s Mathematical Understandings of Tessellations: A Cognitive and Esthetic Synthesis. Dissertation Abstracts International Section A: Humanities and Social Sciences; ProQuest Information & Learning: Ann Arbor, MI, USA, 2011. [Google Scholar]
- Grünbaum, B.; Shephard, G.C. Tilings and Patterns; W.H. Freeman and Company: New York, NY, USA, 1987. [Google Scholar]
- Nadal, M.; Munar, E.; Marty, G.; Cela-Conde, C.J. Visual complexity and beauty appreciation: Explaining the divergence of results. Empir. Stud. Arts 2010, 28, 173–191. [Google Scholar] [CrossRef]
- Cutting, J.E.; Garvin, J.J. Fractal curves and complexity. Percept. Psychophys. 1987, 42, 365–370. [Google Scholar] [CrossRef] [PubMed]
- Bies, A.J.; Blanc-Goldhammer, D.R.; Boydston, C.R.; Taylor, R.P.; Sereno, M.E. Aesthetic responses to exact fractals driven by physical complexity. Front. Hum. Neurosci. 2016, 10, 1–17. [Google Scholar] [CrossRef] [PubMed]
- Coxeter, H.S.M. Regular Polytopes, 3rd ed.; Dover Publications: Mineola, NY, USA, 1973. [Google Scholar]
- Escher, M.C. Escher: His Life and Complete Graphic Work; Locher, J.L., Ed.; Abrams: New York, NY, USA, 1982. [Google Scholar]
- Golomb, S.W. Polyominoes. Puzzles, Patterns, Problems, and Packings, 2nd ed.; Princeton University Press: Princeton, NJ, USA, 1994. [Google Scholar]
- Penrose, R. Pentaplexity. Eureka 1978, 39, 16–22. [Google Scholar]
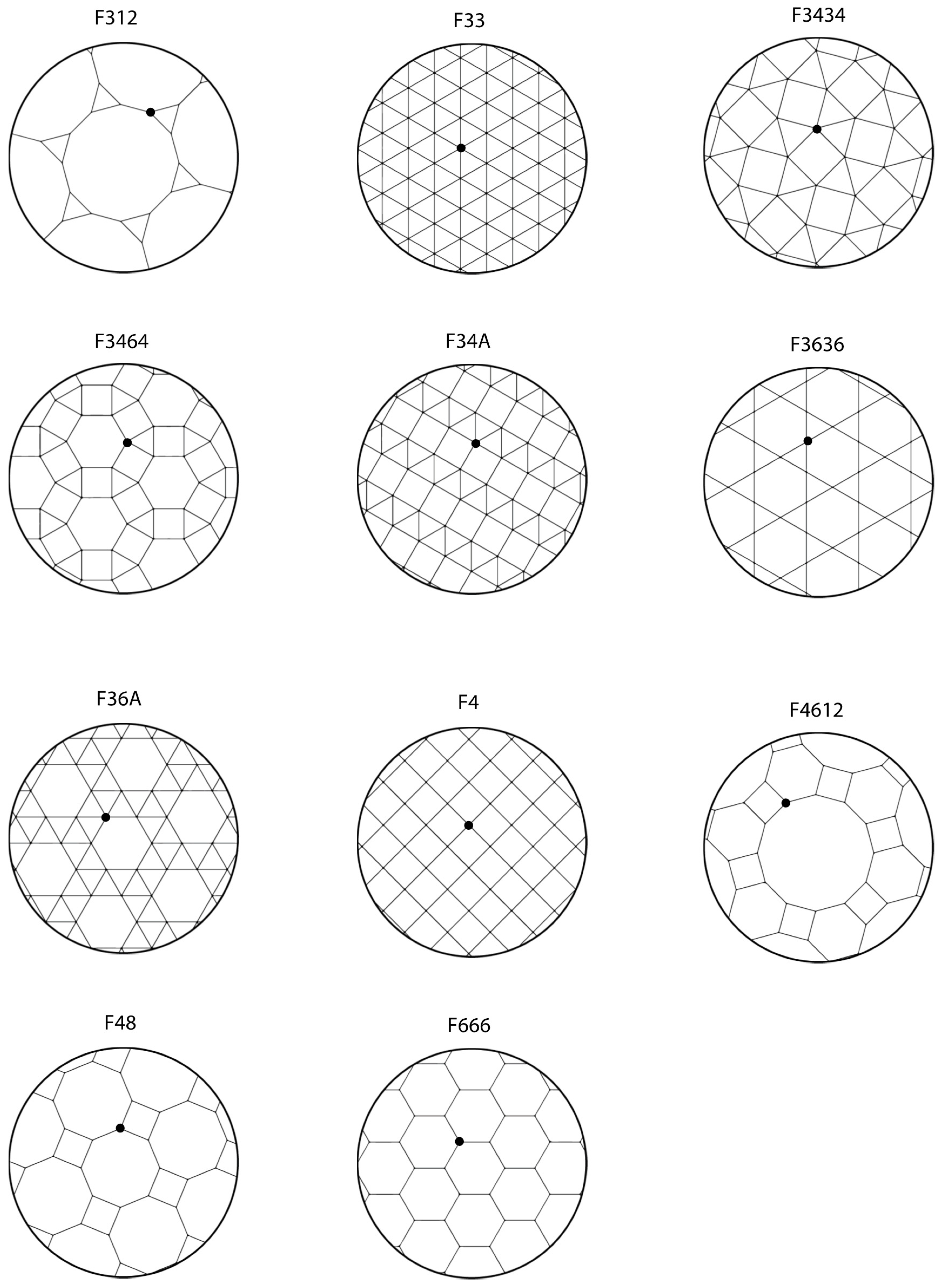

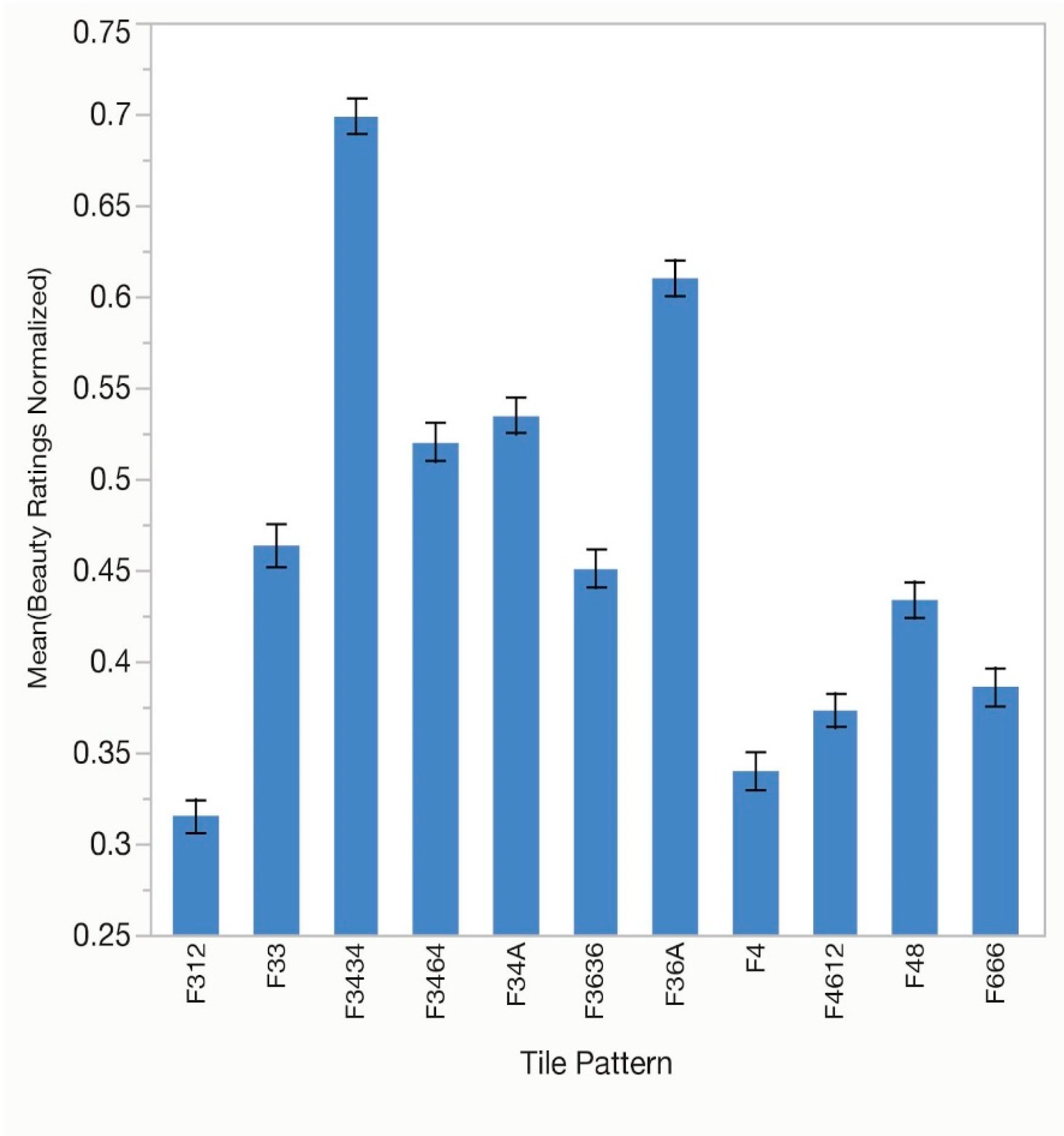


| File Notation | Standard Notation | Expanded Notation | Symmetry Group | Polygon Types | Polygons in Pattern | Polygons around Vertex | Preference Ranking |
|---|---|---|---|---|---|---|---|
| F3434 | 32.4.3.4 | 3.3.4.3.4 | p4g | TS | 2 | 5 | 1 |
| F36A | 34.6 | 3.3.3.3.6 | p6 | TH | 2 | 5 | 2 |
| F34A | 33.42 | 3.3.3.4.4 | cmm | TS | 2 | 5 | 3 |
| F3464 | 3.4.6.4 | 3.4.6.4 | p6m | TSH | 3 | 4 | 4 |
| F33 | 36 | 3.3.3.3.3.3 | p6m | T | 1 | 6 | 5 |
| F3636 | 3.6.3.6 | 3.6.3.6 | p6m | TH | 2 | 4 | 6 |
| F48 | 4.82 | 4.8.8 | p4m | SO | 2 | 3 | 7 |
| F666 | 63 | 6.6.6 | p6m | H | 1 | 3 | 8 |
| F4612 | 4.6.12 | 4.6.12 | p6m | SHD | 3 | 3 | 9 |
| F4 | 44 | 4.4.4.4 | p4m | S | 1 | 4 | 10 |
| F312 | 3.122 | 3.12.12 | p6m | TD | 2 | 3 | 11 |
| Symmetry Group | Lattice | Symmetry Types | Rotation Orders | Other Features |
|---|---|---|---|---|
| cmm | Rhombus | Rf, Rt | 2 | Rf in two perpendicular directions |
| p4g | Square | Rf, Rt, Gl | 2, 4 | Rf in two perpendicular directions |
| Order 2 Rt centers are at the intersections of Rf axes | ||||
| p4m | Square | Rf, Rt, Gl | 2, 4 | Rf lines intersect at 45° |
| All Rt centers lie on Rf axes | ||||
| p6 | Hexagon | Rt | 2, 3, 6 | No Rf; No Gl |
| p6m | Hexagon | Rf, Rt, Gl | 2, 3, 6 | Rf in six directions |
| Possibly the most complex | ||||
| pgg | Rectangular | Rt, Gl | 2 | No reflections. Gl in two perpendicular directions |
| Rt centers not located on Gl axes | ||||
| pmm | Rectangular | Rf, Rt | 2 | Rf in two perpendicular directions |
| Rt centers at the intersection of Rf axes |
| File Notation | Standard Notation | Symmetry Group | Polygon Types | Polygons in Pattern | Polygons Vertex 1 | Polygons Vertex 2 | Preference Ranking |
|---|---|---|---|---|---|---|---|
| F346G | 3^3.4^2;3.4.6.4 | p6m | TSH | 3 | 5 | 4 | 1 |
| F346C | 3^2.4.3.4; 3.4.6.4 | p6m | TSH | 3 | 5 | 4 | 2 |
| F346D | 3^6; 3^2.4.3.4 | p6m | TS | 2 | 6 | 5 | 3 |
| F346F | 3.4^2.6; 3.4.6.4 | p6m | TSH | 3 | 4 | 4 | 4 |
| F3462A | 3.4.6.4; 4.6.12 | p6m | TSHD | 4 | 4 | 3 | 5 |
| F3462B | 3^6; 3^2.4.12 | p6m | TSD | 3 | 6 | 4 | 6 |
| F36D | 3^6; 3^2.6^2 | p6m | TH | 2 | 6 | 4 | 7 |
| F3412 | 3.4.3.12; 3.12^2 | p4m | TSD | 3 | 4 | 3 | 8 |
| F34B | 3^3.4^2;3^2.4.3.4 | p4g | TS | 2 | 5 | 5 | 9 |
| F346E | 3^4.6; 3^2.6^2 | cmm | TH | 2 | 5 | 4 | 10 |
| F34C | 3^3.4^2;3^2.4.3.4 | pgg | TS | 2 | 5 | 5 | 11 |
| F36C | 3^6; 3^4.6 | p6m | TH | 2 | 6 | 5 | 12 |
| F346G | 3^3.4^2;3.4.6.4 | p6 | TH | 2 | 6 | 5 | 13 |
| F346C | 3^2.4.3.4; 3.4.6.4 | pmm | TH | 2 | 4 | 4 | 14 |
| F346D | 3^6; 3^2.4.3.4 | pmm | TS | 2 | 6 | 5 | 15 |
| F346F | 3.4^2.6; 3.4.6.4 | cmm | TSH | 3 | 4 | 4 | 16 |
| F3462A | 3.4.6.4; 4.6.12 | pmm | TSH | 3 | 4 | 4 | 17 |
| F3462B | 3^6; 3^2.4.12 | cmm | TS | 2 | 6 | 5 | 18 |
| F36D | 3^6; 3^2.6^2 | cmm | TS | 2 | 5 | 4 | 19 |
| F3412 | 3.4.3.12; 3.12^2 | cmm | TS | 2 | 5 | 4 | 20 |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Friedenberg, J. The Perceived Beauty of Regular Polygon Tessellations. Symmetry 2019, 11, 984. https://doi.org/10.3390/sym11080984
Friedenberg J. The Perceived Beauty of Regular Polygon Tessellations. Symmetry. 2019; 11(8):984. https://doi.org/10.3390/sym11080984
Chicago/Turabian StyleFriedenberg, Jay. 2019. "The Perceived Beauty of Regular Polygon Tessellations" Symmetry 11, no. 8: 984. https://doi.org/10.3390/sym11080984
APA StyleFriedenberg, J. (2019). The Perceived Beauty of Regular Polygon Tessellations. Symmetry, 11(8), 984. https://doi.org/10.3390/sym11080984




