New Chaotic Systems with Two Closed Curve Equilibrium Passing the Same Point: Chaotic Behavior, Bifurcations, and Synchronization
Abstract
1. Introduction
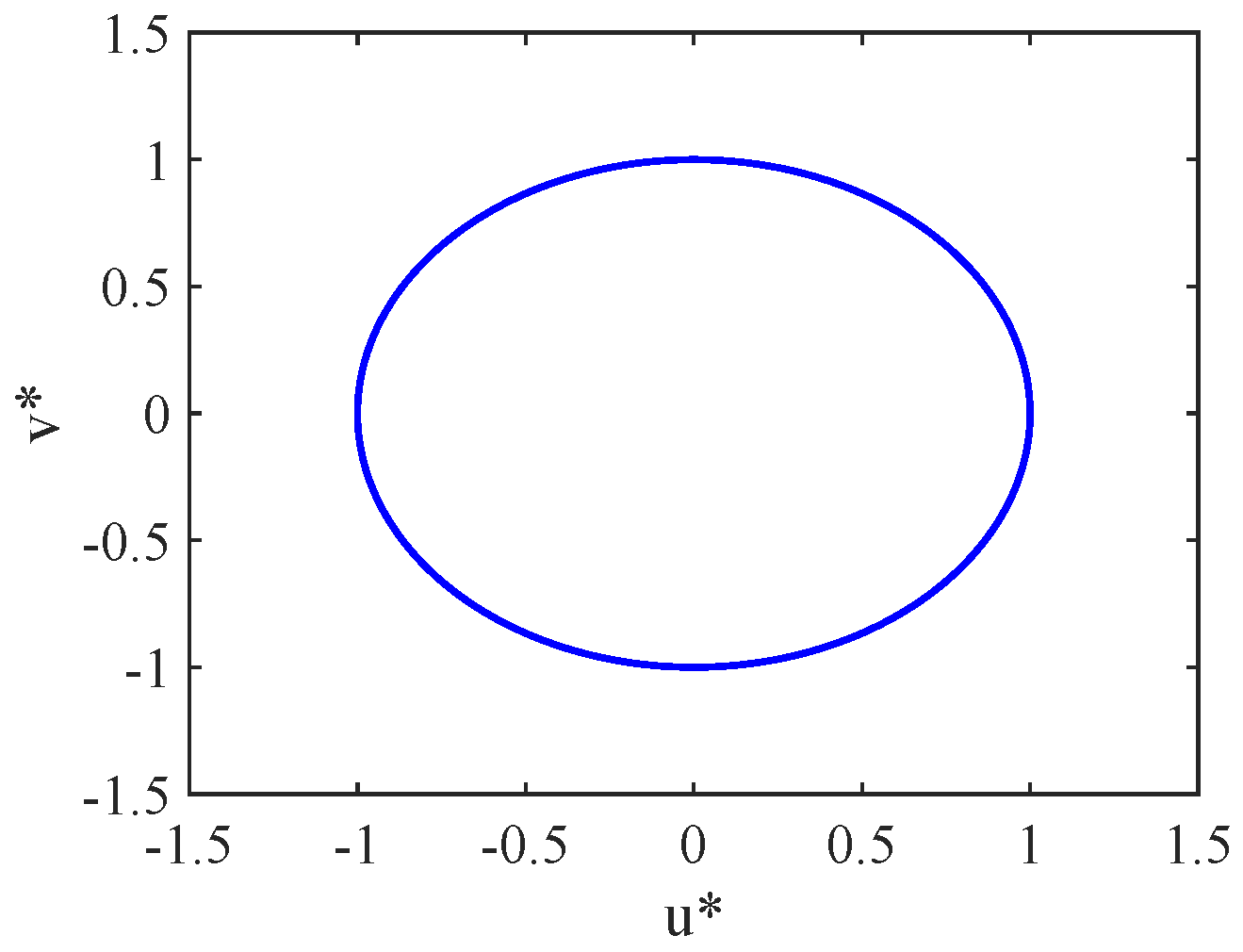
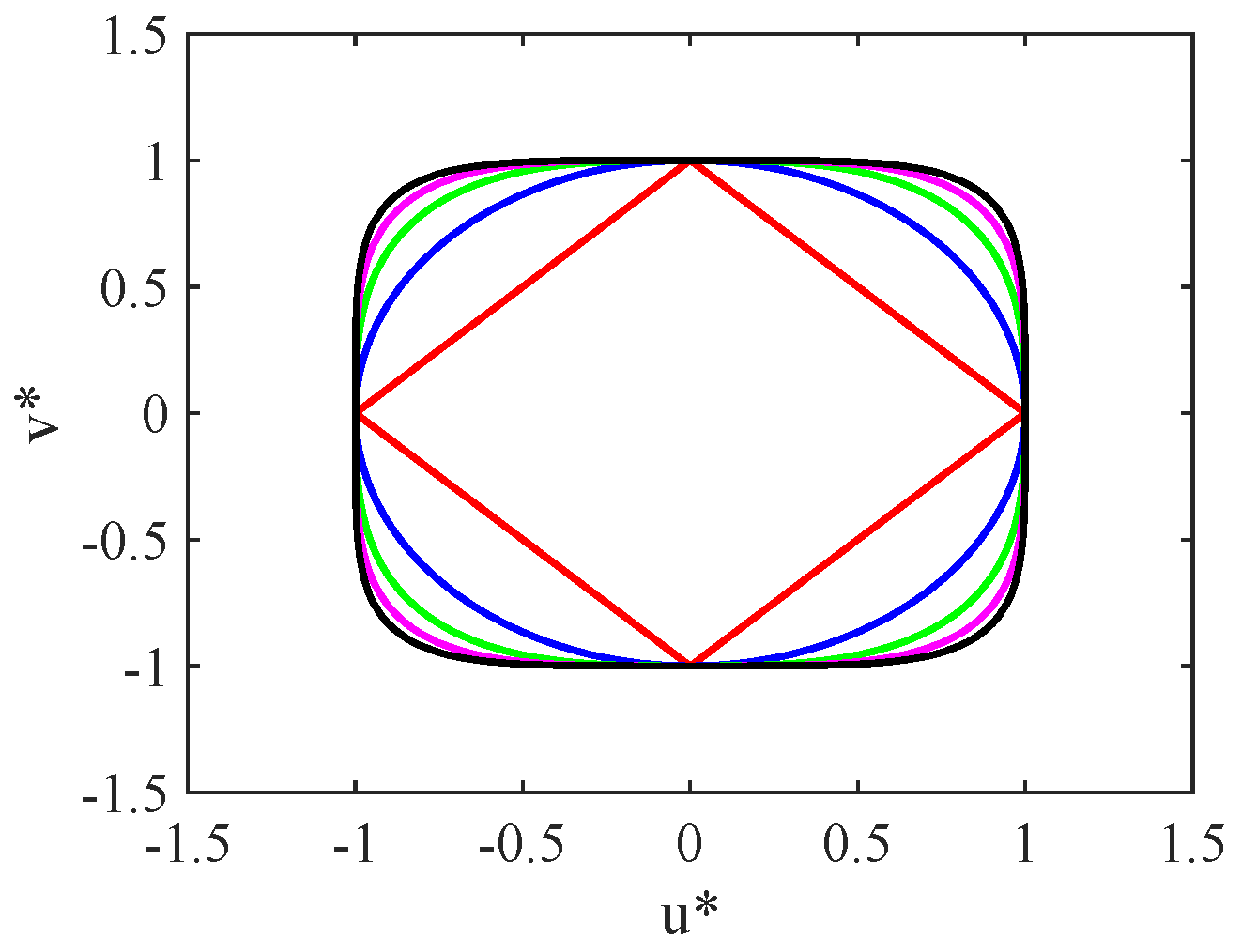
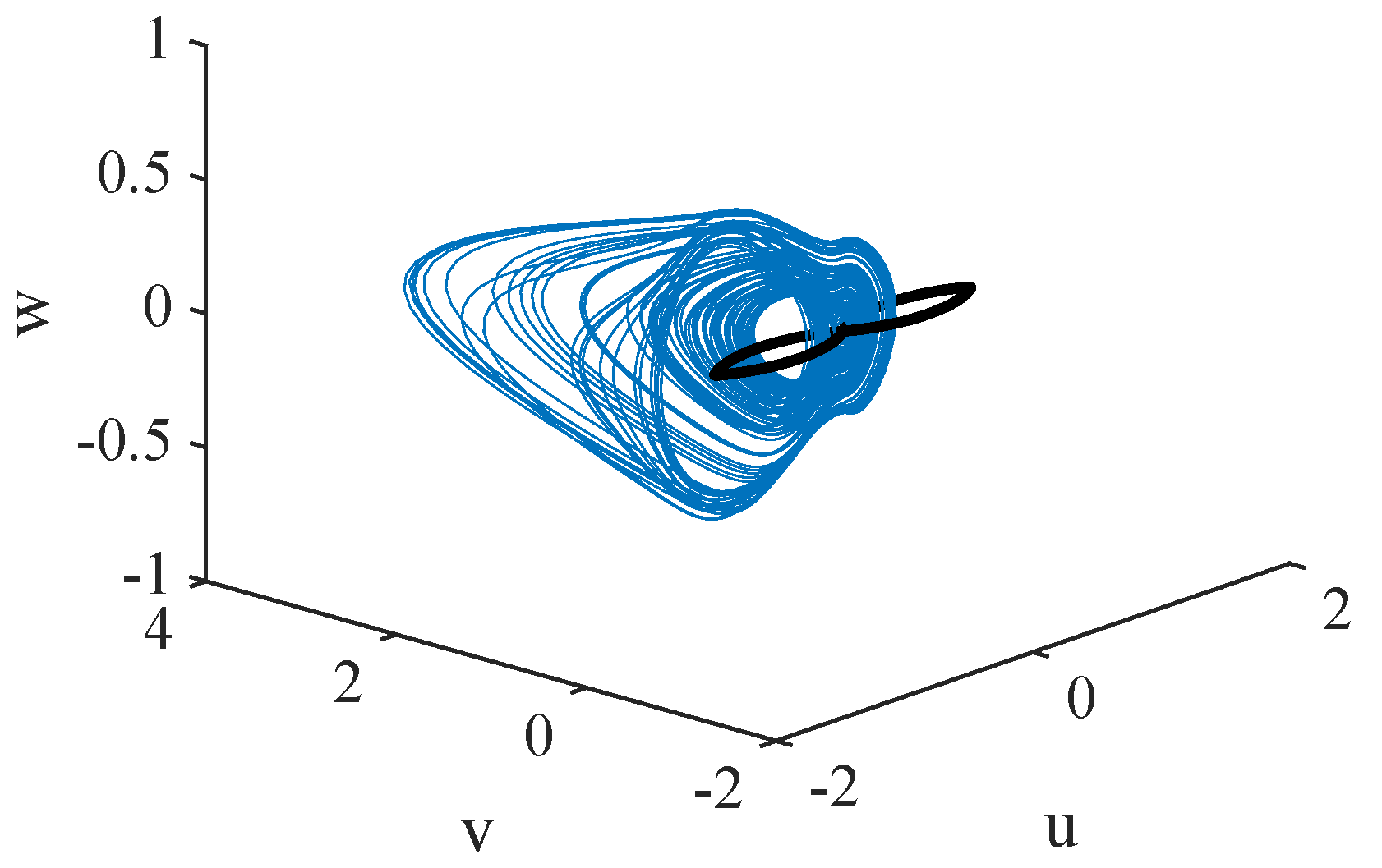
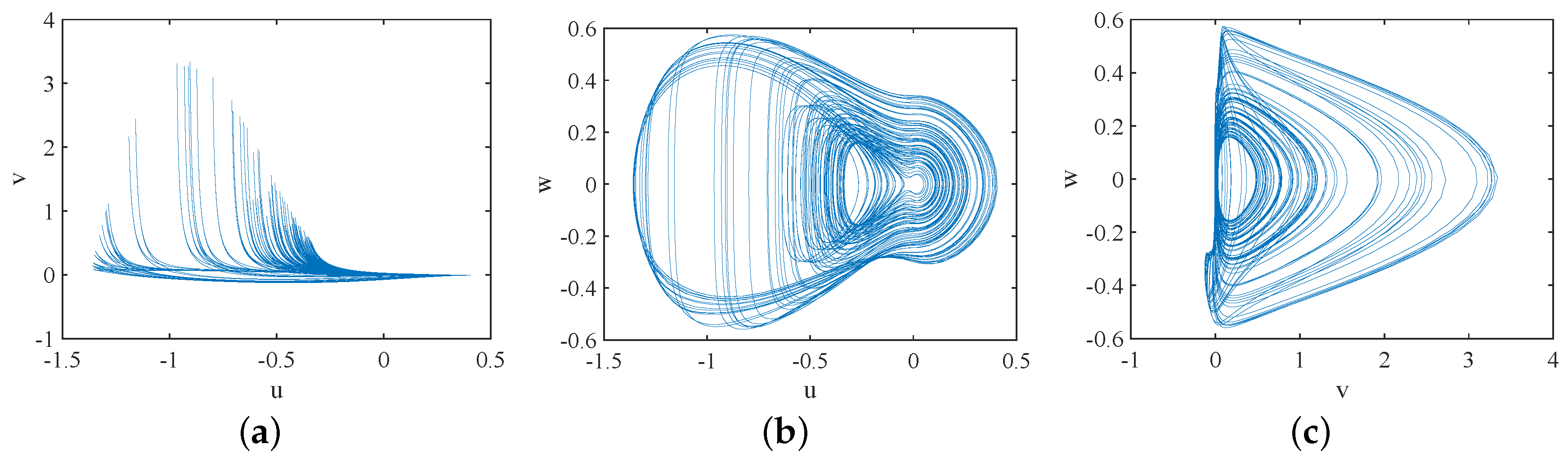
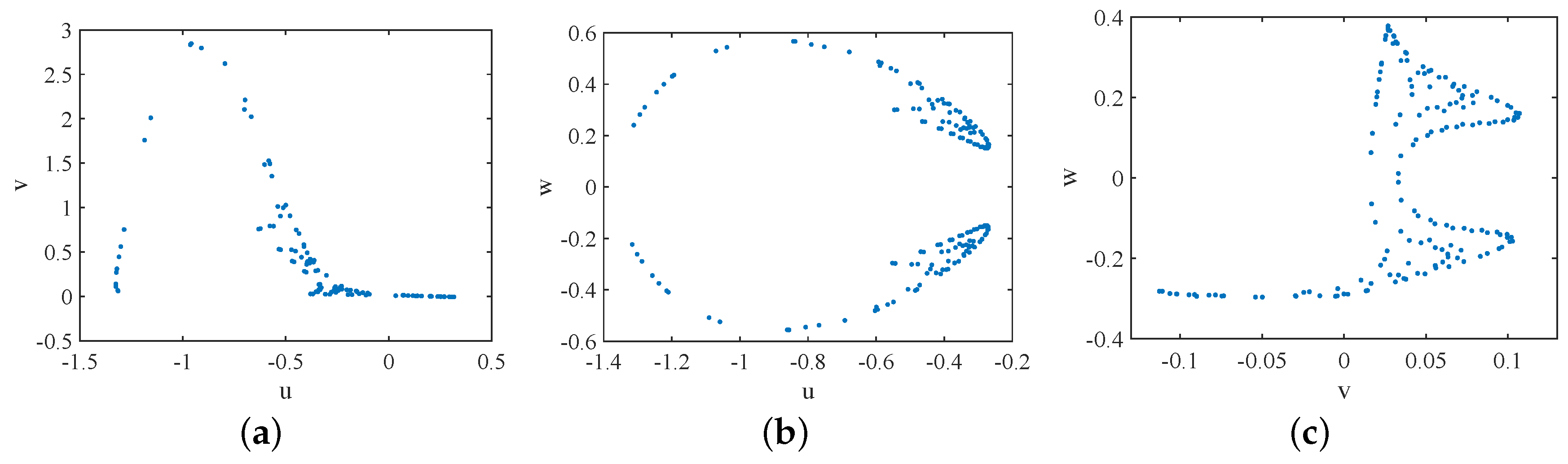
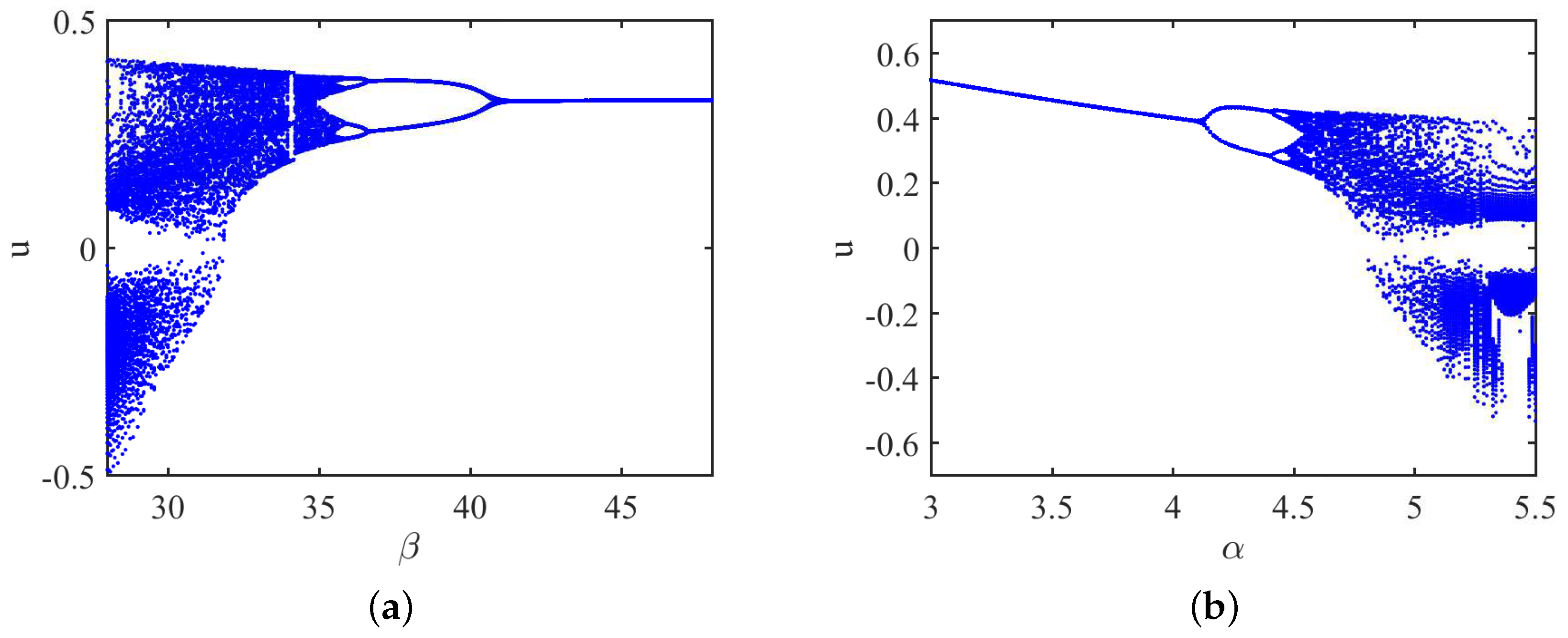
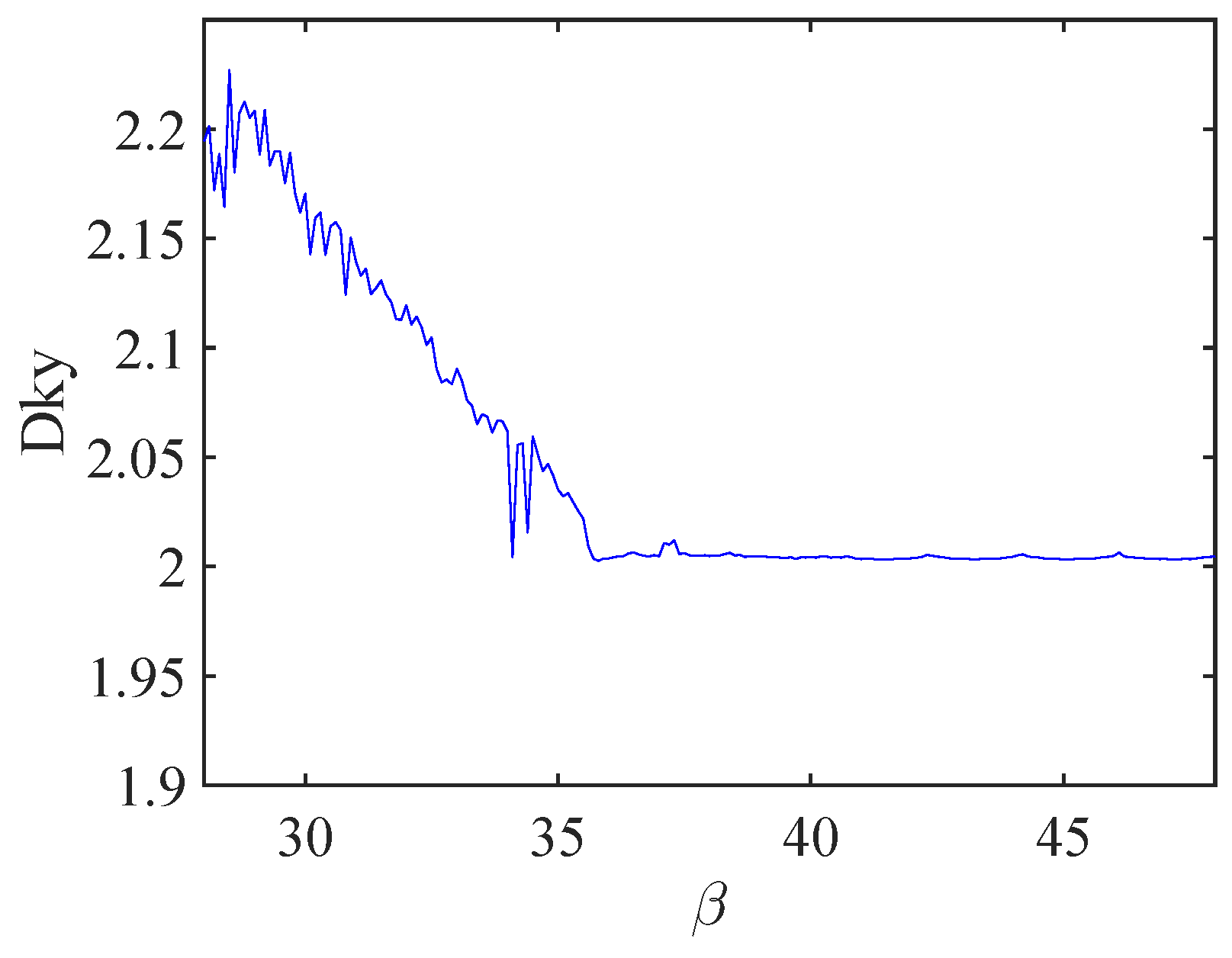
2. A New Family with Two Closed Curve Equilibrium
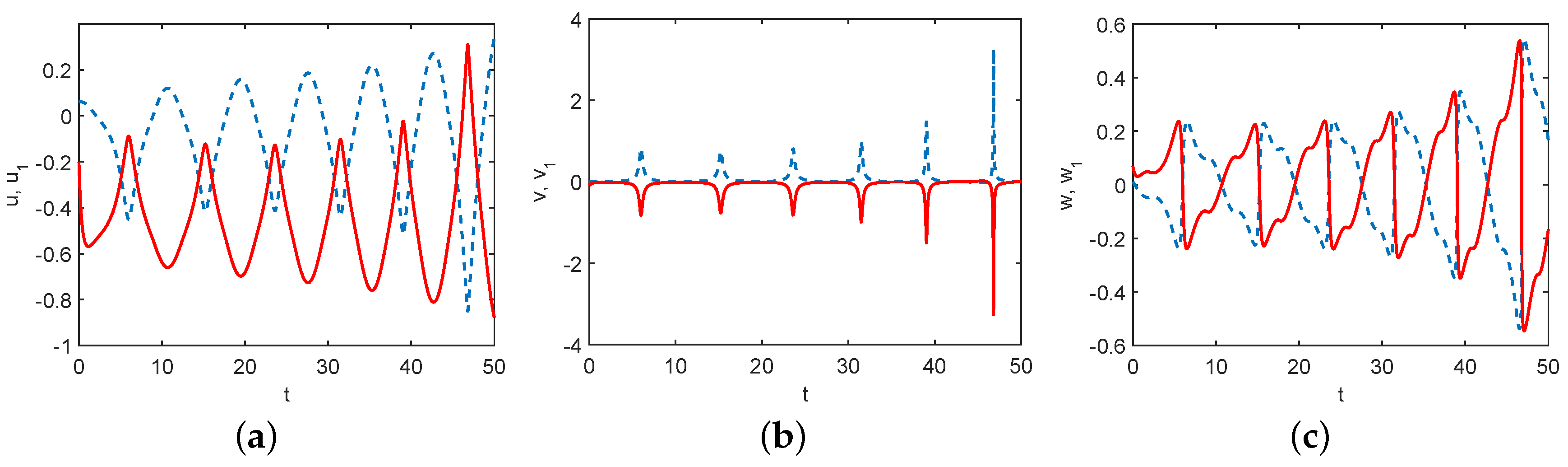
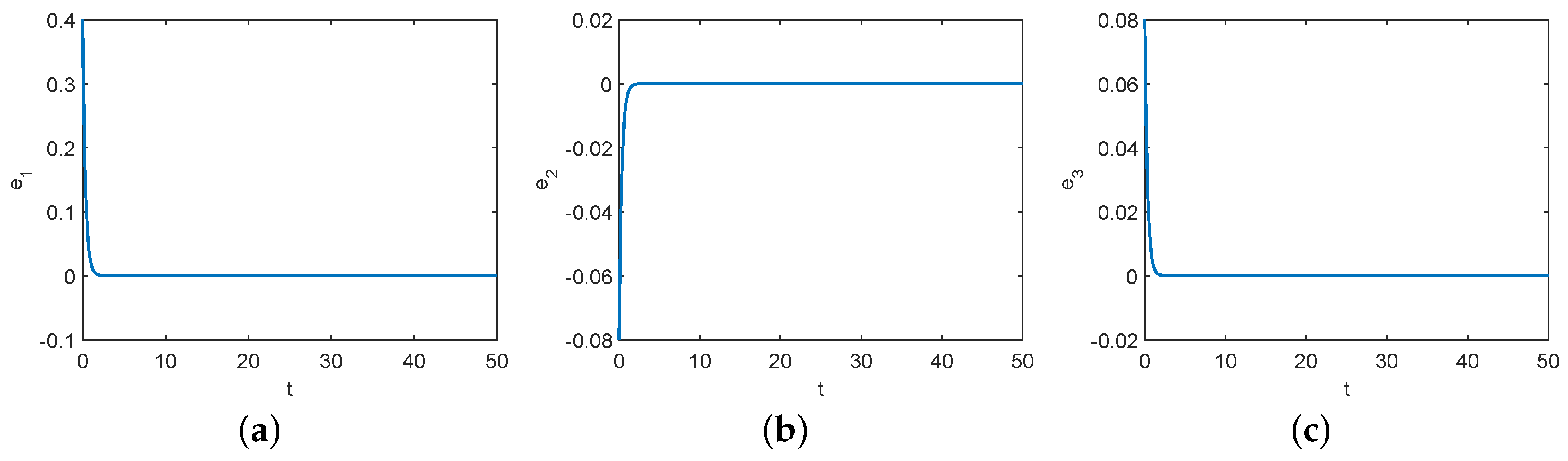
3. Anti-Synchronization of New Systems
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lorenz, E.N. Deterministic nonperiodic flow. J. Atmos. Sci. 1963, 20, 130–141. [Google Scholar] [CrossRef]
- Sprott, J.C. Some simple chaotic flows. Phys. Rev. E 1994, 50, R647–R650. [Google Scholar] [CrossRef]
- Sprott, J.C. Elegant Chaos Algebraically Simple Chaotic Flows; World Scientific: Singapore, 2010; pp. 10–11. [Google Scholar]
- Azar, A.T.; Vaidyanathan, S. Advances in Chaos Theory and Intelligent Control; Springer: Berlin, Germany, 2016; pp. 50–61. [Google Scholar]
- Pehlivan, I.; Moroz, I.M.; Vaidyanathan, S. Analysis, synchronization and circuit design of a novel butterfly attractor. J. Sound Vib. 2014, 333, 5077–5096. [Google Scholar] [CrossRef]
- Akgul, A.; Moroz, I.; Pehlivan, I.; Vaidyanathan, S. A new four-scroll chaotic attractor and its engineering applications. Optik 2016, 127, 5491–5499. [Google Scholar] [CrossRef]
- Pham, V.T.; Volos, C.; Jafari, S.; Wei, Z.; Wang, X. Constructing a novel no-equilibrium chaotic system. Int. J. Bifurc. Chaos 2014, 24, 1450073. [Google Scholar] [CrossRef]
- Molaie, M.; Jafari, S.; Sprott, J.C.; Golpayegani, S. Simple chaotic flows with one stable equilibrium. Int. J. Bifurc. Chaos 2013, 23, 1350188. [Google Scholar] [CrossRef]
- Jafari, S.; Sprott, J.C. Simple chaotic flows with a line equilibrium. Chaos Solitons Fractals 2013, 57, 79–84. [Google Scholar] [CrossRef]
- Gotthans, T.; Petrzela, J. New class of chaotic systems with circular equilibrium. Nonlin. Dyn. 2015, 81, 1143–1149. [Google Scholar] [CrossRef]
- Gotthans, T.; Sprott, J.C.; Petrzela, J. Simple chaotic flow with circle and square equilibrium. Int. J. Bifurc. Chaos 2016, 26, 1650137. [Google Scholar] [CrossRef]
- Pham, V.T.; Jafari, S.; Volos, C.; Giakoumis, A.; Vaidyanathan, S.; Kapitaniak, T. A chaotic system with equilibria located on the rounded square loop and its circuit implementation. IEEE Trans. Circuits Syst. II Express Briefs 2016, 63, 878–882. [Google Scholar] [CrossRef]
- Zhu, X.; Du, W.-S. A new family of chaotic systems with different closed curve equilibrium. Mathematics 2019, 7, 94. [Google Scholar] [CrossRef]
- Bondarenko, V.E. Information processing, memories, and synchronization in chaotic neural network with the time delay. Complexity 2005, 11, 39–52. [Google Scholar] [CrossRef]
- Cicek, S.; Ferikoglu, A.; Pehlivan, I. A new 3D chaotic system: Dynamical analysis, electronic circuit design, active control synchronization and chaotic masking communication application. Optik 2016, 127, 4024–4030. [Google Scholar] [CrossRef]
- Leonov, G.; Kuznetsov, N.; Seldedzhi, S.; Vagaitsev, V. Hidden oscillations in dynamical systems. Trans. Syst. Contr. 2011, 6, 54–67. [Google Scholar]
- Dudkowski, D.; Jafari, S.; Kapitaniak, T.; Kuznetsov, N.V.; Leonov, G.A.; Prasad, A. Hidden attractors in dynamical systems. Phys. Rep. 2016, 637, 1–50. [Google Scholar] [CrossRef]
- Sprott, J.C. A proposed standard for the publication of new chaotic systems. Int. J. Bifurc. Chaos 2011, 21, 2391–2394. [Google Scholar] [CrossRef]
- Wang, X.; Pham, V.T.; Volos, C. Dynamics, circuit design, and synchronization of a new chaotic system with closed curve equilibrium. Complexity 2017, 2017, 7138971. [Google Scholar] [CrossRef]
- Wolf, A.; Swift, J.B.; Swinney, H.L.; Vastano, J.A. Determining Lyapunov exponents from a time series. Phys. D Nonlinear Phenom. 1985, 16, 285–317. [Google Scholar] [CrossRef]
- Pecora, L.M.; Carroll, T.L. Synchronization in chaotic systems. Phys. Rev. Lett. 1990, 64, 821–824. [Google Scholar] [CrossRef]
- Boccaletti, S.; Kurths, J.; Osipov, G.; Valladares, D.L.; Zhou, C.S. The synchronization of chaotic systems. Phys. Rep. A 2002, 366, 1–101. [Google Scholar] [CrossRef]
- Klein, E.; Mislovaty, R.; Kanter, I.; Kinzel, W. Public-channel cryptography using chaos synchronization. Phys. Rev. E 2005, 72, 016214. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.N.; Chen, L.; Cai, Z.S.; Zhao, X.Z. Experimental study of chaos synchronization in the Belousov-Zhabotinsky chemical system. Chaos Solitons Fractals 2004, 22, 767–771. [Google Scholar] [CrossRef]
- Glass, L. Synchronization and rhythmic processes in physiology. Nature 2001, 410, 277–284. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.H.; Zhou, S.B. Chaos synchronization for bi-directional coupled two-neuron systems with discrete delays. Lect. Notes Comput. Sci. 2005, 3496, 351–356. [Google Scholar]
- Yousefi, S.; Maistrenko, Y.; Popovych, S. Complex dynamics in a simple model of interdependent open economies. Discret. Dyn. Nat. Soc. 2000, 5, 161–177. [Google Scholar] [CrossRef]




© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, X.; Du, W.-S. New Chaotic Systems with Two Closed Curve Equilibrium Passing the Same Point: Chaotic Behavior, Bifurcations, and Synchronization. Symmetry 2019, 11, 951. https://doi.org/10.3390/sym11080951
Zhu X, Du W-S. New Chaotic Systems with Two Closed Curve Equilibrium Passing the Same Point: Chaotic Behavior, Bifurcations, and Synchronization. Symmetry. 2019; 11(8):951. https://doi.org/10.3390/sym11080951
Chicago/Turabian StyleZhu, Xinhe, and Wei-Shih Du. 2019. "New Chaotic Systems with Two Closed Curve Equilibrium Passing the Same Point: Chaotic Behavior, Bifurcations, and Synchronization" Symmetry 11, no. 8: 951. https://doi.org/10.3390/sym11080951
APA StyleZhu, X., & Du, W.-S. (2019). New Chaotic Systems with Two Closed Curve Equilibrium Passing the Same Point: Chaotic Behavior, Bifurcations, and Synchronization. Symmetry, 11(8), 951. https://doi.org/10.3390/sym11080951






