Some Applications of the (G′/G,1/G)-Expansion Method for Finding Exact Traveling Wave Solutions of Nonlinear Fractional Evolution Equations
Abstract
1. Introduction
2. Conformable Fractional Derivative and Its Properties
- (1)
- .
- (2)
- .
- (3)
- .
- (4)
- .
- (5)
- , provided that is differentiable.
3. Algorithm of the -Expansion Method
4. Applications of the -Expansion Method
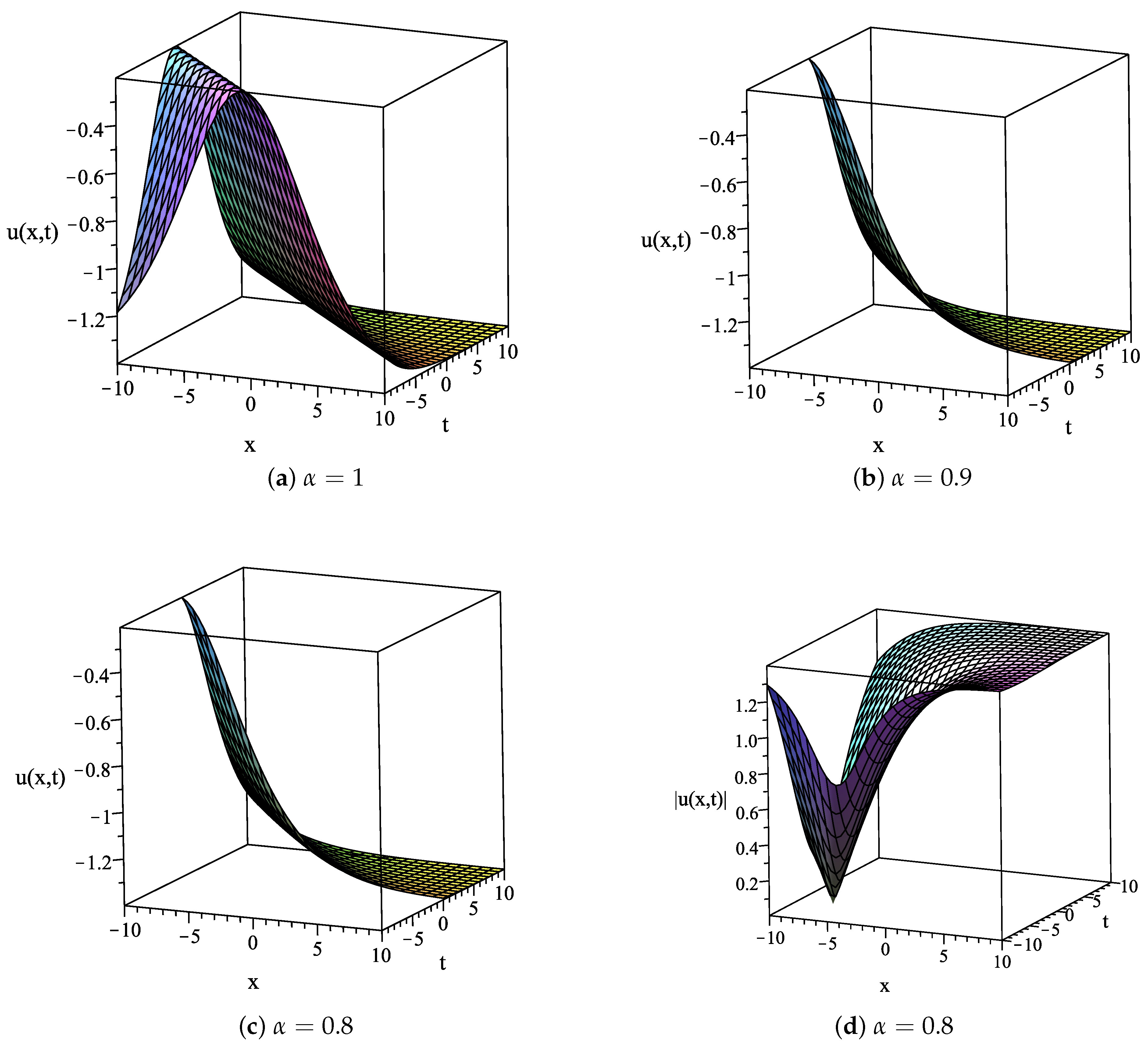
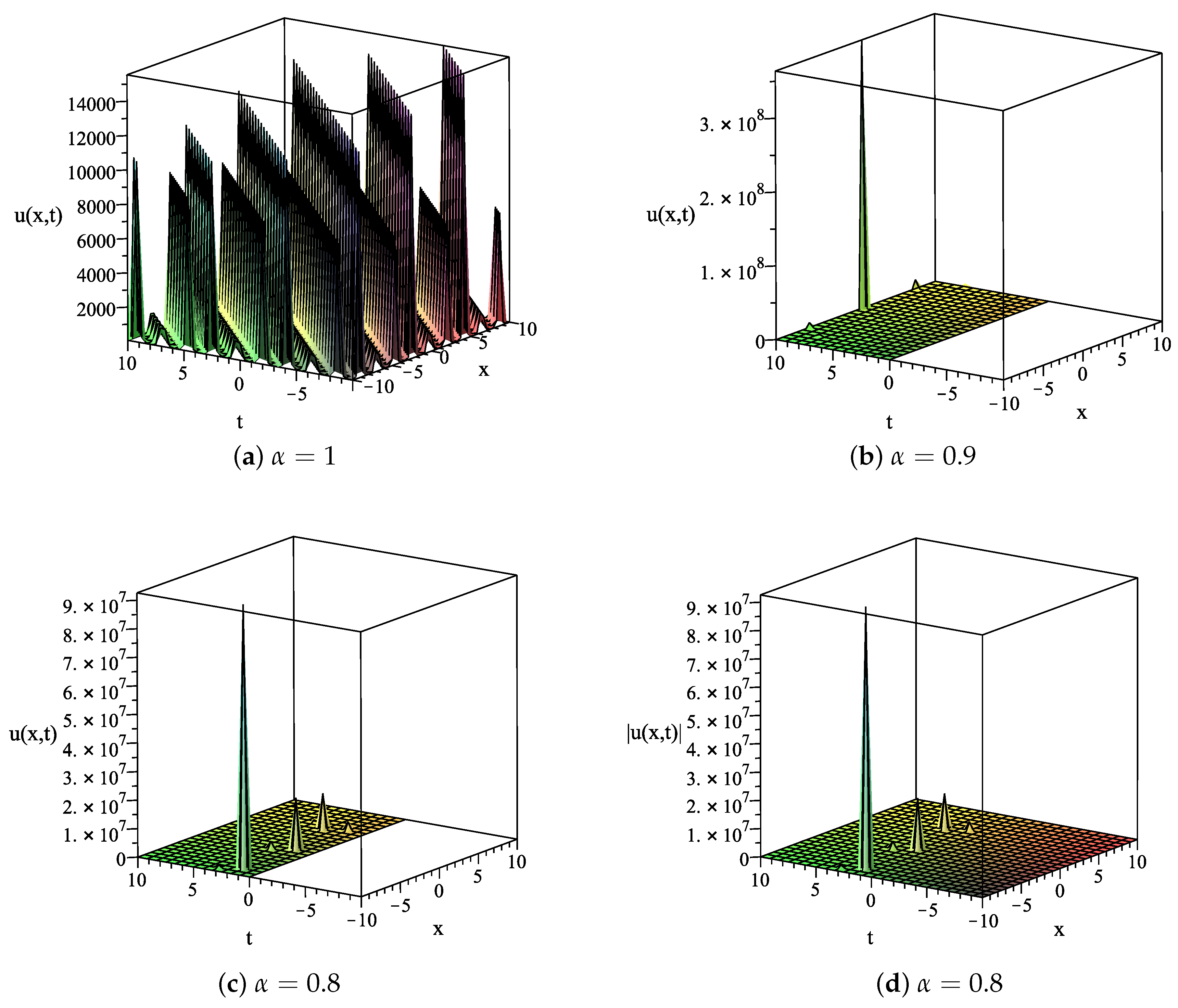
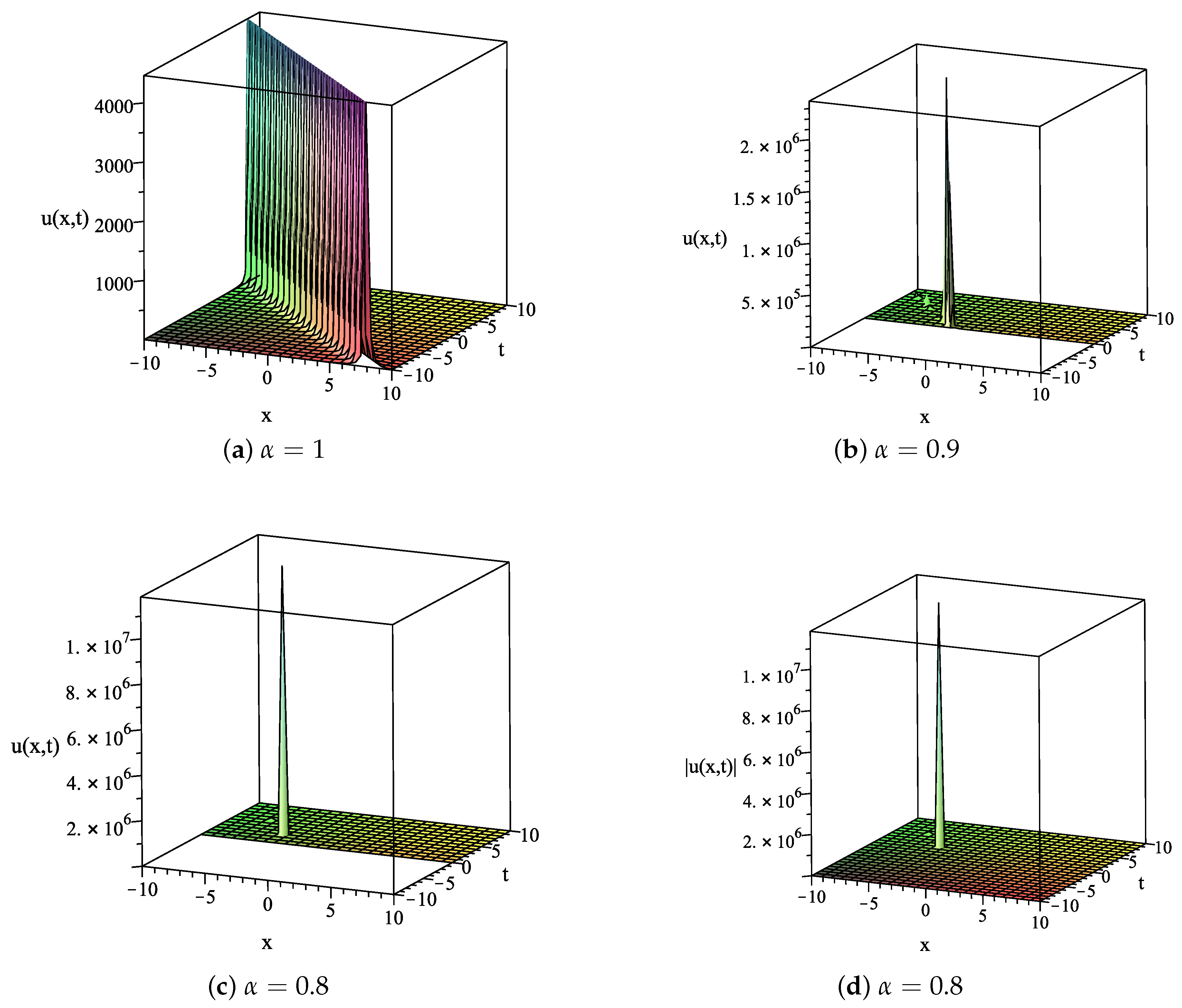
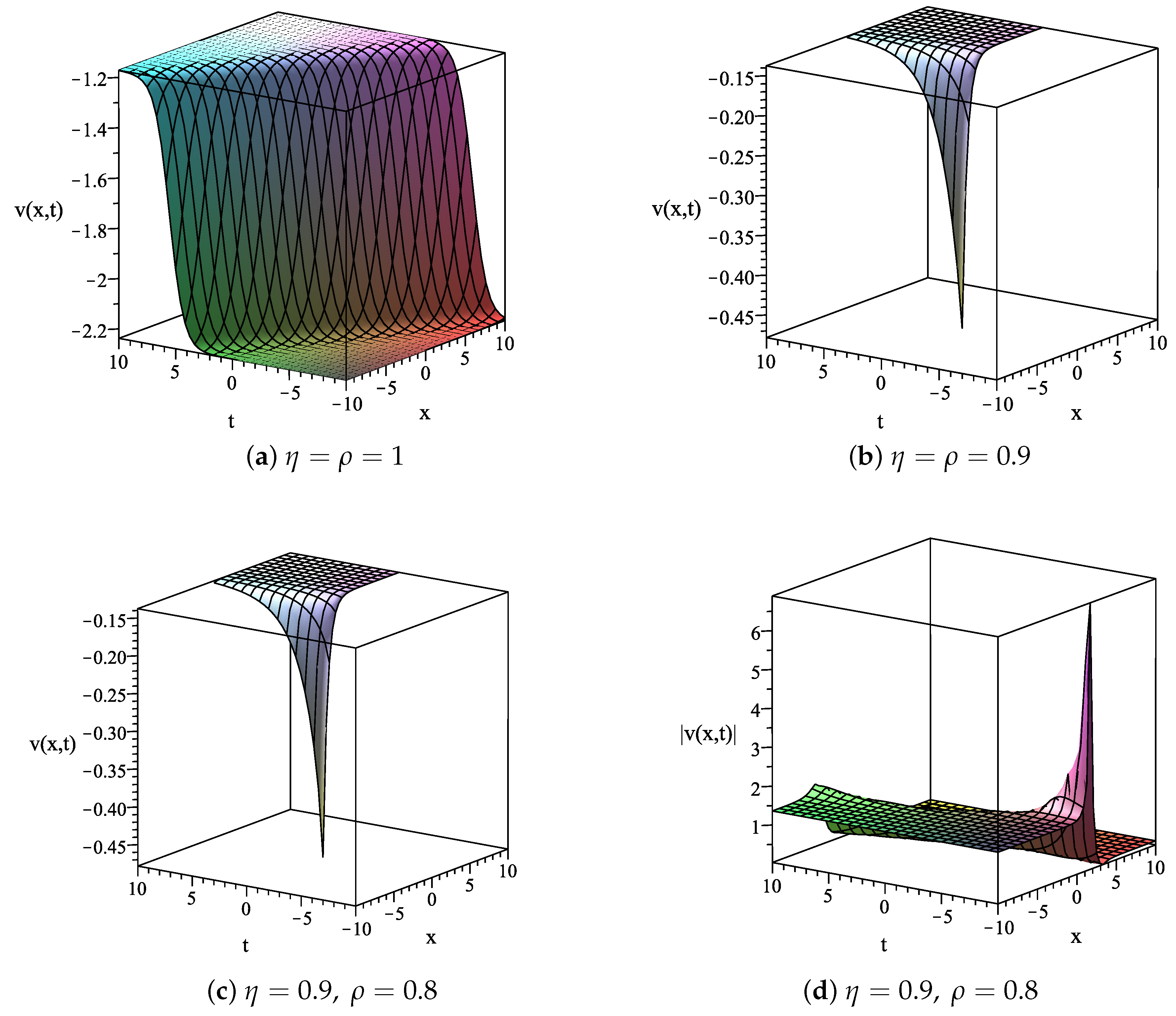
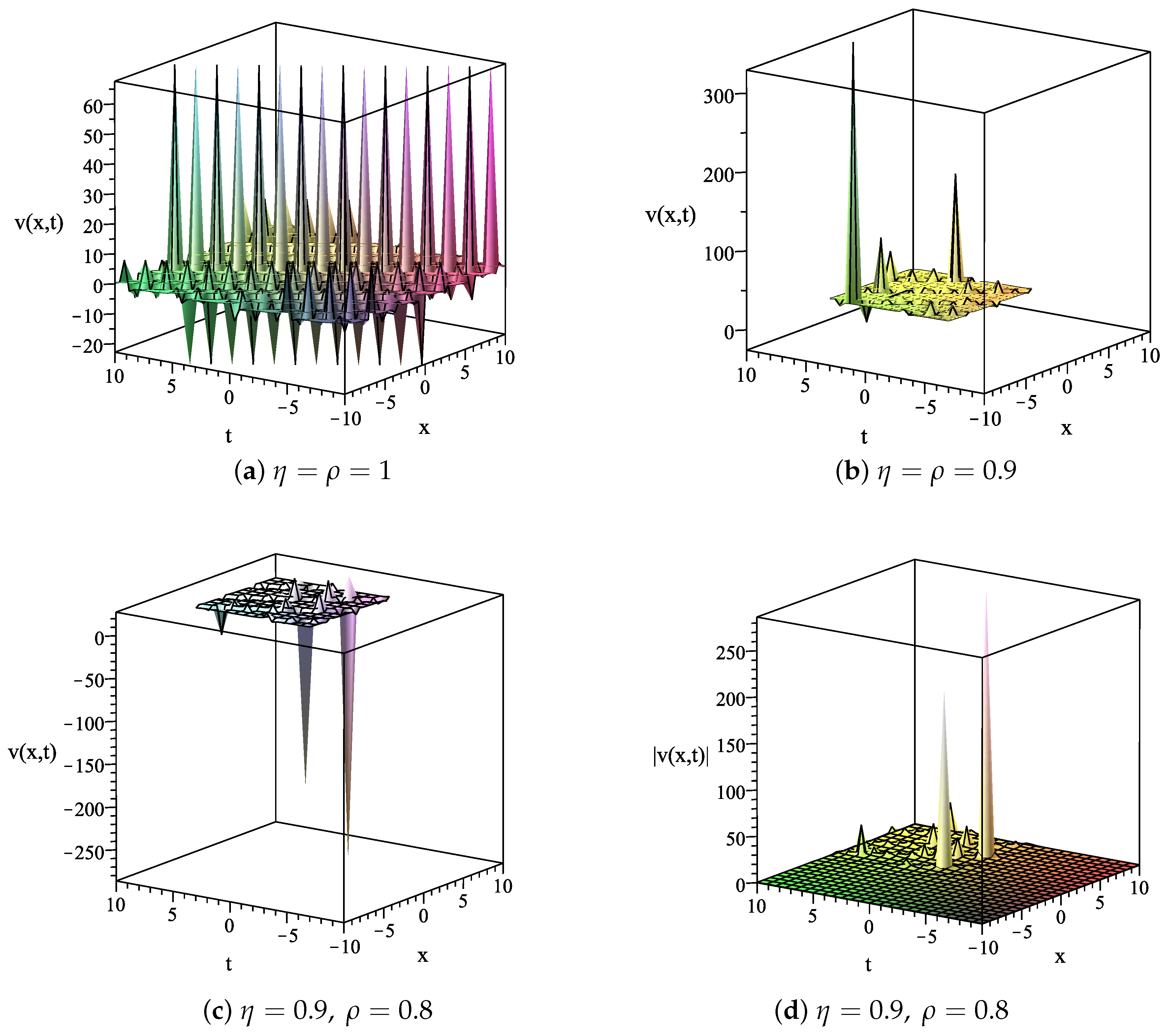
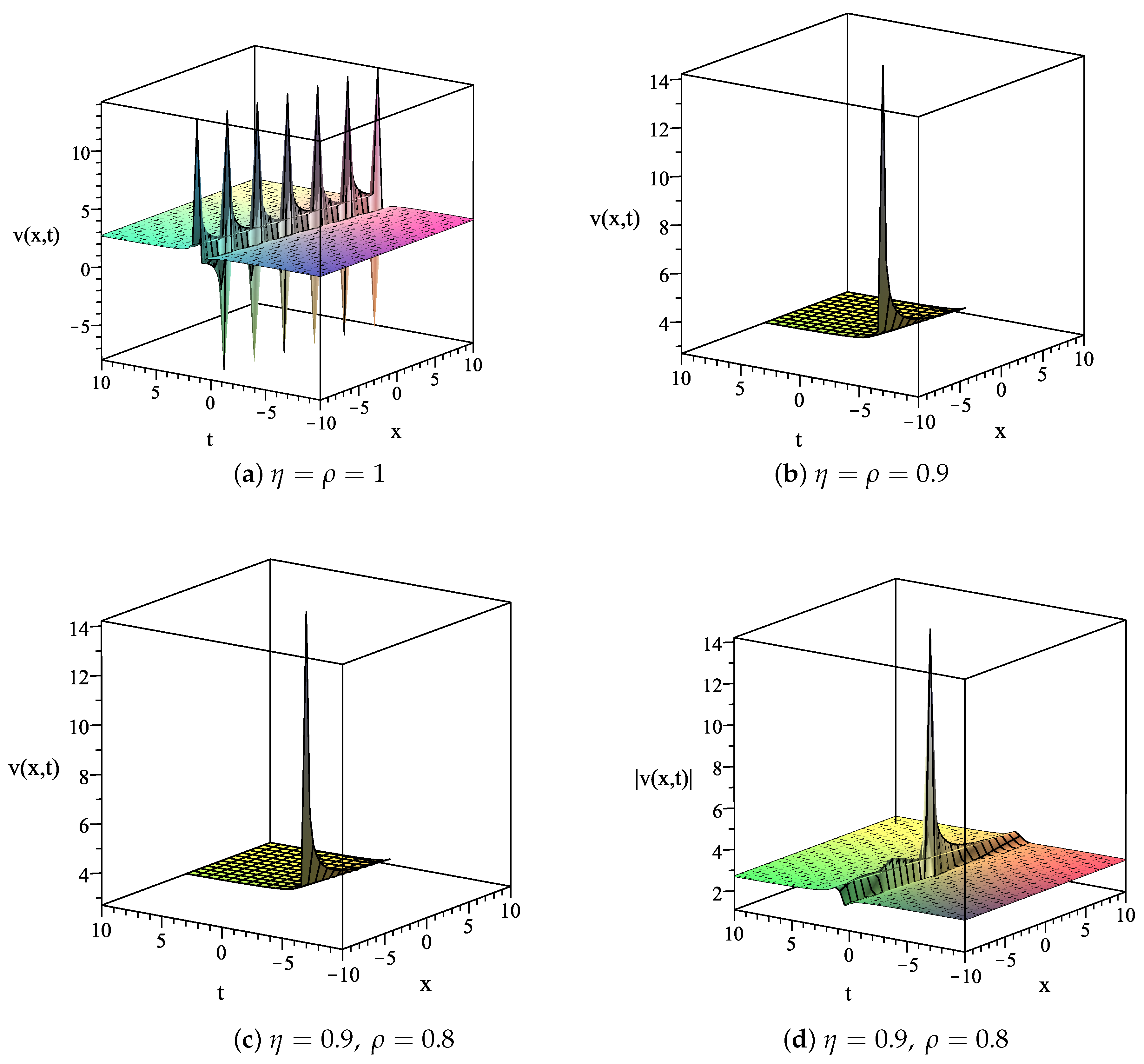
4.1. The Time-Fractional (2+1)-Dimensional Extended Quantum Zakharov-Kuznetsov Equation
4.2. The Space-Time-Fractional Generalized Hirota-Satsuma Coupled KdV System
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hayward, R.; Biancalana, F. Constructing new nonlinear evolution equations with supersymmetry. J. Phys. Math. Theor. 2018, 51, 275202. [Google Scholar] [CrossRef]
- Sendi, C.T.; Manafian, J.; Mobasseri, H.; Mirzazadeh, M.; Zhou, Q.; Bekir, A. Application of the ITEM for solving three nonlinear evolution equations arising in fluid mechanics. Nonlinear Dyn. 2019, 95, 669–684. [Google Scholar] [CrossRef]
- Marhic, M.; Boggio, J.C. Exact solutions for four-wave mixing crosstalk in one-pump fiber optical parametric amplifiers. In Proceedings of the 2008 IEEE/LEOS Winter Topical Meeting Series, Sorrento, Italy, 14–16 January 2008; pp. 69–70. [Google Scholar]
- Wazwaz, A.M. Partial Differential Equations and Solitary Waves Theory; Springer Science & Business Media: Berlin, Germany, 2010. [Google Scholar]
- Bazyar, M.H.; Song, C. Analysis of transient wave scattering and its applications to site response analysis using the scaled boundary finite-element method. Soil Dyn. Earthq. Eng. 2017, 98, 191–205. [Google Scholar] [CrossRef]
- Ahmad, I. Local meshless method for PDEs arising from models of wound healing. Appl. Math. Model. 2017, 48, 688–710. [Google Scholar]
- Sirisubtawee, S.; Kaewta, S. New modified Adomian decomposition recursion schemes for solving certain types of nonlinear fractional two-point boundary value problems. Int. J. Math. Math. Sci. 2017, 2017, 5742965. [Google Scholar] [CrossRef]
- Asma, M.; Othman, W.; Wong, B.; Biswas, A. Optical soliton perturbation with quadratic-cubic nonlinearity by Adomian decomposition method. Optik 2018, 164, 632–641. [Google Scholar] [CrossRef]
- Ünlü, C.; Jafari, H.; Baleanu, D. Revised variational iteration method for solving systems of nonlinear fractional-order differential equations. In Abstract and Applied Analysis; Hindawi: London, UK, 2013; Volume 2013. [Google Scholar]
- Jafari, H.; Jassim, H.K.; Moshokoa, S.P.; Ariyan, V.M.; Tchier, F. Reduced differential transform method for partial differential equations within local fractional derivative operators. Adv. Mech. Eng. 2016, 8, 1687814016633013. [Google Scholar] [CrossRef]
- Bera, P.; Sil, T. Homotopy perturbation method in quantum mechanical problems. Appl. Math. Comput. 2012, 219, 3272–3278. [Google Scholar] [CrossRef]
- Nik, H.S.; Effati, S.; Shirazian, M. An approximate-analytical solution for the Hamilton-Jacobi-Bellman equation via homotopy perturbation method. Appl. Math. Model. 2012, 36, 5614–5623. [Google Scholar]
- Deng, W. Finite element method for the space and time fractional Fokker–Planck equation. SIAM J. Numer. Anal. 2008, 47, 204–226. [Google Scholar] [CrossRef]
- Theeraek, P.; Phongthanapanich, S.; Dechaumphai, P. Combined adaptive meshing technique and finite volume element method for solving convection–diffusion equation. Jpn. J. Ind. Appl. Math. 2013, 30, 185–202. [Google Scholar] [CrossRef]
- Jhinga, A.; Daftardar-Gejji, V. A new finite-difference predictor-corrector method for fractional differential equations. Appl. Math. Comput. 2018, 336, 418–432. [Google Scholar] [CrossRef]
- Aslan, E.C.; Inc, M. Soliton solutions of NLSE with quadratic-cubic nonlinearity and stability analysis. Waves Random Complex Media 2017, 27, 594–601. [Google Scholar] [CrossRef]
- Baleanu, D.; Uğurlu, Y.; Kilic, B. Improved (G’/G)-Expansion Method for the Time-Fractional Biological Population Model and Cahn-Hilliard Equation. J. Comput. Nonlinear Dyn. 2015, 10, 051016. [Google Scholar] [CrossRef]
- Alam, M.N.; Akbar, M.A. The new approach of the generalized (G′/G)-expansion method for nonlinear evolution equations. Ain Shams Eng. J. 2014, 5, 595–603. [Google Scholar] [CrossRef]
- Sirisubtawee, S.; Koonprasert, S.; Khaopant, C.; Porka, W. Two Reliable Methods for Solving the (3+1)-Dimensional Space-Time Fractional Jimbo-Miwa Equation. Math. Probl. Eng. 2017, 2017, 9257019. [Google Scholar] [CrossRef]
- Sirisubtawee, S.; Koonprasert, S. Exact Traveling Wave Solutions of Certain Nonlinear Partial Differential Equations Using the (G′/G2)-Expansion Method. Adv. Math. Phys. 2018, 2018, 7628651. [Google Scholar] [CrossRef]
- Guner, O.; Bekir, A.; Bilgil, H. A note on exp-function method combined with complex transform method applied to fractional differential equations. Adv. Nonlinear Anal. 2015, 4, 201–208. [Google Scholar] [CrossRef]
- Yépez-Martínez, H.; Gómez-Aguilar, J.; Baleanu, D. Beta-derivative and sub-equation method applied to the optical solitons in medium with parabolic law nonlinearity and higher order dispersion. Optik Int. J. Light Electron Opt. 2018, 155, 357–365. [Google Scholar] [CrossRef]
- Yépez-Martínez, H.; Gómez-Aguilar, J.; Atangana, A. First integral method for non-linear differential equations with conformable derivative. Math. Model. Nat. Phenom. 2018, 13, 14. [Google Scholar] [CrossRef]
- Bulut, H.; Baskonus, H.M.; Pandir, Y. The modified trial equation method for fractional wave equation and time fractional generalized Burgers equation. In Abstract and Applied Analysis; Hindawi: London, UK, 2013; Volume 2013. [Google Scholar]
- Pandir, Y.; Gurefe, Y. New exact solutions of the generalized fractional Zakharov-Kuznetsov equations. Life Sci. J. 2013, 10, 2701–2705. [Google Scholar]
- Taghizadeh, N.; Mirzazadeh, M.; Rahimian, M.; Akbari, M. Application of the simplest equation method to some time-fractional partial differential equations. Ain Shams Eng. J. 2013, 4, 897–902. [Google Scholar] [CrossRef]
- Anderson, J.; Moradi, S.; Rafiq, T. Non-Linear Langevin and Fractional Fokker-Planck Equations for Anomalous Diffusion by Lévy Stable Processes. Entropy 2018, 20, 760. [Google Scholar] [CrossRef]
- Tarasov, V.E. Fractional Dynamics: Applications of Fractional Calculus to Dynamics of Particles, Fields and Media; Springer Science & Business Media: Berlin, Germany, 2011. [Google Scholar]
- Mainardi, F. Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models; World Scientific: Hackensack, NJ, USA, 2010. [Google Scholar]
- West, B.; Bologna, M.; Grigolini, P. Physics of Fractal Operators; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; Wiley: Indianapolis, IN, USA, 1993. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional integrals and derivatives: theory and applications. Teoret Mat Fiz 1993, 3, 397–414. [Google Scholar]
- Sahadevan, R.; Bakkyaraj, T. Invariant analysis of time fractional generalized Burgers and Korteweg-de Vries equations. J. Math. Anal. Appl. 2012, 393, 341–347. [Google Scholar] [CrossRef]
- Rizvi, S.; Ali, K.; Bashir, S.; Younis, M.; Ashraf, R.; Ahmad, M. Exact soliton of (2+1)-dimensional fractional Schrödinger equation. Superlattices Microstruct. 2017, 107, 234–239. [Google Scholar] [CrossRef]
- Mohyud-Din, S.T.; Bibi, S. Exact solutions for nonlinear fractional differential equations using (G′/G2)-expansion method. Alex. Eng. J. 2018, 57, 1003–1008. [Google Scholar] [CrossRef]
- Raza, N.; Abdullah, M.; Butt, A.R.; Murtaza, I.G.; Sial, S. New exact periodic elliptic wave solutions for extended quantum Zakharov-Kuznetsov equation. Opt. Quantum Electron. 2018, 50, 177. [Google Scholar] [CrossRef]
- Ali, M.N.; Osman, M.; Husnine, S.M. On the analytical solutions of conformable time-fractional extended Zakharov–Kuznetsov equation through G′/G2-expansion method and the modified Kudryashov method. SeMA J. 2018, 76, 15–25. [Google Scholar] [CrossRef]
- Guo, S.; Mei, L.; Li, Y.; Sun, Y. The improved fractional sub-equation method and its applications to the space-time fractional differential equations in fluid mechanics. Phys. Lett. A 2012, 376, 407–411. [Google Scholar] [CrossRef]
- Hirota, R.; Satsuma, J. Soliton solutions of a coupled Korteweg-de Vries equation. Phys. Lett. A 1981, 85, 407–408. [Google Scholar] [CrossRef]
- Satsuma, J.; Hirota, R. A coupled KdV equation is one case of the four-reduction of the KP hierarchy. J. Phys. Soc. Jpn. 1982, 51, 3390–3397. [Google Scholar] [CrossRef]
- Neirameh, A. Soliton solutions of the time fractional generalized Hirota-Satsuma coupled KdV system. Appl. Math. Inf. Sci. 2015, 9, 1847–1853. [Google Scholar]
- Saberi, E.; Hejazi, S.R. Lie symmetry analysis, conservation laws and exact solutions of the time-fractional generalized Hirota-Satsuma coupled KdV system. Phys. A Stat. Mech. Appl. 2018, 492, 296–307. [Google Scholar] [CrossRef]
- Halim, A.; Kshevetskii, S.; Leble, S. Numerical integration of a coupled Korteweg-de Vries system. Comput. Math. Appl. 2003, 45, 581–591. [Google Scholar] [CrossRef][Green Version]
- Zhang, H. New exact solutions for two generalized Hirota-Satsuma coupled KdV systems. Commun. Nonlinear Sci. Numer. Simul. 2007, 12, 1120–1127. [Google Scholar] [CrossRef]
- Zigao, C.; Junfen, L.; Fang, L. New Exact Solutions for the Variable-Coefficient Generalized Hirota-Satsuma Coupled KdV System. In Proceedings of the 2010 International Conference on Electrical and Control Engineering, Wuhan, China, 25–27 June 2010; Volume 2010, pp. 1349–1354. [Google Scholar]
- Khater, M.M.; Zahran, E.H.; Shehata, M.S. Solitary wave solution of the generalized Hirota-Satsuma coupled KdV system. J. Egypt. Math. Soc. 2017, 25, 8–12. [Google Scholar] [CrossRef]
- Khalil, R.; Horani, M.A.; Yousef, A.; Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65–70. [Google Scholar] [CrossRef]
- Kumar, D.; Seadawy, A.R.; Joardar, A.K. Modified Kudryashov method via new exact solutions for some conformable fractional differential equations arising in mathematical biology. Chin. J. Phys. 2018, 56, 75–85. [Google Scholar] [CrossRef]
- Guner, O.; Bekir, A.; Ünsal, Ö. Two reliable methods for solving the time fractional Clannish Random Walkers Parabolic equation. Optik Int. J. Light Electron Opt. 2016, 127, 9571–9577. [Google Scholar] [CrossRef]
- Demiray, S.; Ünsal, Ö.; Bekir, A. Exact solutions of nonlinear wave equations using (G′/G,1/G)-expansion method. J. Egypt. Math. Soc. 2015, 23, 78–84. [Google Scholar] [CrossRef]
- Zayed, E.; Alurrfi, K. The (G′/G,1/G)-expansion method and its applications to two nonlinear Schrödinger equations describing the propagation of femtosecond pulses in nonlinear optical fibers. Optik Int. J. Light Electron Opt. 2016, 127, 1581–1589. [Google Scholar] [CrossRef]
- Li, B.; Chen, Y.; Zhang, H. Explicit exact solutions for new general two-dimensional KdV-type and two-dimensional KdV-Burgers-type equations with nonlinear terms of any order. J. Phys. A Math. General 2002, 35, 8253. [Google Scholar] [CrossRef]
- Yan, Z. The extended Jacobian elliptic function expansion method and its application in the generalized Hirota-Satsuma coupled KdV system. Chaos Solitons Fractals 2003, 15, 575–583. [Google Scholar] [CrossRef]
- Rezazadeh, H.; Seadawy, A.R.; Eslami, M.; Mirzazadeh, M. Generalized solitary wave solutions to the time fractional generalized Hirota-Satsuma coupled KdV via new definition for wave transformation. J. Ocean Eng. Sci. 2019, 4, 77–84. [Google Scholar] [CrossRef]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sirisubtawee, S.; Koonprasert, S.; Sungnul, S. Some Applications of the (G′/G,1/G)-Expansion Method for Finding Exact Traveling Wave Solutions of Nonlinear Fractional Evolution Equations. Symmetry 2019, 11, 952. https://doi.org/10.3390/sym11080952
Sirisubtawee S, Koonprasert S, Sungnul S. Some Applications of the (G′/G,1/G)-Expansion Method for Finding Exact Traveling Wave Solutions of Nonlinear Fractional Evolution Equations. Symmetry. 2019; 11(8):952. https://doi.org/10.3390/sym11080952
Chicago/Turabian StyleSirisubtawee, Sekson, Sanoe Koonprasert, and Surattana Sungnul. 2019. "Some Applications of the (G′/G,1/G)-Expansion Method for Finding Exact Traveling Wave Solutions of Nonlinear Fractional Evolution Equations" Symmetry 11, no. 8: 952. https://doi.org/10.3390/sym11080952
APA StyleSirisubtawee, S., Koonprasert, S., & Sungnul, S. (2019). Some Applications of the (G′/G,1/G)-Expansion Method for Finding Exact Traveling Wave Solutions of Nonlinear Fractional Evolution Equations. Symmetry, 11(8), 952. https://doi.org/10.3390/sym11080952