Field Fluctuations and Casimir Energy of 1D-Fermions
Abstract
1. Introduction
2. Fundamentals of the Approach
- Particles moving from left to right with and momentum k, .
- Particles moving from right to left with and momentum k, .
- Anti-particles moving from left to right with and momentum k, .
- Anti-particles moving from right to left with and momentum k, .
3. Spectrum of Fluctuations of the Dirac Field Confined in a Finite Filament
3.1. Construction of the Boundary Spinors and the Boundary Condition
- At the components are determined by the eigenvalue equation . This means that the component of contributes to .
- At the components are determined by the eigenvalue equation . This means that the component of contributes to .
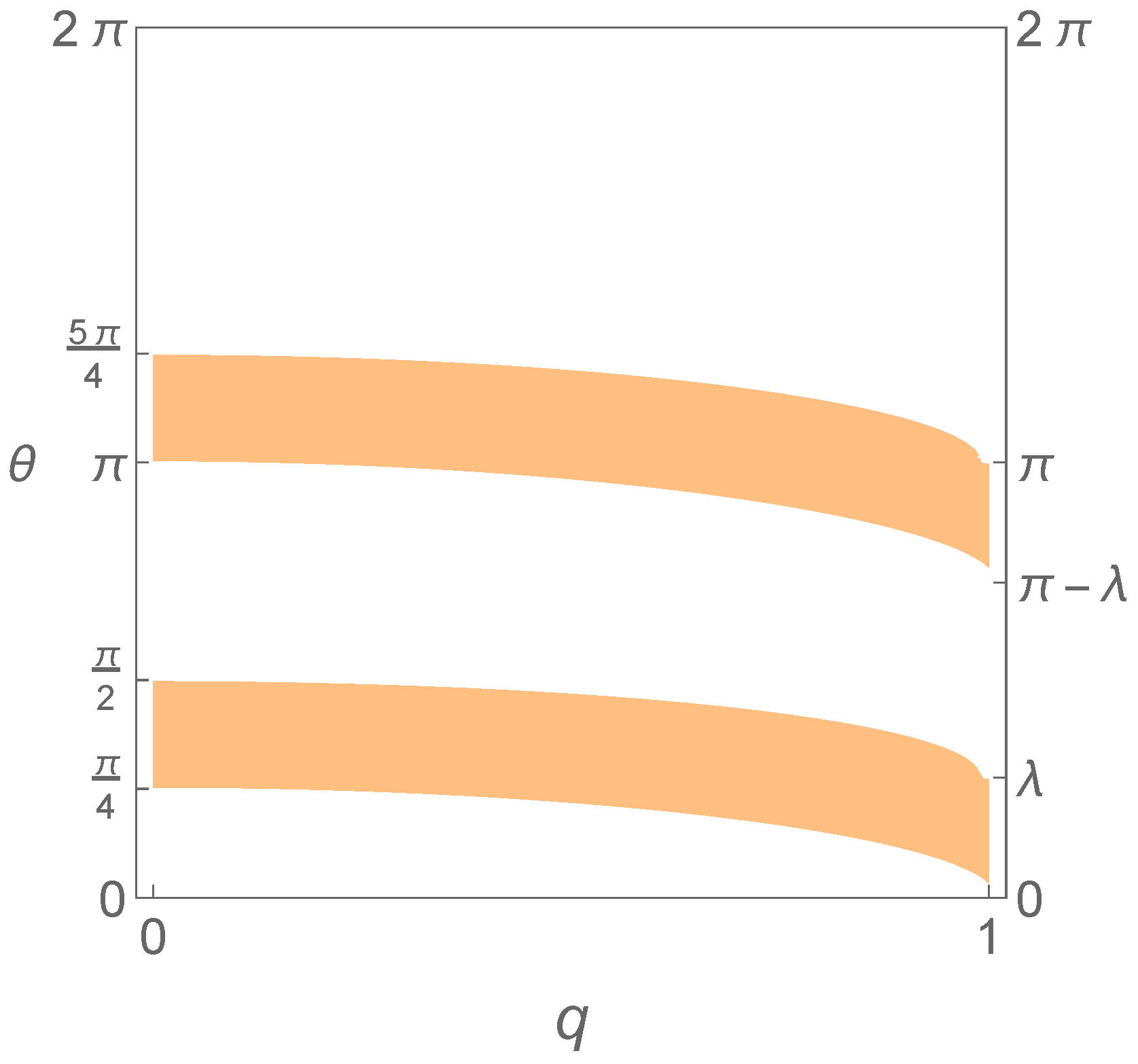
3.2. Normal Modes
3.3. Localised Edge States
4. Casimir Energy of the Dirac Field in 1D
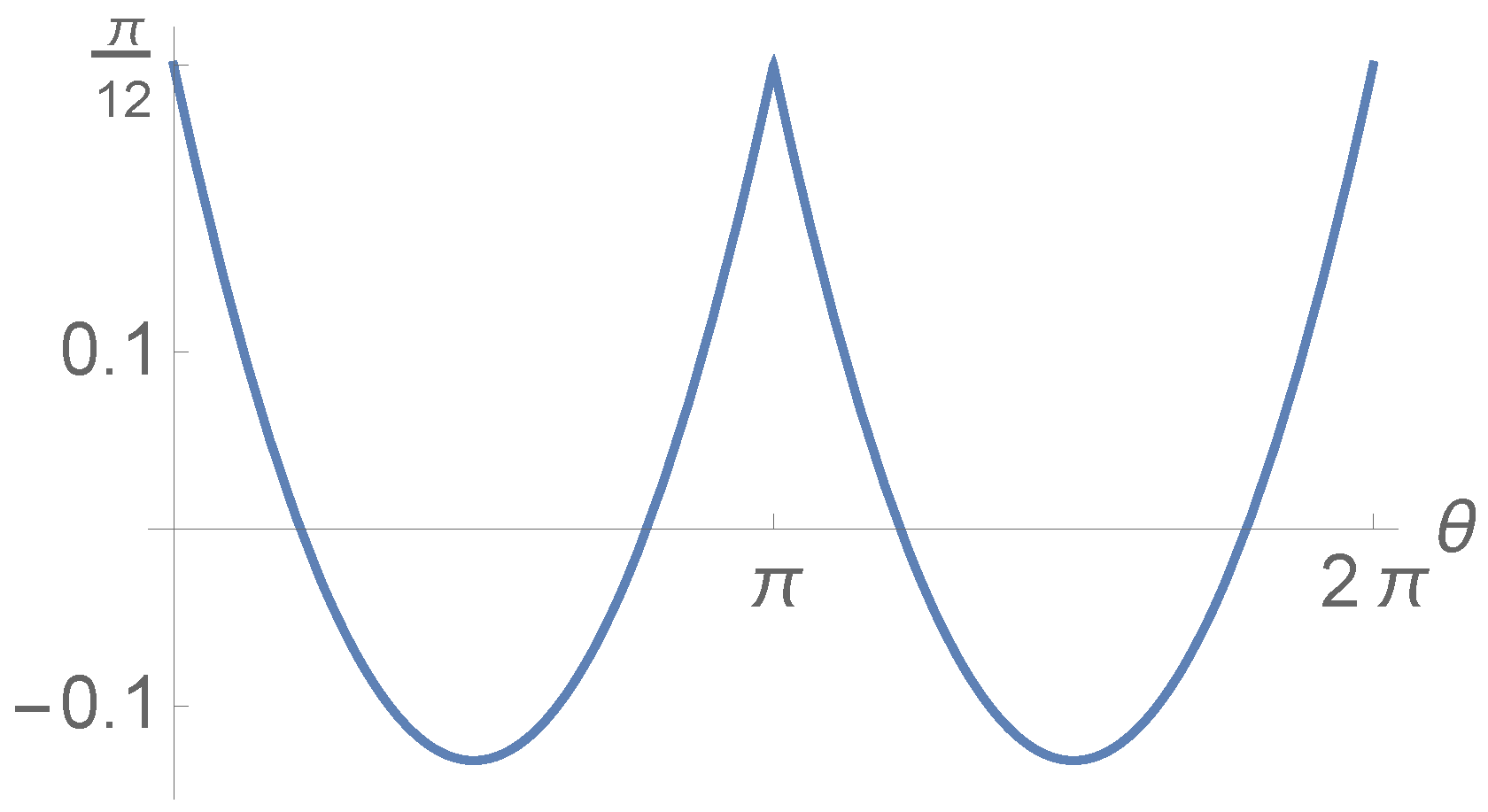
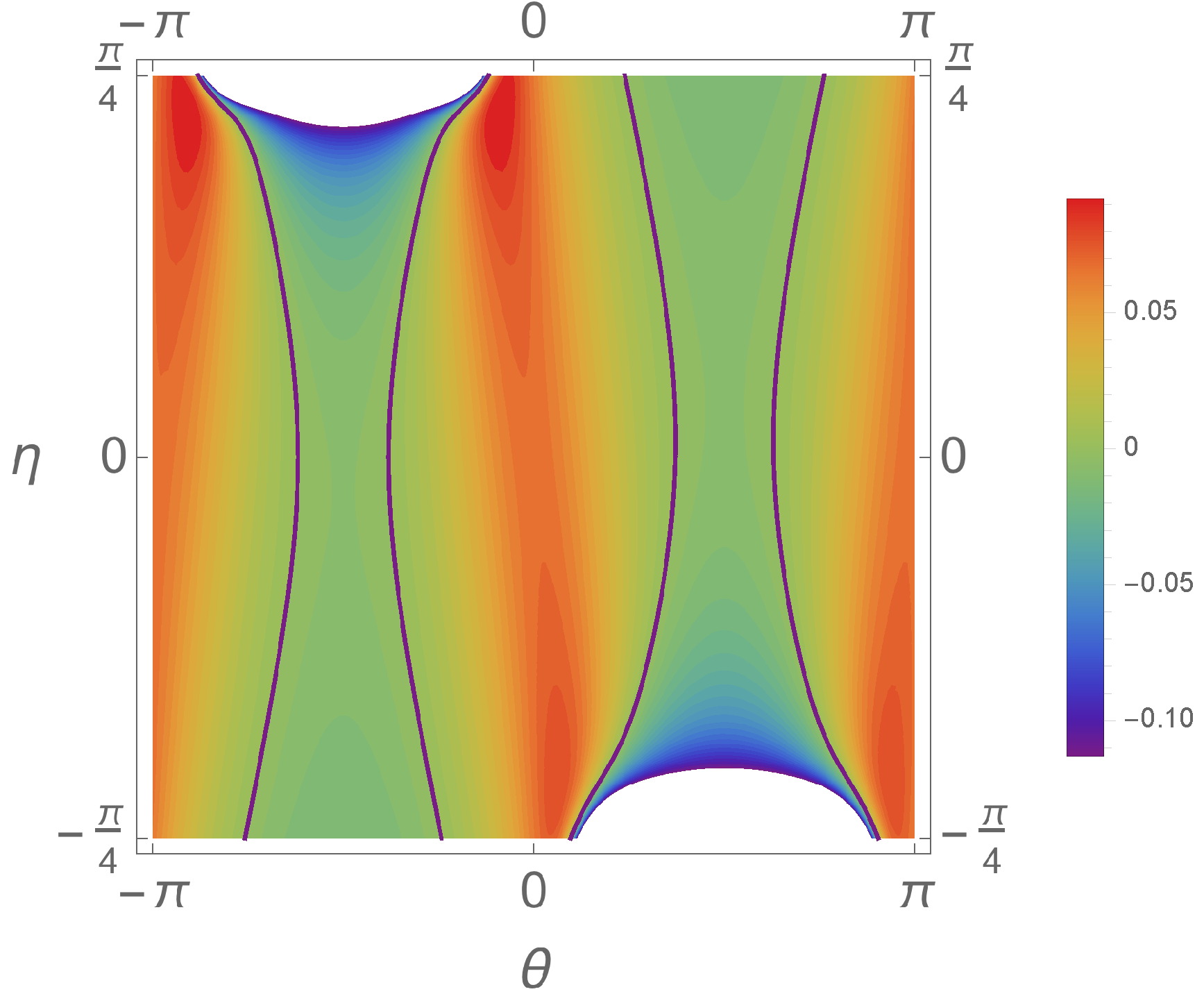
4.1. Casimir Energy of Light Fermions
4.2. Casimir Energy of Heavy Fermions
A Remark on the Sign of the Energy for Heavy Fermions
5. Conclusions and Outlook
Author Contributions
Funding
Conflicts of Interest
References
- Asorey, M.; Ibort, A.; Marmo, G. Global theory of quantum boundary conditions and topology change. Int. J. Mod. Phys. A 2005, 20, 1001. [Google Scholar] [CrossRef]
- Grubb, G. A characterization of the non-local boundary value problems associated with an elliptic operator. Ann. Sc. Norm. Super. Pisa. 1968, 22, 425. [Google Scholar]
- Asorey, M.; Garcia-Alvarez, D.; Munoz-Castaneda, J.M. Casimir Effect and Global Theory of Boundary Conditions. J. Phys. A Math. Gen. 2006, 39, 6127. [Google Scholar] [CrossRef]
- Asorey, M.; Garcia-Alvarez, D.; Munoz-Castaneda, J.M. Vacuum Energy and Renormalization on the Edge. J. Phys. A Math. Theor. 2007, 40, 6767. [Google Scholar] [CrossRef]
- Asorey, M.; Munoz-Castaneda, J.M. Vacuum Boundary Effects. J. Phys. A Math. Theor. 2008, 41, 304004. [Google Scholar] [CrossRef][Green Version]
- Asorey, M.; Munoz-Castaneda, J.M. Attractive and Repulsive Casimir Vacuum Energy with General Boundary Conditions. Nucl. Phys. B 2013, 874, 852. [Google Scholar] [CrossRef]
- Silva, H.O.; Farina, C. A Simple model for the dynamical Casimir effect for a static mirror with time-dependent properties. Phys. Rev. D 2011, 84, 045003. [Google Scholar] [CrossRef]
- Milton, K.A. The Casimir Effect: Physical Manifestations of Zero-Point Energy; World Sci.: Singapore, 2001. [Google Scholar]
- Milonni, P.W. The Quantum Vacuum; Academic Press: San Diego, CA, USA, 1994. [Google Scholar]
- Ibort, A.; Lledó, F.; Pérez-Pardo, J.M. On Self-Adjoint Extensions and Symmetries in Quantum Mechanics. Ann. Henri Poincaré 2015, 1460, 15–54. [Google Scholar] [CrossRef]
- Ibort, A. Three lectures on global boundary conditions and the theory of self-adjoint extensions of the covariant Laplace-Beltrami and Dirac operators on Riemannian manifolds with boundary. AIP Conf. Proc. 2012, 16, 2367–2397. [Google Scholar]
- Pérez-Pardo, J.M.; Barbero-Linán, M.; Ibort, A. Boundary dynamics and topology change in quantum mechanics. Int. J. Geom. Methods Mod. Phys. 2015, 12, 1560011. [Google Scholar] [CrossRef]
- Facchi, P.; Garnero, G.; Ligabo, L. Quantum cavities with alternating boundary conditions. J. Phys. A Math. Theor. 2018, 51, 105301. [Google Scholar] [CrossRef]
- Facchi, P.; Garnero, G.; Marmo, G.; Samuel, J.; Sinha, S. Boundaries without boundaries. Ann. Phys. 2018, 394, 139. [Google Scholar] [CrossRef]
- Asorey, M.; Balachandran, A.P.; Pérez-Pardo, J.M. Edge states at phase boundaries and their stability. Rev. Math. Phys. 2016, 28, 1650020. [Google Scholar] [CrossRef]
- Chodos, A.; Jaffe, R.L.; Johnson, K.; Thorn, C.B.; Weisskopf, V.F. New Extended Model of Hadrons. Phys. Rev. D 1974, 9, 3471. [Google Scholar] [CrossRef]
- Johnson, K. The M.I.T. Bag Model. Acta Phys. Polon. B 1975, 6, 865. [Google Scholar]
- Milton, K.A. Fermionic Casimir Stress on A Spherical Bag. Ann. Phys. 1983, 150, 432. [Google Scholar] [CrossRef]
- Elizalde, E.; Bordag, M.; Kirsten, K. Casimir energy for a massive fermionic quantum field with a spherical boundary. J. Phys. A 1998, 31, 1743. [Google Scholar] [CrossRef][Green Version]
- Asorey, M.; Ibort, A.; Marmo, G. The topology and geometry of self-adjoint and elliptic boundary conditions for Dirac and Laplace operators. Int. J. Geom. Methods Mod. Phys. 2015, 12, 1561007. [Google Scholar] [CrossRef]
- Balachandran, A.P.; Bimonte, G.; Marmo, G.; Simoni, A. Topology change and quantum physics. Nucl. Phys. B 1995, 446, 299. [Google Scholar] [CrossRef]
- Mamaev, S.G.; Trunov, N.N. Vacuum expectation values of the energy-momentum tensor of quantized fields on manifolds with different topologies and geometries. III. Sov. Phys. 1980, 23, 551. [Google Scholar] [CrossRef]
- Vassilevich, D.V. Heat kernel expansion: User’s manual. Phys. Rep. 2003, 388, 279–360. [Google Scholar] [CrossRef]
- Blau, S.K.; Visser, M.; Wipf, A. Zeta functions and the Casimir energy. Nucl. Phys. B 1988, 310, 163–180. [Google Scholar] [CrossRef]
- Kirsten, K. Spectral Functions in Mathematics and Physics; Chapman and Hall/CRC: Boca Raton, FL, USA, 2001. [Google Scholar]
- De Paola, R.D.M.; Rodrigues, R.B.; Svaiter, N.F. Casimir Energy of Massless Fermions in the Slab-bag. Mod. Phys. Lett. A 1999, 14, 2353. [Google Scholar] [CrossRef]
- Elizalde, E.; Santos, F.C.; Tort, A.C. The Casimir Energy of a Massive Fermionic Field Confined in a (d + 1)-dimensional Slab-Bag. Int. J. Mod. Phys. A 2003, 18, 1761. [Google Scholar] [CrossRef]
- Kenneth, O.; Klich, I. Opposites attract: A Theorem about the Casimir force. Phys. Rev. Lett. 2006, 97, 160401. [Google Scholar] [CrossRef]
- Mateos-Guilarte, J.; Munoz-Castaneda, J.M.; Pirozhenko, I.; Santamaria-Sanz, L. One-dimensional scattering of fermions on δ-impurities. arXiv 2019, arXiv:1903.05568v2. [Google Scholar]
- Asorey, M.; Clemente-Gallardo, J.; Munoz-Castaneda, J.M. Path integrals and boundary conditions. J. Phys. Conf. Ser. 2007, 87, 012004. [Google Scholar] [CrossRef]
- Sundberg, P.; Jaffe, R.L. The Casimir effect for fermions in one-dimension. Ann. Phys. (N. Y.) 2004, 309, 442. [Google Scholar] [CrossRef]
- Asorey, M.; Ibort, A.; Marmo, G. Path integrals and boundary conditions. In Proceedings of the Meeting on Fundamental Physics ‘A. Galindo’, Madrid, Spain, 26 November 2004. [Google Scholar]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Donaire, M.; Muñoz-Castañeda, J.M.; Nieto, L.M.; Tello-Fraile, M. Field Fluctuations and Casimir Energy of 1D-Fermions. Symmetry 2019, 11, 643. https://doi.org/10.3390/sym11050643
Donaire M, Muñoz-Castañeda JM, Nieto LM, Tello-Fraile M. Field Fluctuations and Casimir Energy of 1D-Fermions. Symmetry. 2019; 11(5):643. https://doi.org/10.3390/sym11050643
Chicago/Turabian StyleDonaire, Manuel, José María Muñoz-Castañeda, Luis Miguel Nieto, and Marcos Tello-Fraile. 2019. "Field Fluctuations and Casimir Energy of 1D-Fermions" Symmetry 11, no. 5: 643. https://doi.org/10.3390/sym11050643
APA StyleDonaire, M., Muñoz-Castañeda, J. M., Nieto, L. M., & Tello-Fraile, M. (2019). Field Fluctuations and Casimir Energy of 1D-Fermions. Symmetry, 11(5), 643. https://doi.org/10.3390/sym11050643





