Nambu-Jona Lasinio and Nonlinear Sigma Models in Condensed Matter Systems
Abstract
1. Introduction
2. NJL Model and Condensed Matter Systems
2.1. Interacting Fermion
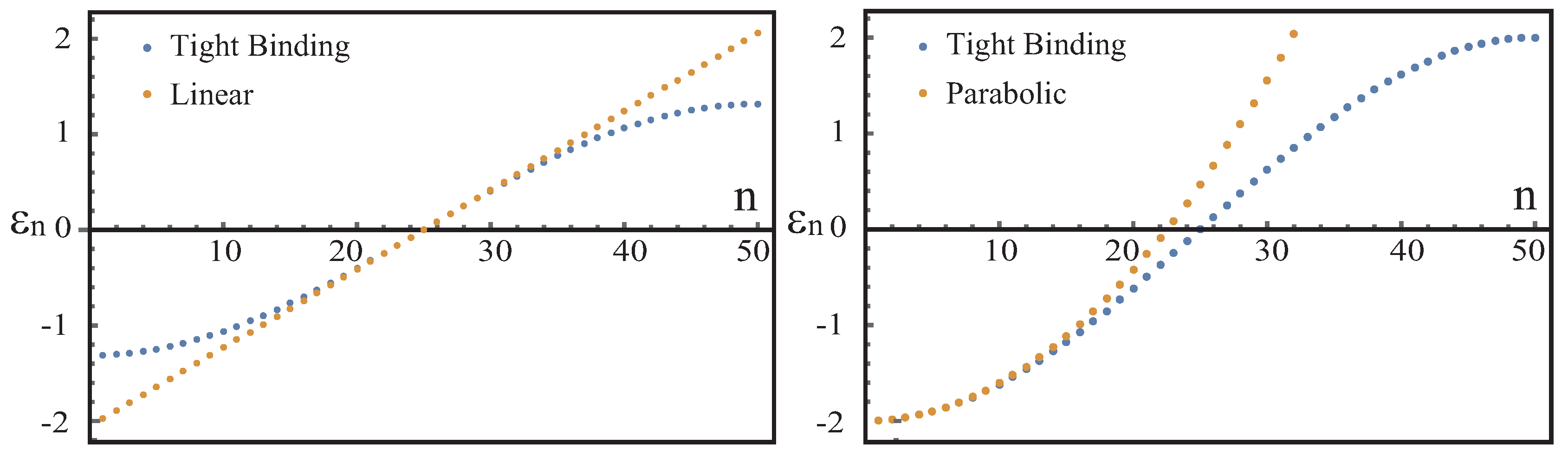
2.2. Discrete Model
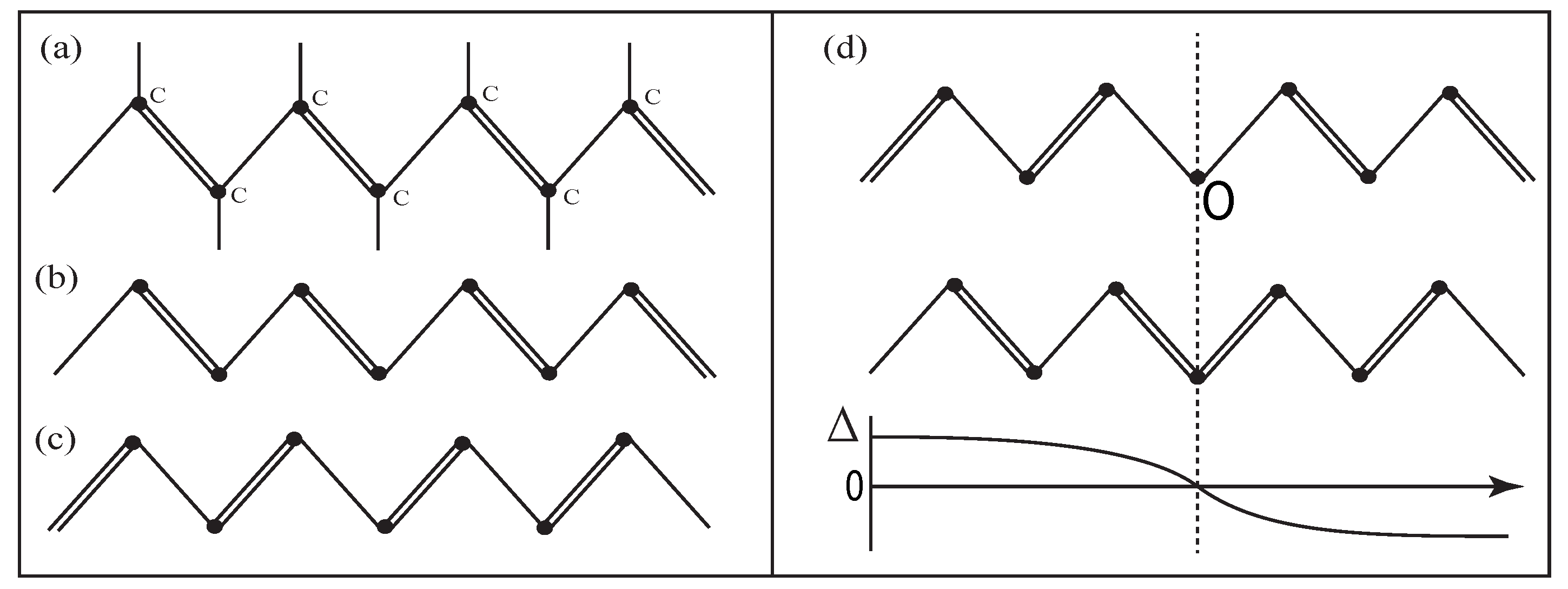
2.3. Conducting Polymers
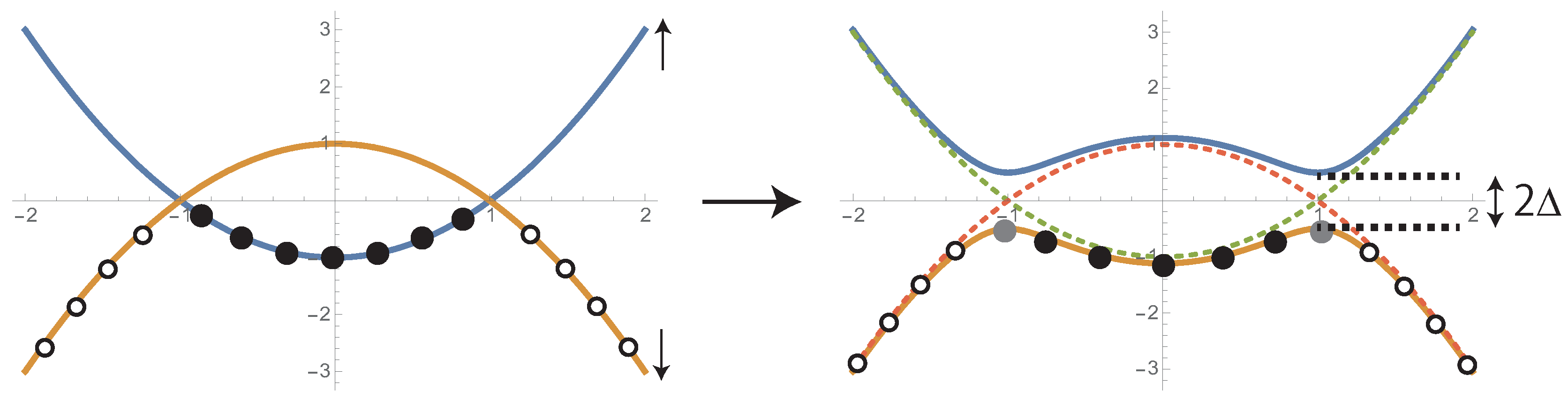
3. Inhomogeneous Solutions
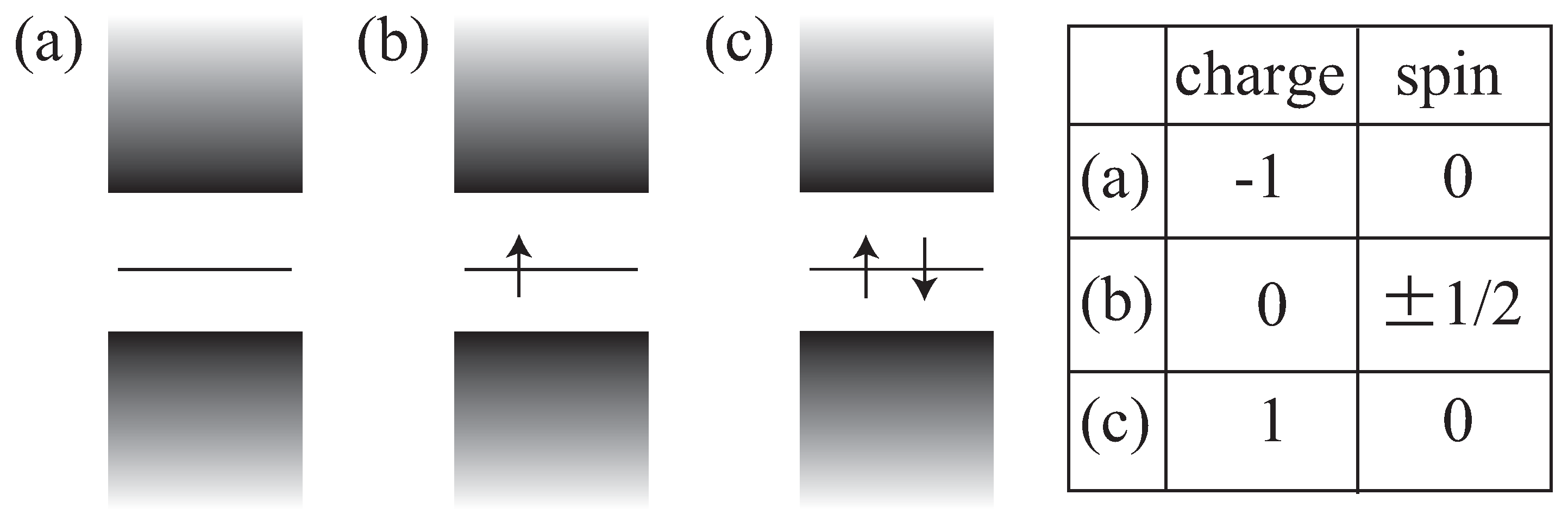
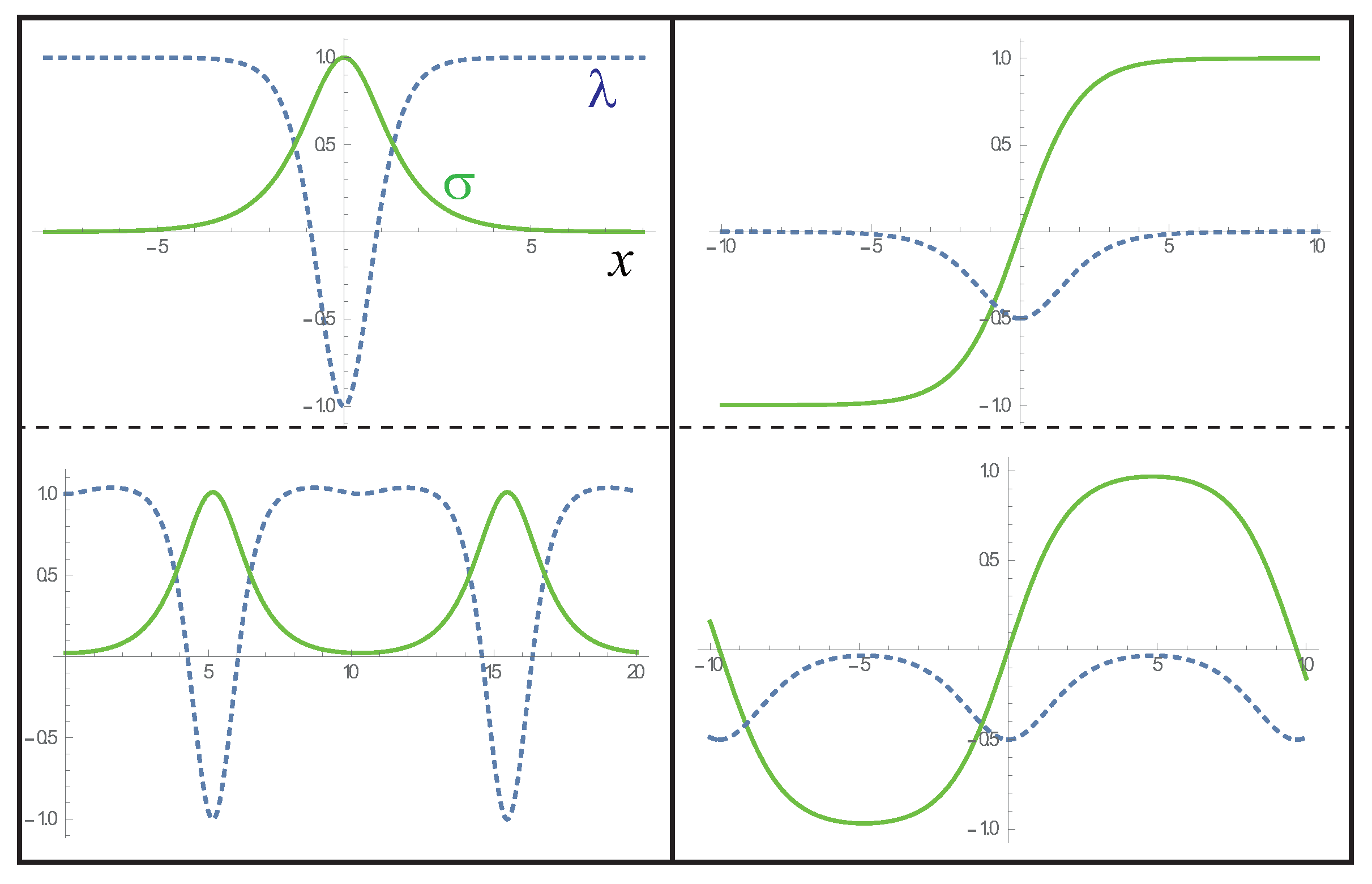
3.1. Kink Solution, Kink Crystal Solution, and Zero Mode
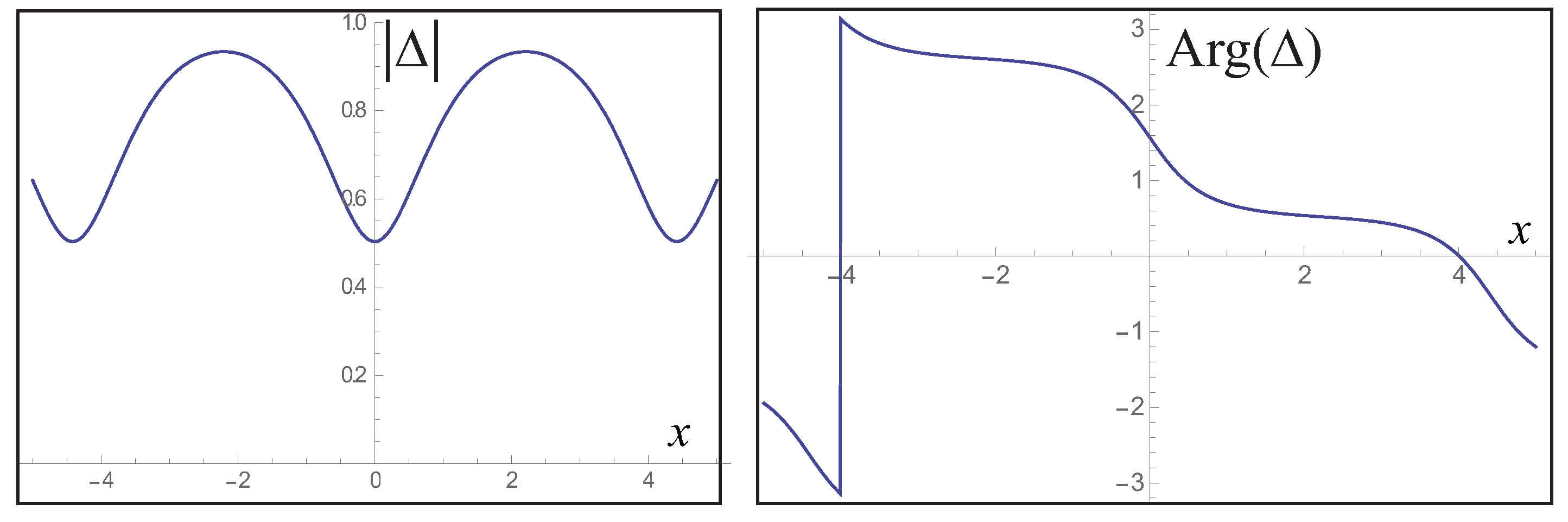
3.2. Nonlinear Schrödinger Equation and Various Inhomogeneous Solutions
3.3. Internal Structures behind the NJL System
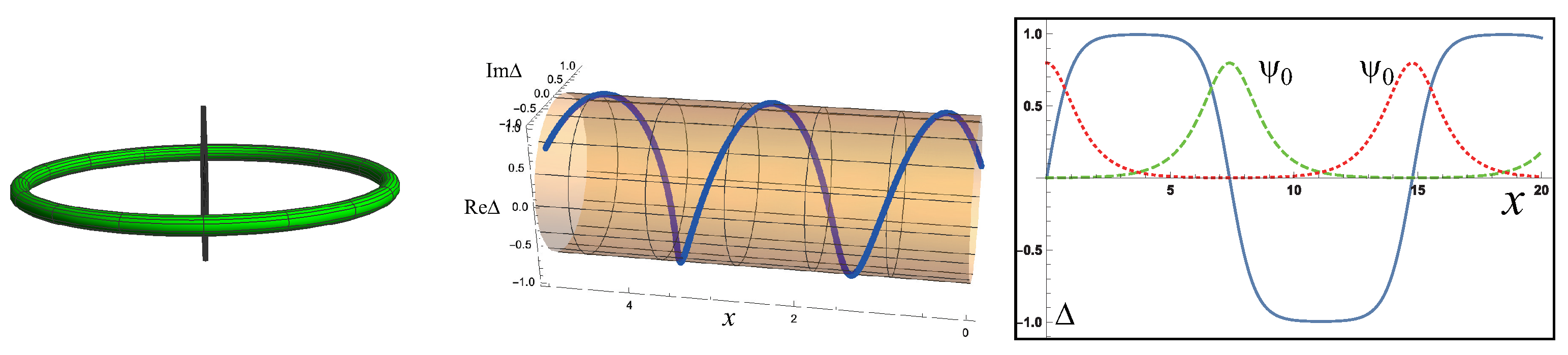
3.4. Inhomogeneous Solutions on a Ring
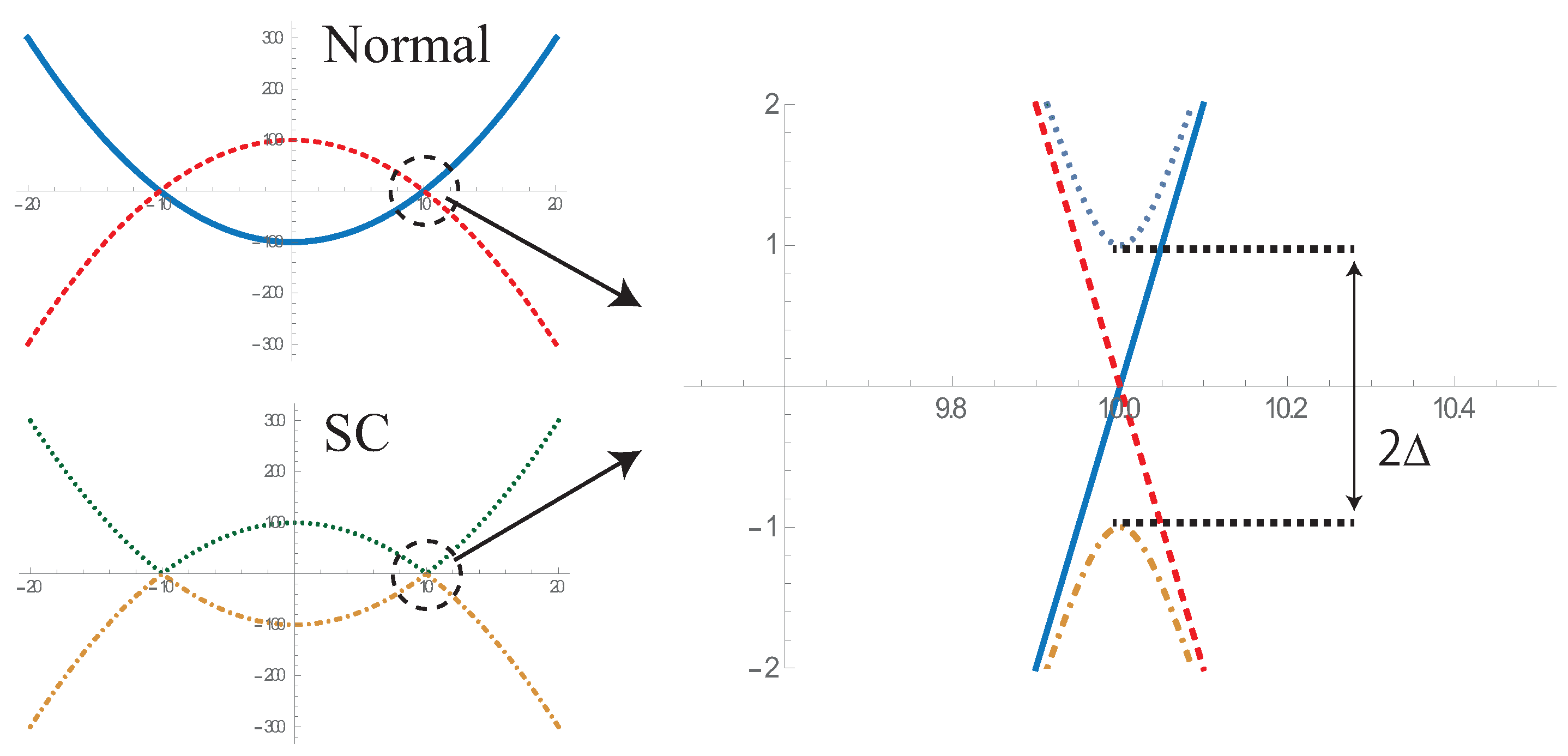
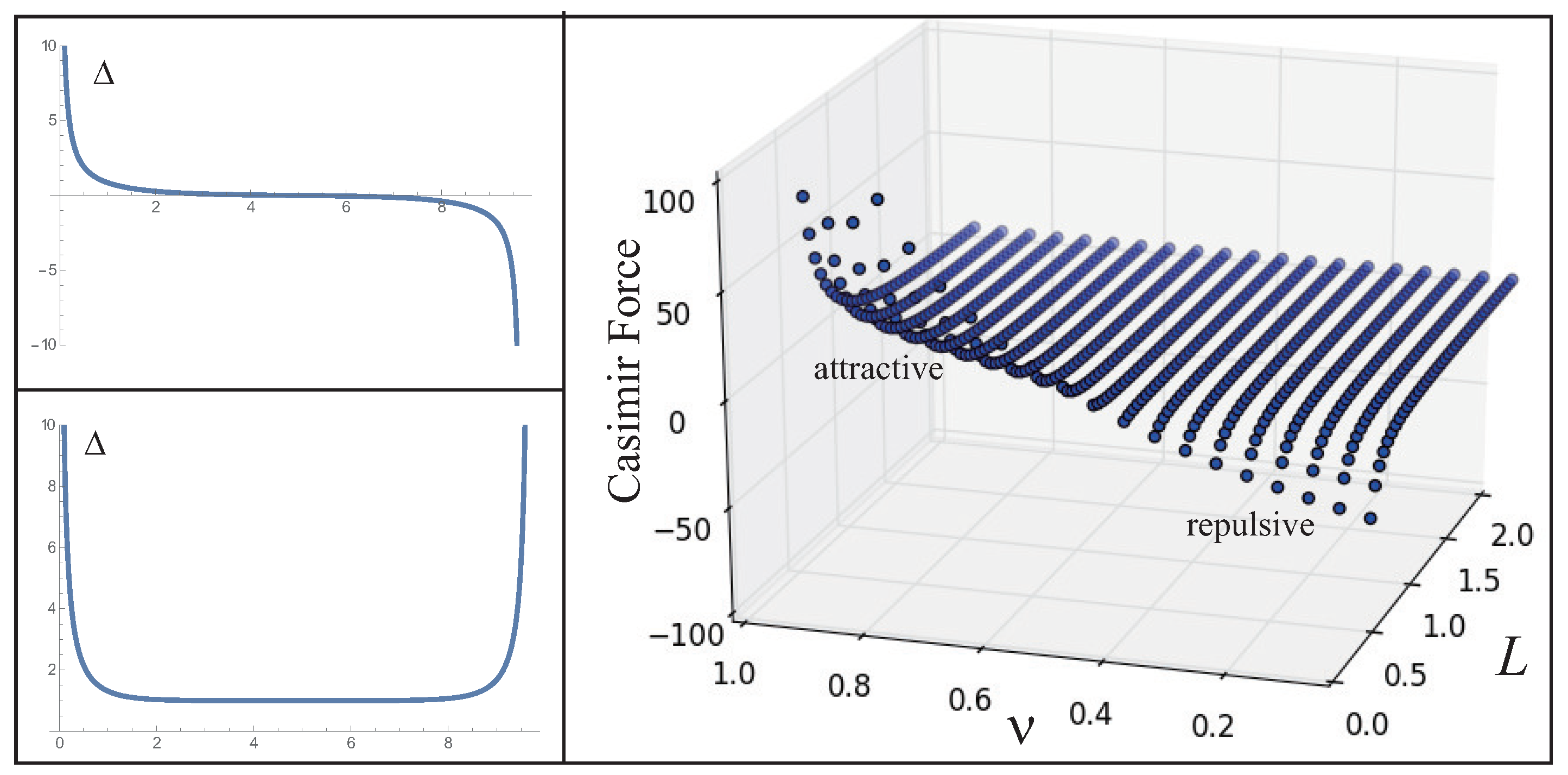
3.5. Finite System and Casimir Force
4. Correspondence between NJL and NL Models
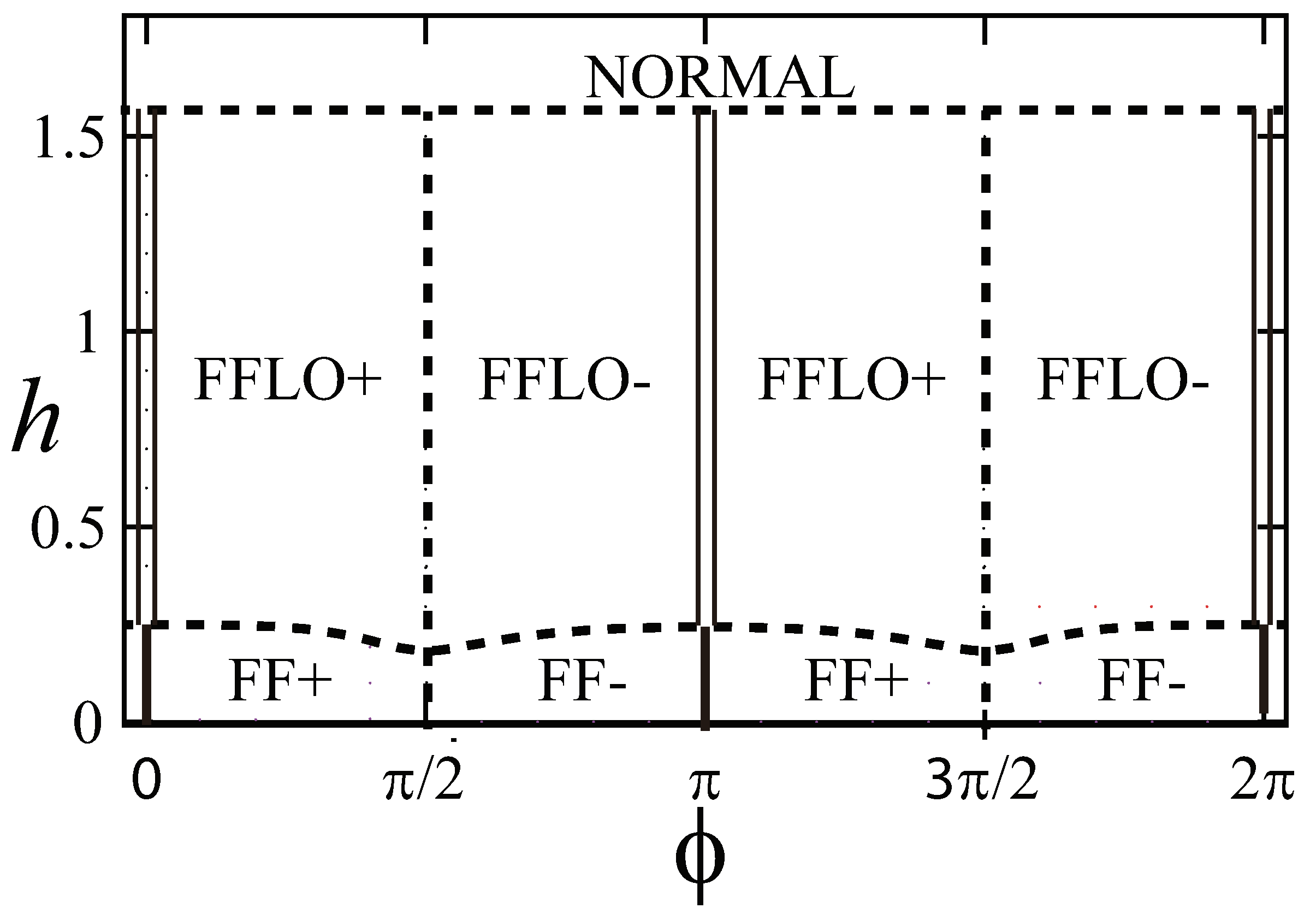
4.1. Model, Method, and Homogeneous Solution
4.2. Inhomogeneous Solutions
4.3. Finite System
4.4. NJL/ Correspondence in 2 + 1 Dimensions
5. Summary
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Landau, L.D. The theory of a Fermi liquid. Sov. Phys. JETP 1956, 30, 1058. [Google Scholar]
- Landau, L.D. Oscillations in a Fermi Liquid. ibid 1957, 32, 59. [Google Scholar]
- Landau, L.D. The Properties of the Green Function for Particles in Statistics. JETP 1958, 34, 262. [Google Scholar]
- Nozieres, P.; Pines, D. Theory of Interacting Fermi Systems; Benjamin: New York, NY, USA, 1965. [Google Scholar]
- Cooper, L.N. Bound Electron Pairs in a Degenerate Fermi gas. Phys. Rev. 1956, 104, 1189. [Google Scholar] [CrossRef]
- Bardeen, J.; Cooper, L.N.; Schrieffer, J.R. Theory of Superconductivity. Phys. Rev. 1957, 108, 1175. [Google Scholar] [CrossRef]
- Ginzburg, V.L.; Landau, L.D. On the Theory of Superconductivity. Zh. Eksp. Teor. Fiz. 1950, 20, 1064. [Google Scholar]
- Landau, L.D. Collected Papers; Pergamon Press: Oxford, UK, 1965; p. 546. [Google Scholar]
- Gor’kov, L.P. Microscopic Derivation of the Ginzburg–Landau Equations in the Theory of Superconductivity. Sov. Phys. JETP 1959, 36, 1364. [Google Scholar]
- Nambu, Y.; Jona-Lasinio, G. Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. I. Phys. Rev. 1961, 122, 345. [Google Scholar] [CrossRef]
- Nambu, Y.; Jona-Lasinio, G. Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. II. Phys. Rev. 1961, 124, 246. [Google Scholar] [CrossRef]
- Nambu, Y. Quasi-Particles and Gauge Invariance in the Theory of Superconductivity. Phys. Rev. 1960, 117, 648. [Google Scholar] [CrossRef]
- Ambegaokar, V.; Kadanoff, L.P. Electromagnetic Properties of Superconductors. Nuovo Cimento 1961, 22, 914. [Google Scholar] [CrossRef]
- Goldstone, J. Field Theories with Superconductor Solutions. Nuovo Cimento 1961, 19, 154. [Google Scholar] [CrossRef]
- Goldstone, J.; Salam, A.; Weinberg, W. Broken Symmetries. Phys. Rev. 1962, 127, 965. [Google Scholar] [CrossRef]
- Anderson, P.W. Plasmons, Gauge Invariance and Mass. Phys. Rev. 1963, 130, 439. [Google Scholar] [CrossRef]
- Guralnik, G.S.; Hagen, C.R.; Kibble, T.W.B. Global Conservation Laws and Massless Particles. Phys. Rev. Lett. 1964, 13, 585. [Google Scholar] [CrossRef]
- Englert, F.; Brout, R. Broken Symmetry and the Mass of Gauge Vector Mesons. Phys. Rev. Lett. 1964, 13, 321. [Google Scholar] [CrossRef]
- Higgs, P.W. Broken Symmetries, Massless Particles and Gauge Fields. Phys. Lett. 1964, 12, 132. [Google Scholar] [CrossRef]
- Higgs, P.W. Broken Symmetries and the Masses of Gauge Bosons. Phys. Rev. Lett. 1964, 13, 508. [Google Scholar] [CrossRef]
- Gross, D.J.; Neveu, A. Dynamical Symmetry Breaking in Asymptotically Free Field Theories. Phys. Rev. D 1974, 10, 3235. [Google Scholar] [CrossRef]
- Hanany, A.; Tong, D. Vortices, Instantons and Branes. J. High Energy Phys. 2003, 2003, 037. [Google Scholar] [CrossRef]
- Hanany, A.; Tong, D. Vortex Strings and Four-Dimensional Gauge Dynamics. J. High Energy Phys. 2004, 2004, 066. [Google Scholar] [CrossRef]
- Auzzi, R.; Bolognesi, S.; Evslin, J.; Konishi, K.; Yung, A. NonAbelian Superconductors: Vortices and Confinement in N = 2 SQCD. Nucl. Phys. B 2003, 673, 187. [Google Scholar] [CrossRef]
- Eto, M.; Isozumi, Y.; Nitta, M.; Ohashi, K.; Sakai, N. Moduli Space of Non-Abelian Vortices. Phys. Rev. Lett. 2006, 96, 161601. [Google Scholar] [CrossRef]
- Eto, M.; Konishi, K.; Marmorini, G.; Nitta, M.; Ohashi, K.; Vinci, W.; Yokoi, N. Non-Abelian Vortices of Higher Winding Numbers. Phys. Rev. D 2006, 74, 065021. [Google Scholar] [CrossRef]
- Tong, D. TASI Lectures on Solitons: Instantons, Monopoles, Vortices and Kinks. hep-th/0509216; Quantum Vortex Strings: A Review. Ann. Phys. 2009, 324, 30. [Google Scholar] [CrossRef]
- Eto, M.; Isozumi, Y.; Nitta, M.; Ohashi, K.; Sakai, N. Solitons in the Higgs phase: The Moduli Matrix Approach. J. Phys. A 2006, 39, R315. [Google Scholar] [CrossRef]
- Shifman, M.; Yung, A. Supersymmetric Solitons and How They Help Us Understand Non-Abelian Gauge Theories. Rev. Mod. Phys. 2007, 79, 1139. [Google Scholar] [CrossRef]
- Shifman, M.; Yung, A. NonAbelian String Junctions as Confined Monopoles. Phys. Rev. D 2004, 70, 045004. [Google Scholar] [CrossRef]
- Haldane, F.D.M. Continuum Dynamics of the 1-D Heisenberg Antiferromagnet: Identification with the O(3) Nonlinear Sigma Model. Phys. Lett. A 1983, 93, 464. [Google Scholar] [CrossRef]
- Haldane, F.D.M. Nonlinear Field Theory of Large-Spin Heisenberg Antiferromagnets: Semiclassically Quantized Solitons of the One-Dimensional Easy-Axis Néel State. Phys. Rev. Lett. 1983, 50, 1153. [Google Scholar] [CrossRef]
- Affleck, I. The Quantum Hall Effects, σ-models at θ = π and Quantum Spin Chains. Nucl. Phys. B 1985, 257, 397. [Google Scholar] [CrossRef]
- Senthil, T.; Vishwanath, A.; Balents, L.; Sachdev, S.; Fisher, M.P.A. Deconfined Quantum Critical Points. Science 2004, 303, 1490. [Google Scholar] [CrossRef]
- Nogueira, F.S.; Sudbo, A. Deconfined Quantum Criticality and Conformal Phase Transition in Two-Dimensional Antiferromagnets. EPL 2013, 104, 56004. [Google Scholar] [CrossRef]
- Pruisken, A.M.M. On Localization in the Theory of the Quantized Hall Effect: A Two-Dimensional Realization of the Theta Vacuum. Nucl. Phys. B 1984, 235, 277. [Google Scholar] [CrossRef]
- Witten, E. A Supersymmetric form of the Nonlinear σ-model in Two-Dimensions. Phys. Rev. D 1977, 16, 2991. [Google Scholar] [CrossRef]
- Filothodoros, E.G.; Petkou, A.C.; Vlachos, N.D. 3d Fermion-Boson Map with Imaginary Chemical Potential. Phys. Rev. D 2017, 95, 065029. [Google Scholar] [CrossRef]
- Nitta, M.; Yoshii, R. Self-Consistent Large-N Analytical Solutions of Inhomogneous Condensates in Quantum Model. J. High Energy Phys. 2017, 12, 145. [Google Scholar] [CrossRef]
- Kondo, J. Resistance Minimum in Dilute Magnetic Alloys. Prog. Theor. Phys. 1964, 32, 37. [Google Scholar] [CrossRef]
- Hewson, A.C. The Kondo Problem to Heavy Fermions—Monograph on the Kondo Effect; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Zhang, S.C.; Hansson, T.H.; Kivelson, S. Effective-Field-Theory Model for the Fractional Quantum Hall Effect. Phys. Rev. Lett. 1989, 62, 82. [Google Scholar] [CrossRef]
- Zee, A. Quantum hall fluids. In Field Theory, Topology and Condensed Matter Physics; Geyer, H.B., Ed.; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 1995; Volume 456. [Google Scholar]
- Jarillo-Herrero, P.; Kong, J.; van der Zant, H.S.J.; Dekke, C.; Kouwenhoven, L.P.; de Franceschi, S. Orbital Kondo Effect in Carbon Nanotubes. Nature 2005, 434, 484. [Google Scholar] [CrossRef]
- Liao, Y.; Rittner, A.S.C.; Paprotta, T.; Li, W.; Partridge, G.B.; Hulet, R.G.; Baur, S.K.; Mueller, E.J. Spin-Imbalance in a One-Dimensional Fermi Gas. Nature 2010, 467, 567. [Google Scholar] [CrossRef]
- Ryu, C.; Andersen, M.F.; Cladé, P.; Natarajan, V.; Helmerson, K.; Phillips, W.D. Observation of Persistent Flow of a Bose-Einstein Condensate in a Toroidal Trap. Phys. Rev. Lett. 2007, 99, 260401. [Google Scholar] [CrossRef]
- Heeger, A.J. Nobel Lecture: Semiconducting and Metallic Polymers: The Fourth Generation of Polymeric Materials. Rev. Mod. Phys. 2001, 73, 681. [Google Scholar] [CrossRef]
- de Gennes, P.G. Superconductivity of Metals and Alloys; Benjamin: New York, NY, USA, 1966. [Google Scholar]
- Andreev, A.F. The Thermal Conductivity of the Intermediate State in Superconductors. Sov. Phys. JETP 1964, 19, 1228. [Google Scholar]
- Leggett, A.J. Modern Trends in the Theory of Condensed Matter; Pekalski, A., Przystawa, J., Eds.; Springer: Berlin, Germany, 1980. [Google Scholar]
- Nozières, P.; Schmitt-Rink, S. Bose Condensation in an Attractive Fermion Gas: From Weak to Strong Coupling Superconductivity. J. Low Temp. Phys. 1985, 59, 195. [Google Scholar] [CrossRef]
- Richardson, R.W. A Restricted Class of Exact Eigenstates of the Pairing-Force Hamiltonian. Phys. Lett. 1963, 3, 277. [Google Scholar] [CrossRef]
- Richardson, R.W.; Sherman, N. Exact Eigenstates of the Pairing-Force Hamiltonian. Nucl. Phys. 1964, 52, 221. [Google Scholar] [CrossRef]
- Richardson, R.W.; Sherman, N. Exactly Solvable Many-Boson Model. J. Math. Phys. 1968, 9, 1327. [Google Scholar] [CrossRef]
- Gaudin, M. Etats et Valeurs Propres de l’Hamiltonien d’Appariement; Internal Report D. Ph. T/DOC-11/DD; Service de Physique Théorique, Centre d’Etudes Nucléaires de Saclay: Essonne, France, 1968. [Google Scholar]
- Gaudin, M. Diagonalisation d’une Classe d’Hamiltoniens de Spin. J. Phys. Fr. 1976, 37, 1087. [Google Scholar] [CrossRef]
- Cambiaggio, M.C.; Rivas, A.M.F.; Saraceno, M. Integrability of the Pairing Hamiltonian. Nucl. Phys. A 1997, 624, 157. [Google Scholar] [CrossRef]
- Sierra, G. Conformal Field Theory and the Exact Solution of the BCS Hamiltonian. Nucl. Phys. B 2000, 572, 517. [Google Scholar] [CrossRef]
- Leggett, A.J. Quantum Liquids; Oxford University Press: New York, NY, USA, 2006. [Google Scholar]
- Su, W.P.; Schrieffer, J.R.; Heeger, A.J. Solitons in Polyacetylene. Phys. Rev. Lett. 1979, 42, 1698. [Google Scholar] [CrossRef]
- Takayama, H.; Lin-Liu, Y.R.; Maki, K. Continuum Model for Solitons in Polyacetylene. Phys. Rev. B 1980, 21, 2388. [Google Scholar] [CrossRef]
- Jackiw, R.; Rebbi, C. Solitons with Fermion Number 1/2. Phys. Rev. D 1976, 13, 3398. [Google Scholar] [CrossRef]
- Su, W.P.; Schrieffer, J.R.; Heeger, A.J. Soliton excitations in Polyacetylene. Phys. Rev. B 1980, 22, 2099. [Google Scholar] [CrossRef]
- Weinberger, B.R.; Ehrenfreund, E.; Pron, A.; Heeger, A.J.; MacDiarmid, A.G. Electron Spin Resonance Studies of Magnetic Soliton Defects in Polyacetylene. J. Chem. Phys. 1980, 72, 4749. [Google Scholar] [CrossRef]
- Nechtschein, M.; Devreux, F.; Genoud, F.; Guglielmi, M.; Holczer, K. Magnetic-Resonance Studies in Undoped Trans-Polyacetylene (CH)x. II. Phys. Rev. B 1983, 27, 61. [Google Scholar] [CrossRef]
- Chodos, A.; Minakata, H. Field Theoretical Tools for Polymer and Particle Physics; Lecture Notes in Physics; Springer-Verlag: Berlin, Germany, 1998; Volume 508. [Google Scholar]
- Goldstone, J.; Wilczek, F. Fractional Quantum Numbers On Solitons. Phys. Rev. Lett. 1981, 47, 986. [Google Scholar] [CrossRef]
- Niemi, A.J.; Semenoff, G.W. Fermion Number Fractionization in Quantum Field Theory. Phys. Rep. 1986, 135, 99. [Google Scholar] [CrossRef]
- Brazovskii, S.A.; Gordynin, S.A.; Kirova, N.N. Exact Solution of the Peierls Model with an Arbitrary Number of Electrons in the Unit Cell. Pis. Zh. Eksp. Teor. Fiz. 1980, 31, 486. [Google Scholar]
- Horovitz, B. Soliton Lattice in Polyacetylene, Spin-Peierls Systems, and Two-Dimensional Sine-Gordon Systems. Phys. Rev. Lett. 1981, 46, 742. [Google Scholar] [CrossRef]
- Fulde, P.; Ferrell, R.A. Superconductivity in a Strong Spin-Exchange Field. Phys. Rev. 1964, 135, A550. [Google Scholar] [CrossRef]
- Larkin, A.I.; Ovchinnikov, Y.N. Nonuniform State of Superconductors. Zh. Eksp. Teor. Fiz. 1964, 47, 1136. [Google Scholar]
- Bar-Sagi, J.; Kuper, C.G. Self-Consistent Pair Potential in an Inhomogeneous Superconductor. Phys. Rev. Lett. 1972, 28, 1556. [Google Scholar] [CrossRef]
- Basar, G.; Dunne, G.V. Self-Consistent Crystalline Condensate in Chiral Gross–Neveu and Bogoliubov–de Gennes Systems. Phys. Rev. Lett. 2008, 100, 200404. [Google Scholar] [CrossRef]
- Basar, G.; Dunne, G.V. Twisted Kink Crystal in the Chiral Gross–Neveu Model. Phys. Rev. D 2008, 78, 065022. [Google Scholar] [CrossRef]
- Eilenberger, G. Transformation of Gorkov’s Equation for Type II Superconductors into Transport-like Equations. Z. Phys. 1968, 214, 195. [Google Scholar] [CrossRef]
- Klotzek, A.; Thies, M. Kink Dynamics, Sinh-Gordon Solitons and Strings in AdS(3) from the Gross-Neveu Model. J. Phys. A 2010, 43, 375401. [Google Scholar] [CrossRef]
- Fitzner, C.; Thies, M. Exact Solution of an N Baryon Problem in the Gross–Neveu Model. Phys. Rev. D 2011, 83, 085001. [Google Scholar] [CrossRef]
- Fitzner, C.; Thies, M. Evidence for Factorized Scattering of Composite States in the Gross–Neveu Model. Phys. Rev. D 2012, 85, 105015. [Google Scholar] [CrossRef]
- Fitzner, C.; Thies, M. Breathers and Their Interaction in the Massless Gross–Neveu Model. Phys. Rev. D 2013, 87, 025001. [Google Scholar] [CrossRef]
- Basar, G.; Dunne, G.V. Gross–Neveu Models, Nonlinear Dirac Equations, Surfaces and Strings. J. High Energy Phys. 2010, 1, 127. [Google Scholar]
- Dunne, G.V.; Fitzner, C.; Thies, M. Baryon-Baryon Scattering in the Gross–Neveu Model: The Large N Solution. Phys. Rev. D 2011, 84, 105014. [Google Scholar] [CrossRef]
- Dunne, G.V.; Thies, M. Time-Dependent Hartree-Fock Solution of Gross–Neveu Models: Twisted-Kink Constituents of Baryons and Breathers. Phys. Rev. Lett. 2013, 111, 121602. [Google Scholar] [CrossRef]
- Dunne, G.V.; Thies, M. Full Time-Dependent Hartree-Fock Solution of Large N Gross–Neveu Models. Phys. Rev. D 2014, 89, 025008. [Google Scholar] [CrossRef]
- Efimkin, D.K.; Galitski, V. Moving Solitons in a One-Dimensional Fermionic Superfluid. Phys. Rev. A 2015, 91, 023616. [Google Scholar] [CrossRef]
- Buzdin, A.I.; Tugushev, V.V. Phase Diagrams of Electronic and Superconductlng Transitions to Soliton Lattice States. Zh. Eksp. Teor. Phys. 1983, 85, 735. [Google Scholar]
- Kunihiro, T.; Hatsuda, T. A Self-Consistent Mean-Field Approach to the Dynamical Symmetry Breaking: The Effective Potential of the Nambu and Jona-Lasinio Model. Prog. Theor. Phys. 1984, 71, 6. [Google Scholar] [CrossRef]
- Correa, F.; Dunne, G.V.; Plyushchay, M.S. The Bogoliubov-de Gennes System, the AKNS Hierarchy, and Nonlinear Quantum Mechanical Supersymmetry. Ann. Phys. 2009, 324, 2522. [Google Scholar] [CrossRef][Green Version]
- Takahashi, D.A.; Tsuchiya, S.; Yoshii, R.; Nitta, M. Fermionic Solutions of Chiral Gross–Neveu and Bogoliubov–de Gennes Systems in Nonlinear Schrödinger Hierarchy. Phys. Lett. B 2012, 718, 2. [Google Scholar] [CrossRef]
- Takahashi, D.A.; Nitta, M. Self-Consistent Multiple Complex-Kink Solutions in Bogoliubov–de Gennes and Chiral Gross–Neveu Systems. Phys. Rev. Lett. 2013, 110, 131601. [Google Scholar] [CrossRef]
- Takahashi, D.A.; Nitta, M. On Reflectionless Nature of Self-Consistent Multi-Soliton Solutions in Bogoliubov-de Gennes and Chiral Gross–Neveu Models. J. Low Temp. Phys. 2014, 175, 250. [Google Scholar] [CrossRef]
- Ablowitz, M.J.; Kaup, D.J.; Newell, A.C.; Segur, H. The Inverse Scattering Transform-Fourier Analysis for Nonlinear Problems. Stud. Appl. Math. 1974, 53, 249. [Google Scholar] [CrossRef]
- Dashen, R.F.; Hasslacher, B.; Neveu, A. Semiclassical Bound States in an Asymptotically Free Theory. Phys. Rev. D 1975, 12, 2443. [Google Scholar] [CrossRef]
- Campbell, D.K.; Bishop, A.R. Solitons in Polyacetylene and Relativistic-Field-Theory Models. Phys. Rev. B 1981, 24, 4859. [Google Scholar] [CrossRef]
- Campbell, D.K.; Bishop, A.R. Soliton Excitations in Polyacetylene and Relativistic Field Theory Models. Nucl. Phys. B 1982, 200, 297. [Google Scholar] [CrossRef]
- Okuno, S.; Onodera, Y. Coexistence of a Soliton and a Polaron in Trans-Polyacetylene. J. Phys. Soc. Jpn. 1983, 52, 3495. [Google Scholar] [CrossRef]
- Feinberg, J. Marginally Stable Topologically Non-Trivial Solitons in the Gross–Neveu Model. Phys. Lett. B 2003, 569, 204. [Google Scholar] [CrossRef][Green Version]
- Feinberg, J. All about the Static Fermion Bags in the Gross–Neveu Model. Ann. Phys. 2004, 309, 166. [Google Scholar] [CrossRef][Green Version]
- Feinberg, J. Kinks and Bound States in the Gross–Neveu Model. Phys. Rev. D 1995, 51, 4503. [Google Scholar] [CrossRef]
- Cooper, F.; Khare, A.; Sukhatme, U. Supersymmetry and Quantum Mechanics. Phys. Rept. 1995, 251, 267. [Google Scholar] [CrossRef]
- Flachi, N.; Nitta, M.; Takada, S.; Yoshii, R. Sign Flip in the Casimir Force for Interacting Fermion Systems. Phys. Rev. Lett. 2017, 119, 031601. [Google Scholar] [CrossRef] [PubMed]
- Machida, K.; Nakanishi, H. Superconductivity under a Ferromagnetic Molecular Field. Phys. Rev. B 1984, 30, 122. [Google Scholar] [CrossRef]
- Sarma, G. On the Influence of a Uniform Exchange Field Acting on the Spins of the Conduction Electrons in a Superconductor. J. Phys. Chem. Solids 1963, 24, 1029. [Google Scholar] [CrossRef]
- Liu, W.V.; Wilczek, F. Interior Gap Superfluidity. Phys. Rev. Lett. 2003, 90, 047002. [Google Scholar] [CrossRef]
- Quan, H.T.; Zhu, J.-X. Interplay between the Fulde-Ferrell-like Phase and Larkin-Ovchinnikov Phase in the Superconducting Ring Pierced by an Aharonov-Bohm Flux. Phys. Rev. B 2010, 81, 014518. [Google Scholar] [CrossRef]
- Yoshida, T.; Yanase, Y. Rotating Fulde-Ferrell-Larkin-Ovchinnikov State in Cold Fermi Gases. Phys. Rev. A 2011, 84, 063605. [Google Scholar] [CrossRef]
- Yoshii, R.; Takada, S.; Tsuchiya, S.; Marmorini, G.; Hayakawa, H.; Nitta, M. Fulde-Ferrell-Larkin-Ovchinnikov States in a Superconducting Ring with Magnetic Fields: Phase Diagram and the First-Order Phase Transitions. Phys. Rev. B 2015, 92, 224512. [Google Scholar] [CrossRef]
- Yoshii, R.; Tsuchiya, S.; Marmorini, G.; Nitta, M. Spin Imbalance Effect on the Larkin-Ovchinnikov- Fulde-Ferrel State. Phys. Rev. B 2011, 84, 024503. [Google Scholar] [CrossRef]
- Yoshii, R.; Marmorini, G.; Nitta, M. Spin Imbalance Effect on Josephson Junction and Grey Soliton. J. Phys. Soc. Jpn. 2012, 81, 094704. [Google Scholar] [CrossRef]
- Yoshida, T.; Sigrist, M.; Yanase, Y. Pair-Density Wave States through Spin-Orbit Coupling in Multilayer Superconductors. Phys. Rev. B 2012, 86, 134514. [Google Scholar] [CrossRef]
- Nickel, D.; Buballa, M. Solitonic Ground States in (Color) Superconductivity. Phys. Rev. D 2009, 79, 054009. [Google Scholar] [CrossRef]
- Takahashi, M.; Mizushima, T.; Machida, K. Fulde–Ferrell–Larkin–Ovchinnikov States in Two-Band Superconductors. J. Phys. Soc. Jpn. 2014, 83, 023703. [Google Scholar]
- Takahashi, M.; Mizushima, T.; Machida, K. Multiband Effects on Fulde-Ferrell-Larkin-Ovchinnikov States of Pauli-Limited Superconductors. Phys. Rev. B 2014, 89, 064505. [Google Scholar] [CrossRef]
- Inagaki, T.; Matsuo, Y.; Shimoji, H. Four-Fermion Interaction Model in D−1⊗S1 and Finite Size Effect. arXiv 2019, arXiv:1903.04244. [Google Scholar]
- Gell-man, M.; Lévy, M. The Axial Vector Current in Beta Decay. Nuovo Cimento 1984, 16, 705. [Google Scholar] [CrossRef]
- Coleman, S.; Jackiw, R.; Politzer, H.D. Spontaneous Symmetry Breaking in the O(N) Model for Large N. Phys. Rev. D 1974, 10, 2491. [Google Scholar] [CrossRef]
- Bolognesi, S.; Konishi, K.; Ohashi, K. Large-N Sigma Model on a Finite Interval. J. High Energy Phys. 2016, 1610, 073. [Google Scholar] [CrossRef]
- Coleman, S.R. There are No Goldstone Bosons in Two-Dimensions. Commun. Math. Phys. 1973, 31, 259. [Google Scholar] [CrossRef]
- Mermin, N.D.; Wagner, H. Absence of Ferromagnetism or Antiferromagnetism in One-Dimensional or Two-Dimensional Isotropic Heisenberg Models. Phys. Rev. Lett. 1966, 17, 1133. [Google Scholar] [CrossRef]
- Nitta, M.; Yoshii, R. Confining Solitons in the Higgs Phase of Model: Self-Consistent Exact Solutions in Large-N Limit. J. High Energy Phys. 2018, 8, 007. [Google Scholar] [CrossRef]
- Gorsky, A.; Pikalov, A.; Vainshtein, A. On Instability of Ground States in 2D CP(N − 1) and O(N) Models at Large N. arXiv 2018, arXiv:1811.05449. [Google Scholar]
- Betti, A.; Bolognesi, S.; Gudnason, S.B.; Konishi, K.; Ohashi, K. Large-N Sigma Model on a Finite Interval and the Renormalized String Energy. J. High Energy Phys. 2018, 1, 106. [Google Scholar] [CrossRef]
- Flachi, A.; Nitta, M.; Takada, S.; Yoshii, R. Casimir Force for the Model. arXiv 2017, arXiv:1708.08807. [Google Scholar]
- Chernodub, M.N.; Goy, V.A.; Molochkov, A.V. Casimir effect and deconfinement phase transition. Phys. Rev. D 2017, 96, 094507. [Google Scholar] [CrossRef]
- Chernodub, M.N.; Goy, V.A.; Molochkov, A.V. Nonperturbative Casimir Effects in Field Theories: Aspects of confinement, dynamical mass generation and chiral symmetry breaking. arXiv 2019, arXiv:1901.04754. [Google Scholar]
- Chernodub, M.N.; Goy, V.A.; Molochkov, A.V.; Nguyen, H.H. Casimir Effect in Yang-Mills Theory in D = 2 + 1. Phys. Rev. Lett. 2018, 121, 191601. [Google Scholar] [CrossRef]
- Pikalov, A. CP(N) model on Regions with Boundary. arXiv 2017, arXiv:1710.00699. [Google Scholar]
- Monin, S.; Shifman, M.; Yung, A. Non-Abelian String of a Finite Length. Phys. Rev. D 2015, 92, 025011. [Google Scholar] [CrossRef]
- Monin, S.; Shifman, M.; Yung, A. Heterotic Non-Abelian String of a Finite Length. Phys. Rev. D 2016, 93, 125020. [Google Scholar] [CrossRef]
- Milekhin, A. CP(N − 1) Model on Finite Interval in the Large N Limit. Phys. Rev. D 2012, 86, 105002. [Google Scholar] [CrossRef]
- Milekhin, A. CP(N) Sigma Model on a Finite Interval Revisited. Phys. Rev. D 2017, 95, 085021. [Google Scholar] [CrossRef]
- Pavshinkin, D. Grassmannian Sigma Model on a Finite Interval. Phys. Rev. D 2018, 97, 025001. [Google Scholar] [CrossRef]
- Nitta, M.; Yoshii, R. Self-Consistent Analytic Solutions in Twisted Model in the Large-N Limit. J. High Energy Phys. 2018, 9, 092. [Google Scholar] [CrossRef]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yoshii, R.; Nitta, M. Nambu-Jona Lasinio and Nonlinear Sigma Models in Condensed Matter Systems. Symmetry 2019, 11, 636. https://doi.org/10.3390/sym11050636
Yoshii R, Nitta M. Nambu-Jona Lasinio and Nonlinear Sigma Models in Condensed Matter Systems. Symmetry. 2019; 11(5):636. https://doi.org/10.3390/sym11050636
Chicago/Turabian StyleYoshii, Ryosuke, and Muneto Nitta. 2019. "Nambu-Jona Lasinio and Nonlinear Sigma Models in Condensed Matter Systems" Symmetry 11, no. 5: 636. https://doi.org/10.3390/sym11050636
APA StyleYoshii, R., & Nitta, M. (2019). Nambu-Jona Lasinio and Nonlinear Sigma Models in Condensed Matter Systems. Symmetry, 11(5), 636. https://doi.org/10.3390/sym11050636



