Analytical Solutions of Fractional Klein-Gordon and Gas Dynamics Equations, via the (G′/G)-Expansion Method
Abstract
1. Introduction
2. Method and Materials
3. Results
4. Discussion
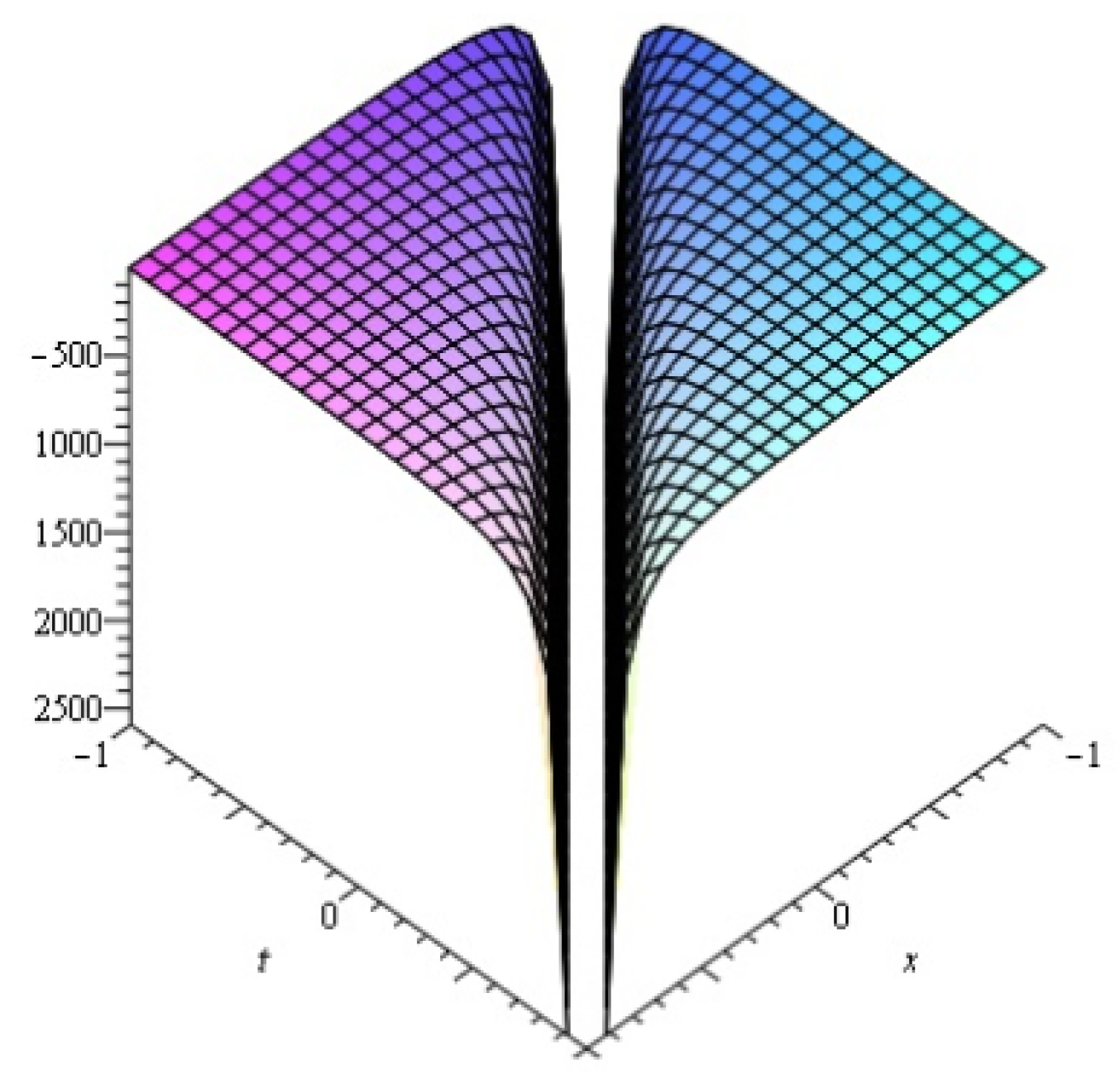
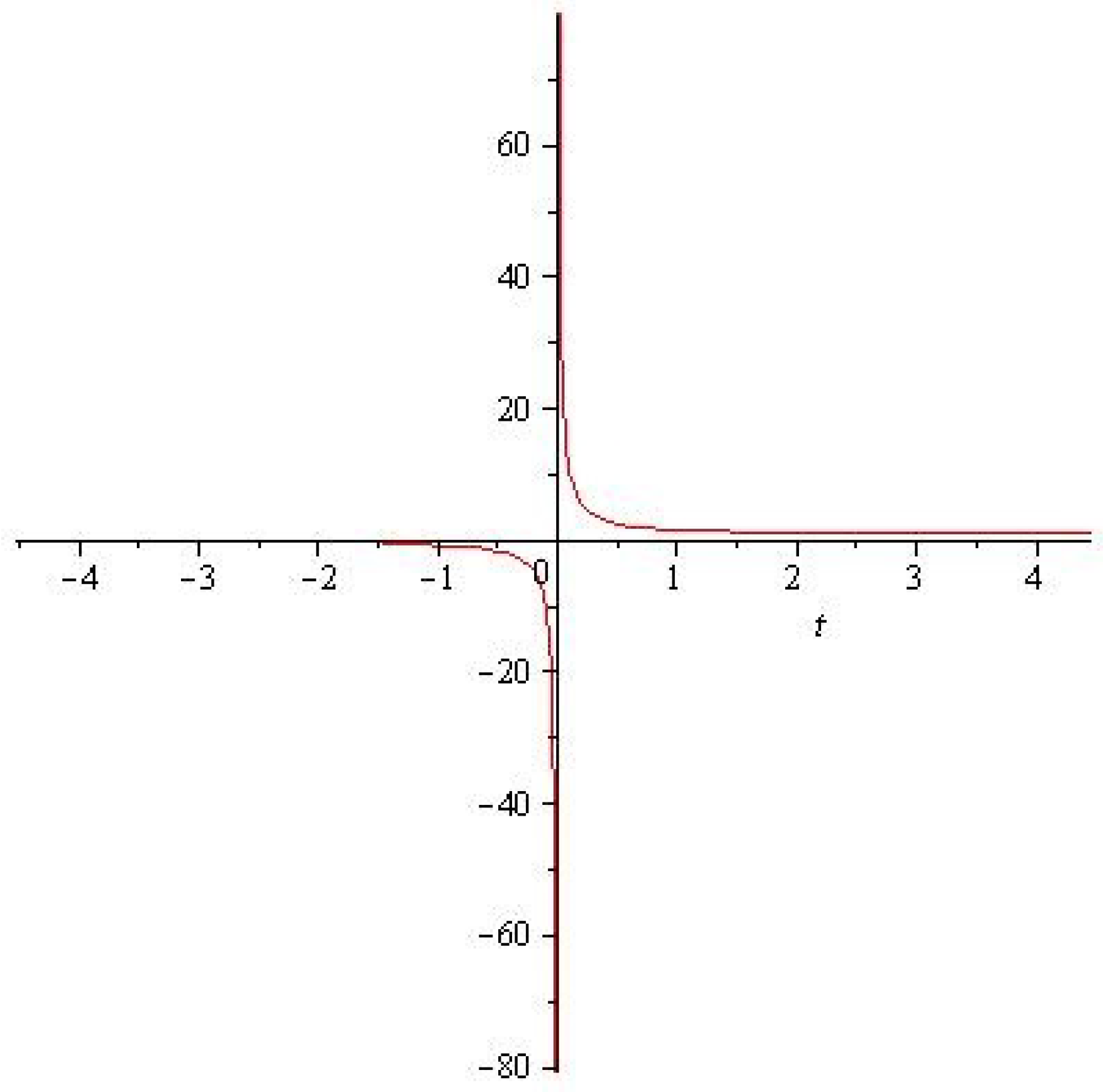
5. Description of Figures
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Almeida, R.; Bastos, N.R.O.; Teresa, M.; Monteiro, T. Modeling some real phenomena by fractional differential equationsh. Math. Methods Appl. Sci. 2016, 39, 4846–4855. [Google Scholar] [CrossRef]
- Sun, H.; Zhang, Y.; Baleanu, D.; Chen, W.; Chen, Y. A new collection of real world applications of fractional calculus in science and engineering. Commun. Nonlinear Sci. Numer. Simul. 2018, 64, 213–231. [Google Scholar] [CrossRef]
- Tarasov, V. Generalized memory: Fractional calculus approach. Fractal Fract. 2018, 2, 23. [Google Scholar] [CrossRef]
- El-Misiery, A.E.M.; Ahmed, E. On a fractional model for earthquakes. Appl. Math. Comput. 2006, 178, 207–211. [Google Scholar] [CrossRef]
- Wu, J. A wavelet operational method for solving fractional partial differential equations numerically. Appl. Math. Comput. 2009, 214, 31–40. [Google Scholar] [CrossRef]
- Zhang, W.; Li, J.; Yang, Y. A fractional diffusion-wave equation with non-local regularization for image denoising. Signal Process. 2014, 103, 6–15. [Google Scholar] [CrossRef]
- Hu, Y.; Øksendal, B. Fractional white noise calculus and applications to finance. Infin. Dimens. Anal. Quantum Probab. Relat. Top. 2003, 6, 1–32. [Google Scholar] [CrossRef]
- Nagy, A.M. Numerical solution of time fractional nonlinear Klein-Gordon equation using Sinc-Chebyshev collocation method. Appl. Math. Comput. 2017, 310, 139–148. [Google Scholar] [CrossRef]
- Garrappa, R. Numerical solution of fractional differential equations: A survey and a software tutorial. Mathematics 2018, 6, 16. [Google Scholar] [CrossRef]
- Jiang, J.; Feng, Y.; Li, S. Exact Solutions to the Fractional Differential Equations with Mixed Partial Derivatives. Axioms 2018, 7, 10. [Google Scholar] [CrossRef]
- Song, J.; Yin, F.; Cao, X.; Lu, F. Fractional variational iteration method versus Adomian’s decomposition method in some fractional partial differential equations. J. Appl. Math. 2013, 2013, 392567. [Google Scholar] [CrossRef]
- Duan, J.; Rach, R.; Baleanu, D.; Wazwaz, A. A review of the Adomian decomposition method and its applications to fractional differential equations. Commun. Fract. Calc. 2012, 3, 73–99. [Google Scholar]
- Mahmood, S.; Shah, R.; Arif, M. Laplace Adomian Decomposition Method for Multi Dimensional Time Fractional Model of Navier-Stokes Equation. Symmetry 2019, 11, 149. [Google Scholar] [CrossRef]
- Wang, Q. Homotopy perturbation method for fractional KdV-Burgers equation. Chaos Solitons Fractals 2008, 35, 843–850. [Google Scholar] [CrossRef]
- Raslan, K.R.; Ali, K.K.; Shallal, M.A. The modified extended tanh method with the Riccati equation for solving the space-time fractional EW and MEW equations. Chaos Solitons Fractals 2017, 103, 404–409. [Google Scholar] [CrossRef]
- Alzaidy, J.F. Fractional sub-equation method and its applications to the space-time fractional differential equations in mathematical physics. Br. J. Math. Comput. Sci. 2013, 3, 153. [Google Scholar] [CrossRef]
- Tasbozan, O.; Çenesiz, Y.; Kurt, A. New solutions for conformable fractional Boussinesq and combined KdV-mKdV equations using Jacobi elliptic function expansion method. Eur. Phys. J. Plus 2016, 131, 244. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Tadjeran, C. Finite difference approximations for fractional advection–dispersion flow equations. J. Comput. Appl. Math. 2004, 172, 65–77. [Google Scholar] [CrossRef]
- Jiang, Y.; Ma, J. High-order finite element methods for time-fractional partial differential equations. J. Comput. Appl. Math. 2011, 235, 3285–3290. [Google Scholar] [CrossRef]
- Zheng, B. Exp-function method for solving fractional partial differential equations. Sci. World J. 2013, 2013, 465723. [Google Scholar] [CrossRef]
- Shang, N.; Zheng, B. Exact solutions for three fractional partial differential equations by the ()-expansion method. Int. J. Appl. Math. 2013, 43, 114–119. [Google Scholar]
- Zhang, Y. Solving STO and KD equations with modified Riemann–Liouville derivative using improved ()-expansion function method. Int. J. Appl. Math. 2015, 45, 16–22. [Google Scholar]
- Shakeel, M.; Mohyud-Din, S.T. New ()-expansion method and its application to the zakharov-kuznetsov-benjamin-bona-mahony (ZK–BBM) equation. J. Assoc. Arab Univ. Basic Appl. Sci. 2015, 18, 66–81. [Google Scholar] [CrossRef]
- Zheng, B. ()-expansion method for solving fractional partial differential equations in the theory of mathematical physics. Commun. Theor. Phys. 2012, 58, 623. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Amer, Y.A.; Shohib, R.M.A. Exact traveling wave solutions for nonlinear fractional partial differential equations using the improved ()-expansion method. Int. J. Eng. 2014, 4, 8269. [Google Scholar]
- Abuteen, E.; Freihat, A.; Al-Smadi, M.; Khalil, H.; Khan, R.A. Approximate series solution of nonlinear, fractional Klein-Gordon equations using fractional reduced differential transform method. arXiv 2017, arXiv:1704.06982. [Google Scholar] [CrossRef]
- Acan, O.; Baleanu, D. A new numerical technique for solving fractional partial differential equations. arXiv 2017, arXiv:1704.02575. [Google Scholar] [CrossRef]
- Chowdhury, M.S.H.; Hashim, I. Application of homotopy-perturbation method to Klein–Gordon and sine-Gordon equations. Chaos Solitons Fractals 2009, 39, 1928–1935. [Google Scholar] [CrossRef]
- Kheiri, H.; Shahi, S.; Mojaver, A. Analytical solutions for the fractional Klein-Gordon equation. Comput. Methods Differ. Equ. 2014, 2, 99–114. [Google Scholar]
- Singh, J.; Kumar, D.; Kılıçman, A. Homotopy perturbation method for fractional gas dynamics equation using Sumudu transform. Abst. Appl. Anal. 2013, 2013, 934060. [Google Scholar] [CrossRef]
- Tamsir, M.; Srivastava, V.K. Revisiting the approximate analytical solution of fractional-order gas dynamics equation. Alex. Eng. J. 2016, 55, 867–874. [Google Scholar] [CrossRef]
- Alam, M.; Rahman, M.; Islam, R.; Roshid, H. Application of the new extended (G’/G)-expansion method to find exact solutions for nonlinear partial differential equation. Comput. Methods Differ. Equ. 2015, 3, 59–69. [Google Scholar]
- Ozis, T.; Aslan, I. Symbolic computation and construction of new exact traveling wave solutions to Fitzhugh-Nagumo and Klein-Gordon equations. Z. Naturforschung A 2009, 64, 15. [Google Scholar]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, H.; Barak, S.; Kumam, P.; Arif, M. Analytical Solutions of Fractional Klein-Gordon and Gas Dynamics Equations, via the (G′/G)-Expansion Method. Symmetry 2019, 11, 566. https://doi.org/10.3390/sym11040566
Khan H, Barak S, Kumam P, Arif M. Analytical Solutions of Fractional Klein-Gordon and Gas Dynamics Equations, via the (G′/G)-Expansion Method. Symmetry. 2019; 11(4):566. https://doi.org/10.3390/sym11040566
Chicago/Turabian StyleKhan, Hassan, Shoaib Barak, Poom Kumam, and Muhammad Arif. 2019. "Analytical Solutions of Fractional Klein-Gordon and Gas Dynamics Equations, via the (G′/G)-Expansion Method" Symmetry 11, no. 4: 566. https://doi.org/10.3390/sym11040566
APA StyleKhan, H., Barak, S., Kumam, P., & Arif, M. (2019). Analytical Solutions of Fractional Klein-Gordon and Gas Dynamics Equations, via the (G′/G)-Expansion Method. Symmetry, 11(4), 566. https://doi.org/10.3390/sym11040566






