Stagnation Point Flow and Heat Transfer over an Exponentially Stretching/Shrinking Sheet in CNT with Homogeneous–Heterogeneous Reaction: Stability Analysis
Abstract
1. Introduction
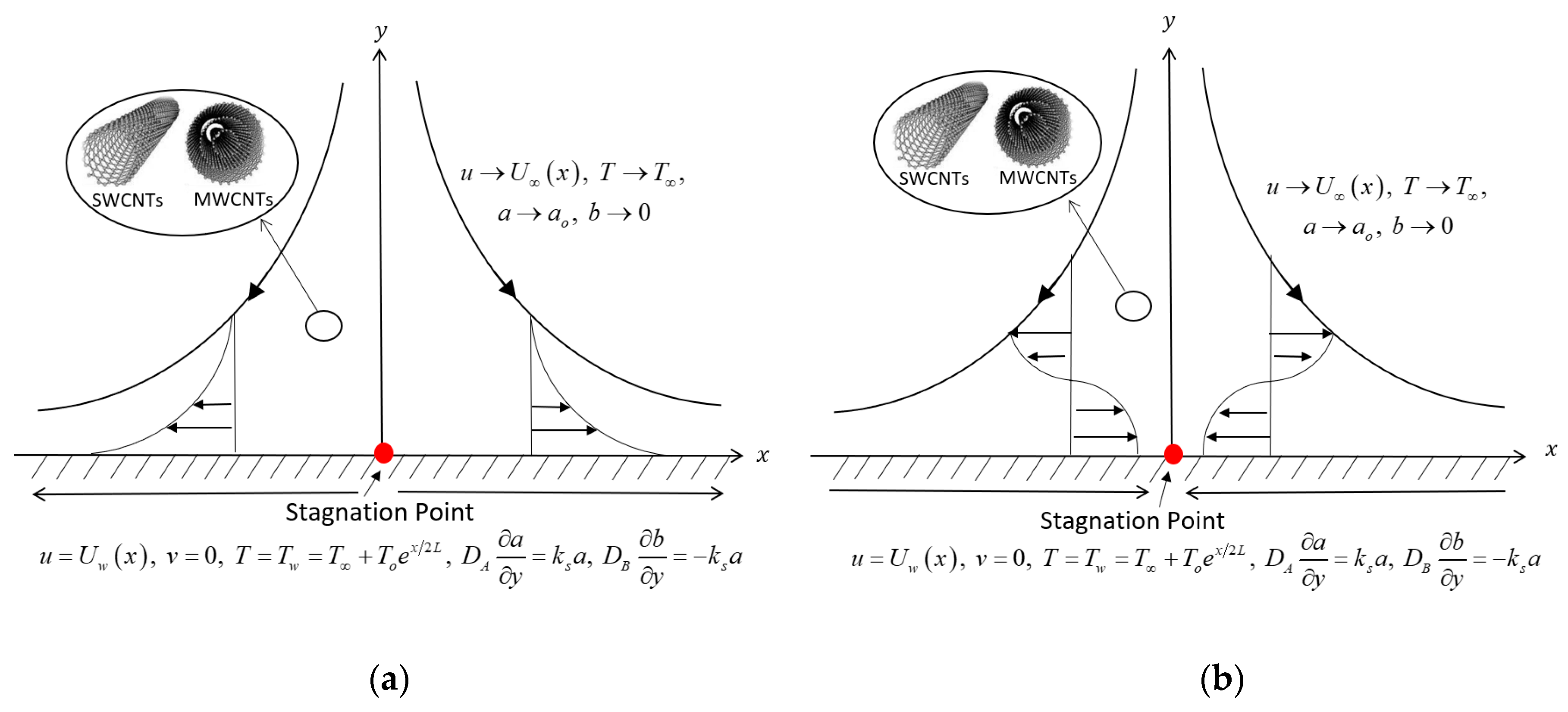
2. Problem Formulation
3. Stability of Solutions
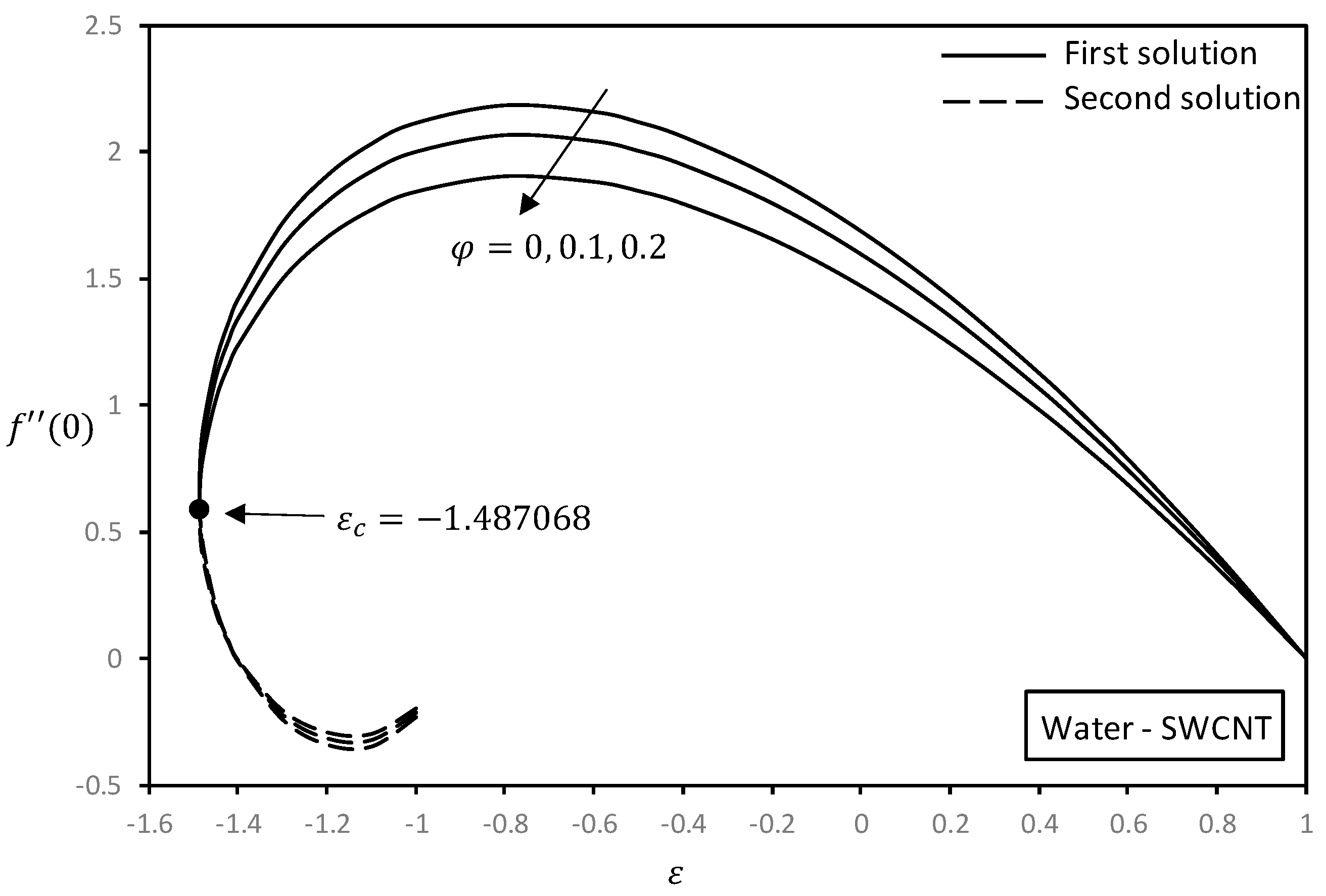
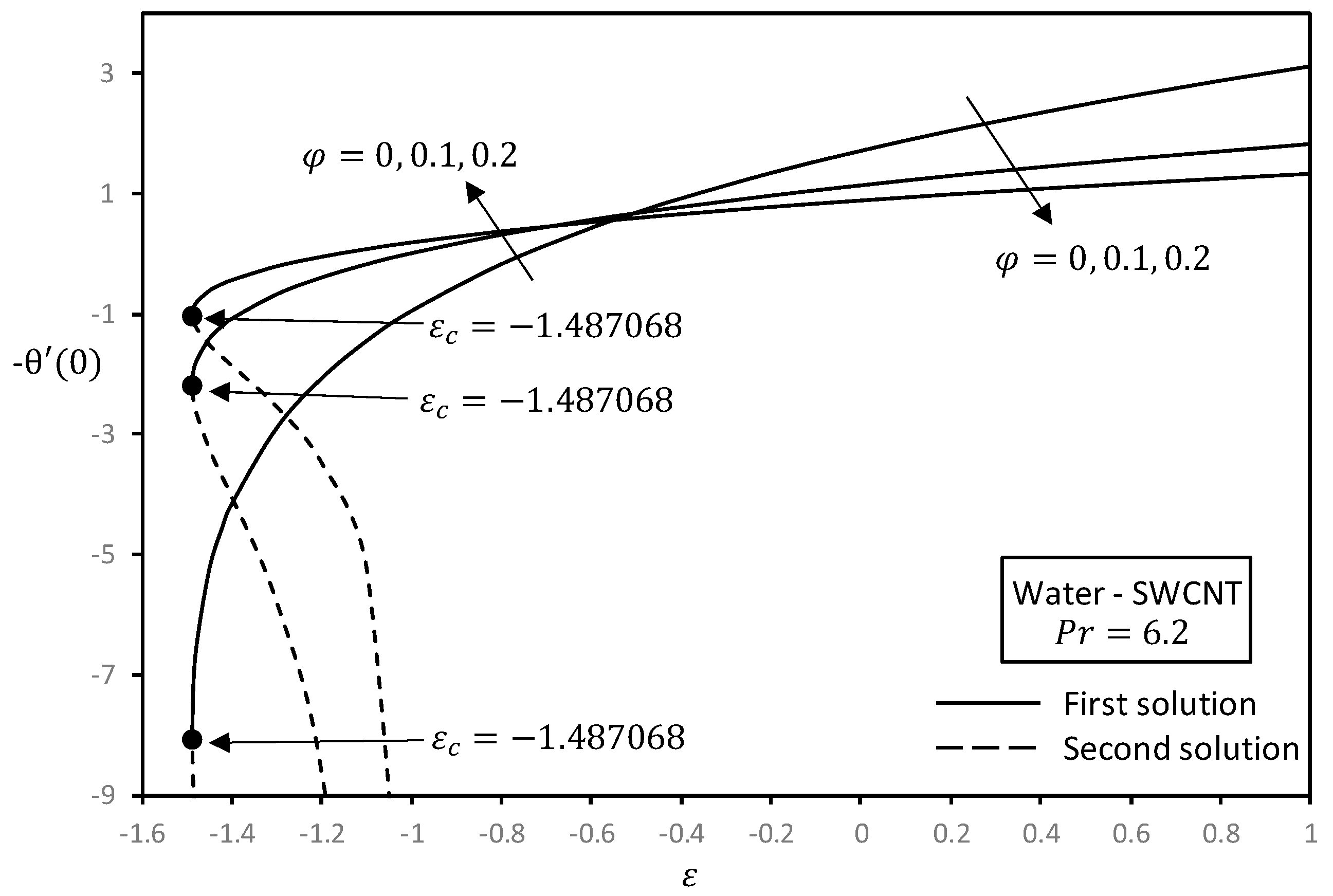
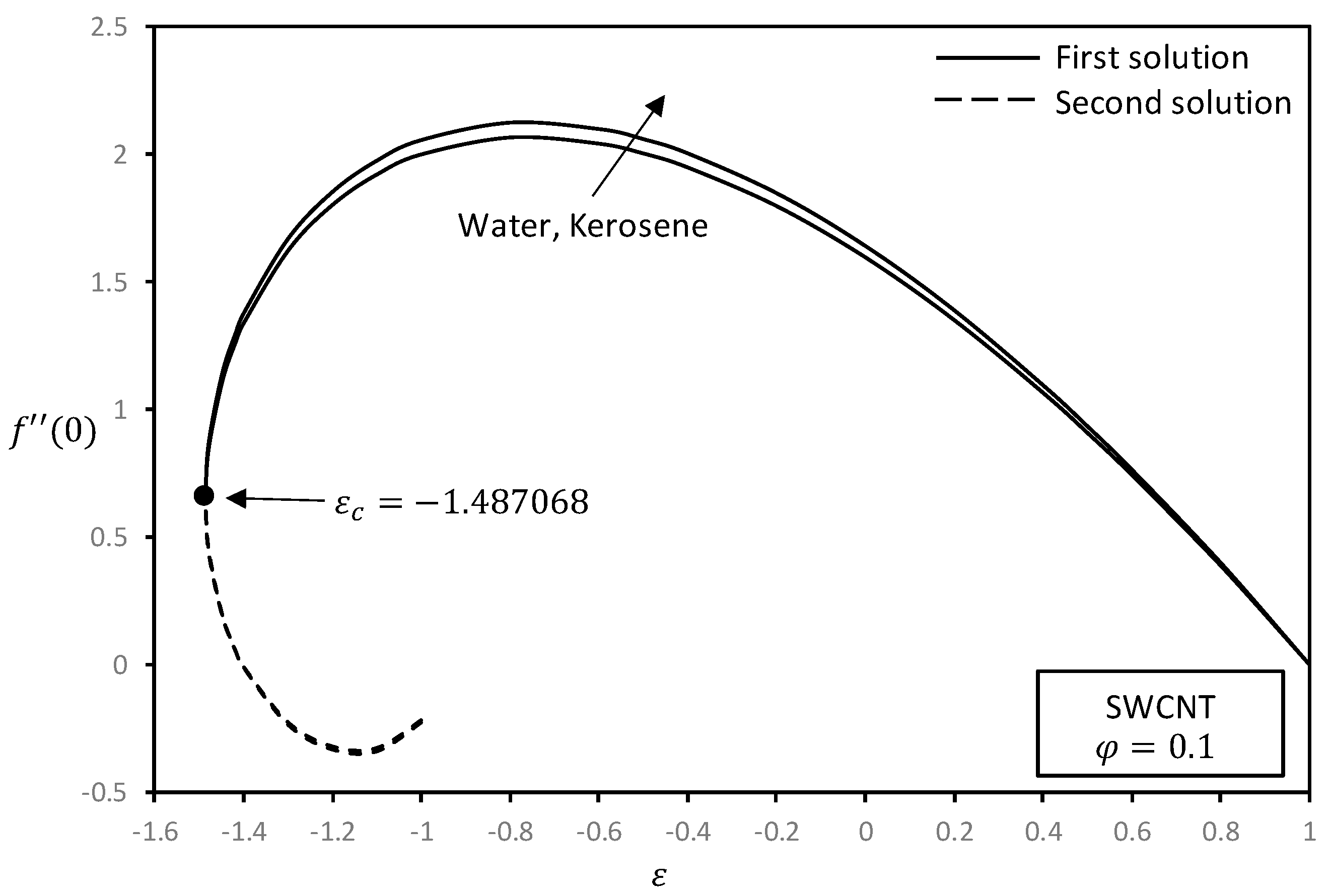
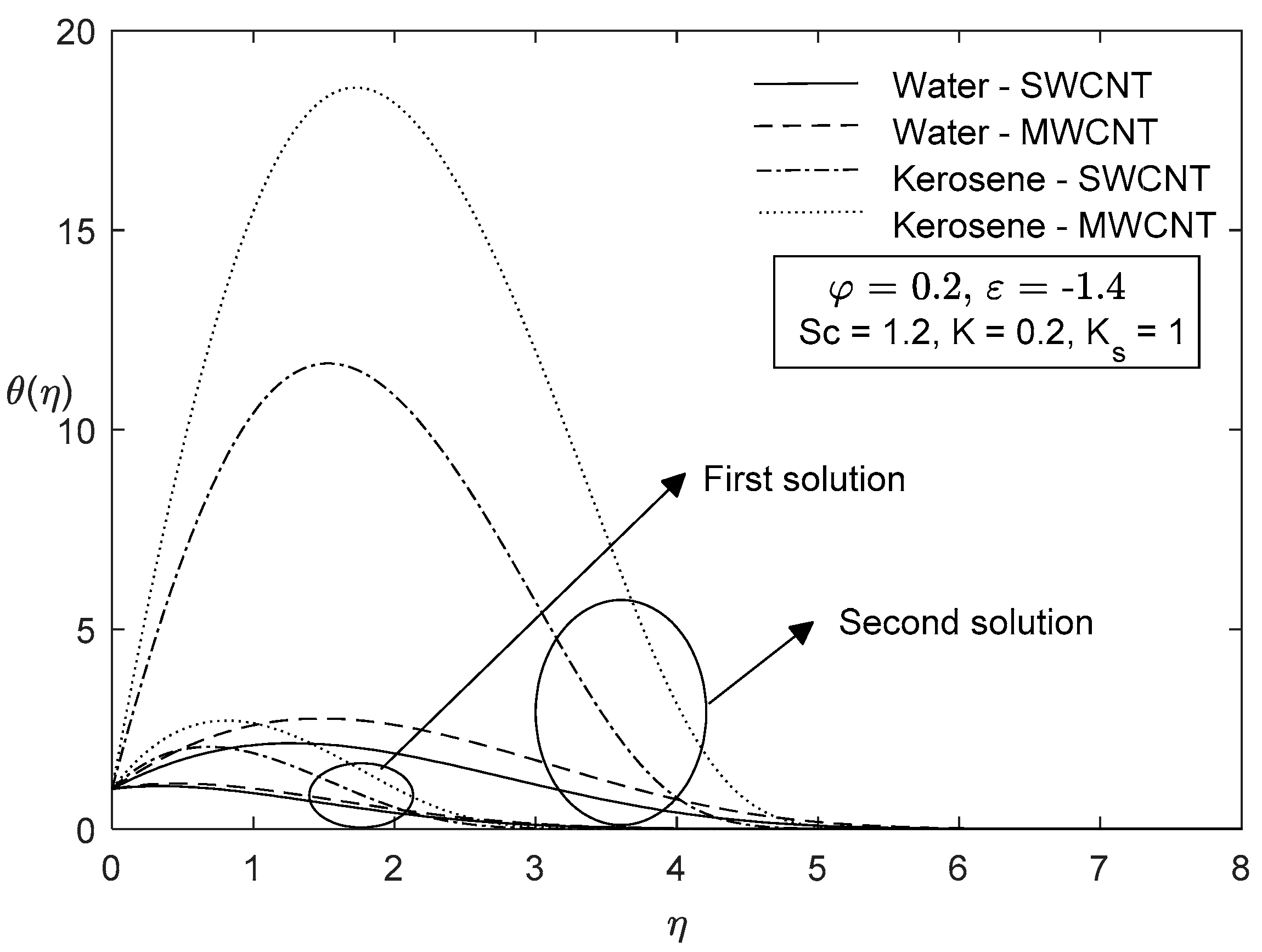
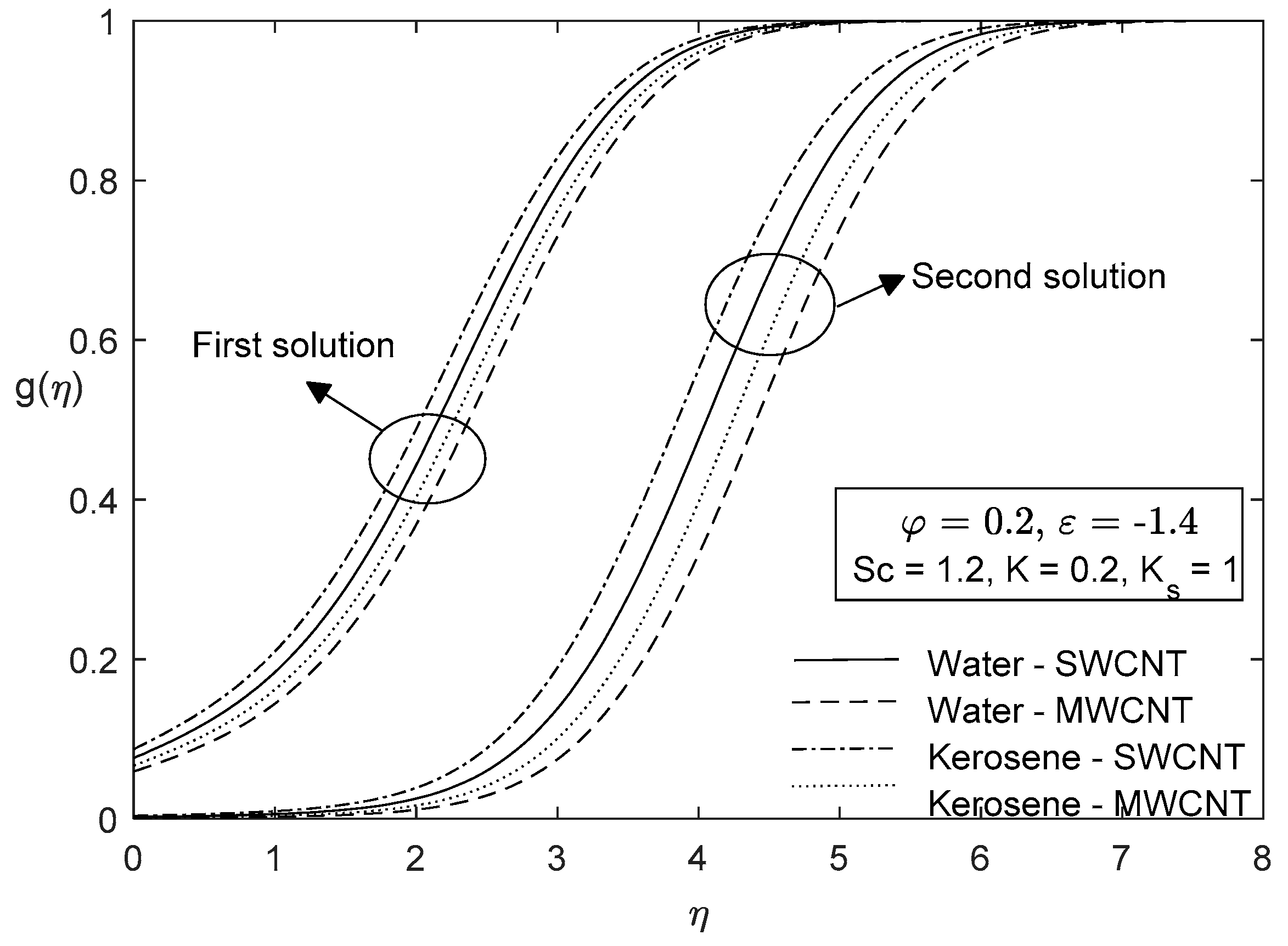
4. Results and Discussion
5. Conclusions
- The skin friction and heat transfer rates increase linearly with nanoparticle volume fraction.
- Kerosene-based CNTs have higher heat transfer rates and skin friction compared to water-based CNTs.
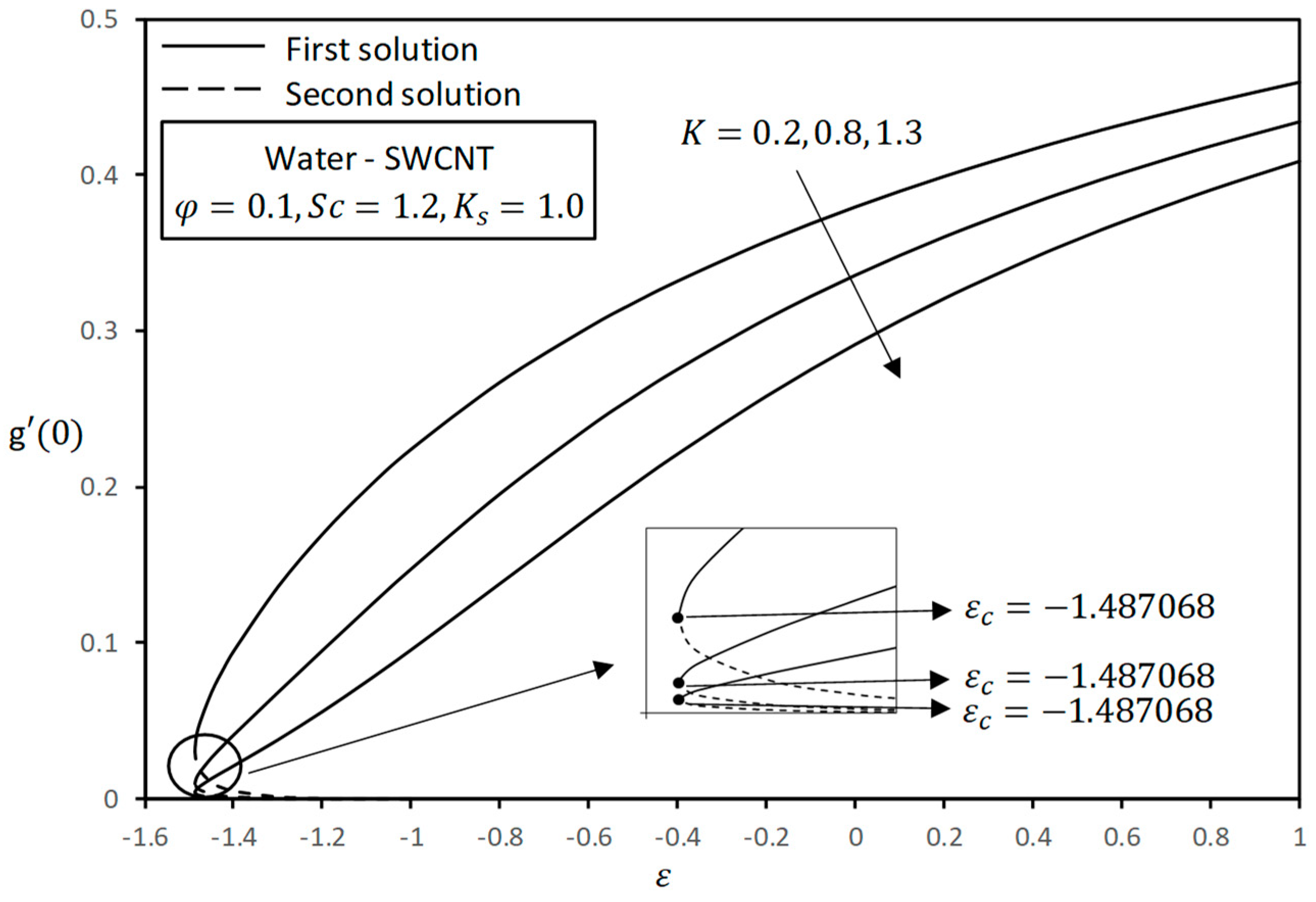
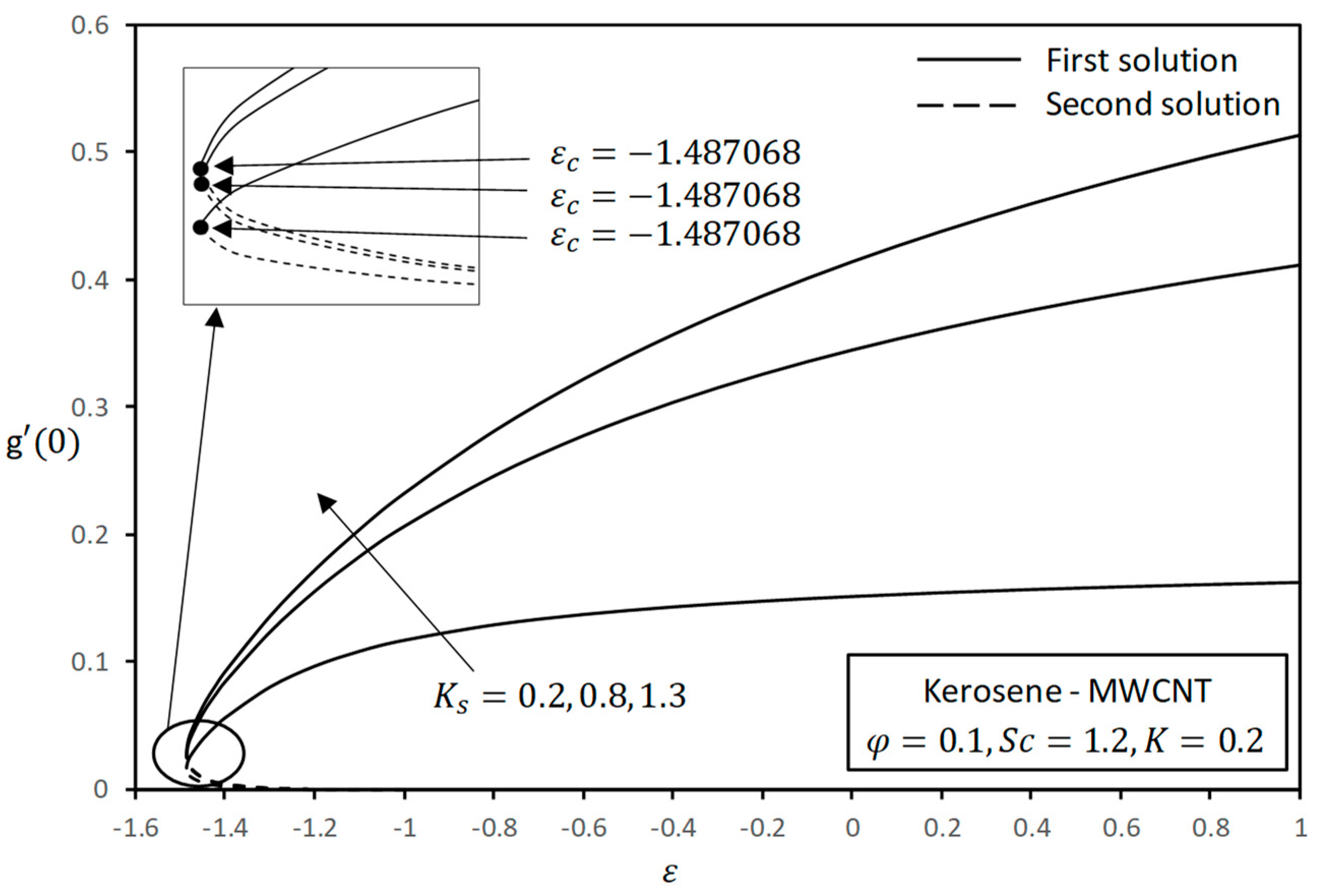
- Single-wall CNTs show greater impact on skin friction and heat transfer rate than multi-wall CNTs in both water and kerosene.
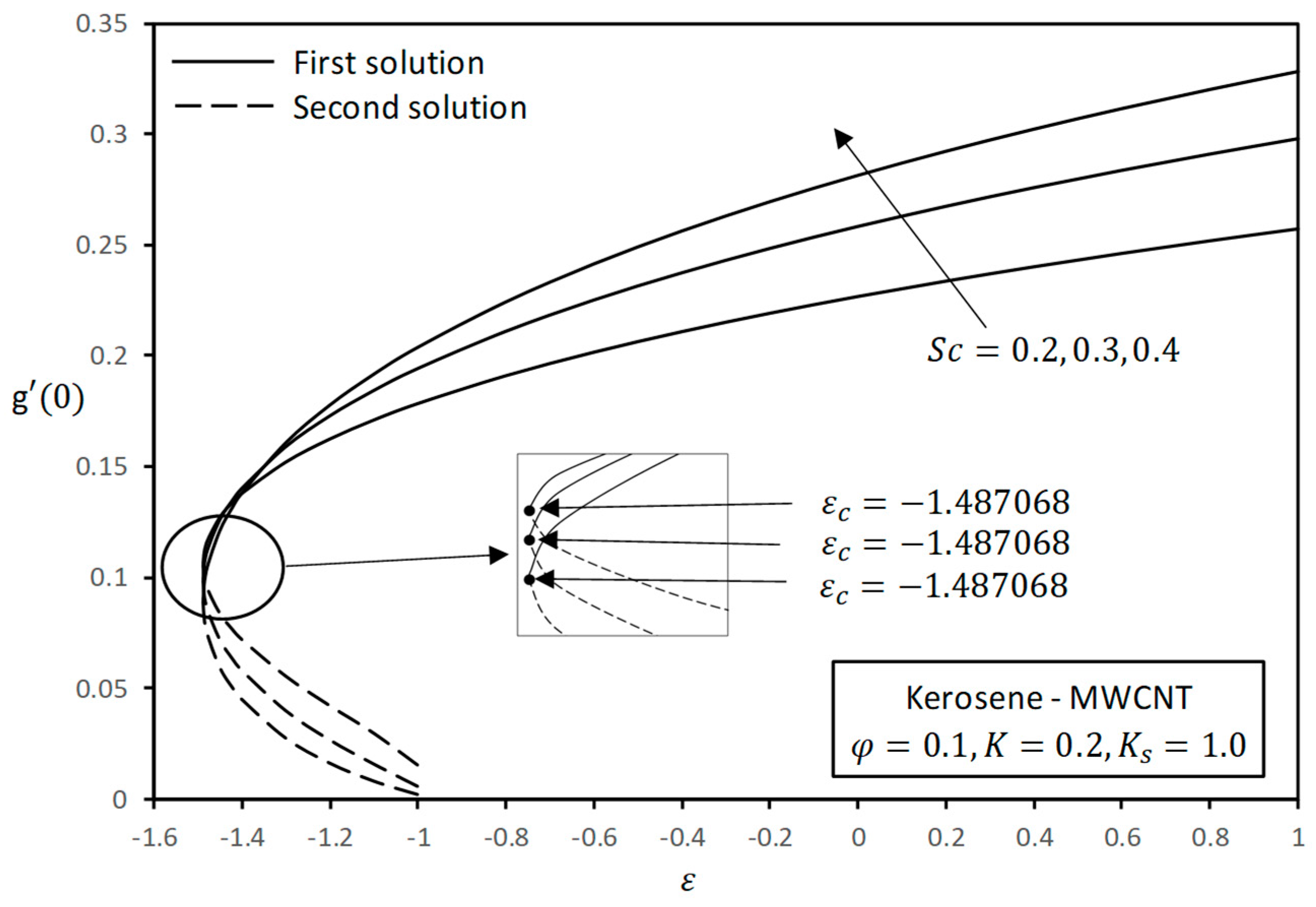
- The concentration of species A at the surface increases with an increase in heterogeneous reaction parameter and Schmidt number , while it decreases when homogeneous reaction parameter is increased.
- The range of solutions were widely expanded for exponentially shrinking cases compared with linear cases.
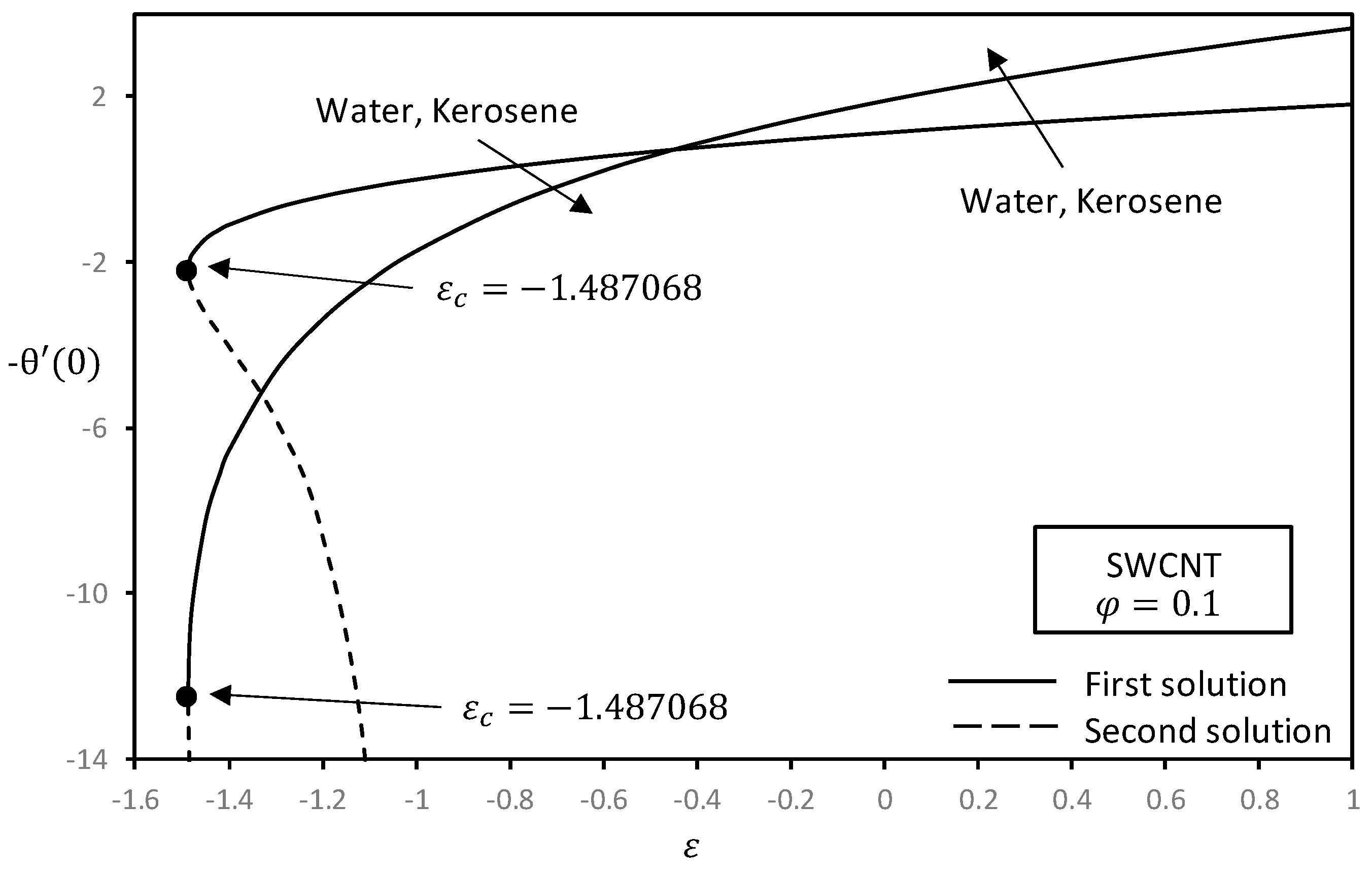
- The existence of unique solutions occurs for an exponentially stretching surface , whereas dual solutions occur for an exponentially shrinking surface .
- The first solution is stable and physically realizable, while the second solution is unstable.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| concentrations of the chemical species | |
| chemical species | |
| constant concentration | |
| constants | |
| skin friction coefficient | |
| specific heat at constant pressure | |
| diffusion coefficients | |
| dimensionless stream function | |
| concentration of species A | |
| concentration of species B | |
| thermal conductivity | |
| strength of the homogeneous reaction | |
| strength of the heterogeneous reaction | |
| constants | |
| constants | |
| characteristic length of a sheet | |
| local Nusselt number | |
| Prandtl number | |
| surface heat flux | |
| local Reynolds numbers | |
| Schmidt number | |
| time | |
| temperature | |
| temperature constant | |
| velocity components along the x- and y- directions, respectively | |
| stretching/shrinking velocity | |
| velocity of inviscid flow | |
| cartesian coordinates along the surface and normal to it, respectively | |
| Greek symbols | |
| thermal diffusivity | |
| ratio of the diffusion coefficient | |
| nanoparticle volume fraction | |
| dimensionless temperature | |
| unknown eigenvalues | |
| stretching/shrinking parameter | |
| kinematic viscosity | |
| dynamic viscosity | |
| fluid density | |
| heat capacity of the fluid | |
| dimensionless time variable | |
| surface shear stress | |
| stream function | |
| similarity variable | |
| Subscripts | |
| condition at the surface of the plate | |
| ambient condition | |
| carbon nanotubes | |
| fluid | |
| nanofluid | |
| Superscript | |
| differentiation with respect to | |
References
- Halelfadl, S.; Maré, T.; Estellé, P. Efficiency of carbon nanotubes water based nanofluids as coolants. Exp. Therm. Fluid Sci. 2014, 53, 104–110. [Google Scholar] [CrossRef]
- Javey, A.; Kong, J. (Eds.) Carbon Nanotube Electronics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Liu, M.S.; Lin, M.C.C.; Huang, I.T.; Wang, C.C. Enhancement of thermal conductivity with carbon nanotube for nanofluids. Int. Commun. Heat Mass Transf. 2005, 32, 1202–1210. [Google Scholar] [CrossRef]
- Biercuk, M.J.; Llaguno, M.C.; Radosavljevic, M.; Hyun, J.K.; Johnson, A.T.; Fischer, J.E. Carbon nanotube composites for thermal management. Appl. Phys. Lett. 2002, 80, 2767–2769. [Google Scholar] [CrossRef]
- Pour, M.S.; Nassab, S.A.G. Numerical investigation of forced laminar convection flow of nanofuids over a backward facing step under bleeding condition. J. Mech. 2012, 28, N7–N12. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Pop, I. Unsteady boundary-layer flow and heat transfer of a nanofluid over a permeable stretching/shrinking sheet. Int. J. Heat Mass Transf. 2012, 55, 2102–2109. [Google Scholar] [CrossRef]
- Batool, K.; Ashraf, M. Stagnation point flow and heat transfer of a magneto-micropolar fluid towards a shrinking sheet with mass transfer and chemical reaction. J. Mech. 2013, 29, 411–422. [Google Scholar] [CrossRef]
- Magyari, E.; Keller, B. Heat and mass transfer in the boundary layers on an exponentially stretching continuous surface. J. Phys. D Appl. Phys. 1999, 32, 577. [Google Scholar] [CrossRef]
- Sanjayanand, E.; Khan, S.K. On heat and mass transfer in a viscoelastic boundary layer flow over an exponentially stretching sheet. Int. J. Therm. Sci. 2006, 45, 819–828. [Google Scholar] [CrossRef]
- Bidin, B.; Nazar, R. Numerical solution of the boundary layer flow over an exponentially stretching sheet with thermal radiation. Eur. J. Sci. Res 2009, 33, 710–717. [Google Scholar]
- Nadeema, S.; Hayat, T.; Malika, M.Y.; Rajputa, S.A. Thermal radiation effects on the flow by an exponentially stretching surface: A series solution. Z. Für Nat. A 2010, 65, 495–503. [Google Scholar] [CrossRef]
- Ishak, A. MHD boundary layer flow due to an exponentially stretching sheet with radiation effect. Sains Malaysiana 2011, 40, 391–395. [Google Scholar]
- Nadeem, S.; Zaheer, S.; Fang, T. Effects of thermal radiation on the boundary layer flow of a Jeffrey fluid over an exponentially stretching surface. Numer. Algorithms 2011, 57, 187–205. [Google Scholar] [CrossRef]
- Nadeem, S.; Lee, C. Boundary layer flow of nanofluid over an exponentially stretching surface. Nanoscale Res. Lett. 2012, 7, 94. [Google Scholar] [CrossRef]
- Zaib, A.; Bhattacharyya, K.; Shafie, S. Unsteady boundary layer flow and heat transfer over an exponentially shrinking sheet with suction in a copper-water nanofluid. J. Cent. South Univ. 2015, 22, 4856–4863. [Google Scholar] [CrossRef]
- Baag, S.; Mishra, S.R.; Hoque, M.M.; Anika, N.N. Magnetohydrodynamic Boundary Layer Flow Over an Exponentially Stretching Sheet Past a Porous Medium with Uniform Heat Source. J. Nanofluids 2018, 7, 570–576. [Google Scholar] [CrossRef]
- Hiemenz, K. Die Grenzschicht an einem in den gleichformigen Flussigkeitsstrom eingetauchten geraden Kreiszylinder. Dinglers Polytech. J. 1911, 326, 321–324. [Google Scholar]
- Bhattacharyya, K.; Vajravelu, K. Stagnation-point flow and heat transfer over an exponentially shrinking sheet. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 2728–2734. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Pop, I. Boundary layer stagnation-point flow and heat transfer over an exponentially stretching/shrinking sheet in a nanofluid. Int. J. Heat Mass Transf. 2012, 55, 8122–8128. [Google Scholar] [CrossRef]
- Merkin, J.H. A model for isothermal homogeneous-heterogeneous reactions in boundary-layer flow. Math. Comput. Model. 1996, 24, 125–136. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Pop, I. On the stagnation-point flow towards a stretching sheet with homogeneous–heterogeneous reactions effects. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 4296–4302. [Google Scholar] [CrossRef]
- Hayat, T.; Farooq, M.; Alsaedi, A. Homogeneous-heterogeneous reactions in the stagnation point flow of carbon nanotubes with Newtonian heating. AIP Adv. 2015, 5, 027130. [Google Scholar] [CrossRef]
- Hayat, T.; Hussain, Z.; Alsaedi, A.; Ahmad, B. Heterogeneous-homogeneous reactions and melting heat transfer effects in flow with carbon nanotubes. J. Mol. Liq. 2016, 220, 200–207. [Google Scholar] [CrossRef]
- Khan, M.I.; Hayat, T.; Khan, M.I.; Alsaedi, A. A modified homogeneous-heterogeneous reactions for MHD stagnation flow with viscous dissipation and Joule heating. Int. J. Heat Mass Transf. 2017, 113, 310–317. [Google Scholar] [CrossRef]
- Hayat, T.; Ayub, T.; Muhammad, T.; Alsaedi, A. Three-dimensional flow with Cattaneo–Christov double diffusion and homogeneous-heterogeneous reactions. Results Phys. 2017, 7, 2812–2820. [Google Scholar] [CrossRef]
- Hayat, T.; Muhammad, K.; Muhammad, T.; Alsaedi, A. Melting Heat in Radiative Flow of Carbon Nanotubes with Homogeneous-Heterogeneous Reactions. Commun. Theor. Phys. 2018, 69, 441. [Google Scholar] [CrossRef]
- Javed, M.; Farooq, M.; Ahmad, S.; Anjum, A. Melting heat transfer with radiative effects and homogeneous- heterogeneous reaction in thermally stratified stagnation flow embedded in porous medium. J. Cent. South Univ. 2018, 25, 2701–2711. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occurring in mixed convection in a porous medium. J. Eng. Math. 1986, 20, 171–179. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Merrill, K.; Beauchesne, M.; Previte, J.; Paullet, J.; Weidman, P. Final steady flow near a stagnation point on a vertical surface in a porous medium. Int. J. Heat Mass Transf. 2006, 49, 4681–4686. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Ishak, A. Flow and heat transfer over a shrinking sheet: A stability analysis. Int. J. Mech. Aerosp. Ind. Mechatron. Eng. 2014, 8, 905–909. [Google Scholar]
- Nazar, R.; Noor, A.; Jafar, K.; Pop, I. Stability analysis of three-dimensional flow and heat transfer over a permeable shrinking surface in a Cu-water nanofluid. Int. J. Math. Comput. Phys. Electr. Comput. Eng. 2014, 8, 782–788. [Google Scholar]
- Awaludin, I.S.; Weidman, P.D.; Ishak, A. Stability analysis of stagnation-point flow over a stretching/shrinking sheet. AIP Adv. 2016, 6, 045308. [Google Scholar] [CrossRef]
- Najib, N.; Bachok, N.; Arifin, N.M.; Senu, N. Boundary layer flow and heat transfer of nanofluids over a moving plate with partial slip and thermal convective boundary condition: Stability analysis. Int. J. Mech. 2017, 11, 19–24. [Google Scholar]
- Najib, N.; Bachok, N.; Arifin, N.M. Stability of Dual Solutions in Boundary Layer Flow and Heat Transfer over an Exponentially Shrinking Cylinder. Indian J. Sci. Technol. 2017, 9. [Google Scholar] [CrossRef]
- Anuar, N.S.; Bachok, N.; Pop, I. A Stability Analysis of Solutions in Boundary Layer Flow and Heat Transfer of Carbon Nanotubes over a Moving Plate with Slip Effect. Energies 2018, 11, 3243. [Google Scholar] [CrossRef]
- Chaudhary, M.A.; Merkin, J.H. A simple isothermal model for homogeneous-heterogeneous reactions in boundary-layer flow. I Equal diffusivities. Fluid Dyn. Res. 1995, 16, 311. [Google Scholar] [CrossRef]
- Oztop, H.F.; Abu-Nada, E. Numerical study of natural convection in partially heated rectangular enclosures filled with nanofluids. Int. J. Heat Fluid Flow 2008, 29, 1326–1336. [Google Scholar] [CrossRef]
- Xue, Q.Z. Model for thermal conductivity of carbon nanotube-based composites. Phys. B Condens. Matter 2005, 368, 302–307. [Google Scholar] [CrossRef]
- Khan, W.A.; Khan, Z.H.; Rahi, M. Fluid flow and heat transfer of carbon nanotubes along a flat plate with Navier slip boundary. Appl. Nanosci. 2014, 4, 633–641. [Google Scholar] [CrossRef]
- Wang, C.Y. Stagnation flow towards a shrinking sheet. Int. J. Non-Linear Mech. 2008, 43, 377–382. [Google Scholar] [CrossRef]
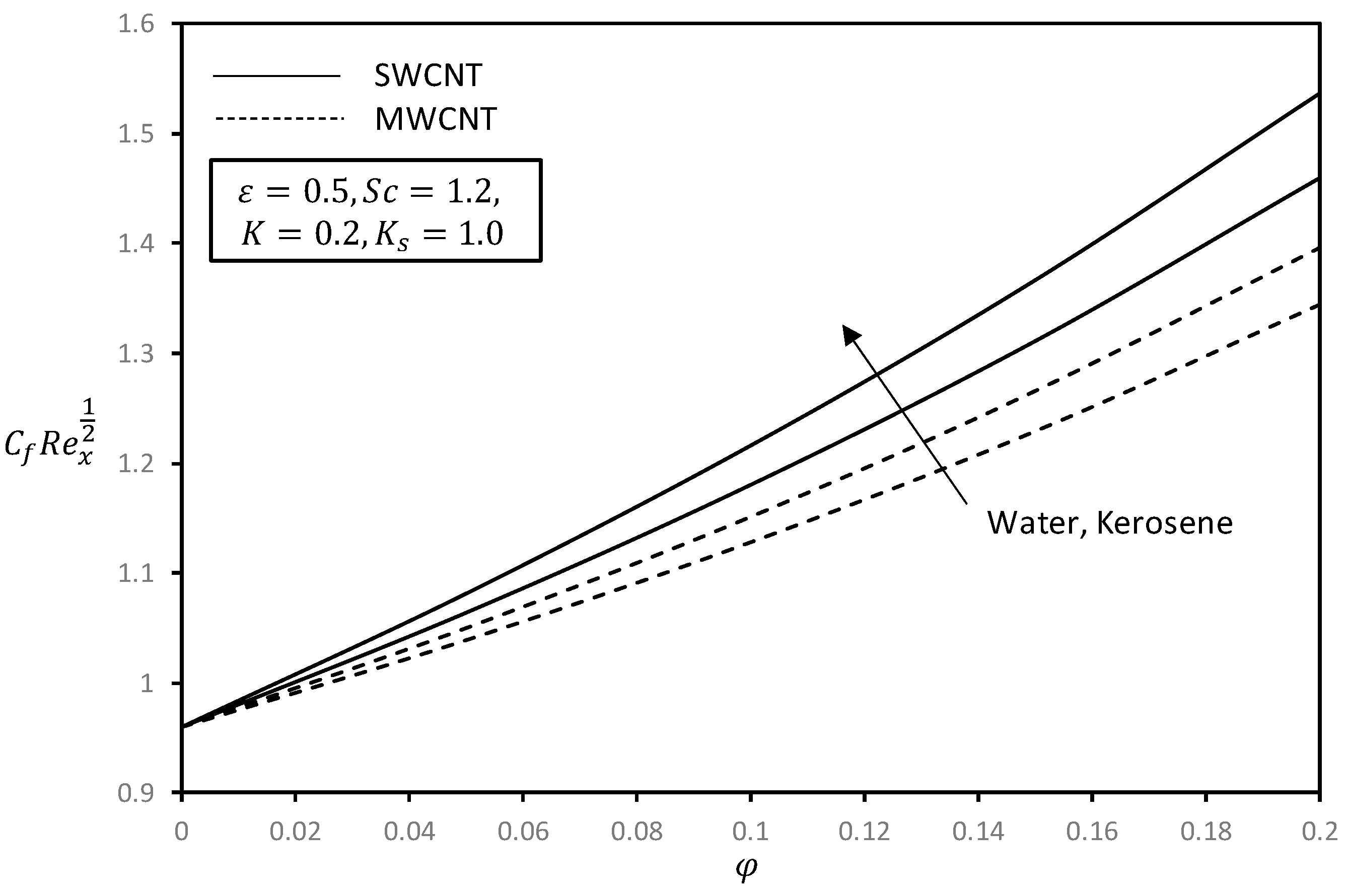

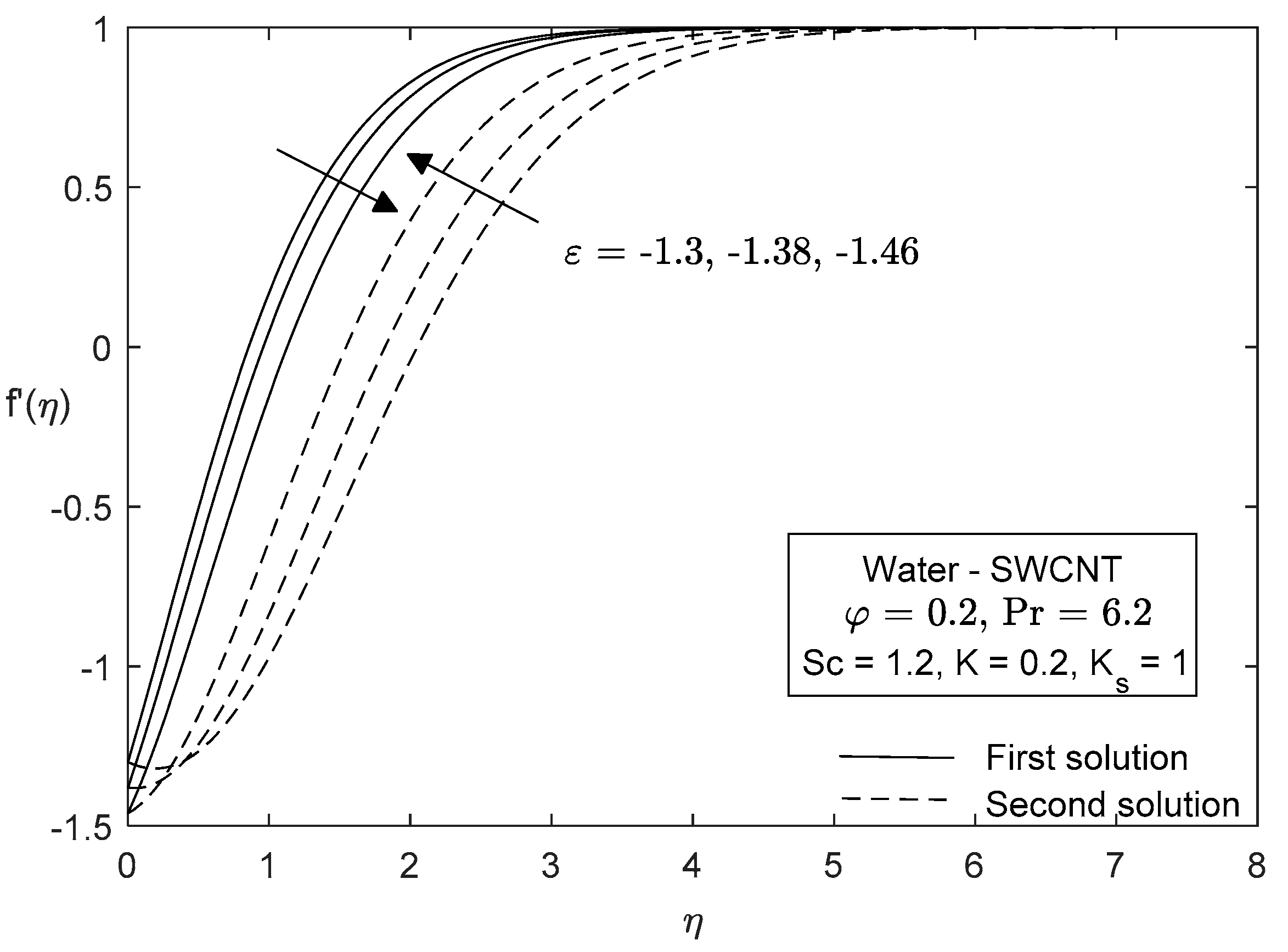
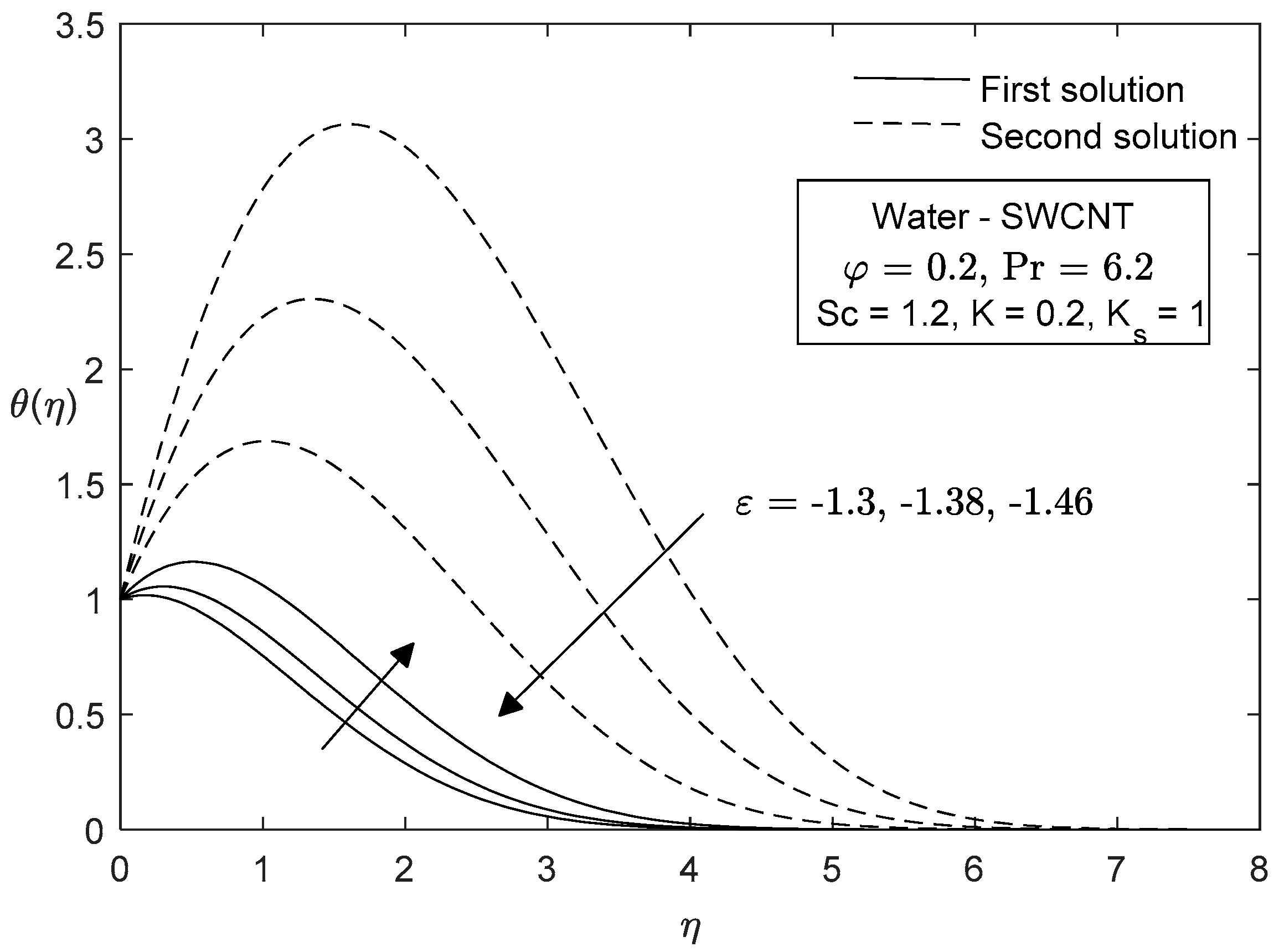

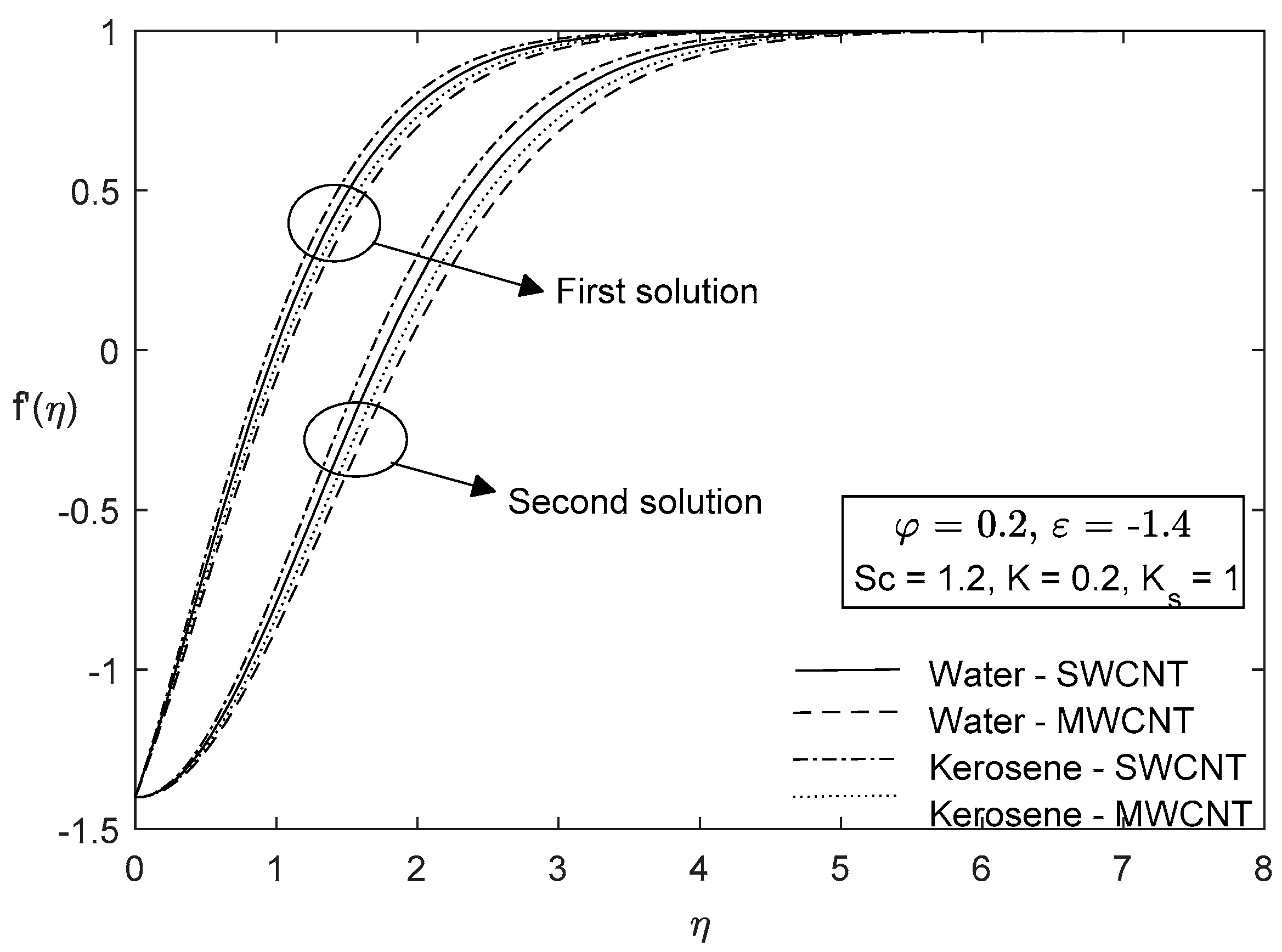
| Physical Properties | Base Fluids | Nanoparticle | ||
|---|---|---|---|---|
| Water (Pr = 6.2) | Kerosene (Pr = 21) | SWCNT | MWCNT | |
| 997 | 783 | 2600 | 1600 | |
| 4179 | 2090 | 425 | 796 | |
| 0.613 | 0.145 | 6600 | 3000 | |
| First Solution | Second Solution | |
|---|---|---|
| −1.48706 | 0.0147 | −0.0147 |
| −1.487 | 0.0413 | −0.0413 |
| −1.485 | 0.2271 | −0.2261 |
| −1.482 | 0.3557 | −0.3533 |
| −1.48 | 0.4202 | −0.4168 |
| −1.42 | 1.2926 | −1.2599 |
| −1.4 | 1.4705 | −1.4278 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anuar, N.S.; Bachok, N.; Arifin, N.M.; Rosali, H. Stagnation Point Flow and Heat Transfer over an Exponentially Stretching/Shrinking Sheet in CNT with Homogeneous–Heterogeneous Reaction: Stability Analysis. Symmetry 2019, 11, 522. https://doi.org/10.3390/sym11040522
Anuar NS, Bachok N, Arifin NM, Rosali H. Stagnation Point Flow and Heat Transfer over an Exponentially Stretching/Shrinking Sheet in CNT with Homogeneous–Heterogeneous Reaction: Stability Analysis. Symmetry. 2019; 11(4):522. https://doi.org/10.3390/sym11040522
Chicago/Turabian StyleAnuar, Nur Syazana, Norfifah Bachok, Norihan Md Arifin, and Haliza Rosali. 2019. "Stagnation Point Flow and Heat Transfer over an Exponentially Stretching/Shrinking Sheet in CNT with Homogeneous–Heterogeneous Reaction: Stability Analysis" Symmetry 11, no. 4: 522. https://doi.org/10.3390/sym11040522
APA StyleAnuar, N. S., Bachok, N., Arifin, N. M., & Rosali, H. (2019). Stagnation Point Flow and Heat Transfer over an Exponentially Stretching/Shrinking Sheet in CNT with Homogeneous–Heterogeneous Reaction: Stability Analysis. Symmetry, 11(4), 522. https://doi.org/10.3390/sym11040522





