Detection of Communities within the Multibody System Dynamics Network and Analysis of Their Relations
Abstract
1. Introduction
2. Methodology
2.1. Automatically Gathering Information
- (1)
- Get publication data. In the first phase ResNetBot obtains information from all publications (journal papers, conference proceedings and books) that contain the words ’multibody’ or ’multi-body’ in the title, abstract or keywords and are stored in the bot database.
- (2)
- Get author data. For each unique author identification number (Author ID) extracted in the previous phase, ResNetBot obtains and stores the information available in Scopus about the author: Affiliations, publications and dates, number of citations and h-index.
- (3)
- Collect collaboration networks. For each Author ID, the bot gets the Author IDs from the co-authors it has collaborated with, along with their affiliation, city and country.
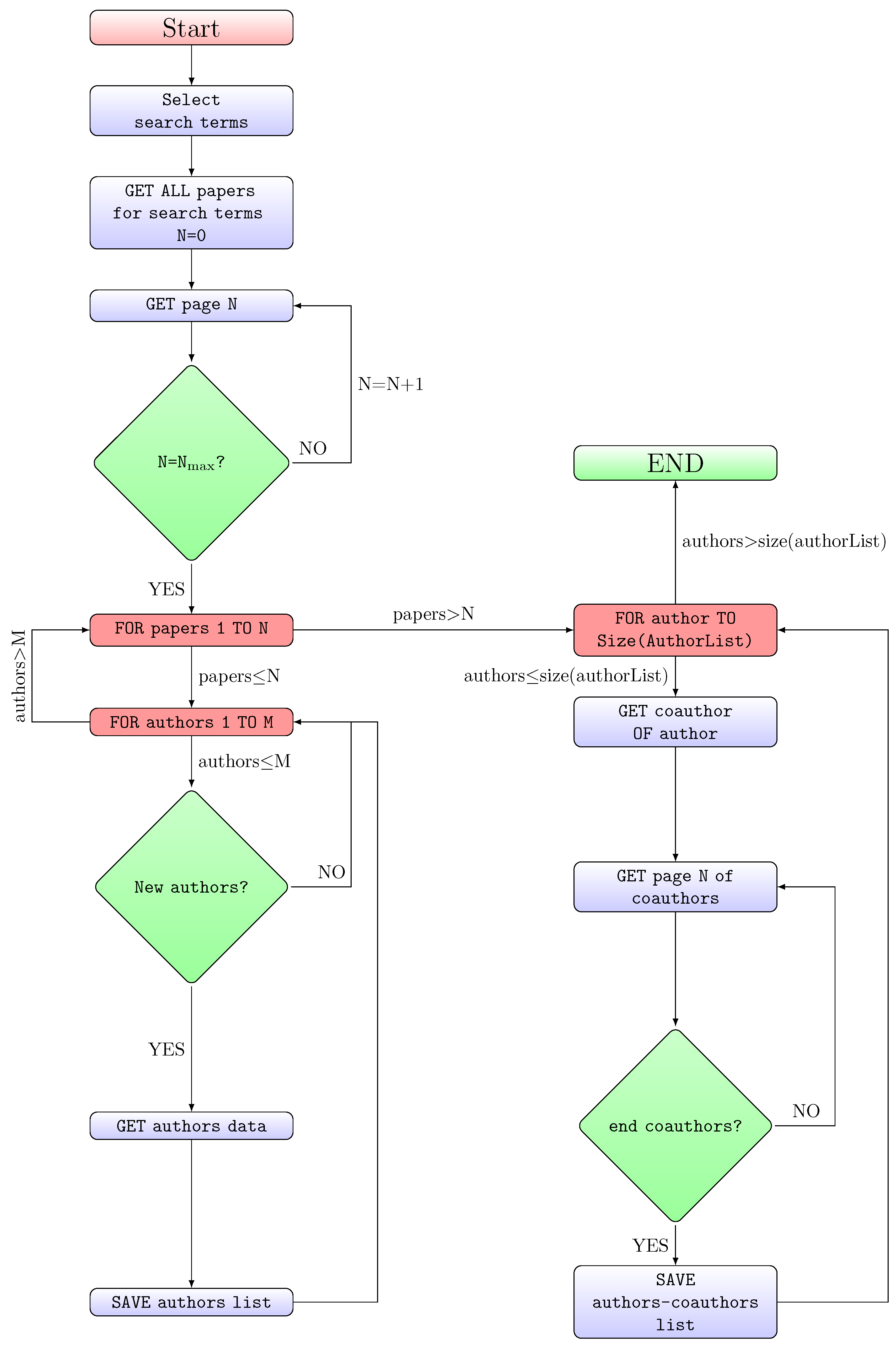
2.2. Graphical Detection of Communities
3. Analysis of Scientific Production and Community Detection
(AUTHKEY(multibod*) OR AUTHKEY(multi-bod*) OR ABS(multibod*) OR ABS(multi-bod*) OR TITLE(multibod*) OR TITLE(multi-bod*)).
3.1. Detection of a Main MBS Community
- The most used keyword is multibody dynamics.
- Multibody systems documents are mainly concerned with dynamics.
- Simulation is a key issue in the community.
- Flexibility appears as a top issue in the community.
- It is interesting that one of the well known approaches for flexible multibody systems, the absolute nodal coordinate formulation, has achieved a high position in the rank, showing the large development that this formulation achieved in the last years.
- ‘Vehicle dynamics’, ‘optimization’ and ‘friction’ are highly important issues according to their position in the rank of used keywords.
3.2. Detection of Thematic Communities
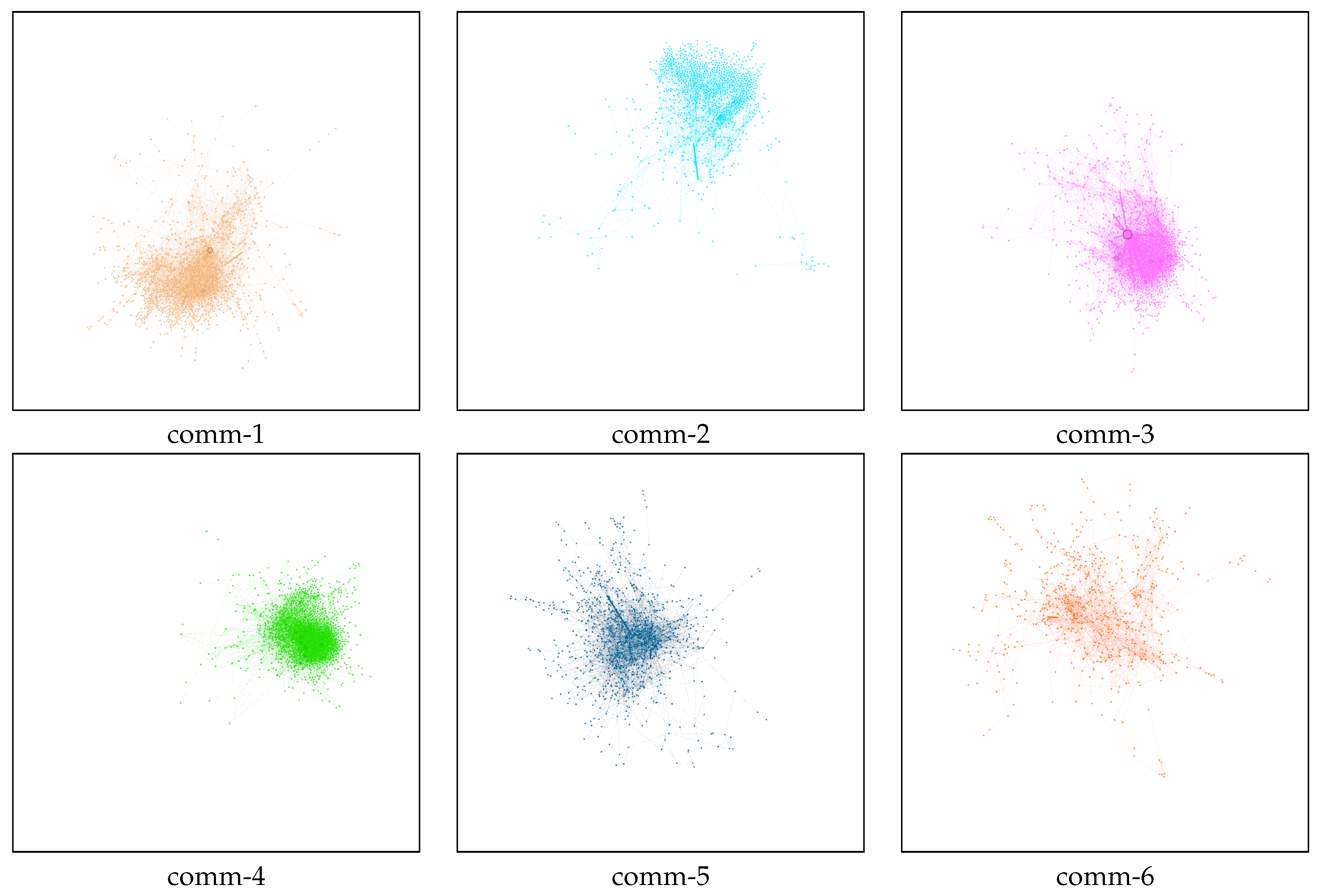
- comm-1
- Flexible multibody systems in general. This is the largest community in terms of published documents, with a total of 1103. The documents in it cover many aspects related with the flexibility in the presence of large rotations and deformations. Significant attention has been paid in this community to the pioneering work of Kane et al. [56] about centrifugal stiffening in rotating beams. A very complete review of the work in this community was carried out by Noor and Wasfy [5].
- comm-2
- Railway. This community, with 1027 documents, deals with the dynamics of railway systems in general. There are many documents that focus on the problem of wheel/rail contact. As a highly cited reference, the work of Shabana et al. [57] presents an elastic contact method which seems to be highly efficient for simulation purposes.
- comm-3
- Absolute nodal coordinate formulation. Interestingly, a well structured community devoted to the development and application of the absolute nodal coordinate formulation is found with 963 documents. According to the shape and location of this community, see Appendix A, the documents are clearly attracted by the documents of this community but also by some of the flexible multibody systems in general. A particular book by Shabana [17] is a highly cited document. There is an extensive review of the work done by this community by Gerstmyer et al. [39].
- comm-4
- Contact and non-ideal joints. A community dedicated to contact problems with 890 references is found. This community is easily identified by the modularity class tool and appears well concentrated, see green community in Appendix A. Many documents have paid attention to elastic or continuous contact models with the work of Lankarani and Nikravesh [58] and Machado et al. [59] as highly visible references. Clearance in joints is also a highly investigated topic within this community.
- comm-5
- Computational aspects of differential algebraic equations (DAE). The particular numerical problems presented in differential algebraic equations (DAE) have attracted many researchers over time. Problems such as the numerical fulfillment of constraint equations, the numerical stability of integrators, etc., are present in this community with 841 documents. The review work of Bauchau and Laulusa [60] and the one about projection methods by Bayo and Ledesma [61] are highly cited within this community.
- comm-6
- Optimization and sensitivity. Optimization and sensitivity analysis in multibody systems deserves the interest of many researchers because of their benefits for design. The work of D. Bestle [62] has been a pioneer in this community and counts 592 references in our analysis.
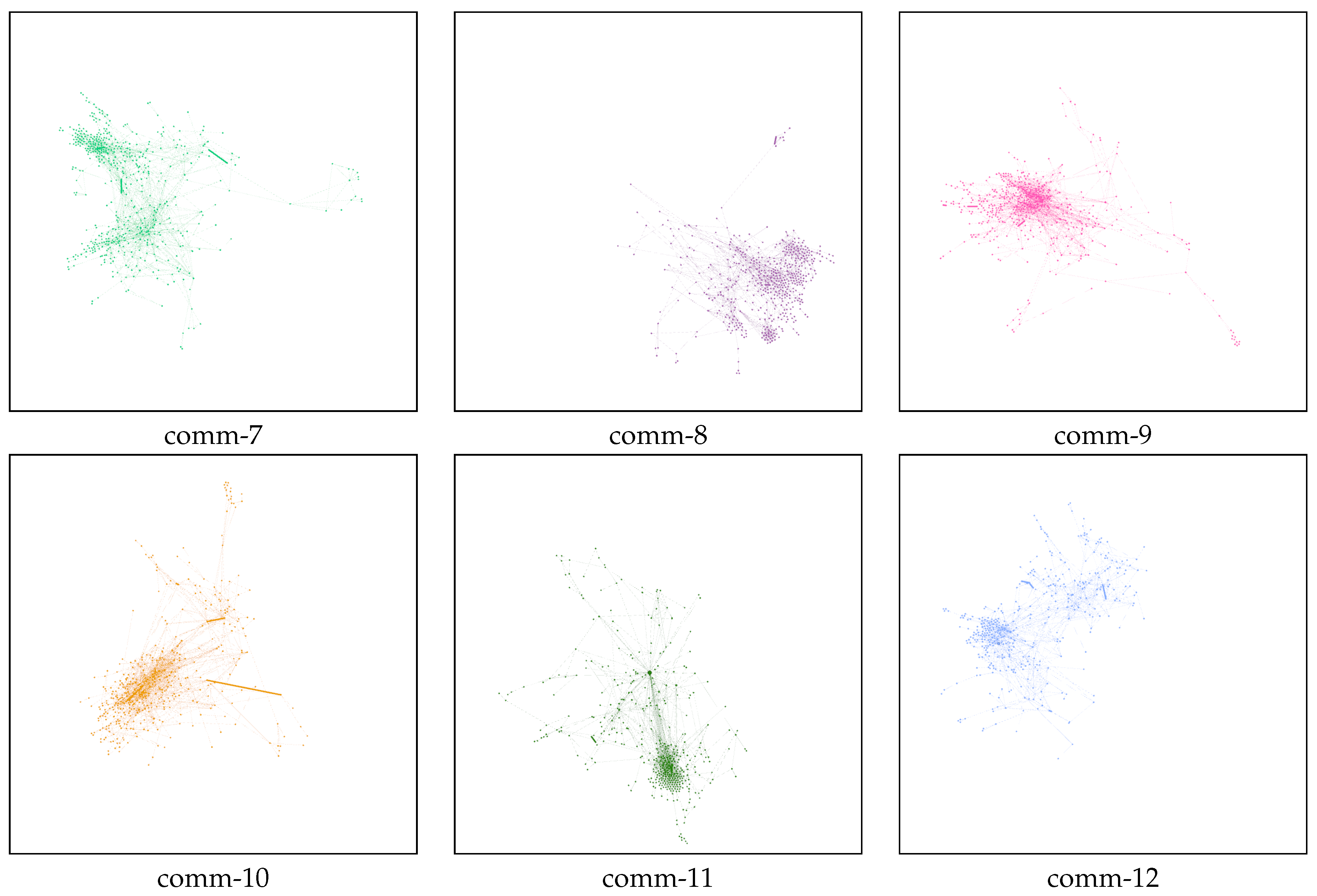
- comm-7
- Two-wheelers. The dynamics of motorcycles and bicycles have been a specific topic of research for many authors of the multibody system network. The work of Cossalter and Lot [63] about a motorcycle multibody model is a remarkable reference that identifies the goals of this community well. An already old review by W. Kortüm [64] has received many citations from documents from a community that includes 525 works.
- comm-8
- Biomechanics. The use of multibody dynamics techniques in biomechanics has experienced a huge development in recent years, with even the release of proprietary (AnyBody) as well as open software (OpenSim) highly extended in the community which, counts 517 documents. Multibody models for biomechanics usually include the dynamics of the biological actuators and are redundantly actuated, requiring the use of optimization methods. A highly cited work is the one by Damsgaard et al. [65] where the software AnyBody is presented to the community. Some of the results of the authors of this community have served as a basis for other applied and fundamental investigations, see, for example references [66,67].
- comm-9
- Accurate and efficient integrators. This community of 459 documents is mainly devoted to the development of efficient and accurate numerical integrators. The documents belonging to this community are well connected to those in the flexible multibody systems in general and the absolute nodal coordinate formulation communities. The topic of energy and momentum conserving numerical methods is one that is more present among the documents of this community. The work of Bauchau [68] and Betsch [69] have received many citations from the community.
- comm-10
- Robots as multibody systems. This community with 429 documents is concerned mainly with the applications of multibody systems techniques to robotics, including robots with flexible linkages, trajectory planning through optimization or the use of recursive formulations. There appears a highly cited book by A. Jain [70] that exemplifies the goals of this community.
- comm-11
- The vibration and transfer matrix method. Multibody systems containing elastic or restoring forces are susceptible of vibration. Linear and non-linear equations of motion are many times obtained with the methods of multibody systems. The transfer matrix method, which is connected to vibrating systems and extended by Rui et al. [71] to general multibody systems has a high impact in the works belonging to this community, which counts 421 documents.
- comm-12
- Aeronautic applications. The methods of multibody systems have encountered a large development and application in aeronautics as reflects this community with 406 documents. Problems of aeroelasticity, modeling of specific parts as rotors, landing gears, etc., or the stability of flight are presented in the documents of this community. The work of Masarati et al. [72] has been highly cited within the community.
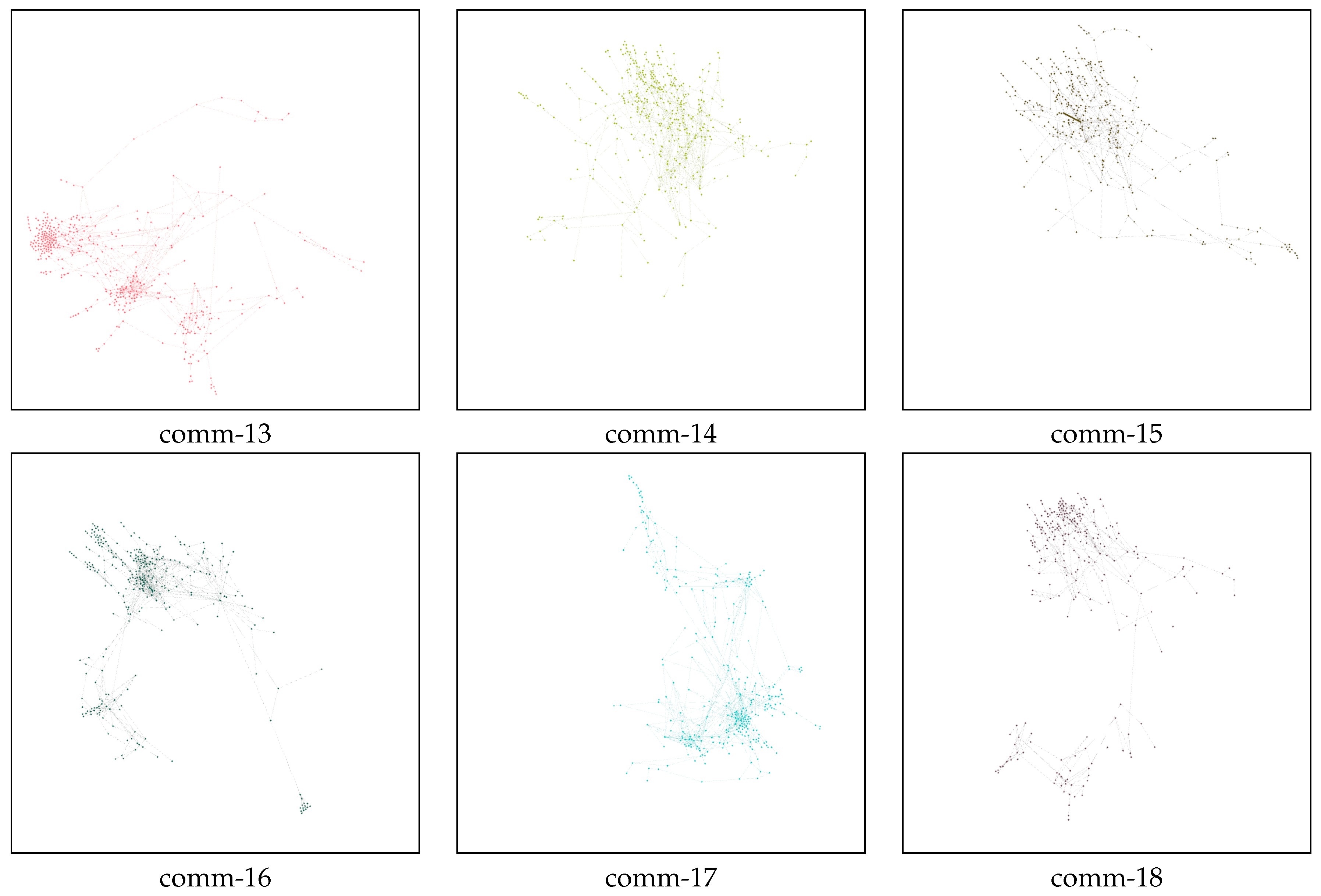
- comm-13
- Marine applications. A total of 401 documents are encountered in this community, where hydrodynamic interaction, floatation and drift issues are of interest and are analyzed together with multibody models. There is a recent review of applications of MBS to ships and offshore structures by Lee and Roh [73].
- comm-14
- Powertrain elements. This community counts 399 documents that are mainly devoted to important aspects of powertrain dynamics as explained by Novotny and Pistek [74]. The high angular speeds in powertrain systems together with the flexibility of some components were the causes for the application of MBS techniques in this field, giving birth to a specific community. The dynamics of crankshafts is a topic of high interest in this community.
- comm-15
- Road vehicle dynamics. Multibody system dynamics has encountered a large application in vehicle system dynamics. The existence of this community with 321 documents probes this fact. The generation of a full vehicle multibody model with detailed suspension systems or the analysis of ride comfort or handling are hot topics in this community. The book by Blundell and Harty [75] is a highly cited reference in the community.
- comm-16
- Wind turbines. The analysis and simulation of wind turbines is the subject of many papers from this community of 308 documents. Within it, the modeling and simulation of gear box dynamics is a hot topic as reported by Helsen et al. [76]. In addition, modal analysis and aeroelasticity issues are also accounted for in many documents.
- comm-17
- Complex terrain and tracked vehicles. A community with 301 documents dedicated to the modeling and simulation of tracked vehicles has also been found. Among others, the work of Rubinstein and Hitron [77] has received many citations. Due to the special nature of this kind of vehicle, this community pays great attention to the modeling of complex terrains.
- comm-18
- Non-smooth contact. This community of 258 documents in our analysis focuses also on contact, but with a slightly different hint related to the discretization of the contact surfaces. Non matching discretizations are studied with the help of the concept of dual Lagrange multiplier space, see reference [78]. In general, the works found in this community are strongly related with the finite element method in multibody contact situations, see Laursen and Simo [79], with geometric problems arising from the different discretizations of the contact surfaces. Friction and frictionless situations are considered with a focus on the formulation of equations and constraints more than on the frictional phenomenon.
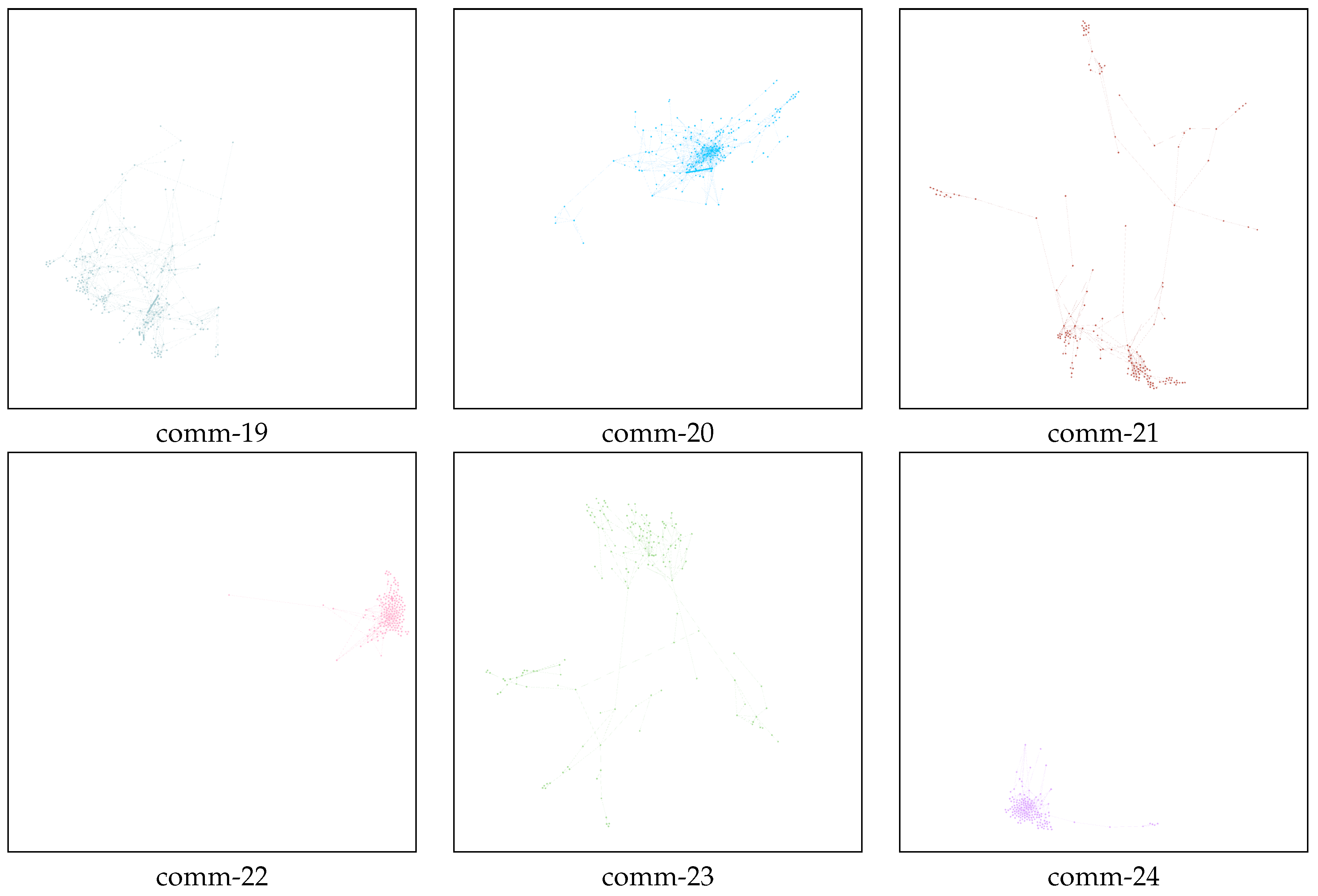
- comm-19
- Space applications. There appeared a community with 227 documents that applies the methods of multibody system dynamics to space problems as attitude control of satellites or assembly of space structures by robotic manipulators. Control issues both for linear and nonlinear multibody systems are important in this kind of application, and is most of the time realized by autonomous systems. As an example, the work of Umetani and Yoshida [80] has a significant impact in the community.
- comm-20
- Friction. A community with a size of 196 documents around friction is found. Since friction requires contact, this community is related with both the contact and non-ideal joints (comm-4) and the non-smooth contact (comm-18) communities. It might make sense to group together the three mentioned communities into a large one (1295 documents). However, the particular hint of this community seems to be the friction phenomenon itself and for this reason the entity of the community has been respected. Complementary problems have been useful for the modeling of friction forces as, for example, reported by Anitescu and Potra [81].
- comm-21
- Flapping wings. A community with a size of 182 documents mainly focused on the analysis and modeling of flapping wings has been detected. Within this community one may find documents dealing with insect flight, micro aerial vehicles or different kinds of ornithopters, see reference [82]. Flapping wings are modeled as flexible structures for which the methods of flexible multibody systems encountered a challenging application.
- comm-22
- Accident reconstruction. Multibody system dynamics has been successfully applied to the reconstruction of accidents. Among other studies the kind of accident due to impacts of vehicle/pedestrian or falls are the most common. The dynamic simulation has been used to evaluate potential injuries as well as safety conditions. For example, in the highly cited document by Crocetta et al. [83] the influence of the vehicle front-end design on pedestrian ground impact is investigated. This community counts 169 documents in this analysis.
- comm-23
- Fatigue and life assessment. A community of 162 documents with the fatigue of materials as a main issue has been detected. Flexible multibody dynamics methods have proven to be useful for the evaluation of alternating stresses that can be used together with fatigue failure criteria for life assessment of components. As an example, the work of Dietz et al. [84] in the context of railway dynamics has received many citations.
- comm-24
- Computer numerical control (CNC) machining. This is the smallest community analyzed in this paper, with a size of 152 documents. Machining by computer numerical control (CNC) units is also fertile soil for application of multibody system dynamics. In this community, the evaluation of geometrical errors in the trajectory of the tool and their compensation are of major importance. CNC machines have different designs, with many axes and large mobility, that are successfully modeled with multibody system dynamics techniques. As a highly representative reference, one may take the work of Zhu et al. [85].
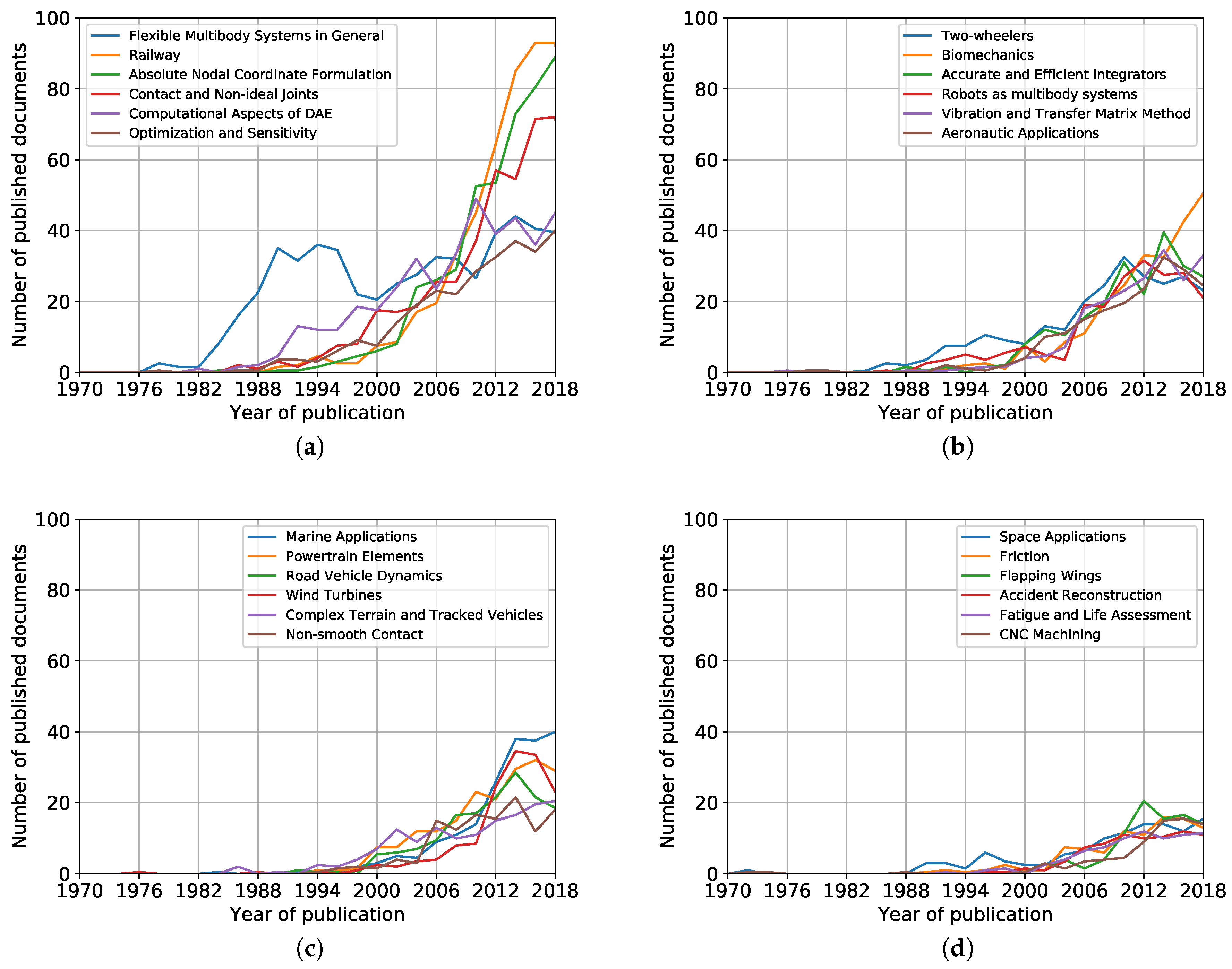
3.3. Activity of the Communities along the Time
3.4. Most Used Journals by MBS Community Members
3.5. Most Used Conference Proceedings by MBS Community Members
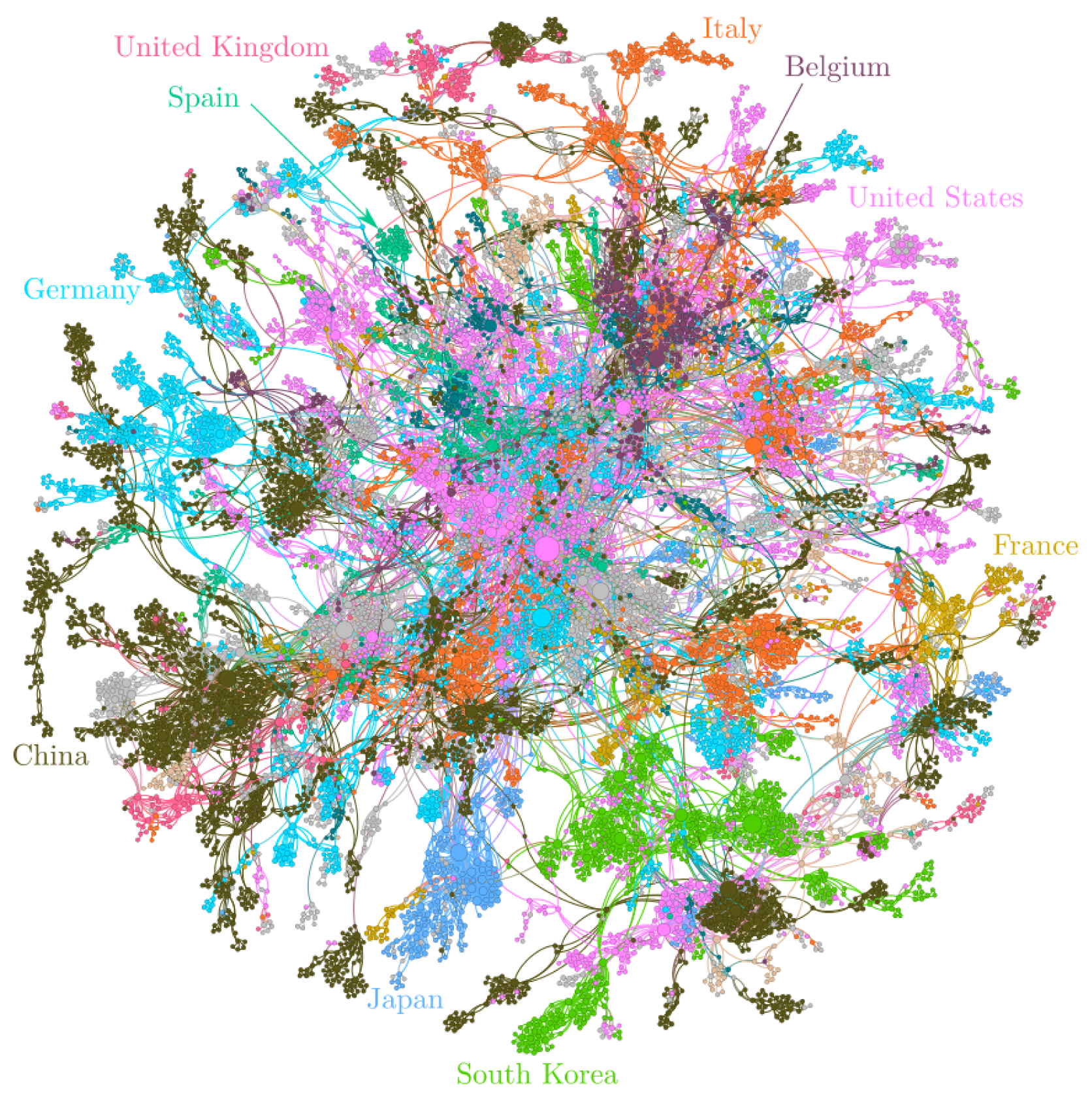
3.6. Analysis of Collaborations of Authors by Country
4. Summary and Conclusions
- ResNetBot was highly efficient at collecting a huge amount of data from the abstract and citation database, SCOPUS. Such an amount of data could be analyzed by using the software Gephi.
- There appear to be 32 communities from the analysis of the modularity. In this paper, only the 24 largest communities were studied. The community detection carried out showed a reasonable result since the documents conforming to a community have meaningful similarities and common objectives.
- The five largest communities are flexible multibody systems in general, railway, absolute nodal coordinate formulation, contact and non-ideal joints, computational aspects of DAE.
- The recent activity analyzed by communities predicts an increasing interest in railway, absolute nodal coordinate formulation, contact and non-ideal joints and biomechanics.
- The fifteen journals most used for publishing by researchers were obtained, with the journal Multibody System Dynamics being the one that has published the largest number of papers from the analyzed network.
- The fifteen conference proceedings most used for publishing by researchers were obtained. The list included conferences from fields like astronautical sciences or offshore engineering.
- Authors from many countries are contributing to the multibody system dynamics communities. The most active countries in the network have been found. Their cooperation was analyzed, obtaining interesting patterns of collaborations in the graph.
Author Contributions
Funding
Conflicts of Interest
Appendix A. Fingerprint Images of all Detected Communities
References
- Erdman, A.G.; Sandor, G.N. Diseño de Mecanismos, Análisis y Síntesis, tercera ed.; Prentice Hall: México city, Mexico, 1998. [Google Scholar]
- Wittenburg, J. Dynamics of Systems of Rigid Bodies; Teubner: Stuttgart, Germany, 1977. [Google Scholar]
- Schiehlen, W. Multibody system dynamics: Roots and perspectives. Multibody Syst. Dyn. 1997, 1, 149–188. [Google Scholar] [CrossRef]
- Valverde, J.; Escalona, J.; Mayo, J.; Domínguez, J. Dynamic analysis of a light structure in outer space: Short Electrodynamic Tether. Multibody Syst. Dyn. 2003, 10, 125–146. [Google Scholar] [CrossRef]
- Wasfy, T.M.; Noor, A.K. Computational strategies for flexible multibody systems. Appl. Mech. Rev. 2003, 56, 553–613. [Google Scholar] [CrossRef]
- Shabana, A.A. Flexible multibody dynamics: Review of past and recent developments. Multibody Syst. Dyn. 1997, 1, 189–222. [Google Scholar] [CrossRef]
- Schiehlen, W. Research trends in multibody system dynamics. Multibody Syst. Dyn. 2007, 18, 3–13. [Google Scholar] [CrossRef]
- Gantmájer, F. Mecánica Analítica; MIR: Moscú, Russia, 1996. [Google Scholar]
- Bremer, H. On the dynamics of elastic multibody systems. Appl. Mech. Rev. 1999, 52, 275–303. [Google Scholar] [CrossRef]
- Nikravesh, P.E. Computer-Aided Analysis of Mechanical Systems; Prentice-Hall International, Inc.: Upper Saddle River, NJ, USA, 1988. [Google Scholar]
- Paul, B.; Krajcinovic, K. Computer analysis of machines with planar motion: Part 1—Kinematics; Part 2—Dynamics. ASME J. Appl. Mech. 1970, 37, 697–712. [Google Scholar] [CrossRef]
- Haug, E. Elements and Methods of Computational Dynamics. In Computer Aided Analysis and Optimization of Mechanical System Dynamics; Haug, E., Ed.; Springer: Berlin/Heidelberg, Germany, 1984; pp. 3–38. [Google Scholar]
- García de Jalón, J.; Unda, J.; Avello, A.; Jiménez, J. Dynamic analysis of three-dimensional mechanisms in natural coordinates. ASME J. Mech. Transm. Autom. Des. 1987, 109, 460–465. [Google Scholar] [CrossRef]
- García de Jalón, J.; Bayo, E. Kinematic and Dynamic Simulation of Multibody Systems—The Real-Time Challenge; Springer: New York, NY, USA, 1993. [Google Scholar]
- Shabana, A. Computational Dynamics, 2nd ed.; Wiley-Interscience: New York, NY, USA, 2001. [Google Scholar]
- Unda, J.; de Jalón, J.G.; Losantos, F.; Enparantza, R. A comparative study on some different formulations of the dynamic equations of constrained mechanical systems. ASME J. Mech. Transm. Autom. Des. 1987, 109, 466–474. [Google Scholar] [CrossRef]
- Shabana, A. Dynamics of Multibody Systems; Cambriedge University Press: New York, NY, USA, 1998. [Google Scholar]
- Vukasovic, N.; Celigüeta, J.; de Jalón, J.G. Flexible multibody dynamics based on a fully cartesian system of support coordinates. ASME J. Mech. Des. 1993, 115, 294–299. [Google Scholar] [CrossRef]
- Mayo, J. Análisis Geométricamente No Lineal en Dinámica de Mecanismos Flexibles. Ph.D. Thesis, Escuela Técnica Superior de Ingenieros Industriales, Universidad de Sevilla, Sevilla, Spain, 1993. [Google Scholar]
- Belytschko, T.; Hsieh, B. Non-linear transient finite element analysis with convected co-ordinates. Int. J. Numer. Methods Eng. 1973, 7, 255–271. [Google Scholar] [CrossRef]
- Simo, J. A finite strain beam formulation. The three-dimensional dynamic problem. Part I. Comput. Methods Appl. Mech. Eng. 1985, 49, 55–70. [Google Scholar] [CrossRef]
- Simo, J.; Vu-Quoc, L. A three-dimensional finite-strain rod model. Part II: Computational aspects. Comput. Methods Appl. Mech. Eng. 1986, 58, 79–116. [Google Scholar] [CrossRef]
- Simo, J.; Vu-Quoc, L. On the dynamics of flexible beams under large overall motions-The plane case: Part I and Part II. ASME J. Appl. Mech. 1986, 53, 849–863. [Google Scholar] [CrossRef]
- Avello, A. Dinámica de Mecanismos Flexibles con Coordenadas Cartesianas y Teoría de Grandes Deformaciones. Ph.D. Thesis, Universidad de Navarra, San Sebastian, Spain, 1990. [Google Scholar]
- Shabana, A.A.; Hussien, H.; Escalona, J.L. Application of the absolute nodal coordinate formulation to large rotation and large deformation problems. ASME J. Mech. Des. 1998, 120, 188–195. [Google Scholar] [CrossRef]
- Shabana, A. Computer implementation of the absolute nodal coordinate formulation for flexible multibody dynamics. Nonlinear Dyn. 1998, 16, 293–306. [Google Scholar] [CrossRef]
- Berzeri, M.; Campanelli, M.; Shabana, A. Definition of the elastic forces in the finite-element absolute nodal coordinate formulation and the floating frame of reference formulation. Multibody Syst. Dyn. 2001, 5, 21–54. [Google Scholar] [CrossRef]
- Berzeri, M.; Shabana, A.A. Development of simple models for the elastic forces in the absolute nodal co-ordinate formulation. J. Sound Vib. 2000, 235, 539–565. [Google Scholar] [CrossRef]
- Omar, M.; Shabana, A. A two-dimensional shear deformable beam for large rotation and deformation problems. J. Sound Vib. 2001, 243, 565–573. [Google Scholar] [CrossRef]
- Yakoub, R.; Shabana, A. Three dimensional absolute nodal coordinate formulation for beam elements: Implementation and Applications. ASME J. Mech. Des. 2001, 123, 614–621. [Google Scholar] [CrossRef]
- Shabana, A.; Yakoub, R. Three-dimensional absolute nodal coordinate formulation for beam elements: Theory. ASME J. Mech. Des. 2001, 123, 606–613. [Google Scholar] [CrossRef]
- Shabana, A.A. Finite element incremental approach and exact rigid body inertia. ASME J. Mech. Des. 1996, 118, 171–178. [Google Scholar] [CrossRef]
- Mikkola, A.; Shabana, A. A non-incremental finite element procedure for the analysis of large deformation of plates and shells in mechanical system applications. Multibody Syst. Dyn. 2003, 9, 283–309. [Google Scholar] [CrossRef]
- Sopanen, J.; Mikkola, A. Description of elastic forces in absolute nodal coordinate formulation. Nonlinear Dyn. 2003, 34, 53–74. [Google Scholar] [CrossRef]
- Sopanen, J.; Mikkola, A. Studies on the Stiffness Properties of the Absolute Nodal Coordinate Formulation for Three-dimensional Beams. In Proceedings of the ASME DETC and CIE Conference; American Society of Mechanical Engineers Digital Collection: Little Falls, NJ, USA, 2003. [Google Scholar]
- Kerkkanen, K.S.; Sopanen, J.T.; Mikkola, A. A geometrically nonlinear beam element for multibody applications. J. Mech. Des. Trans. ASME 2005, 127, 621–630. [Google Scholar]
- Dufva, K.; Sopanen, J.; Mikkola, A. A two-dimensional shear deformable beam element based on the absolute nodal coordinate formulation. J. Sound Vib. 2005, 280, 719–738. [Google Scholar] [CrossRef]
- Dufva, K.; Sopanen, J.; Mikkola, A. Three-dimensional beam element based on a cross-sectional coordinate system approach. Nonlinear Dyn. 2005, in press. [Google Scholar] [CrossRef]
- Gerstmayr, J.; Sugiyama, H.; Mikkola, A. Review on the absolute nodal coordinate formulation for large deformation analysis of multibody systems. J. Comput. Nonlinear Dyn. 2013, 8. [Google Scholar] [CrossRef]
- Bastian, M.; Heymann, S.; Jacomy, M. Gephi: An Open Source Software for Exploring and Manipulating Networks. In Proceedings of the Third International AAAI Conference on Weblogs and Social Media, San Jose, CA, USA, 17–20 May 2009. [Google Scholar]
- De la Cruz-Lovera, C.; Perea-Moreno, A.J.; de la Cruz-Fernández, J.L.; Alvarez-Bermejo, J.; Manzano-Agugliaro, F. Worldwide research on energy efficiency and sustainability in public buildings. Sustainability 2017, 9, 1294. [Google Scholar] [CrossRef]
- Montoya, F.G.; Alcayde, A.; Baños, R.; Manzano-Agugliaro, F. A fast method for identifying worldwide scientific collaborations using the Scopus database. Telemat. Inform. 2018, 35, 168–185. [Google Scholar] [CrossRef]
- Montoya, F.; Baños, R.; Alcayde, A.; Montoya, M.; Manzano-Agugliaro, F. Power quality: Scientific collaboration networks and research trends. Energies 2018, 11, 2067. [Google Scholar] [CrossRef]
- Alcayde, A.; G Montoya, F.; Baños, R.; Perea-Moreno, A.J.; Manzano-Agugliaro, F. Analysis of Research Topics and Scientific Collaborations in Renewable Energy Using Community Detection. Sustainability 2018, 10, 4510. [Google Scholar] [CrossRef]
- Oracle. Json. Available online: http://www.json.org (accessed on 24 July 2018).
- Valderrama-Zurián, J.C.; Aguilar-Moya, R.; Melero-Fuentes, D.; Aleixandre-Benavent, R. A systematic analysis of duplicate records in Scopus. J. Informetr. 2015, 9, 570–576. [Google Scholar] [CrossRef]
- Franceschini, F.; Maisano, D.; Mastrogiacomo, L. Empirical analysis and classification of database errors in Scopus and Web of Science. J. Informetr. 2016, 10, 933–953. [Google Scholar] [CrossRef]
- Google. OpenRefine. Available online: http://openrefine.org (accessed on 24 July 2018).
- Baxter, R.; Christen, P.; Churches, T. A comparison of fast blocking methods for record linkage. In Proceedings of the ACM SIGKDD’03 Workshop on Data Cleaning, Record Linkage, and Object Consolidation, Washington, DC, USA, 27 August 2003; pp. 25–27. [Google Scholar]
- Jin, L.; Li, C.; Mehrotra, S. Efficient record linkage in large data sets. In Proceedings of the Eighth International Conference on Database Systems for Advanced Applications (DASFAA 2003), Kyoto, Japan, 26–28 March 2003; pp. 137–146. [Google Scholar]
- Cavnar, W.; Trenkle, J. N-gram-based text categorization. In Proceedings of the SDAIR-94, 3rd Annual Symposium on Document Analysis and Information Retrieval, Las Vegas, NV, USA, 11–13 April 1994; pp. 161–175. [Google Scholar]
- Abidin, A.F.; Mohamed, A.; Shareef, H. Intelligent detection of unstable power swing for correct distance relay operation using S-transform and neural networks. Expert Syst. Appl. 2011, 38, 14969–14975. [Google Scholar] [CrossRef]
- Montoya, F.G.; Alcayde, A.; Sánchez, P.; Gil, C.; Montoya, M.; Gómez, J. Comparative analysis of power variables in high performance embedded and x86 architectures using GNU/Linux. Comput. Electr. Eng. 2011, 37, 541–549. [Google Scholar] [CrossRef]
- Newman, M.E.; Girvan, M. Finding and evaluating community structure in networks. Phys. Rev. E 2004, 69, 026113. [Google Scholar] [CrossRef]
- Blondel, V.; Guillaume, J.L.; Lambiotte, R.; Lefebvre, E. Fast Unfolding of Communities in Large Networks. J. Stat. Mech. Theory Exp. 2008, 2008. [Google Scholar] [CrossRef]
- Kane, T.; Ryan, R.; Banerjee, A. Dynamics of a cantilever beam attached to a moving base. J. Guid. Control Dyn. 1987, 10, 139–151. [Google Scholar] [CrossRef]
- Shabana, A.; Zaazaa, K.; Escalona, J.; Sany, J. Development of elastic force model for wheel/rail contact problems. J. Sound Vib. 2004, 269, 295–325. [Google Scholar] [CrossRef]
- Lankarani, H.; Nikravesh, P. Contact force model with hysteresis damping for impact analysis of multibody systems. J. Mech. Transm. Autom. Des. 1990, 112, 369–376. [Google Scholar] [CrossRef]
- Machado, M.; Moreira, P.; Flores, P.; Lankarani, H. Compliant contact force models in multibody dynamics: Evolution of the Hertz contact theory. Mech. Mach. Theory 2012, 53, 99–121. [Google Scholar] [CrossRef]
- Bauchau, O.; Laulusa, A. Review of contemporary approaches for constraint enforcement in multibody systems. J. Comput. Nonlinear Dyn. 2008, 3, 1–8. [Google Scholar] [CrossRef]
- Bayo, E.; Ledesma, R. Augmented Lagrangian and mass-orthogonal projection methods for constrained multibody dynamics. Nonlinear Dyn. 1996, 9, 113–130. [Google Scholar] [CrossRef]
- Bestle, D. Analyzing and optimizing multibody systems. Mech. Struct. Mach. 1992, 20, 67–92. [Google Scholar] [CrossRef]
- Cossalter, V.; Lot, R. A motorcycle multi-body model for real time simulations based on the natural coordinates approach. Veh. Syst. Dyn. 2002, 37, 423–447. [Google Scholar] [CrossRef]
- Kortüm, W. Review of Multibody Computer, Codes for Vehicle system Dynamics. Veh. Syst. Dyn. 1993, 22, 3–31. [Google Scholar] [CrossRef]
- Damsgaard, M.; Rasmussen, J.; Christensen, S.; Surma, E.; de Zee, M. Analysis of musculoskeletal systems in the AnyBody Modeling System. Simul. Model. Pract. Theory 2006, 14, 1100–1111. [Google Scholar] [CrossRef]
- Zagrodny, B.; Ludwicki, M.; Wojnicz, W.; Mrozowski, J.; Awrejcewicz, J. Cooperation of mono-and bi-articular muscles: Human lower limb. J. Musculoskelet. Neuronal Interact. 2018, 18, 176–182. [Google Scholar]
- Wojnicz, W.; Zagrodny, B.; Ludwicki, M.; Awrejcewicz, J.; Wittbrodt, E. A two dimensional approach for modelling of pennate muscle behaviour. Biocybern. Biomed. Eng. 2017, 37, 302–315. [Google Scholar] [CrossRef]
- Bauchau, O. Computational Schemes for Flexible, Nonlinear Multi-Body Systems. Multibody Syst. Dyn. 1998, 2, 169–225. [Google Scholar] [CrossRef]
- Betsch, P. The discrete null space method for the energy consistent integration of constrained mechanical systems. Part I: Holonomic constraints. Comput. Methods Appl. Mech. Eng. 2005, 194, 5159–5190. [Google Scholar] [CrossRef]
- Jain, A. Robot and Multibody Dynamics: Analysis and Algorithms; Springer: Berlin, Germany, 2011; pp. 1–510. [Google Scholar] [CrossRef]
- Rui, X.; Wang, G.; Zhang, J. Transfer Matrix Method for Multibody Systems: Theory and Applications; Wiley: Hoboken, NJ, USA, 2017; pp. 1–732. [Google Scholar] [CrossRef]
- Masarati, P.; Morandini, M.; Mantegazza, P. An efficient formulation for general-purpose multibody/multiphysics analysis. J. Comput. Nonlinear Dyn. 2014, 9. [Google Scholar] [CrossRef]
- Lee, H.W.; Roh, M.I. Review of the multibody dynamics in the applications of ships and offshore structures. Ocean Eng. 2018, 167, 65–76. [Google Scholar] [CrossRef]
- Novotny, P.; Pistek, V. New efficient methods for powertrain vibration analysis. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2010, 224, 611–629. [Google Scholar] [CrossRef]
- Blundell, M.; Harty, D. The Multibody Systems Approach to Vehicle Dynamics; Elsevier: Amsterdam, The Netherlands, 2014; pp. 1–741. [Google Scholar] [CrossRef]
- Helsen, J.; Vanhollebeke, F.; Marrant, B.; Vandepitte, D.; Desmet, W. Multibody modelling of varying complexity for modal behaviour analysis of wind turbine gearboxes. Renew. Energy 2011, 36, 3098–3113. [Google Scholar] [CrossRef]
- Rubinstein, D.; Hitron, R. A detailed multi-body model for dynamic simulation of off-road tracked vehicles. J. Terramech. 2004, 41, 163–173. [Google Scholar] [CrossRef]
- Wohlmuth, B.; Krause, R. Monotone multigrid methods on nonmatching grids for nonlinear multibody contact problems. SIAM J. Sci. Comput. 2003, 25, 324–347. [Google Scholar] [CrossRef]
- Laursen, T.; Simo, J. A continuum-based finite element formulation for the implicit solution of multibody, large deformation-frictional contact problems. Int. J. Numer. Methods Eng. 1993, 36, 3451–3485. [Google Scholar] [CrossRef]
- Umetani, Y.; Yoshida, K. Resolved Motion Rate Control of Space Manipulators with Generalized Jacobian Matrix. IEEE Trans. Robot. Autom. 1989, 5, 303–314. [Google Scholar] [CrossRef]
- Anitescu, M.; Potra, F. Formulating Dynamic Multi-Rigid-Body Contact Problems with Friction as Solvable Linear Complementarity Problems. Nonlinear Dyn. 1997, 14, 231–247. [Google Scholar] [CrossRef]
- Pfeiffer, A.; Lee, J.S.; Han, J.H.; Baier, H. Ornithopter Flight Simulation Based on Flexible Multi-Body Dynamics. J. Bionic Eng. 2010, 7, 102–111. [Google Scholar] [CrossRef]
- Crocetta, G.; Piantini, S.; Pierini, M.; Simms, C. The influence of vehicle front-end design on pedestrian ground impact. Accid. Anal. Prev. 2015, 79, 56–69. [Google Scholar] [CrossRef] [PubMed]
- Dietz, S.; Netter, H.; Sachau, D. Fatigue life prediction of a railway bogie under dynamic loads through simulation. Veh. Syst. Dyn. 1998, 29, 385–402. [Google Scholar] [CrossRef]
- Zhu, S.; Ding, G.; Qin, S.; Lei, J.; Zhuang, L.; Yan, K. Integrated geometric error modeling, identification and compensation of CNC machine tools. Int. J. Mach. Tools Manuf. 2012, 52, 24–29. [Google Scholar] [CrossRef]


| Keyword | # of Appearances | % of Appearance |
|---|---|---|
| multibody dynamics | 1254 | 3.41 |
| multibody system | 751 | 2.04 |
| simulation | 302 | 0.82 |
| dynamics | 275 | 0.75 |
| multibody | 252 | 0.69 |
| flexible multibody dynamics | 226 | 0.61 |
| multibody system dynamics | 199 | 0.54 |
| flexible multibody system | 194 | 0.53 |
| multibody simulation | 166 | 0.45 |
| finite element method | 162 | 0.44 |
| absolute nodal coordinate formulation | 142 | 0.39 |
| vehicle dynamics | 140 | 0.38 |
| optimization | 125 | 0.34 |
| friction | 120 | 0.33 |
| multibody model | 114 | 0.31 |
| Most Commonly Used Keywords in the Following Communities: Flexible Multibody Systems in General (comm-1), Railway (comm-2), Absolute Nodal Coordinate Formulation (comm-3), Contact and Non-ideal Joints (comm-4), Computational Aspects of DAE (comm-5) and Optimization and Sensitivity (comm-6). | |||||
|---|---|---|---|---|---|
| comm-1 | comm-2 | comm-3 | comm-4 | comm-5 | comm-6 |
| flexible multibody system (90) | wheel-rail contact (77) | absolute nodal coordinate formulation (178) | clearance joint (52) | differential-algebraic Equations (35) | flexible multibody system (41) |
| dynamics (33) | multibody simulation (37) | flexible multibody system (133) | friction (51) | dynamics (21) | sensitivity analysis (39) |
| elastic multibody system (24) | railway vehicle (36) | large deformation (38) | contact force (43) | vehicle dynamics (19) | optimization (24) |
| finite element method (23) | railway dynamics (33) | dynamics (29) | impact (42) | simulation (19) | crashworthiness (23) |
| model reduction (23) | wear (32) | finite element method (27) | contact (35) | real-time simulation (17) | topology optimization (17) |
| model order reduction (20) | vehicle dynamics (32) | simulation (25) | clearance (26) | redundant constraints (14) | vehicle dynamics (16) |
| simulation (18) | derailment (27) | absolute nodal coordinate (24) | dynamics (26) | constrained multibody system (14) | simulation (16) |
| modal analysis (15) | finite element method (25) | finite element (18) | contact dynamics (25) | numerical integration (12) | parameter identification (15) |
| rigid-flexible coupling (14) | vehicle-track interaction (25) | modeling (15) | joint clearance (25) | subsystem synthesis method (11) | dynamics (12) |
| dynamic stiffening (13) | simulation (21) | large displacements (14) | revolute clearance joint (24) | flexible multibody system (10) | elastic multibody system (11) |
| modeling (11) | high-speed train (20) | floating frame of reference (13) | contact model (17) | dae (10) | suspension (10) |
| floating frame of reference (10) | rolling contact fatigue (19) | flexible multibody system dynamics (12) | contact mechanics (17) | equations of motion (10) | optimal control (9) |
| stability (10) | locomotive (19) | model reduction (11) | simulation (17) | computer aided analysis (8) | adjoint variable method (8) |
| bifurcation (10) | co-simulation (19) | floating frame of reference formulation (11) | flexible multibody system (16) | impact (8) | vibrations (8) |
| component mode synthesis (10) | multibody model (18) | sliding joint (11) | unilateral constraints (15) | multibody simulation (8) | structural optimization (7) |
| Most Commonly Used Keywords in the Following Communities: Two-wheelers (comm-7), Biomechanics (comm-8), Accurate and Efficient Integrators (comm-9), Robots as Multibody Systems (comm-10), Vibration and Transfer Matrix Method (comm-11) and Aeronautic Applications (comm-12). | |||||
|---|---|---|---|---|---|
| comm-7 | comm-8 | comm-9 | comm-10 | comm-11 | comm-12 |
| vehicle dynamics (27) | biomechanics (55) | flexible multibody dynamics (27) | dynamics (19) | transfer matrix method (80) | co-simulation (22) |
| motorcycle (26) | finite element analysis (34) | optimal control (24) | spatial operator algebra (11) | dynamics (21) | multibody simulation (18) |
| simulation (25) | multibody model (30) | lie groups (20) | high-speed train (11) | simulation (19) | stability (14) |
| modeling (22) | inverse dynamics (24) | differential-algebraic Equations (14) | simulation (10) | multiple launch rocket system (16) | aeroelasticity (12) |
| motorcycle dynamics (21) | simulation (21) | aeroelasticity (12) | polynomial chaos (9) | launch dynamics (16) | dynamics (8) |
| stability (20) | optimization (17) | time integration (12) | optimization (9) | vibration (10) | rotor (7) |
| dynamics (15) | gait (16) | constrained mechanical system (11) | uncertainty quantification (9) | discrete time transfer matrix method (9) | landing gear (7) |
| graph theory (12) | kinematics (15) | dynamics (9) | aerodynamics (8) | transfer matrix (8) | solver coupling (7) |
| bicycle dynamics (11) | multibody simulation (14) | natural coordinate (9) | inverse dynamics (8) | control (8) | turbocharger (6) |
| bond graph (11) | validation (14) | wind turbine (8) | uncertainty (8) | multibody simulation (8) | tiltrotor (6) |
| weave (10) | multibody dynamics analysis (12) | conserving time integration (7) | parallel computing (7) | natural frequency (8) | multibody model (5) |
| control (8) | musculoskeletal model (11) | finite rotations (7) | recursive algorithm (7) | rigid-flexible coupling (8) | helicopter (5) |
| flexible multibody system (8) | contact mechanics (11) | simulation (7) | flexible multibody system (6) | virtual prototype (7) | rotor dynamics (5) |
| wobble (8) | whiplash (10) | multibody model (6) | vehicle dynamics (6) | flexible multibody system (7) | vibration control (5) |
| optimal control (8) | knee (10) | nonlinear dynamics (6) | forward dynamics (6) | vibration control (7) | flight dynamics (5) |
| Most Commonly Used Keywords in the Following Communities: Marine Applications (comm-13), Powertrain Elements (comm-14), Road Vehicle Dynamics (comm-15), Wind Turbines (comm-16), Complex Terrain and Tracked Vehicles (comm-17) and Non-smooth Contact (comm-18). | |||||
|---|---|---|---|---|---|
| comm-13 | comm-14 | comm-15 | comm-16 | comm-17 | comm-18 |
| simulation (15) | vibration (23) | simulation (35) | wind turbine (49) | tracked vehicle (42) | finite element (14) |
| floating crane (14) | finite element method (19) | modelling (19) | gearbox (15) | dynamic simulation (14) | friction (11) |
| numerical simulation (14) | noise (18) | suspension (17) | drivetrain (12) | simulation (11) | multibody contact (11) |
| computational fluid dynamics (12) | diesel engine (17) | adams (15) | modal analysis (11) | terramechanics (9) | domain decomposition (9) |
| wave energy converter (10) | dynamics (14) | vehicle dynamics (14) | wind turbine gearbox (9) | virtual prototype (7) | frictional contact (8) |
| hydrodynamic interaction (9) | crankshaft (14) | dynamics (12) | drive train (9) | recurdyn (6) | finite element method (7) |
| dynamic response (8) | torsional vibration (12) | multibody model (10) | gear dynamics (8) | cvt (6) | dual lagrange multipliers (7) |
| virtual prototype (8) | simulation (12) | ride comfort (10) | dynamic response (8) | dynamics (5) | durability (7) |
| flexible multibody system dynamics (8) | flexible multibody dynamics (9) | vehicle handling (7) | simulation (8) | collaborative simulation (5) | linear elasticity (7) |
| dynamics (7) | crank train (8) | optimization (7) | flexible multibody (7) | rough terrain (5) | primal-dual active set strategy (6) |
| finite element method (7) | fatigue life (6) | leaf spring (7) | aeroelasticity (7) | deep ocean mining system (5) | fatigue life (6) |
| bond graph (6) | virtual prototype (6) | vibration (6) | flexible blade (7) | multibody dynamic model (5) | mortar finite element method (6) |
| drift force (6) | mixed lubrication (6) | virtual prototyping (6) | reliability (7) | suspension system (4) | scalability (6) |
| fpso (6) | harshness (6) | dynamic simulation (6) | dynamics (6) | electric vehicle (4) | contact problems (5) |
| hydrodynamics (6) | ic engine (6) | tyre model (5) | superelement (6) | track link (4) | multibody contact problem (5) |
| Most Commonly Used Keywords in the Following Communities: Space Applications (comm-19), Friction (comm-20), Flapping Wings (comm-21), Accident Reconstruction (comm-22), Fatigue and Life Assessment (comm-23) and CNC Machining (comm-24). | |||||
|---|---|---|---|---|---|
| comm-19 | comm-20 | comm-21 | comm-22 | comm-23 | comm-24 |
| optimal control (10) | coulomb friction (10) | co-simulation (12) | accident reconstruction (37) | fatigue life (12) | geometric error (29) |
| space robot (7) | contact (10) | vehicle (8) | head injury (21) | finite element method (10) | multibody system theory (26) |
| object manipulation (7) | friction (9) | semi-active suspension (8) | pedestrian (19) | fatigue life prediction (9) | error modeling (21) |
| attitude control (7) | parallel computing (7) | simulation (7) | multibody simulation (16) | fatigue analysis (6) | error compensation (20) |
| on-orbit servicing (7) | rigid bodies (6) | machine tool (6) | numerical simulation (11) | high-speed train (6) | machine tool (14) |
| motion planning (6) | collision detection (6) | dynamics (6) | madymo (10) | vibration (6) | sensitivity analysis (14) |
| numerical simulation (6) | contact dynamics (5) | flapping flight (6) | pedestrian safety (9) | simulation (6) | error identification (8) |
| flexibility (5) | rigid body dynamics (5) | insect flight (5) | impact biomechanics (7) | rigid-flexible coupling (6) | machining accuracy (8) |
| space manipulator system (5) | simulation (5) | modeling (5) | accident analysis (6) | fem (6) | five-axis machine tool (5) |
| underactuated system (5) | frictional contact (4) | ride comfort (5) | falls (6) | fatigue (5) | force ellipsoid (5) |
| space robots (5) | optimization (4) | vehicle engineering (4) | biomechanics (6) | virtual prototype (5) | multibody kinematics (5) |
| dynamics modelling (5) | complementarity (4) | control (4) | optimization (6) | dynamic stress analysis (5) | error measurement (4) |
| robotics (5) | modified state space (3) | aerial refueling (4) | vehicle-pedestrian collision (6) | carbody structure (5) | stiffness field (4) |
| rigid-flexible multibody system (5) | dynamics (3) | butterfly (4) | crash simulation (6) | recurdyn (5) | ultra-precision machine (4) |
| nonlinear control (4) | femdem (3) | optimization (4) | multibody model (5) | lunar lander (5) | homogeneous coordinate transformation (4) |
| Journal Name | % of Papers Published in the Journal |
|---|---|
| Multibody System Dynamics | 7.76 |
| SAE Technical Papers | 4.22 |
| Vehicle System Dynamics | 4.20 |
| Nonlinear Dynamics | 2.61 |
| Journal of Computational and Nonlinear Dynamics | 2.57 |
| Proceedings of the Institution of Mechanical Engineers, Part K: Journal of Multi-body Dynamics | 2.44 |
| Mechanism and Machine Theory | 1.91 |
| International Journal for Numerical Methods in Engineering | 1.37 |
| Journal of Sound and Vibration | 1.35 |
| Journal of Mechanical Science and Technology | 1.28 |
| Zhendong yu Chongji/Journal of Vibration and Shock | 1.27 |
| Computer Methods in Applied Mechanics and Engineering | 1.25 |
| Journal of Guidance, Control, and Dynamics | 1.21 |
| Computers and Structures | 1.19 |
| Civil-Comp Proceedings | 1.07 |
| Conference Name | % of Papers Published in the Proceedings |
|---|---|
| Proceedings of the ASME Design Engineering Technical Conference | 12.7 |
| Proceedings of the ECCOMAS Thematic Conference on Multibody Dynamics 2013 | 2.19 |
| ASME International Mechanical Engineering Congress and Exposition, Proceedings (IMECE) | 2.16 |
| Proceedings of the ASME International Design Engineering Technical Conferences and Computers and Information in Engineering Conference—DETC2005 | 2.13 |
| Proceedings of the ECCOMAS Thematic Conference on Multibody Dynamics 2015, Multibody Dynamics 2015 | 2.04 |
| Proceedings of the International Offshore and Polar Engineering Conference | 1.95 |
| 2007 Proceedings of the ASME International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, DETC2007 | 1.82 |
| Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering—OMAE | 1.70 |
| Annual Forum Proceedings—AHS International | 1.67 |
| Collection of Technical Papers—AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference | 1.45 |
| Proceedings of the International Astronautical Congress, IAC | 1.42 |
| Proceedings of the 8th ECCOMAS Thematic Conference on MULTIBODY DYNAMICS 2017, MBD 2017 | 1.36 |
| Proceedings - IEEE International Conference on Robotics and Automation | 1.36 |
| Advances in the Astronautical Sciences | 1.36 |
| 5th Asian Conference on Multibody Dynamics 2010, ACMD 2010 | 1.30 |
| Conference Name | % of Papers Published in the Proceedings |
|---|---|
| Proceedings of the ASME Design Engineering Technical Conference | 17.4 |
| Proceedings of the ECCOMAS Thematic Conference on Multibody Dynamics | 5.51 |
| Proceedings of the International Astronautical Congress, IAC | 2.08 |
| Country | Percentage of Authors by Country |
|---|---|
| China | 22.05 |
| United States | 16.33 |
| Germany | 11.43 |
| Italy | 8.38 |
| South Korea | 6.34 |
| Japan | 3.66 |
| United Kingdom | 3.21 |
| Spain | 2.83 |
| Belgium | 2.69 |
| France | 2.68 |
| Canada | 2.51 |
| Netherlands | 2.36 |
| Austria | 1.88 |
| Sweden | 1.08 |
| Finland | 1.04 |
| Portugal | 1.04 |
| Denmark | 0.80 |
| Australia | 0.71 |
| Switzerland | 0.67 |
| Czech Republic | 0.59 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
García-Vallejo, D.; Alcayde, A.; López-Martínez, J.; Montoya, F.G. Detection of Communities within the Multibody System Dynamics Network and Analysis of Their Relations. Symmetry 2019, 11, 1525. https://doi.org/10.3390/sym11121525
García-Vallejo D, Alcayde A, López-Martínez J, Montoya FG. Detection of Communities within the Multibody System Dynamics Network and Analysis of Their Relations. Symmetry. 2019; 11(12):1525. https://doi.org/10.3390/sym11121525
Chicago/Turabian StyleGarcía-Vallejo, Daniel, Alfredo Alcayde, Javier López-Martínez, and Francisco G. Montoya. 2019. "Detection of Communities within the Multibody System Dynamics Network and Analysis of Their Relations" Symmetry 11, no. 12: 1525. https://doi.org/10.3390/sym11121525
APA StyleGarcía-Vallejo, D., Alcayde, A., López-Martínez, J., & Montoya, F. G. (2019). Detection of Communities within the Multibody System Dynamics Network and Analysis of Their Relations. Symmetry, 11(12), 1525. https://doi.org/10.3390/sym11121525





