Nonlinear Dynamic Modelling of Two-Point and Symmetrically Supported Pipeline Brackets with Elastic-Porous Metal Rubber Damper
Abstract
1. Introduction
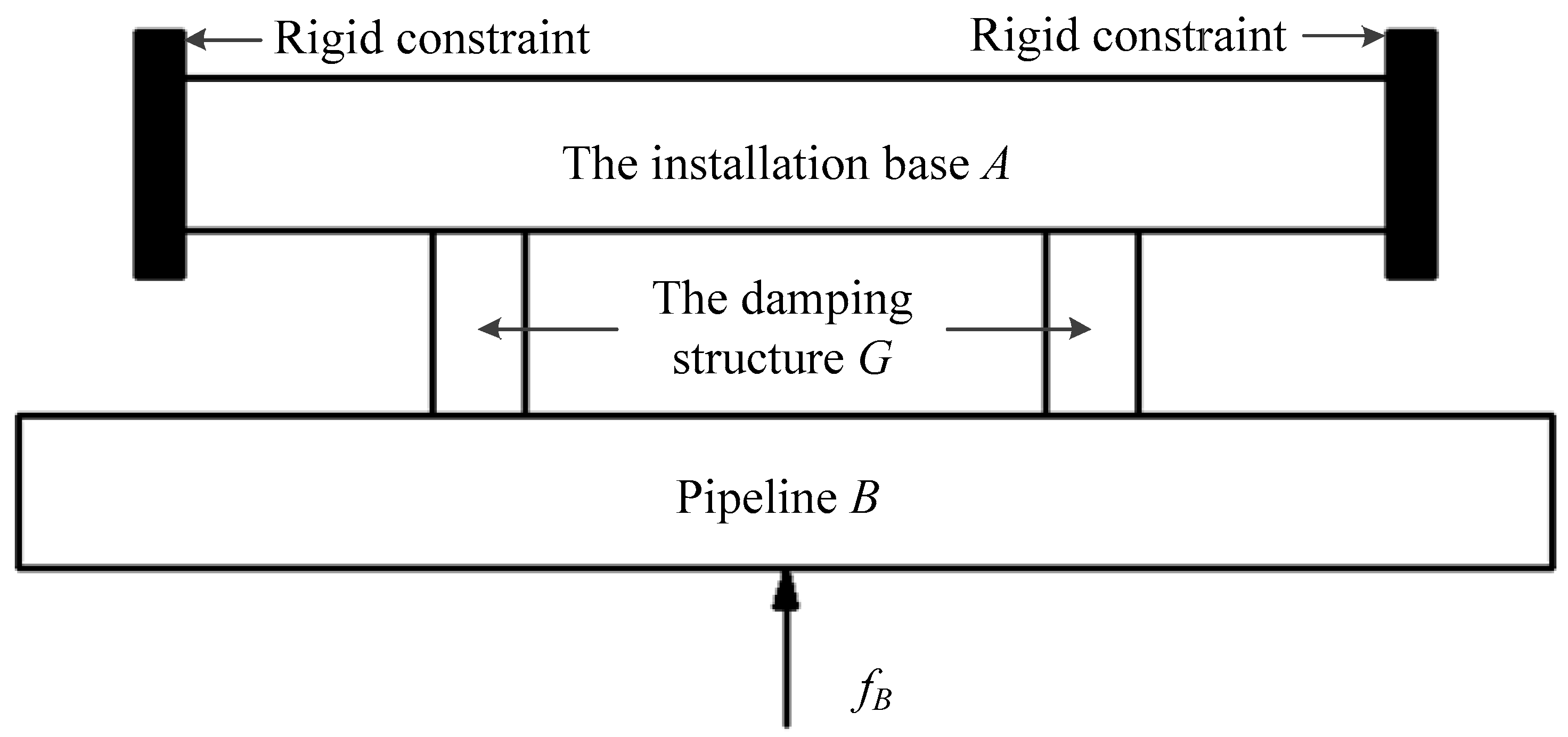
2. Description of Pipeline Vibration Isolation System
2.1. Model Simplifications
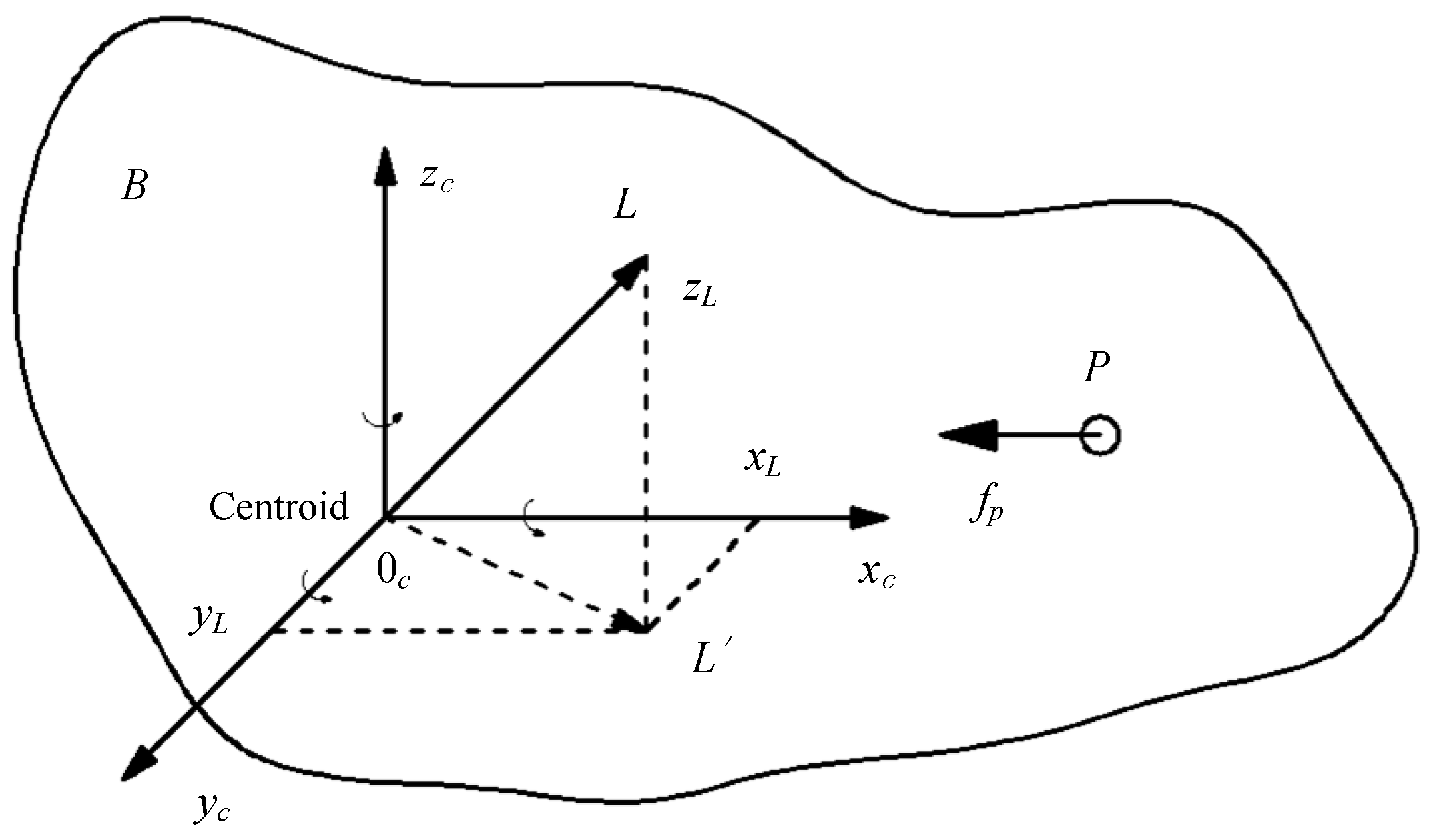
2.2. Junction Definition
3. Dynamic Modelling
3.1. Elastic Partial Impulse Response Function Matrix on I
3.2. Impulse Response Function Matrix on J
3.3. Response Analysis of Arbitrary Points on A and B Caused by Connecting Force
3.4. Dynamics Model
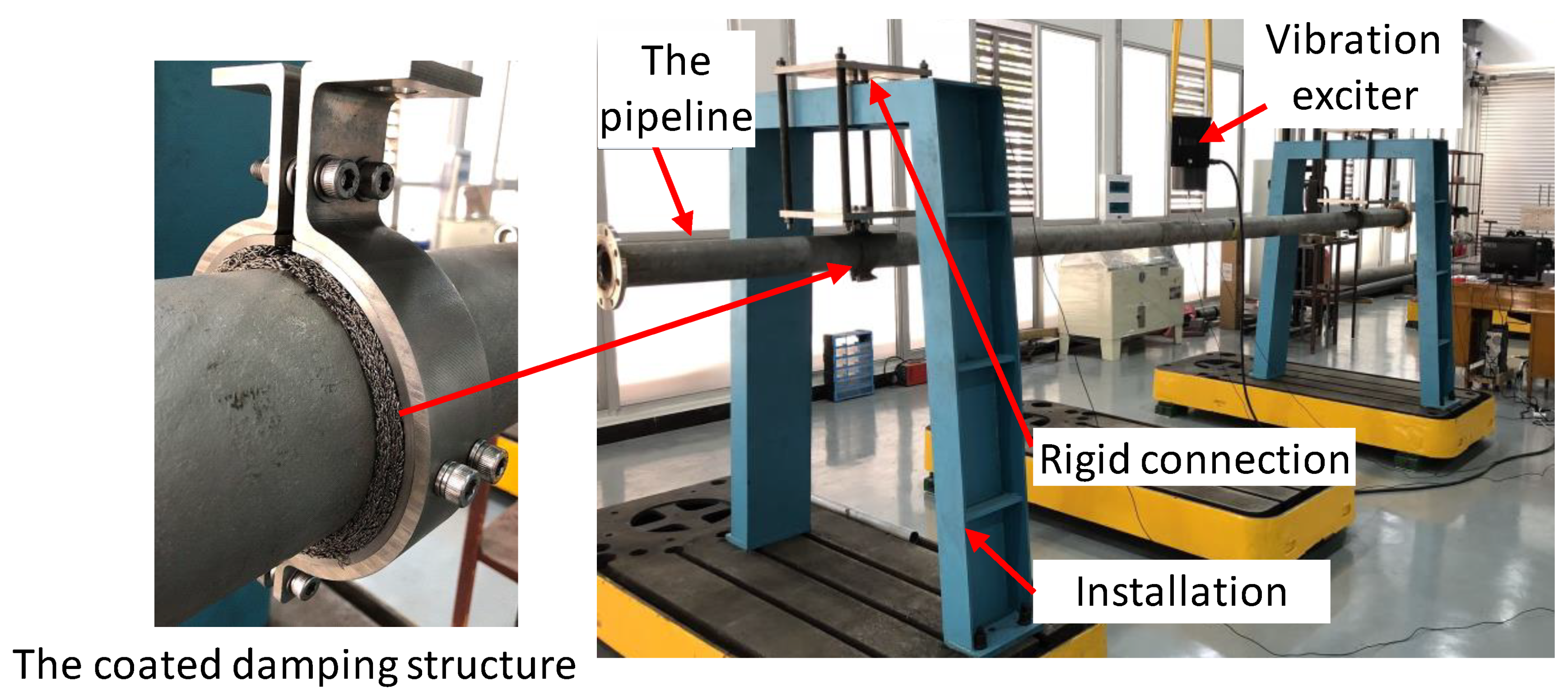
4. Experimental Verification
4.1. Case Description
4.2. Nonlinearity and Energy Consumption
4.3. Dynamic Test and Its Result
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Li, Y.D.; Yang, Y.R. Vibration analysis of conveying fluid pipe via He’s variational iteration method. Appl. Math. Model. 2016, 43, 409–420. [Google Scholar]
- Zhou, K.; Xiong, F.R.; Jiang, N.B.; Dai, H.L.; Yan, H.; Wang, L.; Ni, Q. Nonlinear vibration control of a cantilevered fluid-conveying pipe using the idea of nonlinear energy sink. Nonlinear Dyn. 2019, 95, 1435–1456. [Google Scholar] [CrossRef]
- Zhang, T.; Qu, Y.H.; Zhang, Y.O.; Lv, B.L. Nonlinear dynamics of straight fluid-conveying pipes with general boundary conditions and additional springs and masses. Appl. Math. Model. 2016, 40, 7880–7900. [Google Scholar] [CrossRef]
- Bao, R. Efficient dynamics analysis of large-deformation flexible beams by using the absolute nodal coordinate transfer matrix method. Multibody Syst. Dyn. 2014, 32, 535–549. [Google Scholar]
- Lin, W.; Qiao, N. Nonlinear dynamics of a fluid-conveying curved pipe subjected to motion-limiting constraints and a harmonic excitation. J. Fluids Struct. 2008, 24, 96–110. [Google Scholar] [CrossRef]
- Zhang, Z.W.; Wang, Y.J.; Wang, W.; Tian, Y.L. Periodic Solution of the Strongly Nonlinear Asymmetry System with the Dynamic Frequency Method. Symmetry 2019, 11, 676. [Google Scholar] [CrossRef]
- Meng, D.; Guo, H.Y.; Xu, S.P. Non-linear dynamic model of a fluid-conveying pipe undergoing overall motions. Appl. Math. Model. 2011, 35, 781–796. [Google Scholar] [CrossRef]
- Yu, D.L.; Wen, J.H.; Zhao, H.G. Vibration reduction by using the idea of phononic crystals in a pipe-conveying fluid. J. Sound Vib. 2008, 318, 193–205. [Google Scholar] [CrossRef]
- Bi, K.M.; Hao, H. Using pipe-in-pipe systems for subsea pipeline vibration control. Eng. Struct. 2016, 109, 75–84. [Google Scholar] [CrossRef]
- Modarres-Sadeghi, Y.; Paıdoussis, M.P. Nonlinear dynamics of extensible fluid-conveying pipes, supported at both ends. J. Fluids Struct. 2009, 25, 535–543. [Google Scholar] [CrossRef]
- Amabili, M. Nonlinear damping in large-amplitude vibrations: Modelling and experiments. Nonlinear Dyn. 2018, 93, 5–18. [Google Scholar] [CrossRef]
- Kong, X.R.; Li, H.Q.; Wu, C. Dynamics of 1-dof and 2-dof energy sink with geometrically nonlinear damping: Application to vibration suppression. Nonlinear Dyn. 2018, 91, 733–754. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Sarafrazi, S.R. Nonlinear dynamic structural analysis using dynamic relaxation with zero damping. Eng. Struct. 2011, 89, 1274–1285. [Google Scholar] [CrossRef]
- Zhai, J.; Li, J.; Wei, D.; Gao, P.; Yan, Y.; Han, Q. Vibration control of an aero pipeline system with active constraint layer damping treatment. Appl. Sci. 2019, 9, 2094. [Google Scholar] [CrossRef]
- Dong, G.X.; Zhang, Y.H.; Luo, Y.J.; Xie, S.; Zhang, X.N. Enhanced isolation performance of a high-static-low-dynamic stiffness isolator with geometric nonlinear damping. Nonlinear Dyn. 2018, 93, 2339–2356. [Google Scholar] [CrossRef]
- Gao, K.; Gao, W.; Wu, D. Nonlinear dynamic stability analysis of Euler-Bernoulli beam-columns with damping effects under thermal environment. Nonlinear Dyn. 2017, 90, 2423–2444. [Google Scholar] [CrossRef]
- Wang, G.P.; Rong, B.; Tao, L.; Rui, X.T. Riccati discrete time transfer matrix method for dynamic modeling and simulation of an underwater towed system. J. Appl. Mech. 2012, 79, 041014. [Google Scholar] [CrossRef]
- Rong, B.; Lu, K.; Rui, X.T.; Li, X.J.; Tao, L.; Wang, G.P. Nonlinear dynamics analysis of pipe conveying fluid by Riccati absolute nodal coordinate transfer matrix method. Nonlinear Dyn. 2018, 92, 699–708. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Sarafrazi, S.R.; Rezaiee, H. Efficiency of dynamic relaxation methods in nonlinear analysis of truss and frame structures. Comput. Struct. 2012, 112, 295–310. [Google Scholar] [CrossRef]
- Yang, P.; Bai, H.B.; Xue, X.; Xiao, K.; Zhao, X. Vibration reliability characterization and damping capability of annular periodic metal rubber in the non-molding direction. Mech. Syst. Signal Process. 2019, 132, 622–639. [Google Scholar] [CrossRef]
- Chandrasekhar, K.; Rongong, J.; Cross, E. Mechanical behaviour of tangled metal wire devices. Mech. Syst. Signal Process. 2019, 118, 13–29. [Google Scholar] [CrossRef]
- Zhang, D.Y.; Fabrizio, S.; Ma, Y.H. Dynamic mechanical behavior of nickel-based superalloy metal rubber. Mater. Des. 2014, 56, 69–77. [Google Scholar] [CrossRef]
- Wang, H.; Rongong, J.A.; Tomlinson, G.R. Nonlinear static and dynamic properties of metal rubber dampers. Int. Conf. Noise Vib. Eng. 2010, 10, 1301–1316. [Google Scholar]
- Wu, K.N.; Bai, H.B.; Xue, X.; Li, T.; Li, M. Energy dissipation characteristics and dynamic modeling of the coated damping structure for metal rubber of bellows. Metals 2018, 8, 562. [Google Scholar] [CrossRef]
- Ma, Y.H.; Zhang, Q.C.; Zhang, D.Y.; Scarpa, F.; Liu, B.L.; Hong, J. A novel smart rotor support with shape memory alloy metal rubber for high temperatures and variable amplitude vibrations. Smart Mater. Struct. 2014, 23, 125016. [Google Scholar] [CrossRef]
- Kwon, S.C.; Jo, M.S.; Oh, H.U. Experimental Validation of Fly-Wheel Passive Launch and On-Orbit Vibration Isolation System by Using a Superelastic SMA Mesh Washer Isolator. Int. J. Aerosp. Eng. 2017, 2017, 1–16. [Google Scholar] [CrossRef]
- Xiao, K.; Bai, H.B.; Xue, X.; Wu, Y.W. Damping characteristics of metal rubber in the pipeline coating system. Shock. Vib. 2018, 42, 1–11. [Google Scholar] [CrossRef]
- Ma, Y.H.; Zhang, Q.C.; Zhang, D.Y.; Scarpa, F.; Gao, D.; Hong, J. Size-dependent mechanical behavior and boundary layer effects in entangled metallic wire material systems. J. Mater. Sci. 2017, 52, 3741–3756. [Google Scholar] [CrossRef]
- Catania, G.; Strozzi, M. Damping oriented design of thin-walled mechanical components by means of multi-layer coating technology. Coatings 2018, 8, 73. [Google Scholar] [CrossRef]
- Hou, J.F.; Bai, H.B.; Li, D.W. Damping capacity measurement of elastic porous wire-mesh material in wide temperature range. J. Mater. Process. Technol. 2008, 206, 412–418. [Google Scholar] [CrossRef]
- Zhang, D.Y.; Fabrizio, S.; Ma, Y.H.; Boba, K.; Hong, J.; Lu, H.W. Compression mechanics of nickel-based superalloy metal rubber. Mater. Sci. Eng. A 2013, 580, 305–312. [Google Scholar] [CrossRef]
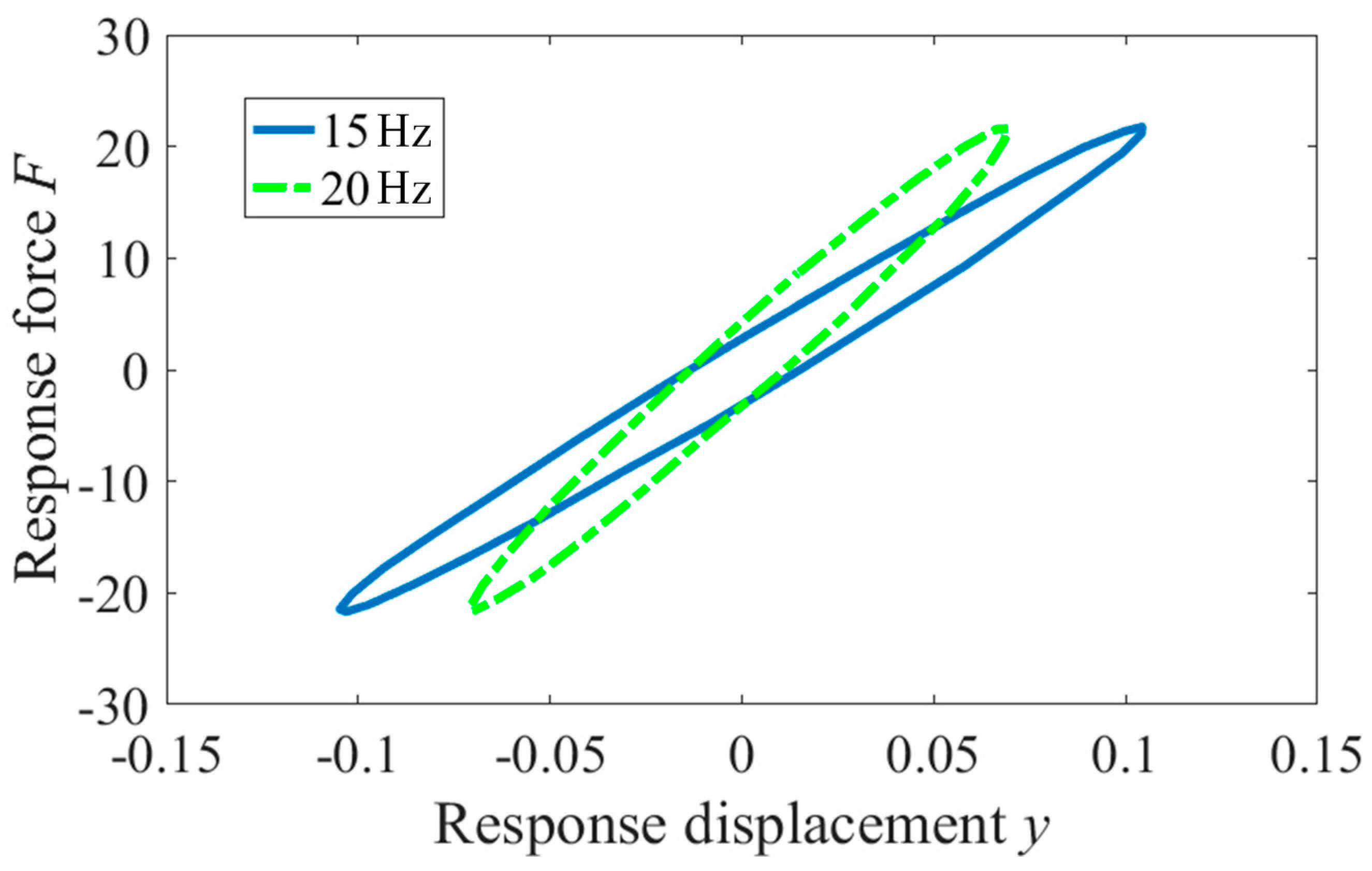
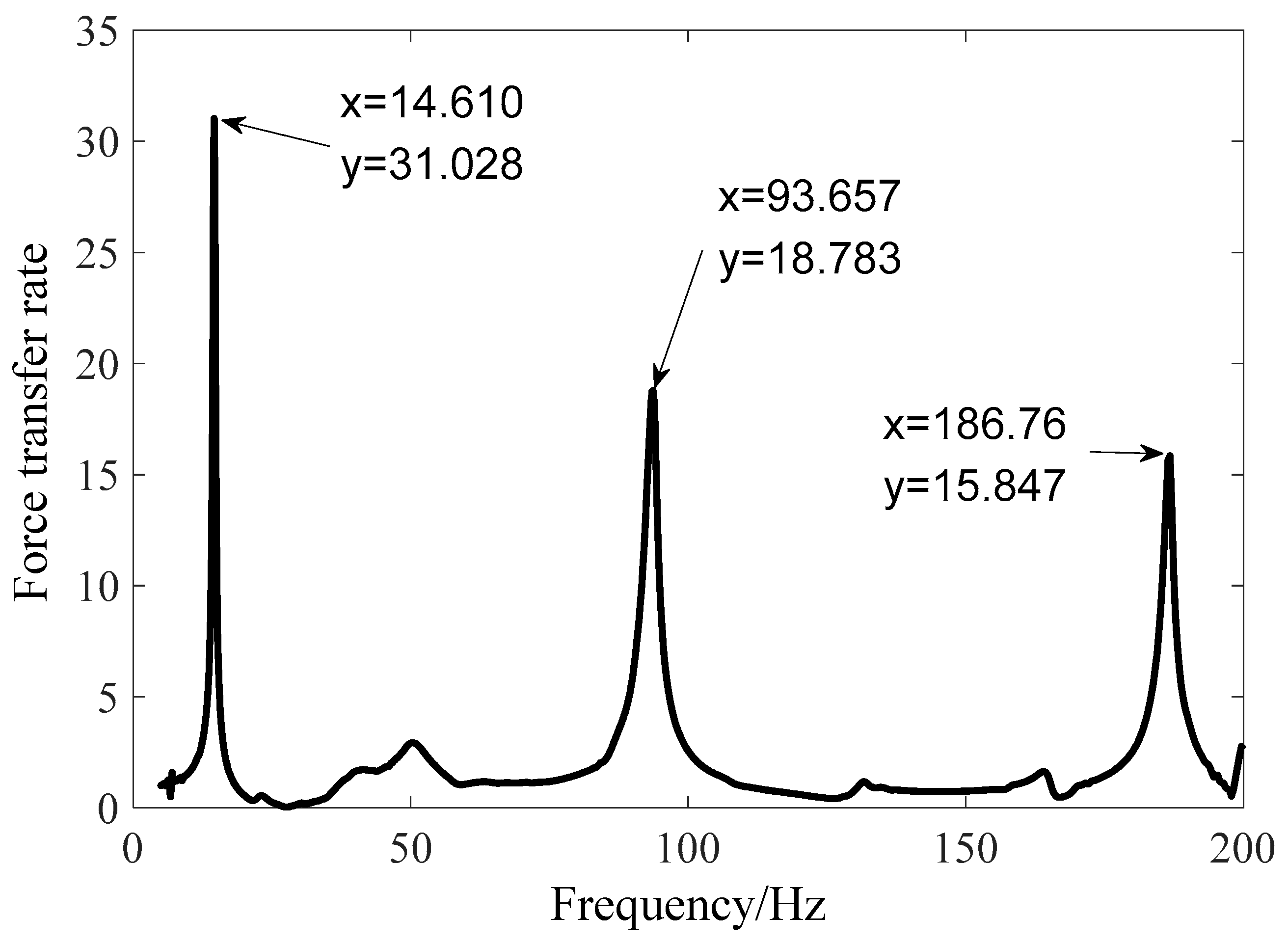

| Frequency (Hz) | Energy Dissipation ΔW (N·mm) | Maximum Elastic Potential Energy W (N·mm) | Loss Factor η |
|---|---|---|---|
| 15 | 1.016 | 1.136 | 0.142 |
| 20 | 0.822 | 0.752 | 0.174 |
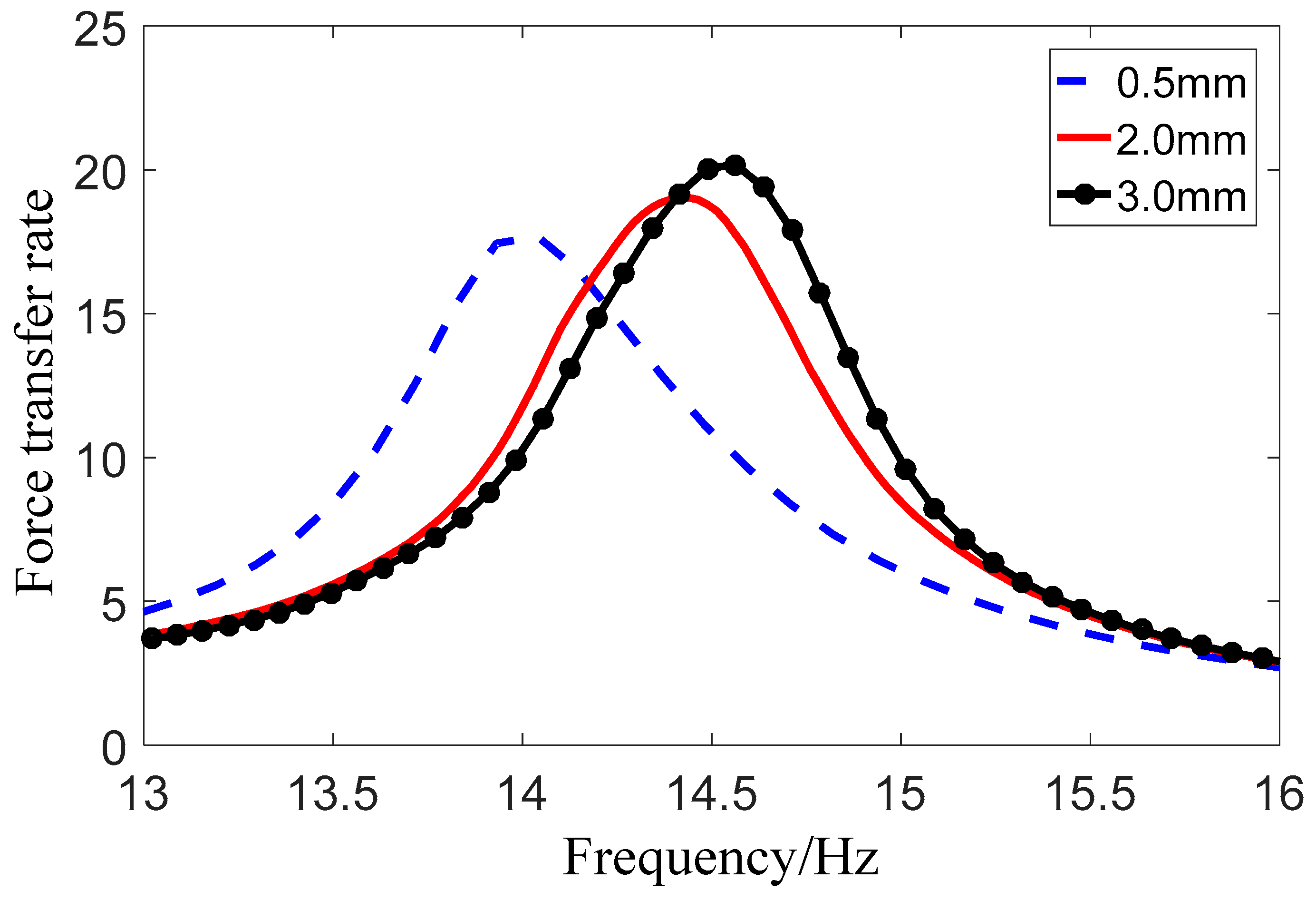
| Energy Dissipation Characteristics | Pre-Tightening Conditions (mm) | ||
|---|---|---|---|
| 0.5 | 2 | 3 | |
| Natural Frequency ωn (Hz) | 13.996 | 14.420 | 14.538 |
| Peak of the Force Transfer Rate TAm | 17.682 | 19.025 | 20.182 |
| Structural Loss Factor η | 0.0533 | 0.0454 | 0.0435 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, X.; Ruan, S.; Li, A.; Bai, H.; Xiao, K. Nonlinear Dynamic Modelling of Two-Point and Symmetrically Supported Pipeline Brackets with Elastic-Porous Metal Rubber Damper. Symmetry 2019, 11, 1479. https://doi.org/10.3390/sym11121479
Xue X, Ruan S, Li A, Bai H, Xiao K. Nonlinear Dynamic Modelling of Two-Point and Symmetrically Supported Pipeline Brackets with Elastic-Porous Metal Rubber Damper. Symmetry. 2019; 11(12):1479. https://doi.org/10.3390/sym11121479
Chicago/Turabian StyleXue, Xin, Shixin Ruan, Angxi Li, Hongbai Bai, and Kun Xiao. 2019. "Nonlinear Dynamic Modelling of Two-Point and Symmetrically Supported Pipeline Brackets with Elastic-Porous Metal Rubber Damper" Symmetry 11, no. 12: 1479. https://doi.org/10.3390/sym11121479
APA StyleXue, X., Ruan, S., Li, A., Bai, H., & Xiao, K. (2019). Nonlinear Dynamic Modelling of Two-Point and Symmetrically Supported Pipeline Brackets with Elastic-Porous Metal Rubber Damper. Symmetry, 11(12), 1479. https://doi.org/10.3390/sym11121479





