Certain Results for the Twice-Iterated 2D q-Appell Polynomials

Abstract

In this paper, the class of the twice-iterated 2D q-Appell polynomials is introduced. The generating function, series definition and some relations including the recurrence relations and partial q-difference equations of this polynomial class are established. The determinant expression for the twice-iterated 2D q-Appell polynomials is also derived. Further, certain twice-iterated 2D q-Appell and mixed type special q-polynomials are considered as members of this polynomial class. The determinant expressions and some other properties of these associated members are also obtained. The graphs and surface plots of some twice-iterated 2D q-Appell and mixed type 2D q-Appell polynomials are presented for different values of indices by using Matlab. Moreover, some areas of potential applications of the subject matter of, and the results derived in, this paper are indicated.

1. Introduction, Definitions and Preliminaries

The subject of q-calculus leads to a new method for computations and classifications of q-series and q-polynomials. In fact, the subject of q-calculus was initiated in the 1920s. However, it has gained considerable popularity and importance during the last three decades or so. In the past decade, q-calculus was developed into an interdisciplinary subject and it served as a bridge between mathematics and physics. The field has been expanded explosively due mainly to its applications in diverse areas of physics such as cosmic strings and black holes [1], conformal quantum mechanics [2], nuclear and high energy physics [3], fluid mechanics, combinatorics, having connection with commutativity relations, number theory, and Lie algebra. The definitions and notations of the q-calculus reviewed here are taken from [4] (see also [5,6]).

The q-analogue of the Pochhammer symbol ${(\alpha )}_m$, which is also called the q-shifted factorial, defined by

$${(\alpha ;q)}_0=1\mathrm{and}{(\alpha ;q)}_m=\prod _{r=0}^{m-1}(1-\alpha q^r)(m\in \mathbb{N};\alpha \in \mathbb{C}).$$

(1)

The q-analogues of a complex number $\alpha$ and of the factorial function are defined as follows:

$${[\alpha ]}_q=\frac{1-q^{\alpha }}{1-q}(q\in \mathbb{C}\backslash \{1\};\alpha \in \mathbb{C})$$

(2)

and

$$\begin{array}{cc}\hfill & {[m]}_q=\sum _{s=1}^m{q}^{s-1},{[0]}_q=0,{[m]}_q!=\prod _{s=1}^m{[s]}_q={[1]}_q{[2]}_q{[3]}_q\cdots {[m]}_q\mathrm{and}{[0]}_q!=1\hfill \\ \hfill & (m\in \mathbb{N};q\in \mathbb{C}\backslash \{0,1\}),\hfill \end{array}$$

(3)

where $\mathbb{N}$ is the set of positive integers.

The q-binomial coefficients ${\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q$ are defined by

$${\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q=\frac{{(q;q)}_m}{{(q;q)}_s{(q;q)}_{m-s}}=\frac{{[m]}_q!}{{[s]}_q!{[m-s]}_q!}(s=0,1,2,\cdots ,m).$$

(4)

The q-analogue of the classical derivative $Df$ or $\frac{d}{dt}f$ of a function f at a point $t\in \mathbb{C}\backslash \{0\}$ is defined by

$$D_{q}f(t)=\frac{f(t)-f(qt)}{(1-q)t}(0<|q|<1;t\ne 0).$$

(5)

We also note that

$$\begin{array}{cc}(\mathrm{i})& \underset{q\to 1}{lim}{D}_{q}f(t)=\frac{df(t)}{dt},\mathrm{where}\frac{d}{dt}\mathrm{denotes}\mathrm{the}\mathrm{classical}\mathrm{ordinary}\mathrm{derivative},\hfill \\ (\mathrm{ii})& D_q\left(a_{1}f(t)+a_{2}g(t)\right)=a_1{D}_{q}f(t)+a_2{D}_{q}g(t),\hfill \\ (\mathrm{iii})& D_q(fg)(t)=f(qt)D_{q}g(t)+g(t)D_{q}f(t)=f(t)D_{q}g(t)+D_{q}f(t)g(qt),\hfill \\ (\mathrm{vi})& D_q\left(\frac{f(t)}{g(t)}\right)=\frac{g(t)D_{q}f(t)-f(t)D_{q}g(t)}{g(t)g(qt)}=\frac{g(qt)D_{q}f(t)-f(qt)D_{q}g(t)}{g(t)g(qt)}.\hfill \end{array}$$

The two familiar q-analogues of the exponential function $e^t$ are given by

$$e_q(t):=\sum _{m=0}^{\infty }\frac{t^m}{{[m]}_q!}=\frac{1}{{\left((1-q)x;q\right)}_{\infty }}{,0<|q|<1,|x|<|1-q|}^{-1}$$

(6)

and

$$E_q(t):=\sum _{m=0}^{\infty }{q}^{\frac{1}{2}m(m-1)}\frac{t^m}{{[m]}_q!}={(-(1-q);q)}_{\infty }(0<|q|<1;t\in \mathbb{C}).$$

(7)

The above-defined q-exponential functions $e_q(t)$ and $E_q(t)$ satisfy the following properties:

$$\begin{array}{c}\hfill D_q{e}_q(t)=e_q(t),D_q{E}_q(t)=E_q(qt),\end{array}$$

(8)

$$\begin{array}{c}\hfill e_q(t)E_q(-t)=E_q(t)e_q(-t)=1.\end{array}$$

(9)

The class of Appell polynomials was introduced and characterized completely by Appell [7] in 1880. Further, Throne [8], Sheffer [9] and Varma [10] studied this class of polynomials from different points of view. For some subsequent and recent developments associated with the Appell polynomials, one may refer to the works [11,12,13,14].

In the year 1954, Sharma and Chak [15] introduced a q-analogue of the Appell polynomials and called this sequence of polynomials as q-Harmonics. Later, in the year 1967, Al-Salam [16] introduced the class of the q-Appell polynomials ${\{{\mathcal{A}}_{m,q}(x)\}}_{m=0}^{\infty }$ and studied some of their properties. Some characterizations of the q-Appell polynomials were presented by Srivastava [17] in the year 1982. These polynomials arise in numerous problems of applied mathematics, theoretical physics, approximation theory and many other branches of the mathematical sciences [7,18,19,20]. The polynomials ${\mathcal{A}}_{m,q}(x)$ (of degree m) are called q-Appell polynomials, provided that they satisfy the following q-differential equation:

$$D_{q,x}\{{\mathcal{A}}_{m,q}(x)\}={[m]}_q{\mathcal{A}}_{m-1,q}(x)(m\in {\mathbb{N}}_0=\mathbb{N}\cup \{0\};q\in \mathbb{C};0<|q|<1).$$

(10)

Recently, Keleshteri and Mahmudov [21] introduced the 2D q-Appell polynomials (2DqAP) ${\left\{{\mathcal{A}}_{m,q}(x_1,x_2)\right\}}_{m=0}^{\infty }$ which are defined by means of the following generating function:

$${\mathcal{A}}_q(t)e_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}(0<q<1),$$

(11)

where

$${\mathcal{A}}_q(t)=\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}\frac{t^m}{{[m]}_q!},{\mathcal{A}}_q(t)\ne 0\mathrm{and}{\mathcal{A}}_{0,q}=1.$$

(12)

We write

$${\mathcal{A}}_{m,q}:={\mathcal{A}}_{m,q}(0,0),$$

where ${\mathcal{A}}_{m,q}$ denotes the 2D q-Appell numbers.

For $x_2=0$, the 2DqAP ${\mathcal{A}}_{m,q}(x_1,x_2)$ reduce to the q-Appell polynomials ${\mathcal{A}}_{m,q}(x)$ (see, for example, [16,17,22]), that is,

$${\mathcal{A}}_{m,q}(x_1,0)={\mathcal{A}}_{m,q}(x_1),$$

(13)

where ${\mathcal{A}}_{m,q}(x)$ are defined by

$${\mathcal{A}}_q(t)e_q(xt)=\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}(x)\frac{t^m}{{[m]}_q!}(0<q<1)$$

(14)

and ${\mathcal{A}}_{m,q}$ given by

$${\mathcal{A}}_{m,q}:={\mathcal{A}}_{m,q}(0)$$

denotes the q-Appell numbers.

The explicit form of the 2DqAP ${\mathcal{A}}_{m,q}(x_1,x_2)$ in terms qAP ${\mathcal{A}}_{m,q}(x)$ is given as follows (see [21]):

$${\mathcal{A}}_{m,q}(x_1,x_2)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{\frac{1}{2}(m-s)(m-s-1)}{\mathcal{A}}_{s,q}(x_1)x_2^{m-s}.$$

(15)

The function ${\mathcal{A}}_q(t)$ may be called the determining function for the set ${\mathcal{A}}_{m,q}(x_1,x_2)$. Based on suitable selections for the function ${\mathcal{A}}_q(t)$, the following different members belonging to the family of the 2D q-Appell polynomials ${\mathcal{A}}_{m,q}(x_1,x_2)$ can be obtained:

I. 

If ${\mathcal{A}}_q(t)=\frac{t}{e_q(t)-1}$, the 2DqAP ${\mathcal{A}}_{m,q}(x_1,x_2)$ reduce to the 2D q-Bernoulli polynomials (2DqBP) ${\mathfrak{B}}_{m,q}(x_1,x_2)$ (see [23,24]), that is,

$${\mathcal{A}}_{m,q}(x_1,x_2)={\mathfrak{B}}_{m,q}(x_1,x_2),$$

where ${\mathfrak{B}}_{m,q}(x_1,x_2)$ are defined by

$$\frac{t}{e_q(t)-1}{e}_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\mathfrak{B}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}$$

(16)

and ${\mathfrak{B}}_{m,q}$ given by

$${\mathfrak{B}}_{m,q}:={\mathfrak{B}}_{m,q}(0,0)$$

denotes the 2D q-Bernoulli numbers.

II. 

If ${\mathcal{A}}_q(t)=\frac{2}{e_q(t)+1}$, the 2DqAP ${\mathcal{A}}_{m,q}(x_1,x_2)$ reduce to the 2D q-Euler polynomials (2DqEP) ${\mathcal{E}}_{m,q}(x_1,x_2)$ (see [23,24]), that is,

$${\mathcal{A}}_{m,q}(x_1,x_2)={\mathcal{E}}_{m,q}(x_1,x_2),$$

where ${\mathcal{E}}_{m,q}(x_1,x_2)$ are defined by

$$\frac{2}{e_q(t)+1}{e}_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\mathcal{E}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}$$

(17)

and ${\mathcal{E}}_{m,q}$ given by

$${\mathcal{E}}_{m,q}:={\mathcal{E}}_{m,q}(0,0)$$

denotes the 2D q-Euler numbers.

III. 

If ${\mathcal{A}}_q(t)=\frac{2t}{e_q(t)+1}$, the 2DqAP ${\mathcal{A}}_{m,q}(x_1,x_2)$ reduce to the 2D q-Genocchi polynomials (2DqGP) ${\mathcal{G}}_{m,q}(x_1,x_2)$ (see [23,24]; see also [25]), that is,

$${\mathcal{A}}_{m,q}(x_1,x_2)={\mathcal{G}}_{m,q}(x_1,x_2),$$

where ${\mathcal{G}}_{m,q}(x_1,x_2)$ are defined by

$$\frac{2t}{e_q(t)+1}{e}_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\mathcal{G}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}$$

(18)

and ${\mathcal{G}}_{m,q}:={\mathcal{G}}_{m,q}(0,0)$ denotes the 2D q-Genocchi numbers.

We recall here that, in a recent paper, Khan and Riyasat [26] introduced the twice-iterated q-Appell polynomials ${\mathcal{A}}_{m,q}^{[2]}(x)$ which are defined by means of the following generating function:

$${\dot{\mathcal{A}}}_q(t){\ddot{\mathcal{A}}}_q(t)e_q(xt)=\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(x)\frac{t^m}{{[m]}_q!}(0<q<1).$$

(19)

In this paper, the class of the twice-iterated 2D q-Appell polynomials is introduced by means of generating functions, recurrence relations, partial q-difference equations, and series and determinant expressions. Further, several results are obtained for the members corresponding to this polynomial family. In Section 2, the twice-iterated 2D q-Appell polynomials are introduced by means of the generating functions and series definition. Also, the recurrence relation and q-difference equations involving the twice-iterated 2D q-Appell polynomials are derived. In Section 3, a determinant expression for the twice-iterated 2D q-Appell polynomials is established. In Section 4, the determinant expressions and some other properties of the members belonging to the family of the twice-iterated 2D q-Appell polynomials are obtained. Section 5 provides several graphical representations and surface plots associated with several members of families of q-polynomials which have investigated in this paper. Finally, in Section 6, we present some concluding remarks and observations.

2. Twice-Iterated 2D q-Appell Polynomials

In order to introduce the twice-iterated 2D q-Appell polynomials (2I2DqAP), we consider two different sets of the 2D q-Appell polynomials ${\dot{\mathcal{A}}}_{m,q}(x_1,x_2)$ and ${\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)$ such that

$${\dot{\mathcal{A}}}_q(t)e_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\dot{\mathcal{A}}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}(0<q<1),$$

(20)

where

$${\dot{\mathcal{A}}}_q(t)=\sum _{m=0}^{\infty }{\dot{\mathcal{A}}}_{m,q}\frac{t^m}{{[m]}_q!},{\dot{\mathcal{A}}}_q(t)\ne 0\mathrm{and}{\dot{\mathcal{A}}}_{0,q}=1;$$

(21)

$${\ddot{\mathcal{A}}}_q(t)e_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}(0<q<1),$$

(22)

where

$${\ddot{\mathcal{A}}}_q(t)=\sum _{m=0}^{\infty }{\ddot{\mathcal{A}}}_{m,q}\frac{t^m}{{[m]}_q!},{\ddot{\mathcal{A}}}_q(t)\ne 0\mathrm{and}{\ddot{\mathcal{A}}}_{0,q}=1;$$

(23)

$${\ddot{\mathcal{A}}}_q(t)e_q(x_{1}t)=\sum _{m=0}^{\infty }{\ddot{\mathcal{A}}}_{m,q}(x_1)\frac{t^m}{{[m]}_q!}(0<q<1).$$

(24)

The generating function for the 2I2DqAP is asserted by the following result.

Theorem 1.

The generating function for the twice-iterated 2D q-Appell polynomials ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ is given by

$${\dot{\mathcal{A}}}_q(t){\ddot{\mathcal{A}}}_q(t)e_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!}(0<q<1).$$

(25)

Proof. 

By expanding the first q-exponential function $e_q(x_{1}t)$ in the left-hand side of the Equation (20) and then replacing the powers of x, that is, $x_1^0,x_1,x_1^2,\cdots ,x_1^m$ by the polynomials ${\ddot{\mathcal{A}}}_{0,q}(x_1),{\ddot{\mathcal{A}}}_{1,q}(x_1),$ ${\ddot{\mathcal{A}}}_{2,q}(x_1),\cdots ,{\ddot{\mathcal{A}}}_{m,q}(x_1)$ in the left-hand side and $x_1$ by ${\ddot{\mathcal{A}}}_{1,q}(x_1)$ in the right-hand side of the resultant equation, we have

$${\dot{\mathcal{A}}}_q(t)\left(1+{\ddot{\mathcal{A}}}_{1,q}(x_1)\frac{t}{{[1]}_q!}+{\ddot{\mathcal{A}}}_{2,q}(x_1)\frac{t^2}{{[2]}_q!}+\cdots +{\ddot{\mathcal{A}}}_{m,q}(x_1)\frac{t^m}{{[m]}_q!}+\cdots \right)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\dot{\mathcal{A}}}_{m,q}({\ddot{\mathcal{A}}}_{1,q}(x_1),x_2)\frac{t^m}{{[m]}_q!}.$$

(26)

Moreover, by summing up the series in the left-hand side and then using the Equation (24) in the resulting equation, we get

$${\dot{\mathcal{A}}}_q(t){\ddot{\mathcal{A}}}_q(t)e_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\dot{\mathcal{A}}}_{m,q}({\ddot{\mathcal{A}}}_{1,q}(x_1),x_2)\frac{t^m}{{[m]}_q!}.$$

(27)

Finally, denoting the resulting 2I2DqAP in the right-hand side of the above equation by ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$, that is,

$${\dot{\mathcal{A}}}_{m,q}({\ddot{\mathcal{A}}}_{1,q}(x_1),x_2)={\mathcal{A}}_{m,q}^{[2]}(x_1,x_2),$$

(28)

the assertion (25) of Theorem 1 is proved. □

Remark 1.

For $x_2=0$, the 2I2DqAP ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ reduce to the twice-iterated q-Appell polynomials (see [26]) such that

$${\mathcal{A}}_{m,q}^{[2]}(x_1):={\mathcal{A}}_{m,q}^{[2]}(x_1,0).$$

(29)

It is also noted that

$${\mathcal{A}}_{m,q}:={\mathcal{A}}_{m,q}(0)={\mathcal{A}}_{m,q}(0,0).$$

(30)

We next give the series definition for the 2I2DqAP ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ by proving the following result.

Theorem 2.

The twice-iterated 2D q-Appell polynomials ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ are given by the following series expression:

$${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\dot{\mathcal{A}}}_{s,q}{\ddot{\mathcal{A}}}_{m-s,q}(x_1,x_2).$$

(31)

Proof. 

In view of the Equations (21) and (22), the Equation (25) can be written as follows:

$$\sum _{s=0}^{\infty }{\dot{\mathcal{A}}}_{s,q}\frac{t^s}{{[s]}_q!}\sum _{m=0}^{\infty }{\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}=\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!},$$

(32)

which, on using the Cauchy product rule, gives

$$\sum _{m=0}^{\infty }\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\dot{\mathcal{A}}}_{s,q}{\ddot{\mathcal{A}}}_{m-s,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}=\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!}.$$

(33)

Equating the coefficients of like powers of t in both sides of the above equation, we arrive at the assertion (31) of Theorem 2. □

Remark 2.

For $x_2=0$, the series expression (31) becomes

$${\mathcal{A}}_{m,q}^{[2]}(x_1)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\dot{\mathcal{A}}}_{s,q}{\ddot{\mathcal{A}}}_{m-s,q}(x_1),$$

(34)

which is a known result [26] (p. 5, Equation (2.8)).

We now state and prove the following result.

Theorem 3.

The following relation between the twice-iterated 2D q-Appell polynomials ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ and the twice-iterated q-Appell polynomials ${\mathcal{A}}_{m,q}(x_1)$ holds true:

$${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{\frac{1}{2}s(s-1)}{x}_2^s{\mathcal{A}}_{m-s,q}^{[2]}(x_1).$$

(35)

Proof. 

Using the Equations (7) and (19) in the left-hand side of the generating function (25), we get

$$\begin{array}{c}\hfill \sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!}=\left(\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(x_1)\frac{t^m}{{[m]}_q!}\right)\left(\sum _{m=0}^{\infty }{q}^{\frac{1}{2}m(m-1)}\frac{{(x_{2}t)}^m}{{[m]}_q!}\right),\end{array}$$

(36)

which, on applying the Cauchy product rule in the left-hand side, yields

$$\begin{array}{c}\hfill \sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!}=\sum _{m=0}^{\infty }\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{\frac{1}{2}s(s-1)}{x}_2^s{\mathcal{A}}_{m-s,q}^{[2]}(x_1)\frac{t^m}{{[m]}_q!}.\end{array}$$

(37)

Finally, equating the coefficients of like powers of t on both sides of this last equation, we obtain the assertion (35) of Theorem 3. □

Remark 3.

By taking $x_2=1$ in the result (35), we get

$${\mathcal{A}}_{m,q}^{[2]}(x_1,1)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{\frac{1}{2}s(s-1)}{\mathcal{A}}_{m-s,q}^{[2]}(x_1).$$

(38)

Remark 4.

The following statements are equivalent:

$$(a){\mathcal{A}}_{m,q}^{[2]}(x_1,-x_2)={(-1)}^m{\mathcal{A}}_{m,q}^{[2]}(0,x_2)$$

(39)

and

$$(b){\mathcal{A}}_{m,q}^{[2]}(x_1)={(-1)}^m{\mathcal{A}}_{m,q}^{[2]}(0)$$

(40)

In order to derive the q-recurrence relations and the q-difference equations for the twice-iterated 2D q-Appell polynomials by using the lowering operators that are, in fact, the q-derivative operator $D_q$, we first prove the following lemma.

Lemma 1.

The twice-iterated 2D q-Appell polynomials ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ satisfy the following operational relations:

$$D_{q,x_1}\left({\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\right)={[m]}_q{\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2),$$

(41)

$$D_{q,x_2}\left({\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\right)={[m]}_q{\mathcal{A}}_{m-1,q}^{[2]}(x_1,q{x}_2),$$

(42)

$${\mathcal{A}}_{m-s,q}^{[2]}(x_1,x_2)=\frac{{[m-s]}_q!}{{[m]}_q!}{D}_{q,x_1}^s{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$$

(43)

and

$$q^{\frac{s(s-1)}{2}}{\mathcal{A}}_{m-s,q}^{[2]}(x_1,q^s{x}_2)=\frac{{[m-s]}_q!}{{[m]}_q!}{D}_{q,x_2}^s{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2).$$

(44)

Proof. 

In view of the Equation (25), the proof of the above lemma requires a direct use of the identity (5). We, therefore, skip the details involved. □

We now derive the q-recurrence relations for the 2I2DqAP ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$.

Theorem 4.

The twice-iterated 2D q-Appell polynomials ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ satisfy the following linear homogeneous recurrence relation:

$${\mathcal{A}}_{m,q}^{[2]}(q{x}_1,x_2)=\frac{1}{{[m]}_q}\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{m-s}({\alpha }_s+x_2{\beta }_s+{\gamma }_s){\mathcal{A}}_{m-s,q}^{[2]}(x_1,x_2)+x_1{q}^m{\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2),$$

(45)

where

$$\begin{array}{c}\hfill \begin{array}{c}\hfill t\frac{{\ddot{\mathcal{A}}}_q(t)D_{q,t}{\dot{\mathcal{A}}}_q(t)}{{\dot{\mathcal{A}}}_q(qt){\ddot{\mathcal{A}}}_q(qt)}=\sum _{m=0}^{\infty }{\alpha }_m\frac{t^m}{{[m]}_q!},t\frac{{\dot{\mathcal{A}}}_q(t){\ddot{\mathcal{A}}}_q(t)}{{\dot{\mathcal{A}}}_q(qt){\ddot{\mathcal{A}}}_q(qt)}=\sum _{m=0}^{\infty }{\beta }_m\frac{t^m}{{[m]}_q!},\\ \hfill t\frac{D_{q,t}{\ddot{\mathcal{A}}}_q(t)}{{\ddot{\mathcal{A}}}_q(qt)}=\sum _{m=0}^{\infty }{\gamma }_m\frac{t^m}{{[m]}_q!}.\end{array}\end{array}$$

(46)

Proof. 

Consider the following generating function:

$${\mathsf{G}}_q(q{x}_1,x_2,t)={\dot{\mathcal{A}}}_q(t){\ddot{\mathcal{A}}}_q(t)e_q(q{x}_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(q{x}_1,x_2)\frac{t^m}{{[m]}_q!}.$$

(47)

By taking the q-derivative of the Equation (47) partially with respect to t, we get

$$\begin{array}{cc}\hfill & D_{q,t}\left({\mathsf{G}}_q(q{x}_1,x_2,t)\right)=x_2{\dot{\mathcal{A}}}_q(t){\ddot{\mathcal{A}}}_q(t)e_q(qxt)E_q(q{x}_{2}t)+q{x}_1{\dot{\mathcal{A}}}_q(qt){\ddot{\mathcal{A}}}_q(qt)e_q(qxt)E_q(q{x}_{2}t)\hfill \\ \hfill & +\left(D_{q,t}{\dot{\mathcal{A}}}_q(t)\right){\ddot{\mathcal{A}}}_q(t)e_q(qxt)E_q(q{x}_{2}t)+{\dot{\mathcal{A}}}_q(qt)\left(D_{q,t}{\ddot{\mathcal{A}}}_q(t)\right)e_q(qxt)E_q(q{x}_{2}t).\hfill \end{array}$$

(48)

Thus, upon factorizing ${\mathsf{G}}_q(q{x}_1,x_2,t)$ occurring in the left-hand side and multiplying both sides of the identity (48) by t, we find that

$$\begin{array}{cc}\hfill & t{D}_{q,t}\left({\mathsf{G}}_q(q{x}_1,x_2,t)\right)\hfill \\ \hfill & ={\mathsf{G}}_q(q{x}_1,x_2,t)\left(t\frac{{\ddot{\mathcal{A}}}_q(t)D_{q,t}{\dot{\mathcal{A}}}_q(t)}{{\dot{\mathcal{A}}}_t(qt){\ddot{\mathcal{A}}}_q(qt)}+x_{2}t\frac{{\dot{\mathcal{A}}}_q(t){\ddot{\mathcal{A}}}_q(t)}{{\dot{\mathcal{A}}}_q(qt){\ddot{\mathcal{A}}}_q(qt)}+t\frac{D_{q,t}{\ddot{\mathcal{A}}}_q(t)}{{\ddot{\mathcal{A}}}_q(qt)}+qt{x}_1\right).\hfill \end{array}$$

(49)

In view of the assumption (46) and the Equation (47), the Equation (49) becomes

$$\begin{array}{cc}\hfill & \sum _{m=0}^{\infty }{[m]}_q{\mathcal{A}}_{m,q}^{[2]}(q{x}_1,x_2)\frac{t^m}{{[m]}_q!}\hfill \\ \hfill & =\sum _{m=0}^{\infty }{q}^m{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!}\left(\sum _{m=0}^{\infty }{\alpha }_m\frac{t^m}{{[m]}_q!}+x_2\sum _{m=0}^{\infty }{\beta }_m\frac{t^m}{{[m]}_q!}+\sum _{m=0}^{\infty }{\gamma }_m\frac{t^m}{{[m]}_q!}+q{x}_1\right),\hfill \end{array}$$

(50)

which, on using the Cauchy product rule, gives

$$\begin{array}{cc}\hfill & \sum _{m=0}^{\infty }{[m]}_q{\mathcal{A}}_{m,q}^{[2]}(q{x}_1,x_2)\frac{t^m}{{[m]}_q!}\hfill \\ \hfill & =\sum _{m=0}^{\infty }\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{m-s}({\alpha }_s+x_2{\beta }_s+{\gamma }_s){\mathcal{A}}_{m-s,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!}\hfill \\ \hfill & +x_1\sum _{m=0}^{\infty }{[m]}_q{q}^m{\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!}.\hfill \end{array}$$

(51)

Finally, upon equating the coefficients of like powers of t on both sides of the above equation and dividing both sides of the resulting equation by ${[m]}_q$, we get the assertion (45) of Theorem 4. □

We now state and prove the following result.

Theorem 5.

The following recurrence relation for the twice-iterated 2D q-Appell polynomials ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ holds true:

$${\mathcal{A}}_{m,q}^{[2]}(q{x}_1,x_2)=q^{m-1}\left(\frac{{\ddot{\mathcal{A}}}_q(t)D_{q,t}{\dot{\mathcal{A}}}_q(t)}{{\dot{\mathcal{A}}}_q(qt){\ddot{\mathcal{A}}}_q(qt)}+x_2\frac{{\dot{\mathcal{A}}}_q(t){\ddot{\mathcal{A}}}_q(t)}{{\dot{\mathcal{A}}}_q(qt){\ddot{\mathcal{A}}}_q(qt)}+\frac{D_{q,t}{\ddot{\mathcal{A}}}_q(t)}{{\ddot{\mathcal{A}}}_q(qt)}+q{x}_1\right){\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2).$$

(52)

Proof. 

We first use the Equation (47) in both sides of the Equation (49). Then, after some simplification, by equating the coefficients of like powers of t on both sides of the resulting equation, we arrive at the assertion (52) of Theorem 5. □

We next derive the q-difference equations which are satisfied by the twice-iterated 2D q-Appell polynomials.

Theorem 6.

The twice-iterated 2D q-Appell polynomials ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ are the solutions of the following q-difference equations:

$$\left(\sum _{s=0}^m\frac{q^{m-s}}{{[s]}_q}({\alpha }_s+x_2{\beta }_s+{\gamma }_s)D_{q,x_1}^s+x_1{q}^m{D}_{q,x_1}\right){\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)-{[m]}_q{\mathcal{A}}_{m,q}^{[2]}(q{x}_1,x_2)=0$$

(53)

or

$$\sum _{s=0}^m\frac{q^{m-s}}{{[s]}_q}\left({\alpha }_s+x_2\frac{{\beta }_s}{q^s}+{\gamma }_s\right)D_{q,x_2}^s{\mathcal{A}}_{m,q}^{[2]}\left(x_1,\frac{x_2}{q^s}\right)+x_1{q}^m{D}_{q,x_2}{\mathcal{A}}_{m,q}^{[2]}\left(x_1,\frac{x_2}{q}\right)-{[m]}_q{\mathcal{A}}_{m,q}^{[2]}(q{x}_1,x_2)=0.$$

(54)

Proof. 

The proof of the assertions (53) and (54) of Theorem 6 would follow directly upon using the Equations (43) and (44), respectively, in the recurrence relation (45). □

In the next section (Section 3 below), the determinant forms for the 2I2DqAP are established.

3. The Twice-Iterated 2D q-Appell Polynomials from the Determinant Viewpoint

One of the important aspects of the study of any polynomial system is to find its potentially useful determinant representation. Recently, Keleshteri and Mahmudov [21] introduced the determinant definitions for the q-Appell polynomials and the 2D q-Appell polynomials. These polynomials are useful in finding the solutions of some general linear interpolation problems and can also be used for computational purposes. Khan and Riyasat [26], on the other hand, established the determinant expressions for the twice-iterated q-Appell polynomials. This fact provides motivation for us to establish the determinant definitions and the determinant expressions for the twice-iterated 2D q-Appell polynomials 2I2DqAP by proving the following result.

Theorem 7.

The 2I2DqAP ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ of degree m are defined by

$${\mathcal{A}}_{0,q}^{[2]}(x_1,x_2)=\frac{1}{{\mathcal{B}}_{0,q}},$$

(55)

$$\begin{array}{c}\hfill \begin{array}{c}\hfill {\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)=\frac{{(-1)}^m}{{({\mathcal{B}}_{0,q})}^{m+1}}\left|\begin{array}{cccccc}1& {\ddot{\mathcal{A}}}_{1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{2,q}(x_1,x_2)& \cdots & {\ddot{\mathcal{A}}}_{m-1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_m(x_1,x_2)\\ {\mathcal{B}}_{0,q}& {\mathcal{B}}_{1,q}& {\mathcal{B}}_{2,q}& \cdots & {\mathcal{B}}_{m-1,q}& {\mathcal{B}}_{m,q}\\ 0& {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& \cdots & {\left[\genfrac{}{}{0pt}{}{m-1}{1}\right]}_q{\mathcal{B}}_{m-2,q}& {\left[\genfrac{}{}{0pt}{}{m}{1}\right]}_q{\mathcal{B}}_{m-1,q}\\ 0& 0& {\mathcal{B}}_{0,q}& \cdots & {\left[\genfrac{}{}{0pt}{}{m-1}{2}\right]}_q{\mathcal{B}}_{m-3,q}& {\left[\genfrac{}{}{0pt}{}{m}{2}\right]}_q{\mathcal{B}}_{m-2,q}\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮\\ 0& 0& 0& \cdots & {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}\end{array}\right|,\end{array}\\ \hfill {\mathcal{B}}_{m,q}=-\frac{1}{{\dot{\mathcal{A}}}_{0,q}}\left(\sum _{s=1}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\dot{\mathcal{A}}}_{s,q}{\mathcal{B}}_{m-s,q}\right)(m\in \mathbb{N}),\end{array}$$

(56)

where ${\mathcal{B}}_{0,q}\ne 0$, ${\mathcal{B}}_{0,q}=\frac{1}{{\dot{\mathcal{A}}}_{0,q}}$ and ${\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)(m\in {\mathbb{N}}_0)$ are the q-Appell polynomials of degree m.

Proof. 

Consider ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ as a sequence of the 2I2DqAP defined by the Equation (25). Also let ${\dot{\mathcal{A}}}_{m,q}$ and ${\mathcal{B}}_{m,q}$ be two numerical sequences (the coefficients of the q-Taylor series expansions of functions) such that

$${\dot{\mathcal{A}}}_q(t)={\dot{\mathcal{A}}}_{0,q}+{\dot{\mathcal{A}}}_{1,q}\frac{t}{{[1]}_q!}+{\dot{\mathcal{A}}}_{2,q}\frac{t^2}{{[2]}_q!}+\cdots +{\dot{\mathcal{A}}}_{m,q}\frac{t^m}{{[m]}_q!}+\cdots (m\in {\mathbb{N}}_0;{\dot{\mathcal{A}}}_{0,q}\ne 0)$$

(57)

and

$${\widehat{\dot{\mathcal{A}}}}_q(t)={\mathcal{B}}_{0,q}+{\mathcal{B}}_{1,q}\frac{t}{{[1]}_q!}+{\mathcal{B}}_{2,q}\frac{t^2}{{[2]}_q!}+\cdots +{\mathcal{B}}_{m,q}\frac{t^m}{{[m]}_q!}+\cdots (m\in {\mathbb{N}}_0;{\mathcal{B}}_{0,q}\ne 0),$$

(58)

also satisfying the following condition:

$${\dot{\mathcal{A}}}_q(t){\widehat{\dot{\mathcal{A}}}}_q(t)=1.$$

(59)

On using the Cauchy product rule for the two-series product ${\dot{\mathcal{A}}}_q(t){\widehat{\dot{\mathcal{A}}}}_q(t)$, we get

$$\begin{array}{cc}\hfill {\dot{\mathcal{A}}}_q(t){\widehat{\dot{\mathcal{A}}}}_q(t)& =\sum _{m=0}^{\infty }{\dot{\mathcal{A}}}_{m,q}\frac{t^m}{{[m]}_q!}\sum _{m=0}^{\infty }{\mathcal{B}}_{m,q}\frac{t^m}{{[m]}_q!}\hfill \\ \hfill & =\sum _{m=0}^{\infty }\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\dot{\mathcal{A}}}_{s,q}{\mathcal{B}}_{m-s,q}\frac{t^m}{{[m]}_q!}.\hfill \end{array}$$

Consequently, we have

$$\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\dot{\mathcal{A}}}_{s,q}{\mathcal{B}}_{m-s,q}=\left\{\begin{array}{c}1,ifm=0,\hfill \\ 0,ifm\in \mathbb{N}.\hfill \end{array}\right.$$

(60)

that is,

$$\left\{\begin{array}{c}{\mathcal{B}}_{0,q}=\frac{1}{{\dot{\mathcal{A}}}_{0,q}};\hfill \\ {\mathcal{B}}_{m,q}=-\frac{1}{{\dot{\mathcal{A}}}_{0,q}}\left(\sum _{s=1}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\dot{\mathcal{A}}}_{s,q}{\mathcal{B}}_{m-s,q}\right)(m\in {\mathbb{N}}_0).\hfill \end{array}\right.$$

(61)

Next, upon multiplying both sides of the Equation (25) by ${\widehat{\dot{\mathcal{A}}}}_q(t)$, we get

$${\dot{\mathcal{A}}}_q(t){\widehat{\dot{\mathcal{A}}}}_q(t){\ddot{\mathcal{A}}}_q(t)e_q(x_{1}t)E_q(x_{2}t)={\widehat{\dot{\mathcal{A}}}}_q(t)\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!}.$$

(62)

Further, in view of the Equations (22), (58) and (59), the above Equation (62) becomes

$$\sum _{m=0}^{\infty }{\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}=\sum _{m=0}^{\infty }{\mathcal{B}}_{m,q}\frac{t^m}{{[m]}_q!}\sum _{m=0}^{\infty }{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)\frac{t^m}{{[m]}_q!}.$$

(63)

Now, on using the Cauchy product rule for the two series in the right-hand side of the Equation (63), we obtain the following infinite system for the unknowns ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$:

$$\left\{\begin{array}{c}{\mathcal{B}}_{0,q}{\mathcal{A}}_{0,q}^{[2]}(x_1,x_2)=1;\hfill \\ {\mathcal{B}}_{1,q}{\mathcal{A}}_{0,q}^{[2]}(x_1,x_2)+{\mathcal{B}}_{0,q}{\mathcal{A}}_{1,q}^{[2]}(x_1,x_2)={\ddot{\mathcal{A}}}_{1,q}(x_1,x_2),\hfill \\ {\mathcal{B}}_{2,q}{\mathcal{A}}_{0,q}^{[2]}(x_1,x_2)+{\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}{\mathcal{A}}_{1,q}^{[2]}(x_1,x_2)+{\mathcal{B}}_{0,q}{\mathcal{A}}_{2,q}^{[2]}(x_1,x_2)={\ddot{\mathcal{A}}}_{2,q}(x_1,x_2),\hfill \\ ⋮\hfill \\ {\mathcal{B}}_{m-1,q}{\mathcal{A}}_{0,q}^{[2]}(x_1,x_2)+{\left[\genfrac{}{}{0pt}{}{m-1}{1}\right]}_q{\mathcal{B}}_{m-2,q}{\mathcal{A}}_{1,q}^{[2]}(x_1,x_2)+\cdots +{\mathcal{B}}_{0,q}{\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2)={\ddot{\mathcal{A}}}_{m-1,q}(x_1,x_2),\hfill \\ {\mathcal{B}}_{m,q}{\mathcal{A}}_{0,q}^{[2]}(x_1,x_2)+{\left[\genfrac{}{}{0pt}{}{m}{1}\right]}_q{\mathcal{B}}_{m-1,q}{\mathcal{A}}_{1,q}^{[2]}(x_1,x_2)+\cdots +{\mathcal{B}}_{0,q}{\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)={\ddot{\mathcal{A}}}_{m,q}(x_1,x_2),\hfill \\ ⋮\hfill \end{array}\right.$$

(64)

Obviously, the first equation of the system (64) leads to our first assertion (55). The coefficient matrix of the system (64) is lower triangular, so this helps us to obtain the unknowns ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ by applying the Cramer rule to the first $m+1$ equations of the system (64). Accordingly, we can obtain

$${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)=\frac{\left|\begin{array}{cccccc}{\mathcal{B}}_{0,q}& 0& 0& \cdots & 0& 1\\ {\mathcal{B}}_{1,q}& {\mathcal{B}}_{0,q}& 0& \cdots & 0& {\ddot{\mathcal{A}}}_{1,q}(x_1,x_2)\\ {\mathcal{B}}_{2,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& {\mathcal{B}}_{0,q}& \cdots & 0& {\ddot{\mathcal{A}}}_{2,q}(x_1,x_2)\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮\\ {\mathcal{B}}_{m-1,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{1}\right]}_q{\mathcal{B}}_{m-2,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{2}\right]}_q{\mathcal{B}}_{m-3,q}& \cdots & {\mathcal{B}}_{0,q}& {\ddot{\mathcal{A}}}_{m-1,q}(x_1,x_2)\\ {\mathcal{B}}_{m,q}& {\left[\genfrac{}{}{0pt}{}{m}{1}\right]}_q{\mathcal{B}}_{m-1,q}& {\left[\genfrac{}{}{0pt}{}{m}{2}\right]}_q{\mathcal{B}}_{m-2,q}& \cdots & {\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}& {\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)\end{array}\right|}{\left|\begin{array}{cccccc}{\mathcal{B}}_{0,q}& 0& 0& \cdots & 0& 1\\ {\mathcal{B}}_{1,q}& {\mathcal{B}}_{0,q}& 0& \cdots & 0& 0\\ {\mathcal{B}}_{2,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& {\mathcal{B}}_{0,q}& \cdots & 0& 0\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮\\ {\mathcal{B}}_{m-1,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{1}\right]}_q{\mathcal{B}}_{m-2,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{2}\right]}_q{\mathcal{B}}_{m-3,q}& \cdots & {\mathcal{B}}_{0,q}& 0\\ {\mathcal{B}}_{m,q}& {\left[\genfrac{}{}{0pt}{}{m}{1}\right]}_q{\mathcal{B}}_{m-1,q}& {\left[\genfrac{}{}{0pt}{}{m}{2}\right]}_q{\mathcal{B}}_{m-2,q}& \cdots & {\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}& {\mathcal{B}}_{0,q}\end{array}\right|},$$

(65)

where $m\in \mathbb{N}$. Thus, upon expanding the determinant in the denominator and taking the transpose of the determinant in the numerator, we get

$$\begin{array}{c}\hfill {\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)=\frac{1}{{({\mathcal{B}}_{0,q})}^{m+1}}\left|\begin{array}{cccccc}{\mathcal{B}}_{0,q}& {\mathcal{B}}_{1,q}& {\mathcal{B}}_{2,q}& \cdots & {\mathcal{B}}_{m-1,q}& {\mathcal{B}}_{n,q}\\ 0& {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& \cdots & {\left[\genfrac{}{}{0pt}{}{m-1}{1}\right]}_q{\mathcal{B}}_{m-2,q}& {\left[\genfrac{}{}{0pt}{}{m}{1}\right]}_q{\mathcal{B}}_{m-1,q}\\ 0& 0& {\mathcal{B}}_{0,q}& \cdots & {\left[\genfrac{}{}{0pt}{}{m-1}{2}\right]}_q{\mathcal{B}}_{m-3,q}& {\left[\genfrac{}{}{0pt}{}{m}{2}\right]}_q{\mathcal{B}}_{m-2,q}\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮\\ 0& 0& 0& \cdots & {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}\\ 1& {\ddot{\mathcal{A}}}_{1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{2,q}(x_1,x_2)& \cdots & {\ddot{\mathcal{A}}}_{m-1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)\end{array}\right|.\end{array}$$

(66)

Finally, after m circular row exchanges, that is, after moving the $j\mathrm{th}$ row to the $(j+1)\mathrm{st}$ position for $j=1,2,3,\cdots ,m-1$, we arrive at our assertion (56) of Theorem 7. □

Theorem 8.

The following identity for the 2I2DqAP ${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)$ holds true:

$${\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)=\frac{1}{{\mathcal{B}}_{0,q}}\left({\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)-\sum _{s=0}^{m-1}{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\mathcal{B}}_{m-s,q}{\mathcal{A}}_{s,q}^{[2]}(x_1,x_2)\right)(m\in \mathbb{N}).$$

(67)

Proof. 

Expanding the determinant in the Equation (56) with respect to the ${(m+1)}^{\mathrm{st}}$ row, we get

$$\begin{array}{c}\hfill \begin{array}{c}\hfill {\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)=\frac{{(-1)}^m}{{({\mathcal{B}}_{0,q})}^{m+1}}{\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}\left|\begin{array}{cccccc}1& {\ddot{\mathcal{A}}}_{1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{2,q}(x_1,x_2)& \cdots & \cdots & {\ddot{\mathcal{A}}}_{m-1,q}(x_1,x_2)\\ {\mathcal{B}}_{0,q}& {\mathcal{B}}_{1,q}& {\mathcal{B}}_{2,q}& \cdots & \cdots & {\mathcal{B}}_{m-1,q}\\ 0& {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-1}{1}\right]}_q{\mathcal{B}}_{m-2,q}\\ 0& 0& {\mathcal{B}}_{0,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-1}{2}\right]}_q{\mathcal{B}}_{m-3,q}\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮\\ 0& 0& 0& \cdots & {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{m-2}\right]}_q{\mathcal{B}}_{1,q}\end{array}\right|\end{array}\end{array}$$

$$\begin{array}{c}\hfill \begin{array}{c}\hfill +\frac{{(-1)}^m}{{({\mathcal{B}}_{0,q})}^{m+1}}\left|\begin{array}{ccccccc}1& {\ddot{\mathcal{A}}}_{1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{2,q}(x_1,x_2)& \cdots & \cdots & {\ddot{\mathcal{A}}}_{m-2,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)\\ {\mathcal{B}}_{0,q}& {\mathcal{B}}_{1,q}& {\mathcal{B}}_{2,q}& \cdots & \cdots & {\mathcal{B}}_{m-1,q}& {\mathcal{B}}_{m-1,q}\\ 0& {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-2}{1}\right]}_q{\mathcal{B}}_{m-3,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{1}\right]}_q{\mathcal{B}}_{m-2,q}\\ 0& 0& {\mathcal{B}}_{0,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-2}{2}\right]}_q{\mathcal{B}}_{m-4,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{2}\right]}_q{\mathcal{B}}_{m-3,q}\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮& ⋮\\ 0& 0& 0& \cdots & \cdots & {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{m-2}\right]}_q{\mathcal{B}}_{1,q}\end{array}\right|\end{array}\end{array}$$

$$\begin{array}{c}\hfill \begin{array}{c}\hfill =\frac{-1}{{\mathcal{B}}_{0,q}}{\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}{\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2)+\frac{{(-1)}^m}{{({\mathcal{B}}_{0,q})}^m}\\ \hfill \left|\begin{array}{ccccccc}1& {\ddot{\mathcal{A}}}_{1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{2,q}(x_1,x_2)& \cdots & \cdots & {\ddot{\mathcal{A}}}_{m-2,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)\\ {\mathcal{B}}_{0,q}& {\mathcal{B}}_{1,q}& {\mathcal{B}}_{2,q}& \cdots & \cdots & {\mathcal{B}}_{m-1,q}& {\mathcal{B}}_{m-1,q}\\ 0& {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-2}{1}\right]}_q{\mathcal{B}}_{m-3,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{1}\right]}_q{\mathcal{B}}_{m-2,q}\\ 0& 0& {\mathcal{B}}_{0,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-2}{2}\right]}_q{\mathcal{B}}_{m-4,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{2}\right]}_q{\mathcal{B}}_{m-3,q}\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮& ⋮\\ 0& 0& 0& \cdots & \cdots & {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{m-2}\right]}_q{\mathcal{B}}_{1,q}\end{array}\right|.\end{array}\end{array}$$

Next, by applying the same argument for the last determinant, we find that

$$\begin{array}{c}\hfill \begin{array}{c}\hfill {\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)=\frac{-1}{{\mathcal{B}}_{0,q}}{\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}{\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2)+\frac{{(-1)}^m}{{({\mathcal{B}}_{0,q})}^m}{\left[\genfrac{}{}{0pt}{}{m-1}{m-2}\right]}_q{\mathcal{B}}_{2,q}\\ \hfill \left|\begin{array}{ccccccc}1& {\ddot{\mathcal{A}}}_{1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{2,q}(x_1,x_2)& \cdots & \cdots & {\ddot{\mathcal{A}}}_{m-3,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{m-2,q}(x_1,x_2)\\ {\mathcal{B}}_{0,q}& {\mathcal{B}}_{1,q}& {\mathcal{B}}_{2,q}& \cdots & \cdots & {\mathcal{B}}_{m-3,q}& {\mathcal{B}}_{m-2,q}\\ 0& {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-3}{1}\right]}_q{\mathcal{B}}_{m-4,q}& {\left[\genfrac{}{}{0pt}{}{m-2}{1}\right]}_q{\mathcal{B}}_{m-3,q}\\ 0& 0& {\mathcal{B}}_{0,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-3}{2}\right]}_q{\mathcal{B}}_{m-5,q}& {\left[\genfrac{}{}{0pt}{}{m-2}{2}\right]}_q{\mathcal{B}}_{m-4,q}\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮& ⋮\\ 0& 0& 0& \cdots & \cdots & {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{m-2}{m-3}\right]}_q{\mathcal{B}}_{1,q}\end{array}\right|\end{array}\end{array}$$

$$\begin{array}{c}\hfill \begin{array}{c}\hfill +\frac{{(-1)}^{m+2}}{{({\mathcal{B}}_{0,q})}^m}{\mathcal{B}}_{0,q}\left|\begin{array}{ccccccc}1& {\ddot{\mathcal{A}}}_{1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{2,q}(x_1,x_2)& \cdots & \cdots & {\ddot{\mathcal{A}}}_{m-3,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)\\ {\mathcal{B}}_{0,q}& {\mathcal{B}}_{1,q}& {\mathcal{B}}_{2,q}& \cdots & \cdots & {\mathcal{B}}_{m-3,q}& {\mathcal{B}}_{m-1,q}\\ 0& {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-3}{1}\right]}_q{\mathcal{B}}_{m-4,q}& {\left[\genfrac{}{}{0pt}{}{m}{1}\right]}_q{\mathcal{B}}_{m-2,q}\\ 0& 0& {\mathcal{B}}_{0,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-3}{2}\right]}_q{\mathcal{B}}_{m-5,q}& {\left[\genfrac{}{}{0pt}{}{m}{2}\right]}_q{\mathcal{B}}_{m-2,q}\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮& ⋮\\ 0& 0& 0& \cdots & \cdots & {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{m-2}\right]}_q{\mathcal{B}}_{2,q}\end{array}\right|\end{array}\end{array}$$

$$\begin{array}{c}\hfill \begin{array}{c}\hfill =\frac{-1}{{\mathcal{B}}_{0,q}}{\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}{\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2)-\frac{-1}{({\mathcal{B}}_{0,q})}{\left[\genfrac{}{}{0pt}{}{m-1}{m-2}\right]}_q{\mathcal{B}}_{2,q}{\mathcal{A}}_{m-2,q}^{[2]}(x_1,x_2)+\frac{{(-1)}^{m-2}}{{({\mathcal{B}}_{0,q})}^{m-1}}\\ \hfill \left|\begin{array}{ccccccc}1& {\ddot{\mathcal{A}}}_{1,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{2,q}(x_1,x_2)& \cdots & \cdots & {\ddot{\mathcal{A}}}_{m-3,q}(x_1,x_2)& {\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)\\ {\mathcal{B}}_{0,q}& {\mathcal{B}}_{1,q}& {\mathcal{B}}_{2,q}& \cdots & \cdots & {\mathcal{B}}_{m-3,q}& {\mathcal{B}}_{m-1,q}\\ 0& {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q{\mathcal{B}}_{1,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-3}{1}\right]}_q{\mathcal{B}}_{m-4,q}& {\left[\genfrac{}{}{0pt}{}{m}{1}\right]}_q{\mathcal{B}}_{m-2,q}\\ 0& 0& {\mathcal{B}}_{0,q}& \cdots & \cdots & {\left[\genfrac{}{}{0pt}{}{m-3}{2}\right]}_q{\mathcal{B}}_{m-5,q}& {\left[\genfrac{}{}{0pt}{}{m}{2}\right]}_q{\mathcal{B}}_{m-2,q}\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮& ⋮\\ 0& 0& 0& \cdots & \cdots & {\mathcal{B}}_{0,q}& {\left[\genfrac{}{}{0pt}{}{m-1}{m-2}\right]}_q{\mathcal{B}}_{2,q}\end{array}\right|.\end{array}\end{array}$$

Again, we apply the same technique recursively until we arrive at the following consequence:

$$\begin{array}{c}\hfill {\mathcal{A}}_{m,q}^{[2]}(x_1,x_2)=\frac{-1}{{\mathcal{B}}_{0,q}}{\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}{\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2)-\frac{1}{({\mathcal{B}}_{0,q})}{\left[\genfrac{}{}{0pt}{}{m-1}{m-2}\right]}_q{\mathcal{B}}_{2,q}{\mathcal{A}}_{m-2,q}^{[2]}(x_1,x_2)\\ \hfill -\cdots -\frac{1}{{({\mathcal{B}}_{0,q})}^2}\left|\begin{array}{cc}1& {\ddot{\mathcal{A}}}_{n,q}(x_1,x_2)\\ {\mathcal{B}}_{0,q}& {\mathcal{B}}_{m,q}\end{array}\right|\end{array}$$

$$\begin{array}{c}\hfill =\frac{-1}{{\mathcal{B}}_{0,q}}{\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q{\mathcal{B}}_{1,q}{\mathcal{A}}_{m-1,q}^{[2]}(x_1,x_2)-\frac{1}{({\mathcal{B}}_{0,q})}{\left[\genfrac{}{}{0pt}{}{m-1}{m-2}\right]}_q{\mathcal{B}}_{2,q}{\mathcal{A}}_{m-2,q}^{[2]}(x_1,x_2)\\ \hfill -\cdots -\frac{1}{({\mathcal{B}}_{0,q})}{\mathcal{B}}_{m,q}{\mathcal{A}}_{0,q}^{[2]}(x_1,x_2)+\frac{1}{{\mathcal{B}}_{0,q}}{\ddot{\mathcal{A}}}_{n,q}(x_1,x_2).\end{array}$$

(68)

Finally, upon summing up the series in the left-hand side of the Equation (68), we arrive at the assertion (67) of Theorem 8. □

Corollary 1.

The following identity for the 2DqAP ${\ddot{\mathcal{A}}}_{n,q}(x_1,x_2)$ holds true:

$${\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\mathcal{B}}_{m-s,q}{\mathcal{A}}_{k,q}^{[2]}(x_1,x_2)(m\in \mathbb{N}).$$

(69)

4. Several Members of the Twice-Iterated 2D $\mathit{q}$-Appell Polynomials

During the last two decades, much research work has been done for different members of the family of the q-Appell polynomials and the 2D q-Appell polynomials. By making suitable selections for the functions ${\dot{\mathcal{A}}}_q(t)$ and ${\ddot{\mathcal{A}}}_q(t)$, the members belonging to the family of the twice-iterated 2D q-Appell polynomials ${\mathcal{A}}_{k,q}^{[2]}(x_1,x_2)$ can be obtained. The 2D q-Bernoulli polynomials ${\mathfrak{B}}_{m,q}(x_1,x_2)$, the 2D q-Euler polynomials ${\mathcal{E}}_{m,q}(x_1,x_2)$ and the 2D q-Genocchi polynomials ${\mathcal{G}}_{m,q}(x_1,x_2)$ are important members of the 2D q-Appell family. Therefore, in this section, we first introduce the 2D q-Euler based Bernoulli polynomials (2DqEBP) ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ and the 2D q-Genocchi based Bernoulli polynomials (2DqGBP) ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ by means of their respective generating functions and series definitions. We then explore other properties of these members.

4.1. The 2D q-Euler–Bernoulli Polynomials

Since, for

$${\mathcal{A}}_q(t)=\frac{2}{e_q(t)+1}\mathrm{and}{\mathcal{A}}_q(t)=\frac{t}{e_q(t)-1},$$

the 2DqAP ${\mathcal{A}}_{m,q}(x_1,x_2)$ reduce to the 2DqEP ${\mathcal{E}}_{m,q}(x_1,x_2)$ and the 2DqBP ${\mathfrak{B}}_{m,q}(x_1,x_2)$, respectively. Therefore, for the same choices of $A_q(t)$, that is,

$${\dot{\mathcal{A}}}_q(t)=\frac{2}{e_q(t)+1}\mathrm{and}{\ddot{\mathcal{A}}}_q(t)=\frac{t}{e_q(t)-1},$$

the 2I2DqAP reduce to 2DqEBP ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ and are defined by means of generating functions as follows:

$$\frac{2t}{\left(e_q(t)+1\right)\left(e_q(t)-1\right)}{e}_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}(0<q<1).$$

(70)

The 2DqEBP ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ of degree m are defined by the following series:

$${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\mathfrak{B}}_{s,q}{\mathcal{E}}_{m-s,q}(x_1,x_2).$$

(71)

The following relation between the 2DqEBP ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ and the qEBP ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1)$ holds true:

$${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{\frac{1}{2}s(s-1)}{x}_2^s{}_{\mathcal{E}}{\mathfrak{B}}_{m-s,q}(x_1),$$

(72)

which, for $x_2=1$, yields

$${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,1)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{\frac{1}{2}s(s-1)}{}_{\mathcal{E}}{\mathfrak{B}}_{m-s,q}(x_1).$$

(73)

The 2DqEBP ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ satisfy the following recurrence relation:

$$\begin{array}{c}{}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(q{x}_1,x_2)=q^{m-2}\\ ·\left(\frac{t\left(e_q(qt)-1\right)\left(x_2\left(e_q(qt)+1\right)-e_q(t)\right)+\left(e_q(t)-t{e}_q(t)-1\right)\left(e_q(t)+1\right)}{t\left(e_q(t)+1\right)\left(e_q(t)-1\right)}+q^2{x}_1\right){}_{\mathcal{E}}{\mathfrak{B}}_{m-1,q}(x_1,x_2).\end{array}$$

(74)

Further, by taking

$${\mathcal{B}}_{0,q}=1,$$

$${\mathcal{B}}_{j,q}=\frac{1}{[j+1]}(j\in \mathbb{N})$$

and

$${\ddot{\mathcal{A}}}_{m,q}(x_1,x_2)={\mathcal{E}}_{m,q}(x_1,x_2)$$

in the Equation (56), we obtain the determinant definition of the 2DqEBP ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ as given below.

Definition 1.

The 2D q-Euler–Bernoulli polynomials ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ of degree m are defined by

$$\begin{array}{c}\hfill {}_{\mathcal{E}}{\mathfrak{B}}_{0,q}(x_1,x_2)=1,\end{array}$$

(75)

$$\begin{array}{c}\hfill \begin{array}{c}\hfill {}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)={(-1)}^m\left|\begin{array}{cccccc}1& {\mathcal{E}}_{1,q}(x_1,x_2)& {\mathcal{E}}_{2,q}(x_1,x_2)& \cdots & {\mathcal{E}}_{m-1,q}(x_1,x_2)& {\mathcal{E}}_m(x_1,x_2)\\ 1& \frac{1}{{[2]}_q}& \frac{1}{{[3]}_q}& \cdots & \frac{1}{{[m]}_q}& \frac{1}{{[m+1]}_q}\\ 0& 1& {\left[\genfrac{}{}{0pt}{}{2}{1}\right]}_q\frac{1}{{[2]}_q}& \cdots & {\left[\genfrac{}{}{0pt}{}{m-1}{1}\right]}_q\frac{1}{{[m-1]}_q}& {\left[\genfrac{}{}{0pt}{}{m}{1}\right]}_q\frac{1}{{[m]}_q}\\ 0& 0& 1& \cdots & {\left[\genfrac{}{}{0pt}{}{m-1}{2}\right]}_q\frac{1}{{[m-2]}_q}& {\left[\genfrac{}{}{0pt}{}{m}{2}\right]}_q\frac{1}{{[m-1]}_q}\\ ⋮& ⋮& ⋮& \ddots & ⋮& ⋮\\ 0& 0& 0& \cdots & 1& {\left[\genfrac{}{}{0pt}{}{m}{m-1}\right]}_q\frac{1}{{[2]}_q}\end{array}\right|\end{array}\\ \hfill (m\in \mathbb{N}),\end{array}$$

(76)

where ${\mathcal{E}}_{m,q}(x_1,x_2)(m\in {\mathbb{N}}_0)$ are the 2D q-Euler polynomials of degree m.

4.2. The 2D q-Genocchi–Bernoulli Polynomials

Since, for

$${\mathcal{A}}_q(t)=\frac{2t}{e_q(t)+1}\mathrm{and}{\mathcal{A}}_q(t)=\frac{t}{e_q(t)-1},$$

the 2DqAP ${\mathcal{A}}_{m,q}(x_1,x_2)$ reduce to the 2DqGP ${\mathcal{G}}_{m,q}(x_1,x_2)$ and the 2DqBP ${\mathfrak{B}}_{m,q}(x_1,x_2)$, respectively. Therefore, for the same choices of $A_q(t)$, that is,

$${\dot{\mathcal{A}}}_q(t)=\frac{2t}{e_q(t)+1}\mathrm{and}{\ddot{\mathcal{A}}}_q(t)=\frac{t}{e_q(t)-1},$$

the 2I2DqAP reduce to 2DqGBP ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ and are defined by means of generating functions as follows:

$$\frac{2t^2}{\left(e_q(t)+1\right)\left(e_q(t)-1\right)}{e}_q(x_{1}t)E_q(x_{2}t)=\sum _{m=0}^{\infty }{}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)\frac{t^m}{{[m]}_q!}(0<q<1).$$

(77)

The 2DqGBP ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ of degree m are defined by the following series:

$${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{\mathfrak{B}}_{s,q}{\mathcal{G}}_{m-s,q}(x_1,x_2).$$

(78)

The following relation between the 2DqGBP ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ and the qGBP ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1)$ holds true:

$${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{\frac{1}{2}s(s-1)}{x}_2^s{}_{\mathcal{G}}{\mathfrak{B}}_{m-s,q}(x_1),$$

(79)

which, for $x_2=1$, gives

$${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,1)=\sum _{s=0}^m{\left[\genfrac{}{}{0pt}{}{m}{s}\right]}_q{q}^{\frac{1}{2}s(s-1)}{}_{\mathcal{G}}{\mathfrak{B}}_{m-s,q}(x_1).$$

(80)

The 2DqGBP ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ satisfy the following recurrence relation:

$$\begin{array}{c}{}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(q{x}_1,x_2)=q^{m-3}·\left(\frac{\left(e_q(qt)+1\right)\left(e_q(t)-t{e}_q(t)+1+x_{2}t\left(e_q(qt)+1\right)\right)}{t\left(e_q(t)+1\right)\left(e_q(t)-1\right)}\right.\hfill \\ \hfill \left.+\frac{q\left(e_q(t)-t{e}_q(t)-1\right)\left(e_q(t)+1\right)}{t\left(e_q(t)+1\right)\left(e_q(t)-1\right)}+q^3{x}_1\right){}_{\mathcal{G}}{\mathfrak{B}}_{m-1,q}(x_1,x_2).\end{array}$$

(81)

In the next section (Section 5 below), we give some graphical representations and the surface plots of some of the members of the twice-iterated 2D q-Appell polynomials.

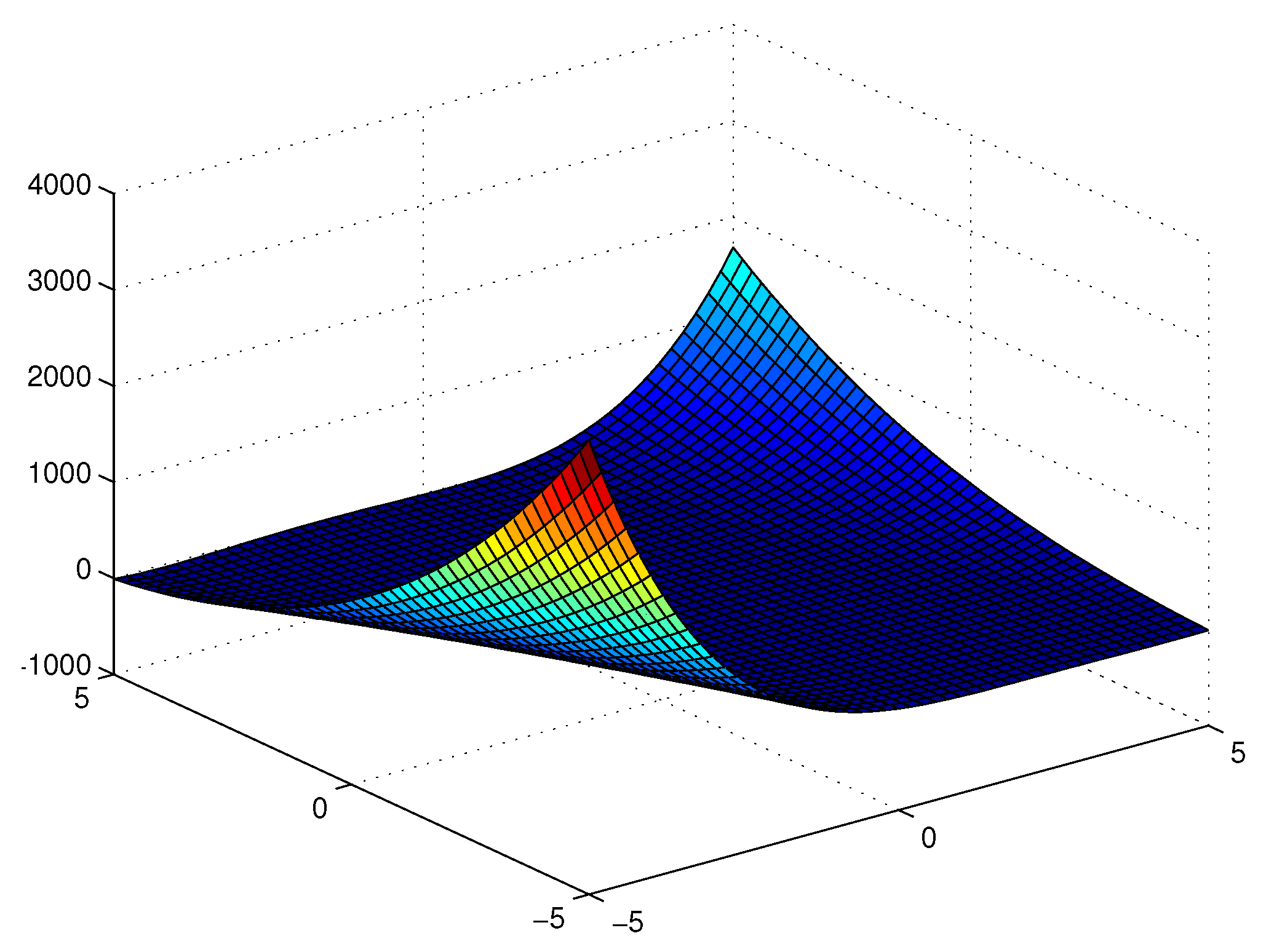
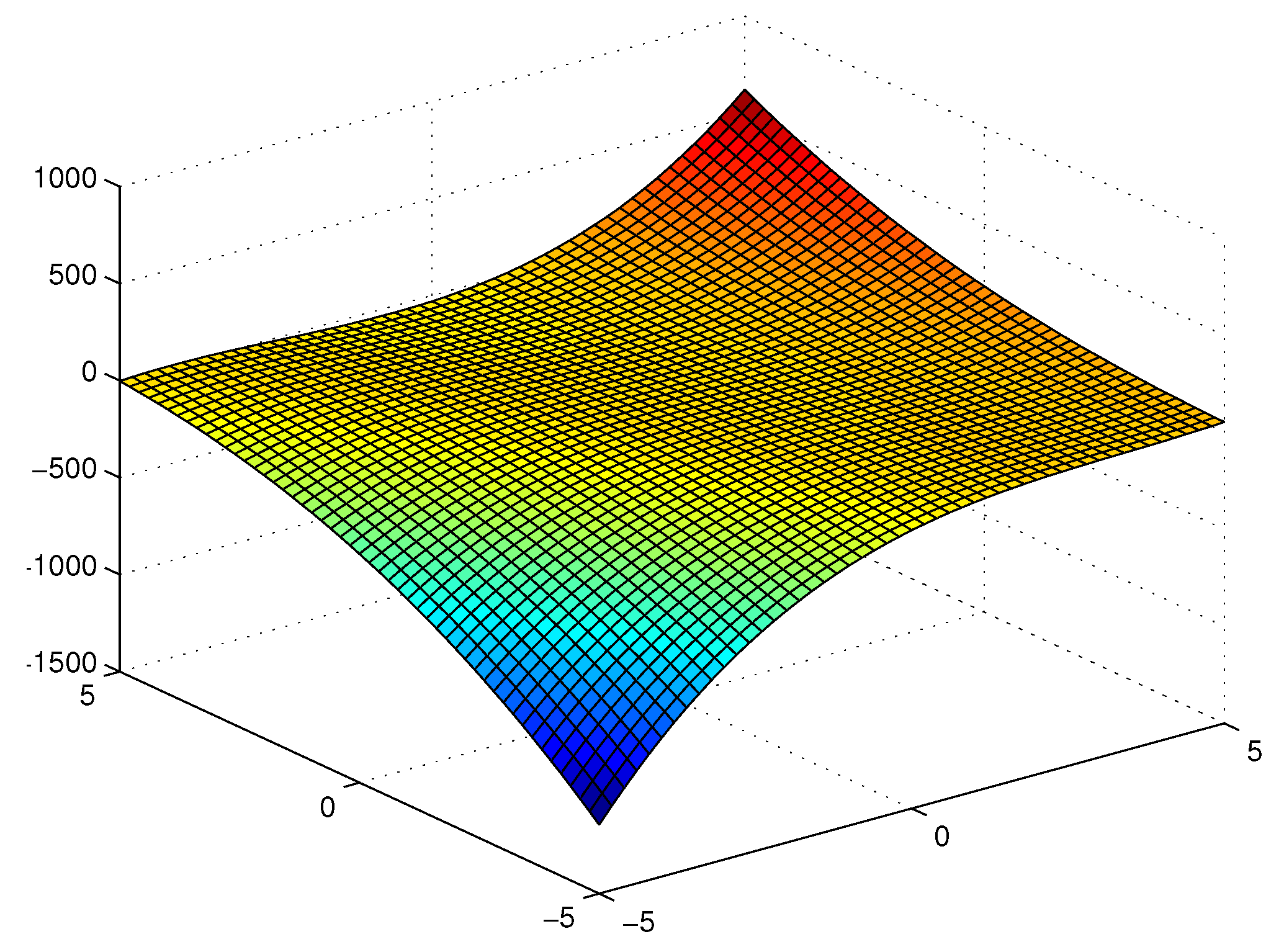
5. Graphical Representations and Surface Plots

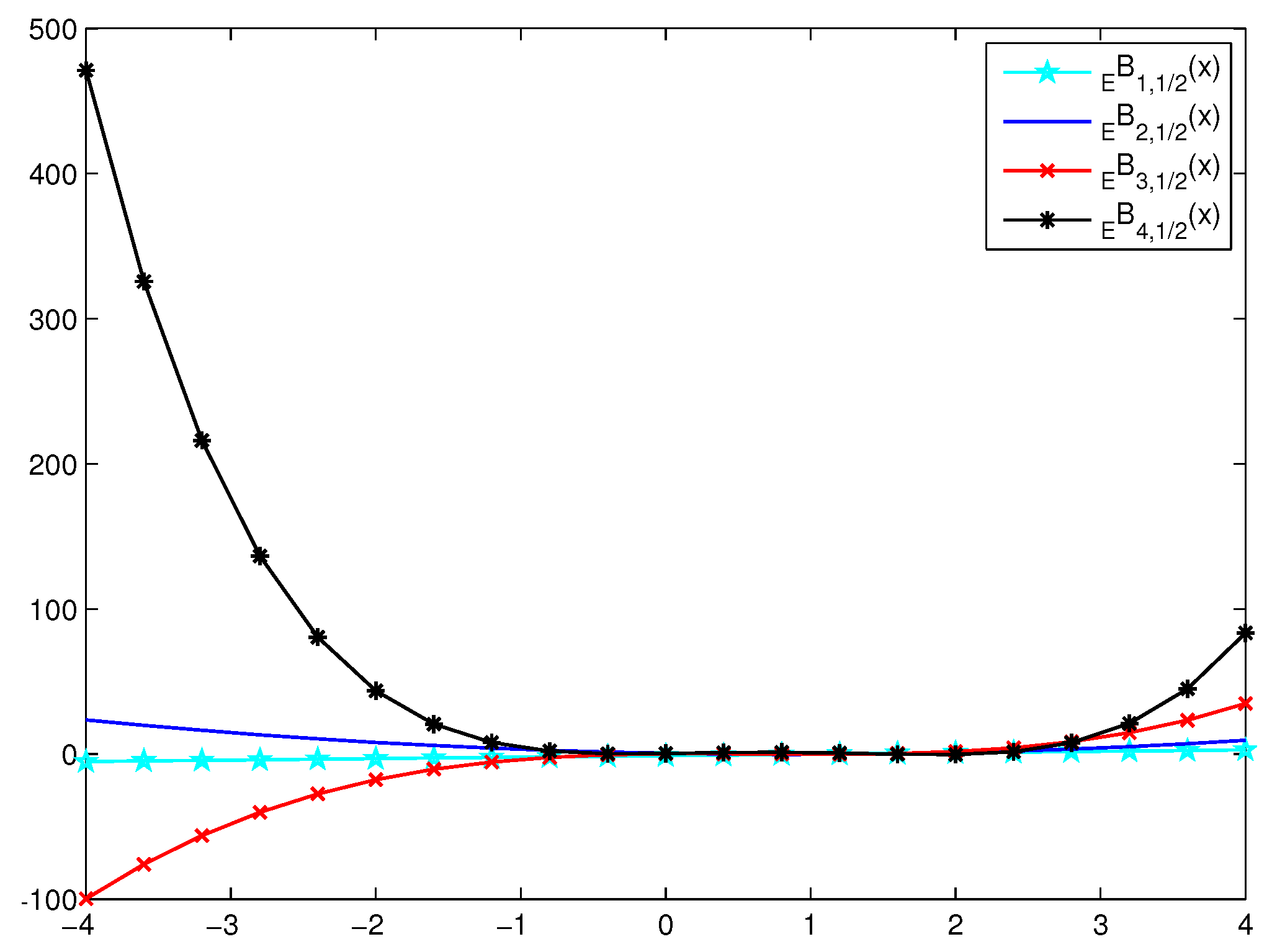
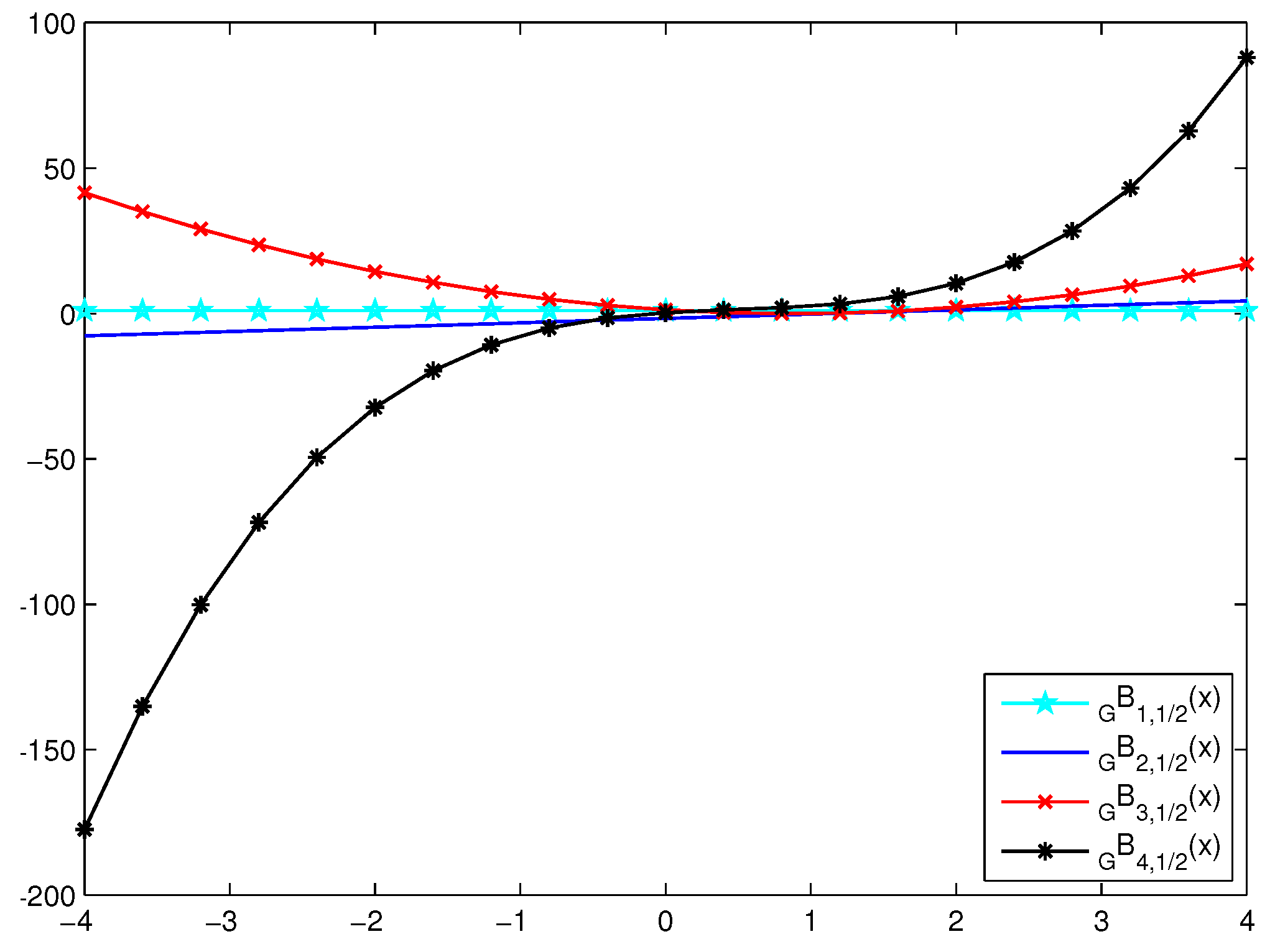
Here, in this section, the graphs of the q-Euler–Bernoulli polynomials (qEBP) ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x)$, q-Genocchi- Bernoulli polynomials (qGBP) ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x)$ and the surface plots of the 2DqEBP ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ and the 2DqGBP ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ are presented.

To draw the plot of the qEBP ${}_{\mathcal{E}}{\mathfrak{B}}_{m,q}(x)$ and the qGBP ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x)$, we choose $q=\frac{1}{2}$ and consider the values of the first four q-Euler–Bernoulli polynomials and of the first four q-Genocchi–Bernoulli polynomials, the expressions of these polynomials are given in Table 1.

Table 1. Expressions of the first four ${}_{\mathcal{E}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$ and ${}_{\mathcal{G}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$.

m	0	1	2	3	3
${}_{\mathcal{E}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$	1	$x-\frac{7}{6}$	$x^2-\frac{7}{4}x+\frac{79}{168}$	$x^3-\frac{49}{24}{x}^2+\frac{79}{96}x+\frac{379}{2880}$	$x^4-\frac{35}{16}{x}^3+\frac{145}{192}{x}^2+\frac{379}{1536}x+0.0213$
${}_{\mathcal{G}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$	0	1	$\frac{3}{2}x-\frac{7}{4}$	$\frac{7}{4}{x}^2-\frac{49}{16}x+\frac{121}{96}$	$\frac{15}{8}{x}^3-\frac{45}{16}{x}^2+\frac{815}{256}x+\frac{379}{1536}$

Further, by setting $m=4$ and $q=\frac{1}{2}$ in the series definitions (72) and (79) of ${}_{\mathcal{E}}{\mathfrak{B}}_{m,\frac{1}{2}}(x_1,x_2)$ and ${}_{\mathcal{G}}{\mathfrak{B}}_{m,q}(x_1,x_2)$ and using the particular values of ${}_{\mathcal{E}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$ and ${}_{\mathcal{G}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$ from Table 1, we find that

$$\begin{array}{cc}\hfill {}_{\mathcal{E}}{\mathfrak{B}}_{4,\frac{1}{2}}(x_1,x_2)=& x_1^4-\frac{35}{16}{x}_1^3+\frac{145}{192}{x}_1^2+\frac{379}{1536}{x}_1+0.0213+\frac{15}{8}{x}_1^3{x}_2-\frac{245}{64}{x}_1^2{x}_2+\frac{395}{256}{x}_1{x}_2\hfill \\ & +\frac{379}{1536}{x}_2+\frac{35}{32}{x}_1^2{x}_2^2-\frac{245}{128}{x}_1{x}_2^2+\frac{395}{768}{x}_2^2+\frac{15}{64}{x}_1{x}_2^3-\frac{35}{128}{x}_2^3+\frac{1}{64}{x}_2^4\hfill \end{array}$$

(82)

and

$$\begin{array}{cc}\hfill {}_{\mathcal{G}}{\mathfrak{B}}_{3,\frac{1}{2}}(x_1,x_2)=\frac{15}{8}{x}^3-\frac{45}{16}{x}^2+\frac{815}{256}x+\frac{379}{1536}& +\frac{105}{32}{x}_1^2{x}_2-\frac{735}{128}{x}_1{x}_2-\frac{605}{256}{x}_2\hfill \\ & +\frac{105}{64}{x}_1{x}_2^2-\frac{245}{128}{x}_2^2+\frac{15}{64}{x}_2^3.\hfill \end{array}$$

(83)

Next, by using the expression given in Table 1 and the Equations (82) and (83), with the help of Matlab, we get the Figure 1, Figure 2, Figure 3 and Figure 4 below.

Figure 1. Shape of ${}_{\mathcal{E}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$.

Figure 2. Shape of ${}_{\mathcal{G}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$.

Figure 3. Surface plot of ${}_{\mathcal{E}}{\mathfrak{B}}_{4,\frac{1}{2}}(x_1,x_2)$.

Figure 4. Surface plot of ${}_{\mathcal{G}}{\mathfrak{B}}_{4,\frac{1}{2}}(x_1,x_2)$.

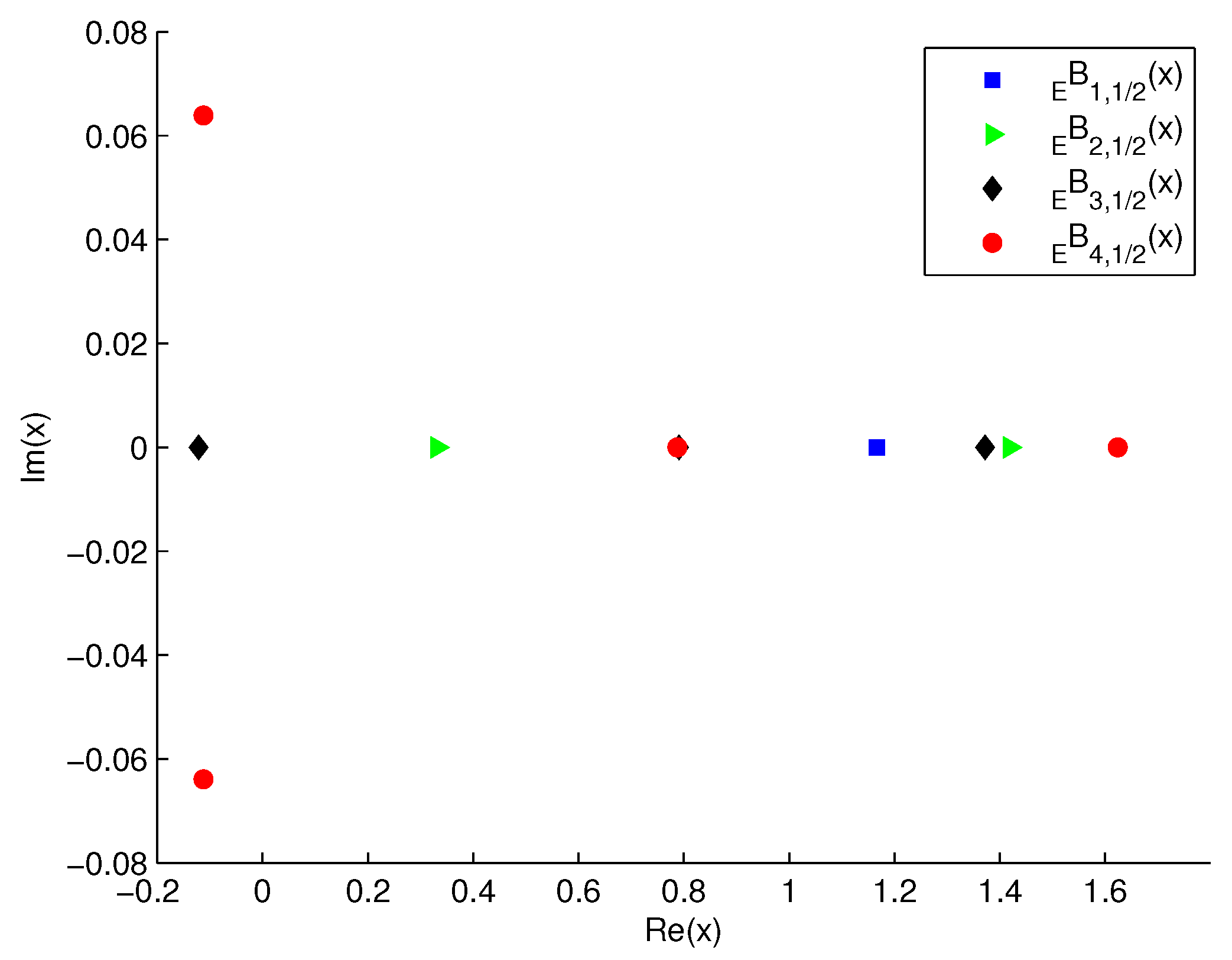
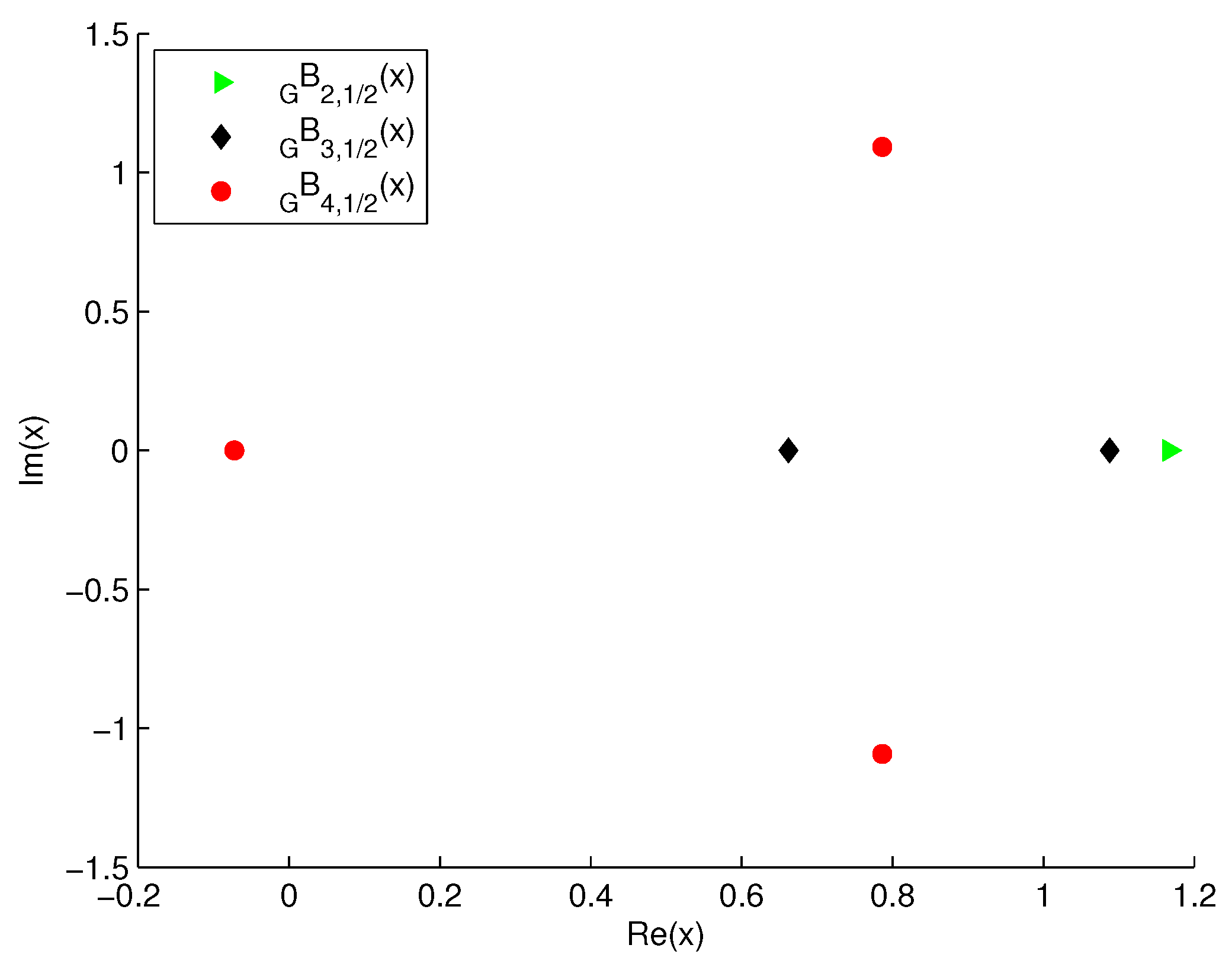
Further, with the help of Matlab, we compute the real and complex zeros of ${}_{\mathcal{E}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$ and ${}_{\mathcal{G}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$ for $m=1,2,3,4$ and $x\in \mathbb{C}$. These zeros are mentioned in Table 2 and Table 3.

Table 2. Real zeros of ${}_{\mathcal{E}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$ and ${}_{\mathcal{G}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$.

m	${}_{\mathcal{E}}{\mathfrak{B}}_{\mathit{m},\frac{1}{2}}\left(\mathit{x}\right)$	${}_{\mathcal{G}}{\mathfrak{B}}_{\mathit{m},\frac{1}{2}}\left(\mathit{x}\right)$
1	$1.1667$	0
2	$0.3315, 1.4185$	$1.1667$
3	$-0.1213, 0.7910, 1.3719$	$0.6620, 1.0880$
4	$0.7878,1.6239$	$-0.0726$

Table 3. Complex zeros of ${}_{\mathcal{E}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$ and ${}_{\mathcal{G}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$.

m	${}_{\mathcal{E}}{\mathfrak{B}}_{\mathit{m},\frac{1}{2}}\left(\mathit{x}\right)$	${}_{\mathcal{G}}{\mathfrak{B}}_{\mathit{m},\frac{1}{2}}\left(\mathit{x}\right)$
1	−	−
2	−	−
3	−	−
4	$-0.1121-0.0639i,-0.1121+0.0639i$	$0.7863-1.0926i,0.7863+1.0926i$

Also, with the help of Matlab, the zeros mentioned in Table 2 and Table 3 are shown in the Figure 5 and Figure 6.

Figure 5. Zeros of ${}_{\mathcal{E}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$.

Figure 6. Zeros of ${}_{\mathcal{G}}{\mathfrak{B}}_{m,\frac{1}{2}}(x)$.

6. Concluding Remarks and Observations

As long ago as 1910, Jackson [27] studied the q-definite integral of an arbitrary function $f(t)$, which is defined as follows:

$${\int }_0^{a}f(t)d_{q}t=(1-q)a\sum _{m=0}^{\infty }{q}^{m}f(a{q}^m)(0<q<1;a\in \mathbb{R})$$

(84)

and

$${\int }_a^{b}f(t)d_{q}t={\int }_0^{b}f(t)d_{q}t-{\int }_0^{a}f(t)d_{q}t.$$

(85)

We note also that

$$D_q{\int }_0^{t}f(x)d_{q}x=f(t).$$

(86)

Applying the double q-integral to both sides of the Equation (42), that is,

$${\int }_0^{x_1}{\int }_0^{x_2}{[m]}_q{\mathcal{A}}_{m-1,q}^{[2]}(t_1,q{t}_2)d_q{t}_1{d}_q{t}_2={\int }_0^{x_1}{\int }_0^{x_2}{D}_{q,t_2}{\mathcal{A}}_{m,q}^{[2]}(t_1,t_2)d_q{t}_1{d}_q{t}_2,$$

(87)

we have

$${[m]}_q{\int }_0^{x_1}{\int }_0^{x_2}{\mathcal{A}}_{m-1,q}^{[2]}(t_1,q{t}_2)d_q{t}_1{d}_q{t}_2={\int }_0^{x_1}\left({\mathcal{A}}_{m,q}^{[2]}(t_1,x_2)-{\mathcal{A}}_{m,q}^{[2]}(t_1,0)\right)d_q{t}_1.$$

(88)

In view of the Equation (41), the above Equation (88) yields

$$\begin{array}{c}\hspace{1em}{[m]}_q{\int }_0^{x_1}{\int }_0^{x_2}{\mathcal{A}}_{m-1,q}^{[2]}(t_1,q{t}_2)d_q{t}_1{d}_q{t}_2\hfill \\ \hspace{1em} ={\int }_0^{x_1}\frac{1}{{[m+1]}_q}\left(D_{q,t_1}{\mathcal{A}}_{m+1,q}^{[2]}(t_1,x_2)-D_{q,t_1}{\mathcal{A}}_{m+1,q}^{[2]}(t_1,0)\right)d_q{t}_1\hfill \\ =\frac{1}{{[m+1]}_q}\left({\mathcal{A}}_{m+1,q}^{[2]}(x_1,x_2)-{\mathcal{A}}_{m+1,q}^{[2]}(0,x_2)-{\mathcal{A}}_{m+1,q}^{[2]}(x_1,0)+{\mathcal{A}}_{m+1,q}^{[2]}(0,0)\right),\hfill \end{array}$$

(89)

which, on using the Equations (13) and (39), becomes

$$\begin{array}{c}{\int }_0^{x_1}{\int }_0^{x_2}{\mathcal{A}}_{m,q}^{[2]}(t_1,q{t}_2)d_q{t}_1{d}_q{t}_2\\ =\frac{1}{{[m+1]}_q{[m+2]}_q}\left({\mathcal{A}}_{m+2,q}^{[2]}(x_1,x_2)-{(-1)}^m{\mathcal{A}}_{m+2,q}^{[2]}(x_1,-x_2)-{\mathcal{A}}_{m+2,q}^{[2]}(x_1)+{\mathcal{A}}_{m+2,q}^{[2]}\right).\end{array}$$

(90)

Further, in view of the Equations (31) and (34), the Equations (90) yields

$$\begin{array}{c}{\int }_0^{x_1}{\int }_0^{x_2}{\mathcal{A}}_{m,q}^{[2]}(t_1,q{t}_2)d_q{t}_1{d}_q{t}_2=\frac{1}{{[m+1]}_q{[m+2]}_q}\\ ·\sum _{s=0}^{m+2}{\left[\genfrac{}{}{0pt}{}{m+2}{s}\right]}_q{\dot{\mathcal{A}}}_{s,q}\left({\ddot{\mathcal{A}}}_{m+2-s,q}(x_1,x_2)-{(-1)}^m{\ddot{\mathcal{A}}}_{m+2-s,q}(x_1,x_2)-{\ddot{\mathcal{A}}}_{m+2-s,q}(x_1)+{\ddot{\mathcal{A}}}_{m+2-s,q}\right).\end{array}$$

(91)

In conclusion, we choose to reiterate the now well-understood fact that the results for the q-analogues, which we have considered in this article for $0<q<1$, can easily be translated into the corresponding results for the so-called $(p,q)$-analogues (with $0<q<p\leqq 1$) by applying some obviously trivial parametric and argument variations, the additional parameter p being redundant. In fact, the so-called $(p,q)$-number ${[n]}_{p,q}$ is given (for $0<q<p\leqq 1$) by (see also [28])

$$\begin{array}{cc}\hfill {[n]}_{p,q}& :=\left\{\begin{array}{cc}{\displaystyle \frac{p^n-q^n}{p-q}}\hfill & (n\in \{1,2,3,\cdots \})\hfill \\ 0\hfill & (n=0)\hfill \end{array}\right.\hfill \\ \hfill & =:p^{n-1}{[n]}_{\frac{q}{p}},\hfill \end{array}$$

(92)

where, for the classical q-number ${[n]}_q$, we have

$$\begin{array}{cc}\hfill {[n]}_q& :=\frac{1-q^n}{1-q}\hfill \\ \hfill & =p^{1-n}\left(\frac{p^n-{(pq)}^n}{p-(pq)}\right)\hfill \\ \hfill & =p^{1-n}{[n]}_{p,pq}.\hfill \end{array}$$

(93)

Consequently, any claimed extensions of most (including the present) investigations involving the classical q-calculus to the corresponding obviously straightforward investigations involving the $(p,q)$-calculus are truly inconsequential.

Further investigations along the lines presented in this paper, which are associated with the various recent generalizations and extensions of the Apostol type Bernoulli, Euler and Genocchi polynomials introduced by, for example, Srivastava et al. (see [29,30]) may be worthy of consideration by the targeted readers.