A Closed-Form Expression of the Instantaneous Rotational Lurch Index to Evaluate Its Numerical Approximation
Abstract
:1. Introduction
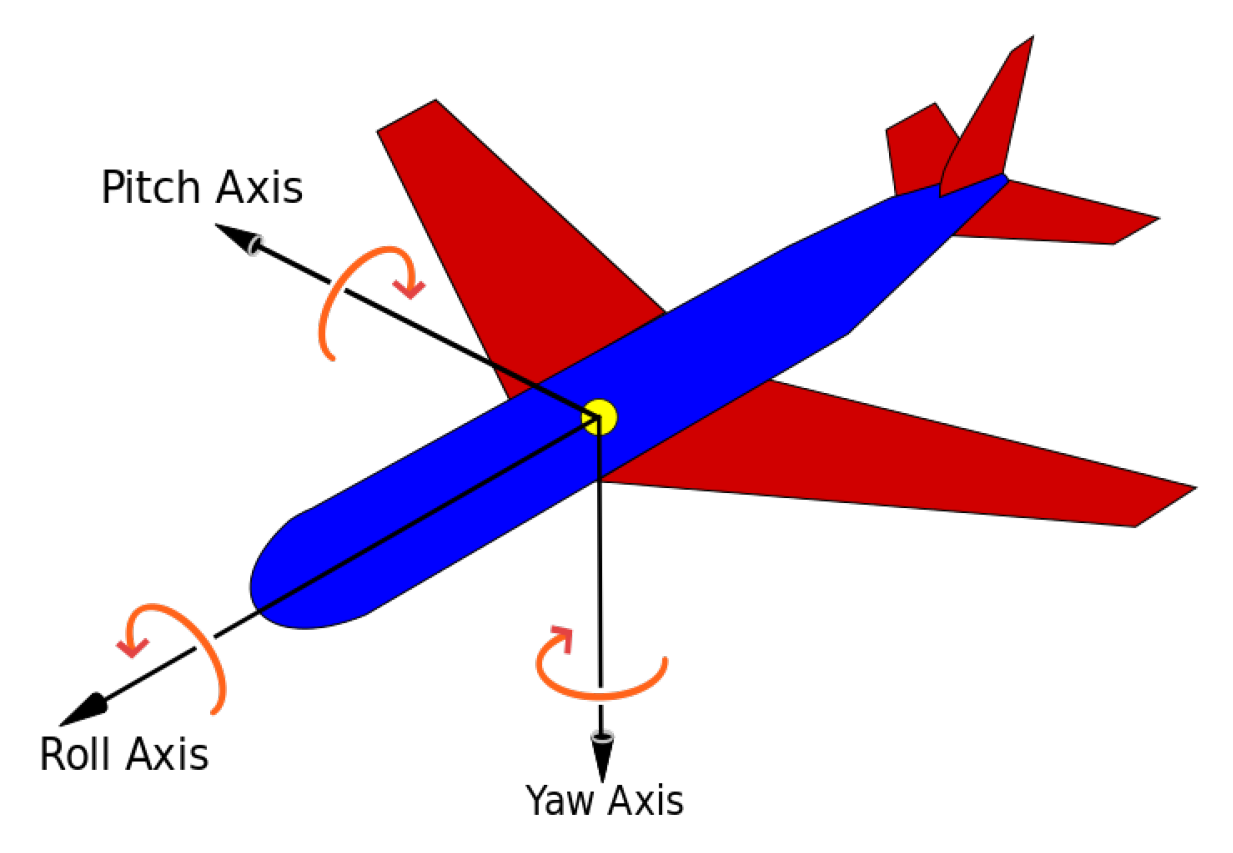
2. Rotational Lurch Index
2.1. Definition of Rotational Lurch Index
2.2. Relationship with Cartesian Lurch and Total Absolute Torsion
3. Closed-Form Expression of the Rotational Lurch Index
4. Numerical Evaluation of the Kinematic Indexes
4.1. Numerical Estimation Formulas
4.2. Remarks on the Precision of the Estimations
5. Numerical Tests
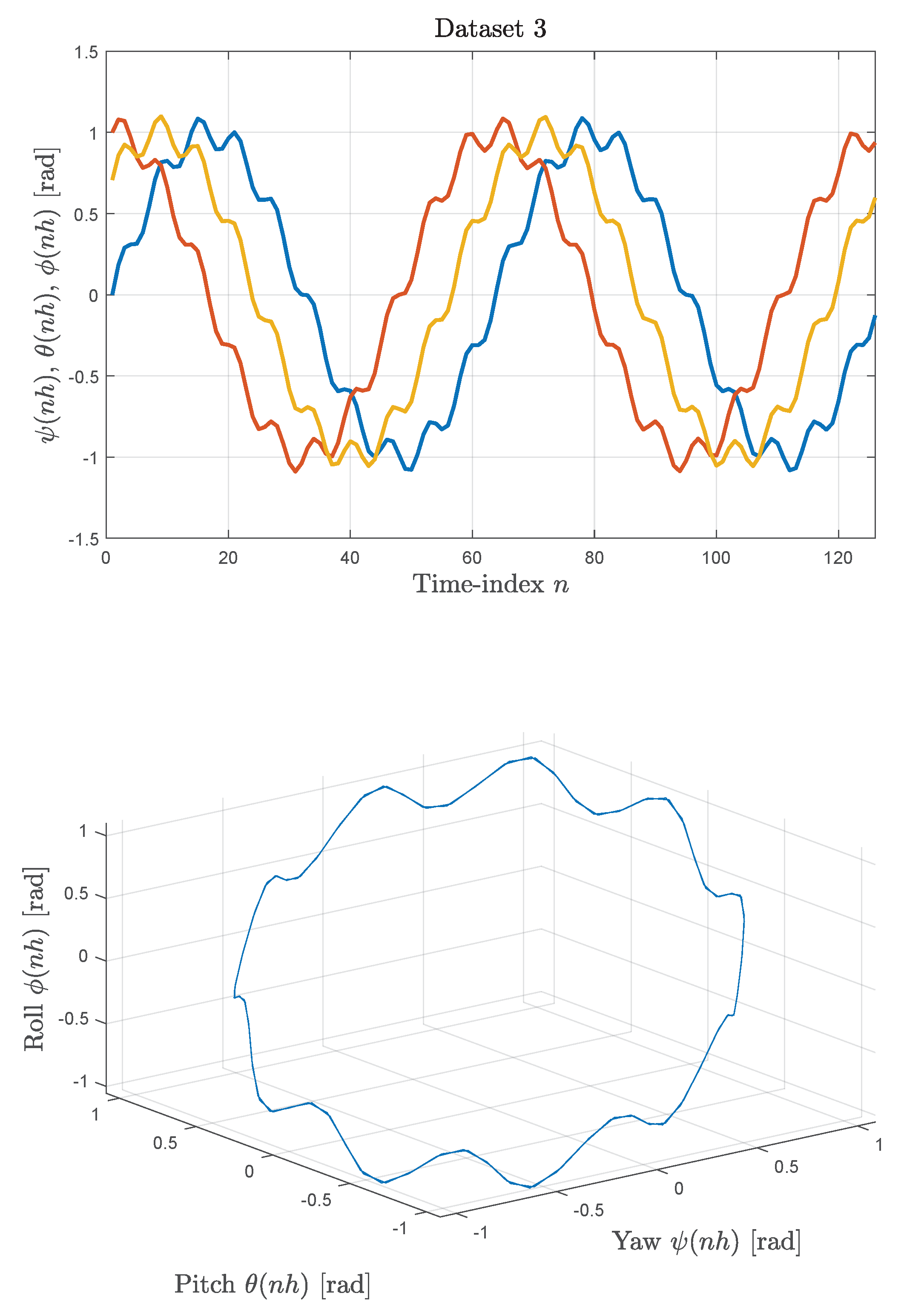
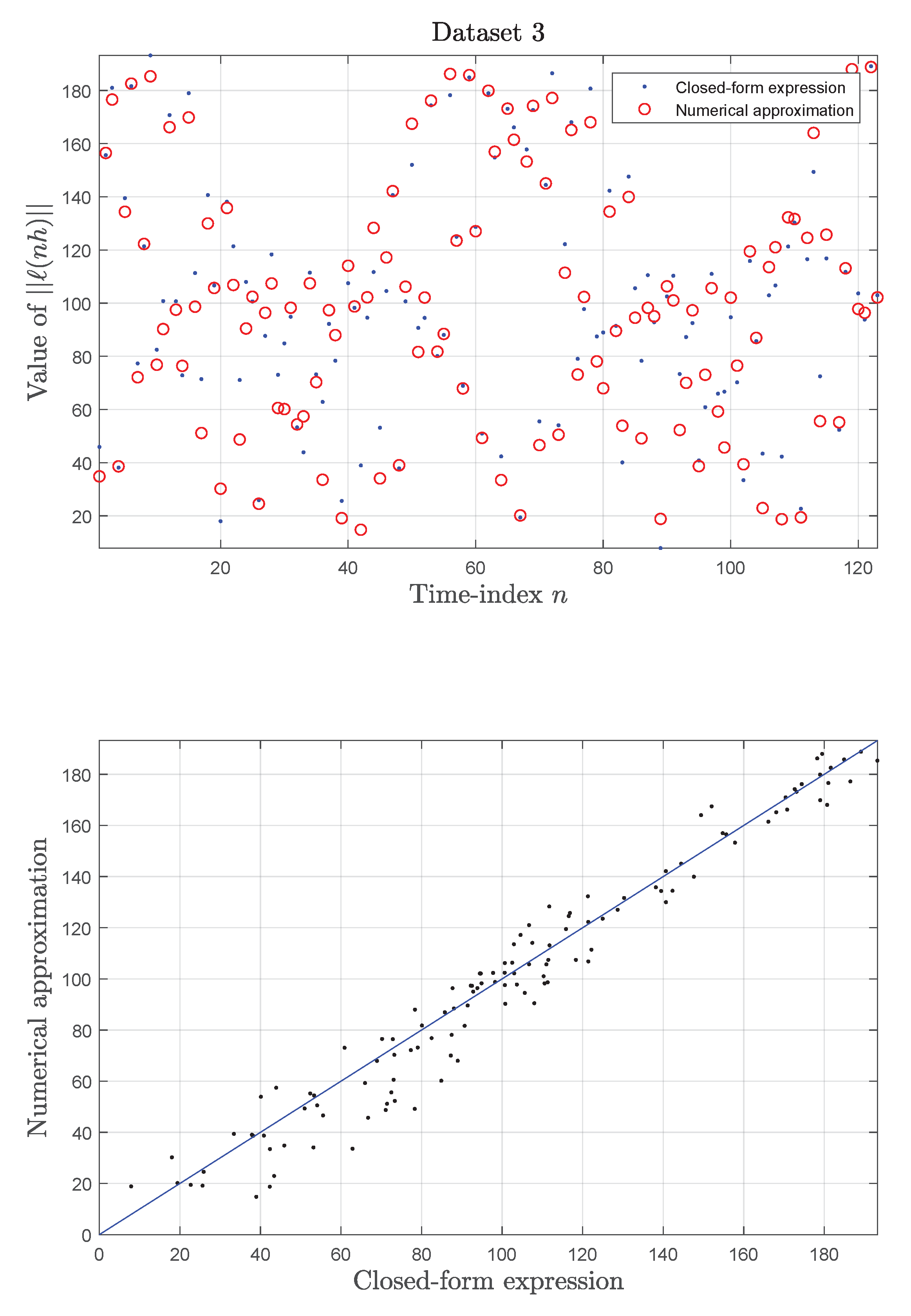
5.1. Numerical Test on Three Synthetic Data Sets
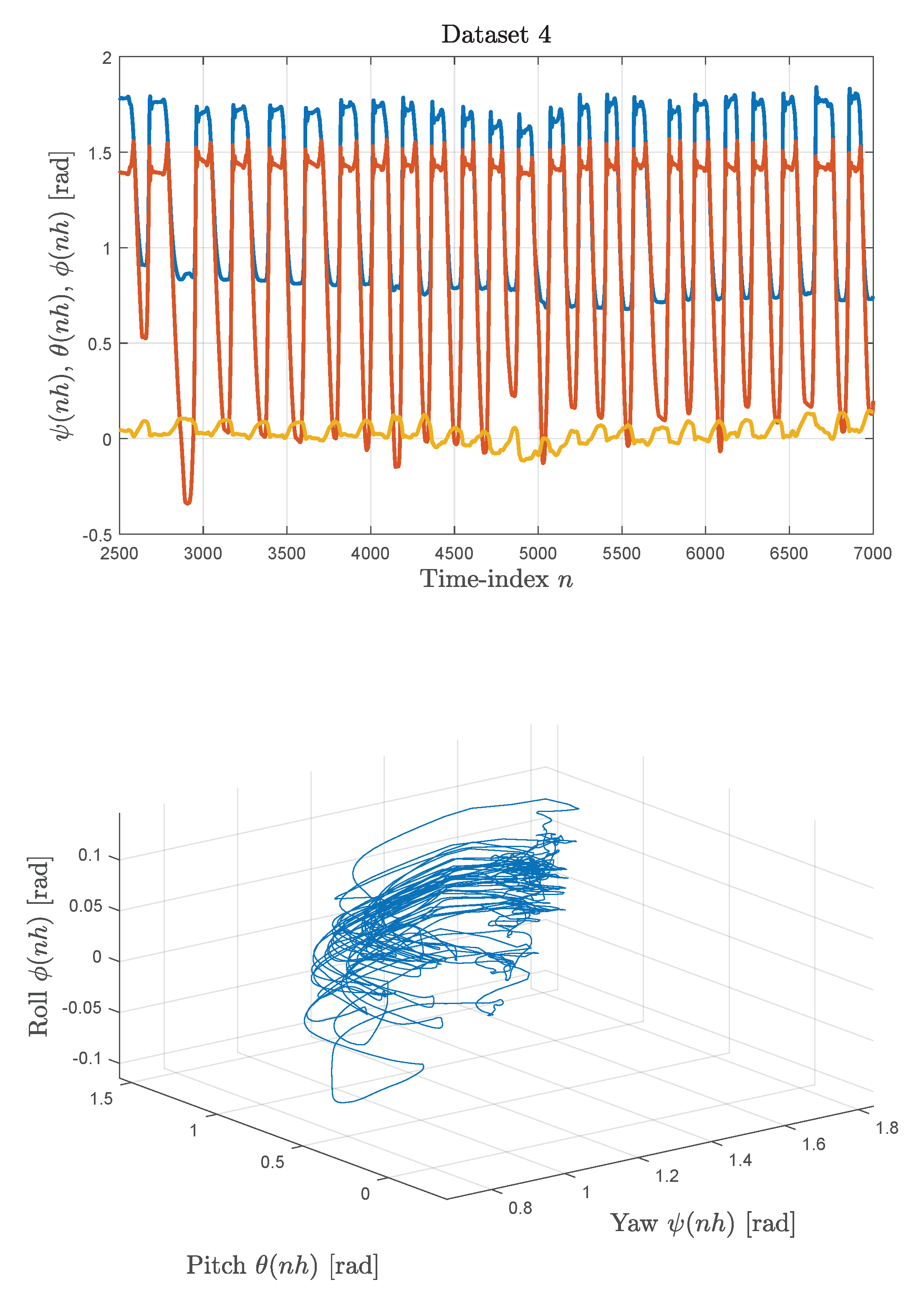
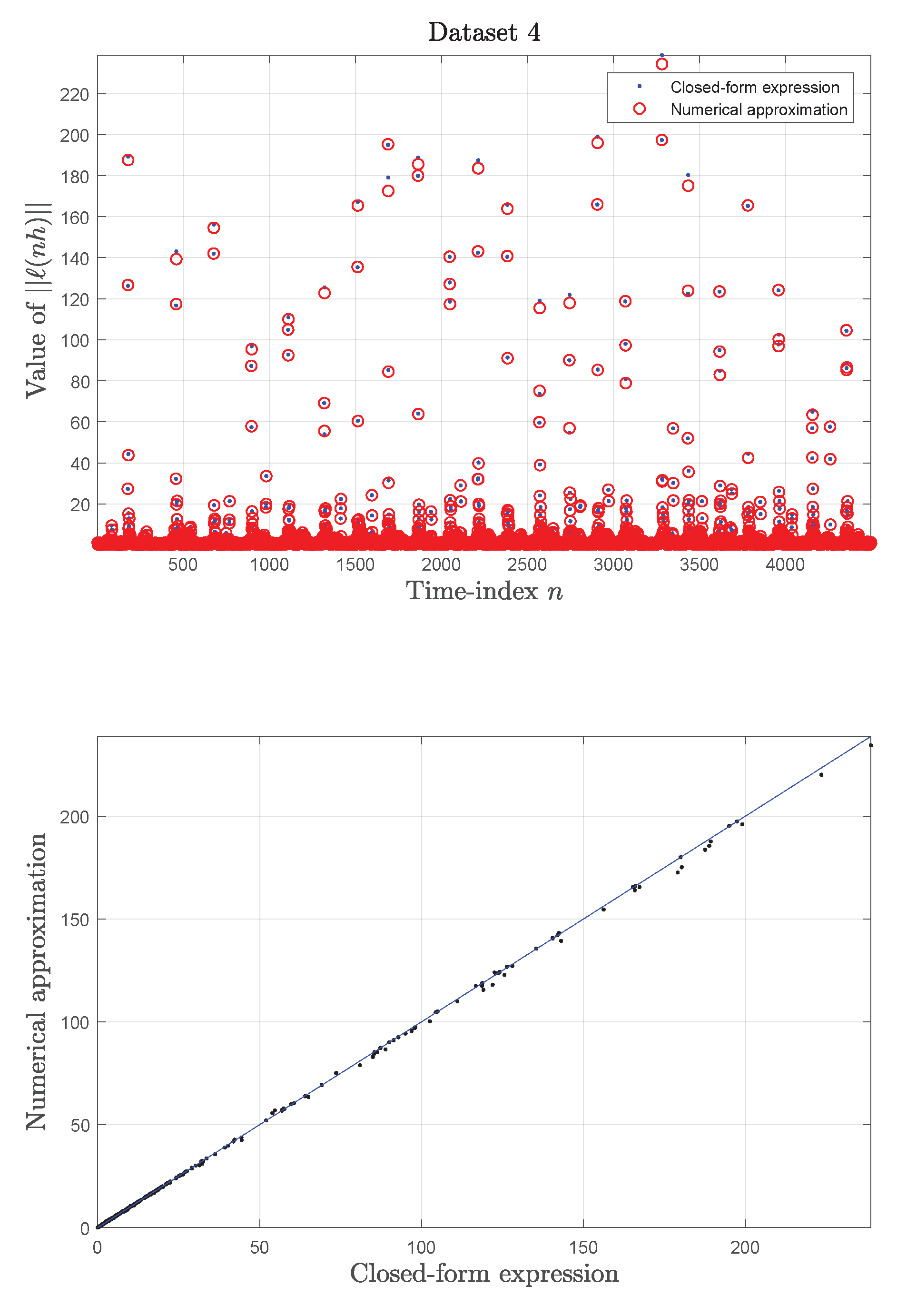
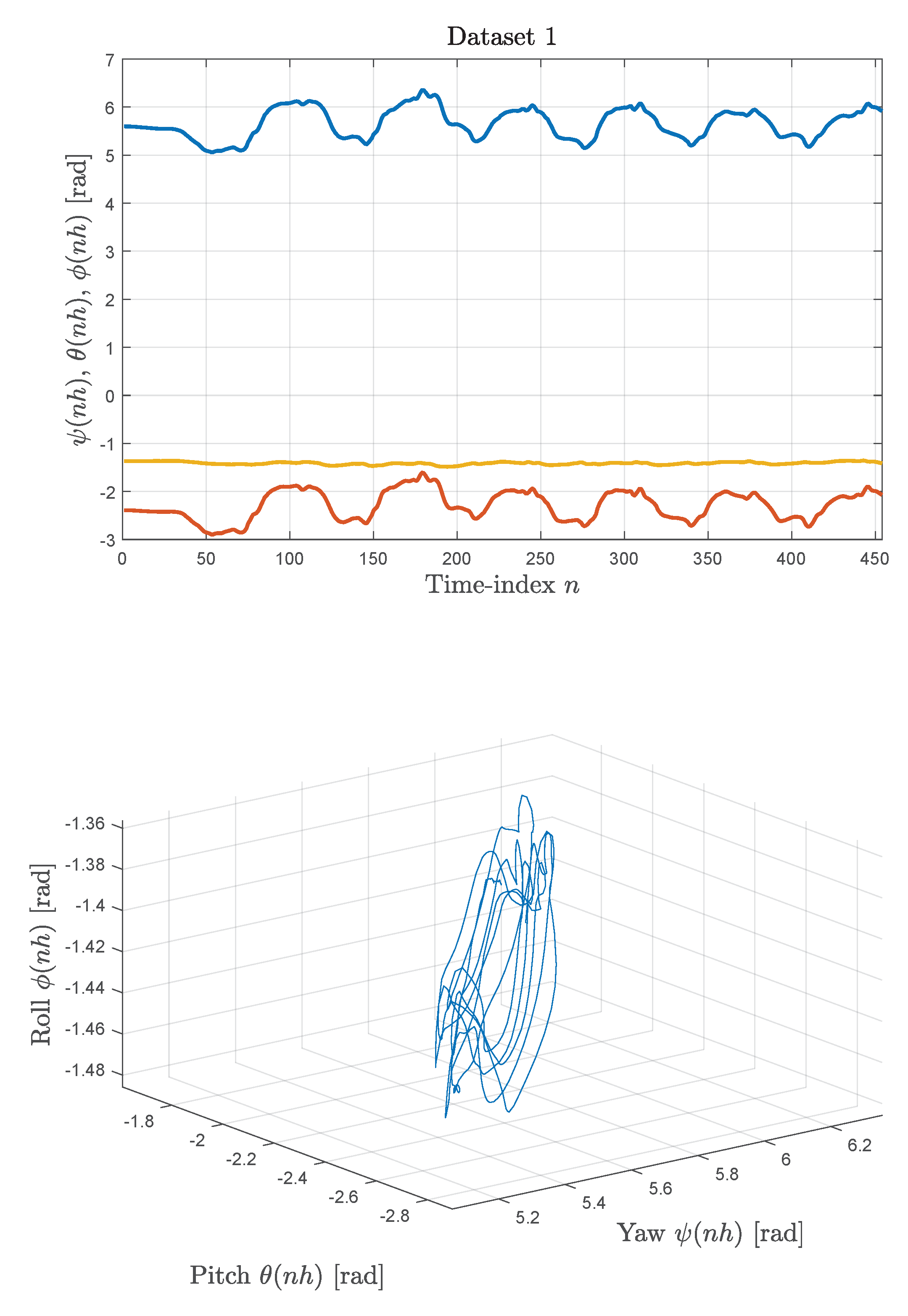
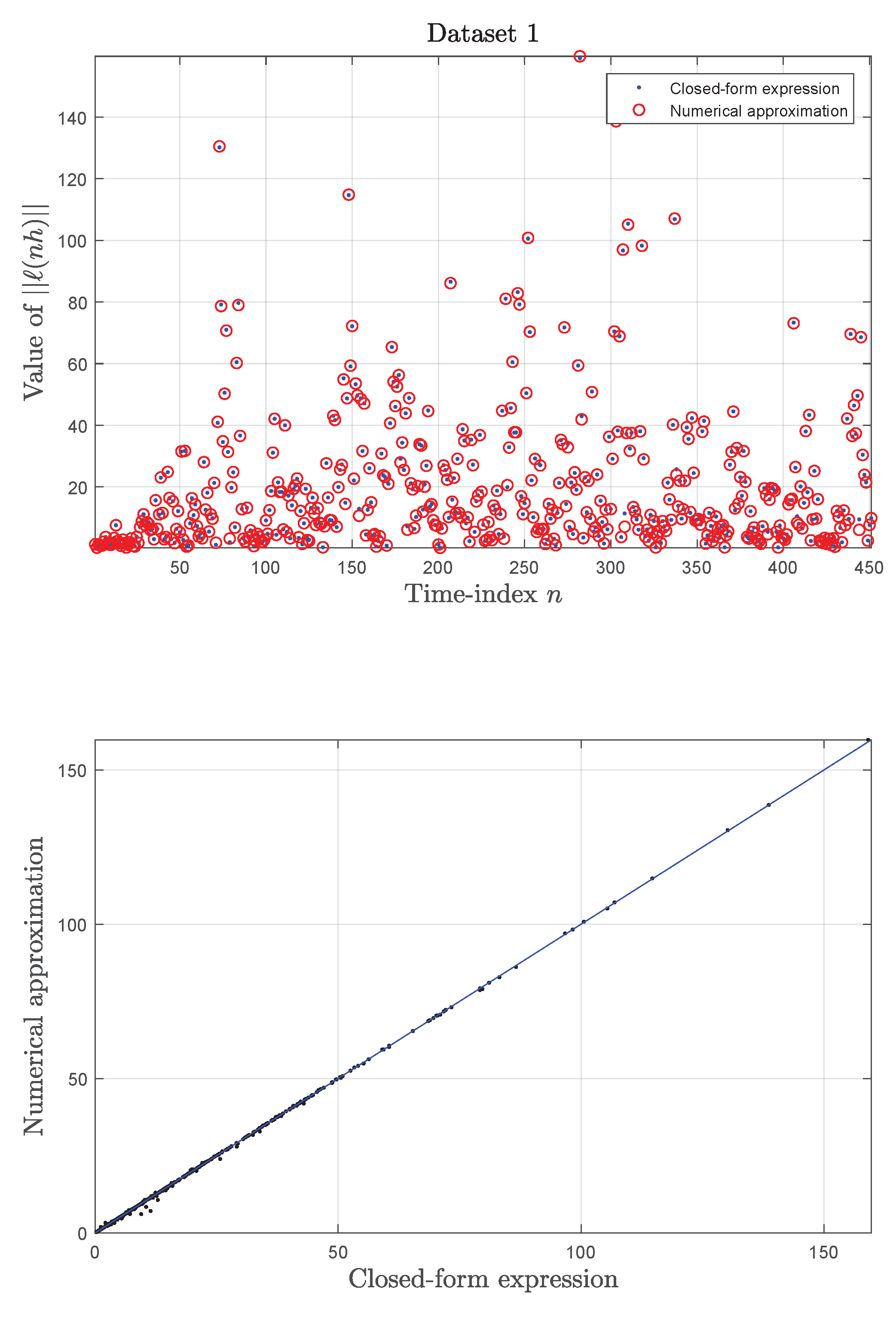
5.2. Numerical Test on Ten Data Sets Acquired by an Inertial Measurement Unit
5.3. Numerical Test on Three Data Sets Acquired by a Smartphone
6. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Gimbal Lock
Appendix B. General Problem of the Weighting of P+H, P−H, P+2h, P−2h
References
- McCarthy, J.M. Introduction to Theoretical Kinematics; MIT Press: Cambridge, MA, USA, 1990. [Google Scholar]
- Marion, J.B.; Thornton, S.T. Classical Dynamics of Systems and Particles, 4th ed.; Thomson Learning: Boston, MA, USA, 1995. [Google Scholar]
- Bearee, R. New damped-jerk trajectory for vibration reduction. Control Eng. Pract. 2014, 28, 112–120. [Google Scholar] [CrossRef]
- Canali, F.; Guarino Lo Bianco, C.; Locatelli, M. Minimum-jerk online planning by a mathematical programming approach. Eng. Optim. 2014, 46, 763–783. [Google Scholar] [CrossRef]
- Lu, Y.-S.; Shieh, R. A jerk-constrained time-optimal servo with disturbance compensation. Control Eng. Pract. 2014, 28, 49–57. [Google Scholar] [CrossRef]
- Mirzabeigy, A.; Yildirim, A. Approximate periodic solution for nonlinear jerk equation as a third-order nonlinear equation via modified differential transform method. Eng. Comput. 2014, 31, 622–633. [Google Scholar] [CrossRef]
- Choi, A.; Joo, S.-B.; Oh, E.; Mun, J.H. Kinematic evaluation of movement smoothness in golf: relationship between the normalized jerk cost of body joints and the clubhead. BioMed. Eng. OnLine 2014, 13, 20. [Google Scholar] [CrossRef] [PubMed]
- Civita, A.; Fiori, S.; Romani, G. A mobile acquisition system and a method for hips sway fluency assessment. Information 2018, 9, 321. [Google Scholar] [CrossRef]
- Fiori, S. Gyroscopic signals smoothness assessment by geometric jolt estimation. Math. Methods Appl. Sci. 2017, 40, 5893–5905. [Google Scholar] [CrossRef]
- Seifert, L.; Orth, D.; Boulanger, J.; Dovgalecs, V.; Hérault, R.; Davids, K. Climbing skill and complexity of climbing wall design: Assessment of jerk as a novel indicator of performance fluency. J. Appl. Biomech. 2014, 30, 619–625. [Google Scholar] [CrossRef] [PubMed]
- Lin, H.-I. A fast and unified method to find a minimum-jerk robot joint trajectory using particle swarm optimization. J. Intell. Robot. Syst. 2014, 75, 379–392. [Google Scholar] [CrossRef]
- Žefran, M.; Kumar, V. Planning of smooth motions on SE(3). In Proceedings of the 1996 IEEE International Conference on Robotics and Automation, Minneapolis, MN, USA, 22–28 April 1996; Volume 1, pp. 121–126. [Google Scholar]
- Scrivener, S.L.; Thompson, R.C. Survey of time-optimal attitude maneuvers. J. Guid. Control Dyn. 1994, 17, 225–233. [Google Scholar] [CrossRef]
- Spring, K.W. Euler parameters and the use of quaternion algebra in the manipulation of finite rotations: A review. Mech. Mach. Theory 1986, 21, 365–373. [Google Scholar] [CrossRef]
- Naidoo, Y.; Stopforth, R.; Bright, G. Quad-rotor unmanned aerial vehicle helicopter modelling & control. Int. J. Adv. Robot. Syst. 2011, 8, 139–149. [Google Scholar]
- Xueshan, Y.; Xiaozhai, Q.; Lee, G.C.; Tong, M.; Jinming, C. Jerk and jerk sensor. In Proceedings of the 14th World Conference on Earthquake Engineering, Beijing, China, 12–17 October 2008; Available online: http://www.iitk.ac.in/nicee/wcee/fourteenth_conf_china/ (accessed on 25 October 2015).
- Dubrovin, B.; Novikov, S.; Fomenko, A. Modern Geometry—Methods and Applications (Part I: The Geometry of Surfaces, Transformation Groups, and Fields), Graduate Texts in Mathematics, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 1991; ISBN 0387976639. [Google Scholar]
- Fiori, S.; Prifti, S. Exact low-order polynomial expressions to compute the Kolmogoroff-Nagumo mean in the affine symplectic group of optical transference matrices. Linear Multilinear Algebr. 2017, 65, 840–856. [Google Scholar] [CrossRef]
- Hardy, G.H.; Wright, E.M. An Introduction to the Theory of Numbers, 5th ed.; Clarendon Press: Oxford, UK, 1979; pp. 7–8. [Google Scholar]
- Stoer, J.; Bulirsch, R. Introduction to Numerical Analysis; Springer: New York, NY, USA, 1980. [Google Scholar]
- Eager, D.; Pendrill, A.-M.; Reistad, N. Beyond velocity and acceleration: jerk, snap and higher derivatives. Eur. J. Phys. 2016, 37, 065008. [Google Scholar] [CrossRef]
- InvenSense Inc. MPU-6000 and MPU-6050 Product Specification, Revision 3.4; InvenSense Inc.: San Jose, CA, USA, 2013. [Google Scholar]
- Vosinakis, S.; Gardeli, A. On the use of mobile devices as controllers for first-person navigation in public installations. Information 2019, 10, 238. [Google Scholar] [CrossRef]
- Josiński, H.; Michalczuk, A.; Świtoński, A.; Mucha, R.; Wojciechowski, K. Quantifying chaotic behavior in treadmill walking. In Intelligent Information and Database Systems, Proceedings of the 2015 Asian Conference on Intelligent Information and Database Systems, Bali, Indonesia, 23–25 March 2015; Lecture Notes in Computer Science Book Series (LNCS); Springer: Cham, Switzerland, 2015; Volume 9012, pp. 317–326. [Google Scholar]
- Vakanski, A.; Jun, H.-P.; Paul, D.; Baker, R. A data set of human body movements for physical rehabilitation exercises. Data 2018, 3, 2. [Google Scholar] [CrossRef] [PubMed]
- Altman, S.L. Rotations, Quaternions, and Double Groups; Dover Publications: Mineola, NY, USA, 1986. [Google Scholar]
| Indices | |||||||
|---|---|---|---|---|---|---|---|
| Data | Data set 1 | 126 | 23.0 | 8900.0 | 8900.0 | 61,433.2 | 61,433.2 |
| Data set 2 | 126 | 14.3 | 33.1 | 33.0 | 366.3 | 366.1 | |
| Data set 3 | 126 | 18.1 | 1221.8 | 1254.4 | 10,740.0 | 11,027.4 | |
| Indices | |||||||
|---|---|---|---|---|---|---|---|
| Data | Data set 1 | 7000 | 18.6 | 218.4 | 218.4 | 2379721.2 | 2379714.2 |
| Data set 2 | 7000 | 27.6 | 238.7 | 238.7 | 1,751,479.4 | 1,751,354.8 | |
| Data set 3 | 7000 | 41.5 | 642.7 | 642.8 | 3,136,393.9 | 3,136,772.0 | |
| Data set 4 | 7000 | 99.7 | 1553.9 | 1559.3 | 3,156,866.2 | 3,167,875.9 | |
| Data set 5 | 7000 | 2.8 | 175.0 | 175.0 | 12,685,692.6 | 12,685,684.0 | |
| Data set 6 | 7000 | 2.9 | 206.6 | 206.6 | 14,548,178.5 | 14,548,208.6 | |
| Data set 7 | 7000 | 4.1 | 214.5 | 214.5 | 10,640,476.9 | 10,640,417.8 | |
| Data set 8 | 7000 | 5.5 | 209.5 | 209.5 | 7,748,235.0 | 7,748,229.0 | |
| Data set 9 | 7000 | 6.6 | 218.7 | 218.7 | 6,710,767.1 | 6,710,600.4 | |
| Data set 10 | 7000 | 7.6 | 223.3 | 223.3 | 5,930,836.2 | 5,930,734.8 | |
| Indices | |||||||
|---|---|---|---|---|---|---|---|
| Data | Data set 1 | 454 | 16.5 | 886.1 | 888.3 | 110510.7 | 110792.8 |
| Data set 2 | 461 | 23.4 | 1881.1 | 1898.6 | 170,905.9 | 172,500.2 | |
| Data set 3 | 457 | 15.4 | 1555.4 | 1562.1 | 210,765.2 | 211,681.1 | |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fiori, S. A Closed-Form Expression of the Instantaneous Rotational Lurch Index to Evaluate Its Numerical Approximation. Symmetry 2019, 11, 1208. https://doi.org/10.3390/sym11101208
Fiori S. A Closed-Form Expression of the Instantaneous Rotational Lurch Index to Evaluate Its Numerical Approximation. Symmetry. 2019; 11(10):1208. https://doi.org/10.3390/sym11101208
Chicago/Turabian StyleFiori, Simone. 2019. "A Closed-Form Expression of the Instantaneous Rotational Lurch Index to Evaluate Its Numerical Approximation" Symmetry 11, no. 10: 1208. https://doi.org/10.3390/sym11101208
APA StyleFiori, S. (2019). A Closed-Form Expression of the Instantaneous Rotational Lurch Index to Evaluate Its Numerical Approximation. Symmetry, 11(10), 1208. https://doi.org/10.3390/sym11101208





