The Circular Hill Problem Regarding Arbitrary Disturbing Forces: The Periodic Solutions that are Emerging from the Equilibria
Abstract
:1. Introduction
2. Proof of Theorems 1 and 3
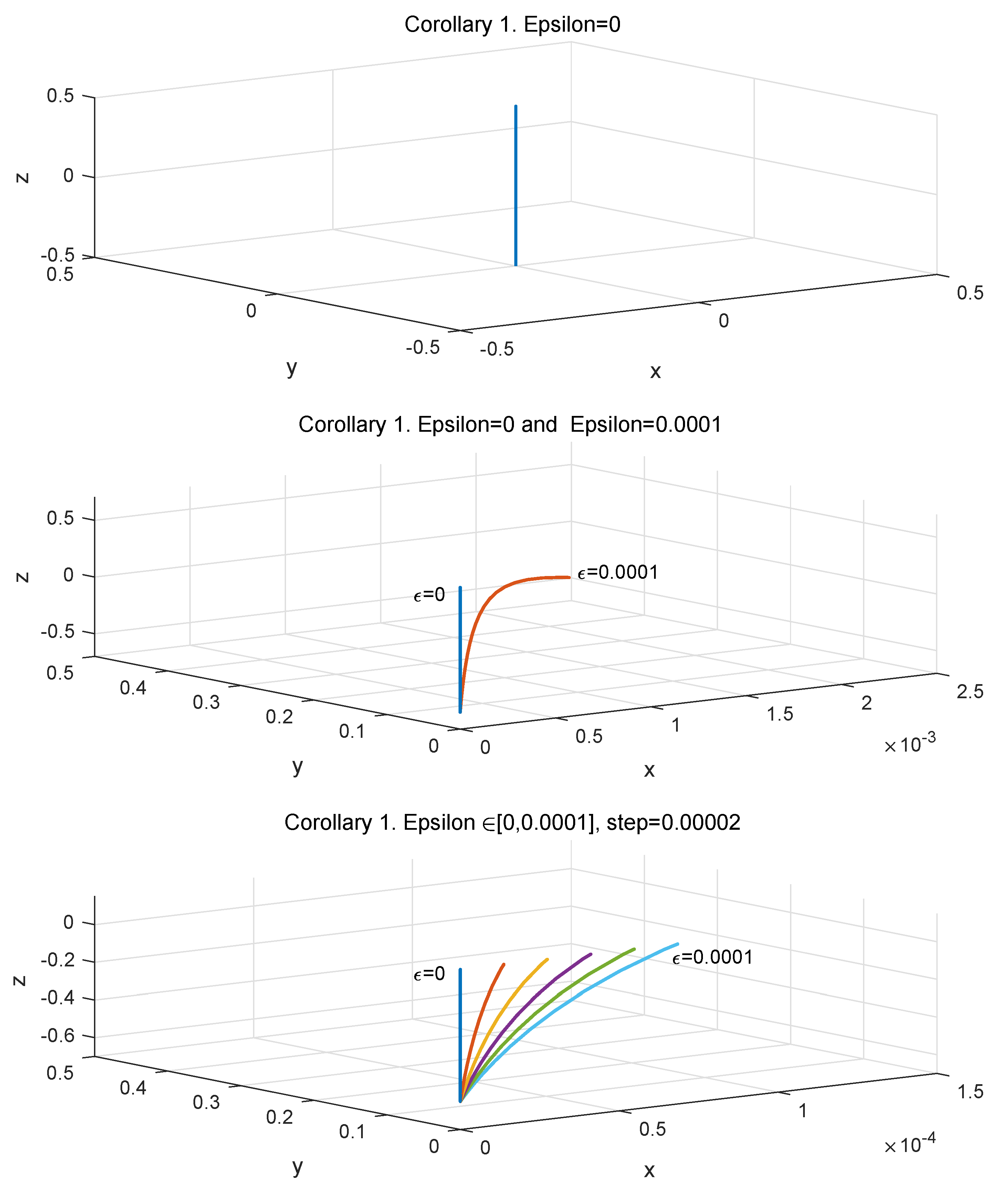
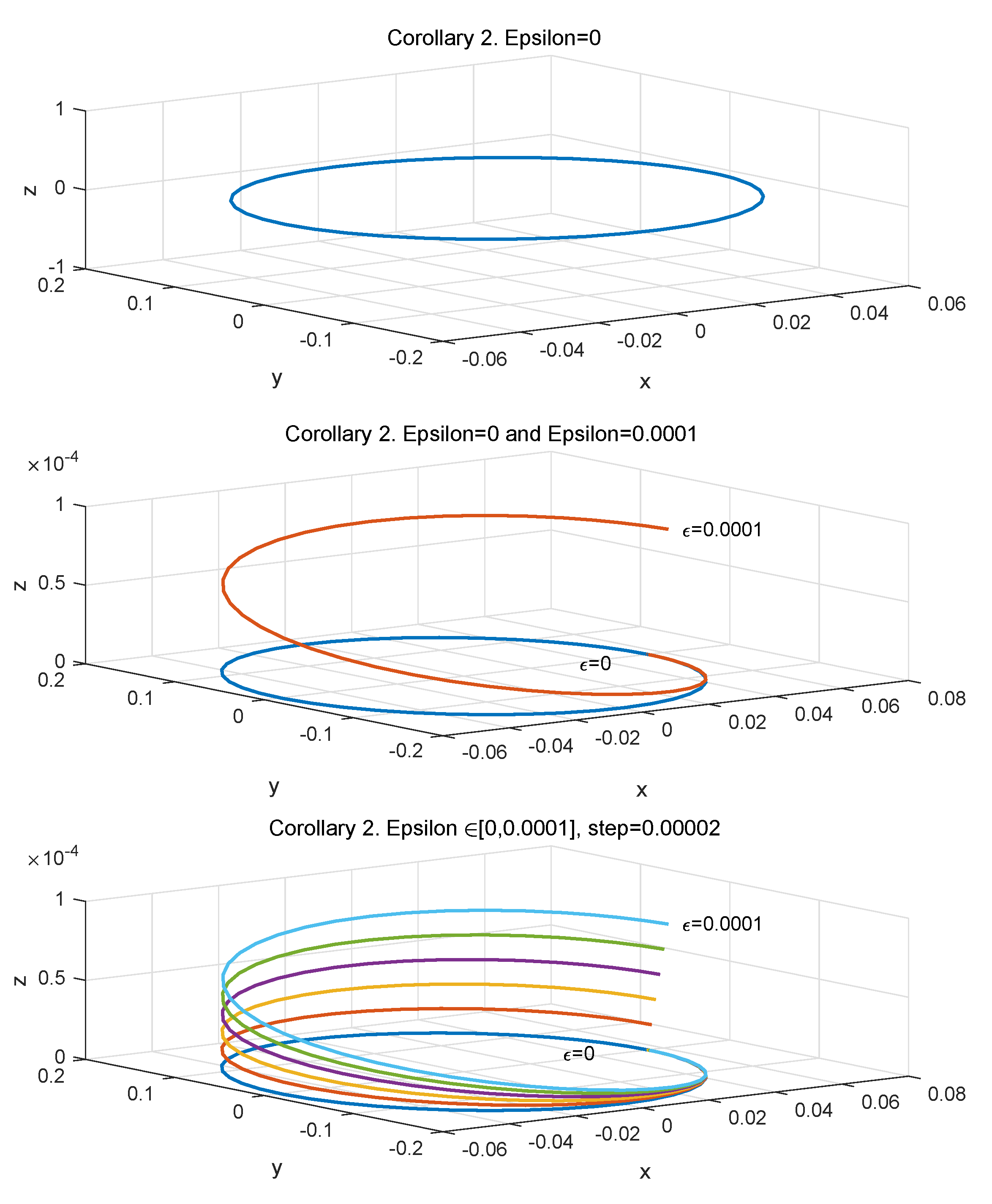
3. Proof of Corollaries 1 and 2
Author Contributions
Funding
Conflicts of Interest
Appendix A. Basic Results on Averaging Theory
References
- Abouelmagd, E.I.; Guirao, J.L.G. On the perturbed restricted three-body problem. Appl. Math. Nonlinear Sci. 2016, 1, 123–144. [Google Scholar] [CrossRef] [Green Version]
- de Bustos, M.T.; Guirao, J.L.G.; Vera, J.A. The spatial Hill Lunar problem: Periodic solutions emerging from equilibria. Dynam. Syst. 2017, 32, 340–353. [Google Scholar] [CrossRef]
- Chauvineau, B.; Mignard, F. Dynamics of Binary Asteroids. I. Hill’s Case. Icarus 1990, 83, 360–381. [Google Scholar] [CrossRef]
- Chauvineau, B. The generalized Hill problem. Case of an external field of force deriving from a central potential. Celest. Mech. Dyn. Astron. 1991, 51, 119–129. [Google Scholar] [CrossRef]
- Llibre, J. On the central configurations of the n-body problem. Appl. Math. Nonlinear Sci. 2017, 2, 509–518. [Google Scholar] [CrossRef]
- Markellos, V.V.; Roy, A.E.; Velgakis, M.J.; Kanavos, S.S. A Photogravitational Hill Problem and radiation effects on Hill Stability of Orbits. Astrophys. Space Sci. 2000, 271, 293–301. [Google Scholar] [CrossRef]
- Michalodimitrakis, M. Hill’s problem: Families of three dimentional periodic orbits (part I). Astrophys. Space Sci. 1980, 68, 253–268. [Google Scholar] [CrossRef]
- Papadakis, K.E. The Planar Photogravitational Hill Problem. Int. J. Bifur. Chaos Appl. Sci. Engrg. 2006, 16, 1809–1821. [Google Scholar] [CrossRef]
- Perdiou, A.E.; Markellos, V.V.; Douskos, C.N. The Hill Problem with oblate secondary: Numerical exploration. Earth Moon Planets 2005, 97, 127–145. [Google Scholar] [CrossRef]
- Pérez-Chavela, E.; Tamayo, C. Relative Equilibria in the 4-Vortex Problem Bifurcating from an Equilateral Triangle Configuration. Appl. Math. Nonlinear Sci. 2016, 1, 301–310. [Google Scholar] [CrossRef] [Green Version]
- Simó, C.; Stuchi, T.J. Central stable/unstable manifolds and the destruction of KAM tori in the planar Hill problem. Physics D 2000, 140, 1–32. [Google Scholar] [CrossRef]
- Malkin, I.G. Some Problems of the Theory of Nonlinear Oscillations; Gosudarstv. Izdat. Tehn.–Teor. Lit.: Moscow, Russia, 1956. [Google Scholar]
- Buica, A.; García, I. Periodic solutions of some perturbed symmetric Euler top. Topol. Methods Nonlinear Anal. 2010, 36, 91–100. [Google Scholar]
- de Bustos, M.T.; Guirao, J.L.G.; Vera, J.A.; Vigo-Aguiar, J. Periodic orbits and C1-integrability in the planar Stark-Zeeman problem. J. Math. Phys. 2012, 53, 082701. [Google Scholar] [CrossRef]
- de Bustos, M.T.; Guirao, J.L.G.; Llibre, J.; Vera, J.A. New families of periodic orbits for a galactic potential. Chaos Solitons Fractals 2016, 82, 97–102. [Google Scholar] [CrossRef]
- de Bustos, M.T.; López, M.A.; Martínez, R. On the periodic auto-oscillations of an electric circuit with periodic imperfections on its variables. Appl. Math. Inf. Sci. 2013, 7, 2105–2111. [Google Scholar] [CrossRef]
- de Bustos, M.T.; López, M.A.; Martínez, R. On the periodic solutions of a linear chain of three identical atoms. Nonlinear Dyn. 2014, 76, 893–903. [Google Scholar] [CrossRef]
- Llibre, J.; Rodrigues, A. On the periodic orbits of Hamiltonian systems. J. Math. Phys. 2010, 51, 042704. [Google Scholar] [CrossRef]
- Roseau, M. Vibrations Non Linéaires et Théorie de la Stabilité; Springer Tracts in Natural Philosophy; Springer: Berlin, Germany, 1966; Volume 8. [Google Scholar]
- Buica, A.; Francoise, J.P.; Llibre, J. Periodic solutions of nonlinear periodic differential systems with a small parameter. Commun. Pure Appl. Anal. 2007, 6, 103–111. [Google Scholar]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
de Bustos, M.T.; López, M.A.; Martínez, R. The Circular Hill Problem Regarding Arbitrary Disturbing Forces: The Periodic Solutions that are Emerging from the Equilibria. Symmetry 2019, 11, 1196. https://doi.org/10.3390/sym11101196
de Bustos MT, López MA, Martínez R. The Circular Hill Problem Regarding Arbitrary Disturbing Forces: The Periodic Solutions that are Emerging from the Equilibria. Symmetry. 2019; 11(10):1196. https://doi.org/10.3390/sym11101196
Chicago/Turabian Stylede Bustos, M. Teresa, Miguel A. López, and Raquel Martínez. 2019. "The Circular Hill Problem Regarding Arbitrary Disturbing Forces: The Periodic Solutions that are Emerging from the Equilibria" Symmetry 11, no. 10: 1196. https://doi.org/10.3390/sym11101196
APA Stylede Bustos, M. T., López, M. A., & Martínez, R. (2019). The Circular Hill Problem Regarding Arbitrary Disturbing Forces: The Periodic Solutions that are Emerging from the Equilibria. Symmetry, 11(10), 1196. https://doi.org/10.3390/sym11101196





