Fermion Scattering in a CPT-Even Lorentz Violation Quantum Electrodynamics
Abstract
1. Introduction
2. Aspects of the Photonic SME Minimal Sector
Photon Propagator and a Lorentz Violation Gauge Choice
3. The Pair Annihilation
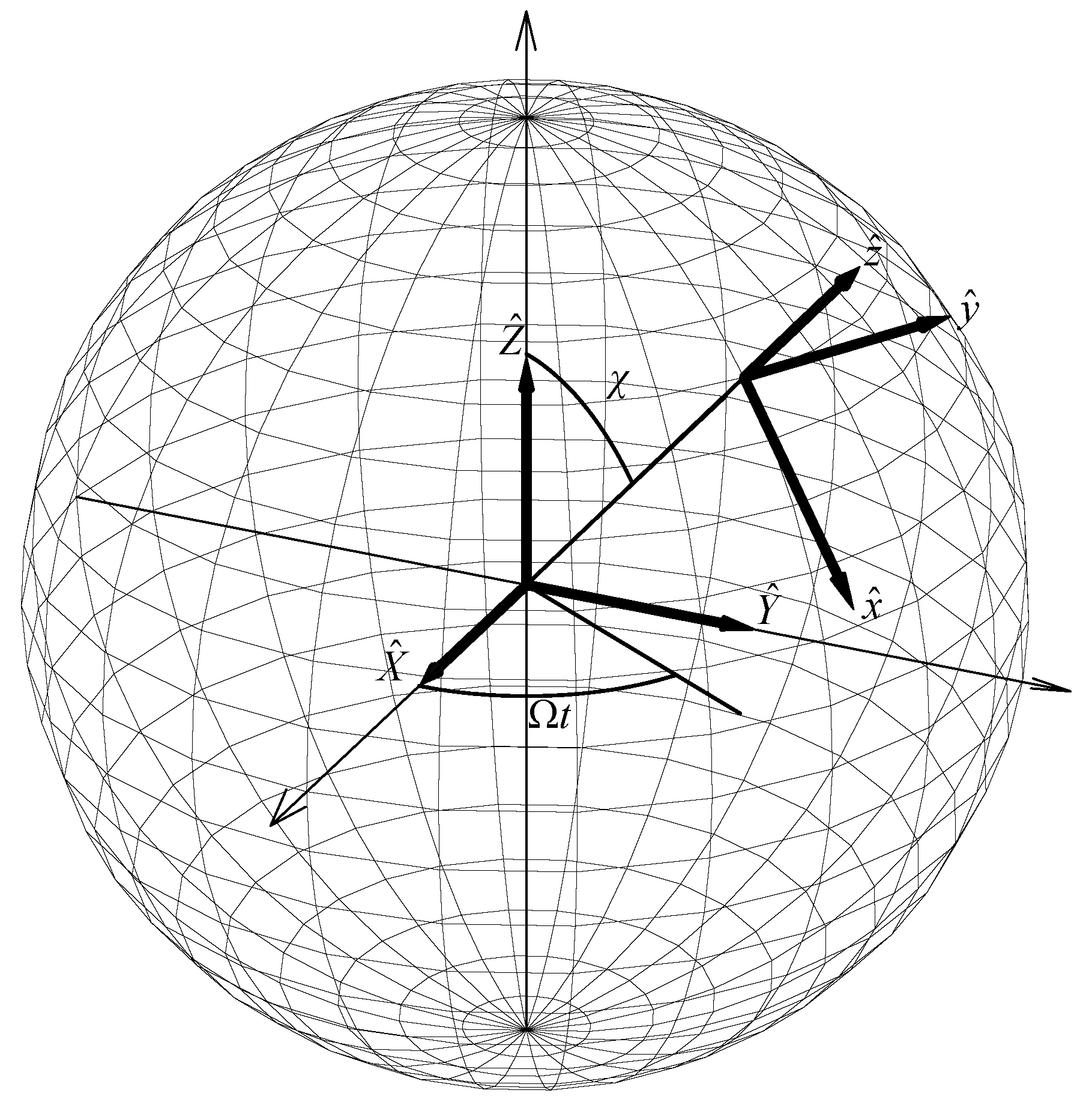
Sidereal Effects
4. The Scattering Process
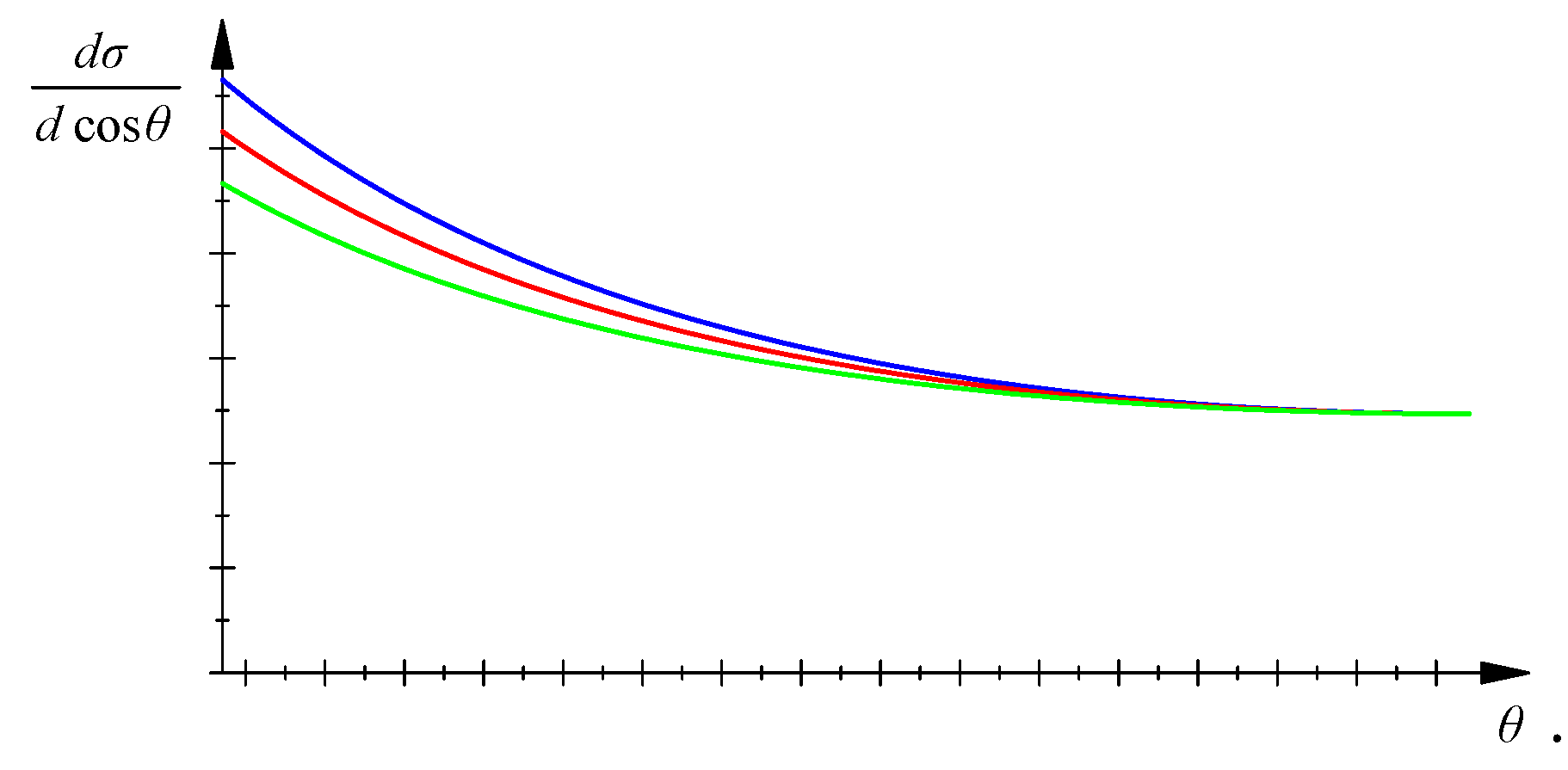
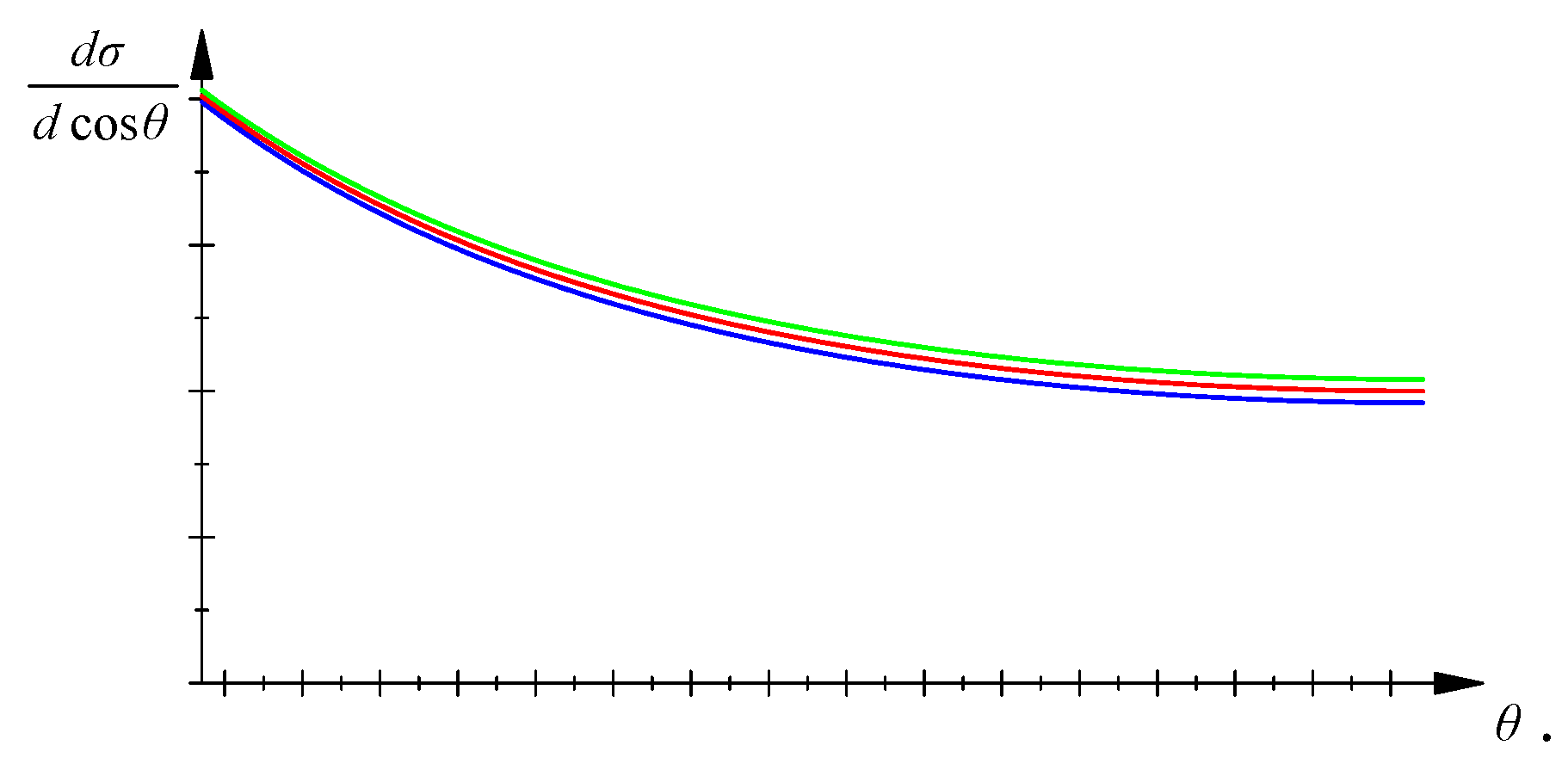
Mott Scattering
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Colladay, D.; Kostelecký, V.A. Lorentz-violating extension of the standard model. Phys. Rev. D 1998, 58, 116002. [Google Scholar] [CrossRef]
- Kosteleckỳ, V.A.; Russell, N. Data tables for Lorentz and C P T violation. Rev. Mod. Phys. 2011, 83, 11. [Google Scholar] [CrossRef]
- Carroll, S.M.; Field, G.B.; Jackiw, R. Limits on a Lorentz- and parity-violating modification of electrodynamics. Phys. Rev. D 1990, 41, 1231–1240. [Google Scholar] [CrossRef]
- Kostelecký, V.A.; Mewes, M. Cosmological Constraints on Lorentz Violation in Electrodynamics. Phys. Rev. Lett. 2001, 87, 251304. [Google Scholar] [CrossRef] [PubMed]
- Kostelecký, V.A.; Mewes, M. Signals for Lorentz violation in electrodynamics. Phys. Rev. D 2002, 66, 056005. [Google Scholar] [CrossRef]
- Kostelecký, V.A.; Mewes, M. Sensitive Polarimetric Search for Relativity Violations in Gamma-Ray Bursts. Phys. Rev. Lett. 2006, 97, 140401. [Google Scholar] [CrossRef] [PubMed]
- Kostelecký, V.A.; Mewes, M. Electrodynamics with Lorentz-violating operators of arbitrary dimension. Phys. Rev. D 2009, 80, 015020. [Google Scholar] [CrossRef]
- Escobar, C.A.; Garcia, M.A. Full C P T-even photon sector of the standard model extension at finite temperature. Phys. Rev. D 2015, 92, 025034. [Google Scholar] [CrossRef]
- Martín-Ruiz, A.; Escobar, C. Casimir effect between ponderable media as modeled by the standard model extension. Phys. Rev. D 2016, 94, 076010. [Google Scholar] [CrossRef]
- Andrianov, A.A.; Soldati, R. Lorentz symmetry breaking in Abelian vector-field models with Wess-Zumino interaction. Phys. Rev. D 1995, 51, 5961–5964. [Google Scholar] [CrossRef]
- Andrianov, A.A.; Soldati, R. Patterns of Lorentz symmetry breaking in QED by CPT-odd interaction. Phys. Lett. B 1998, 435, 449–452. [Google Scholar] [CrossRef]
- Andrianov, A.A.; Soldati, R.; Sorbo, L. Dynamical Lorentz symmetry breaking from a (3+1)-dimensional axion-Wess-Zumino model. Phys. Rev. D 1998, 59, 025002. [Google Scholar] [CrossRef]
- Alfaro, J.; Andrianov, A.; Cambiaso, M.; Giacconi, P.; Soldati, R. Bare and induced lorentz and cpt invariance violations in qed. Int. J. Mod. Phys. A 2010, 25, 3271–3306. [Google Scholar] [CrossRef]
- Zhukovsky, V.C.; Lobanov, A.E.; Murchikova, E.M. Radiative effects in the standard model extension. Phys. Rev. D 2006, 73, 065016. [Google Scholar] [CrossRef]
- Chen, W.F.; Kunstatter, G. Constraint from the Lamb shift and anomalous magnetic moment on radiatively induced Lorentz and CPT violation in quantum electrodynamics. Phys. Rev. D 2000, 62, 105029. [Google Scholar] [CrossRef]
- Adam, C.; Klinkhamer, F. Causality and CPT violation from an Abelian Chern–Simons-like term. Nucl. Phys. B 2001, 607, 247–267. [Google Scholar] [CrossRef]
- Adam, C.; Klinkhamer, F. Photon decay in a CPT-violating extension of quantum electrodynamics. Nucl. Phys. B 2003, 657, 214–228. [Google Scholar] [CrossRef]
- Baêta Scarpelli, A.P.; Belich, H.; Boldo, J.L.; Helayël-Neto, J.A. Aspects of causality and unitarity and comments on vortexlike configurations in an Abelian model with a Lorentz-breaking term. Phys. Rev. D 2003, 67, 085021. [Google Scholar] [CrossRef]
- Casana, R.; Ferreira, M.M.; Gomes, A.R.; dos Santos, F.E.P. Feynman propagator for the nonbirefringent CPT-even electrodynamics of the standard model extension. Phys. Rev. D 2010, 82, 125006. [Google Scholar] [CrossRef]
- Colladay, D.; McDonald, P.; Potting, R. Gupta-Bleuler photon quantization in the standard model extension. Phys. Rev. D 2014, 89, 085014. [Google Scholar] [CrossRef]
- Casana, R.; Ferreira, M., Jr.; dos Santos, F. Gupta-Bleuler quantization of the anisotropic parity-even and C P T-even electrodynamics of a standard model extension. Phys. Rev. D 2014, 90, 105025. [Google Scholar] [CrossRef]
- Casana, R.; Ferreira, M., Jr.; dos Santos, F. Gupta-Bleuler’s quantization of a parity-odd C P T-even electrodynamics of the standard model extension. Phys. Rev. D 2016, 94, 125011. [Google Scholar] [CrossRef]
- Belich, H.; Costa-Soares, T.; Ferreira, M.M., Jr.; Helayël-Neto, J. Non-minimal coupling to a Lorentz-violating background and topological implications. Eur. Phys. J. C 2005, 41, 421–426. [Google Scholar] [CrossRef]
- Belich, H.; Colatto, L.; Costa-Soares, T.; Helayël-Neto, J.; Orlando, M. Magnetic moment generation from non-minimal couplings in a scenario with Lorentz-symmetry violation. Eur. Phys. J. C 2009, 62, 425–432. [Google Scholar] [CrossRef]
- Bakke, K.; Belich, H. Abelian geometric phase for a Dirac neutral particle in a Lorentz. symmetry violation environment. J. Phys. G 2012, 39, 085001. [Google Scholar] [CrossRef]
- Bakke, K.; Belich, H.; Silva, E. Relativistic Landau-Aharonov-Casher quantization based on the Lorentz symmetry violation background. J. Math. Phys. 2011, 52, 063505. [Google Scholar] [CrossRef]
- Bakke, K.; Silva, E.; Belich, H. He–McKellar–Wilkens effect and scalar Aharonov–Bohm effect for a neutral particle based on the Lorentz symmetry violation. J. Phys. G 2012, 39, 055004. [Google Scholar] [CrossRef]
- Bakke, K.; Belich, H.; Silva, E. Relativistic Anandan quantum phase in the Lorentz violation background. Ann. Phys. (Berl.) 2011, 523, 910–918. [Google Scholar] [CrossRef]
- Anacleto, M.A. Lorentz violation correction to the Aharonov-Bohm scattering. Phys. Rev. D 2015, 92, 085035. [Google Scholar] [CrossRef]
- Bakke, K.; Belich, H. Relativistic Landau–He–McKellar–Wilkens quantization and relativistic bound states solutions for a Coulomb-like potential induced by the Lorentz symmetry breaking effects. Ann. Phys. 2013, 333, 272–281. [Google Scholar] [CrossRef]
- Ribeiro, L.; Furtado, C.; Passos, E. An analogy of the quantum hall conductivity in a Lorentz-symmetry violation setup. J. Phys. G 2012, 39, 105004. [Google Scholar] [CrossRef]
- Charneski, B.; Gomes, M.; Maluf, R.V.; da Silva, A.J. Lorentz violation bounds on Bhabha scattering. Phys. Rev. D 2012, 86, 045003. [Google Scholar] [CrossRef]
- Gazzola, G.; Fargnoli, H.; Scarpelli, A.B.; Sampaio, M.; Nemes, M.C. QED with minimal and nonminimal couplings: On the quantum generation of Lorentz-violating terms in the pure photon sector. J. Phys. G 2012, 39, 035002. [Google Scholar] [CrossRef]
- Scarpelli, A.B. QED with chiral nonminimal coupling: aspects of the Lorentz-violating quantum corrections. J. Phys. G 2012, 39, 125001. [Google Scholar] [CrossRef]
- Brito, L.; Fargnoli, H.; Scarpelli, A.B. Aspects of quantum corrections in a Lorentz-violating extension of the Abelian Higgs model. Phys. Rev. D 2013, 87, 125023. [Google Scholar] [CrossRef]
- de Brito, G.; Junior, J.G.; Kroff, D.; Malta, P.; Marques, C. Lorentz violation in simple QED processes. Phys. Rev. D 2016, 94, 056005. [Google Scholar] [CrossRef]
- Santos, A.F.; Khanna, F.C. Lorentz Violation, Möller Scattering, and Finite Temperature. Adv. High Energy Phys. 2018, 2018, 7. [Google Scholar] [CrossRef]
- Casana, R.; Ferreira, M.M.; Passos, E.; dos Santos, F.E.P.; Silva, E.O. New CPT-even and Lorentz-violating nonminimal coupling in the Dirac equation. Phys. Rev. D 2013, 87, 047701. [Google Scholar] [CrossRef]
- Araujo, J.B.; Casana, R.; Ferreira, M.M. General CPT-even dimension-five nonminimal couplings between fermions and photons yielding EDM and MDM. Phys. Lett. B 2016, 760, 302–308. [Google Scholar] [CrossRef]
- Ding, Y.; Kosteleckỳ, V.A. Lorentz-violating spinor electrodynamics and Penning traps. Phys. Rev. D 2016, 94, 056008. [Google Scholar] [CrossRef]
- Mewes, M. Optical-cavity tests of higher-order Lorentz violation. Phys. Rev. D 2012, 85, 116012. [Google Scholar] [CrossRef]
- Schreck, M. Quantum field theoretic properties of Lorentz-violating operators of nonrenormalizable dimension in the photon sector. Phys. Rev. D 2014, 89, 105019. [Google Scholar] [CrossRef]
- Kosteleckỳ, V.A.; Mewes, M. Fermions with Lorentz-violating operators of arbitrary dimension. Phys. Rev. D 2013, 88, 096006. [Google Scholar] [CrossRef]
- Altschul, B. Vacuum Čerenkov Radiation in Lorentz-Violating Theories Without C P T Violation. Phys. Rev. Lett. 2007, 98, 041603. [Google Scholar] [CrossRef] [PubMed]
- Cambiaso, M.; Lehnert, R.; Potting, R. Massive photons and Lorentz violation. Phys. Rev. D 2012, 85, 085023. [Google Scholar] [CrossRef]
- Cambiaso, M.; Lehnert, R.; Potting, R. Asymptotic states and renormalization in Lorentz-violating quantum field theory. Phys. Rev. D 2014, 90, 065003. [Google Scholar] [CrossRef]
- Bailey, Q.G.; Kosteleckỳ, V.A. Lorentz-violating electrostatics and magnetostatics. Phys. Rev. D 2004, 70, 076006. [Google Scholar] [CrossRef]
- Colladay, D.; Kosteleckỳ, V.A. Cross sections and Lorentz violation. Phys. Lett. B 2001, 511, 209–217. [Google Scholar] [CrossRef]
- Kosteleckỳ, V.A.; Lane, C.D. Constraints on Lorentz violation from clock-comparison experiments. Phys. Rev. D 1999, 60, 116010. [Google Scholar] [CrossRef]
- Bluhm, R.; Kosteleckỳ, V.A.; Lane, C.D.; Russell, N. Clock-comparison tests of Lorentz and CPT symmetry in space. Phys. Rev. Lett. 2002, 88, 090801. [Google Scholar] [CrossRef] [PubMed]
- Bluhm, R.; Kosteleckỳ, V.A.; Lane, C.D.; Russell, N. Probing Lorentz and CPT violation with space-based experiments. Phys. Rev. D 2003, 68, 125008. [Google Scholar] [CrossRef]
- Derrick, M.; Fernandez, E.; Fries, R.; Hyman, L.; Kooijman, P.; Loos, J.S.; Musgrave, B.; Price, L.E.; Schlereth, J.; Sugano, K.; et al. New results on the reaction e+ + e− → μ+ + μ−, at = 29 GeV. Phys. Rev. D 1985, 31, 2352. [Google Scholar] [CrossRef]
- Bender, D.; Derrick, M.; Fernandez, E.; Gieraltowski, G.; Hyman, L.; Jaeger, K.; Klem, R.; Kooijman, P.; Kooijman, S.; Loos, J.; et al. Tests of QED at 29 GeV center-of-mass energy. Phys. Rev. D 1984, 30, 515. [Google Scholar] [CrossRef]
- Levi, M.; Blocker, C.; Strait, J.; Abrams, G.; Amidei, D.; Baden, A.; Boyarski, A.; Breidenbach, M.; Burchat, P.; Burke, D.; et al. Weak Neutral Currents in e+ e- Collisions at s = 29 GeV. Phys. Rev. Lett. 1983, 51, 1941. [Google Scholar] [CrossRef]
- Casana, R.; Ferreira, M., Jr.; Maluf, R.; dos Santos, F. Effects of a C P T-even and Lorentz-violating nonminimal coupling on electron-positron scattering. Phys. Rev. D 2012, 86, 125033. [Google Scholar] [CrossRef]


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santos, F.E.P.d.; Ferreira, M.M. Fermion Scattering in a CPT-Even Lorentz Violation Quantum Electrodynamics. Symmetry 2018, 10, 302. https://doi.org/10.3390/sym10080302
Santos FEPd, Ferreira MM. Fermion Scattering in a CPT-Even Lorentz Violation Quantum Electrodynamics. Symmetry. 2018; 10(8):302. https://doi.org/10.3390/sym10080302
Chicago/Turabian StyleSantos, Frederico E. P. dos, and Manoel M. Ferreira. 2018. "Fermion Scattering in a CPT-Even Lorentz Violation Quantum Electrodynamics" Symmetry 10, no. 8: 302. https://doi.org/10.3390/sym10080302
APA StyleSantos, F. E. P. d., & Ferreira, M. M. (2018). Fermion Scattering in a CPT-Even Lorentz Violation Quantum Electrodynamics. Symmetry, 10(8), 302. https://doi.org/10.3390/sym10080302



