Evaluating Water Use Efficiency in China’s Western Provinces Based on a Slacks-Based Measure (SBM)-Undesirable Window Model and a Malmquist Productivity Index
Abstract
1. Introduction
2. Methodology
2.1. SBM-Undesirable Model
2.2. Window Analysis
2.3. Malmquist Productivity Index
3. Data Processing and Empirical Analysis
3.1. Case Study
3.2. Index Selection and Data Source
3.3. Correlation Analysis
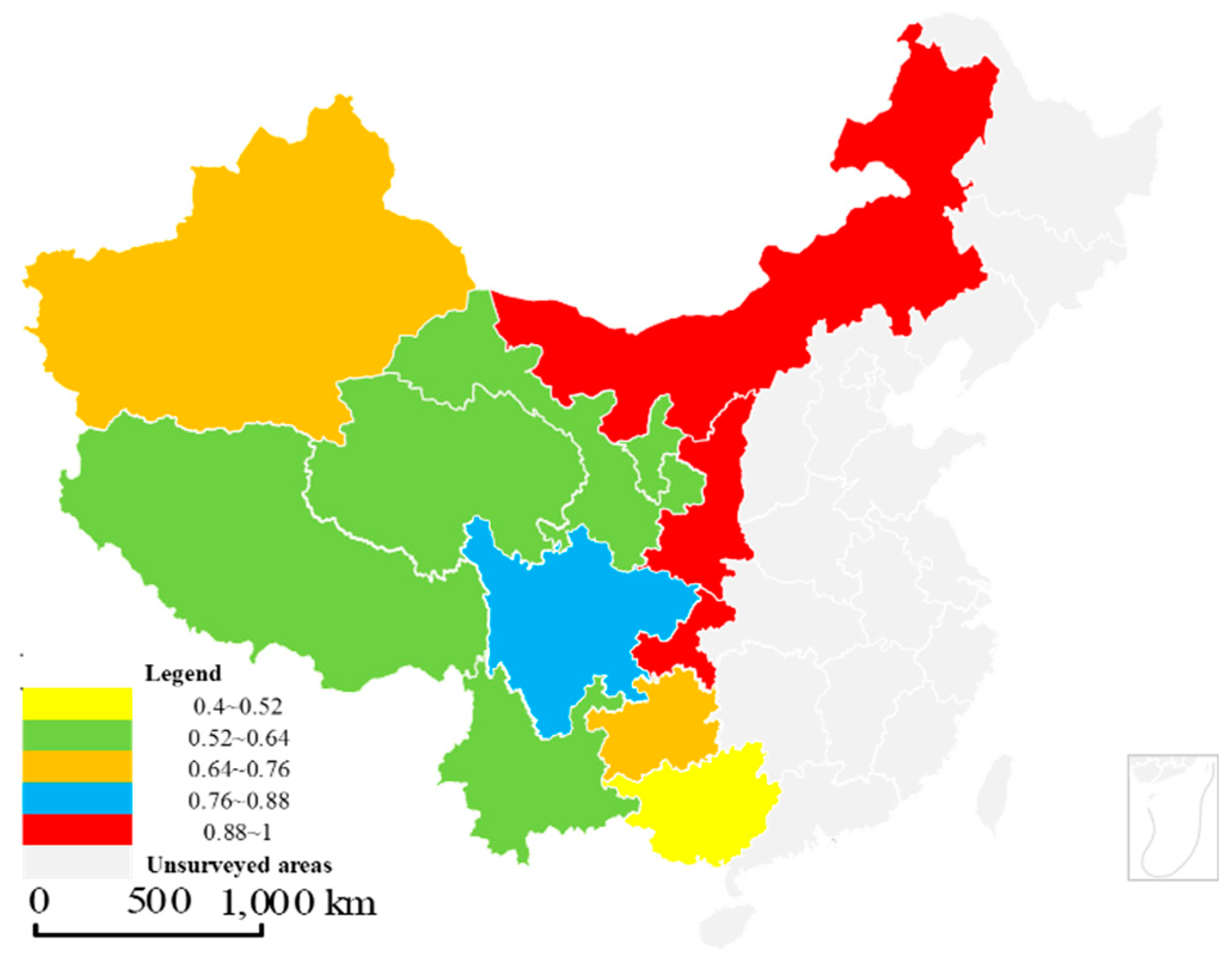
3.4. Overview of Water Use Efficiency
3.5. Non-Parametric Test
3.6. Water Use Efficiency Analysis Based on the Malmquist Productivity Index
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dogru, T.; Bulut, U. Is tourism an engine for economic recovery? Theory and empirical evidence. Tourism Manag. 2018, 67, 425–434. [Google Scholar] [CrossRef]
- Qin, Y.; Luo, Y.Y.; Zhao, Y.Q.; Zhang, J. Research on relationship between tourism income and economic growth based on meta-analysis. Appl. Math. Nonlinear Sci. 2018, 3, 105–114. [Google Scholar] [CrossRef]
- Lu, X.H.; Ke, S.G. Establishment of regional water resources ecological compensation model based on ecological footprint model-take the Yangtze River for example. Resour. Environ. Yangtze Basin 2016, 25, 334–341. [Google Scholar] [CrossRef]
- Liu, X.; Qu, J.S.; Liu, L.; Zeng, J.; Qian, Y.E. Review on climate change adaptation and its risk in western China. Ecol. Econ. 2017, 33, 185–189. [Google Scholar]
- Jia, L.; Gan, H.; Wang, L.; Qin, C.H. Discussion on water resources liabilities. J. Nat. Resour. 2017, 32, 1–11. [Google Scholar] [CrossRef]
- Fuentes, R.; Torregrosa, T.; Ballenilla, E. Conditional order-m efficiency of wastewater treatment plants: The role of environmental factors. Water 2015, 7, 5503–5524. [Google Scholar] [CrossRef]
- Yang, T.F. The changes of the balance of supply and demand of water resources ecological support capacity in watershed of Changjiang River and Zhujiang River-a case of Qiandongnan. Territory Nat. Resour. Study 2017, 1, 47–50. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, B. Water use efficiency and water conservation potential in China. Adv. Sci. Technol. Water Resour. 2015, 35, 5–10. [Google Scholar] [CrossRef]
- Lo Storto, C. Efficiency, conflicting goals and trade-offs: A nonparametric analysis of the water and wastewater service industry in Italy. Sustainability 2018, 10, 919. [Google Scholar] [CrossRef]
- Byrnes, J.; Lin, C.; Dollery, B.; Villano, R. The relative economic efficiency of urban water utilities in regional New South Wales and Victoria. Resour. Energy Econ. 2010, 32, 439–455. [Google Scholar] [CrossRef]
- Ma, H.; Shi, C.; Chou, N.T. China’s water utilization efficiency: An analysis with environmental considerations. Sustainability 2016, 8, 516. [Google Scholar] [CrossRef]
- Guerrini, A.; Romano, G.; Leardini, C.; Martini, M. The effects of operational and environmental variables on efficiency of Danish water and wastewater utilities. Water 2015, 7, 3263–3282. [Google Scholar] [CrossRef]
- Lo Storto, C. Are public-private partnerships a source of greater efficiency in water supply? Results of a non-parametric performance analysis relating to the Italian industry. Water 2013, 5, 2058–2079. [Google Scholar] [CrossRef]
- Deng, G.Y.; Han, J.; Zhang, Z.J. Study on measure of water use efficiency and rebound effect of Chinese provinces. Soft Sci. 2017, 31, 15–19. [Google Scholar] [CrossRef]
- Liao, H.; Dong, Y. Utilization efficiency of water resources in 12 western provinces of China based on the DEA and Malmquist TFP index. Resour. Sci. 2011, 33, 273–279. [Google Scholar]
- Zhu, Y.; Chen, Z.; Jin, Y. Evaluation on utilization efficiency of water resources in Jinhua city. Yangtze River 2016, 47, 43–47. [Google Scholar] [CrossRef]
- Ueda, T.; Hoshiai, Y. Application of principal component analysis for parsimonious summarization of DEA inputs and/or outputs. J. Oper. Res. Soc. Jpn. 2017, 40, 466–478. [Google Scholar] [CrossRef]
- Deng, G.; Li, L.; Song, Y. Provincial water use efficiency measurement and factor analysis in China: Based on SBM-DEA model. Ecol. Indic. 2016, 69, 12–18. [Google Scholar] [CrossRef]
- Toneab, K. Network DEA: A slacks-based measure approach. Eur. J. Oper. Res. 2009, 197, 243–252. [Google Scholar] [CrossRef]
- Ma, H.L.; Ding, Y.Q.; Wang, L. Measurement and convergence analysis of green water utilization efficiency. J. Nat. Resour. 2017, 32, 406–417. [Google Scholar] [CrossRef]
- Tone, K. Dealing with undesirable outputs in DEA: A slacks-based measure (SBM) approach(DEA(1)). Spring operations research meeting of the Japan. Oper. Res. Soc. 2004, 2004, 44–45. [Google Scholar]
- Oviatt, D.P.; Baumann, M.R.; Bennett, J.M.; Garza, R.T. Undesirable effects of working while in college: Work-school conflict, substance use, and health. J. Psychol. 2017, 151, 1–20. [Google Scholar] [CrossRef] [PubMed]
- Gao, X.P.; Sun, B.W.; Zi, T.L.; Zhang, C.; Li, Y.J. Application of improved fuzzy comprehensive water quality assessment based on weight matrix of temporal distribution. China J. Environ. Eng. 2017, 11, 970–976. [Google Scholar] [CrossRef]
- Wang, P.; Zhu, B.; Tao, X.; Xie, R. Measuring regional energy efficiencies in China: A meta-frontier SBM-Undesirable approach. Nat. Hazards 2017, 85, 793–809. [Google Scholar] [CrossRef]
- Liu, F.; Wang, Y. Research on efficiency measurement of China’s high end equipment manufacturing enterprise based on meta-frontier-bootstrap-DEA method. Stat. Inf. Forum 2017, 32, 92–98. [Google Scholar] [CrossRef]
- Charnes, A.; Cooper, W.; Golany, B.; Learner, D.B.; Phillips, F.Y. A multiperiod analysis of market segments and brand efficiency in the competitive carbonated beverage industry. Data Envel. Anal. Theory Methodol. Appl. 1994, 47, 145–165. [Google Scholar] [CrossRef]
- Färe, R.; Grosskopf, S.; Norris, M.; Zhang, Z. Productivity growth, technical progress, and efficiency change in industrialized countries. Am. Econ. Rev. 1994, 84, 66–83. [Google Scholar] [CrossRef]
- Yao, X.; Yan, J.; Wang, L. Industrial heterogeneity offshoring and productivity spillover: Empirical analysis based on wiod database. Econ. Rev. 2017, 4, 57–72. [Google Scholar]
- Al-Refaie, A.; Wu, C.W.; Sawalheh, M. DEA window analysis for assessing efficiency of blistering process in a pharmaceutical industry. Neural Comput. Appl. 2018, 6, 1–15. [Google Scholar] [CrossRef]
- Lu, X.; Xu, C.X. Study the utilization efficiency of water resources in Yangtze River economic based on three-stage DEA and Malmquist index decomposition. Resour. Environ. Yangtze Basin 2017, 26, 7–14. [Google Scholar]
- Zhang, F.T.; Su, W.C. Measurement and projection optimization analysis of water resources use efficiency in Chongqing. China Rural Water Hydropower 2016, 11, 93–96. [Google Scholar]
- Han, Y.Q.; Su, S.P. Static and dynamic analysis on water use efficiency of Fujian Province-Based on DEA-BCC and DEA-Malmquist Model. Resour. Dev. Mark. 2015, 31, 578–583. [Google Scholar]
- Zhang, K.; Ma, J.Z.; Wu, Z.B. Study of correction method of deep water sounding and application. Yangtze River 2015, 46, 48–51. [Google Scholar] [CrossRef]
- Wu, W.B.; Liu, Y.N.; Hu, Z. Comparison of water use efficiency of provincial water resources based on DEA model. China New Technol. Prod. 2008, 7, 190–191. [Google Scholar]
- Dong, Z.F.; Yu, E.Y.; Qiu, L. Water efficiency evaluation of the provincial regions in China based on DEA model. Ecol. Econ. 2012, 10, 43–47. [Google Scholar]
- Zhao, C.; Wang, Y.; Gu, X.M. Water use efficiency of Jiangsu Province based on the data envelopment analysis approach. Acta Ecol. Sin. 2013, 33, 1636–1644. [Google Scholar] [CrossRef]
- Sun, C.Z.; Yan, D. Evaluation of sustainable development of water resources and socio-economy in Dalian based on DEA model. J. Econ. Water Resour. 2008, 26, 1–4. [Google Scholar]
- Li, Z.M.; Liao, H.C. Input and output analysis of water resources across China in 2010. Resour. Sci. 2012, 34, 2274–2281. [Google Scholar]
- Wu, J.Y.; Han, Z.L.; Wu, H.G. Water resource utilization efficiency and its spatial spillover efficiency measure in Liaoning Province. Resour. Dev. Mark. 2017, 33, 417–422. [Google Scholar]
- Wang, Y. Chinese provincial water resources utility efficiency and its influencing factors based on super-efficiency DEA and Tobit model. China Rural Water Hydropower 2015, 41–44. [Google Scholar]
- Jiang, B.Q.; Liu, X. Evaluation on efficiency of water utilization of bohai economic zone based on DEA-Malmquist. Resour. Dev. Market. 2015, 31, 49–51. [Google Scholar]
- Dong, M.Y.; Liao, H.C. Utilization efficiency of water resource in west capital cities based on DEA. Bull. Soil Water Conserv. 2011, 31, 134–139. [Google Scholar] [CrossRef]
- Yu, Y.G.; Liu, L.Y. Regional differences and influence factors of water resource efficiency in China: Based on super efficiency DEA-Tobit. Econ. Geogr. 2017, 37, 12–19. [Google Scholar]
- You, S.Q. Different cities in our country are green research on total factor water resources efficiency. Acad. Exch. 2016, 267, 173–176. [Google Scholar]
- Ma, H.L.; Huang, D.C.; Zhang, J.G. Water resource utility efficiency and its influencing factors considering undesirable goods. China Popul. Environ. 2012, 22, 35–42. [Google Scholar]
- Liu, J.; Bai, Y.; Han, X. The impact of urbanization on innovation on efficiency in China-based on SFA model test in two stage of innovation. Chin. J. Manag. 2017, 14, 704–712. [Google Scholar] [CrossRef]
- Wada, Y.; Gleeson, T.; Esnault, L. Wedge approach to water stress. Nat. Geosci. 2014, 7, 615–617. [Google Scholar] [CrossRef]
- Das, B.; Singh, A.; Panda, S.N.; Yasuda, H. Optimal land and water resources allocation policies for sustainable irrigated agriculture. Land Use Policy 2015, 42, 527–537. [Google Scholar] [CrossRef]
- Salmoral, G.; Willaarts, B.A.; Garrido, A.G.; Guse, B. Fostering integrated land and water management approaches: Evaluating the water footprint of a Mediterranean basin under different agricultural land use scenarios. Land Use Policy 2017, 61, 24–39. [Google Scholar] [CrossRef]
| Index Type | Index Name | References |
|---|---|---|
| Input | Total agricultural water (TA) | [30,31,32,33,34,35,36] |
| Total industrial water (TI) | [14,16,30,31,32,33,34,35,36,37] | |
| Total domestic water (TD) | [14,16,30,32,33,34,35,36,37,38] | |
| Total investment in fixed assets for the whole society (TIS) | [30,31,32,33,35,36,37,38,39,40,41,42] | |
| Output | Regional GDP (RG) | [30,33,38,41,42,43] |
| Waste water discharge (WD) | [20,44,45] |
| TA | TI | TD | TIS | RG | WD | ||
|---|---|---|---|---|---|---|---|
| TA | Pearson correlation | 1 | 0.057 | 0.227 | 0.150 | 0.157 | 0.207 |
| Sig. (2-tailed) | 0.860 | 0.479 | 0.641 | 0.627 | 0.519 | ||
| N | 12 | 12 | 12 | 12 | 12 | 12 | |
| TI | Pearson correlation | 0.057 | 1 | 0932 ** | 0749 ** | 0.778 ** | 0926 ** |
| Sig. (2-tailed) | 0860 | 0.000 | 0.005 | 0.003 | 0.000 | ||
| N | 12 | 12 | 12 | 12 | 12 | 12 | |
| TD | Pearson correlation | 0.227 | 0932 ** | 1 | 0762 ** | 0.789 ** | 0.975 ** |
| Sig. (2-tailed) | 0.479 | 0.000 | 0.004 | 0.002 | 0.000 | ||
| N | 12 | 12 | 12 | 12 | 12 | 12 | |
| TIS | Pearson correlation | 0.150 | 0.749 ** | 0.762 ** | 1 | 0.997 ** | 0823 ** |
| Sig. (2-tailed) | 0.641 | 0.005 | 0.004 | 0.000 | 0.001 | ||
| N | 12 | 12 | 12 | 12 | 12 | 12 | |
| RG | Pearson correlation | 0.157 | 0.778 ** | 0.789 ** | 0.997 ** | 1 | 0.848 |
| Sig. (2-tailed) | 0.627 | 0.003 | 0.002 | 0.000 | 0.000 | ||
| N | 12 | 12 | 12 | 12 | 12 | 12 | |
| WD | Pearson correlation | 0.207 | 0.926 ** | 0.975 ** | 0.823 ** | 0.848 ** | 1 |
| Sig. (2-tailed) | 0.519 | 0.000 | 0.000 | 0.001 | 0.000 | ||
| N | 12 | 12 | 12 | 12 | 12 | 12 | |
| Provinces | W1 | W2 | W3 | W4 | W5 | W6 | W7 | W8 | W9 | Mean |
|---|---|---|---|---|---|---|---|---|---|---|
| Yunnan (P1) | 0.593 | 0.604 | 0.648 | 0.603 | 0.517 | 0.543 | 0.570 | 0.533 | 0.527 | 0.571 |
| Guizhou (P2) | 0.516 | 0.726 | 0.872 | 0.745 | 0.718 | 0.665 | 0.501 | 0.502 | 0.521 | 0.641 |
| Sichuan (P3) | 0.690 | 0.921 | 0.871 | 0.719 | 0.657 | 0.804 | 0.868 | 0.845 | 0.888 | 0.807 |
| Chongqing (P4) | 1.000 | 0.957 | 1.000 | 0.950 | 0.795 | 0.887 | 1.000 | 1.000 | 1.000 | 0.954 |
| Tibet (P5) | 0.851 | 0.482 | 0.478 | 0.499 | 0.440 | 0.703 | 0.644 | 0.742 | 0.859 | 0.633 |
| Shaanxi (P6) | 1.000 | 1.000 | 1.000 | 0.947 | 0.835 | 0.929 | 1.000 | 1.000 | 1.000 | 0.968 |
| Gansu (P7) | 0.935 | 0.946 | 0.824 | 0.687 | 0.487 | 0.442 | 0.475 | 0.496 | 0.473 | 0.640 |
| Qinghai (P8) | 0.412 | 0.458 | 0.545 | 0.578 | 0.465 | 0.512 | 0.548 | 0.591 | 0.596 | 0.523 |
| Ningxia (P9) | 0.619 | 0.632 | 0.826 | 0.844 | 0.655 | 0.488 | 0.530 | 0.538 | 0.509 | 0.627 |
| Xinjiang (P10) | 0.801 | 1.000 | 0.843 | 0.734 | 0.792 | 0.703 | 0.652 | 0.476 | 0.421 | 0.714 |
| Inner Mongolia (P11) | 0.819 | 0.807 | 0.922 | 1.000 | 0.854 | 0.895 | 1.000 | 1.000 | 0.964 | 0.918 |
| Guangxi (P12) | 0.624 | 0.459 | 0.457 | 0.428 | 0.410 | 0.417 | 0.438 | 0.398 | 0.388 | 0.446 |
| Mean | 0.738 | 0.749 | 0.774 | 0.728 | 0.635 | 0.666 | 0.686 | 0.677 | 0.679 |
| Provinces | 2005 | 2006 | 2007 | 2008 | 2009 | 2010 | 2011 | 2012 | 2013 | 2014 | 2015 | Mean |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| P1 | 0.569 | 0.580 | 0.629 | 0.698 | 0.508 | 0.485 | 0.657 | 0.524 | 0.525 | 0.543 | 0.523 | 0.567 |
| P2 | 0.450 | 0.520 | 0.744 | 1.000 | 0.749 | 0.750 | 0.509 | 0.486 | 0.511 | 0.509 | 0.544 | 0.616 |
| P3 | 0.611 | 0.835 | 0.851 | 1.000 | 0.524 | 0.563 | 1.000 | 0.927 | 0.844 | 0.858 | 0.665 | 0.789 |
| P4 | 1.000 | 1.000 | 0.957 | 1.000 | 0.823 | 0.808 | 1.000 | 1.000 | 1.000 | 1.000 | 1.000 | 0.963 |
| P5 | 1.000 | 0.746 | 0.485 | 0.476 | 0.480 | 0.449 | 0.440 | 0.509 | 0.764 | 1.000 | 1.000 | 0.668 |
| P6 | 1.000 | 1.000 | 1.000 | 1.000 | 0.840 | 0.871 | 1.000 | 1.000 | 1.000 | 1.000 | 1.000 | 0.974 |
| P7 | 0.805 | 1.000 | 1.000 | 0.924 | 0.524 | 0.467 | 0.463 | 0.464 | 0.496 | 0.499 | 0.475 | 0.647 |
| P8 | 0.359 | 0.391 | 0.449 | 0.642 | 0.481 | 0.484 | 0.482 | 0.589 | 0.583 | 0.620 | 0.598 | 0.516 |
| P9 | 0.385 | 0.443 | 0.653 | 1.000 | 0.662 | 0.645 | 0.664 | 0.539 | 0.526 | 0.519 | 0.544 | 0.598 |
| P10 | 0.599 | 0.902 | 1.000 | 1.000 | 0.599 | 0.891 | 0.760 | 0.470 | 0.462 | 0.450 | 0.422 | 0.687 |
| P11 | 0.665 | 0.712 | 0.851 | 1.000 | 0.918 | 0.831 | 1.000 | 1.000 | 0.964 | 1.000 | 1.000 | 0.904 |
| P12 | 1.000 | 0.338 | 0.450 | 0.501 | 0.353 | 0.340 | 0.520 | 0.399 | 0.388 | 0.393 | 0.398 | 0.462 |
| Mean | 0.704 | 0.706 | 0.756 | 0.853 | 0.622 | 0.632 | 0.708 | 0.659 | 0.672 | 0.699 | 0.681 |
| W1 | W2 | W3 | W4 | W5 | W6 | W7 | W8 | W9 | |
|---|---|---|---|---|---|---|---|---|---|
| 11.000 | 11.000 | 11.000 | 11.000 | 11.000 | 11.000 | 11.000 | 11.000 | 11.000 | |
| df | 11 | 11 | 11 | 11 | 11 | 11 | 11 | 11 | 11 |
| Asymptotic significance | 0.443 | 0.443 | 0.443 | 0.443 | 0.443 | 0.443 | 0.443 | 0.443 | 0.443 |
| P1 | P2 | P3 | P4 | P5 | P6 | P7 | P8 | P9 | P10 | P11 | P12 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 10.000 | 10.000 | 10.000 | 10.000 | 10.000 | 10.000 | 10.000 | 10.000 | 10.000 | 10.000 | 10.000 | 10.000 | |
| df | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 |
| Asymptotic significance | 0.440 | 0.440 | 0.440 | 0.440 | 0.440 | 0.440 | 0.440 | 0.440 | 0.440 | 0.440 | 0.440 | 0.440 |
| Years | Effch | Techch | Pech | Sech | Tfpch |
|---|---|---|---|---|---|
| 2006 | 0.981 | 1.134 | 0.968 | 1.014 | 1.113 |
| 2007 | 0.969 | 1.111 | 0.983 | 0.986 | 1.077 |
| 2008 | 1.063 | 1.127 | 1.029 | 1.033 | 1.198 |
| 2009 | 0.821 | 1.020 | 0.863 | 0.951 | 0.837 |
| 2010 | 1.029 | 1.105 | 1.022 | 1.008 | 1.138 |
| 2011 | 1.042 | 1.131 | 1.031 | 1.011 | 1.179 |
| 2012 | 0.891 | 1.164 | 0.917 | 0.971 | 1.037 |
| 2013 | 1.054 | 1.041 | 1.011 | 1.043 | 1.098 |
| 2014 | 1.003 | 1.027 | 1.001 | 1.002 | 1.030 |
| 2015 | 0.976 | 1.016 | 0.984 | 0.992 | 0.991 |
| Mean | 0.980 | 1.086 | 0.979 | 1.001 | 1.065 |
| Provinces | Effch | Techch | Pech | Sech | Tfpch |
|---|---|---|---|---|---|
| P1 | 0.928 | 1.066 | 0.938 | 0.990 | 0.989 |
| P2 | 0.912 | 0.992 | 0.912 | 1.000 | 0.905 |
| P3 | 1.015 | 1.017 | 1.007 | 1.008 | 1.033 |
| P4 | 1.000 | 1.094 | 1.000 | 1.000 | 1.094 |
| P5 | 0.941 | 1.373 | 1.000 | 0.941 | 1.292 |
| P6 | 1.038 | 1.088 | 1.000 | 1.038 | 1.129 |
| P7 | 1.000 | 1.059 | 1.000 | 1.000 | 1.059 |
| P8 | 1.007 | 1.012 | 1.000 | 1.007 | 1.019 |
| P9 | 1.000 | 1.232 | 1.000 | 1.000 | 1.232 |
| P10 | 0.949 | 1.027 | 0.921 | 1.030 | 0.974 |
| P11 | 0.981 | 1.055 | 0.982 | 0.999 | 1.035 |
| P12 | 1.000 | 1.075 | 1.000 | 1.000 | 1.075 |
| Mean | 0.980 | 1.086 | 0.979 | 1.001 | 1.065 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, Y.; Yin, L.; Qin, Y.; Wang, Z.; Gong, Y. Evaluating Water Use Efficiency in China’s Western Provinces Based on a Slacks-Based Measure (SBM)-Undesirable Window Model and a Malmquist Productivity Index. Symmetry 2018, 10, 301. https://doi.org/10.3390/sym10080301
Luo Y, Yin L, Qin Y, Wang Z, Gong Y. Evaluating Water Use Efficiency in China’s Western Provinces Based on a Slacks-Based Measure (SBM)-Undesirable Window Model and a Malmquist Productivity Index. Symmetry. 2018; 10(8):301. https://doi.org/10.3390/sym10080301
Chicago/Turabian StyleLuo, Yuyan, Lu Yin, Yong Qin, Zhong Wang, and Yanfeng Gong. 2018. "Evaluating Water Use Efficiency in China’s Western Provinces Based on a Slacks-Based Measure (SBM)-Undesirable Window Model and a Malmquist Productivity Index" Symmetry 10, no. 8: 301. https://doi.org/10.3390/sym10080301
APA StyleLuo, Y., Yin, L., Qin, Y., Wang, Z., & Gong, Y. (2018). Evaluating Water Use Efficiency in China’s Western Provinces Based on a Slacks-Based Measure (SBM)-Undesirable Window Model and a Malmquist Productivity Index. Symmetry, 10(8), 301. https://doi.org/10.3390/sym10080301





