Polyhedral Phenylacetylenes: The Interplay of Aromaticity and Antiaromaticity in Convex Graphyne Substructures
Abstract
:1. Introduction
2. Computational Methods
3. Results
3.1. Energies of formation
3.2. Link topology
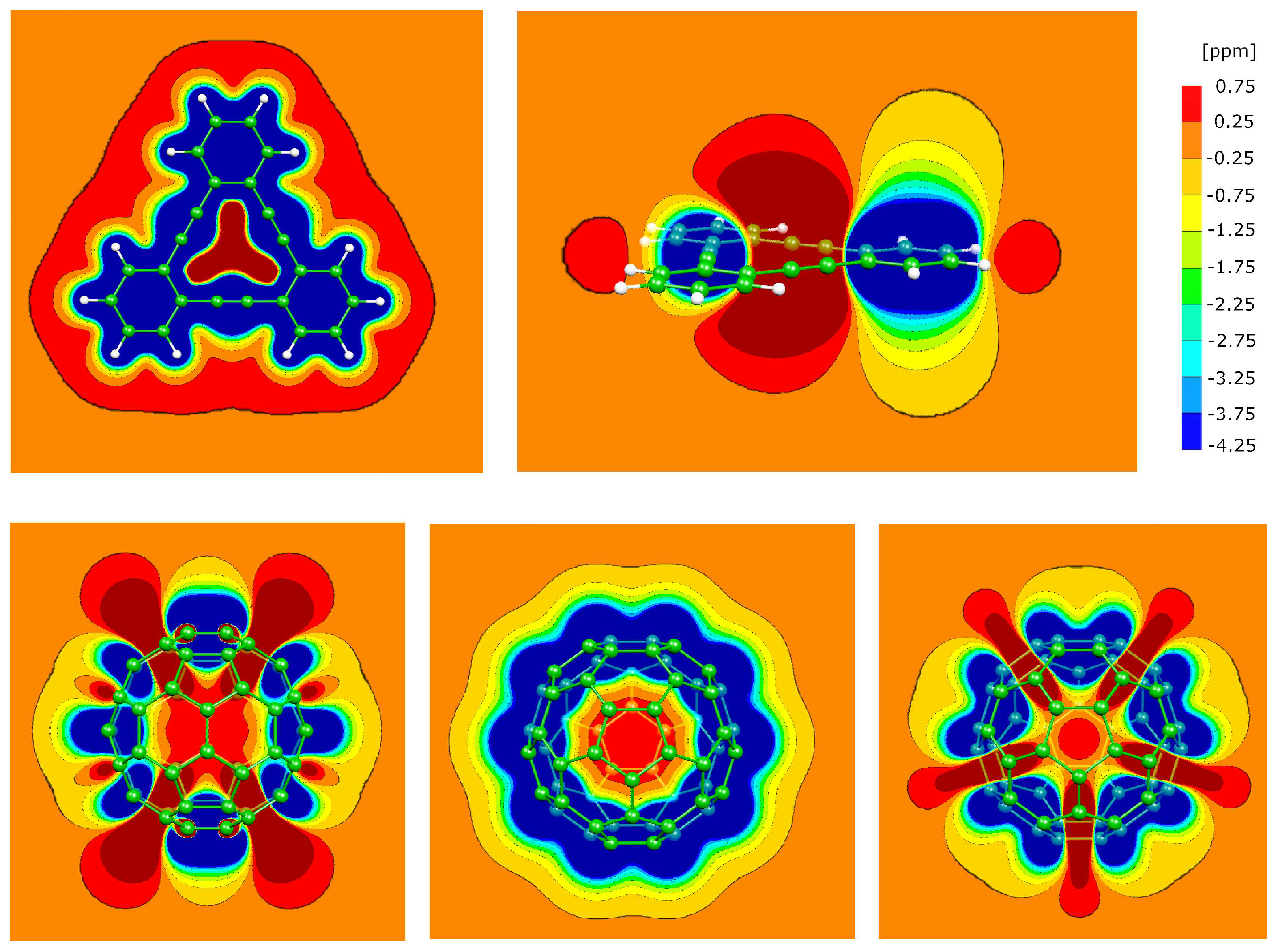
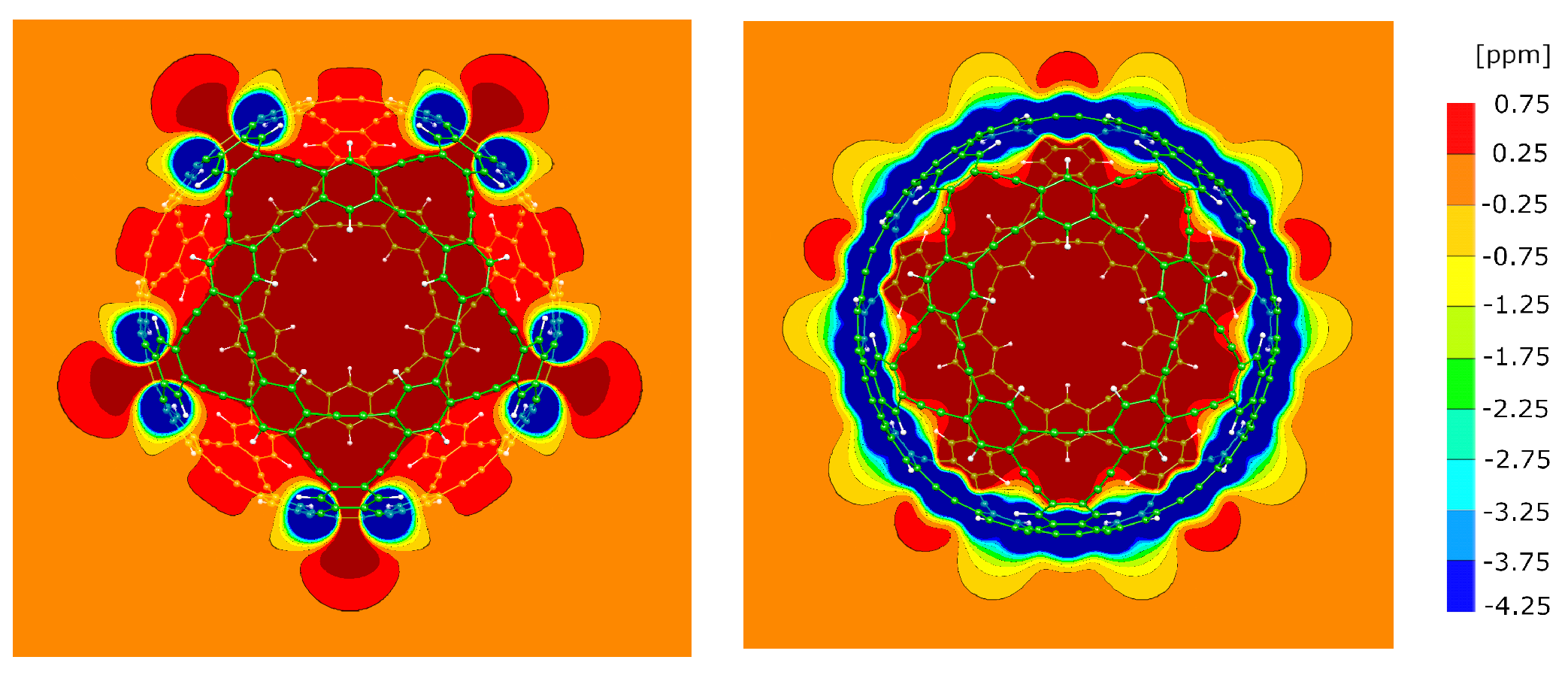
3.3. Cyclic phenylacetylene trimer and conventional Fullerene C
3.4. Necklace-style phenylacetylenes
3.5. The P1 molecule
3.6. The P2 molecule
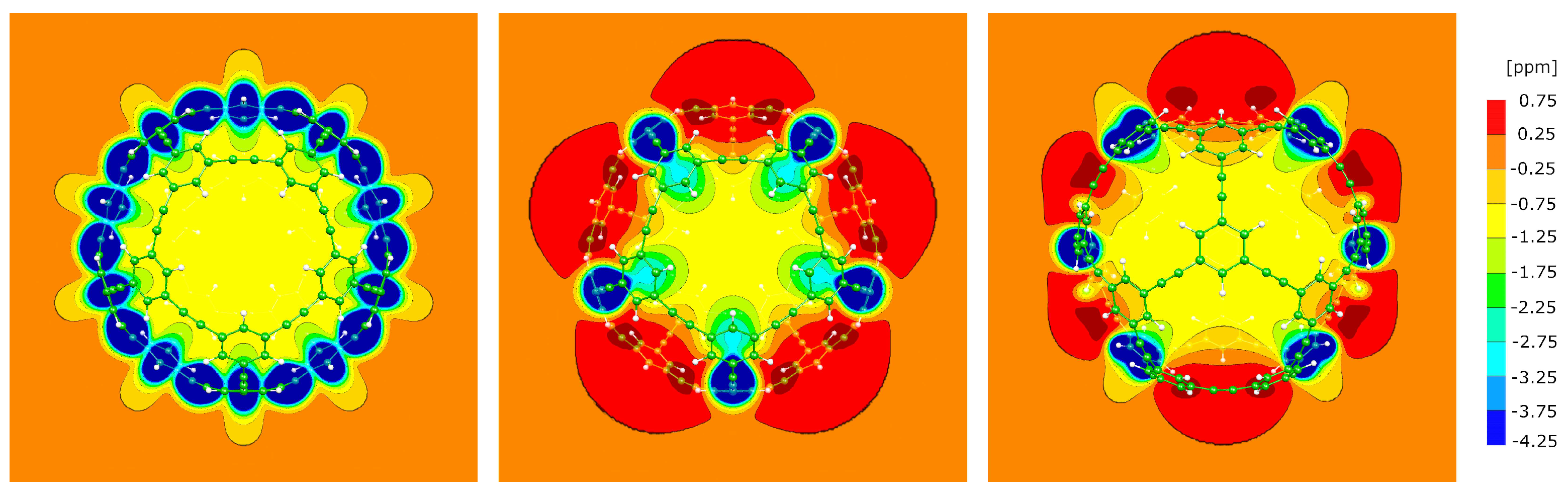
3.7. The P4 molecule
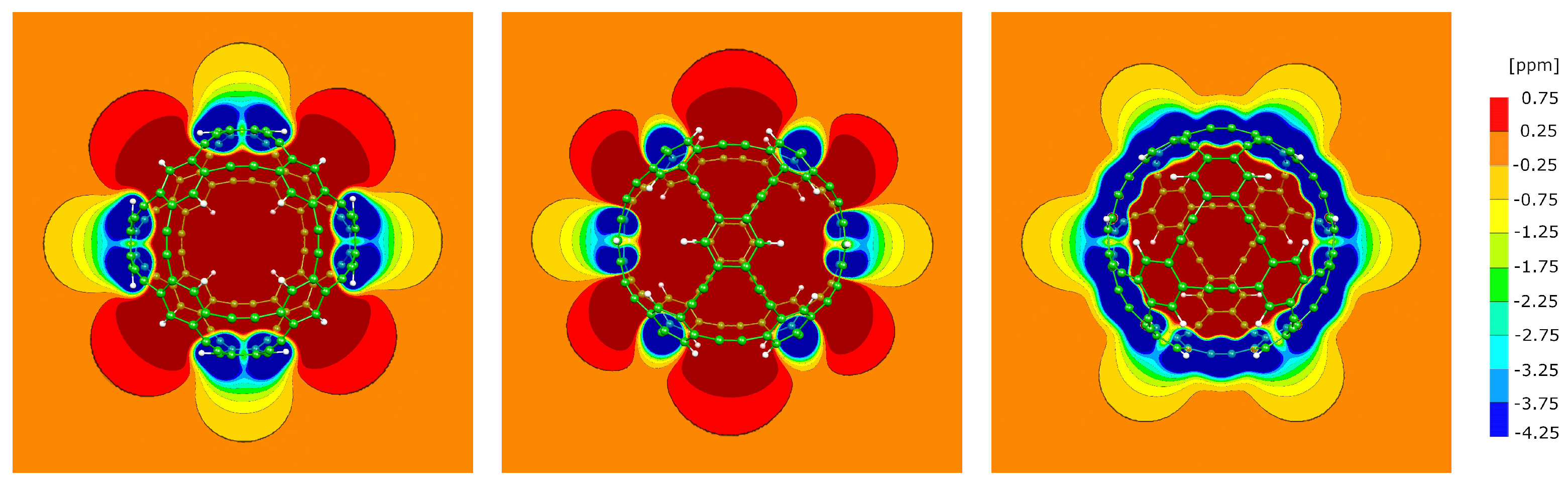
3.8. The A1 molecule
3.9. The A4 molecule
4. Discussion and Conclusions
Acknowledgements
References
- Bacon, R. Growth, Structure, and Properties of Graphite Whiskers. J. Appl. Phys. 1960, 31, 283–290. [Google Scholar] [CrossRef]
- Kroto, H.W.; Heath, J.R.; O’Brien, S.C.; Curl, R.F.; Smalley, R.E. C-60 - Buckminsterfullerene. Nature 1985, 318, 162–163. [Google Scholar] [CrossRef]
- Grossman, J.C.; Cote, M.; Louie, S.G.; Cohen, M.L. Electronic and structural properties of molecular C-36. Chem. Phys. Lett. 1998, 284, 344–349. [Google Scholar] [CrossRef]
- Collins, P.G.; Grossman, J.C.; Cote, M.; Ishigami, M.; Piskoti, C.; Louie, S.G.; Cohen, M.L.; Zettl, A. Scanning tunneling spectroscopy of C-36. Phys. Rev. Lett. 1999, 82, 165–168. [Google Scholar] [CrossRef]
- Klimko, G.T.; Mestechkin, M.M.; Whyman, G.E.; Khmelevsky, S. C-28 and C-48 fullerenes special properties. J. Mol. Struct. 1999, 481, 329–333. [Google Scholar] [CrossRef]
- Romero, A.H.; Sebastiani, D.; Ramírez, R.; Kiwi, M. Is NMR the tool to characterize the structure of C20 isomers? Chem. Phys. Lett. 2002, 366, 134–140. [Google Scholar] [CrossRef]
- Kroto, H.W. C-60 - Buckminsterfullerene, the Celestial Sphere That Fell To Earth. Angew. Chem. Int. Ed. 1992, 31, 111–129. [Google Scholar] [CrossRef]
- Prinzbach, H.; Weiler, A.; Landenberger, P.; Wahl, F.; Worth, J.; Scott, L.T.; Gelmont, M.; Olevano, D.; van Issendorff, B. Gas-phase production and photoelectron spectroscopy of the smallest fullerene, C20. Nature 2000, 407, 60. [Google Scholar] [CrossRef] [PubMed]
- Piskoti, C.; Yarger, J.; Zettl, A. C-36, a new carbon solid. Nature 1998, 393, 771–774. [Google Scholar] [CrossRef]
- Weber, K.; Voss, T.; Heimbach, D.; Weiler, A.; Keller, M.; Worth, J.; Knothe, L.; Exner, K.; Prinzbach, H. From unsaturated dodecahedranes to C-40 cages? Tetrahedron Lett. 2005, 46, 5471–5474. [Google Scholar] [CrossRef]
- Wahl, F.; Weiler, A.; Landenberger, P.; Sackers, E.; Voss, T.; Haas, A.; Lieb, M.; Hunkler, D.; Worth, J.; Knothe, L.; Prinzbach, H. Towards perfunctionalized dodecahedranes - En route to C-20 fullerene. Chem. Eur. J. 2006, 12, 6255–6267. [Google Scholar] [CrossRef]
- Narita, N.; Nagai, S.; Suzuki, S.; Nakao, K. Optimized geometries and electronic structures of graphyne and its family. Phys. Rev. B 1998, 58, 11009–11014. [Google Scholar] [CrossRef]
- Kehoe, J.M.; Kiley, J.H.; English, J.J.; Johnson, C.A.; Petersen, R.C.; Haley, M.M. Carbon networks based on dehydrobenzoannulenes. 3. Synthesis of graphyne substructures. Org. Lett. 2000, 2, 969–972. [Google Scholar] [CrossRef] [PubMed]
- Tahara, K.; Yoshimura, T.; Sonoda, M.; Tobe, Y.; Williams, R.V. Theoretical studies on graphyne substructures: Geometry, aromaticity, and electronic properties of the multiply fused dehydrobenzo[12]annulenes. J. Org. Chem. 2007, 72, 1437–1442. [Google Scholar] [CrossRef]
- Jones, R.O.; Gunnarsson, O. The Density Functional Formalism, Its Applications and Prospects. Rev. Mod. Phys. 1989, 61, 689–746. [Google Scholar] [CrossRef]
- Hutter, J.; others. Computer code CPMD, version 3.12. Copyright IBM Corp. and MPI-FKF Stuttgart 1990-2008. www.cpmd.org.
- Putrino, A.; Sebastiani, D.; Parrinello, M. Generalized Variational Density Functional Perturbation Theory. J. Chem. Phys. 2000, 113, 7102–7109. [Google Scholar] [CrossRef]
- Sebastiani, D.; Parrinello, M. A New Method to Compute NMR Chemical Shifts in Periodic Systems. J. Phys. Chem. A 2001, 105, 1951. [Google Scholar] [CrossRef]
- Sebastiani, D. Current Density Plots and Nucleus Independent Chemical Shift Maps (NICS) from Reciprocal Space Density Functional Perturbation Theory Calculations. ChemPhysChem 2006, 7, 164–175. [Google Scholar] [CrossRef]
- Schleyer, P.v.R.; Maerker, C.; Dransfeld, A.; Jiao, H.; Hommes, N.J.R.v.E. Nucleus–Independent Chemical Shifts: A Simple and Efficient Aromaticity Probe. J. Am. Chem. Soc. 1996, 118, 6317–6318. [Google Scholar] [CrossRef]
- Heine, T.; Schleyer, P.v.R.; Corminboeuf, C.; Seifert, G.; Reviakine, R.; Weber, J. Analysis of Aromatic Delocalization: Individual Molecular Orbital Contributions to Nucleus-Independent Chemical Shifts. J. Phys. Chem. A 2003, 107, 6470–6475. [Google Scholar] [CrossRef]
- Moran, D.; Stahl, F.; Bettinger, H.F.; Schaefer, H.; Schleyer, P.v.R. Towards graphite: Magnetic properties of large polybenzenoid hydrocarbons. J. Am. Chem. Soc. 2003, 125, 6746–6752. [Google Scholar] [CrossRef] [PubMed]
- Corminboeuf, C.; Heine, T.; Seifert, G.; Schleyer, P.v.R.; Weber, J. Induced magnetic fields in aromatic [n]-annulenes - interpretation of NICS tensor components. Phys. Chem. Chem. Phys. 2004, 6, 273–276. [Google Scholar] [CrossRef]
- Sebastiani, D.; Kudin, K. Response Properties of Carbon Nanotubes in Magnetic Fields. ACS Nano 2008, 2, 661–668. [Google Scholar] [CrossRef] [PubMed]
- Kirchner, B.; Sebastiani, D. Visualizing Degrees of Aromaticity. J. Phys. Chem. A 2004, 108, 11728–11732. [Google Scholar] [CrossRef]
- Lazzeretti, P. Assessment of aromaticity via molecular response properties. Phys. Chem. Chem. Phys. 2004, 6, 217–223. [Google Scholar] [CrossRef]
- Merino, G.; Heine, T.; Seifert, G. The induced magnetic field in cyclic molecules. Chem. Eur. J. 2004, 10, 4367–4371. [Google Scholar] [CrossRef] [PubMed]
- Lazzeretti, P. Ring-Current Signatures in Shielding-Density Maps. Chem. Phys. Lett. 2005, 401, 164–169. [Google Scholar]
- Kleinpeter, E.; Fettke, A. Quantification of the (anti)aromaticity of fulvenes subject to ring size. Tetrahedron Lett. 2008, 49, 2776–2781. [Google Scholar] [CrossRef]
- Gonze, X.; Vigneron, J.P. Phys. Rev. B 1989, 39, 13120. [CrossRef]
- Gonze, X. Phys. Rev. A 1995, 52, 1096. [CrossRef]
- Baroni, S.; de Gironcoli, S.; del Corso, A.; Giannozzi, P. Phonons and Related Crystal Properties from Density-Functional Perturbation Theory. Rev. Mod. Phys. 2001, 73, 515. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. The Inhomogeneous Electron Gas. Phys. Rev. 1964, 136, B864. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-Consistent Equations Including Exchange and Correlation Effects. Phys. Rev. 1965, 140, A1133. [Google Scholar] [CrossRef]
- Goedecker, S.; Teter, M.; Hutter, J. Separable Dual-Space Gaussian Pseudopotentials. Phys. Rev. B 1996, 54, 1703. [Google Scholar] [CrossRef]
- Hartwigsen, C.; Goedecker, S.; Hutter, J. Relativistic Separable Dual-Space Gaussian Pseudopotentials from H to Rn. Phys. Rev. B 1998, 58, 3641. [Google Scholar] [CrossRef]
- Becke, A.D. Density-Functional Exchange-Energy Approximation With Correct Asymptotic Behavior. Phys. Rev. A 1988, 38, 3098. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti Correlation-Energy Formula into a Functional of the Electron-Density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
- Johnson, C.E.; Bovey, F.A. Calculation of Nuclear Magnetic Resonance Spectra of Aromatic Hydrocarbons. J. Chem. Phys. 1958, 29, 1012–1014. [Google Scholar] [CrossRef]
- Aihara, J.I. Nucleus-independent chemical shifts and local aromaticities in large polycyclic aromatic hydrocarbons. Chem. Phys. Lett. 2002, 365, 34–39. [Google Scholar] [CrossRef]
- Steiner, E.; Fowler, P.W.; Jenneskens, L.W.; Havenith, R.W.A. Local and global paratropic and diatropic ring currents in pyrene and its cyclopenta-fused congeners. Eur. J. Org. Chem. 2002, 365, 163–169. [Google Scholar] [CrossRef]
- Matsuo, Y.; Tahara, K.; Nakamura, E. Theoretical Studies on Structures and Aromaticity of Finite-Length Armchair Carbon Nanotubes. Org. Lett. 2003, 5, 3181–3184. [Google Scholar] [CrossRef]
- Poater, J.; Fradera, X.; Duran, M.; Sola, M. An Insight into the Local Aromaticities of Polycyclic Aromatic Hydrocarbons and Fullerenes. Chem. Eur. J. 2003, 9, 1113–1122. [Google Scholar] [CrossRef] [PubMed]
- Fowler, P.W.; Soncini, A. Aromaticity, polarisability and ring current. Chem. Phys. Lett. 2004, 383, 507–511. [Google Scholar] [CrossRef]
- Fowler, P.W.; Steiner, E.; Havenith, R.W.A.; Jenneskens, L.W. Current Density, Chemical Shifts and Aromaticity. Magn. Reson. Chem. 2004, 42, S68–S78. [Google Scholar] [CrossRef] [PubMed]


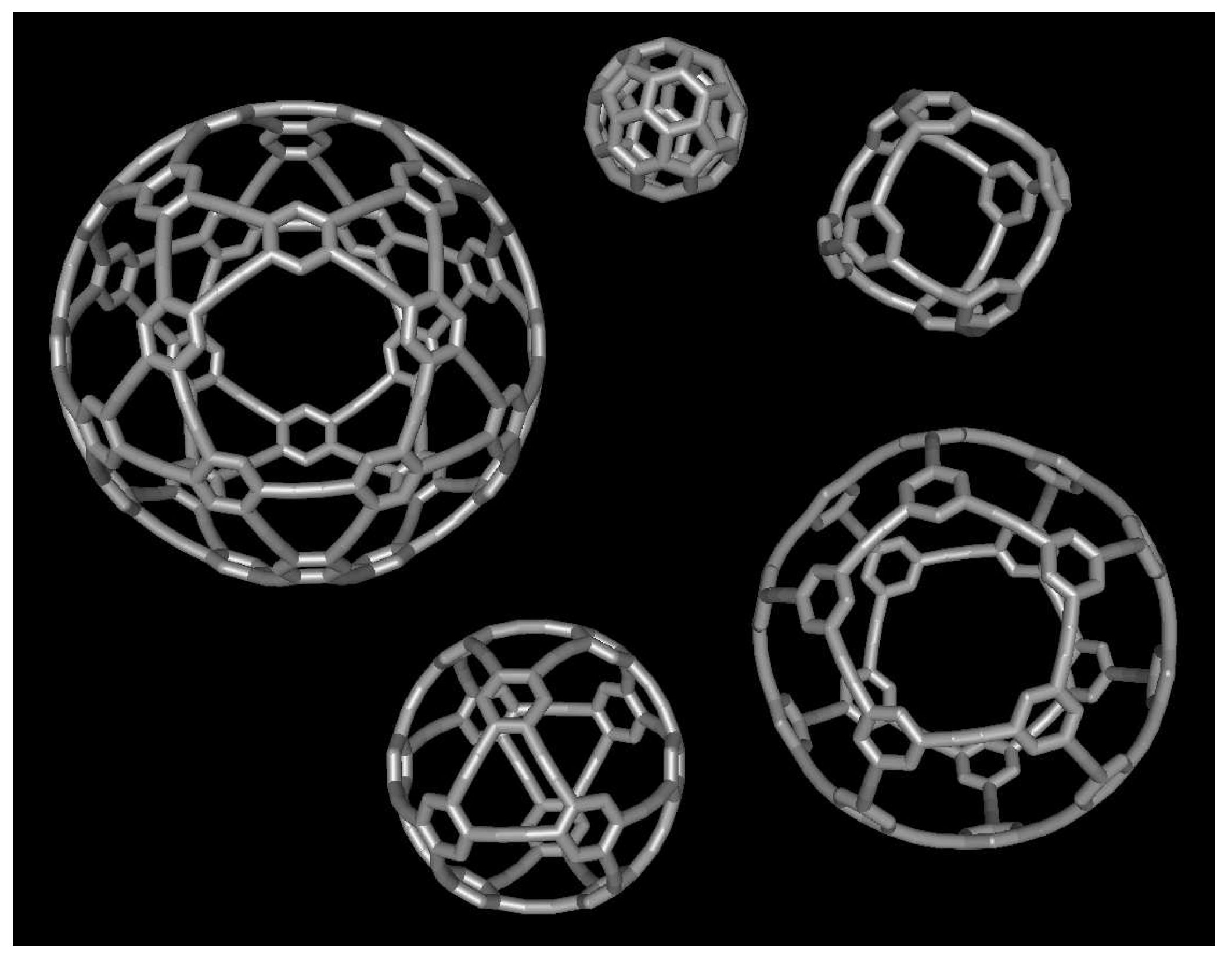



| Polyhedron designation (name) | Vertices (C6) | Edges (C2) | Molecular formula |
|---|---|---|---|
| P1 (tetrahedron) | 4 | 6 | C36H12 |
| P2 (cube) | 8 | 12 | C72H24 |
| P3 (octahedron) | 6 | 12 | – |
| P4 (dodecahedron) | 20 | 30 | C180H60 |
| P5 (icosahedron) | 12 | 30 | – |
| A1 (cuboctahedron) | 12 | 24 | C120H24 |
| A2 (great rhombicosidodecahedron) | 120 | 180 | C1080H360 |
| A3 (great rhombicuboctahedron) | 48 | 72 | C432H144 |
| A4 (icosidodecahedron) | 30 | 60 | C300H60 |
| A5 (small rhombicosidodecahedron) | 60 | 120 | – |
| A6 (small rhombicuboctahedron) | 24 | 48 | – |
| A7 (snub cube) | 24 | 60 | C264H24 |
| A8 (snub dodecahedron) | 60 | 150 | C660H60 |
| A9 (truncated cube) | 24 | 36 | C216H72 |
| A10 (truncated dodecahedron) | 60 | 90 | C540H180 |
| A11 (truncated icosahedron) | 60 | 90 | C540H180 |
| A12 (truncated octahedron) | 24 | 36 | C216H72 |
| A13 (truncated tetrahedron) | 12 | 18 | C108H36 |
| Polyhedron | Formation energy | Number of triple bonds | link |
|---|---|---|---|
| [kcal/mol per C] | topology | ||
| Graphyne sheet | 0.0 | n/a | ortho/meta/para |
| C | -4.31 | n/a | ortho/meta/para |
| PA trimer | 0.10 | 3 | ortho |
| PA hexamer | 0.71 | para | |
| PA decamer | 0.86 | ortho/meta/para | |
| P1 | 3.86 | meta | |
| P2 | 1.56 | meta | |
| P4 | 0.47 | meta | |
| A1 | 1.12 | ortho/meta/para | |
| A4 | 0.25 | ortho/meta/para |
© 2009 by the authors; licensee Molecular Diversity Preservation International, Basel, Switzerland. This article is an open-access article distributed under the terms and conditions of the Creative Commons Attribution license http://creativecommons.org/licenses/by/3.0/.
Share and Cite
Sebastiani, D.; Parker, M.A. Polyhedral Phenylacetylenes: The Interplay of Aromaticity and Antiaromaticity in Convex Graphyne Substructures. Symmetry 2009, 1, 226-239. https://doi.org/10.3390/sym1020226
Sebastiani D, Parker MA. Polyhedral Phenylacetylenes: The Interplay of Aromaticity and Antiaromaticity in Convex Graphyne Substructures. Symmetry. 2009; 1(2):226-239. https://doi.org/10.3390/sym1020226
Chicago/Turabian StyleSebastiani, Daniel, and Matt A. Parker. 2009. "Polyhedral Phenylacetylenes: The Interplay of Aromaticity and Antiaromaticity in Convex Graphyne Substructures" Symmetry 1, no. 2: 226-239. https://doi.org/10.3390/sym1020226
APA StyleSebastiani, D., & Parker, M. A. (2009). Polyhedral Phenylacetylenes: The Interplay of Aromaticity and Antiaromaticity in Convex Graphyne Substructures. Symmetry, 1(2), 226-239. https://doi.org/10.3390/sym1020226




