Factors Affecting Phosphorous in Groundwater in an Alluvial Valley Aquifer: Implications for Best Management Practices
Abstract
:1. Introduction
2. Materials and Methods
2.1. Study Site
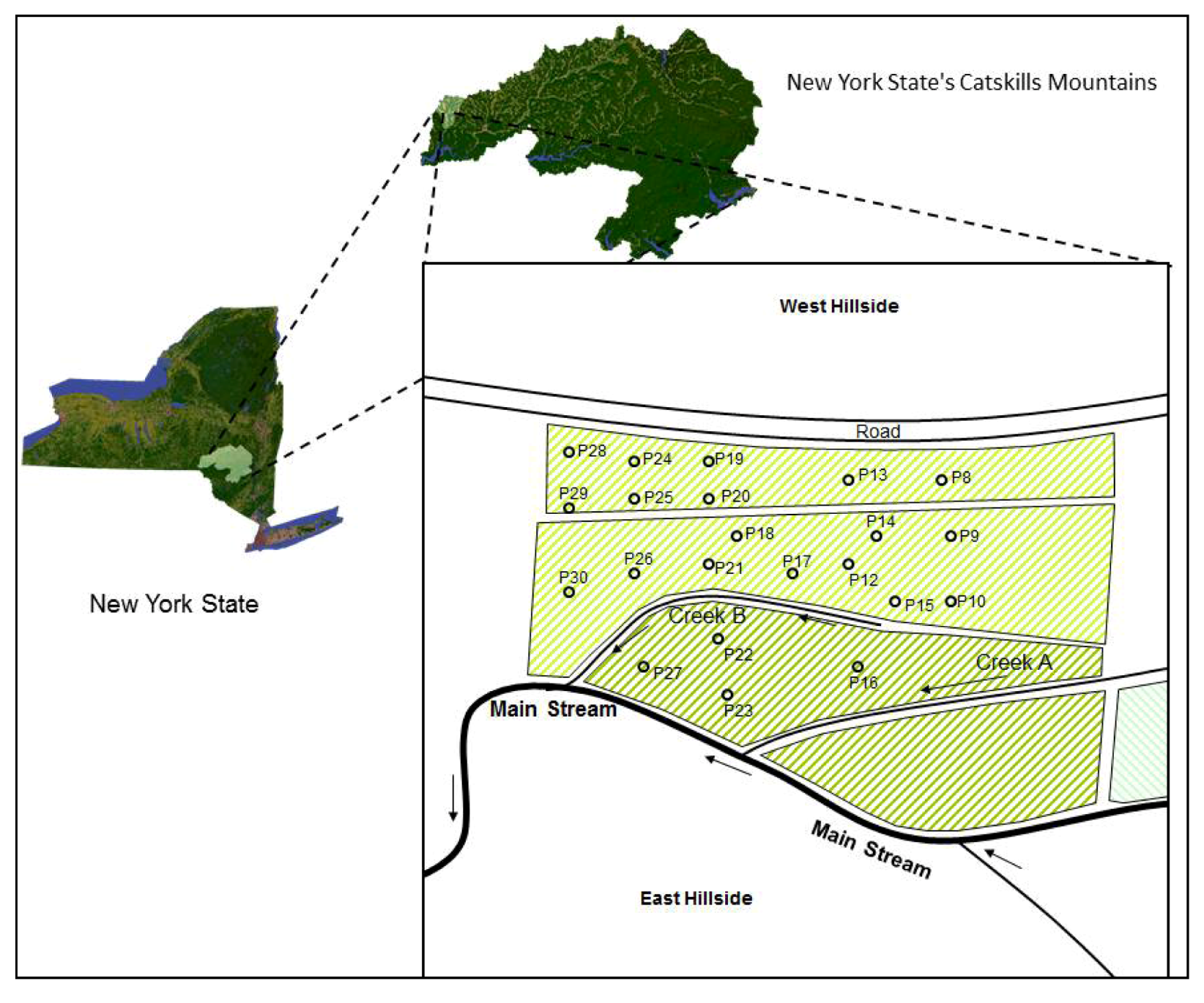
2.2. Groundwater Sampling
2.3. Stream Sampling
2.4. Water Chemistry Analysis
2.5. Modeling of Ground Water Flow and Streamlines
2.6. Spatial Analysis of Ground Water SRP
2.7. Predicting Temperature Effect on SRP Concentrations


 is the damping depth (m) described by Dt, the soil’s thermal diffusivity (m2 d−1) and ω = 2π/365 the radial frequency (d−1), Ta the annual average air temperature (°C), A0 is annual amplitude of the air temperature (°C), and t0 a lag time so that when t0 days have passed (starting at t = 0) the air temperature is equal to the average air temperature, i.e., T(t0, 0) = Ta.
is the damping depth (m) described by Dt, the soil’s thermal diffusivity (m2 d−1) and ω = 2π/365 the radial frequency (d−1), Ta the annual average air temperature (°C), A0 is annual amplitude of the air temperature (°C), and t0 a lag time so that when t0 days have passed (starting at t = 0) the air temperature is equal to the average air temperature, i.e., T(t0, 0) = Ta.3. Results and Discussion
3.1. Precipitation
3.2. Groundwater Flow and Streamlines
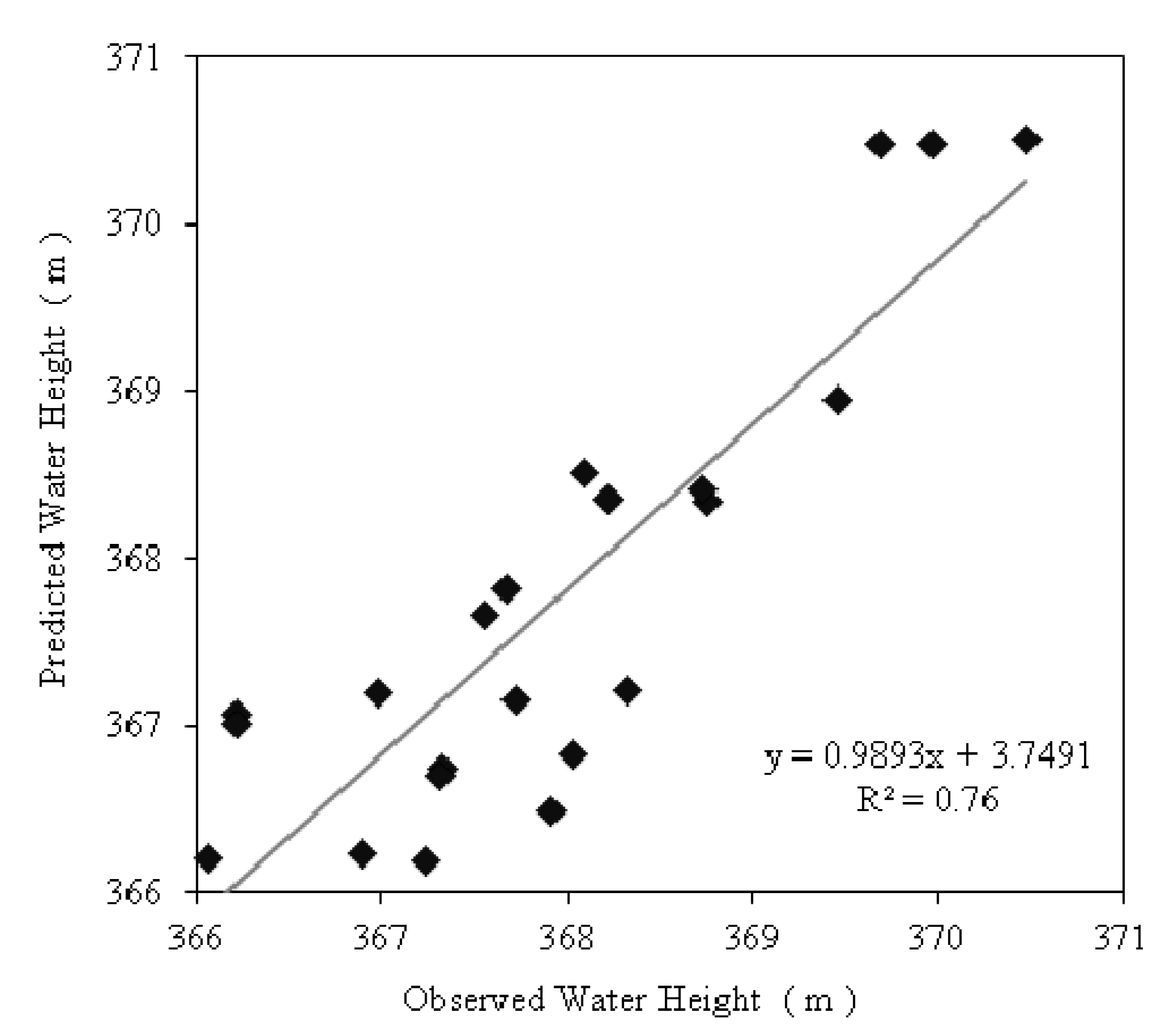
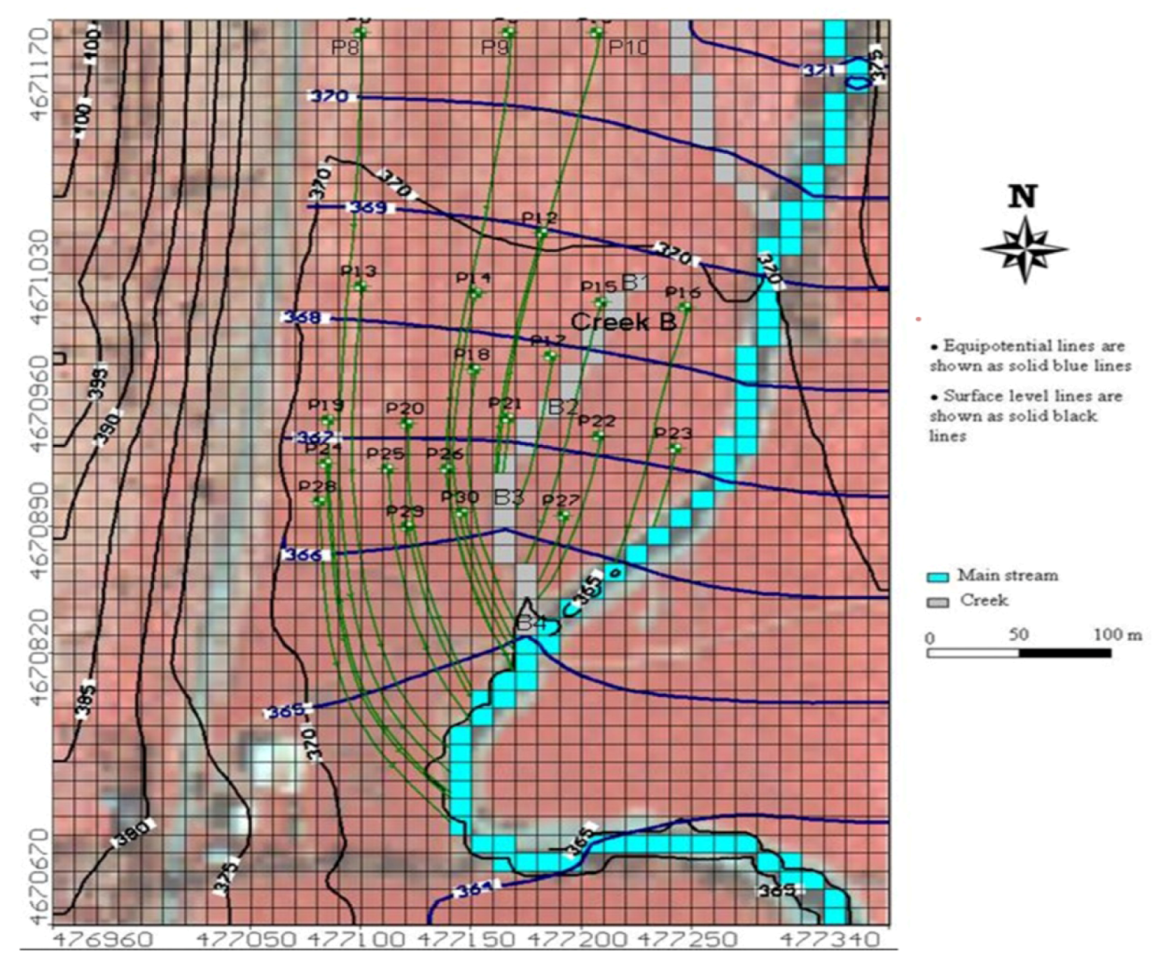
3.3. Groundwater and Stream Flow SRP Concentrations
| Season | Sites (n) | Samples (n) | Minimum SRP | Maximum SRP | Mean SRP | Median SRP | S.E. of Mean | Skewness |
|---|---|---|---|---|---|---|---|---|
| All Piezometers Placed in the Study Area | ||||||||
| Spring | 22 | 148 | 0.010 | 0.099 | 0.032 | 0.025 | 0.005 | 1.93 |
| Summer | 16 | 88 | 0.009 | 0.082 | 0.029 | 0.019 | 0.005 | 1.43 |
| Fall | 22 | 176 | 0.005 | 0.193 | 0.048 | 0.032 | 0.011 | 2.25 |
| Winter | 22 | 130 | 0.003 | 0.069 | 0.028 | 0.024 | 0.003 | 1.23 |
| Piezometers Feeding Creek B | ||||||||
| Spring | 9 | 59 | 0.014 | 0.075 | 0.028 | 0.019 | 0.009 | 2.56 |
| Summer | 7 | 38 | 0.014 | 0.082 | 0.034 | 0.020 | 0.011 | 1.11 |
| Fall | 9 | 72 | 0.005 | 0.186 | 0.051 | 0.035 | 0.019 | 2.15 |
| Winter | 9 | 55 | 0.003 | 0.062 | 0.026 | 0.022 | 0.006 | 1.75 |
| Creek B Stream Flow | ||||||||
| Spring | 4 | 31 | 0.008 | 0.175 | 0.024 | 0.014 | 0.005 | 4.52 |
| Summer | 4 | 42 | 0.006 | 0.239 | 0.035 | 0.028 | 0.006 | 4.73 |
| Fall | 4 | 47 | 0.002 | 0.397 | 0.046 | 0.015 | 0.011 | 2.97 |
| Winter | 4 | 26 | 0.002 | 0.161 | 0.044 | 0.027 | 0.009 | 1.47 |
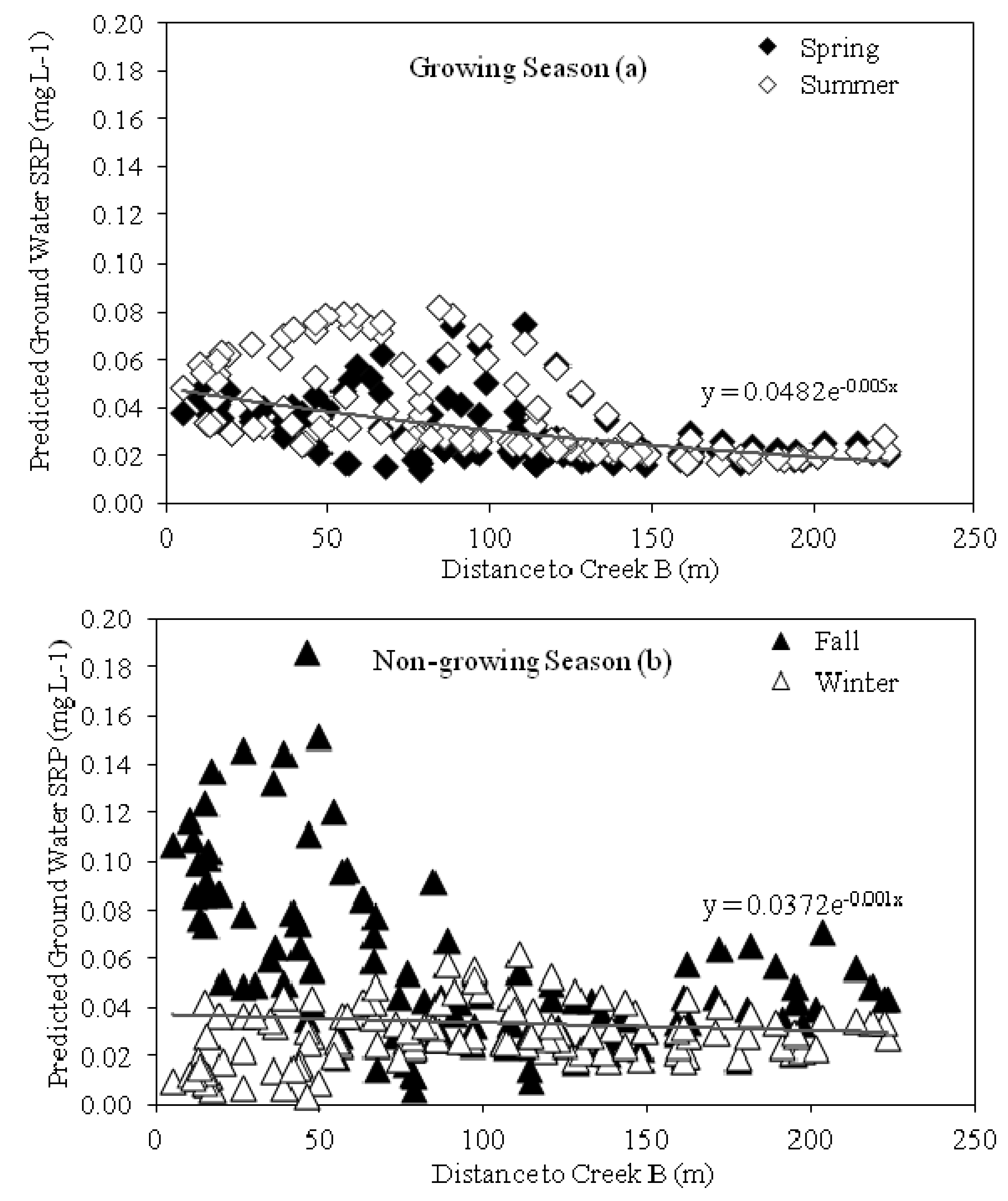
3.4. Spatial Variability of Groundwater SRP
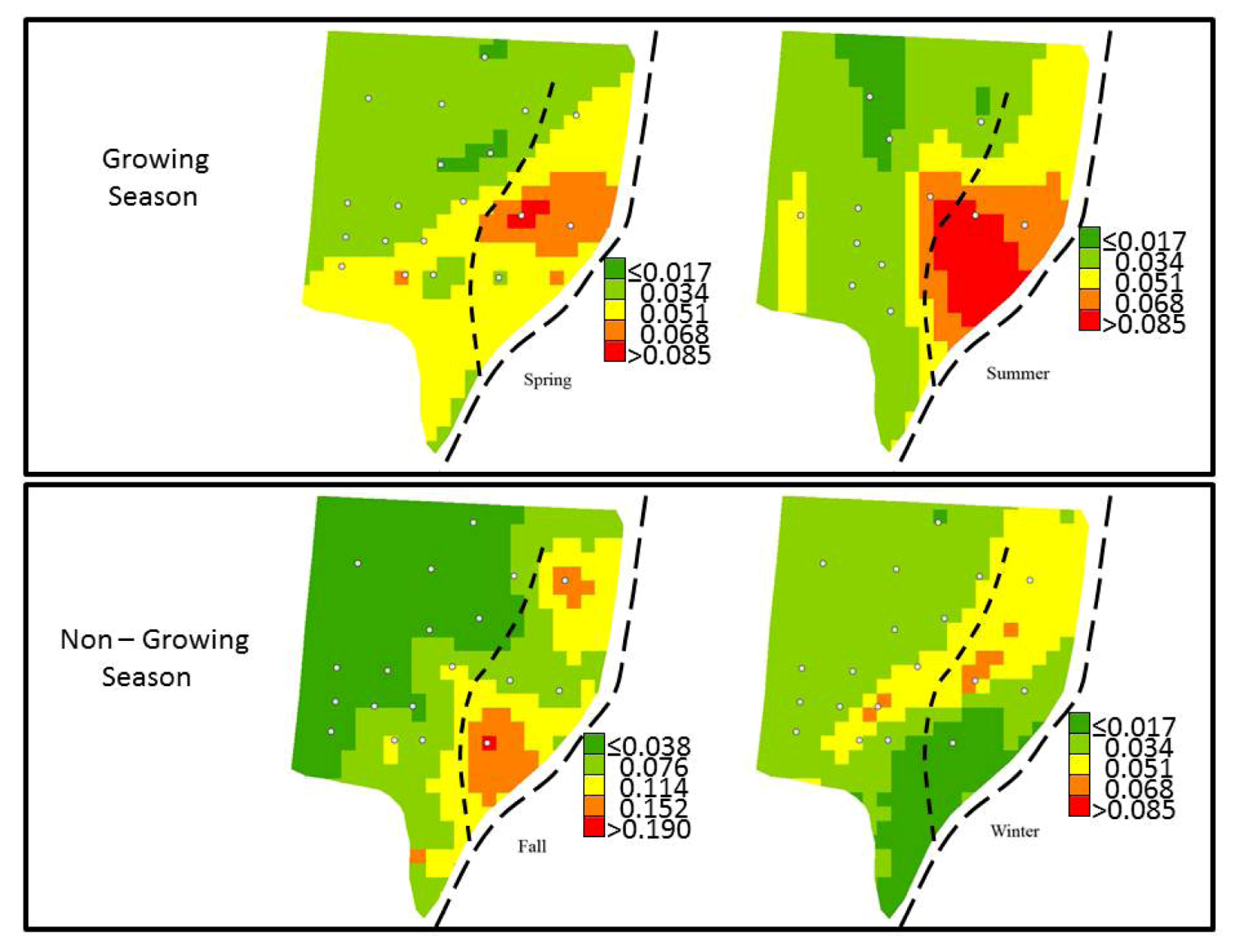
| Season | Mean Predicted SRP | Root-Mean-Square Error | Average Standard Error | Mean Standardized Error | Root-Mean-Square Standardized Error | |
|---|---|---|---|---|---|---|
| Spring | −0.00158 | 0.01970 | 0.02171 | −0.11570 | 0.9522 | |
| Summer | −0.00103 | 0.01451 | 0.02051 | −0.12730 | 0.7551 | |
| Fall | −0.00397 | 0.05156 | 0.06982 | −0.13210 | 0.8944 | |
| Winter | 0.00072 | 0.01473 | 0.02335 | −0.04036 | 0.7134 |

3.5. Relationship between Groundwater SRP Concentrations, Temperature and Groundwater Depth
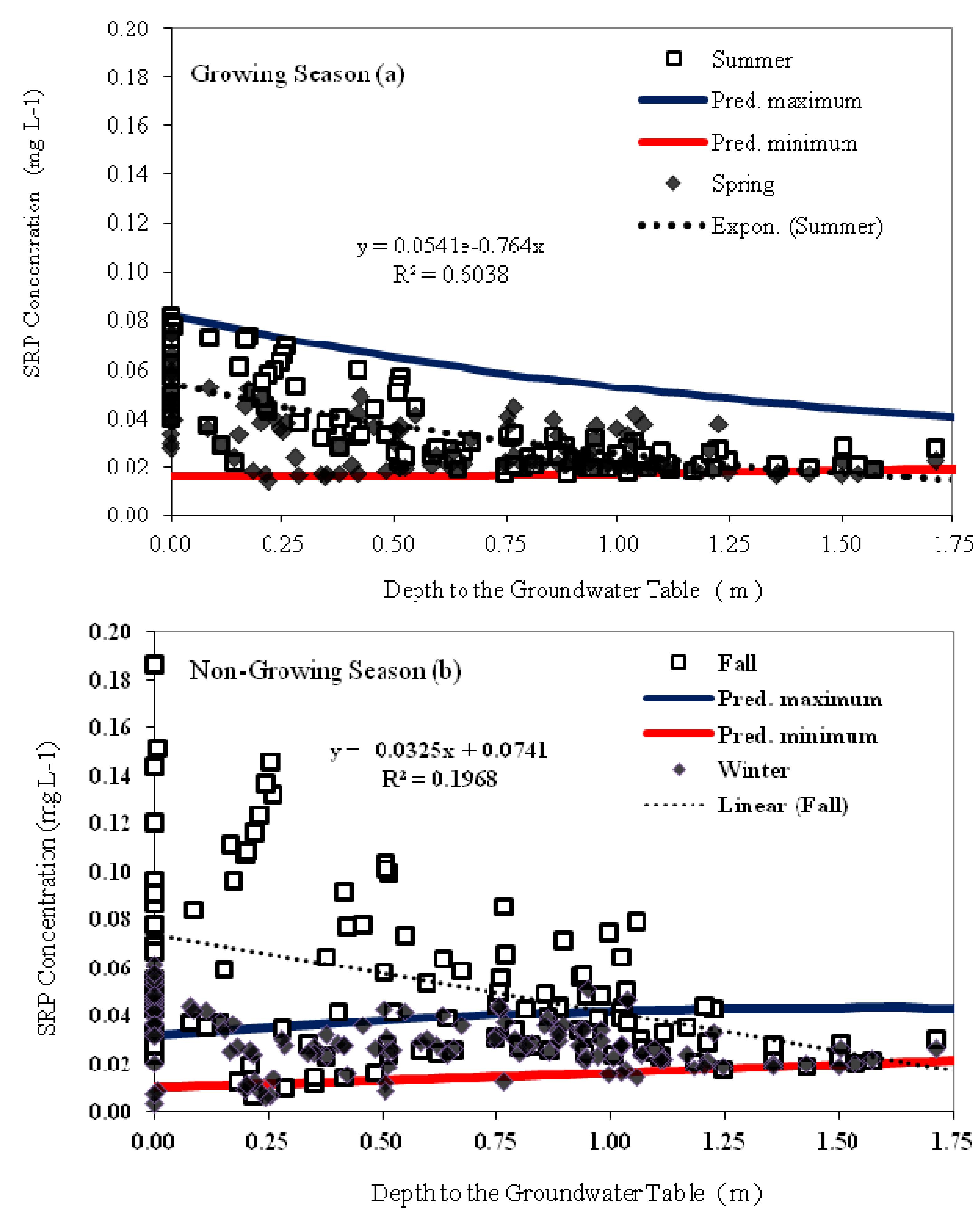
| Parameter | Parameter Description | Parameter Value | Unit |
|---|---|---|---|
| Cref | Reference concentration | 0.03 | mg L−1 |
| X | Factor change for a 10 °C change in temperature | 2.5 | - |
| Ta | Annual average air temperature | 7 | °C |
| ΔT | Annual amplitude | 12 | °C |
| tϕ | Time lag from 1 January | 120 | d |
| DT | Thermal diffusivity | 0.04 | m2/day |
3.6. Best Management Practices
4. Conclusions
Acknowledgements
References
- Puckett, L.J. Identifying the major sources of nutrient water pollution. Environ. Sci. Technol. 1995, 29, 408A–414A. [Google Scholar]
- Sharpley, A.N.; McDowell, R.W.; Weld, J.L.; Kleinman, P.J.A. Assessing site vulnerability to phosphorus loss in an agricultural watershed. J. Environ. Qual. 2001, 30, 2026–2036. [Google Scholar] [CrossRef]
- Domagalski, J.L.; Ator, S.; Coupe, R.; McCarthy, K.; Lampe, D.; Sandstrom, M.; Baker, N. Comparative study of transport processes of nitrogen, phosphorus, and herbicides to streams in five agricultural basins, USA. J. Environ. Qual. 2008, 37, 1158–1169. [Google Scholar] [CrossRef]
- Dingman, S.L. Physical Hydrology, 2nd ed; Prentice Hall: Upper Saddle River, NJ, USA, 2002. [Google Scholar]
- Gburek, W.J.; Sharpley, A.N. Hydrologic controls on phosphorus loss from upland agricultural watersheds. J. Environ. Qual. 1998, 27, 267–277. [Google Scholar] [CrossRef]
- Schilling, K.E.; Jacobson, P. Groundwater nutrient concentrations near an incised midwestern stream: Effects of floodplain lithology and land management. Biogeochemistry 2008, 87, 199–216. [Google Scholar] [CrossRef]
- Sharpley, A.N.; Chapra, S.; Wedepohl, R.; Sims, J.T.; Daniel, T.C.; Reddy, K. Managing agricultural phosphorus for protection of surface waters: Issues and options. J. Environ. Qual. 1994, 23, 437–451. [Google Scholar]
- Steenhuis, T.S.; Bodnar, M.; Geohring, L.D.; Aburime, S.-A.; Wallach, R. A simple model for predicting solute concentration in agricultural tile lines shortly after application. Hydrol. Earth Syst. Sci. Discuss. 1997, 1, 823–833. [Google Scholar] [CrossRef]
- United States Environmental Protection Agency (USEPA), National Water Quality Inventory: Report to Congress; USEPA: Washington, DC, USA, 2009.
- Laboski, C.A.M.; Lamb, J.A. Impact of manure application on soil phosphorus sorption characteristics and subsequent water quality implications. Soil Sci. 2004, 169, 440–448. [Google Scholar]
- New York State Department of Environmental Conservation (NYSDEC), New York State Fact Sheet: Ambient Water Quality Value for Protection of Recreational Uses; Bureau of Technical Services and Research: Albany, NY, USA, 1993.
- Flores-López, F.; Easton, Z.M. A multivariate analysis of covariance to determine the effects of near-stream best management practices on nitrogen and phosphorus concentrations on a dairy farm in the New York Conservation Effects Assessment Project watershed. J. Soil. Water Conserv. 2010, 65, 438–449. [Google Scholar] [CrossRef]
- Kleinman, P.J.A.; Allen, A.L.; Needelman, B.A.; Sharpley, A.N.; Vadas, P.A.; Saporito, L.S.; Folmar, G.J.; Bryant, R.B. Dynamics of phosphorus transfers from heavily manured coastal plain soils to drainage ditches. J. Soil. Water Conserv. 2007, 62, 225–235. [Google Scholar]
- Murray, T.P. Evaluating Level-Lip Spreader Vegetative Filter Strips in Removing Phosphorus from Milkhouse Waste in New York City’s Water Supply Watersheds. Master Thesis, Cornell University, Ithaca, NY, USA, 2001. [Google Scholar]
- Kim, Y.J.; Geohring, L.D.; Jeon, J.H.; Collick, A.S.; Giri, S.K.; Steenhuis, T.S. Evaluation of the effectiveness of vegetative filter strips for phosphorus removal with the use of a tracer. J. Soil Water Conserv. 2006, 61, 293–302. [Google Scholar]
- Bishop, P.L.; Hively, W.D.; Stedinger, J.R.; Rafferty, M.R.; Lojpersberger, J.L.; Bloomfield, J.A. Multivariate analysis of paired watershed data to evaluate agricultural best management practice effects on stream water phosphorus. J. Environ. Qual. 2005, 34, 1087–1101. [Google Scholar] [CrossRef]
- Brannan, K.M.; Mostaghimi, J.A.; Mcclellan, P.W.; Inamdar, S. Animal waste BMP impacts on sediment and nutrient losses in runoff from the Owl Run watershed. Trans. ASAE 2000, 43, 1155–1166. [Google Scholar]
- Easton, Z.M.; Walter, M.T.; Steenhuis, T.S. Combined monitoring and modeling indicate the most effective agricultural best management practices. J. Environ. Qual. 2008, 37, 1798–1809. [Google Scholar] [CrossRef]
- Gitau, M.W.; Veith, T.L.; Gburek, W.J. Farm-level optimization of BMP placement for cost-effective pollution reduction. Trans. ASAE 2004, 47, 1923–1931. [Google Scholar]
- Inamdar, S.P.; Mostaghimi, S.; Mcclellan, P.W.; Brannan, K.M. BMP impacts on sediment and nutrient yields from an agricultural watershed in the coastal plain region. Trans. ASAE 2001, 44, 1191–1200. [Google Scholar]
- Lee, K.-H.; Isenhart, T.M.; Schultz, R.C.; Mickelson, S.K. Multispecies riparian buffers trap sediment and nutrients during rainfall simulations. J. Environ. Qual. 2000, 29, 1200–1205. [Google Scholar]
- Heathwaite, A.; Dils, R. Characterising phosphorus loss in surface and subsurface hydrological pathways. Sci. Total Environ. 2000, 251–252, 523–538. [Google Scholar]
- Brodie, J.E.; Mitchell, A.W. Nutrients in Australian tropical rivers: Changes with agricultural development and implications for receiving environments. Mar. Freshw. Res. 2005, 56, 279–302. [Google Scholar] [CrossRef]
- Rasiah, V.; Moody, P.W.; Armour, J.D. Soluble phosphate in fluctuating groundwater under cropping in the north-eastern wet tropics of Australia. Soil Res. 2011, 49, 329–342. [Google Scholar] [CrossRef]
- Stedinger, J.R.; Vogel, R.M.; Foufoula-Georgiou, E. Frequency analysis of extreme events. In Handbook of Hydrology; Maidment, D.R., Ed.; McGraw-Hill, Inc.: New York, NY, USA, 1993; Chapter 18; pp. 18.11–18.66. [Google Scholar]
- Spalding, R.F.; Exner, M.E. Occurrence of nitrate in groundwater: A review. J. Environ. Qual. 1993, 22, 392–402. [Google Scholar] [CrossRef]
- Wayland, K.G.; Hyndman, D.W.; Boutt, D.; Pijanowski, B.C.; Long, D.T. Modelling the impact of historical land uses on surface-water quality using groundwater flow and solute-transport models. Lakes Reserv. 2002, 7, 189–199. [Google Scholar]
- Obour, A.K.; Silveira, M.L.; Vendramini, J.M.B.; Sollenberger, L.E.; O’Connor, G.A. Fluctuating water table effect on phosphorus release and availability from a Florida Spodosol. Nutr. Cycl. Agroecosyst. 2011, 91, 207–217. [Google Scholar] [CrossRef]
- Martin, H.W.; Ivanoff, D.B.; Graetz, D.A.; Reddy, K.R. Water table effects on histosol drainage water carbon, nitrogen, and phosphorus. J. Environ. Qual. 1997, 26, 1062–1071. [Google Scholar]
- Scott, C.A.; Walter, M.F.; Nagle, G.N.; Walter, M.T.; Sierra, N.V.; Brooks, E.S. Residual phosphorus in runoff from successional forest on abandoned agricultural land: 1. Biogeochemical and hydrological processes. Biogeochemistry 2001, 55, 293–310. [Google Scholar] [CrossRef]
- Duan, S.; Kaushal, S.S.; Groffman, P.M.; Band, L.E.; Belt, K.T. Phosphorus export across an urban to rural gradient in the Chesapeake Bay watershed. J. Geophys Res. Biogeosci. 2012, 117, G01025. [Google Scholar] [CrossRef]
- Lutz, B.D.; Mulholland, P.J.; Bernhardt, E.S. Long-term data reveal patterns and controls on stream water chemistry in a forested stream: Walker Branch, Tennessee. Ecol. Monographs 2012, 82, 367–387. [Google Scholar] [CrossRef]
- Hively, W.D.; Bryant, R.B.; Fahey, T.J. Phosphorus concentrations in overland flow from diverse locations on a New York dairy farm. J. Environ. Qual. 2005, 34, 1224–1233. [Google Scholar] [CrossRef]
- Bailey, J.E.; Ollis, D.F. Biochemical Engineering Fundamentals; McGraw-Hill Inc.: New York, NY, USA, 1986. [Google Scholar]
- Frossard, E.; Condron, L.M.; Oberson, A.; Sinaj, S.; Fardeau, J.C. Process governing phosphorus availability in temperate soils. J. Environ. Qual. 2000, 34, 15–23. [Google Scholar]
- Hansen, N.C.; Daniel, T.C.; Sharpley, A.N.; Lemunyon, J.L. The fate and transport of phosphorus in agricultural systems. J. Soil Water Conserv. 2002, 57, 408–417. [Google Scholar]
- Blecken, G.T.; Zinger, Y.; Deletic, A.; Fletcher, T.D.; Hedstrom, A.; Viklander, M. Laboratory study on stormwater biofiltration Nutrient and sediment removal in cold temperatures. J. Hydrol. 2010, 394, 507–514. [Google Scholar] [CrossRef]
- Bunnell, F.L.; Tait, D.E.N.; Flanagan, P.W.; van Cleve, K. Microbial respiration and substrate weight loss. I. A general model of the influences of abiotic variables. Soil Biol. Biochem. 1977, 9, 33–40. [Google Scholar] [CrossRef]
- Clesceri, N.L.; Curran, S.J.; Sedlak, R.L. Nutrient loads to Wisconsin lakes: Part I. Nitrogen and phosphorus export coefficients. Water Resour. Bull. 1986, 22, 983–990. [Google Scholar] [CrossRef]
- Hanrahan, G.; Gledhill, M.; House, W.A.; Worsfold, P.J. Phosphorus loading in the Frome catchment, UK: Seasonal refinement of the coefficient modeling approach. J. Environ. Qual. 2001, 30, 1738–1746. [Google Scholar] [CrossRef]
- Johnsson, H.; Bergstrom, L.; Jansson, P.E. Simulated nitrogen dynamics and losses in a layered agricultural soil. Agric. Ecosyst. Environ. 1987, 18, 333–356. [Google Scholar] [CrossRef]
- Sharpley, A.N.; Kleinman, P.J.A.; McDowell, R.W.; Gitau, M.; Bryant, R.B. Modeling phosphorus transport in agricultural watersheds: Processes and possibilities. J. Soil Water Conserv. 2002, 57, 425–439. [Google Scholar]
- Kuo, W.L. Spatial and Temporal Analysis of Soil Water and Nitrogen Distribution in Undulating Landscapes Using a GIS-Based Model; Cornell University Press: Ithaca, NY, USA, 1998. [Google Scholar]
- Flores-Lopez, F.; Easton, Z.M.; Geohring, L.D.; Steenhuis, T.S. Factors affecting dissolved phosphorus and nitrate concentrations in ground and surface water for a valley dairy farm in the northeastern United States. Water Environ. Res. 2011, 83, 116–127. [Google Scholar] [CrossRef]
- Soren, J. The Ground-Water Resources of Delaware County, New York; U.S. Bulletin GW-50; New York State Water Resources Commission: Albany, NY, USA, 1963.
- National Climatic Data Center (NCDC), Climatological Data Annual Summary-New York; NCDC: Asheville, NC, USA, 2000.
- Method 4500-P G (Ortho-P) and Method 4500-Ph (Total P). In Apha/Awwa/Wef.: Standard Methods for the Examination of Water and Wastewater; American Public Health Association: Washington, DC, USA, 1999.
- Environmental Systems Research Institute (ESRI), ArcGIS; ESRI: Redlands, CA, USA, 2007.
- Hively, W.D.; Gérard-Marchant, P.; Steenhuis, T.S. Distributed hydrological modeling of total dissolved phosphorus transport in an agricultural landscape, part II: Dissolved phosphorus transport. Hydrol. Earth Sys. Sci. Discuss. 2006, 10, 263–276. [Google Scholar] [CrossRef]
- De Vries, D.A. Thermal properties of soils. In Physics of Plant Environment; van Wijk, W., Ed.; North Holland Pub. Co.: Amsterdam, NL, USA, 1963; pp. 210–235. [Google Scholar]
- Brutsaert, W. Evaporation into the Atmosphere; D. Reidel Publishing Co.: Boston, MA, USA, 1982. [Google Scholar]
- Young, E.O.; Briggs, R.D. Phosphorus concentrations in soil and subsurface water: A field study among cropland and riparian buffers. J. Environ. Qual. 2008, 37, 69–78. [Google Scholar] [CrossRef]
- Carlyle, G.C.; Hill, A.R. Groundwater phosphate dynamics in a river riparian zone: Effects of hydrologic flowpaths, lithology and redox chemistry. J. Hydrol. 2001, 247, 151–168. [Google Scholar] [CrossRef]
- McDowell, R.; Trudgill, S. Variation of phosphorus loss from a small catchment in south Devon, UK. Agric. Ecosyst. Environ. 2000, 79, 143–157. [Google Scholar] [CrossRef]
- Neal, C.; Reynolds, B.; Neal, M.; Hughes, S.; Wickham, H.; Hill, L.; Rowland, P.; Pugh, B. Soluble reactive phosphorus levels in rainfall, cloud water, throughfall, stemflow, soil waters, stream waters and groundwaters for the Upper River Severn area, Plynlimon, mid Wales. Sci. Total Environ. 2003, 314–316, 99–120. [Google Scholar] [CrossRef]
- Mulholland, P.J.; Hill, W.R. Seasonal patterns in streamwater nutrient and dissolved organic carbon concentrations: Separating catchment flow path and in-stream effects. Water Resour. Res. 1997, 33, 1297–1306. [Google Scholar] [CrossRef]
- Correll, D.L.; Jordan, T.E.; Weller, D.E. Effects of precipitation and air temperature on phosphorus fluxes from rhode river watersheds. J. Environ. Qual. 1999, 28, 144–154. [Google Scholar] [CrossRef]
- Duan, S.; Amon, R.; Bianchi, T.S.; Santschi, P.H. Temperature control on soluble reactive phosphorus in the lower Mississippi river? Estuaries Coasts 2011, 34, 78–89. [Google Scholar] [CrossRef]
- Geohring, L.D.; McHugh, O.V.; Walter, M.T.; Steenhuis, T.S.; Akhtar, M.S.; Walter, M.F. Phosphorus transport into subsurface drains by macropores after manure applications: Implications for best manure management practices. Soil Sci. J. 2001, 166, 896–909. [Google Scholar]
- Zhang, W.; Faulkner, J.W.; Giri, S.K.; Geohring, L.D.; Steenhuis, T.S. Effect of soil reduction on phosphorus sorption of an organic-rich silt loam. Soil Sci. Soc. Am. J. 2010, 74, 240–249. [Google Scholar]
- Dittrich, T.M.; Geohring, L.D.; Walter, M.T.; Steenhuis, T.S. Revisiting Buffer Strip Design Standards for Removing Dissolved and Particulate Phosphorus. In Proceedings of Total Maximum Daily Load (TMDL) Environmental Regulations–II Conference, Albuquerque, NM, USA, 8–12 November 2003; 2003; pp. 527–534. [Google Scholar]
- James, E.; Kleinman, P.; Veith, T.; Stedman, R.; Sharpley, A. Phosphorus contributions from pastured dairy cattle to streams of the Cannonsville Watershed, New York. J. Soil. Water Conserv. 2007, 62, 40–47. [Google Scholar]
© 2013 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Flores-López, F.; Easton, Z.M.; Geohring, L.D.; Vermeulen, P.J.; Haden, V.R.; Steenhuis, T.S. Factors Affecting Phosphorous in Groundwater in an Alluvial Valley Aquifer: Implications for Best Management Practices. Water 2013, 5, 540-559. https://doi.org/10.3390/w5020540
Flores-López F, Easton ZM, Geohring LD, Vermeulen PJ, Haden VR, Steenhuis TS. Factors Affecting Phosphorous in Groundwater in an Alluvial Valley Aquifer: Implications for Best Management Practices. Water. 2013; 5(2):540-559. https://doi.org/10.3390/w5020540
Chicago/Turabian StyleFlores-López, Francisco, Zachary M. Easton, Larry D. Geohring, Peter J. Vermeulen, Van R. Haden, and Tammo S. Steenhuis. 2013. "Factors Affecting Phosphorous in Groundwater in an Alluvial Valley Aquifer: Implications for Best Management Practices" Water 5, no. 2: 540-559. https://doi.org/10.3390/w5020540
APA StyleFlores-López, F., Easton, Z. M., Geohring, L. D., Vermeulen, P. J., Haden, V. R., & Steenhuis, T. S. (2013). Factors Affecting Phosphorous in Groundwater in an Alluvial Valley Aquifer: Implications for Best Management Practices. Water, 5(2), 540-559. https://doi.org/10.3390/w5020540






