Definition of Exergetic Efficiency in the Main and Emerging Thermal Desalination Technologies: A Proposal
Abstract
1. Introduction
2. Literature Review
2.1. Multieffect Distillation
| Type of Process | Operating Conditions | Chemical Exergy | Exergy Efficiency | Ref. |
|---|---|---|---|---|
| MED | Seawater (37,000 ppm) | [26] | ||
| Temperature (°C): 75 | ||||
| Effects (n°): 8 | ||||
| MSF-MED | Seawater (45,000 ppm) | [32] | ||
| Temperature (°C): 60 | ||||
| Effects (n°): 12 | ||||
| MED | Seawater | [27] | ||
| Temperature (°C): 66 | ||||
| Effects (n°): 14 | ||||
| MED-TVC | Seawater (42,000 ppm) | [33] | ||
| Temperature (°C): 92 | ||||
| Effects (n°): 6 | ||||
| MED | Seawater (36,000 ppm) | [29] | ||
| Temperature (°C): 60 | ||||
| Effects (n°): 5 | ||||
| MED-TVC | Seawater (40,000 ppm) | [34] | ||
| Temperature (°C): 60 | ||||
| Effects (n°): 12 | ||||
| MED-TVC | Seawater (45,000 ppm) | [28] | ||
| Temperature (°C): 65 | ||||
| Effects (n°): 4 | ||||
| MED-MCV | Seawater (42,000 ppm) | [35] | ||
| Temperature (°C): 100 | ||||
| Effects (n°): 10 | ||||
| MED-TVC | Seawater (45,000 ppm) | [36] | ||
| Temperature (°C): 60 | ||||
| Effects (n°): 4 |
2.2. Multistage Flash Distillation
2.3. Membrane Distillation
| Type of Process | Operating Conditions | Chemical Exergy | Exergy Efficiency | Reference |
|---|---|---|---|---|
| AGMD | Seawater (40,000 ppm) | [46] | ||
| Temperature (°C): 66–35 | ||||
| Flux (kg/s): | ||||
| DCMD | Seawater (35,000 ppm) | [48] | ||
| Temperature (°C): 70–15 | ||||
| Flux (kg/s): 0.027 | ||||
| DCMD | Seawater (30,000 ppm) | [47] | ||
| Temperature (°C): 65–22 | ||||
| Flux (kg/s): 0.002 | ||||
| VMD | Seawater (35,000 ppm) | [49] | ||
| Temperature (°C): 80–77 | ||||
| Flux (kg/s): 0.02 | ||||
| DCMD | Seawater (37,000 ppm) | - | [50] | |
| Temperature (°C): 70–31 | ||||
| Flux (kg/s): | ||||
| DCMD | Tap water (2000 ppm) | - | [51] | |
| Temperature (°C): 80–25 | ||||
| Flux (kg/s): | ||||
| AGMD | Seawater | [52] | ||
| Temperature (°C): 55–27 | ||||
| Flux (kg/s): | ||||
| AGMD | Seawater (35,000 ppm) | [53] | ||
| Temperature (°C): 62–29 | ||||
| Flux (kg/s): | ||||
| DCMD | Seawater (35,000 ppm) | [54] | ||
| Temperature (°C): 77–25 | ||||
| Flux (kg/s): 0.089 | ||||
| MSVMD-MVC | Seawater (113,800 ppm) | [55] | ||
| Temperature (°C): 50–25 | ||||
| Flux (kg/s): 0.061 |
3. Case Studies
3.1. MED-TVC Desalination Plant
3.2. MSF Desalination Plant
3.3. Membrane Distillation Plant Powered by Solar Thermal System
4. Exergy Analysis
Exergy Efficiency
- a.
- Inlet–outlet efficiency.Kotas [63], under the name of rational efficiency, defined exergy efficiency as the ratio between the exergy transformations that constitute the output and the input of the system. Gundersen [64] defined exergy efficiency as the ratio between the useful exergy produced by the system and the total exergy of the system. Rosen and Dinçer [8] defined exergy efficiency as the ratio between the product exergy at the output of the system and the exergy at the input. Considering those definitions, the exergy balance can be expressed asAs before, the ingoing and outgoing exergy quantities may include work, heat, and cooling, with or without mass flows. The overall or total exergetic efficiency, , should be, consequently, defined as
- b.
- Consumed–produced efficiency.The exergy supplied to the system is either delivered in the outputs or destroyed inside the system. This balance can also be expressed in terms of exergy of the fuel (the exergy of the resources), ; exergy of the product (the desired exergy output), ; exergy loss (with effluents), ; or exergy destroyed (within the system due to irreversibilities), .The terms product exergy and fuel or consumption exergy have been widely used in the literature by several authors, with slight differences [15,61,65,66,67], often indicated as the most appropriate definition for exergy efficiency. Szaargut et al. and Tsatsaronis et al. [12,14] proposed taking into account those exergy transfers that were used in the production of the desired exergy from the driving exergy of the system, for which a coherence between the purpose of the system and its exergy analysis is necessary, giving rise to the concepts of fuel exergy and product exergy. In addition, Lazaretto and Tsatsaronis [68] proposed a systematic procedure for the definition of the exergy efficiency for process components.Based on the common energy efficiency definition as the ratio between the product that is obtained (work, heat, refrigeration) and the resources that must be “paid” for, the consumed–produced exergetic efficiency could be defined as the ratio between the useful exergy outputs and the paid exergy inputs [15].
5. Results and Discussion
6. Conclusions
- The use of the thermodynamic properties of seawater from Sharqawy et al. [23] in exergy analysis, as they seem to better represent the thermodynamic behaviour of seawater.
- The consideration of the consumed–produced exergy approach for analysis, which allows one to focus on the desired product of thermal desalination processes and therefore to better describe the final objective of this type of process.
- The taking into account of the increase in chemical exergy in the definition of the exergy efficiency of the process components in which salt separation takes place.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Abbreviations | Description |
| DCMD | Direct-contact membrane distillation |
| AGMD | Air-gap membrane distillation |
| VMD | Vacuum membrane distillation |
| MD | Membrane distillation |
| MED | Multieffect distillation |
| MSF | Multistage flash distillation |
| MVC | Mechanical vapour compression |
| TVC | Thermal vapour compression |
| Symbols | Description |
| cp | Specific heat [kJ/kg °C] |
| E | Exergy [kW] |
| e | Specific exergy [kJ/kg] |
| I | Solar radiation [W/m2] |
| N | Number of moles [kmol or mol] |
| m | Mass flow rate [kg/s] |
| h | Specific enthalpy [kJ/kg] |
| s | Specific entropy [kJ/kg K] |
| p | Pressure [bar] |
| ppm | Parts per million |
| MW | Molar mass [kg/mol] |
| R | Universal constant of gases [kJ/kmol K] |
| T | Temperature [°C or K] |
| W | Mechanical work [kJ] |
| Minimum work of separation [kW] | |
| m | Mass flow rate [kg/s] |
| u | Chemical potential [kJ/kmol] |
| x | Mole fraction |
| w | Mass fraction |
| Exergy efficiency [%] | |
| Dissociation factor of the salts | |
| Subscripts | Description |
| i | Related to chemical species |
| 0 | Dead state |
| D | Destruction |
| F | Fuel |
| L | Loss |
| P | Product |
| no dissoc | Not accounting for the ionic dissociation of salts |
| in | Input |
| out | Output |
| hot | Hot stream |
| cold | Cold stream |
| sol | Solar |
| t | Total |
| s | Salt, saline water |
| w | Pure water |
| Superscripts | Description |
| CH | Chemical |
| KN | Kinetic |
| PH | Physical |
| PT | Potential |
References
- UN-Water. The United Nations World Water Development Report 2020: Water and Climate Change; Technical Report; UNESCO: Paris, France, 2020. [Google Scholar]
- UN-Water. United Nations World Water Development Report 2021: The Value of Water; Technical Report; UNESCO: Paris, France, 2021. [Google Scholar]
- Najim, A. A review of advances in freeze desalination and future prospects. npj Clean. Water. 2022, 5, 15. [Google Scholar] [CrossRef]
- Jones, E.; Qadir, M.; van Vliet, M.T.; Smakhtin, V.; Kang, S.m. The state of desalination and brine production: A global outlook. Sci. Total Environ. 2019, 657, 1343–1356. [Google Scholar] [CrossRef] [PubMed]
- Gude, V.G. Energy storage for desalination processes powered by renewable energy and waste heat sources. Appl. Energy 2015, 137, 877–898. [Google Scholar] [CrossRef]
- Nassrullah, H.; Anis, S.F.; Hashaikeh, R.; Hilal, N. Energy for desalination: A state-of-the-art review. Desalination 2020, 491, 114569. [Google Scholar] [CrossRef]
- Stillwell, A.S.; Webber, M.E. Predicting the specific energy consumption of reverse osmosis desalination. Water 2016, 8, 601. [Google Scholar] [CrossRef]
- Rosen, M.A. Benefits of exergy and needs for increased education and public understanding and applications in industry and policy—Part II: Needs. Int. J. Exergy 2006, 3, 219. [Google Scholar] [CrossRef]
- Grassmann, P. Zur allgemeinen Definition des Wirkungsgrades. Chem. Ing. Tech. 1950, 22, 77–80. [Google Scholar] [CrossRef]
- Baehr, H. Zur Defintion Exergeticshe Wirkuksgrader (to the Definition of Exergetic Efficencies). Brennst.-Wärme-Kraft 1968, 20, 197–200. [Google Scholar]
- Brodyanski, V.M.; Sorin, M.V.; Le Goff, P. The Efficiency of Industrial Processes: Exergy Analysis and Optimization; Elsevier: Amsterdam, The Netherlands, 1994. [Google Scholar]
- Szargut, J.; Morris, D.; Steward, F. Exergy Analysis of Thermal, Chemical, and Metallurgical Processes; Hemisphere Publishing Corporation: New York, NY, USA, 1988. [Google Scholar]
- Kotas, T. The Exergy Method of Thermal Plant Analysis; Krieger Publishing Company: Malabar, FL, USA, 1995. [Google Scholar]
- Tsatsaronis, G.; Winhold, M. Thermoeconomic Analysis of Power Plants; Technical Report, EPRI AP-3651, RP 2029-8; U.S. Department of Energy, Office of Scientific Technical Information: Palo Alto, CA, USA, 1984. [Google Scholar]
- Bejan, A.; Tsatsaronis, G.; Moran, M.J. Thermal Design and Optimization; Wiley: Hoboken, NJ, USA, 1996; p. 542. [Google Scholar]
- Sciubba, E.; Wall, G. A brief Commented History of Exergy From the Beginnings to 2004. Int. J. Thermodyn. 2007, 10, 1–26. [Google Scholar]
- Lior, N.; Zhang, N. Energy, exergy, and Second Law performance criteria. Energy 2007, 32, 281–296. [Google Scholar] [CrossRef]
- Cornelissen, R. Thermodynamics and Sustainable Development: The Use of Exergy Analysis and the Reduction of Irreversibility; Febodruk: Enschede, The Netherlands, 1997. [Google Scholar]
- Gaggioli, R.A. The concept of available energy. Chem. Eng. Sci. 1961, 16, 87–96. [Google Scholar] [CrossRef]
- Tsatsaronis, G. Definitions and nomenclature in exergy analysis and exergoeconomics. Energy 2007, 32, 249–253. [Google Scholar] [CrossRef]
- Ahmed, F.E.; Khalil, A.; Hilal, N. Emerging desalination technologies: Current status, challenges and future trends. Desalination 2021, 517, 115183. [Google Scholar] [CrossRef]
- Fitzsimons, L.; Corcoran, B.; Young, P.; Foley, G. Exergy analysis of water purification and desalination: A study of exergy model approaches. Desalination 2015, 359, 212–224. [Google Scholar] [CrossRef]
- Sharqawy, M.H.; Lienhard V, J.H.; Zubair, S.M. On exergy calculations of seawater with applications in desalination systems. Int. J. Therm. Sci. 2011, 50, 187–196. [Google Scholar] [CrossRef]
- Cerci, Y. Exergy analysis of a reverse osmosis desalination plant in California. Desalination 2002, 142, 257–266. [Google Scholar] [CrossRef]
- García-Rodríguez, L.; Gómez-Camacho, C. Exergy analysis of the SOL-14 plant (Plataforma Solar de Almería, Spain). Desalination 2001, 137, 251–258. [Google Scholar] [CrossRef]
- Piacentino, A. Application of advanced thermodynamics, thermoeconomics and exergy costing to a Multiple Effect Distillation plant: In-depth analysis of cost formation process. Desalination 2015, 371, 88–103. [Google Scholar] [CrossRef]
- Carballo, J.A.; Bonilla, J.; Roca, L.; De la Calle, A.; Palenzuela, P.; Alarcón-Padilla, D.C. Optimal operating conditions analysis for a multi-effect distillation plant according to energetic and exergetic criteria. Desalination 2018, 435, 70–76. [Google Scholar] [CrossRef]
- Elsayed, M.L.; Mesalhy, O.; Mohammed, R.H.; Chow, L.C. Exergy and thermo-economic analysis for MED-TVC desalination systems. Desalination 2018, 447, 29–42. [Google Scholar] [CrossRef]
- Moghimi, M.; Emadi, M.; Mirzazade Akbarpoor, A.; Mollaei, M. Energy and exergy investigation of a combined cooling, heating, power generation, and seawater desalination system. Appl. Therm. Eng. 2018, 140, 814–827. [Google Scholar] [CrossRef]
- Macedonio, F.; Drioli, E. An exergetic analysis of a membrane desalination system. Desalination 2010, 261, 293–299. [Google Scholar] [CrossRef]
- Sharaf Eldean, M.A.; Soliman, A.M. A novel study of using oil refinery plants waste gases for thermal desalination and electric power generation: Energy, exergy & cost evaluations. Appl. Energy 2017, 195, 453–477. [Google Scholar] [CrossRef]
- Soliman, M.N.; Guen, F.Z.; Ahmed, S.A.; Saleem, H.; Khalil, M.J.; Zaidi, S.J. Energy consumption and environmental impact assessment of desalination plants and brine disposal strategies. Process Saf. Environ. Prot. 2021, 147, 589–608. [Google Scholar] [CrossRef]
- Khalilzadeh, S.; Hossein Nezhad, A. Utilization of waste heat of a high-capacity wind turbine in multi effect distillation desalination: Energy, exergy and thermoeconomic analysis. Desalination 2018, 439, 119–137. [Google Scholar] [CrossRef]
- Askari, I.B.; Ameri, M.; Calise, F. Energy, exergy and exergo-economic analysis of different water desalination technologies powered by Linear Fresnel solar field. Desalination 2018, 425, 37–67. [Google Scholar] [CrossRef]
- Khouya, A. Performance evaluation of a MED-MVC desalination plant driven by a concentrated photovoltaic thermal system and an organic Rankine cycle. Energy Convers. Manag. 2022, 274, 116428. [Google Scholar] [CrossRef]
- Fergani, Z.; Triki, Z.; Menasri, R.; Tahraoui, H.; Kebir, M.; Amrane, A.; Moula, N.; Zhang, J.; Mouni, L. Analysis of Desalination Performance with a Thermal Vapor Compression System. Water 2023, 15, 1225. [Google Scholar] [CrossRef]
- Kahraman, N.; Cengel, Y.A. Exergy analysis of a MSF distillation plant. Energy Convers. Manag. 2005, 46, 2625–2636. [Google Scholar] [CrossRef]
- Nafey, A.S.; Fath, H.E.; Mabrouk, A.A. Exergy and thermoeconomic evaluation of MSF process using a new visual package. Desalination 2006, 201, 224–240. [Google Scholar] [CrossRef]
- Mabrouk, A.A.; Nafey, A.S.; Fath, H.E. Analysis of a new design of a multi-stage flash-mechanical vapor compression desalination process. Desalination 2007, 204, 482–500. [Google Scholar] [CrossRef]
- Al-Weshahi, M.A.; Anderson, A.; Tian, G. Exergy efficiency enhancement of MSF desalination by heat recovery from hot distillate water stages. Appl. Therm. Eng. 2013, 53, 226–233. [Google Scholar] [CrossRef]
- Al Ghamdi, A.; Mustafa, I. Exergy analysis of a MSF desalination plant in Yanbu, Saudi Arabia. Desalination 2016, 399, 148–158. [Google Scholar] [CrossRef]
- Almerri, A.H.; Al-Obaidi, M.A.; Alsadaie, S.; Mujtaba, I.M. Modelling and simulation of industrial multistage flash desalination process with exergetic and thermodynamic analysis. A case study of Azzour seawater desalination plant. Chem. Prod. Process Model. 2023, 18, 73–95. [Google Scholar] [CrossRef]
- Tayyeban, E.; Deymi-Dashtebayaz, M.; Dadpour, D. Multi objective optimization of MSF and MSF-TVC desalination systems with using the surplus low-pressure steam (an energy, exergy and economic analysis). Comput. Chem. Eng. 2022, 160, 107708. [Google Scholar] [CrossRef]
- Tayefeh, M. Exergy and economic analysis of a novel integration of compressed air energy storage with multi-effect distillation and multi-stage flash systems. J. Energy Storage 2022, 55, 105534. [Google Scholar] [CrossRef]
- Alotaibi, S.A.; Ibrahim, O.M.; Salamah, F.H. Energy and exergy analysis of three major recirculating multi-stage flashing desalination plants in Kuwait. J. Eng. Res. 2024. [Google Scholar] [CrossRef]
- Banat, F.; Jwaied, N. Exergy analysis of desalination by solar-powered membrane distillation units. Desalination 2008, 230, 27–40. [Google Scholar] [CrossRef]
- Mrayed, S.; Maccioni, D.; Leslie, G. Efficiency of Membrane Distillation to Produce Fresh Water. Int. J. Chem. Mol. Nucl. Mater. Metall. Eng. 2013, 7, 991–995. [Google Scholar]
- Al-Obaidani, S.; Curcio, E.; Macedonio, F.; Di Profio, G.; Al-Hinai, H.; Drioli, E. Potential of membrane distillation in seawater desalination: Thermal efficiency, sensitivity study and cost estimation. J. Membr. Sci. 2008, 323, 85–98. [Google Scholar] [CrossRef]
- Miladi, R.; Frikha, N.; Gabsi, S. Exergy analysis of a solar-powered vacuum membrane distillation unit using two models. Energy 2017, 120, 872–883. [Google Scholar] [CrossRef]
- Ali, E. Energy efficient configuration of Membrane Distillation units for brackish water desalination using exergy analysis. Chem. Eng. Res. Des. 2017, 125, 245–256. [Google Scholar] [CrossRef]
- Ali, E.; Orfi, J.; Najib, A. Assessing the Thermal Efficiency of Brackish Water Desalination by Membrane Distillation Using Exergy Analysis. Arab. J. Sci. Eng. 2018, 43, 2413–2424. [Google Scholar] [CrossRef]
- Lai, C.; Zhang, H. An efficient and economic evacuated U-tube solar collector powered air gap membrane distillation hybrid system for seawater desalination. J. Clean. Prod. 2023, 394, 136382. [Google Scholar] [CrossRef]
- Mibarki, N.; Triki, Z.; Belhadj, A.E.; Tahraoui, H.; Amrane, A.; Cheikh, S.; Hadadi, A.; Bouchelkia, N.; Kebir, M.; Zhang, J.; et al. Energy and Exergy Analysis of Solar Air Gap Membrane Distillation System for Seawater Desalination. Water 2023, 15, 1201. [Google Scholar] [CrossRef]
- Okati, V.; Moghadam, A.J.; Farzaneh-Gord, M.; Moein-Jahromi, M. Thermo-economical and environmental analyses of a Direct Contact Membrane Distillation (DCMD) performance. J. Clean. Prod. 2022, 340, 130613. [Google Scholar] [CrossRef]
- Lin, B.; Malmali, M. Energy and exergy analysis of multi-stage vacuum membrane distillation integrated with mechanical vapor compression. Sep. Purif. Technol. 2023, 306, 122568. [Google Scholar] [CrossRef]
- Curcio, E.; Drioli, E. Membrane Distillation and Related Operations—A Review. Sep. Purif. Rev. 2005, 34, 35–86. [Google Scholar] [CrossRef]
- Eshoul, N.; Almutairi, A.; Lamidi, R.; Alhajeri, H.; Alenezi, A. Energetic, Exergetic, and Economic Analysis of MED-TVC Water Desalination Plant with and without Preheating. Water 2018, 10, 305. [Google Scholar] [CrossRef]
- Nasr, J.; Reza, M. Exergy Analysis of the Optimized MSFD Type of Brackish Water Desalination Process. Iran J. Chem. Chem. Eng. 2017, 36, 191–208. [Google Scholar]
- Schwantes, R.; Cipollina, A.; Gross, F.; Koschikowski, J.; Pfeifle, D.; Rolletschek, M.; Subiela, V. Membrane distillation: Solar and waste heat driven demonstration plants for desalination. Desalination 2013, 323, 93–106. [Google Scholar] [CrossRef]
- Petela, R. Exergy of undiluted thermal radiation. Sol. Energy 2003, 74, 469–488. [Google Scholar] [CrossRef]
- Gaggioli, R.A. Second Law Analysis for Process and Energy Engineering. In Efficiency and Costing; American Chemical Society: Washington, DC, USA, 1983; pp. 3–50. [Google Scholar] [CrossRef]
- Marmolejo-Correa, D.; Gundersen, T. A comparison of exergy efficiency definitions with focus on low temperature processes. Energy 2012, 44, 477–489. [Google Scholar] [CrossRef]
- Kotas, T. Exergy Criteria of Performance for Thermal Plant: Second of Two Papers on Exergy Techniques in Thermal Plant Analysis. Int. J. Heat Fluid Flow 1980, 2, 147–163. [Google Scholar] [CrossRef]
- Gundersen, T. An Introduction to: The Concept of Exergy and Energy Quality; Department of Energy and Process Engineering Norwegian University of Science and Technology: Trondheim, Norway, 2009; Volume 4, pp. 1–25. [Google Scholar]
- Moran, M.J.; Shapiro, H.N.; Bailey, M.B. Fundamentals of Engineering Thermodynamics, 9th ed.; Wiley: Hoboken, NJ, USA, 2018. [Google Scholar]
- Tsatsaronis, G. Thermoeconomic analysis and optimization of energy systems. Prog. Energy Combust. Sci. 1993, 19, 227–257. [Google Scholar] [CrossRef]
- Martinaitis, V.; Streckienė, G.; Biekša, D.; Bielskus, J. The exergy efficiency assessment of heat recovery exchanger for air handling units, using a state property—Coenthalpy. Appl. Therm. Eng. 2016, 108, 388–397. [Google Scholar] [CrossRef]
- Lazzaretto, A.; Tsatsaronis, G. SPECO: A systematic and general methodology for calculating efficiencies and costs in thermal systems. Energy 2006, 31, 1257–1289. [Google Scholar] [CrossRef]
- Cao, H.; Mao, Y.; Wang, W.; Gao, Y.; Zhang, M.; Zhao, X.; Sun, J.; Song, Z. Thermal-exergy efficiency trade-off optimization of pressure retarded membrane distillation based on TOPSIS model. Desalination 2022, 523, 115446. [Google Scholar] [CrossRef]
- Zhu, X.; Han, J.; Ge, Y.; Zhu, W.; Yang, J. Multi-objective optimization of a small-scale solar-hot-spring-geothermal brackish water direct contact membrane distillation system. Energy Convers. Manag. 2022, 270, 116282. [Google Scholar] [CrossRef]
- Woldemariam, D.; Martin, A.; Santarelli, M. Exergy analysis of air-gap membrane distillation systems for water purification applications. Appl. Sci. 2017, 7, 301. [Google Scholar] [CrossRef]


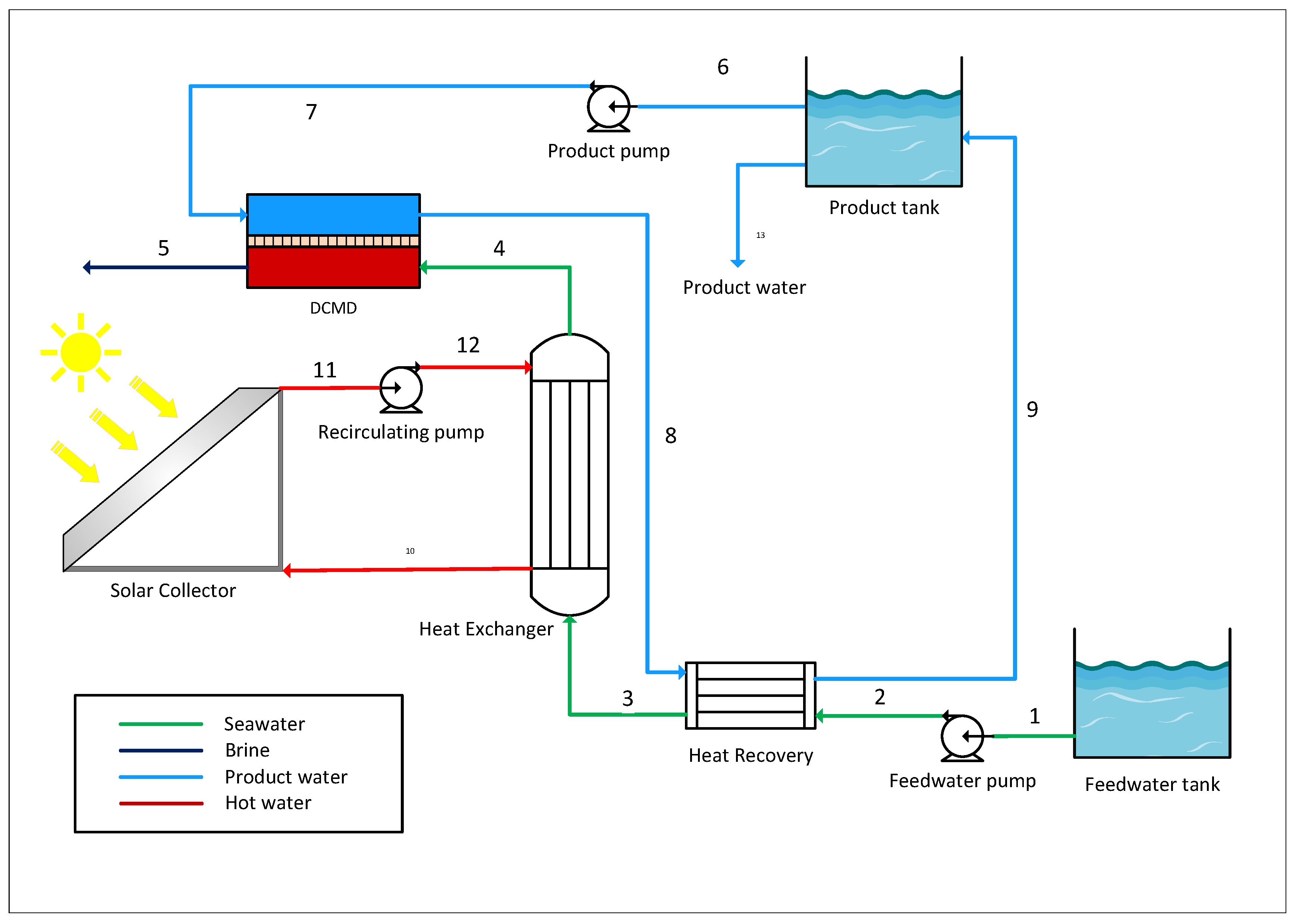
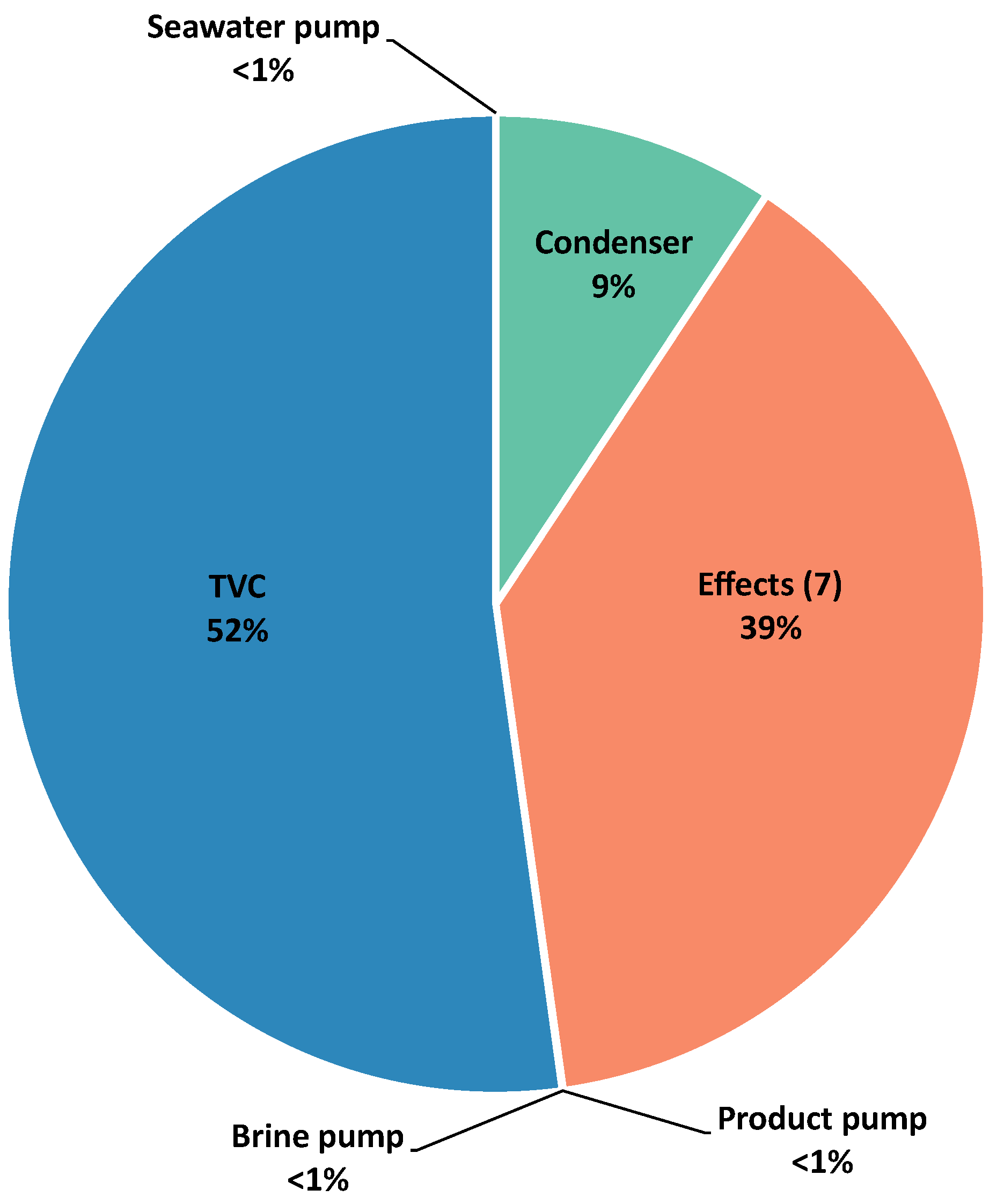
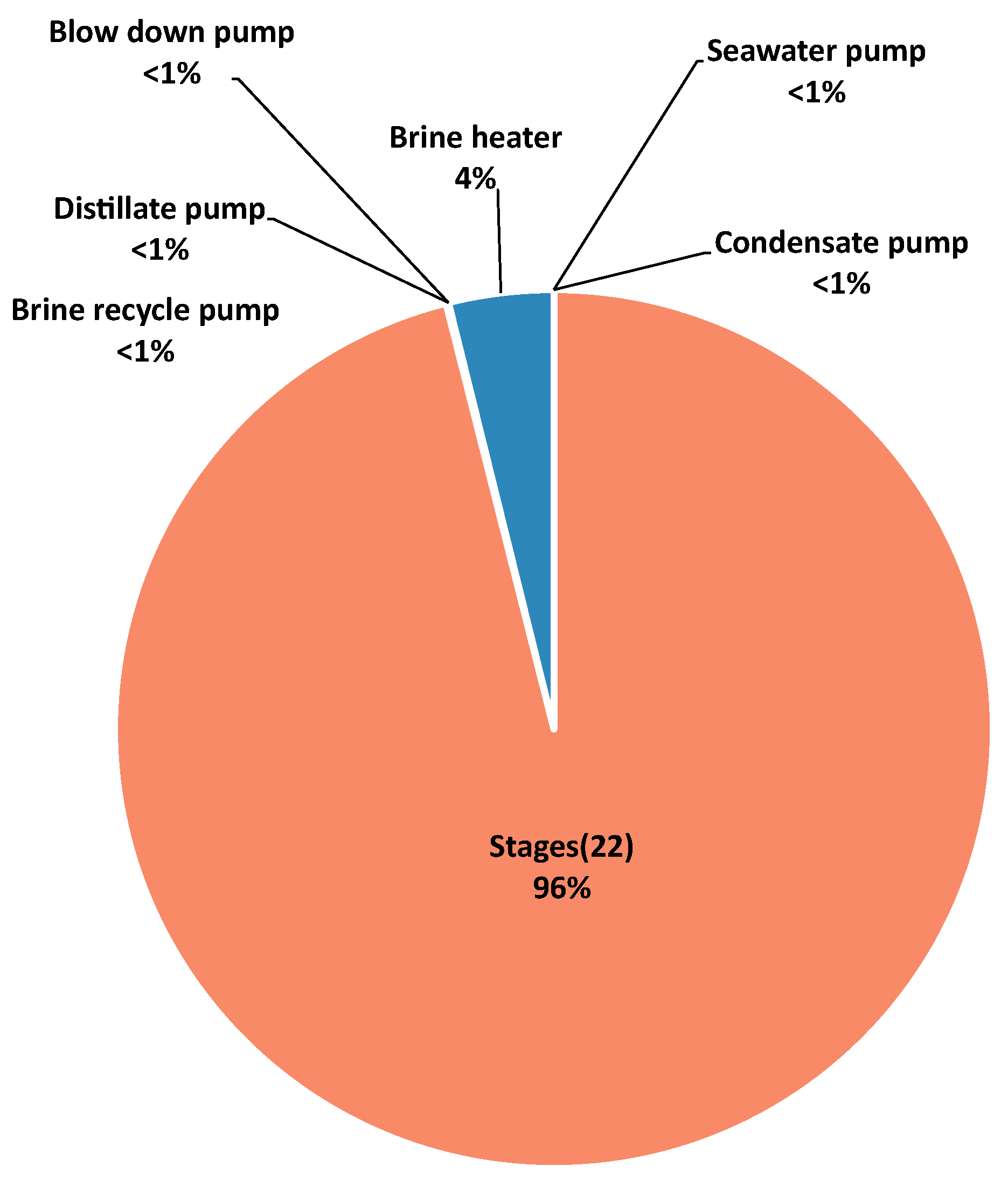

| Technology | Electrical Energy (kWh/m3) | Thermal Energy (kWh/m3) | Total Energy Consumption (kWh/m3) |
|---|---|---|---|
| MSF | 2.5–5 | 40–120 | 21–59 |
| MED | 2–2.5 | 30–120 | 15–57 |
| MD | 1.5–4 | 4–40 | 3–22 |
| SWRO | 3–6 | - | 3–6 |
| ED | 1–3.5 | - | 1–3.5 |
| BWRO | 0.5–3 | - | 0.5–3 |
| Type of Process | Operating Conditions | Chemical Exergy | Exergy Efficiency | Ref. |
|---|---|---|---|---|
| MSF | Seawater (46,500) | [37] | ||
| Temperature (°C): 90 | ||||
| Stages (n°): 22 | ||||
| MSF-BR | Seawater (45,000) | [38] | ||
| Temperature (°C): 109 | ||||
| Stages (n°): 21 | ||||
| MSF-MVC | Seawater (48,620) | [39] | ||
| Temperature (°C): 110 | ||||
| Stages (n°): 20 | ||||
| MSF | Seawater (45,000) | [40] | ||
| Temperature (°C): 114 | ||||
| Stages (n°): 28 | ||||
| MSF | Seawater (45,000 ppm) | - | [41] | |
| Temperature (°C): 110 | ||||
| Stages (n°): 28 | ||||
| MSF | Seawater (44,000 ppm) | [42] | ||
| Temperature (°C): 90 | ||||
| Stages (n°): 24 | ||||
| MSF-TVC | Wastewater (51,400 ppm) | - | [43] | |
| Temperature (°C): 115 | ||||
| Stages (n°): 25 | ||||
| MED-MSF | Seawater (35,000 ppm) | [44] | ||
| Temperature (°C): 70–80 | ||||
| Stages (n°): 14 + 14 | ||||
| MSF | Seawater (45,000 ppm) | [45] | ||
| Temperature (°C): 90–110 | ||||
| Stages (n°): 23–26 |
| Component | ||
|---|---|---|
| Pump | ||
| Heat exchanger | ||
| Condenser |
| Process | ||
|---|---|---|
| MED-TVC | ||
| MSF | ||
| DCMD |
| Component | ||||
|---|---|---|---|---|
| Seawater pump | 99.81 | 25.61 | 25.57 | 99.81 |
| Condenser | 12.86 | 677.90 | 42.50 | 6.27 |
| Distillation units | 55.11 | 2888.00 | 257.50 | 8.91 |
| Brine pump | 99.72 | 30.14 | 28.32 | 93.96 |
| Product pump | 99.94 | 13.98 | 13.43 | 96.10 |
| TVC | 62.1 | 8612.00 | 5052.00 | 58.66 |
| Total plant | 21.35 | 7723.00 | 239.00 | 3.095 |
| Component | ||||
|---|---|---|---|---|
| Seawater pump | 99.98 | 97.16 | 97.14 | 99.98 |
| Distillation units | 80.61 | 17,591.00 | 11.74 | 0.07 |
| Brine recycle pump | 99.44 | 258.40 | 255.20 | 98.73 |
| Distillate pump | 99.92 | 20.04 | 19.98 | 99.74 |
| Blow-down pump | 99.98 | 21.82 | 21.80 | 99.93 |
| Brine heater | 99.22 | 3592.00 | 3453.00 | 96.12 |
| Condensate pump | 99.98 | 0.88 | 0.87 | 98.72 |
| Total plant | 17.08 | 3991.00 | 63.12 | 1.58 |
| Component | ||||
|---|---|---|---|---|
| Feed pump | 96.13 | 0.03 | 0.001 | 96.12 |
| Permeate pump | 99.90 | 0.03 | 0.002 | 98.85 |
| Distillate pump | 99.98 | 0.02 | 0.004 | 98.28 |
| Heat exchanger | 74.37 | 3.98 | 3.35 | 15.77 |
| Heat recovery | 79.43 | 1.60 | 0.42 | 73.74 |
| DCMD module | 98.28 | 1.87 | 0.12 | 93.60 |
| Solar collector | 95.67 | 4.49 | 0.54 | 88.06 |
| Product tank | 60.58 | 0.42 | 0.16 | 60.58 |
| Total plant | 1.28 | 4.53 | 0.02 | 0.37 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arakcheeva El Kori, N.; Blanco-Marigorta, A.M.; Melián Martel, N. Definition of Exergetic Efficiency in the Main and Emerging Thermal Desalination Technologies: A Proposal. Water 2024, 16, 1254. https://doi.org/10.3390/w16091254
Arakcheeva El Kori N, Blanco-Marigorta AM, Melián Martel N. Definition of Exergetic Efficiency in the Main and Emerging Thermal Desalination Technologies: A Proposal. Water. 2024; 16(9):1254. https://doi.org/10.3390/w16091254
Chicago/Turabian StyleArakcheeva El Kori, Nenna, Ana M. Blanco-Marigorta, and Noemi Melián Martel. 2024. "Definition of Exergetic Efficiency in the Main and Emerging Thermal Desalination Technologies: A Proposal" Water 16, no. 9: 1254. https://doi.org/10.3390/w16091254
APA StyleArakcheeva El Kori, N., Blanco-Marigorta, A. M., & Melián Martel, N. (2024). Definition of Exergetic Efficiency in the Main and Emerging Thermal Desalination Technologies: A Proposal. Water, 16(9), 1254. https://doi.org/10.3390/w16091254







