Influence Mechanism of Water Level Variation on Deformation of Steep and Toppling Bedding Rock Slope
Abstract
1. Introduction
2. Geological Background
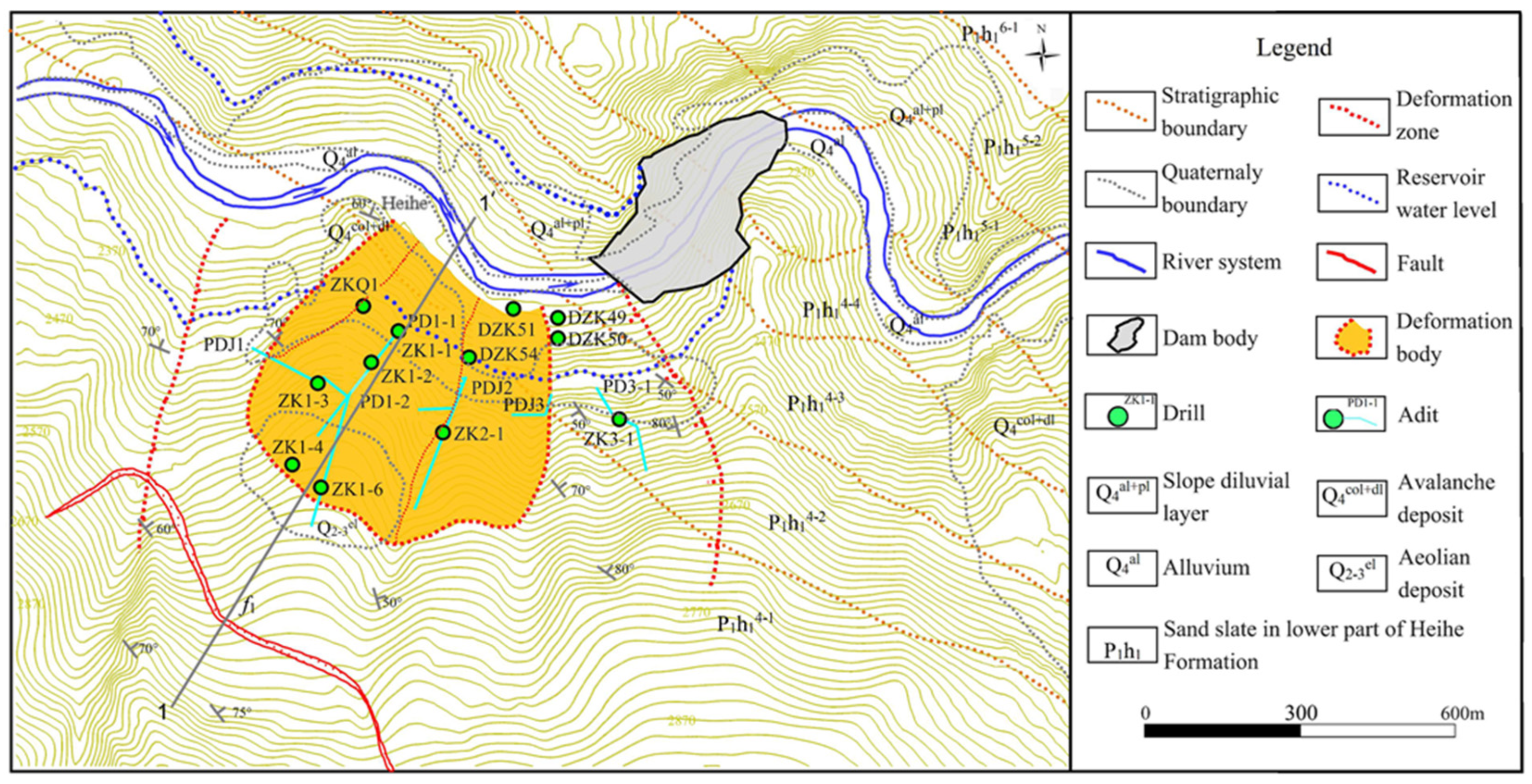
3. Engineering Geological Zoning
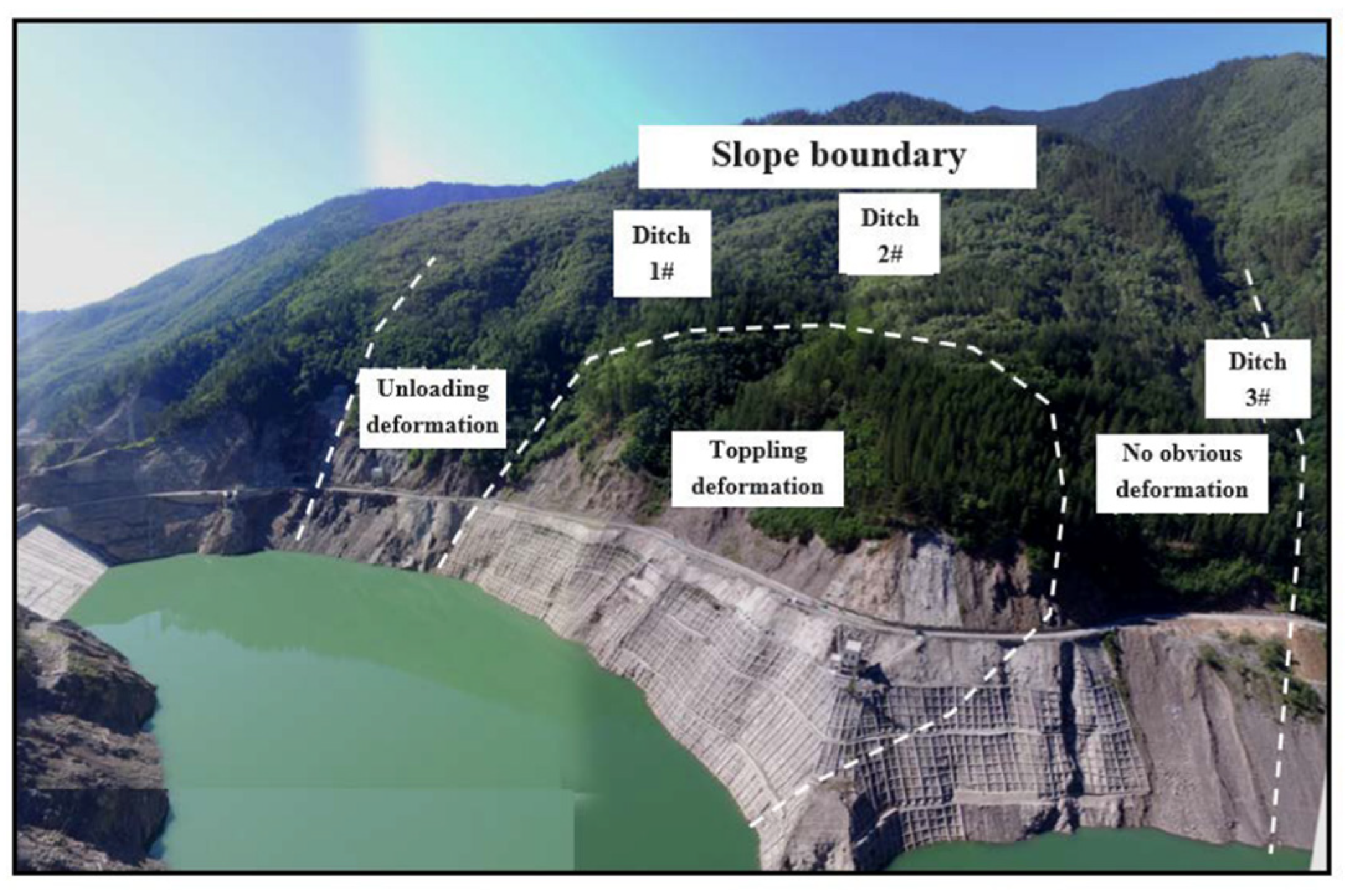
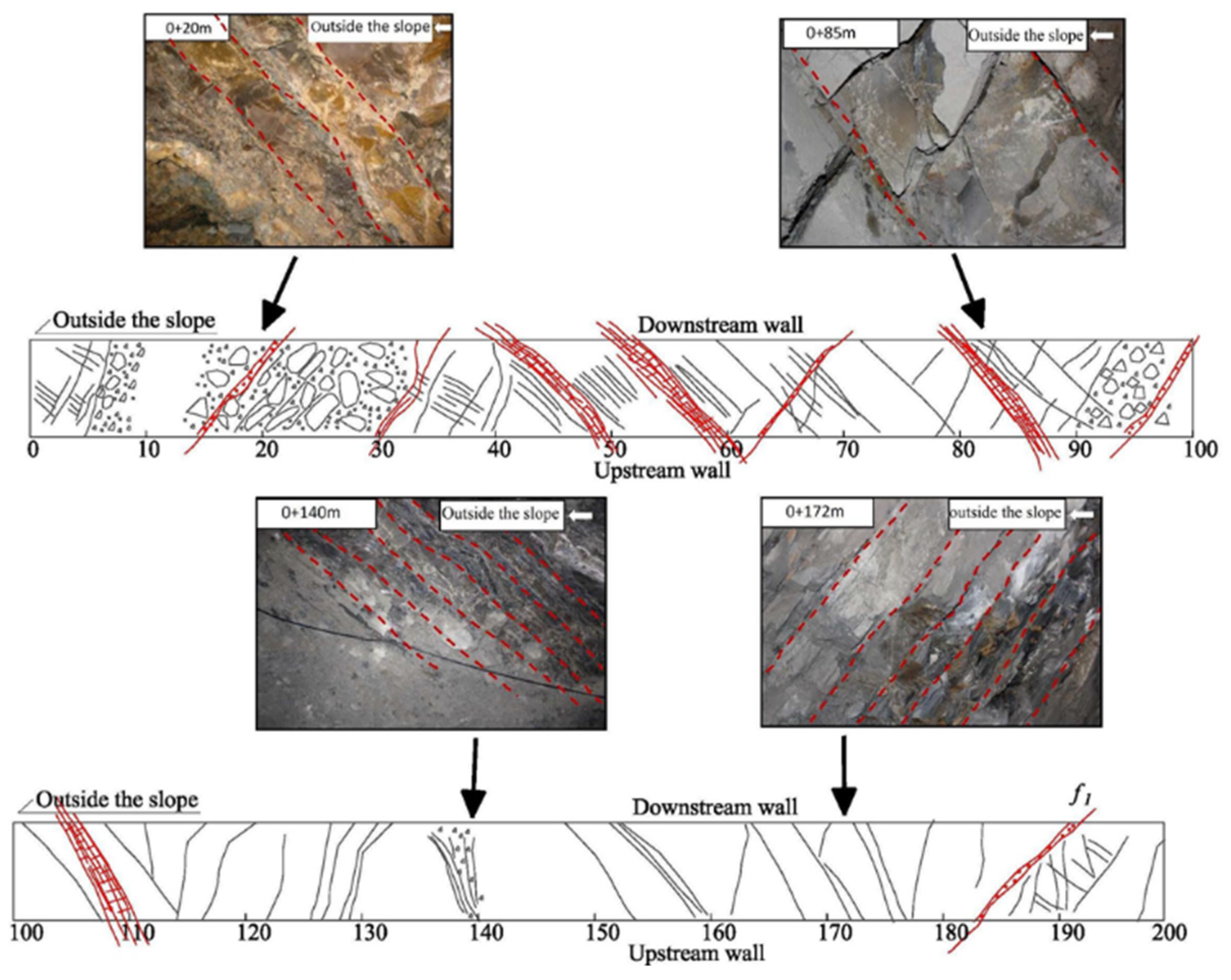
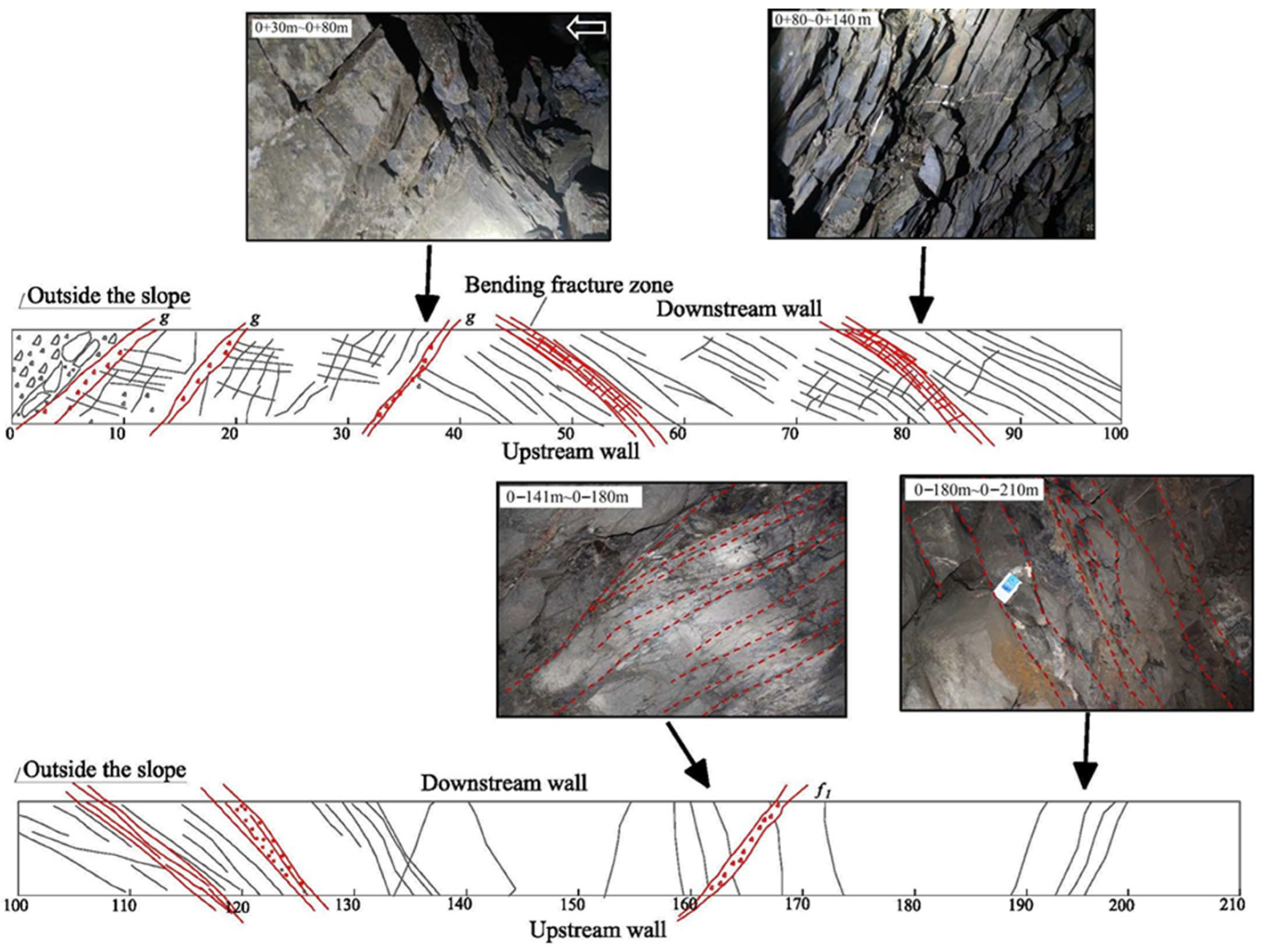
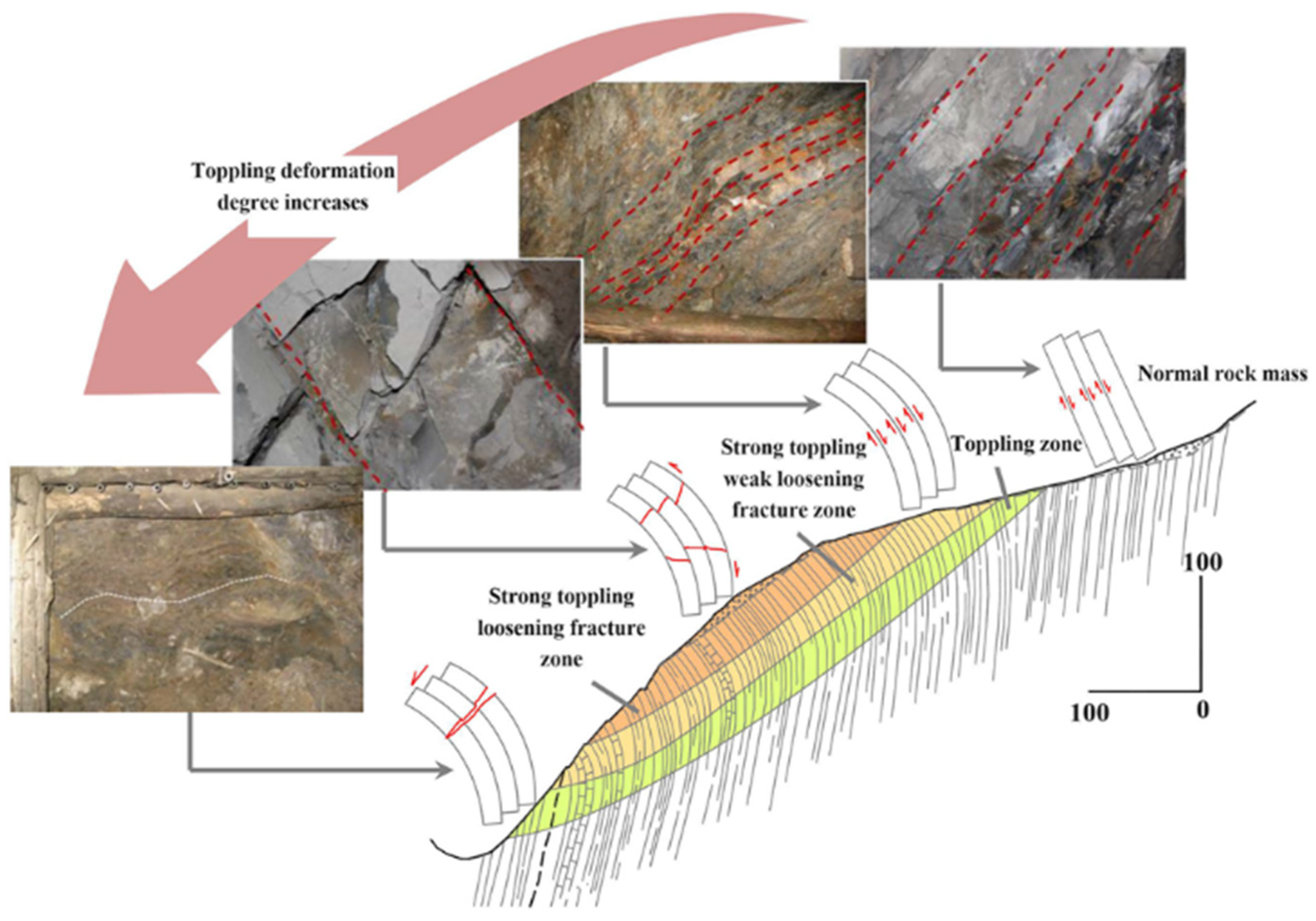
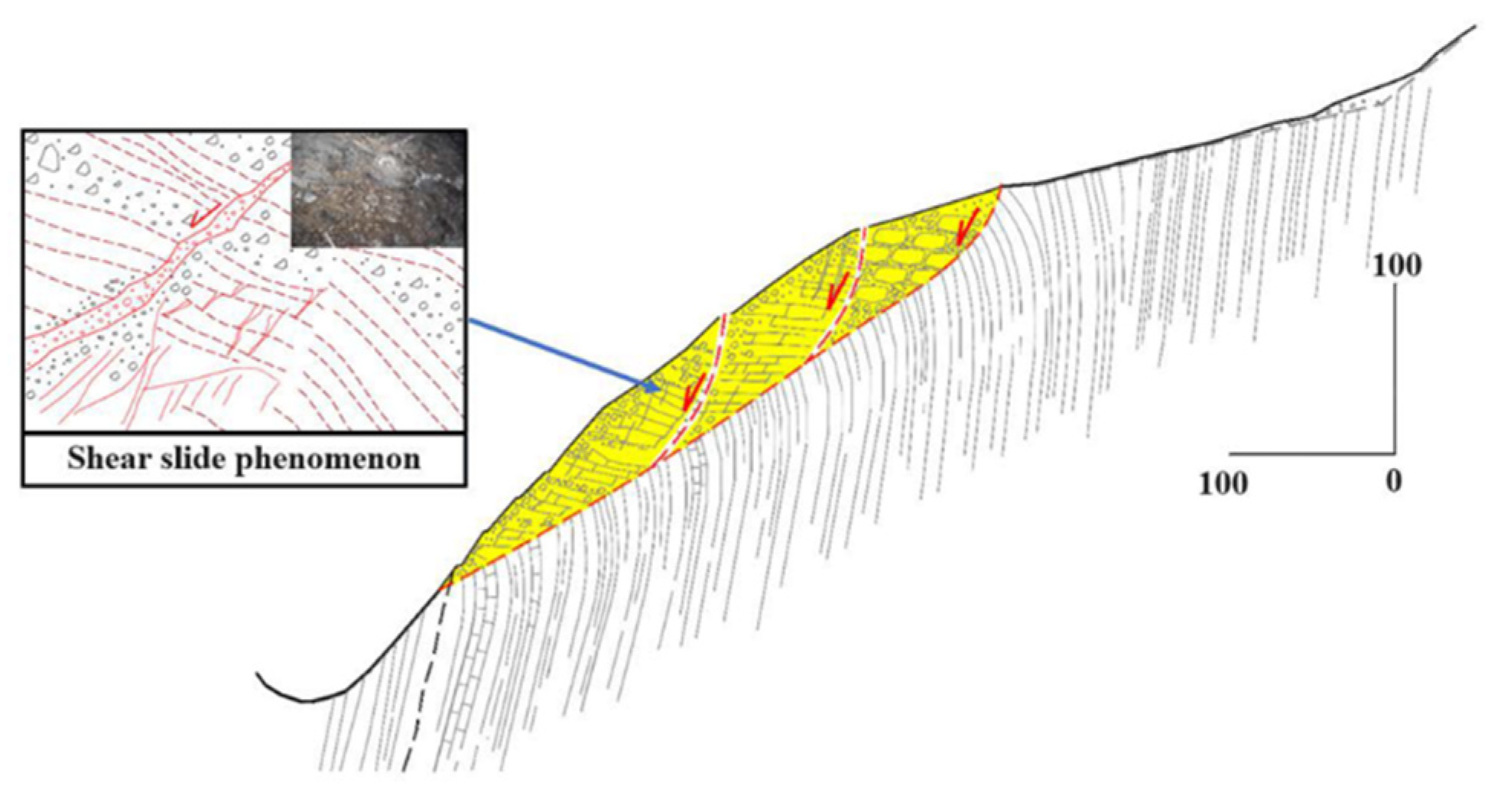
3.1. Slope Toppling Deformation Characteristics
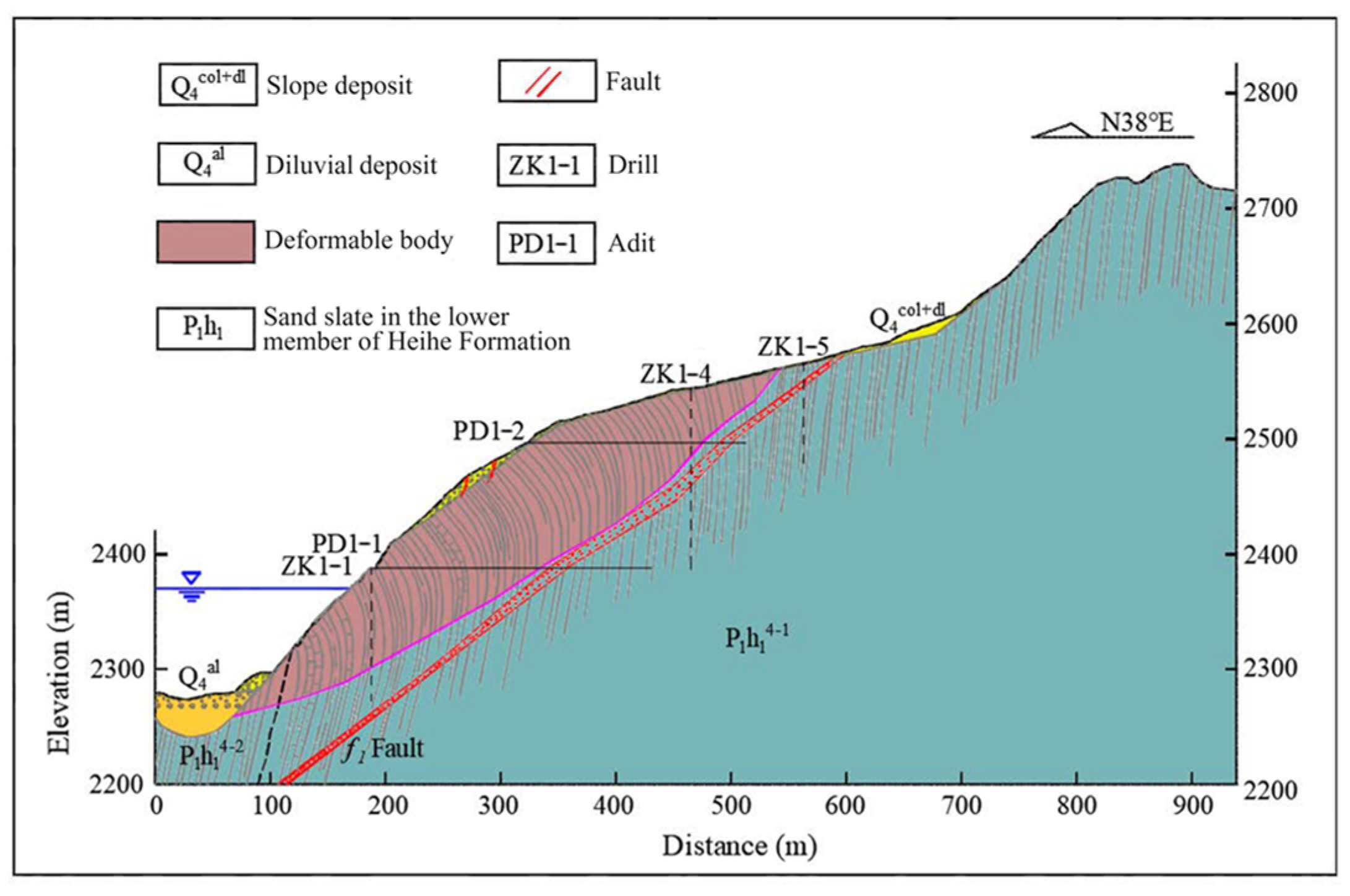
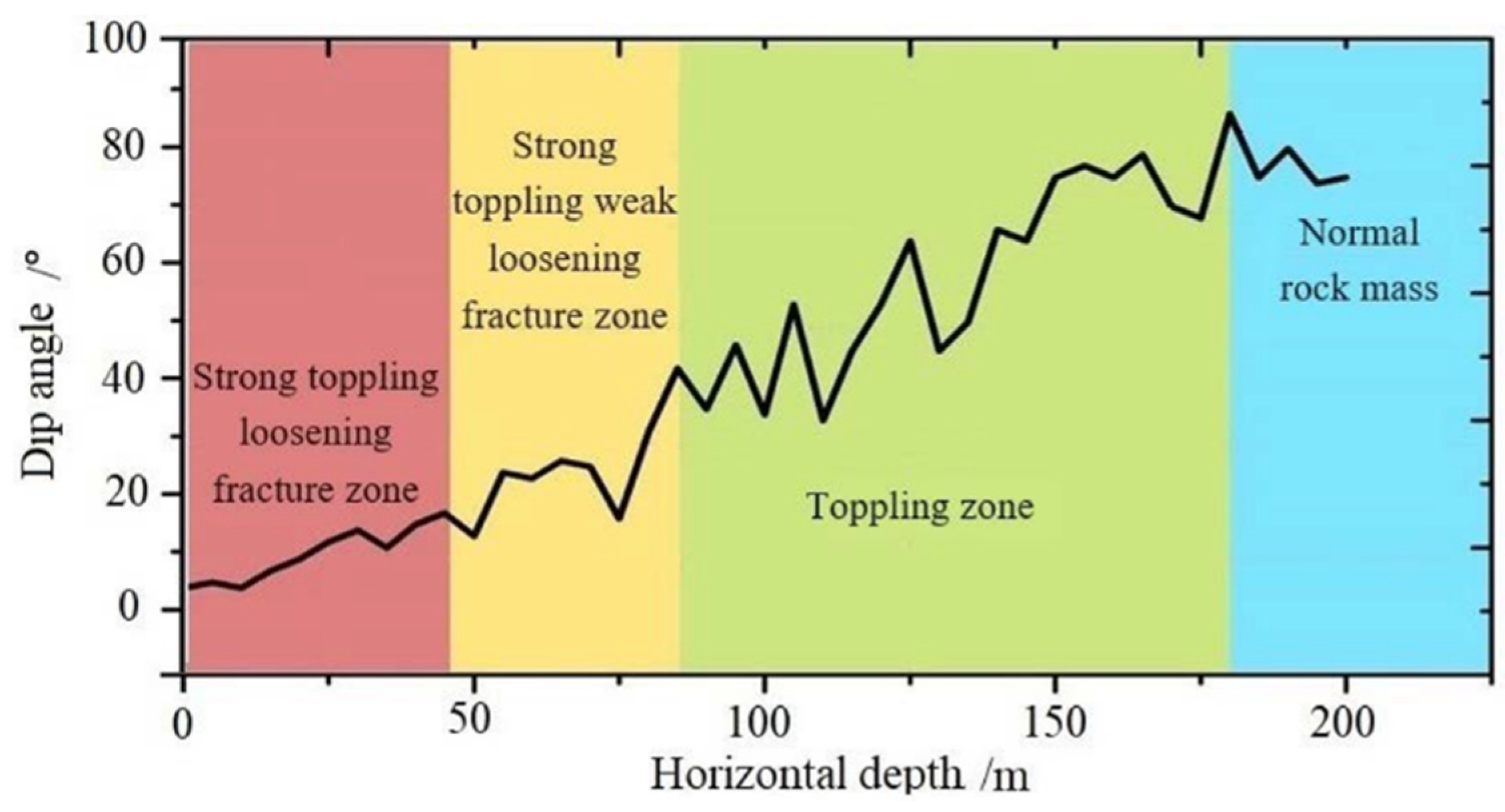
3.2. Slope Toppling Deformation Zoning
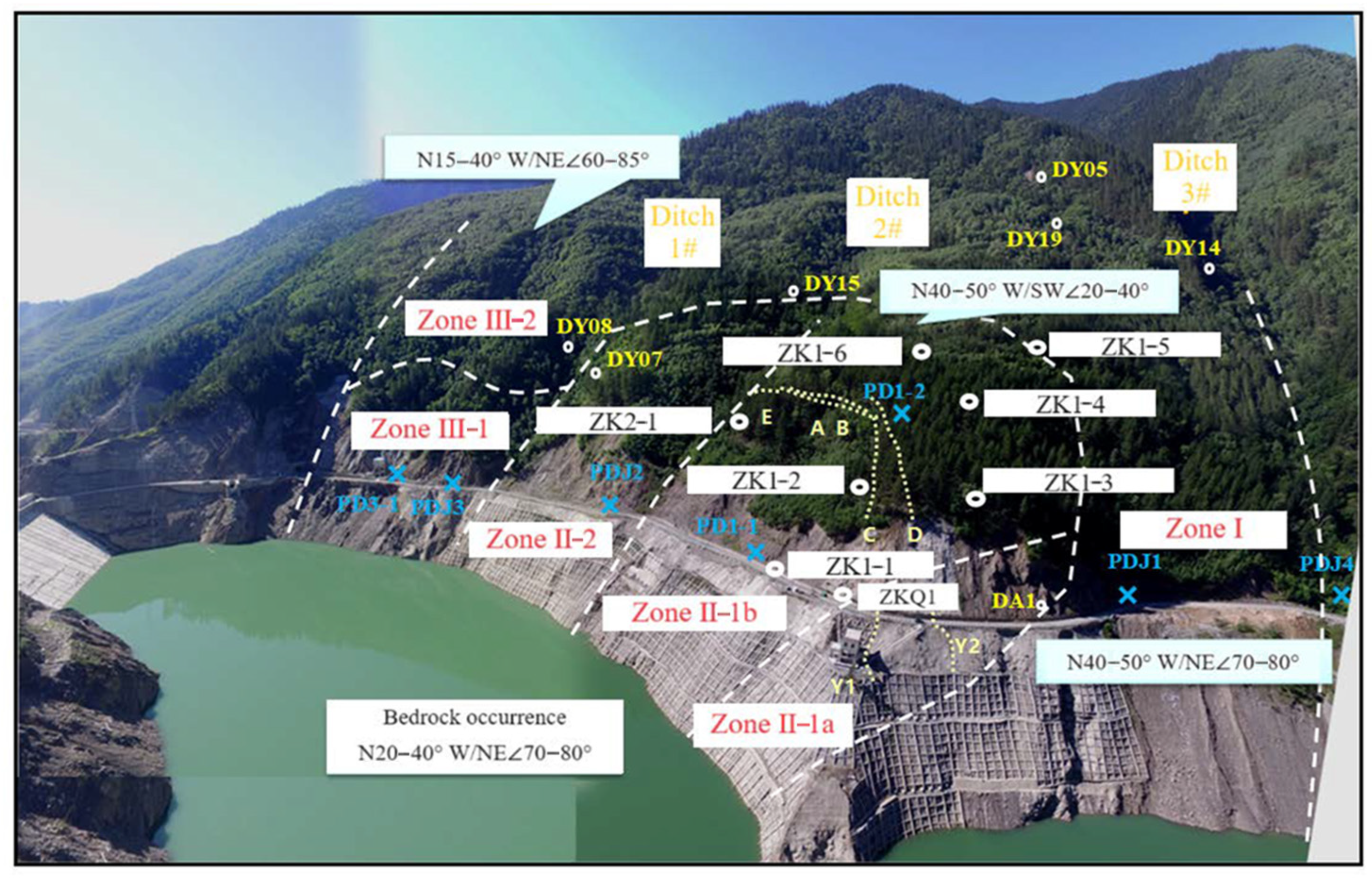
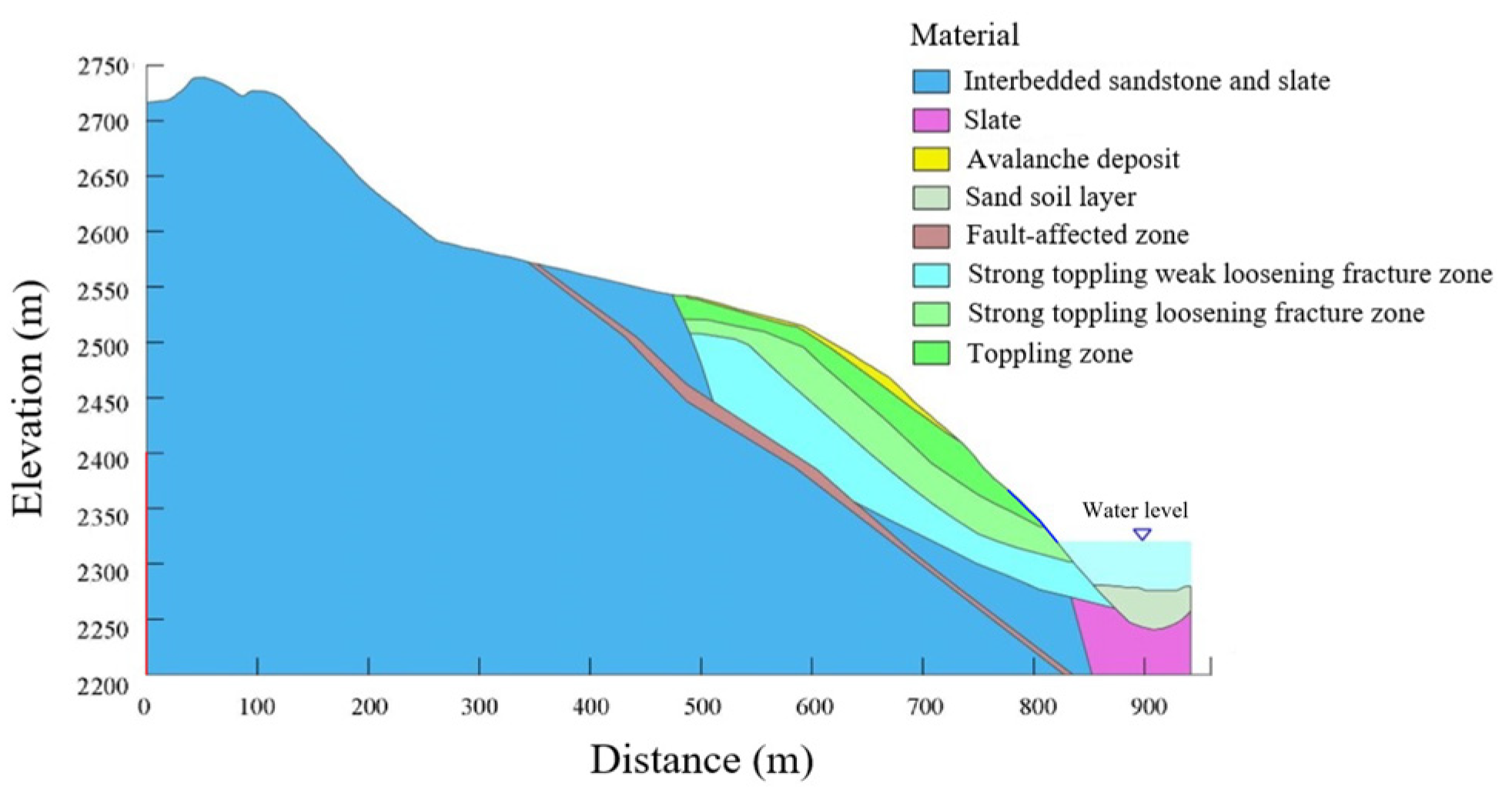
3.3. Slope Engineering Geological Zoning
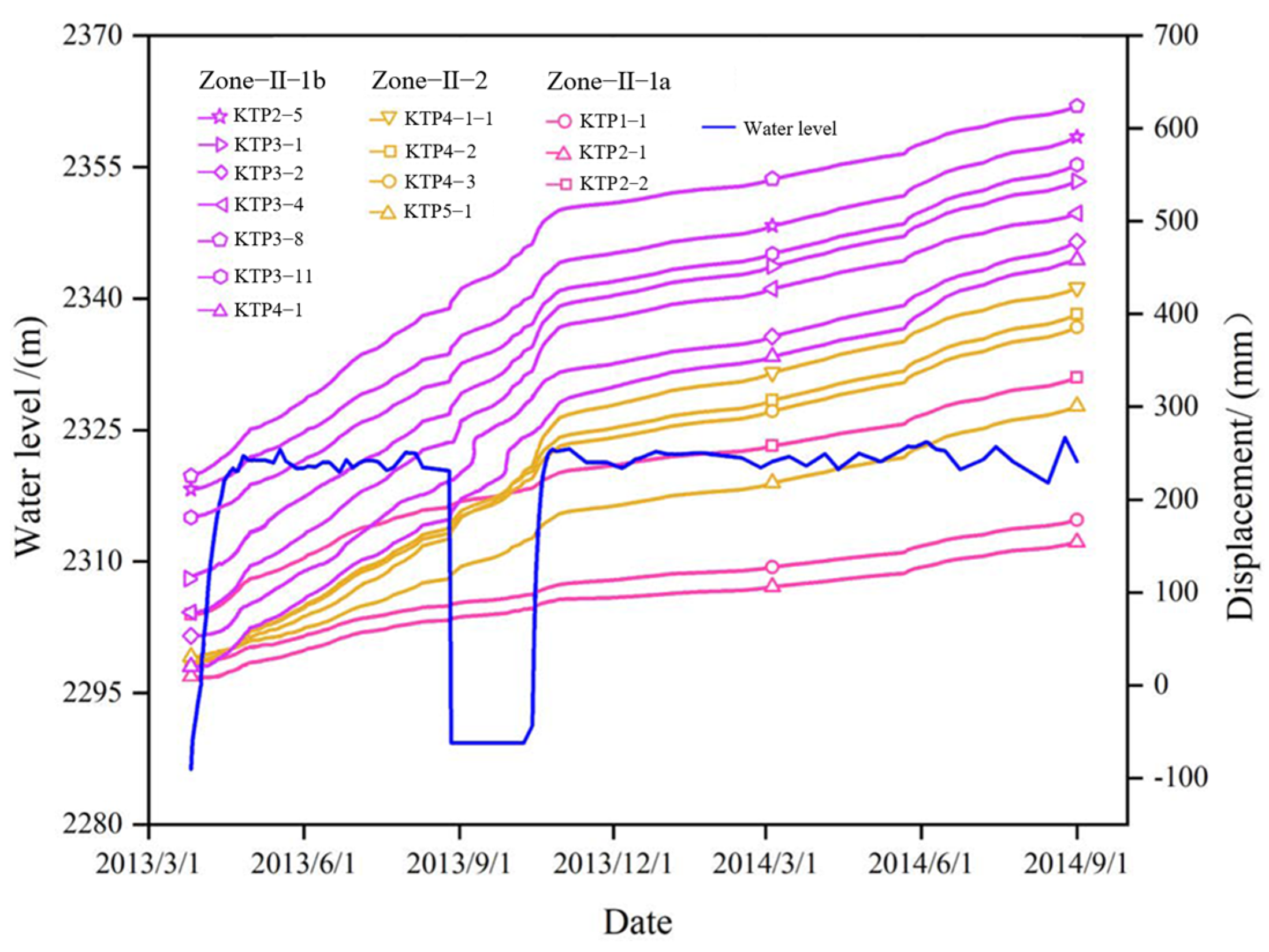
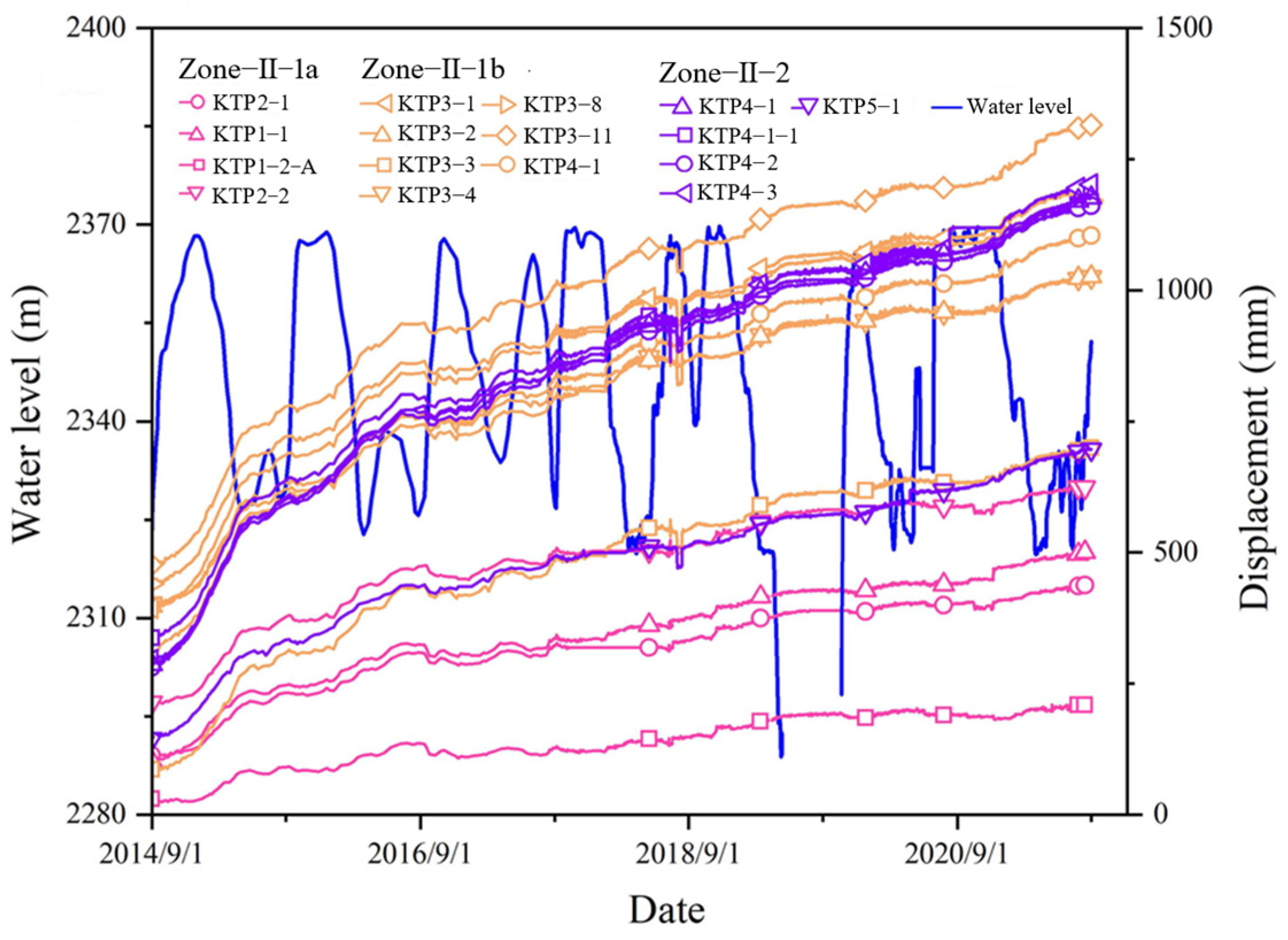
4. Deformation Monitoring
4.1. Monitoring Point Arrangement
4.2. Monitoring Result Analyses
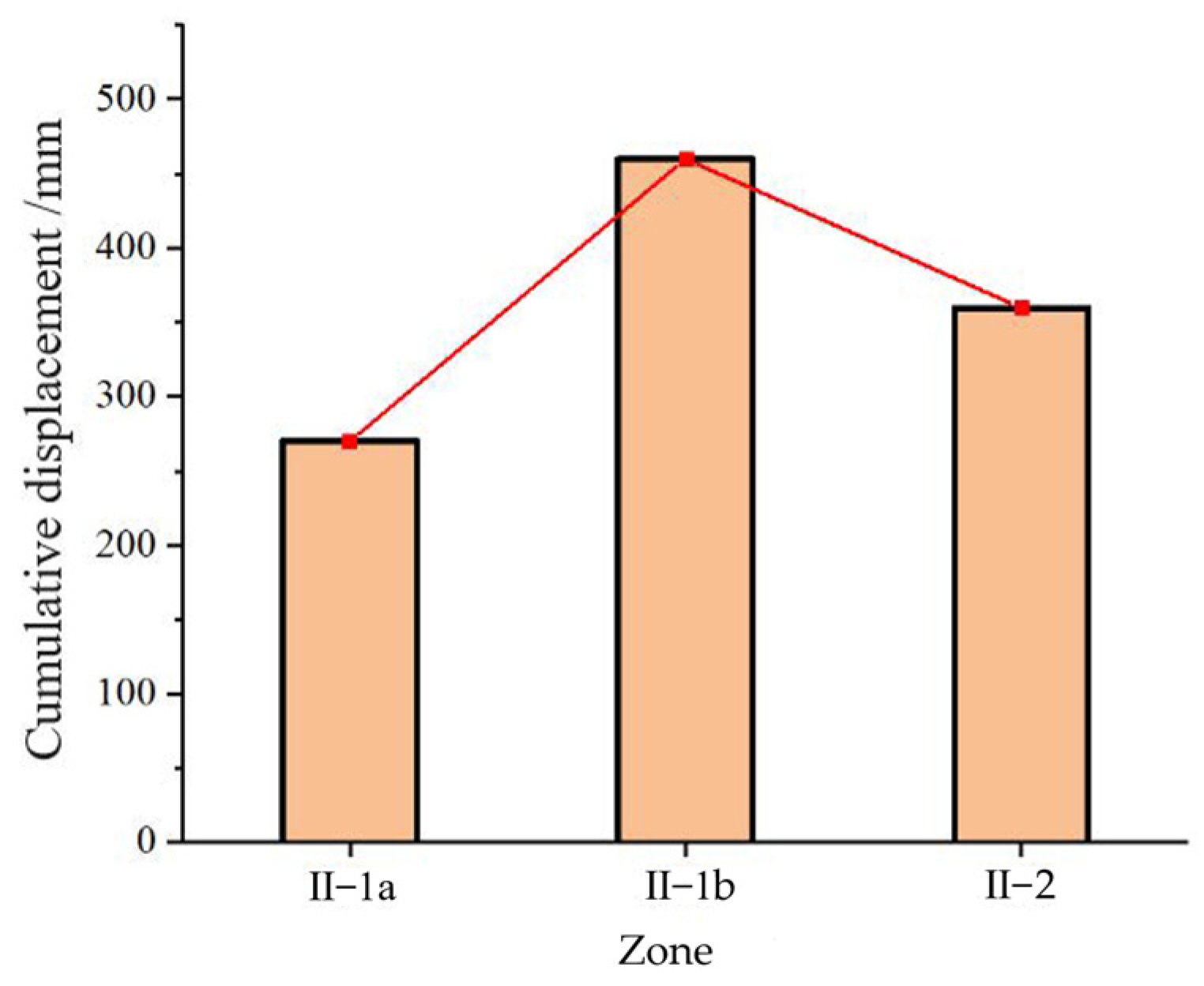
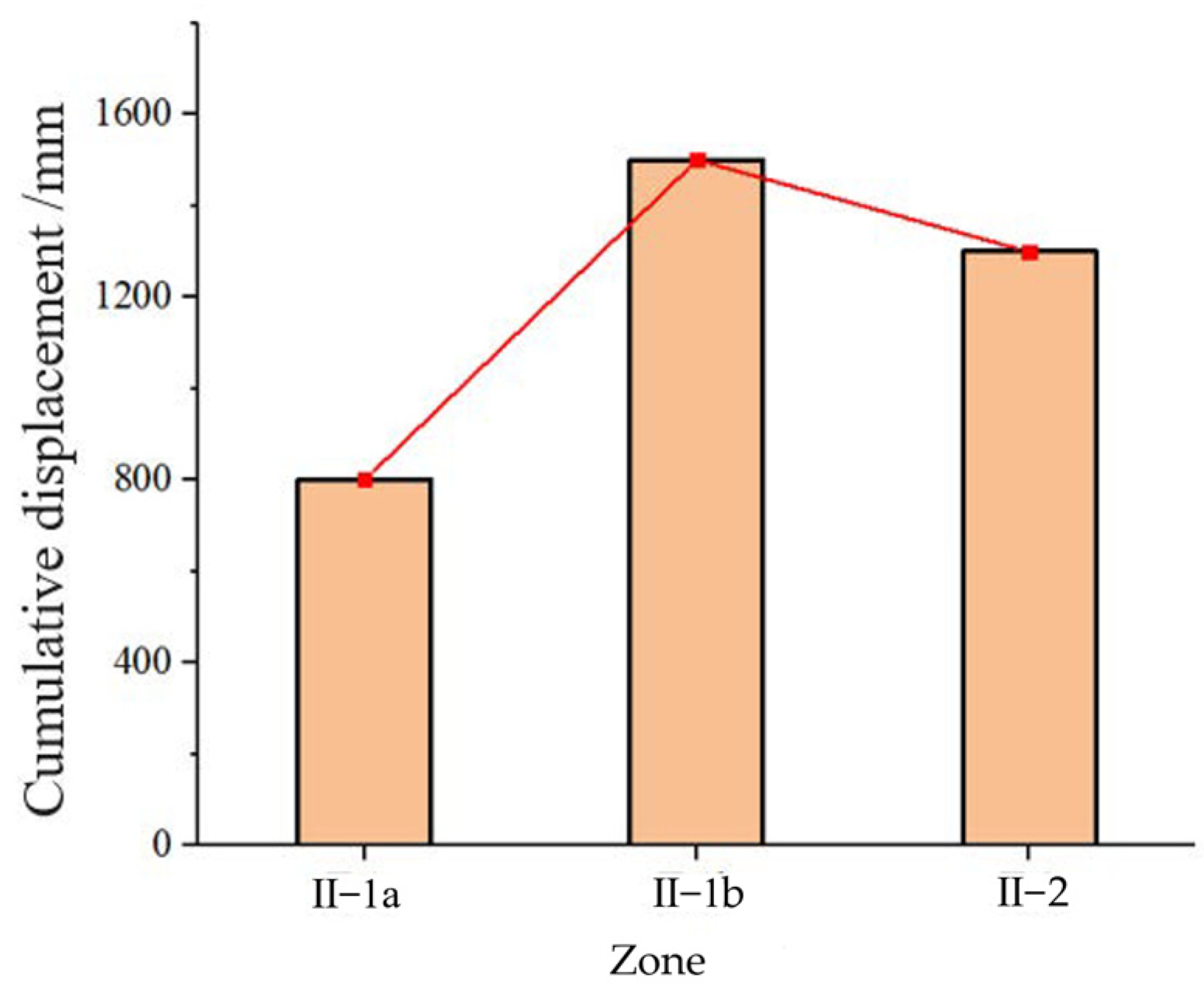
4.3. Surface Deformation Zoning
5. Influence Mechanism of Water Level Variation
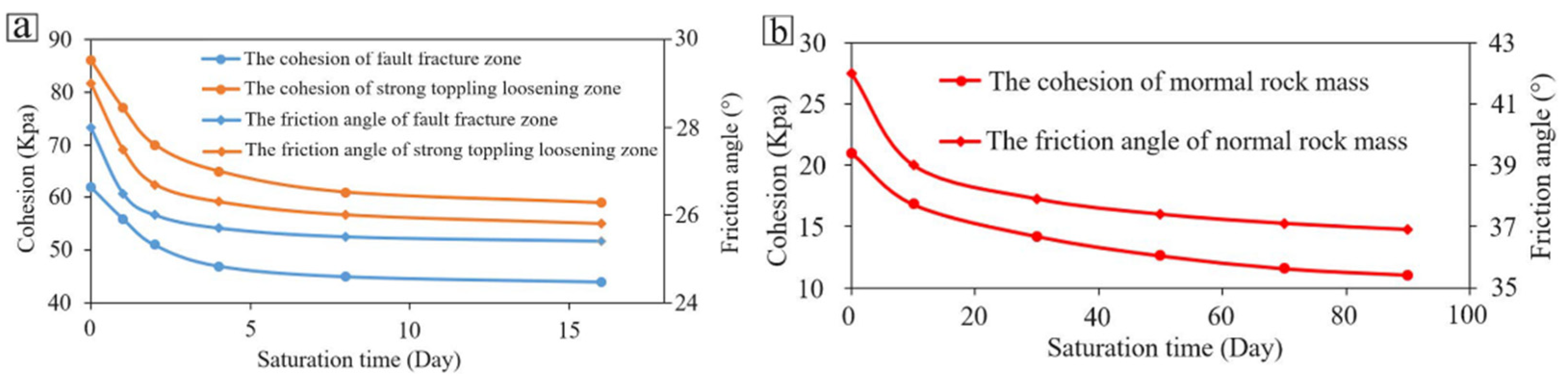
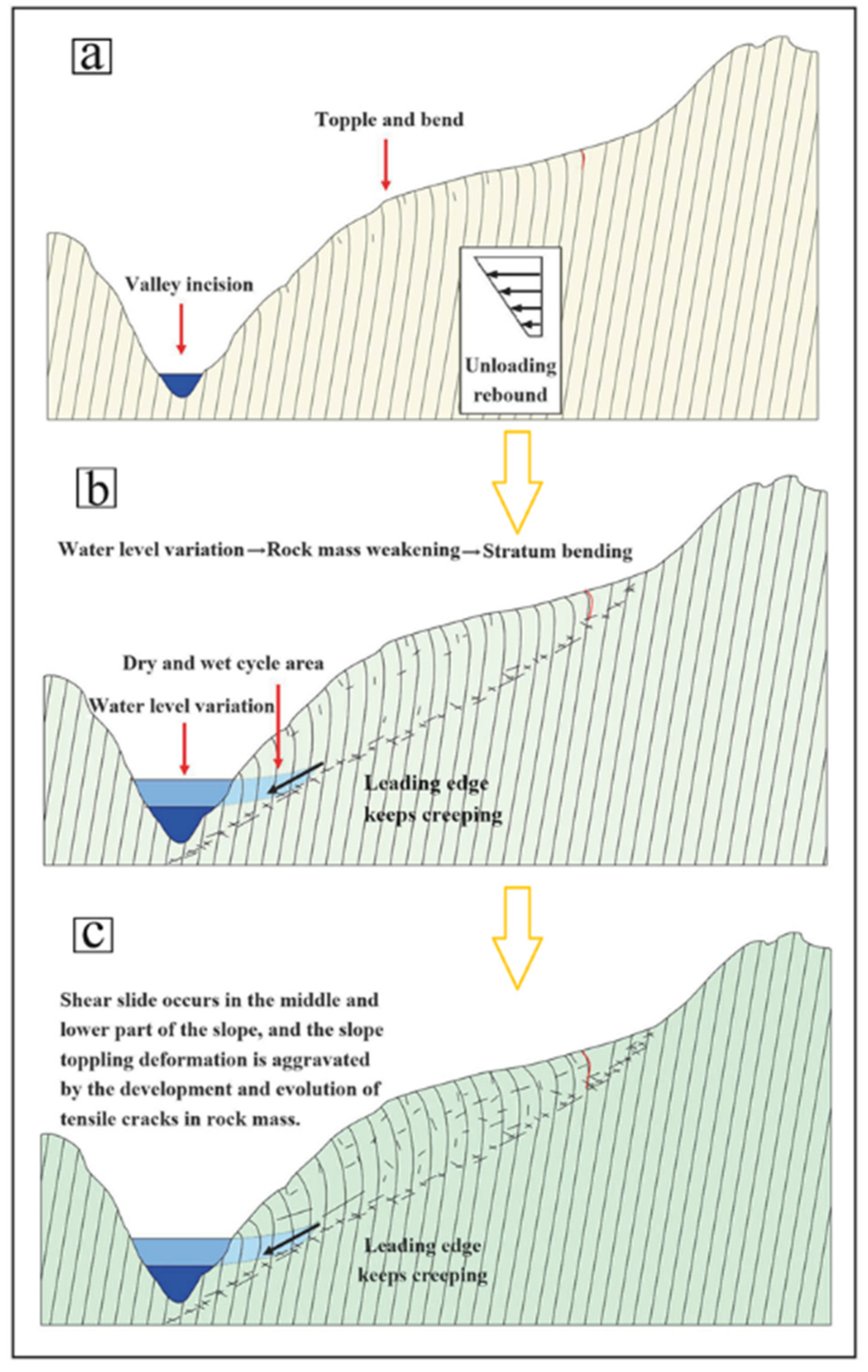
5.1. Deterioration of Rock Mass Caused by Water Level Variation
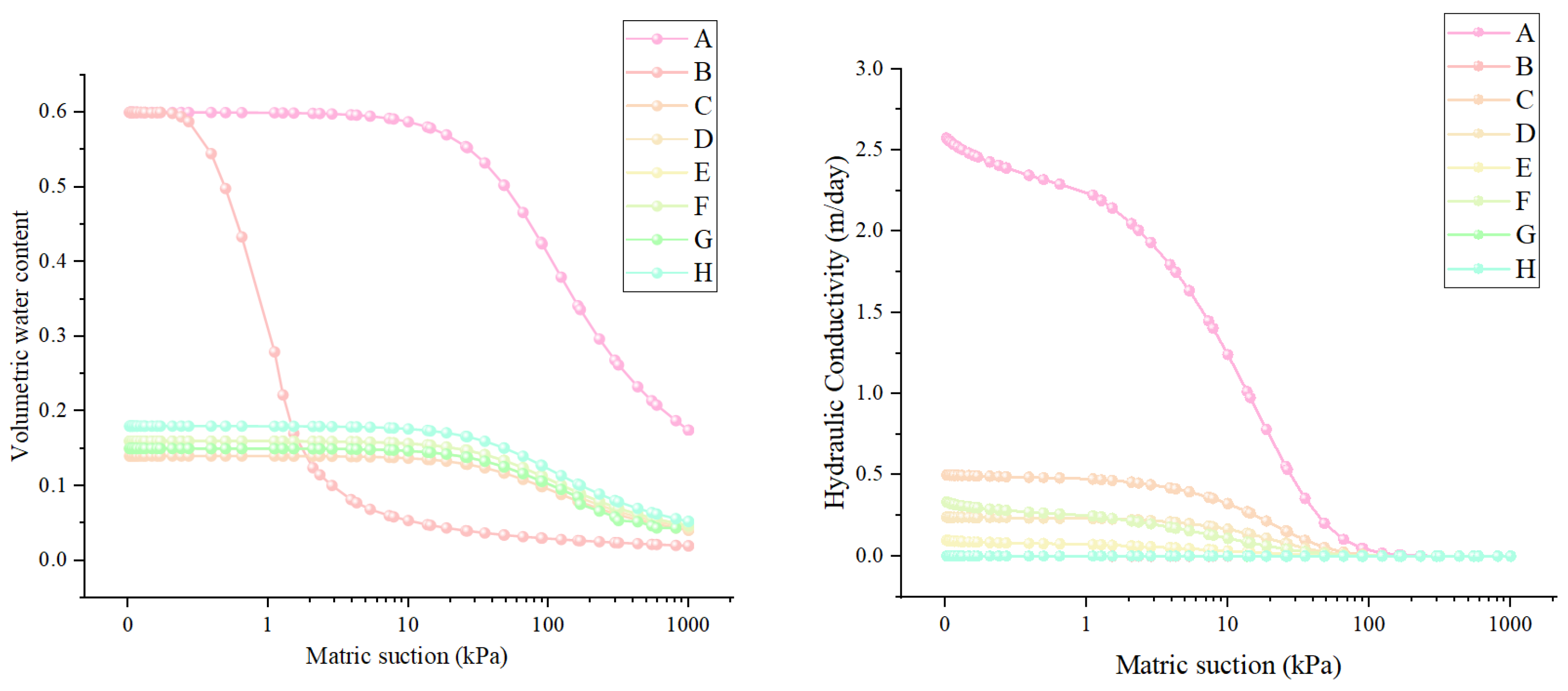
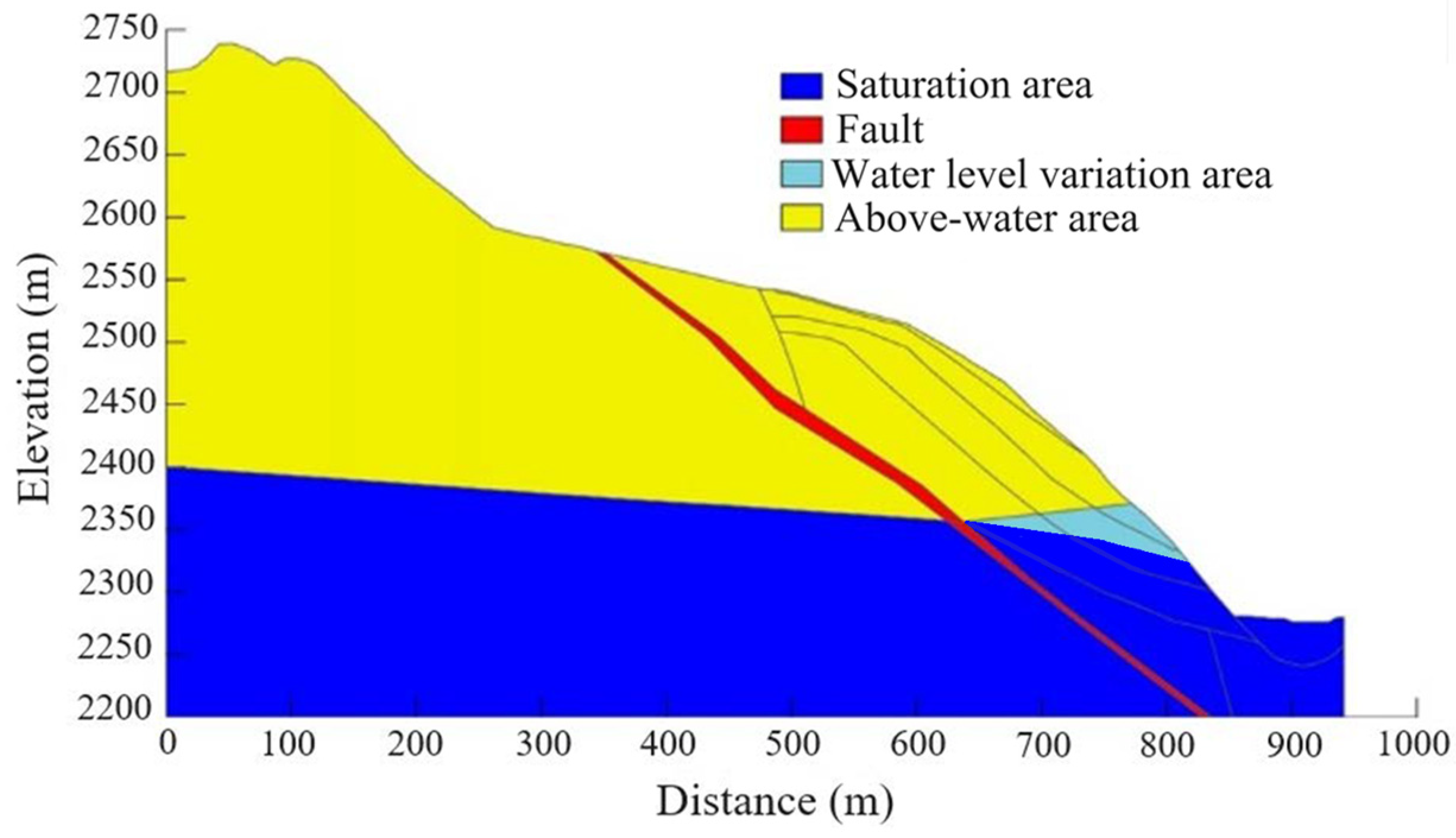
5.2. Influence of Water Level Variation on Seepage Field
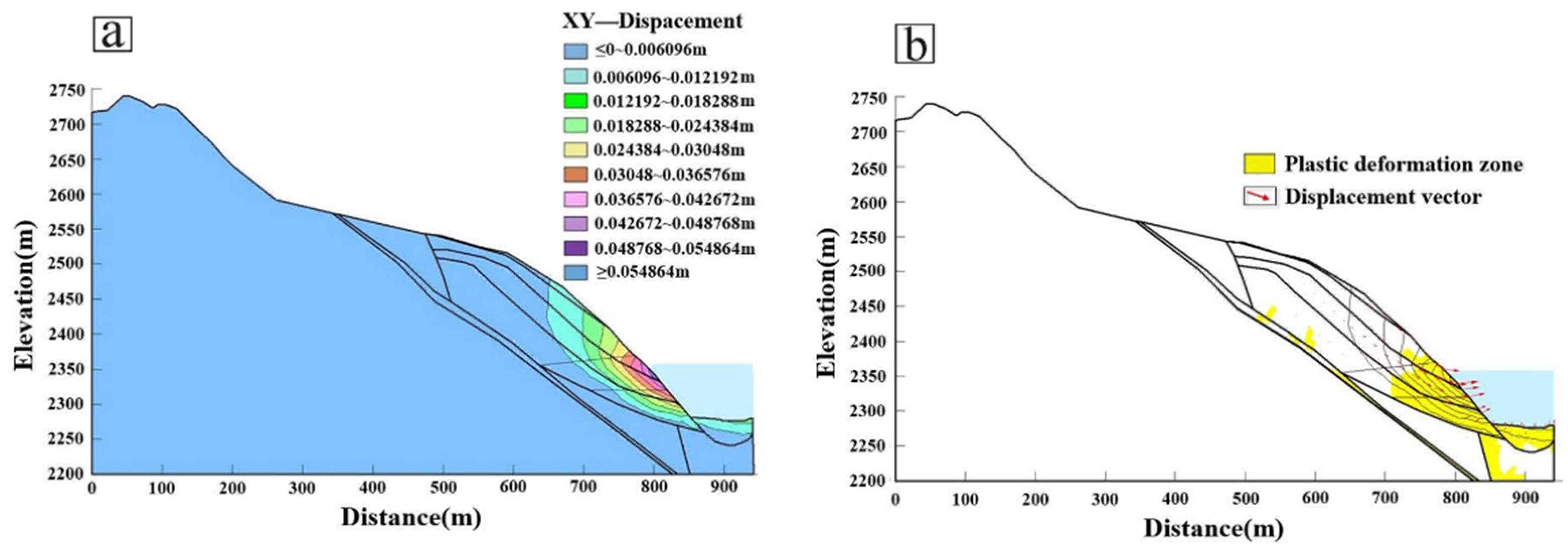
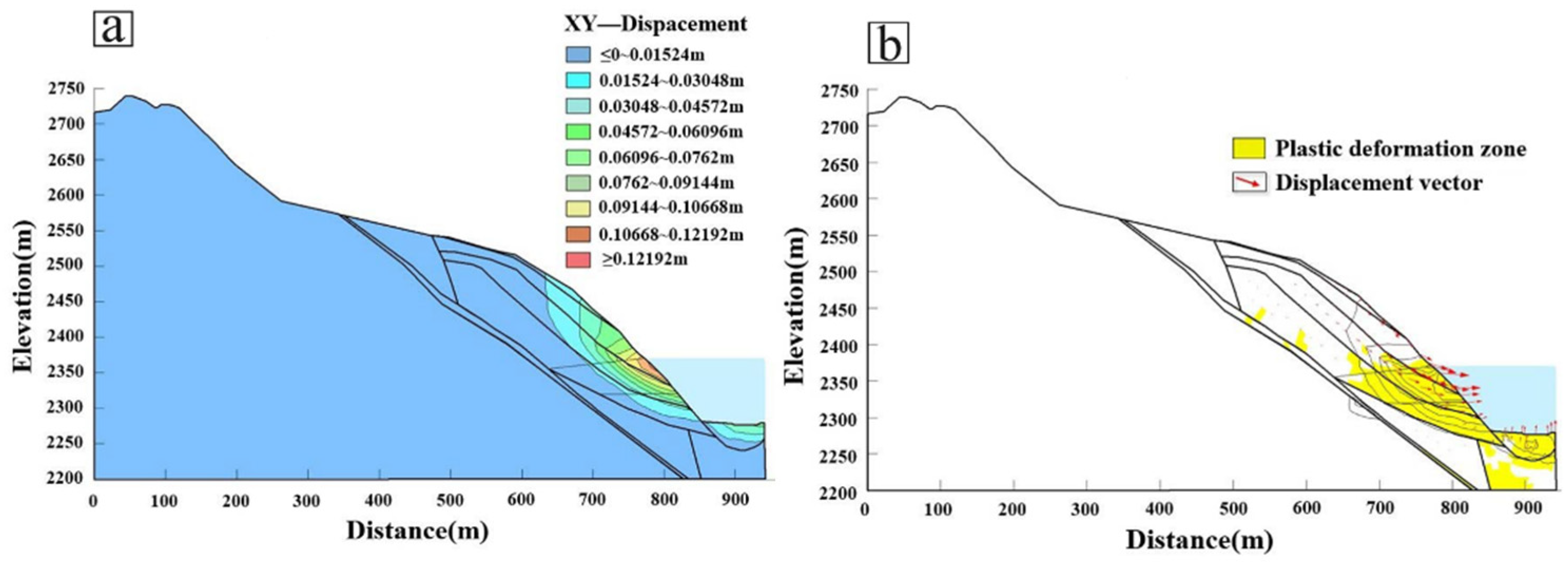
5.3. Slope Deformation Response under Water Level Variation
5.4. Discussion
6. Conclusions
- (1)
- Water level variation significantly impacts the deformation of the steep toppling bedding rock slope. Regardless of whether the water level increases or decreases, the slope deformation intensifies. Notably, the decrease in the water level has a more pronounced influence on the slope deformation, with a greater increase in the overall deformation and deformation rate.
- (2)
- By analyzing the dynamic characteristics of the groundwater seepage field, as well as the strength test under saturation and dry and wet cycling under different rise and decrease rates of the reservoir water level, it is revealed that the increase and decrease in the water level change the range of the seepage line and hydraulic gradient in the slope, and deteriorate the strength of the rock mass, which accelerates the process of slope creep fracture and bending and toppling.
- (3)
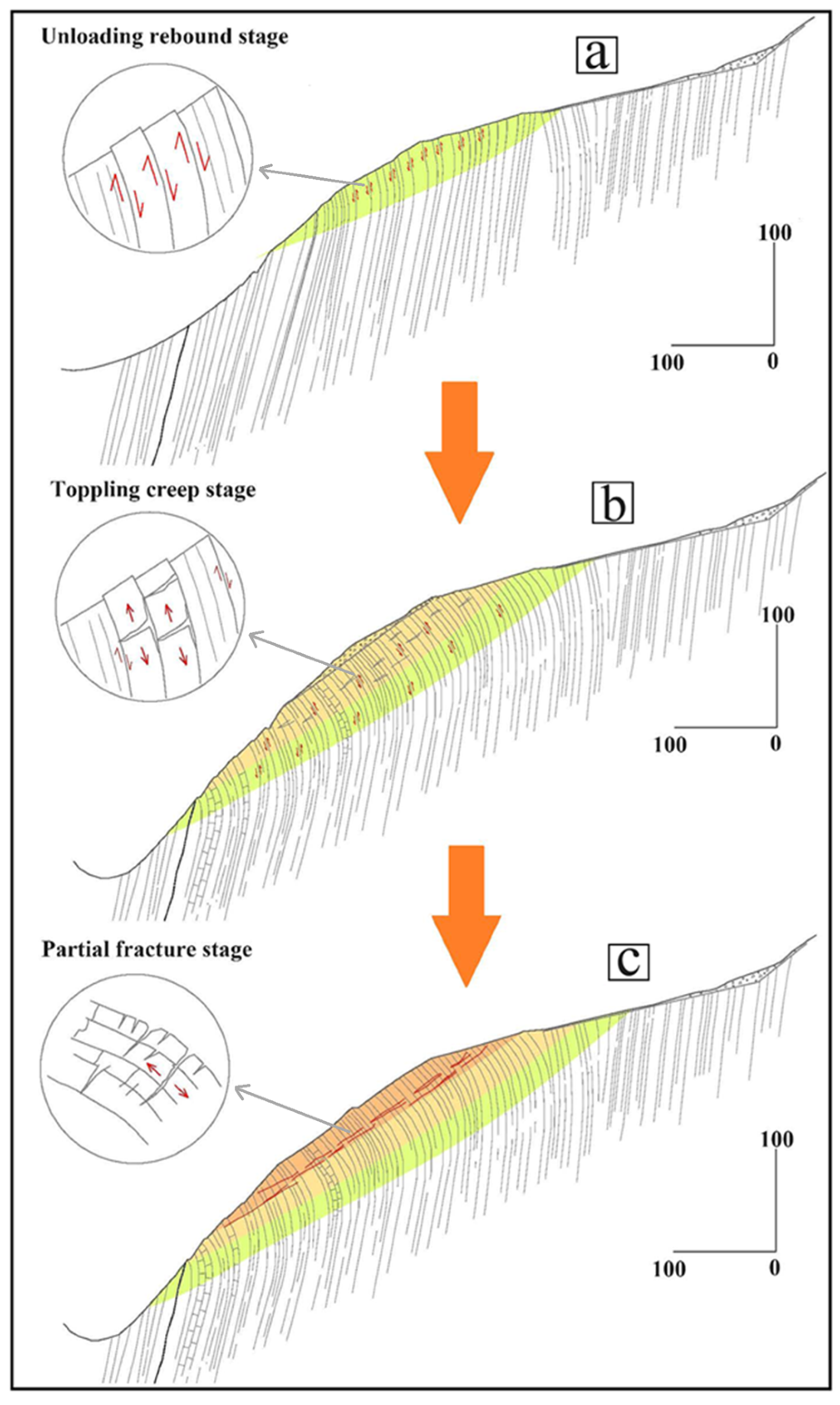
- The research shows that the evolution process of slope toppling deformation is divided into the following three stages: the unloading rebound stage, toppling creep stage and partial fracture stage. Based on the analyses of the deformation boundary, rock mass structure and deformation and failure characteristics, the deformation and failure modes are summarized as the shear dislocation, bending deformation fracture and toppling fracture.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, W. Study on the influence of water level rise and fall on the stability of dumping deformation based on numerical simulation. Henan Water Conserv. South-North Water Divers. 2022, 51, 86–87. (In Chinese) [Google Scholar]
- Miao, F.; Wu, Y.; Török, Á.; Li, L.; Xue, Y. Centrifugal model test on a riverine landslide in the Three Gorges Reservoir induced by rainfall and water level fluctuation. Geosci. Front. 2022, 13, 101378. [Google Scholar] [CrossRef]
- Chen, M.; Zheng, G.; Liu, Z.-D.; Liu, J.-J.; Lu, H.-Y. Study on the relationship between dumping deformation pattern and macro deformation characteristics of anti-dip stratiform rocky slope. Eng. Sci. Technol. 2024. (In Chinese) [Google Scholar] [CrossRef]
- Dong, M.; Zhang, F.; Lv, J.; Hu, M.; Li, Z. Study on deformation and failure law of soft-hard rock interbedding toppling slope base on similar test. Bull. Eng. Geol. Environ. 2020, 79, 4625–4637. [Google Scholar] [CrossRef]
- Hou, T.-S.; Xu, G.-L.; Zhang, D.-Q.; Liu, H.-Y. Stability analysis of Gongjiacun landslide in the three Gorges Reservoir area under the action of reservoir water level fluctuation and rainfall. Nat. Hazards 2022, 114, 1647–1683. [Google Scholar] [CrossRef]
- Chen, Q. Numerical Simulation Study on the Failure Mechanism of Dumping Deformation of Steeply Dipping Rocky Slope. Master’s Thesis, Chengdu University of Technology, Chengdu, China, 2011. (In Chinese). [Google Scholar]
- Dai, Z.; Zhang, L.; Wang, Y.; Jiang, Z.; Xu, S. Deformation and failure response characteristics and stability analysis of bedding rock slope after underground adverse slope mining. Bull. Eng. Geol. Environ. 2021, 80, 4405–4422. [Google Scholar] [CrossRef]
- Chi, E.A.; Tao, T.J.; Zhao, M.S.; Kang, Q. Failure Mode Analysis of Bedding Rock Slope Affected by Rock Mass Structural Plane. Appl. Mech. Mater. 2014, 602–605, 594–597. [Google Scholar] [CrossRef]
- Jiang, Z.; Wang, H.; Xie, W. Deformation mechanism of deposit landslide induced by fluctuations of reservoir water level based on physical model tests. Environ. Earth Sci. 2021, 80, 410. [Google Scholar] [CrossRef]
- Li, Y.; Wang, S.P.; Zhang, Z.H.; Li, S.J. Study on the Relationship between Factor of Safety and Angle of Dip of Bedding Rock Slope when Exposed to Reservoir Water Level Fluctuation. Adv. Mater. Res. 2012, 446–449, 3894–3899. [Google Scholar] [CrossRef]
- Bu, L.; Xu, T.; Zhang, Y.J.; Xu, Q. Stability Analysis of Steep Bedding Rock Slope with Strength Reduction Method. Appl. Mech. Mater. 2014, 501–504, 399–402. [Google Scholar] [CrossRef]
- Ren, G.; Xia, M.; Li, G.; Liu, C.; Zhang, F.-R. Study on the deformation and failure characteristics of steeply dipping rocky slopes. Rock Mech. Eng. 2009, 28 (Suppl. 1), 3193–3200. (In Chinese) [Google Scholar]
- Ren, G.; Xia, M.; Zeng, Q.; Jia, Y.; Lv, S.-D.; Lu, D.-L.; Liu, R.-Q.; Zhao, H.-Y.; Gou, F.-M. Characteristics and formation mechanism of typical slide-dump landslides in the main stream of Bailongjiang River. J. Chengdu Univ. Technol. (Nat. Sci. Ed.) 2015, 42, 18–25. (In Chinese) [Google Scholar]
- Wang, R.; Zheng, Y.; Chen, C.; Zhang, W.; Sun, C. Stability Analysis of Antidip Bedding Rock Slopes with Very Low-Persistent Cross Joints Using a Limit-Equilibrium Model and Fracture Mechanics. Int. J. Geomech. 2023, 23, 04023039. [Google Scholar] [CrossRef]
- Wang, R.; Zheng, Y.; Chen, C.; Zhang, W. Theoretical and numerical analysis of flexural toppling failure in soft-hard interbedded anti-dip rock slopes. Eng. Geol. 2023, 312, 106923. [Google Scholar] [CrossRef]
- Xiong, X.; Shi, Z.; Xiong, Y.; Peng, M.; Ma, X.; Zhang, F. Unsaturated slope stability around the Three Gorges Reservoir under various combinations of rainfall and water level fluctuation. Eng. Geol. 2019, 261, 105231. [Google Scholar] [CrossRef]
- Cao, Z.; Tang, J.; Zhao, X.; Zhang, Y.; Wang, B.; Li, L.; Guo, F. Failure Mechanism of Colluvial Landslide Influenced by the Water Level Change in the Three Gorges Reservoir Area. Geofluids 2021, 2021, 6865129. [Google Scholar] [CrossRef]
- Fu, W.; Zhang, J.; Zhan, Y. Study on failure mechanism and stability of soil slope on reservoir bank under water level rise and fall. Subgrade Eng. 2021, 6, 43–47. (In Chinese) [Google Scholar] [CrossRef]
- Hu, X.; Tang, H.; Li, C.; Sun, R. Stability of Huangtupo riverside slumping mass II# under water level fluctuation of Three Gorges Reservoir. J. Earth Sci. 2012, 23, 326–334. [Google Scholar] [CrossRef]
- Shoaib, M.; Yang, W.; Liang, Y.; Rehman, G. Stability and Deformation Analysis of Landslide under Coupling Effect of Rainfall and Reservoir Drawdown. Civ. Eng. J. 2021, 7, 1098–1111. [Google Scholar] [CrossRef]
- Ou, M. Research on the influence of rapid rise and fall of water level on the stability of earth dam. Hydraul. Sci. Cold Zone Eng. 2022, 5, 11–14. (In Chinese) [Google Scholar]
- Pang, L.; Li, C.; Liu, D.; Zhang, F.; Chen, B. Response of Guobu Slope Displacement to Rainfall and Reservoir Water Level with Time-Series InSAR and Wavelet Analysis. Appl. Sci. 2023, 13, 5141. [Google Scholar] [CrossRef]
- Xu, P.; Chen, J.; Huang, R.; Yan, M. Discussion on the deformation mechanism of Jiefanggou anti-dipping high slope of Jinping Hydropower Station. J. Eng. Geol. 2004, 12, 247–252. (In Chinese) [Google Scholar]
- Xu, W.; Wang, L.; Hu, R. Flow-solid interaction characteristics and stability analysis of large soil-rock mixed slope under the action of reservoir water level rise. Chin. J. Rock Mech. Eng. 2009, 28, 1491–1498. (In Chinese) [Google Scholar]
- Yang, B.; Yin, K.; Xiao, T.; Chen, L.; Du, J. Annual variation of landslide stability under the effect of water level fluctuation and rainfall in the Three Gorges Reservoir, China. Environ. Earth Sci. 2017, 76, 564. [Google Scholar] [CrossRef]
- Yang, H.; Song, K.; Chen, L.; Qu, L. Hysteresis effect and seasonal step-like creep deformation of the Jiuxianping landslide in the Three Gorges Reservoir region. Eng. Geol. 2023, 317, 107089. [Google Scholar] [CrossRef]
- Yang, Z.; Xi, W.; Yang, Z.; Shi, Z.; Huang, G.; Guo, J.; Yang, D. Time-Lag Response of Landslide to Reservoir Water Level Fluctuations during the Storage Period: A Case Study of Baihetan Reservoir. Water 2023, 15, 2732. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, Z.; Xue, S.; Wang, R.; Xiao, M. Stability analysis of a typical landslide mass in the Three Gorges Reservoir under varying reservoir water levels. Environ. Earth Sci. 2020, 79, 243–254. [Google Scholar] [CrossRef]
- Jadid, R.; Montoya, B.M.; Bennett, V.; Gabr, M.A. Effect of repeated rise and fall of water level on seepage-induced deformation and related stability analysis of Princeville levee. Eng. Geol. 2020, 266, 105458. [Google Scholar] [CrossRef]
- Liao, K.; Wu, Y.; Miao, F. System reliability analysis of landslides subjected to fluctuation of reservoir water level: A case study in the Three Gorges Reservoir area, China. Bull. Eng. Geol. Environ. 2022, 81, 225. [Google Scholar] [CrossRef]
- Liu, H.; Li, Y.; Chen, L. Mechanism of difference in motion mode in the northern and southern sections of the Huya fault zone on the eastern margin of the Tibetan Plateau: Constraints from numerical simulation. Chin. J. Geophys. 2023, 66, 2757–2771. (In Chinese) [Google Scholar]









| Number | Length (m) | Width (m) | Depth (m) | Platform Height (m) | Shape | Strike Angle |
|---|---|---|---|---|---|---|
| 1 | 25 | 2 × 10−2– 3 × 10−2 | 1 × 10−1 | 1 × 10−2– 2 × 10−2 | Dentation | 10–20° NW |
| 2 | 30 | 3 × 10−3– 5 × 10−3 | Les than 1 × 10−2 | 30° NW | ||
| 3 | 55 | 2 × 10−1– 3 × 10−1 | 1–2 | 3 × 10−1– 4 × 10−1 | Dentation | 50° NW |
| 4 | 110 | SN-50° NW | ||||
| 5 | 90 | 2 × 10−1– 3 × 10−1 | 3 | 2 × 10−2– 5 × 10−2 | Dentation and wavy | 13° NW-SN |
| 6 | 140 | 2 × 10−1– 5 × 10−1 | 3 | 2 × 10−2– 5 × 10−2 | Dentation | |
| 7 | 29 | 5 × 10−2 | 1 × 10−1– 2 × 10−1 | 50–80° NW |
| Category | A | B | C | D | E | F | G | H |
|---|---|---|---|---|---|---|---|---|
| Osmotic coefficient (m/s) | 3 × 10−5 | 3 × 10−5 | 6 × 10−6 | 3 × 10−6 | 1 × 10−6 | 3 × 10−6 | 9 × 10−9 | 1 × 10−8 |
| Saturated water content (%) | 60 | 60 | 14 | 15 | 16 | 16 | 15 | 18 |
| Density (g/cm3) | 2.14 | 2.51 | 7.65 | 2.51 | 2.5 | 2.39 | 2.5 | 2.39 |
| Poisson’s ratio | 0.33 | 0.25 | 0.35 | 0.33 | 0.33 | 0.33 | 0.25 | 0.24 |
| Cohesion (×10 kPa) | 0 | 0 | 7.5 | 20 | 27 | 10 | 43 | 40 |
| Frictional angle (°) | 28 | 42.5 | 26.5 | 28.5 | 29 | 26.5 | 41 | 40 |
| Elasticity modulus (×106 kPa) | 0.1 | 5 | 0.35 | 0.75 | 1 | 0.35 | 6.5 | 5 |
| Water Level Fluctuation | V1 (m/day) | V2 (m/day) | V3 (m/day) |
|---|---|---|---|
| 2320–2370 (rise) | 0.4 | 0.8 | 1.6 |
| 2370–2320 (decrease) | 0.4 | 0.8 | 1.6 |
| Category | Density (g/cm3) | Deformation Modulus (×105 kPa) | Poisson’s Rate | Cohesion (×10 kPa) | Frictional Angle (°) |
|---|---|---|---|---|---|
| I | 2.1 | 1 | 0.35 | 0 | 28 |
| C | 2.35 | 3.5 | 0.35 | 7.5 | 26.5 |
| D | 2.45 | 7.5 | 0.35 | 20 | 28.5 |
| E | 2.45 | 15 | 0.3 | 28 | 29 |
| J | 2.45 | 20 | 0.35 | 38 | 37 |
| K | 2.45 | 40 | 0.3 | 28 | 32 |
| F | 2.35 | 3.5 | 0.35 | 10 | 26.5 |
| L | 2.45 | 65 | 0.25 | 42 | 42 |
| Category | Water Level Variation Cycles | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|---|
| L | Elasticity modulus (×106 kPa) | 6.50 | 5.75 | 5.40 | 5.01 | 4.81 |
| Viscoelasticity modulus (×106 kPa) | 10.89 | 9.63 | 9.04 | 8.40 | 8.05 | |
| Poisson’s rate | 0.30 | 0.31 | 0.31 | 0.31 | 0.31 | |
| Cohesion (kPa) | 252 | 202 | 177 | 160 | 150 | |
| Frictional angle (°) | 39.0 | 37.9 | 37.3 | 37.0 | 36.8 | |
| F | Elasticity modulus (×106 kPa) | 0.35 | 0.30 | 0.29 | 0.27 | 0.25 |
| Viscoelasticity modulus (×106 kPa) | 0.59 | 0.51 | 0.48 | 0.46 | 0.43 | |
| Poisson’s rate | 0.38 | 0.39 | 0.39 | 0.39 | 0.39 | |
| Cohesion (kPa) | 60 | 48 | 42 | 38 | 36 | |
| Frictional angle (°) | 24.2 | 23.3 | 22.9 | 22.7 | 22.5 | |
| E | Elasticity modulus (×106 kPa) | 1.5 | 1.32 | 1.24 | 1.16 | 0.63 |
| Viscoelasticity modulus (×106 kPa) | 2.45 | 2.16 | 2.03 | 1.89 | 1.03 | |
| Poisson’s rate | 0.34 | 0.35 | 0.35 | 0.35 | 0.35 | |
| Cohesion (kPa) | 168 | 134 | 118 | 106 | 100 | |
| Frictional angle (°) | 26.5 | 25.6 | 25.1 | 24.9 | 24.7 | |
| C | Elasticity modulus (×106 kPa) | 0.75 | 0.67 | 0.62 | 0.59 | 0.55 |
| Viscoelasticity modulus (×106 kPa) | 1.19 | 1.06 | 0.98 | 0.93 | 0.88 | |
| Poisson’s rate | 0.38 | 0.39 | 0.39 | 0.39 | 0.39 | |
| Cohesion (kPa) | 150 | 120 | 106 | 95 | 89 | |
| Frictional angle (°) | 26.0 | 25.1 | 24.7 | 24.5 | 24.3 | |
| D | Elasticity modulus (×106 kPa) | 0.35 | 0.30 | 0.29 | 0.27 | 0.25 |
| Viscoelasticity modulus (×106 kPa) | 0.59 | 0.51 | 0.48 | 0.46 | 0.43 | |
| Poisson’s rate | 0.38 | 0.39 | 0.39 | 0.39 | 0.39 | |
| Cohesion (kPa) | 450 | 360 | 317 | 285 | 268 | |
| Frictional angle (°) | 24.2 | 23.3 | 22.9 | 22.7 | 22.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, T.; Ran, W.; Wei, K.; Guo, J.; Chen, S.; Li, X.; Chen, M.; Pei, X. Influence Mechanism of Water Level Variation on Deformation of Steep and Toppling Bedding Rock Slope. Water 2024, 16, 2706. https://doi.org/10.3390/w16192706
Li T, Ran W, Wei K, Guo J, Chen S, Li X, Chen M, Pei X. Influence Mechanism of Water Level Variation on Deformation of Steep and Toppling Bedding Rock Slope. Water. 2024; 16(19):2706. https://doi.org/10.3390/w16192706
Chicago/Turabian StyleLi, Tiantao, Weiling Ran, Kaihong Wei, Jian Guo, Shihua Chen, Xuan Li, Mingyang Chen, and Xiangjun Pei. 2024. "Influence Mechanism of Water Level Variation on Deformation of Steep and Toppling Bedding Rock Slope" Water 16, no. 19: 2706. https://doi.org/10.3390/w16192706
APA StyleLi, T., Ran, W., Wei, K., Guo, J., Chen, S., Li, X., Chen, M., & Pei, X. (2024). Influence Mechanism of Water Level Variation on Deformation of Steep and Toppling Bedding Rock Slope. Water, 16(19), 2706. https://doi.org/10.3390/w16192706






