Author Contributions
Conceptualization, X.L. (Xinglu Liu) and X.L. (Xiaofeng Luo); methodology, X.L. (Xiaofeng Luo) and C.L.; software, X.L. (Xinglu Liu); validation, X.L. (Xinglu Liu), G.Z. and W.D.; formal analysis, X.L. (Xinglu Liu); investigation, X.L. (Xinglu Liu), C.L. and G.Z; writing—original draft preparation, X.L. (Xinglu Liu); writing—review and editing, X.L. (Xiaofeng Luo) and C.L.; figure, X.L. (Xinglu Liu) and C.L.; supervision, W.D. All authors have read and agreed to the published version of the manuscript.
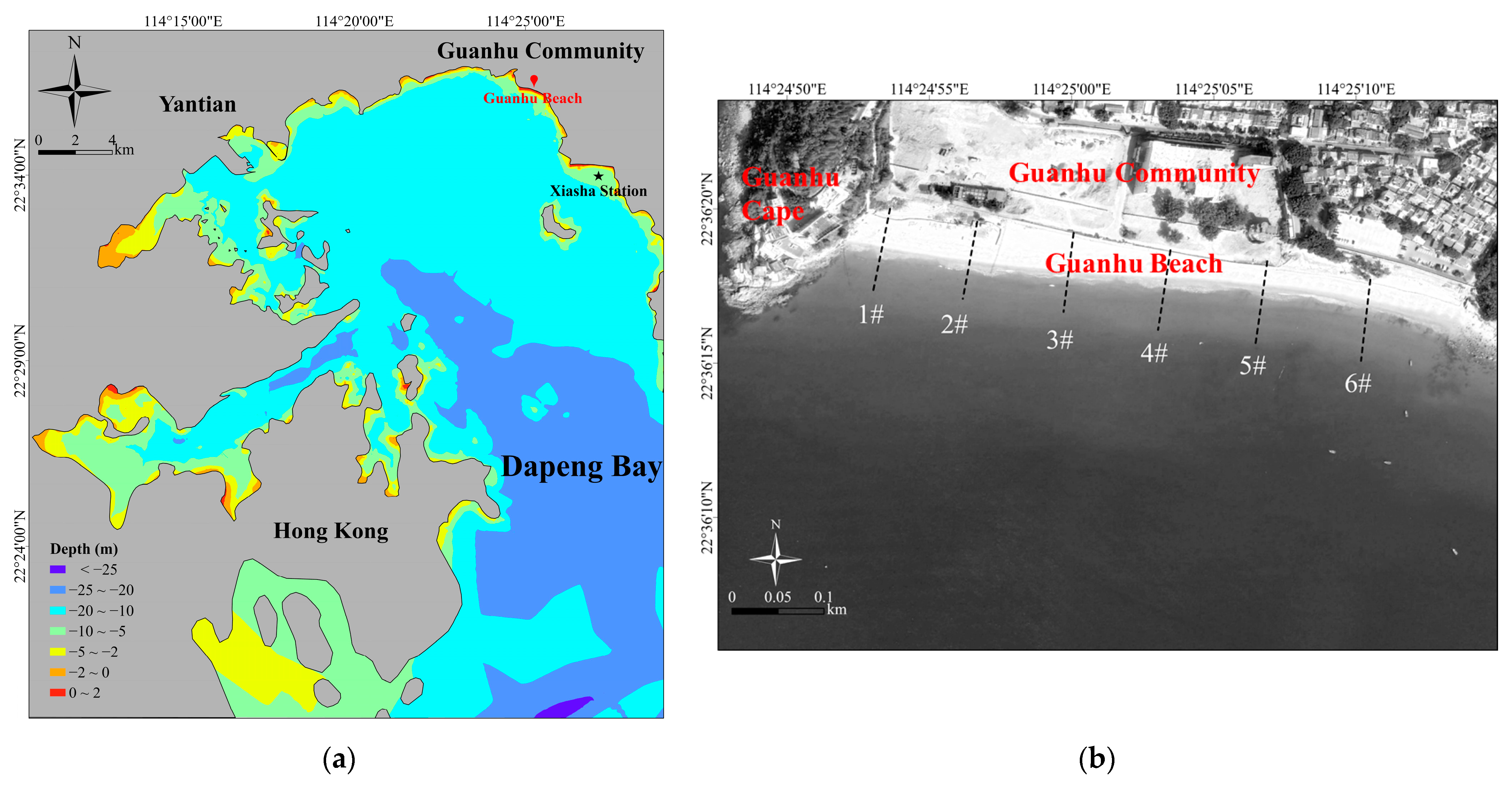
Figure 1.
The description of the study area with the focus area on Guanhu Beach: (a) The topography and bathymetry of Dapeng Bay. (b) The distribution of Guanhu Beach and measurement profile.
Figure 1.
The description of the study area with the focus area on Guanhu Beach: (a) The topography and bathymetry of Dapeng Bay. (b) The distribution of Guanhu Beach and measurement profile.
Figure 2.
Wave conditions of the study area (measured by Xiasha station with a collection frequency of every two hours): (a) Significant wave height. (b) Wave direction frequency.
Figure 2.
Wave conditions of the study area (measured by Xiasha station with a collection frequency of every two hours): (a) Significant wave height. (b) Wave direction frequency.
Figure 3.
Field survey conduction: (a) Guanhu beach. (b) Real Time Kinematic (RTK) measurement.
Figure 3.
Field survey conduction: (a) Guanhu beach. (b) Real Time Kinematic (RTK) measurement.
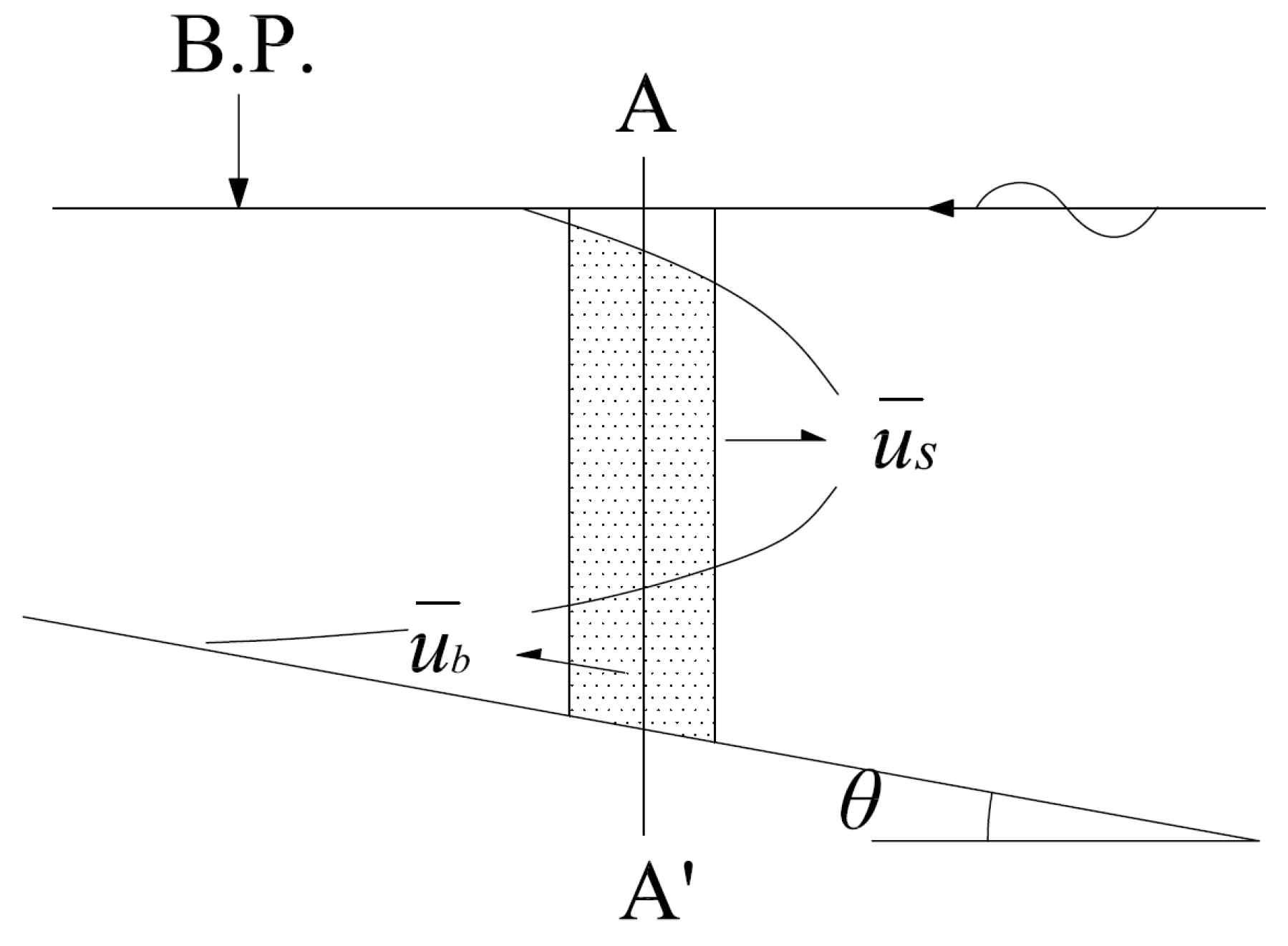
Figure 4.
Illustration of the Bagnold energy conservation method: B.P. stands for Barometric Pressure; A-A’ is a vertical cross-section located just outside the breaking point of the waves.
Figure 4.
Illustration of the Bagnold energy conservation method: B.P. stands for Barometric Pressure; A-A’ is a vertical cross-section located just outside the breaking point of the waves.
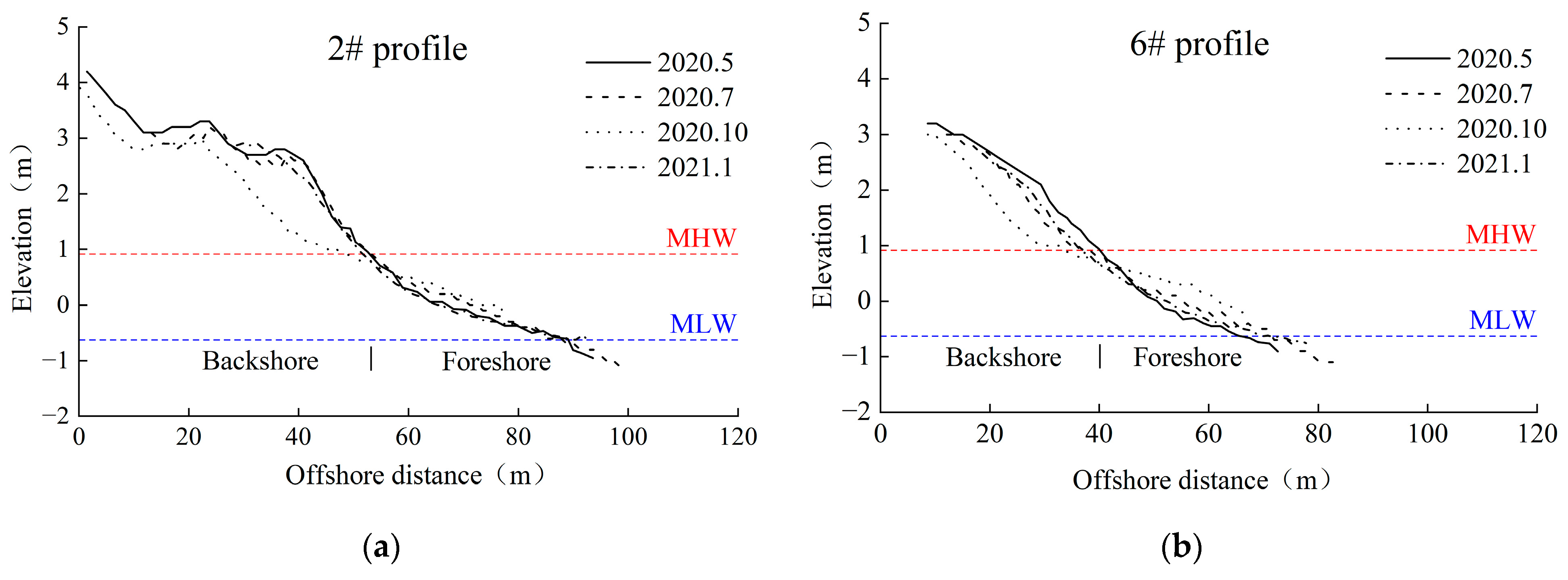
Figure 5.
Seasonal variation in typical profile: (a) Beach evolution of 2# profile. (b) Beach evolution of 6# profile. The berm at Guan Hu Beach has a consistent elevation of 3 m and a width of 30 m. Between July and October 2020, significant erosion was observed, resulting in a change in the foreshore slope from 1:8 to 1:25.
Figure 5.
Seasonal variation in typical profile: (a) Beach evolution of 2# profile. (b) Beach evolution of 6# profile. The berm at Guan Hu Beach has a consistent elevation of 3 m and a width of 30 m. Between July and October 2020, significant erosion was observed, resulting in a change in the foreshore slope from 1:8 to 1:25.
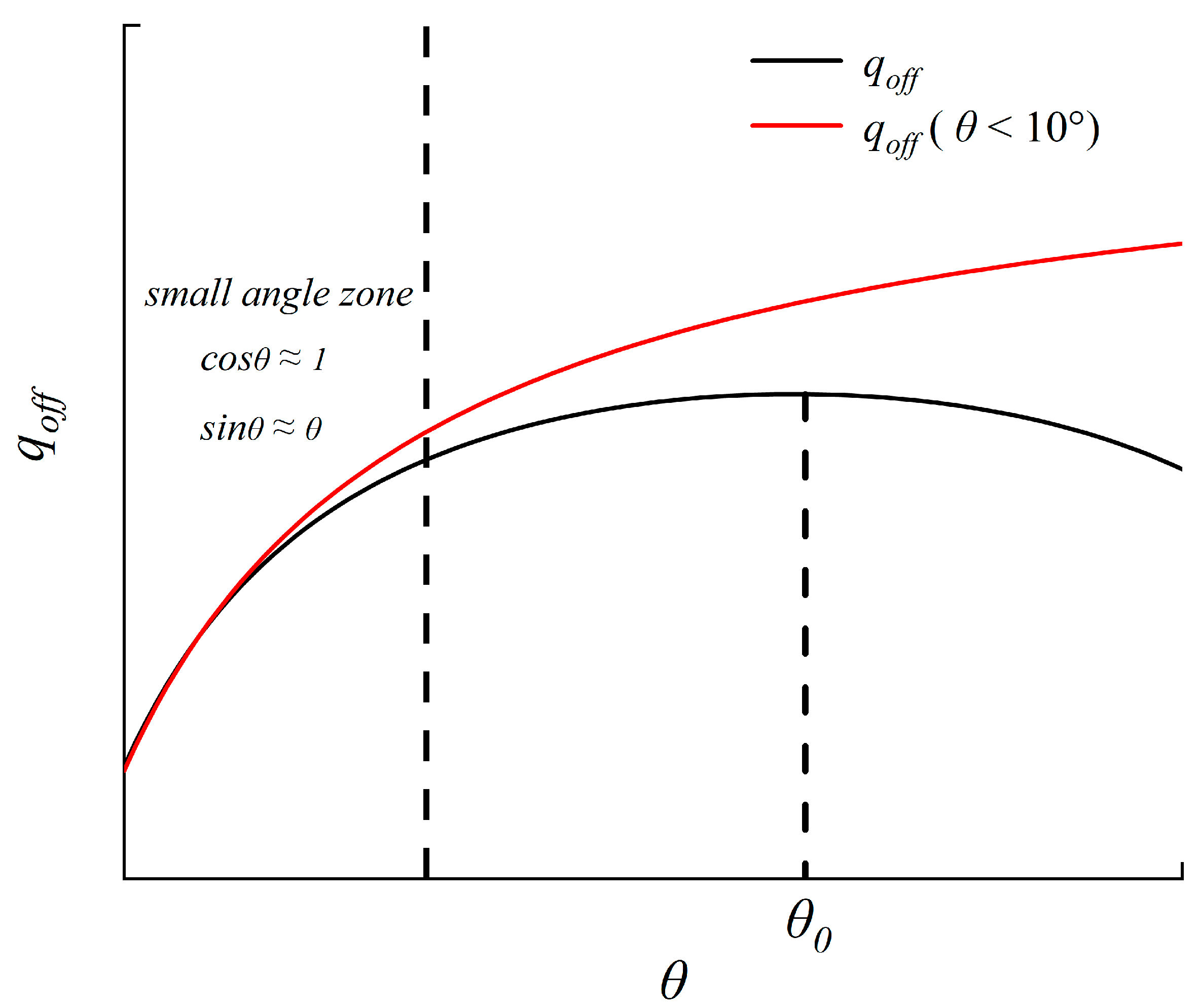
Figure 6.
Relationship between qoff and θ. The black line represents Equation (18), while the red line represents Equation (19). In the small-angle zone with angles less than 10°, the values of the two equations can be considered similar, as the red line nearly coincides with the black line.
Figure 6.
Relationship between qoff and θ. The black line represents Equation (18), while the red line represents Equation (19). In the small-angle zone with angles less than 10°, the values of the two equations can be considered similar, as the red line nearly coincides with the black line.
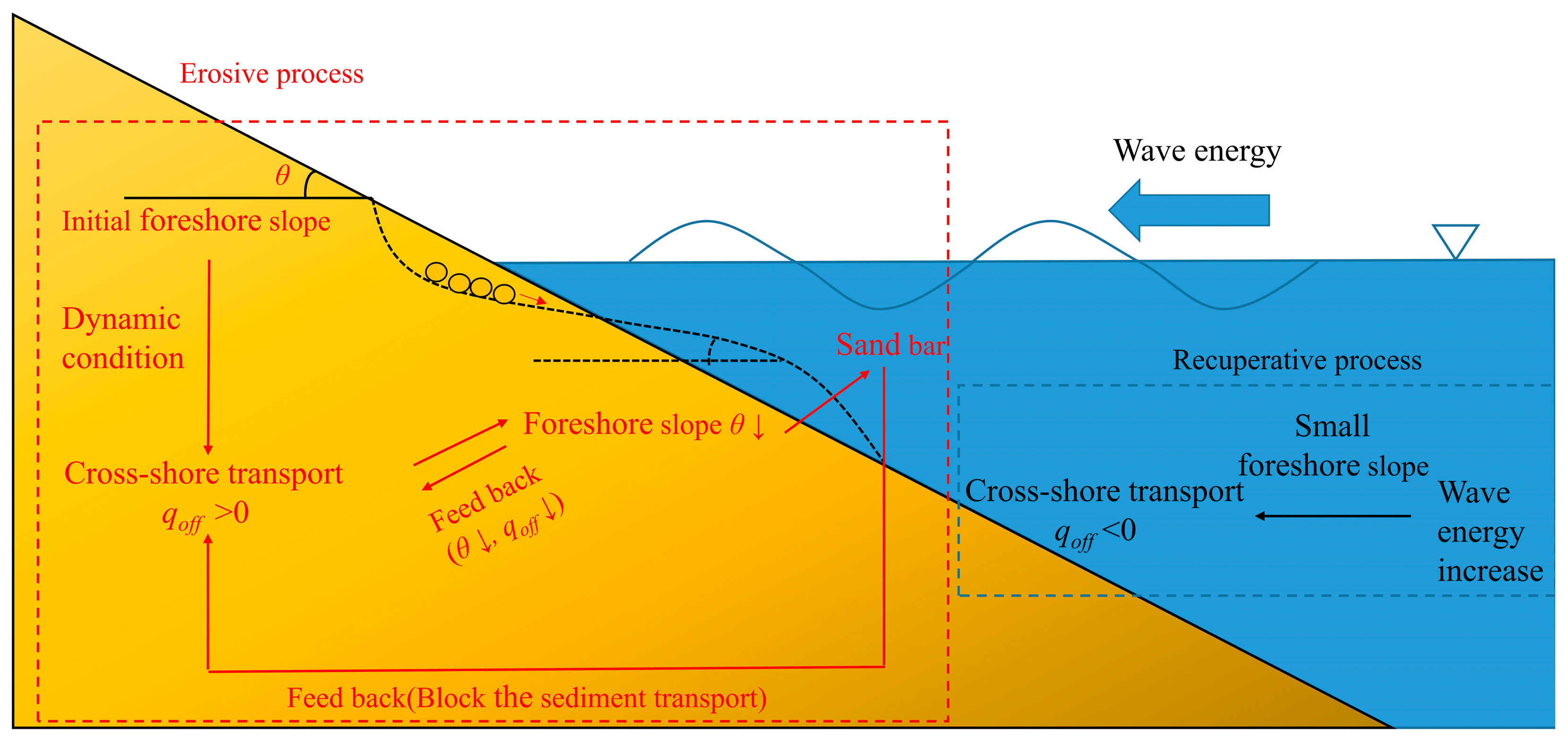
Figure 7.
The feedback process between cross-shore sediment transport and foreshore slope. The red box illustrates the erosive process, while the blue box illustrates the recuperative process. Together, these elements contribute to the seasonal changes observed on the beach.
Figure 7.
The feedback process between cross-shore sediment transport and foreshore slope. The red box illustrates the erosive process, while the blue box illustrates the recuperative process. Together, these elements contribute to the seasonal changes observed on the beach.
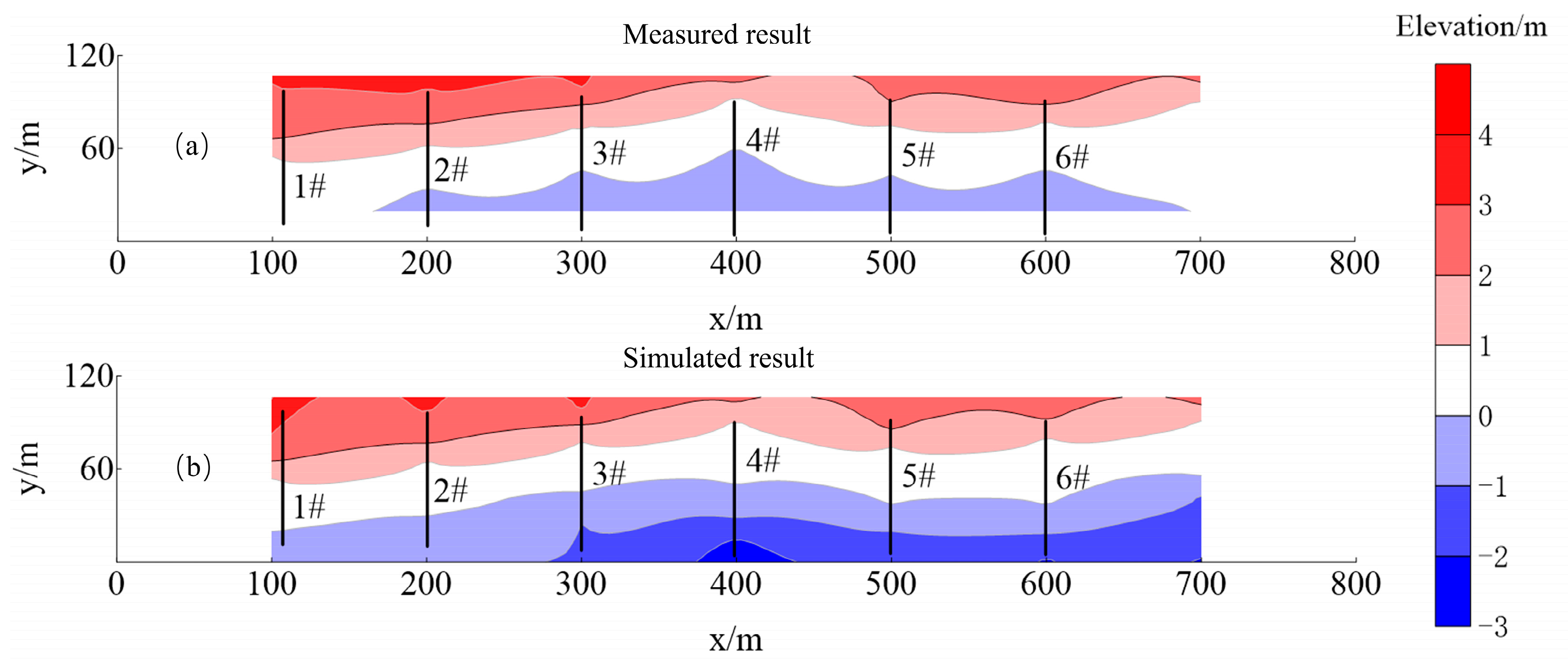
Figure 8.
Plan view of the field and numerical model: (a) Measured beach elevation in October 2020. (b) Simulated beach elevation in October 2020. The white and red regions represent land, while the blue area represents the sea. The area included in the simulation is slightly larger than the measured area.
Figure 8.
Plan view of the field and numerical model: (a) Measured beach elevation in October 2020. (b) Simulated beach elevation in October 2020. The white and red regions represent land, while the blue area represents the sea. The area included in the simulation is slightly larger than the measured area.
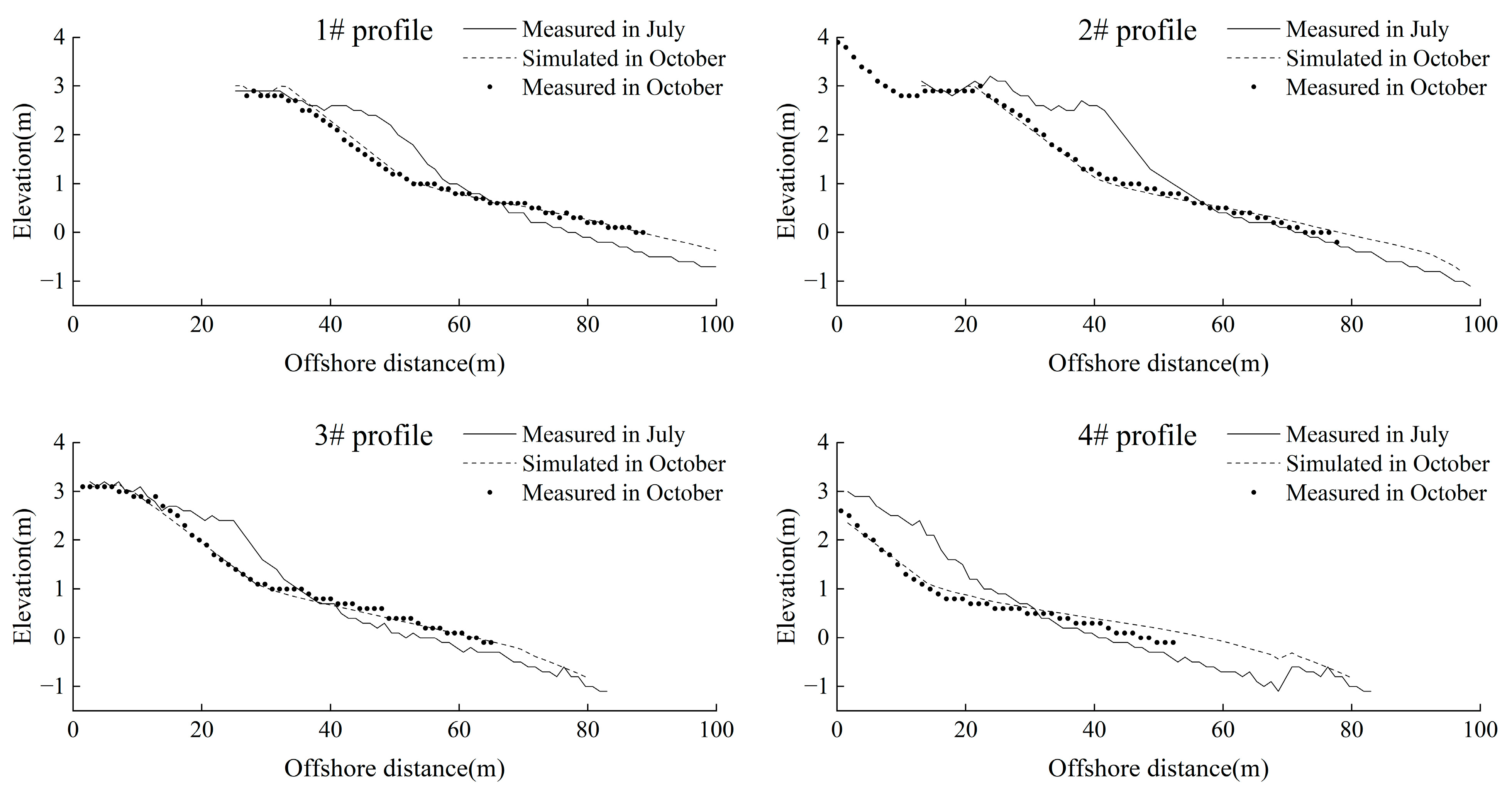
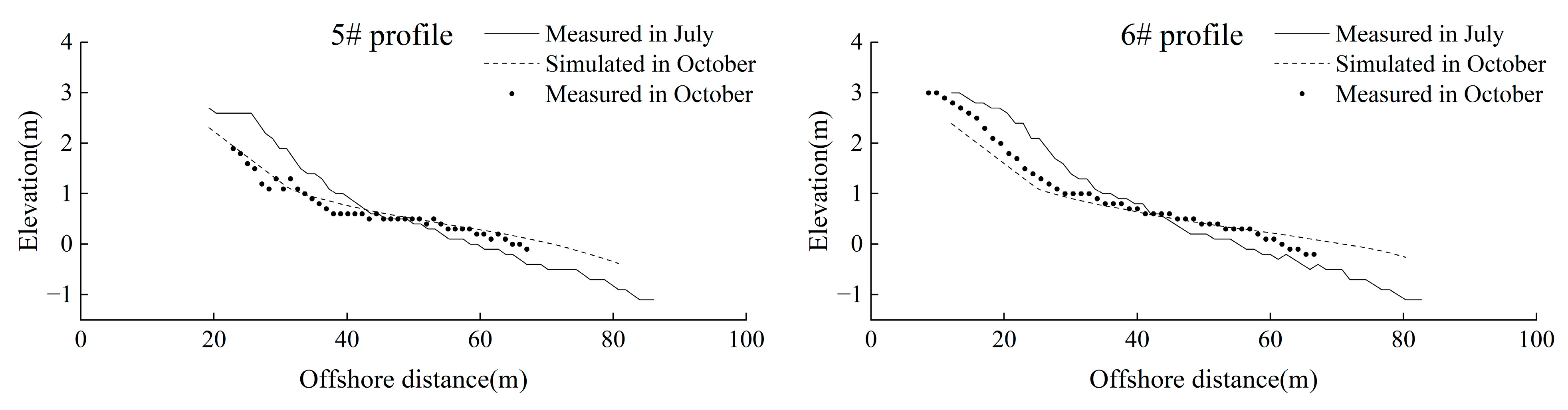
Figure 9.
Xbeach model profile validation (successively from 1# profile to 6# profile). The Brier Skill Scores for each profile exceed 0.8, while the coefficient of determination is over 0.9. These results demonstrate that the two-dimensional model effectively simulates changes in beach profiles in response to actual dynamic conditions.
Figure 9.
Xbeach model profile validation (successively from 1# profile to 6# profile). The Brier Skill Scores for each profile exceed 0.8, while the coefficient of determination is over 0.9. These results demonstrate that the two-dimensional model effectively simulates changes in beach profiles in response to actual dynamic conditions.
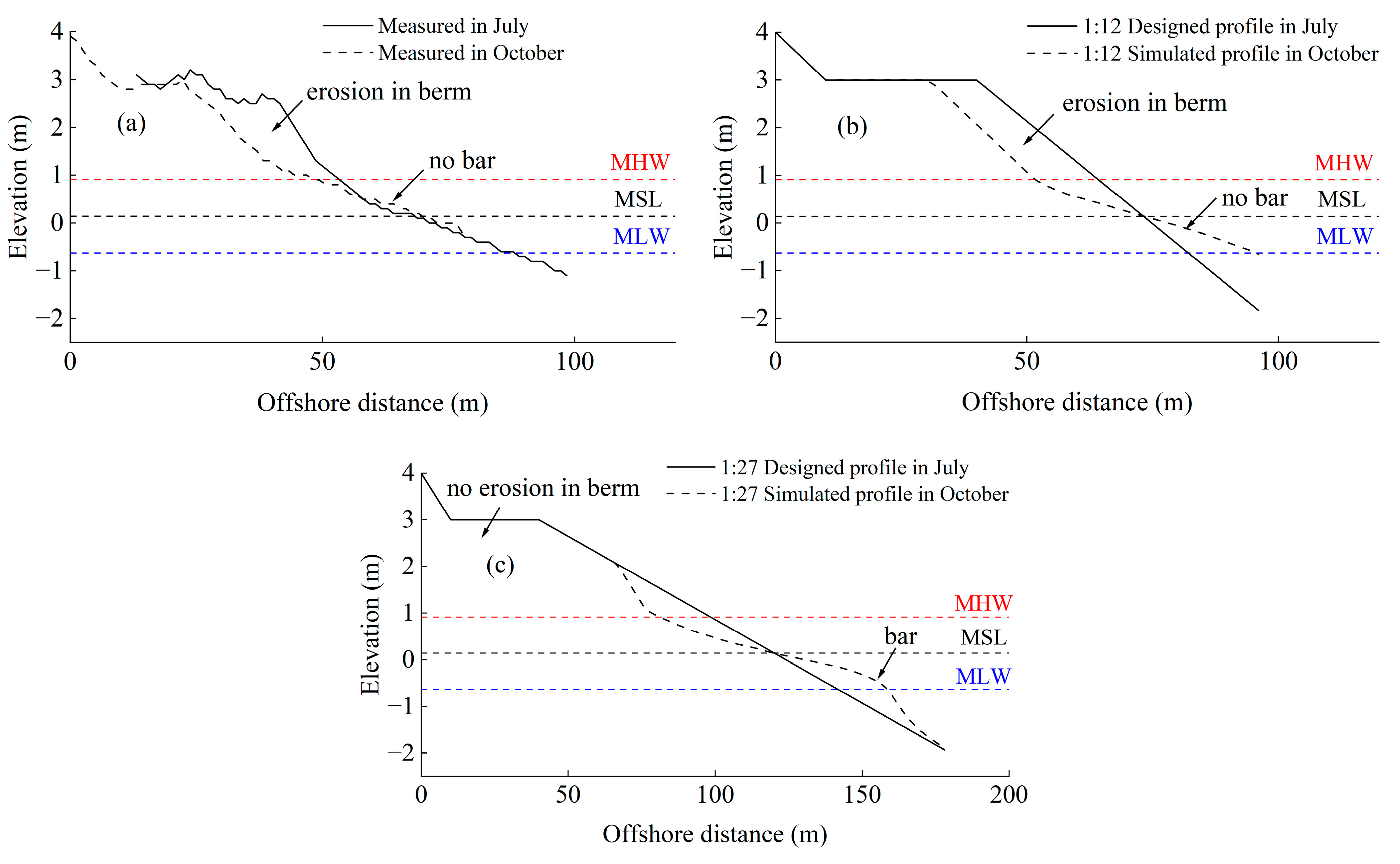
Figure 10.
Various beach profile evolution under monsoon wave conditions: (a) 1:8 initial foreshore slope (b) 1:12 initial foreshore slope; (c) 1:27 initial foreshore slope. The steep foreshore slopes (a,b) experienced direct erosion of the berm, without the formation of a sandbar, while the gentler foreshore slope (c) showed no berm erosion and resulted in the establishment of a stable sandbar.
Figure 10.
Various beach profile evolution under monsoon wave conditions: (a) 1:8 initial foreshore slope (b) 1:12 initial foreshore slope; (c) 1:27 initial foreshore slope. The steep foreshore slopes (a,b) experienced direct erosion of the berm, without the formation of a sandbar, while the gentler foreshore slope (c) showed no berm erosion and resulted in the establishment of a stable sandbar.
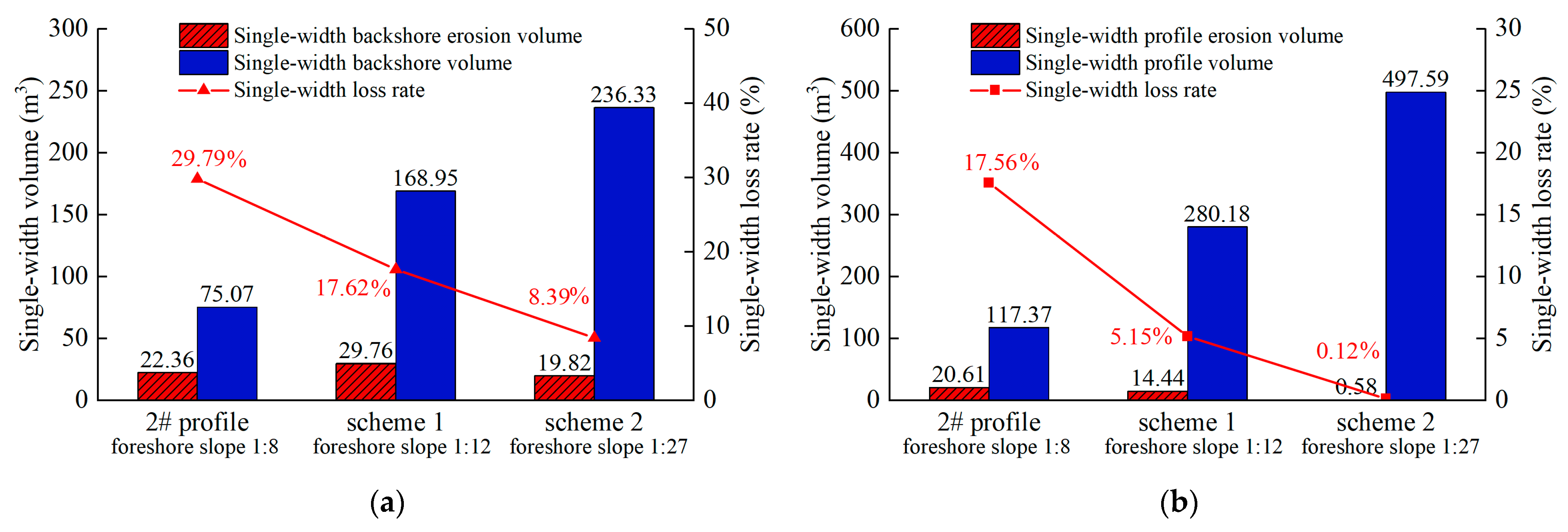
Figure 11.
Erosion and loss rate in single width of profiles with different foreshore slopes under monsoon wave conditions: (a) Only count the backshore erosion. (b) Count the whole profile erosion. Both the backshore erosion rate and the overall profile erosion rate decrease as the slope of the foreshore becomes gentler.
Figure 11.
Erosion and loss rate in single width of profiles with different foreshore slopes under monsoon wave conditions: (a) Only count the backshore erosion. (b) Count the whole profile erosion. Both the backshore erosion rate and the overall profile erosion rate decrease as the slope of the foreshore becomes gentler.
Figure 12.
Storm surge dynamic conditions under Typhoon Mangkhut. Wave data are sourced from Xiasha Station.
Figure 12.
Storm surge dynamic conditions under Typhoon Mangkhut. Wave data are sourced from Xiasha Station.
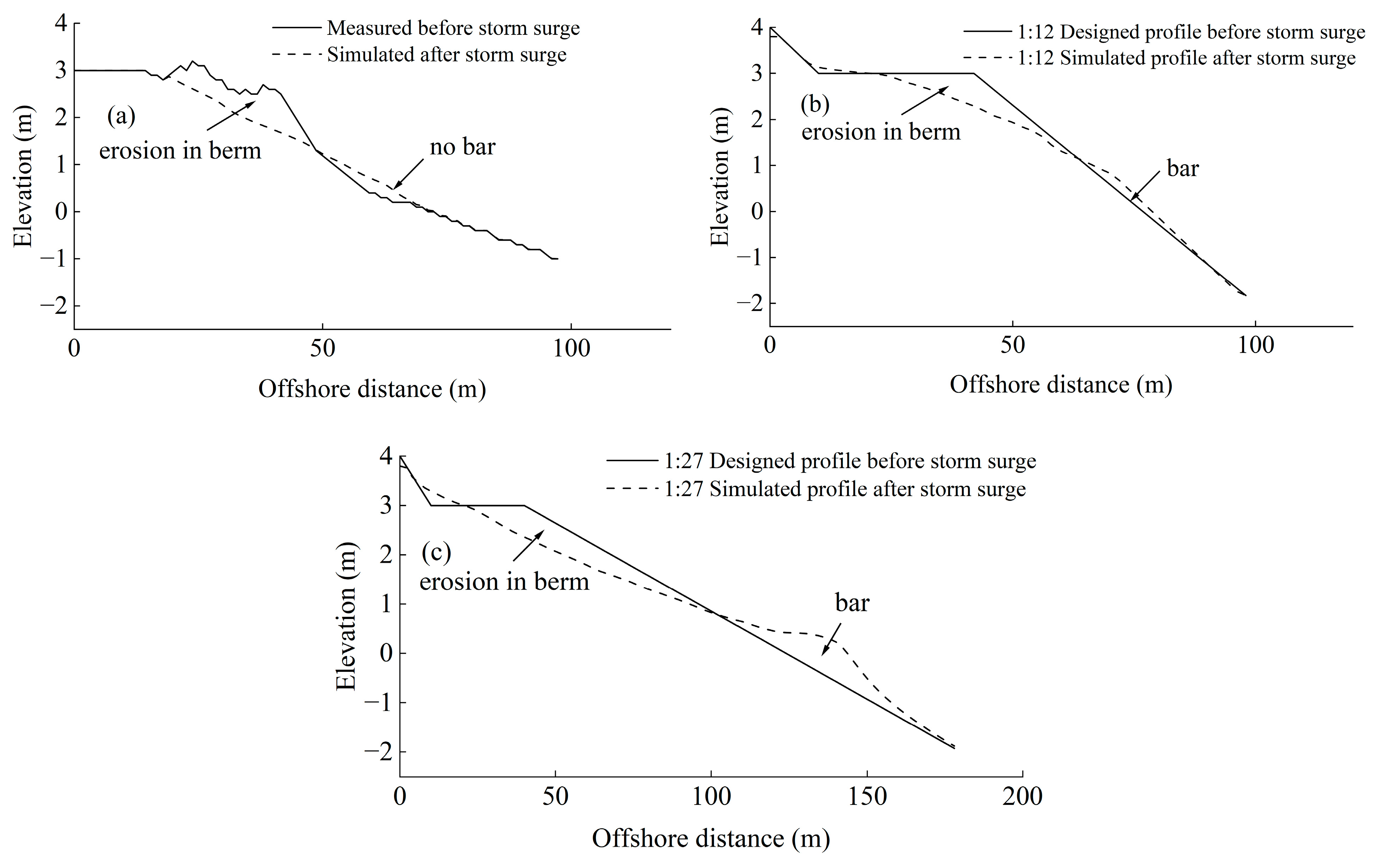
Figure 13.
Various beach profile evolution under storm surge conditions: (a) 1:8 initial foreshore slope; (b) 1:12 initial foreshore slope; (c) 1:27 initial foreshore slope. During storm surge conditions, direct erosion of the berm occurred, with (a) not resulting in the formation of a sandbar. However, as the foreshore slope became gentler, both (b) and (c) produced sandbars, with the size of the sandbar increasing as the foreshore slope decreased.
Figure 13.
Various beach profile evolution under storm surge conditions: (a) 1:8 initial foreshore slope; (b) 1:12 initial foreshore slope; (c) 1:27 initial foreshore slope. During storm surge conditions, direct erosion of the berm occurred, with (a) not resulting in the formation of a sandbar. However, as the foreshore slope became gentler, both (b) and (c) produced sandbars, with the size of the sandbar increasing as the foreshore slope decreased.
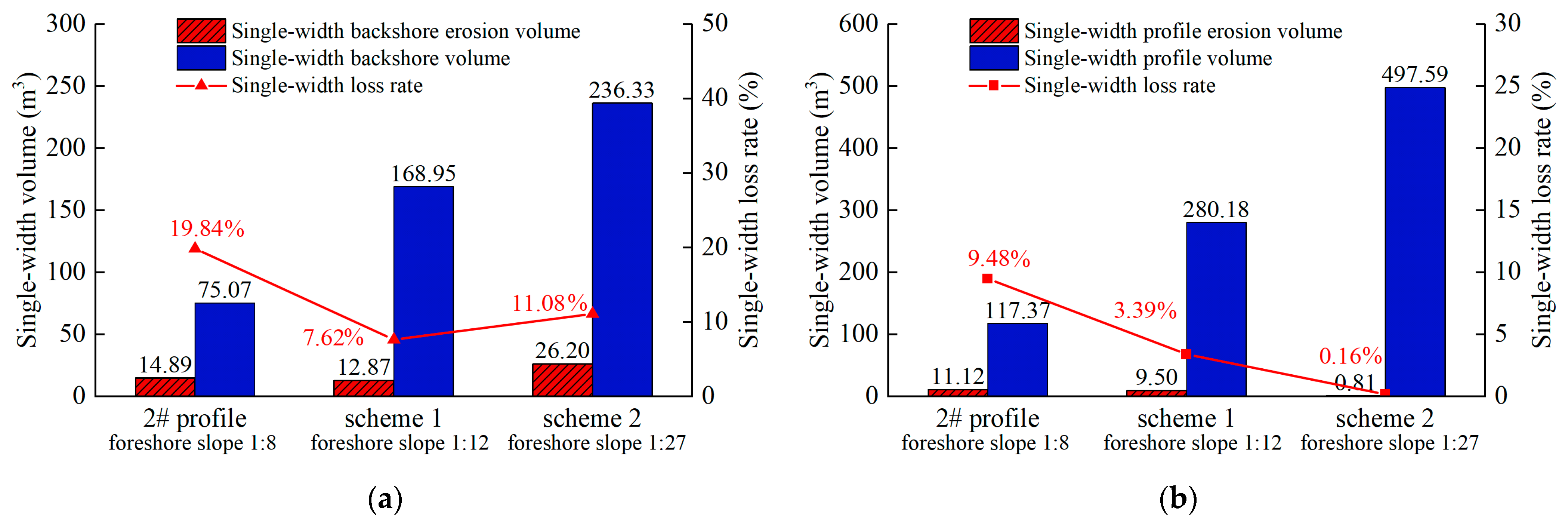
Figure 14.
Erosion and loss rate in single width of profiles with different foreshore slopes under storm surge conditions: (a) Only count the backshore erosion. (b) Count the whole profile erosion. The overall net erosion rate of the profile decreases as the foreshore slope becomes gentler. In contrast, the erosion rate of the backshore shows irregular patterns due to the complex effects of storm surges, resulting in chaotic adjustments without a clear trend.
Figure 14.
Erosion and loss rate in single width of profiles with different foreshore slopes under storm surge conditions: (a) Only count the backshore erosion. (b) Count the whole profile erosion. The overall net erosion rate of the profile decreases as the foreshore slope becomes gentler. In contrast, the erosion rate of the backshore shows irregular patterns due to the complex effects of storm surges, resulting in chaotic adjustments without a clear trend.
Table 1.
Default and Setup Values of Model Parameters.
Table 1.
Default and Setup Values of Model Parameters.
| Parameter | Default | Value | Detail |
|---|
| wavemodel | surfbeat | surfbeat | focus on swash zone |
| nx | 50 | 72 | related to beach width and measurement interval |
| ny | 2 | 6 | related to beach length |
| vardx | 0 | 1 | uneven mesh |
| Alfa/° | 0 | 67.5 | angle of x-axis from east |
| D50/m | 0.0002 | 0.0002 | average median particle size of native sediment |
| posdwn | 1 | −1 | bathymetry is specified positive up |
| CFL | 0.7 | 0.7 | maximum Courant–Friedrichs–Lewy number |
| Tstop/s | 2000 | 743,040 | stop time of simulation |
| morfac | 0 | 10 | morphological acceleration factor |
| wbctype | params | parametric | wave boundary condition type |
Table 2.
Cross-shore sediment transport of the beach profile in October.
Table 2.
Cross-shore sediment transport of the beach profile in October.
| Parameter | Profile |
|---|
| 2# | 6# |
|---|
| Hs/m | 0.33 | 0.33 |
| T/s | 5.26 | 5.26 |
| D50/mm | 0.221 | 0.203 |
| tanθ | 0.059 | 0.057 |
| C | 0.80 | 1.03 |
Table 3.
Evaluation values for XBeach model.
Table 3.
Evaluation values for XBeach model.
| Assessed Value | Profile |
|---|
| 1# | 2# | 3# | 4# | 5# | 6# |
|---|
| BBS | 0.963 | 0.917 | 0.922 | 0.843 | 0.857 | 0.855 |
| R2 | 0.995 | 0.992 | 0.994 | 0.992 | 0.951 | 0.992 |
Table 4.
Parameters of beach profiles.
Table 4.
Parameters of beach profiles.
| Name | Beach Berm Elevation | Beach Berm Width | Slope |
|---|
| 2# profile | 3.0 | 30 | 1:8 |
| Scheme 1 | 3.0 | 30 | 1:12 |
| Scheme 2 | 3.0 | 30 | 1:27 |
Table 5.
Erosion and sandbar development characteristics of profiles with different foreshore slopes under monsoon wave conditions.
Table 5.
Erosion and sandbar development characteristics of profiles with different foreshore slopes under monsoon wave conditions.
| Condition | Name | Slope | Berm Erosion | Bar | Bar Height (m) | Bar Length (m) |
|---|
| Monsoon wave | 2# profile | 1:8 | √ | × | / | / |
| Scheme 1 | 1:12 | √ | × | / | / |
| Scheme 2 | 1:27 | × | √ | 4 | 58 |
Table 6.
Erosion and sandbar development characteristics of profiles with different foreshore slopes under storm surge conditions.
Table 6.
Erosion and sandbar development characteristics of profiles with different foreshore slopes under storm surge conditions.
| Condition | Name | Slope | Berm Erosion | Bar | Bar Height (m) | Bar Length (m) |
|---|
| Storm surge | 2# profile | 1:8 | √ | × | / | / |
| Scheme 1 | 1:12 | √ | √ | 2 | 24 |
| Scheme 2 | 1:27 | √ | √ | 6 | 76 |