The Straightening of a River Meander Leads to Extensive Losses in Flow Complexity and Ecosystem Services
Abstract
1. Introduction
2. Methods
CFD Model
3. Hydraulic Complexity Variables
4. Results
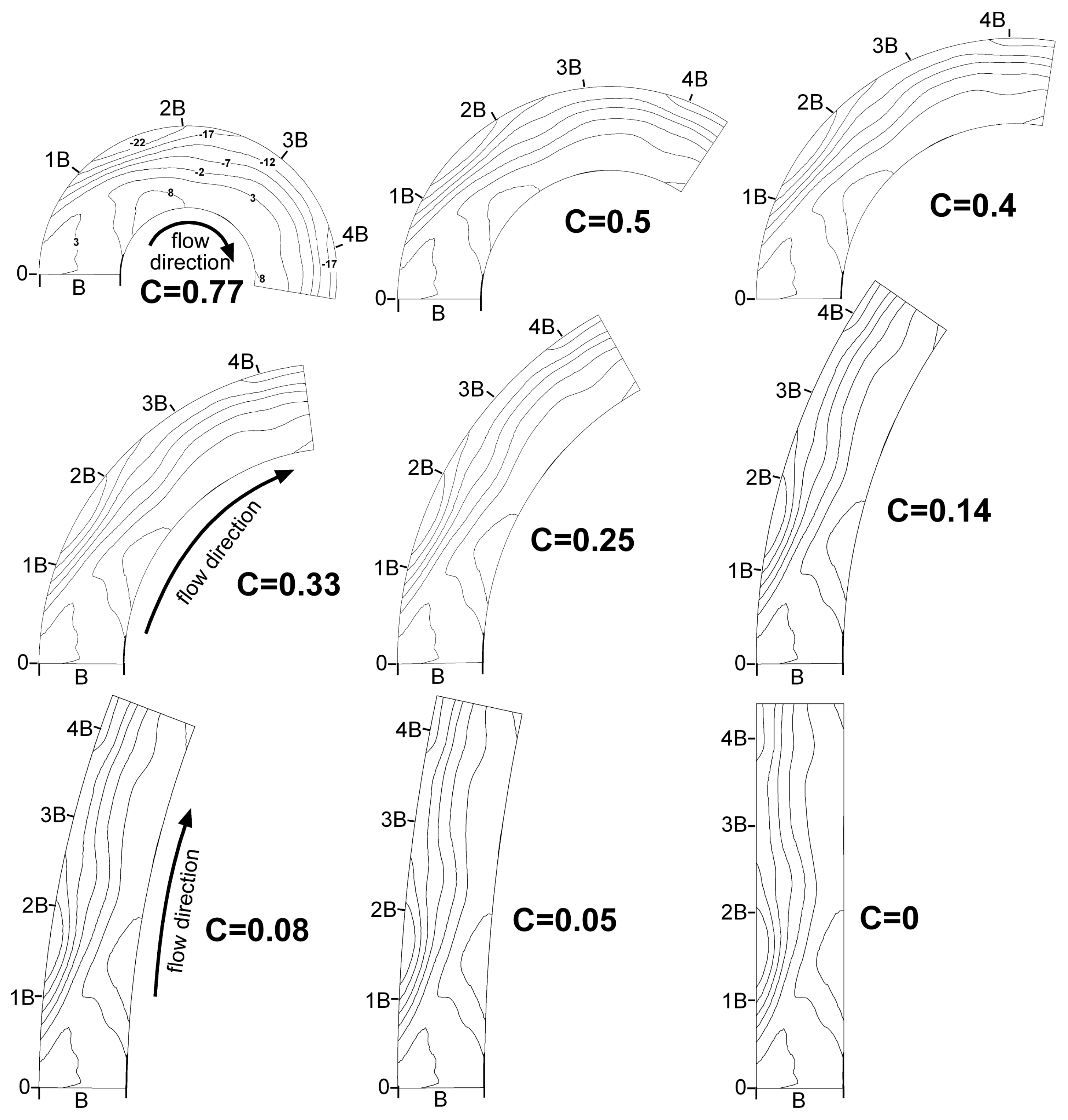
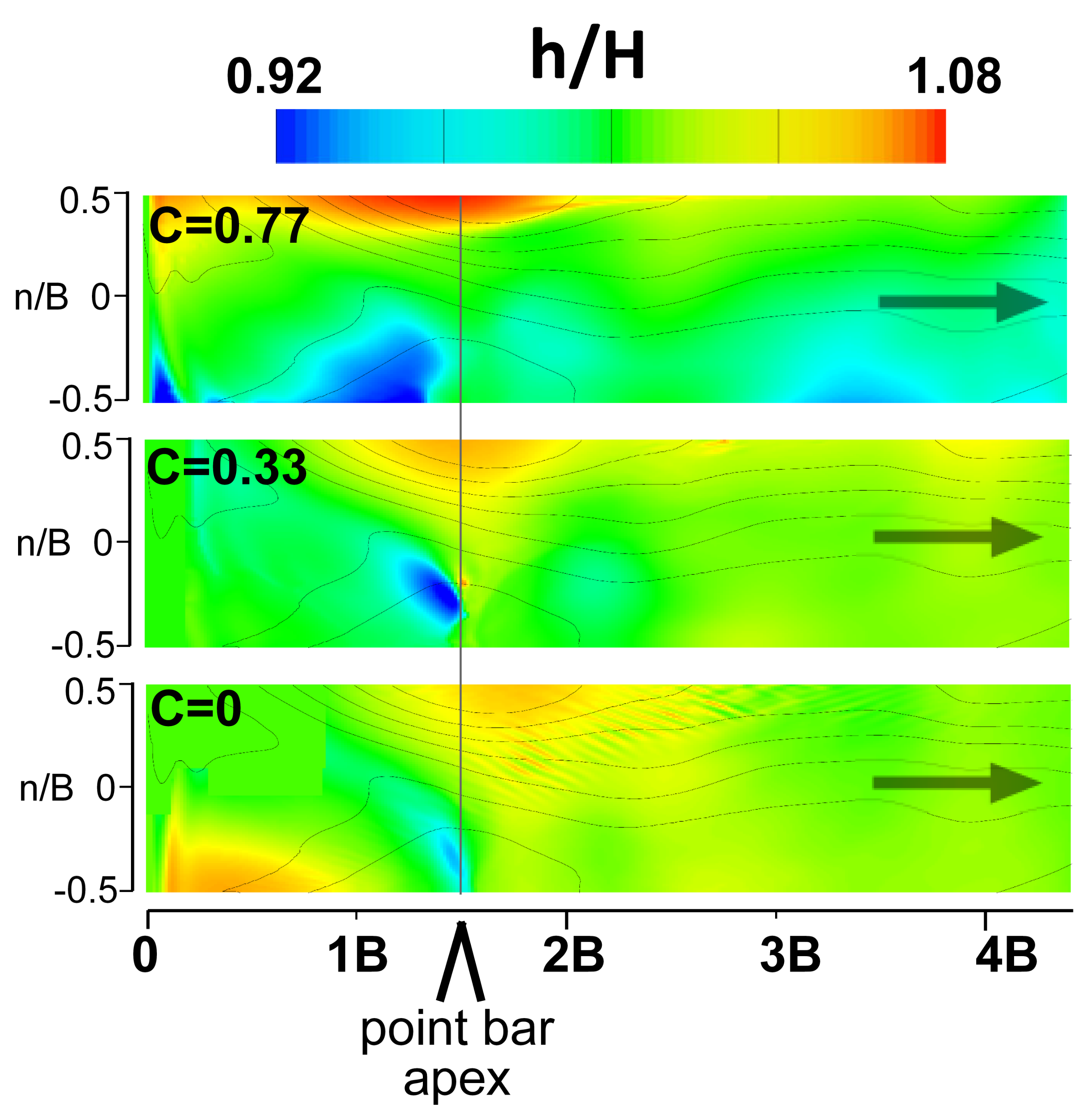
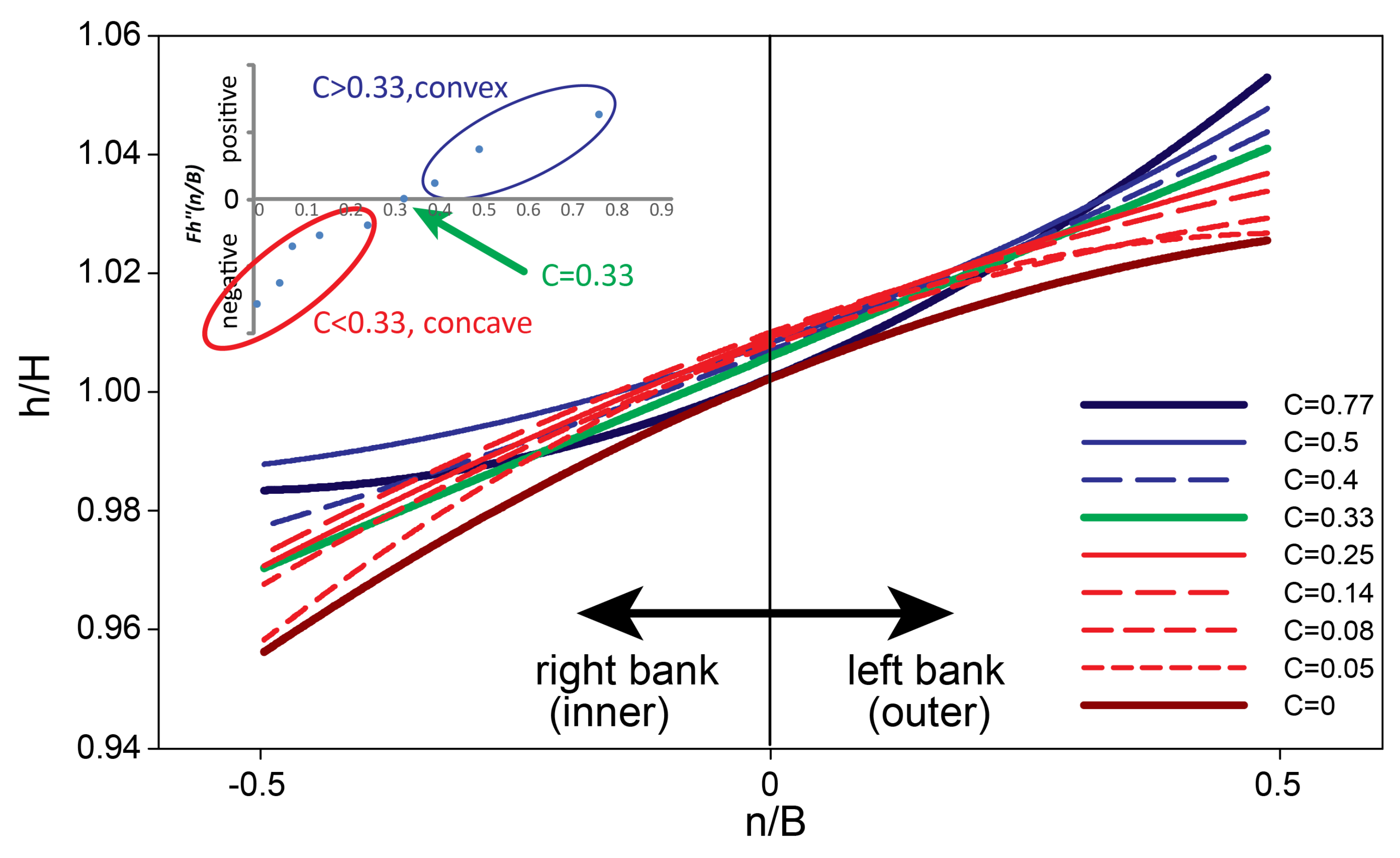
4.1. Flow Surface Elevation
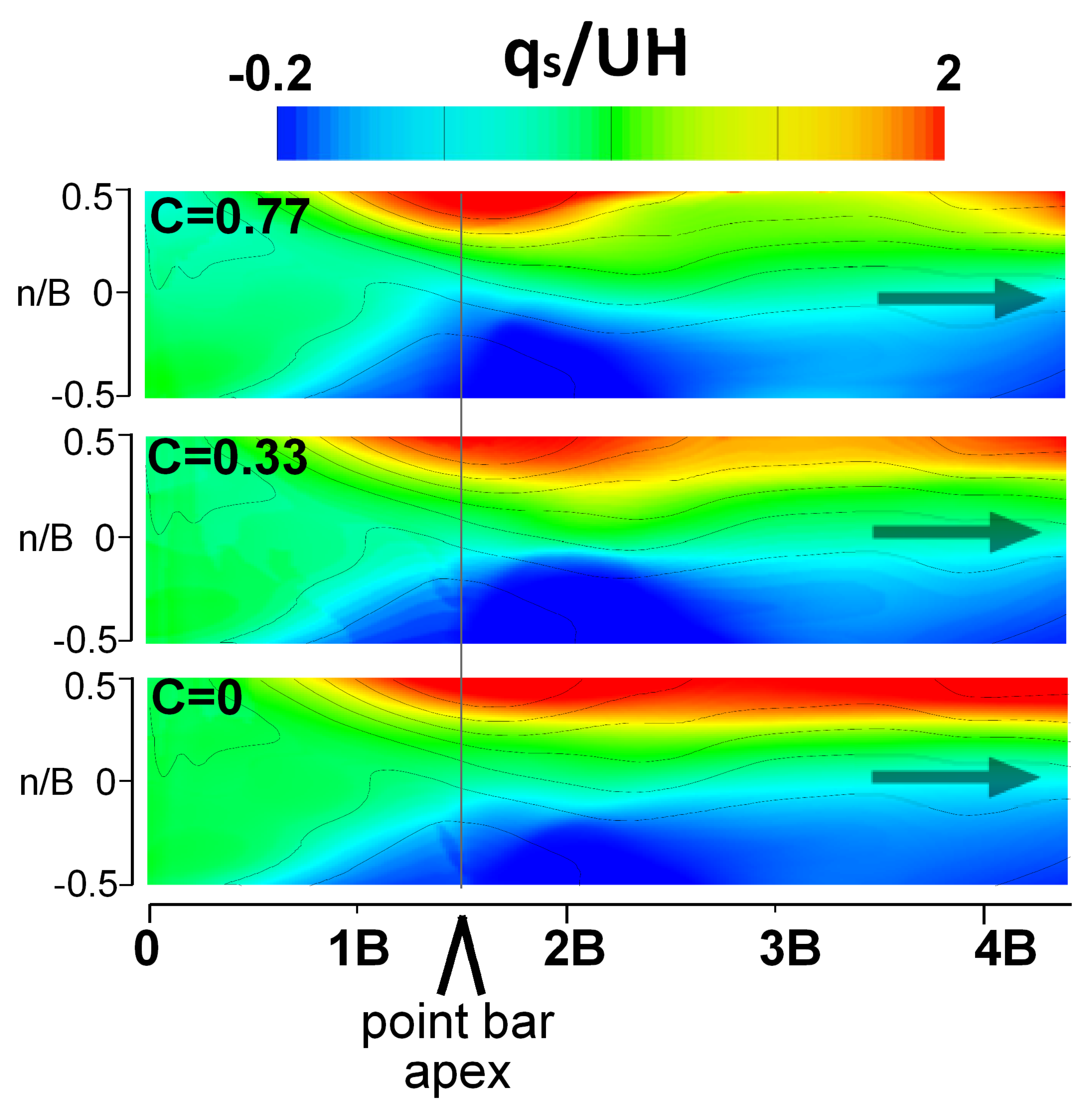
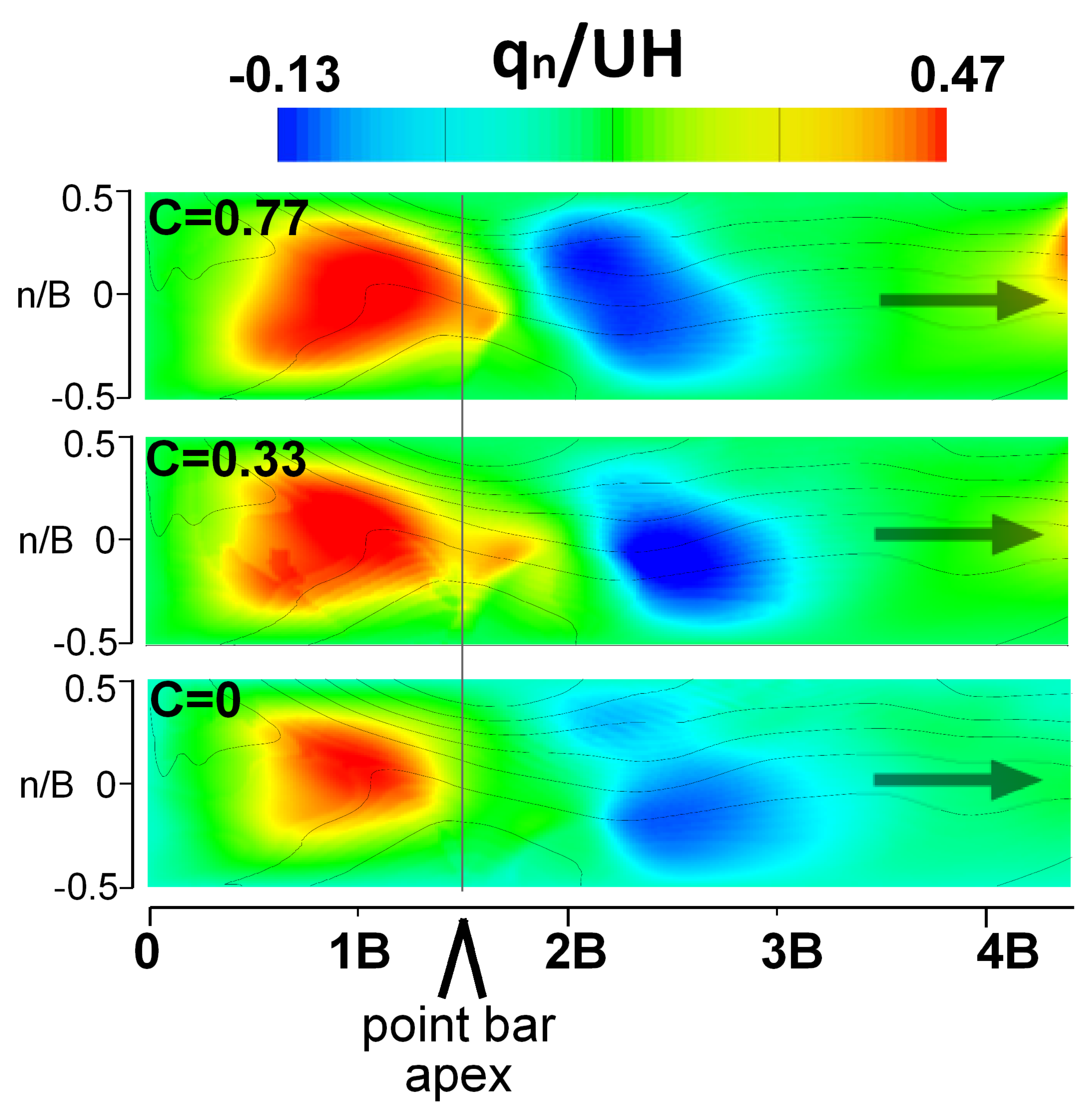
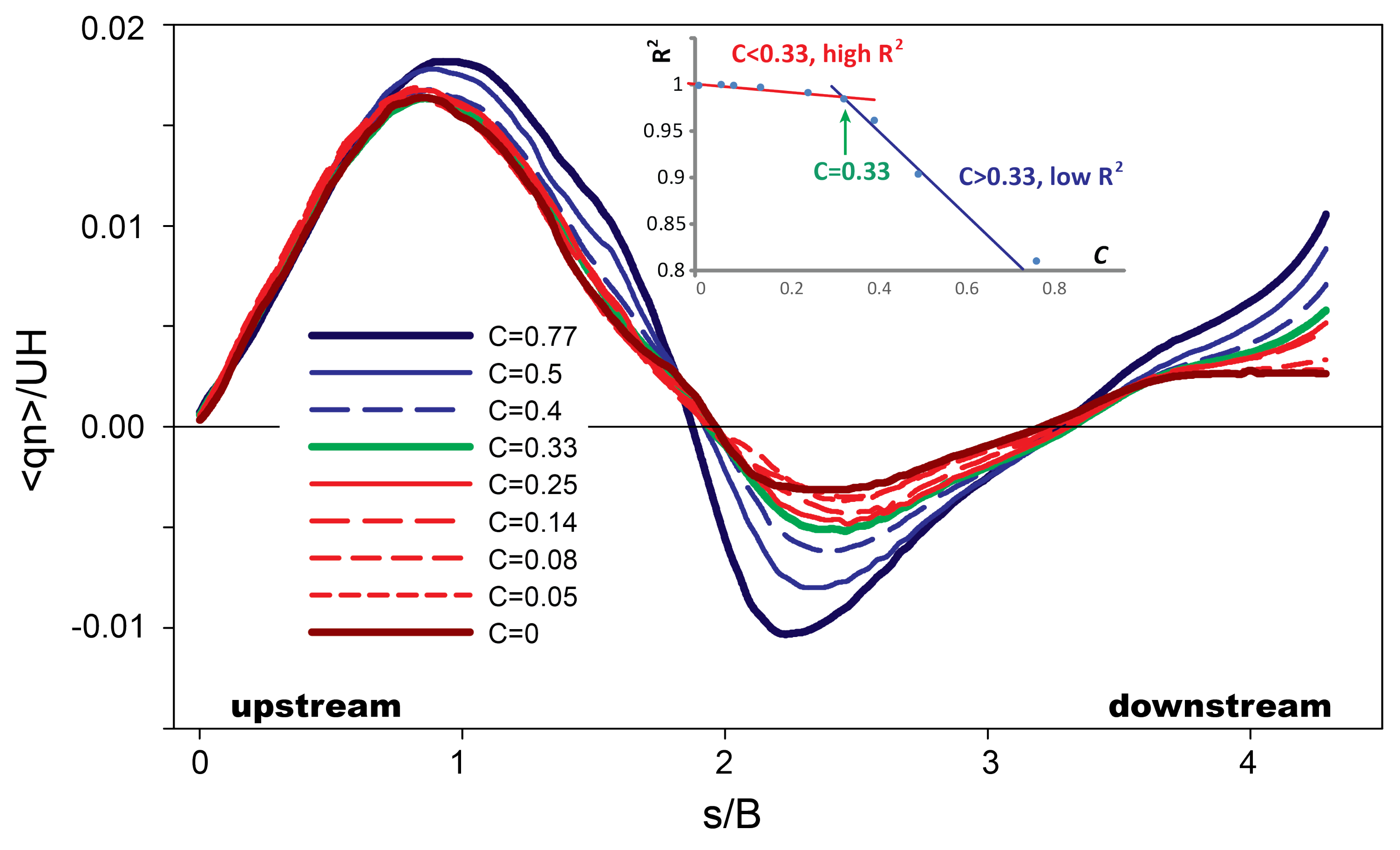
4.2. Streamwise and Transverse Unit Discharge
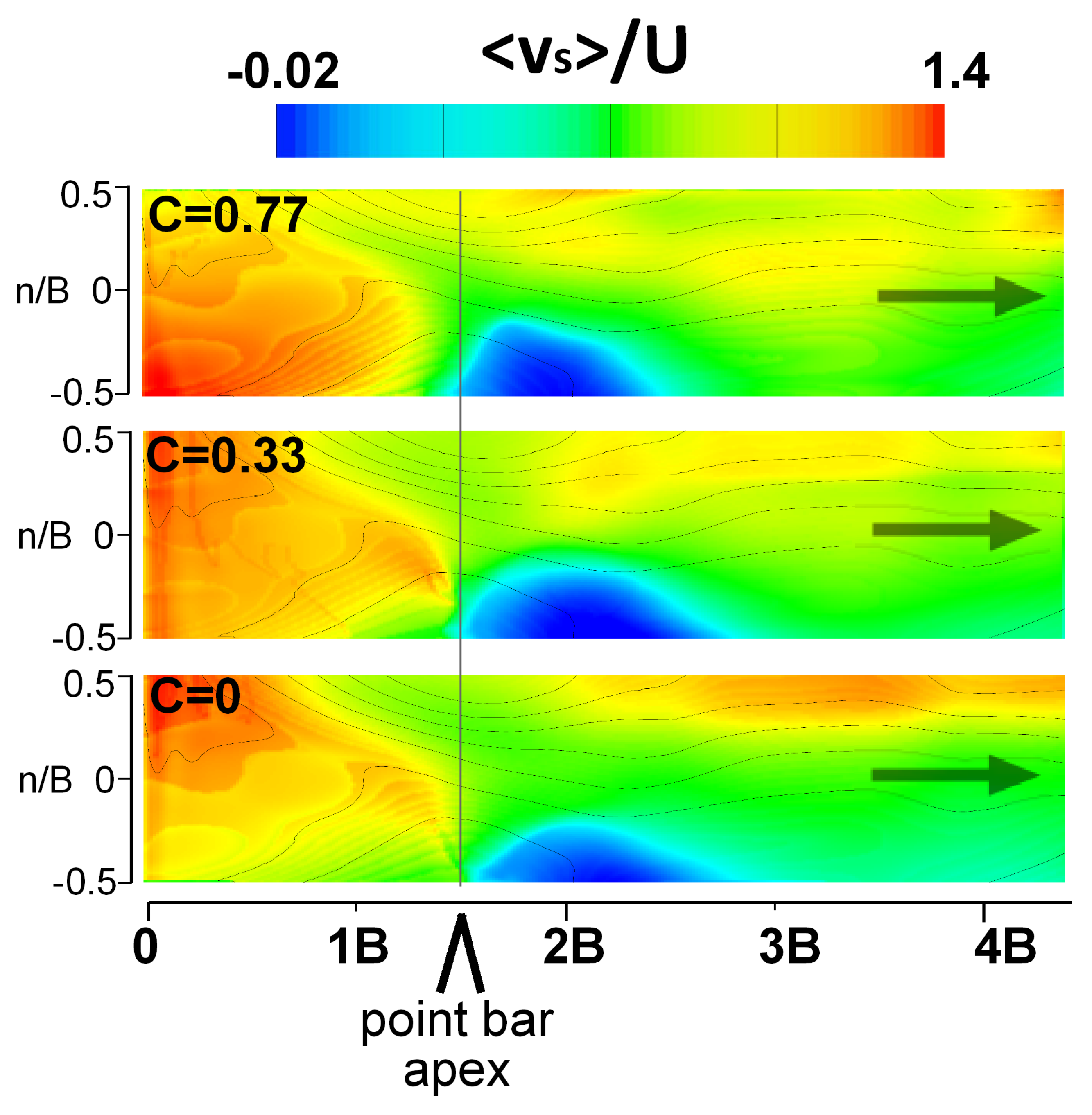
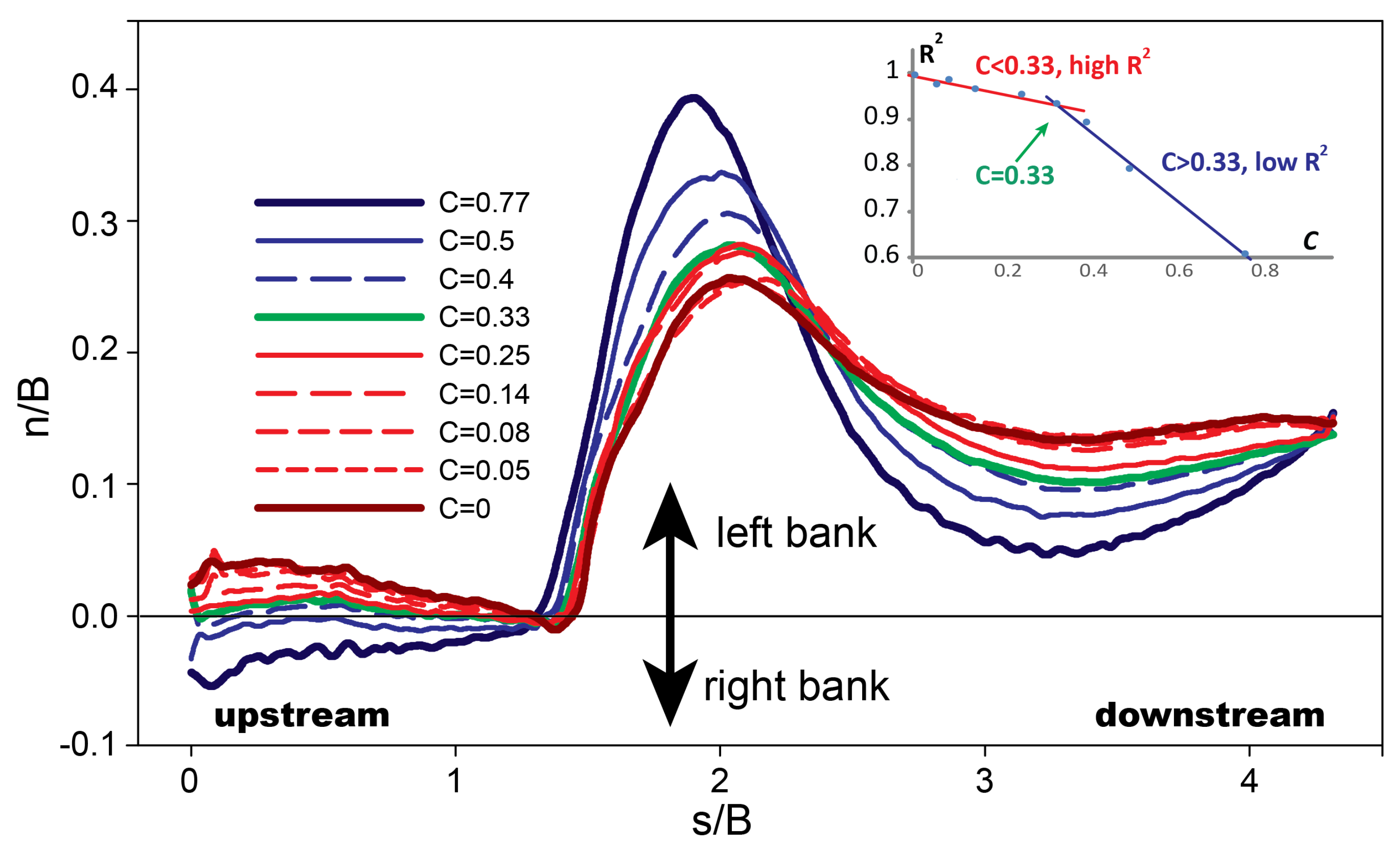
4.3. Streamwise Velocity Distribution
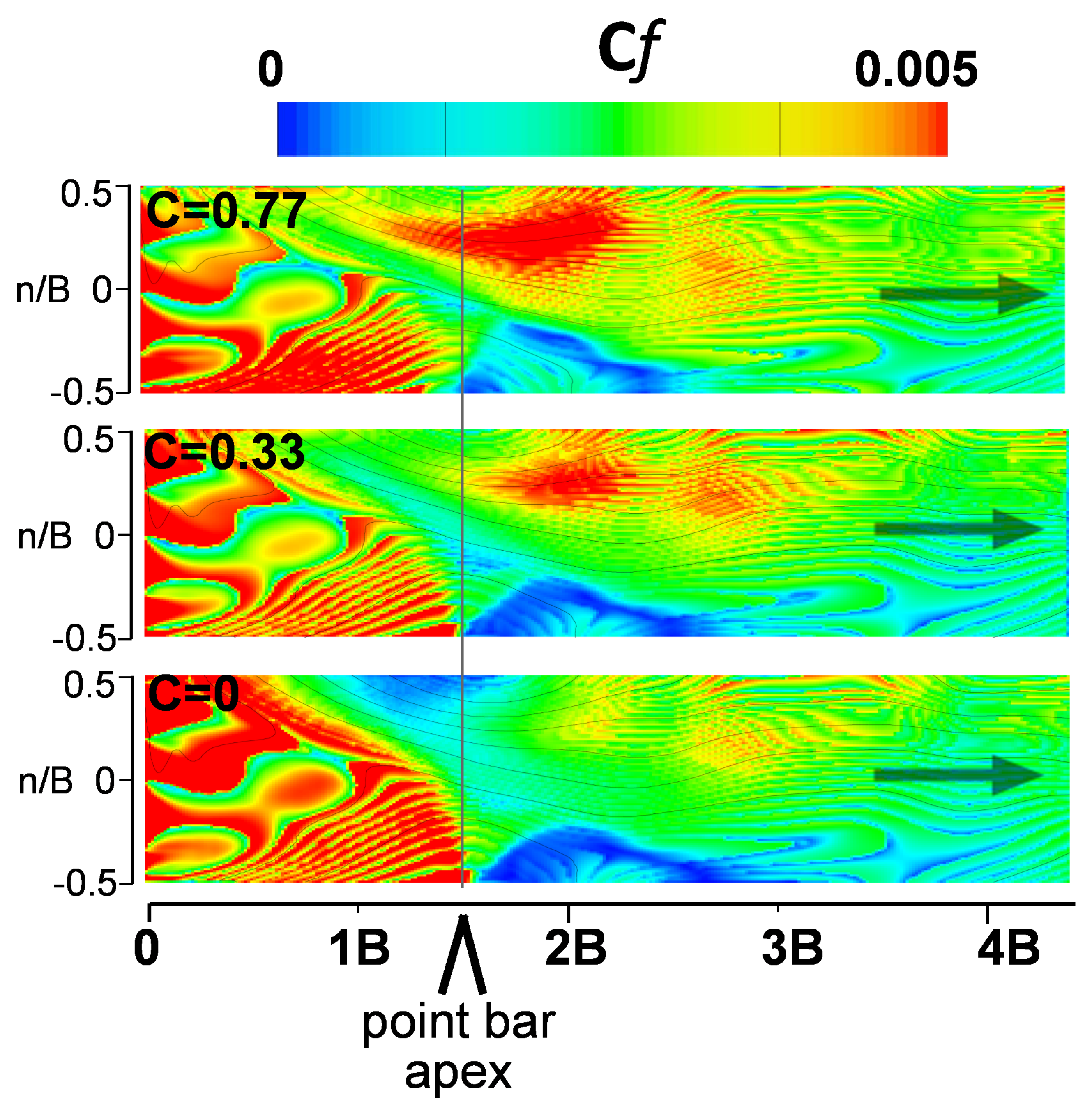
4.4. Bed Shear Distribution
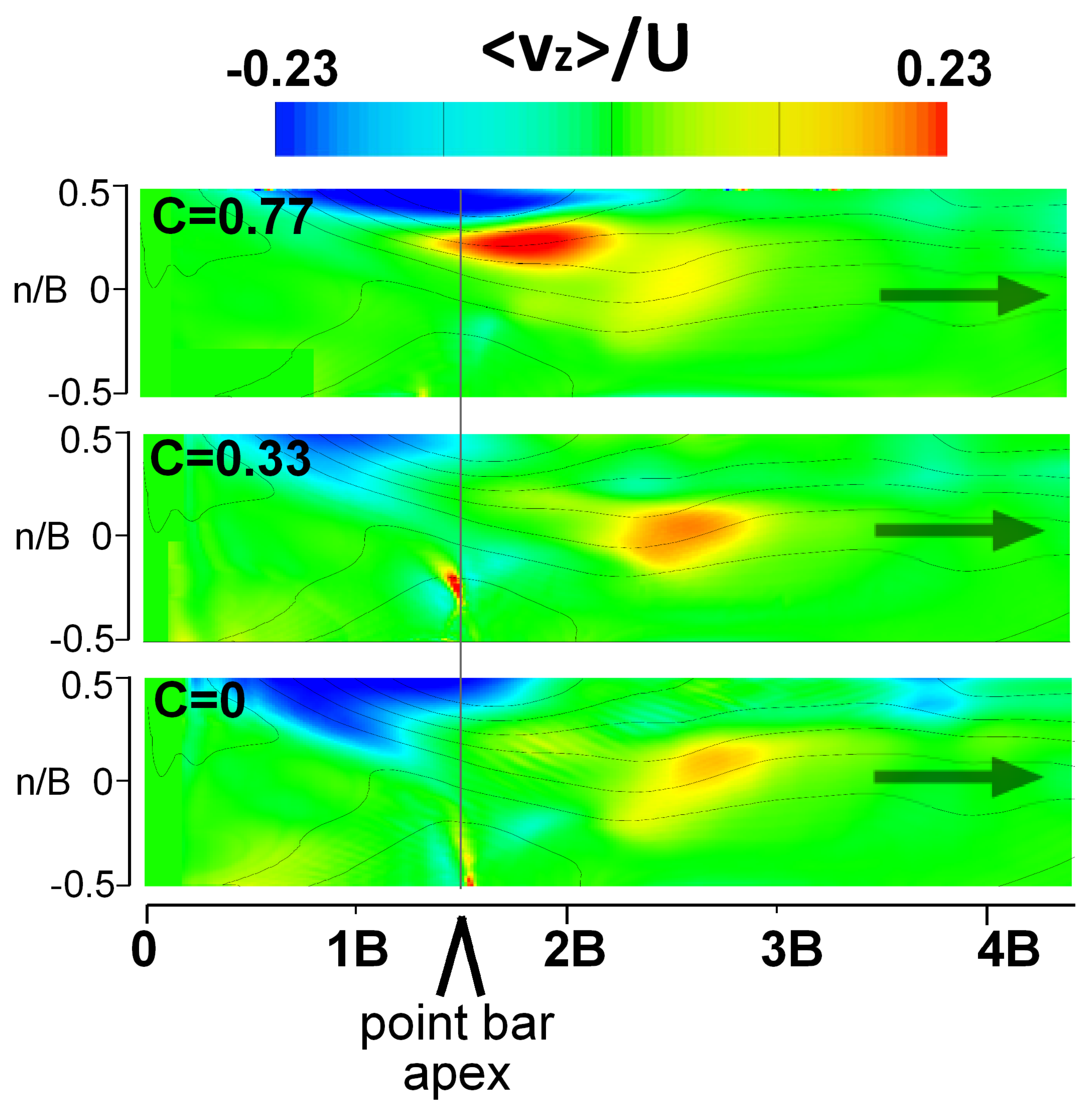
4.5. Vertical Hyporheic Exchange
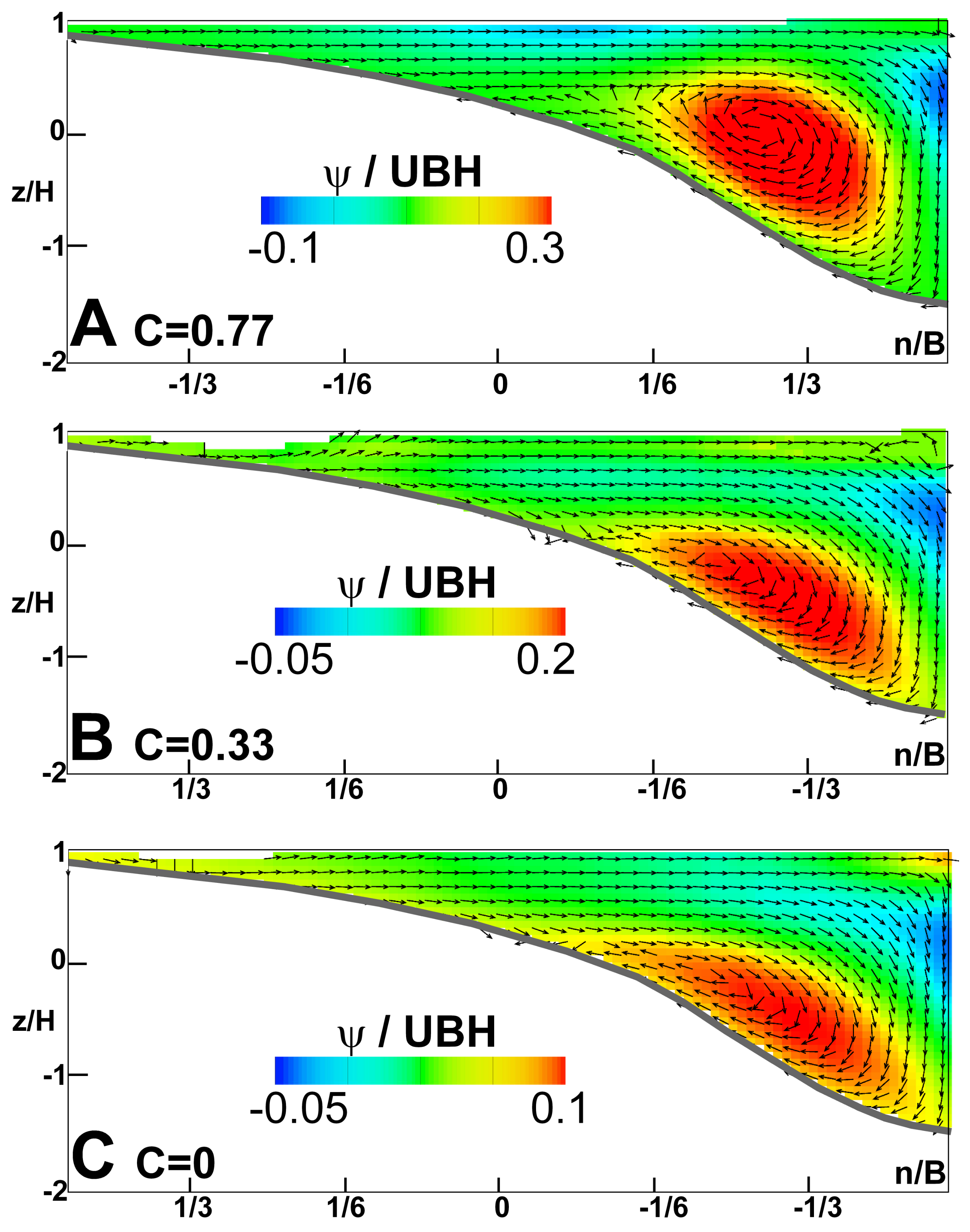
4.6. Secondary Circulation Patterns
5. Discussion and Conclusions
- The loss of large magnitude bed shear stresses in the pool at the meander apex and establishment of a larger zone of near-zero bed shear about the point bar (Figure 9), and the related loss of large swaths of upwelling flow of groundwater into the channel at the apex along the outer bank and downstream of the apex along the inner half of the channel (Figure 10).
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Langbein, W.; Leopold, L. River Meanders—Theory of Minimum Variance (Geological Survey Professional Paper 422-H); U.S. Government Printing Office: Washington, DC, USA, 1966.
- Leopold, L.B.; Wolman, M.G. River meanders. Bull. Geol. Soc. Am. 1960, 71, 769–793. [Google Scholar] [CrossRef]
- Wohl, E. Rivers in the Landscape; John Wiley & Sons: Hoboken, NJ, USA, 2020. [Google Scholar]
- Dietrich, W.E.; Smith, J.D. Influence of the point bar on flow through curved channels. Water Resour. Res. 1983, 19, 1173–1192. [Google Scholar] [CrossRef]
- Harvey, J.W.; Bencala, K. The effects of streambed topography on surface-subsurface water exchange in mountains catchments. Water Resour. Res. 1993, 29, 89–98. [Google Scholar] [CrossRef]
- Bridge, J.S. Rivers and Floodplains: Forms, Processes, and Sedimentary Record; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Schumm, S.A. Patterns of alluvial rivers. Annu. Rev. Earth Planet. Sci. 1985, 13, 5–27. [Google Scholar] [CrossRef]
- Vermeulen, B.; Hoitink, A.J.F.; Labeur, R.J. Flow structure caused by a local cross-sectional area increase and curvature in a sharp river bend. J. Geophys. Res. Earth Surf. 2015, 120, 1771–1783. [Google Scholar] [CrossRef]
- Konsoer, K.M.; Rhoads, B.L.; Best, J.L.; Langendoen, E.J.; Abad, J.D.; Parsons, D.R.; Garcia, M.H. Three-dimensional flow structure and bed morphology in large elongate meander loops with different outer bank roughness characteristics. Water Resour. Res. 2016, 52, 9621–9641. [Google Scholar] [CrossRef]
- Li, B.D.; Zhang, X.H.; Tang, H.S.; Tsubaki, R. Influence of deflection angles on flow behaviours in openchannel bends. J. Mt. Sci. 2018, 15, 2292–2306. [Google Scholar] [CrossRef]
- Gualtieri, C.; Abdi, R.; Ianniruberto, M.; Filizola, N.; Endreny, T.A. A 3D analysis of spatial habitat metrics about the confluence of Negro and Solimões rivers, Brazil. Ecohydrology 2020, 13, e2166. [Google Scholar] [CrossRef]
- Gualtieri, C.; Ianniruberto, M.; Filizola, N.; Santos, R.; Endreny, T. Hydraulic complexity at a large river confluence in the Amazon basin. Ecohydrology 2017, 10, e1863. [Google Scholar] [CrossRef]
- Kozarek, J.; Hession, W.; Dolloff, C.; Diplas, P. Hydraulic complexity metrics for evaluating in-stream brook trout habitat. J. Hydraul. Eng. 2010, 136, 1067–1076. [Google Scholar] [CrossRef]
- McCoy, E.D.; Bell, S.S.; Mushinsky, H.R. Habitat structure: Synthesis and perspectives. In Habitat Structure; Springer: Berlin, Germany, 1991; pp. 427–430. [Google Scholar]
- Re-Engineering Britain’s Rivers. The Economist. 6 March 2020. Available online: https://www.latestnigeriannews.com/news/8279579/reengineering-britains-rivers.html (accessed on 12 April 2020).
- Palmer, M.A.; Bernhardt, E.; Allan, J.; Lake, P.S.; Alexander, G.; Brooks, S.; Carr, J.; Clayton, S.; Dahm, C.; Follstad Shah, J.; et al. Standards for ecologically successful river restoration. J. Appl. Ecol. 2005, 42, 208–217. [Google Scholar] [CrossRef]
- Abad, J.D.; Rhoads, B.L.; Güneralp, İ.; García, M.H. Flow structure at different stages in a meander-bend with bendway weirs. J. Hydraul. Eng. 2008, 134, 1052–1063. [Google Scholar] [CrossRef]
- Blanckaert, K.; Schnauder, I.; Sukhodolov, A.; van Balen, W.; Uijttewaal, W. Meandering: Field Experiments, Laboratory Experiments and Numerical Modeling. Technical Report. 2009. Available online: https://infoscience.epfl.ch/record/146621/files/2009-695-Blanckaert_et_al-Meandering_field_experiments_laboratory_experiments_and_numerical.pdf (accessed on 12 April 2020).
- Constantinescu, G.; Koken, M.; Zeng, J. The structure of turbulent flow in an open channel bend of strong curvature with deformed bed: Insight provided by detached eddy simulation. Water Resour. Res. 2011, 47. [Google Scholar] [CrossRef]
- Sawyer, A.H.; Bayani Cardenas, M.; Buttles, J. Hyporheic exchange due to channel-spanning logs. Water Resour. Res. 2011, 47. [Google Scholar] [CrossRef]
- Zhou, T.; Endreny, T. Meander hydrodynamics initiated by river restoration deflectors. Hydrol. Process. 2012, 26, 3378–3392. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Van Balen, W.; Uijttewaal, W.; Blanckaert, K. Large-eddy simulation of a curved open-channel flow over topography. Phys. Fluids 2010, 22, 075108. [Google Scholar] [CrossRef]
- Blanckaert, K. Topographic steering, flow recirculation, velocity redistribution, and bed topography in sharp meander bends. Water Resour. Res. 2010, 46. [Google Scholar] [CrossRef]
- Zeng, J.; Constantinescu, G.; Blanckaert, K.; Weber, L. Flow and bathymetry in sharp open-channel bends: Experiments and predictions. Water Resour. Res. 2008, 44. [Google Scholar] [CrossRef]
- Elliott, A.H.; Brooks, N.H. Transfer of nonsorbing solutes to a streambed with bed forms: Laboratory experiments. Water Resour. Res. 1997, 33, 137–151. [Google Scholar] [CrossRef]
- Zhou, T.; Endreny, T.A. Reshaping of the hyporheic zone beneath river restoration structures: Flume and hydrodynamic experiments. Water Resour. Res. 2013, 49, 5009–5020. [Google Scholar] [CrossRef]
- Lane, S.; Bradbrook, K.; Richards, K.; Biron, P.; Roy, A. The application of computational fluid dynamics to natural river channels: Three-dimensional versus two-dimensional approaches. Geomorphology 1999, 29, 1–20. [Google Scholar] [CrossRef]
- Vardy, A. Fluid Principles; McGraw-Hill International Series in Civil Engineering; McGraw-Hill: London, UK, 1990. [Google Scholar]
- Rozovskii, I.L. Flow of Water in Bends of Open Channels; Academy of Sciences of the Ukrainian SSR: Kiev, Ukraine, 1957. [Google Scholar]
- Blanckaert, K.; De Vriend, H.J. Secondary flow in sharp open-channel bends. J. Fluid Mech. 2004, 498, 353–380. [Google Scholar] [CrossRef]
- Johannesson, H.; Parker, G. Linear theory of river meanders. River Meand. 1989, 12, 181–213. [Google Scholar] [CrossRef]
- Camporeale, C.; Perona, P.; Porporato, A.; Ridolfi, L. Hierarchy of models for meandering rivers and related morphodynamic processes. Rev. Geophys. 2007, 45. [Google Scholar] [CrossRef]
- He, L. Distribution of primary and secondary currents in sine-generated bends. Water SA 2018, 44, 118–129. [Google Scholar] [CrossRef]
- Liao, J.C.; Beal, D.N.; Lauder, G.V.; Triantafyllou, M.S. Fish exploiting vortices decrease muscle activity. Science 2003, 302, 1566–1569. [Google Scholar] [CrossRef]
- Crispell, J.K.; Endreny, T.A. Hyporheic exchange flow around constructed in-channel structures and implications for restoration design. Hydrol. Process. 2009, 1168, 1158–1168. [Google Scholar] [CrossRef]
- Hester, E.T.; Gooseff, M.N. Moving Beyond the Banks: Hyporheic Restoration Is Fundamental to Restoring Ecological Services and Functions of Streams. Environ. Sci. Technol. 2010, 44, 1521–1525. [Google Scholar] [CrossRef]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, T.; Endreny, T. The Straightening of a River Meander Leads to Extensive Losses in Flow Complexity and Ecosystem Services. Water 2020, 12, 1680. https://doi.org/10.3390/w12061680
Zhou T, Endreny T. The Straightening of a River Meander Leads to Extensive Losses in Flow Complexity and Ecosystem Services. Water. 2020; 12(6):1680. https://doi.org/10.3390/w12061680
Chicago/Turabian StyleZhou, Tian, and Theodore Endreny. 2020. "The Straightening of a River Meander Leads to Extensive Losses in Flow Complexity and Ecosystem Services" Water 12, no. 6: 1680. https://doi.org/10.3390/w12061680
APA StyleZhou, T., & Endreny, T. (2020). The Straightening of a River Meander Leads to Extensive Losses in Flow Complexity and Ecosystem Services. Water, 12(6), 1680. https://doi.org/10.3390/w12061680





