Characterisation of the Water Renewal in a Macro-Tidal Marina Using Several Transport Timescales
Abstract
1. Introduction
2. Materials and Methods
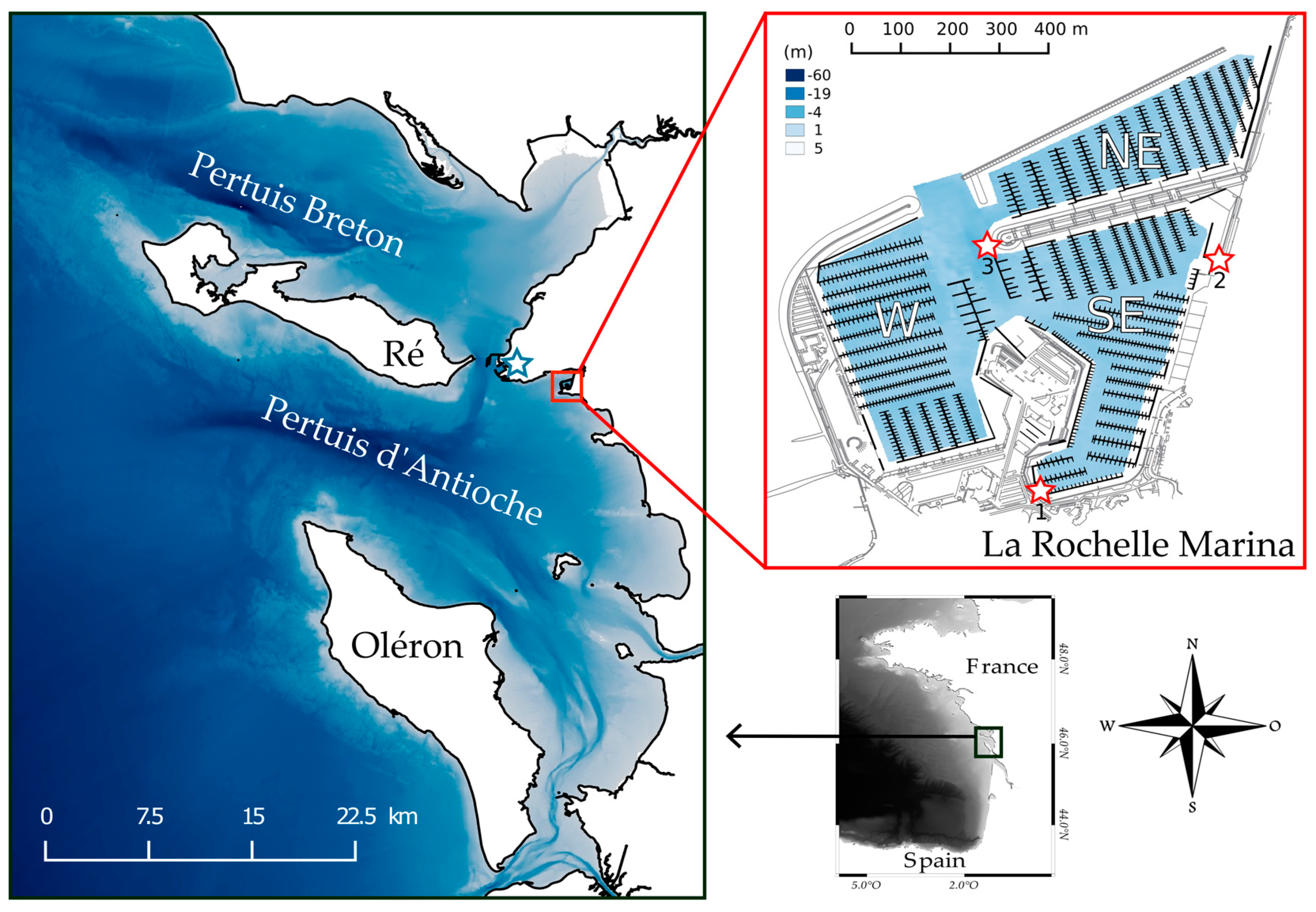
2.1. Study Site
2.1.1. La Rochelle Marina
2.1.2. Geomorphology and Hydrodynamics of the Coastal Area
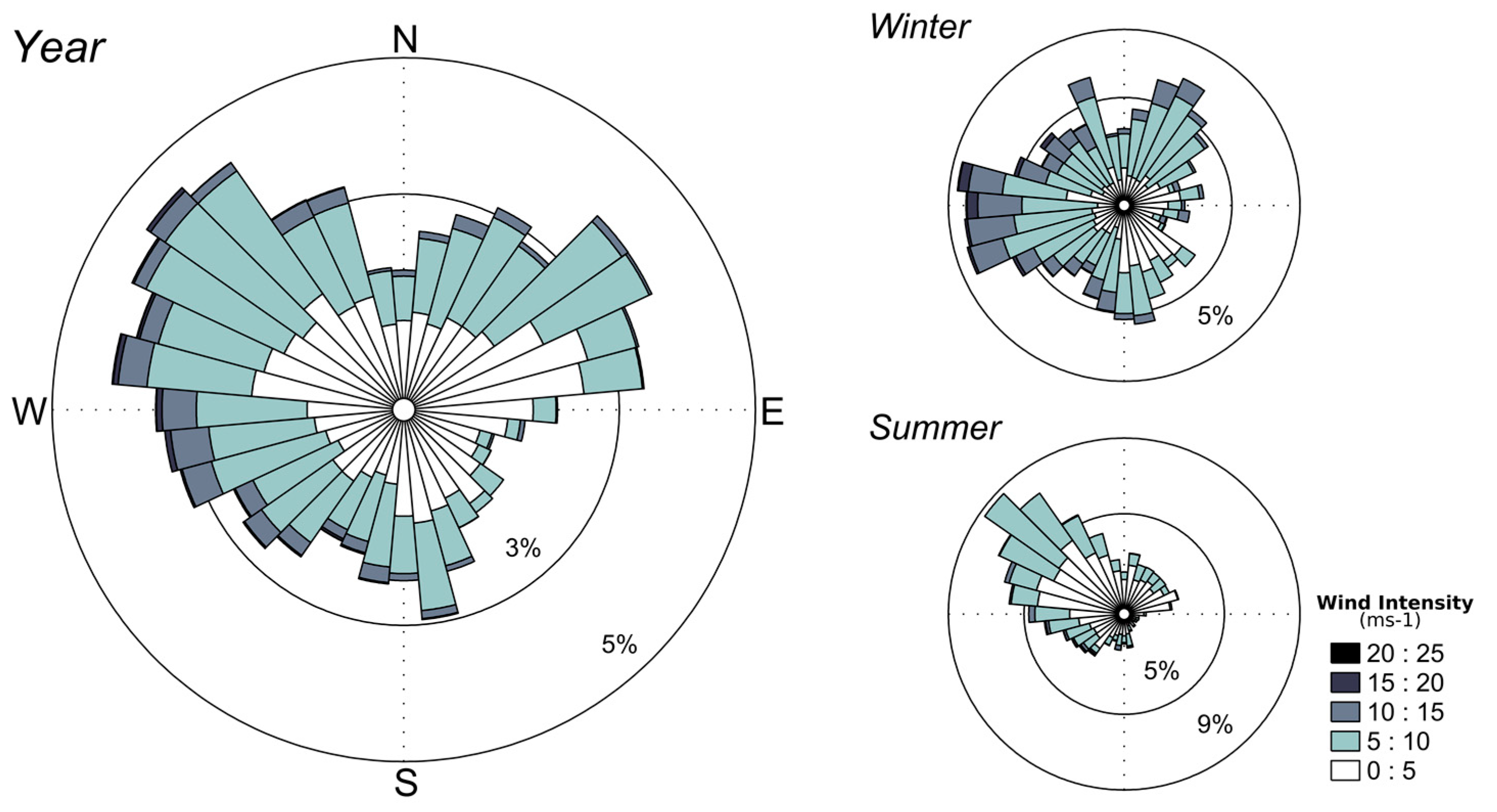
2.1.3. Meteorological Context
2.2. Numerical Model Implementation
2.3. Water Transport Timescales
2.3.1. Flushing Time
2.3.2. Residence and Exposure time
2.3.3. Estimation of the Return-Flow
2.4. General Simulation Set Up
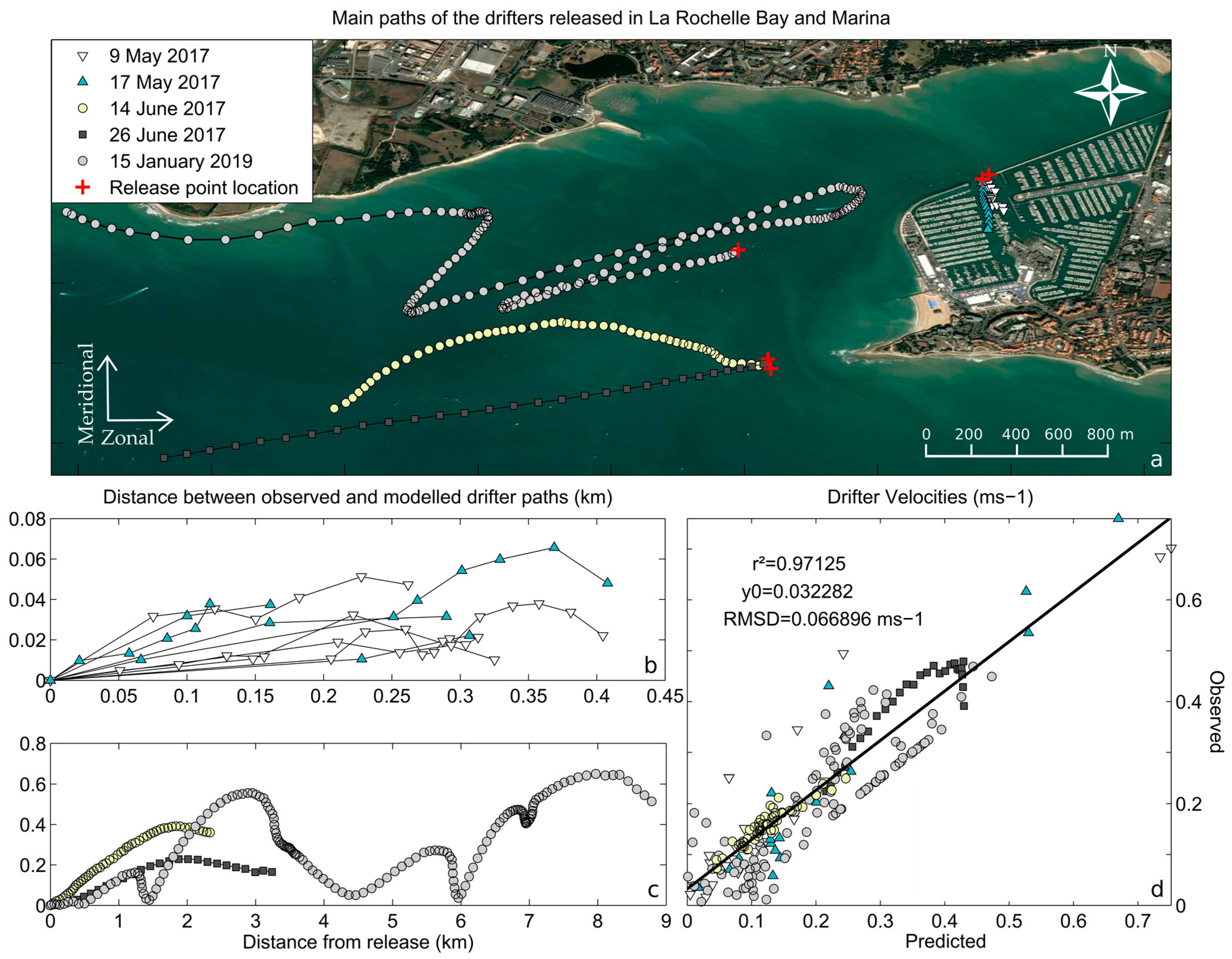
3. Lagrangian Validation
4. Results
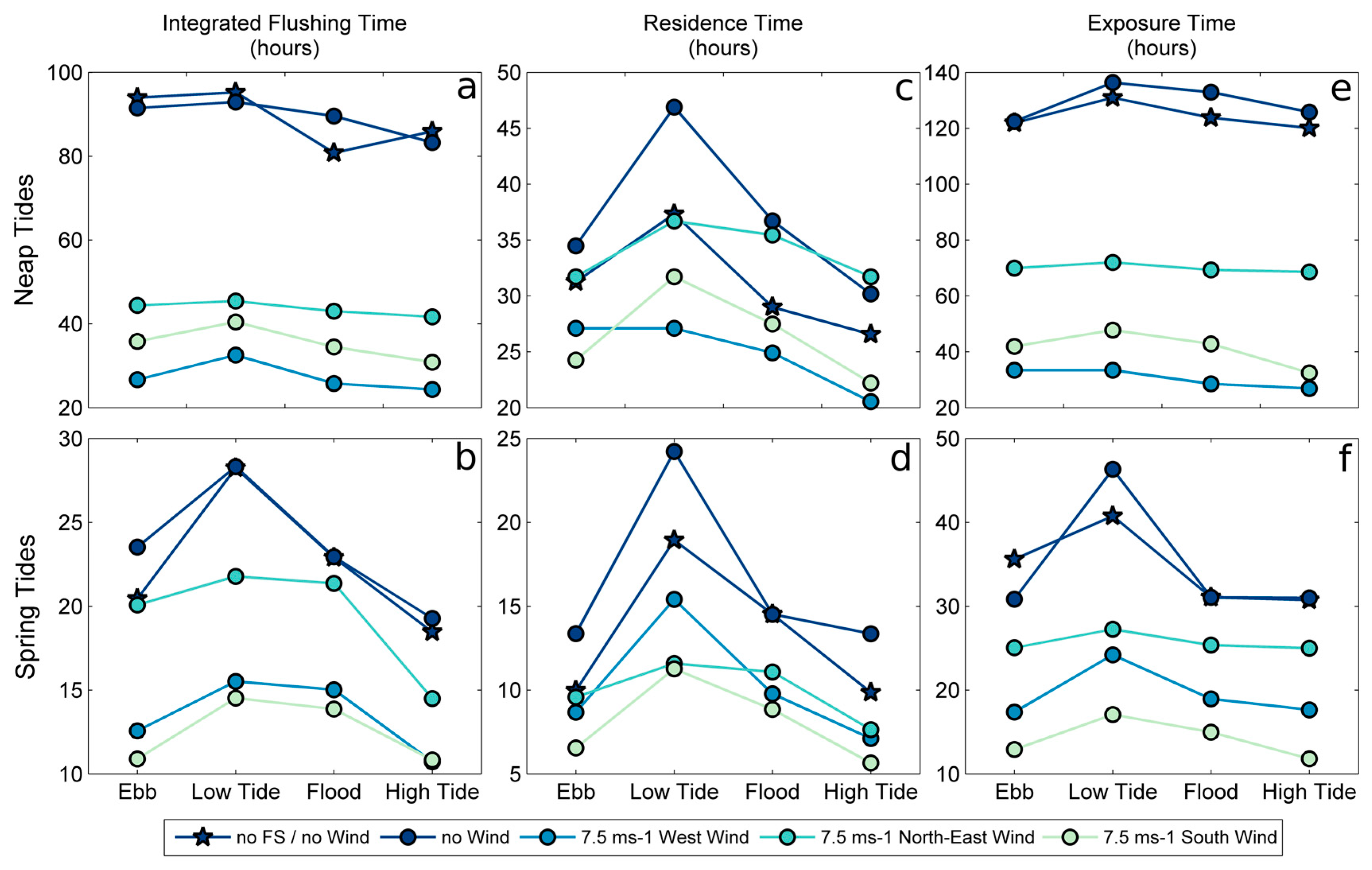
4.1. Integrated Flushing Time
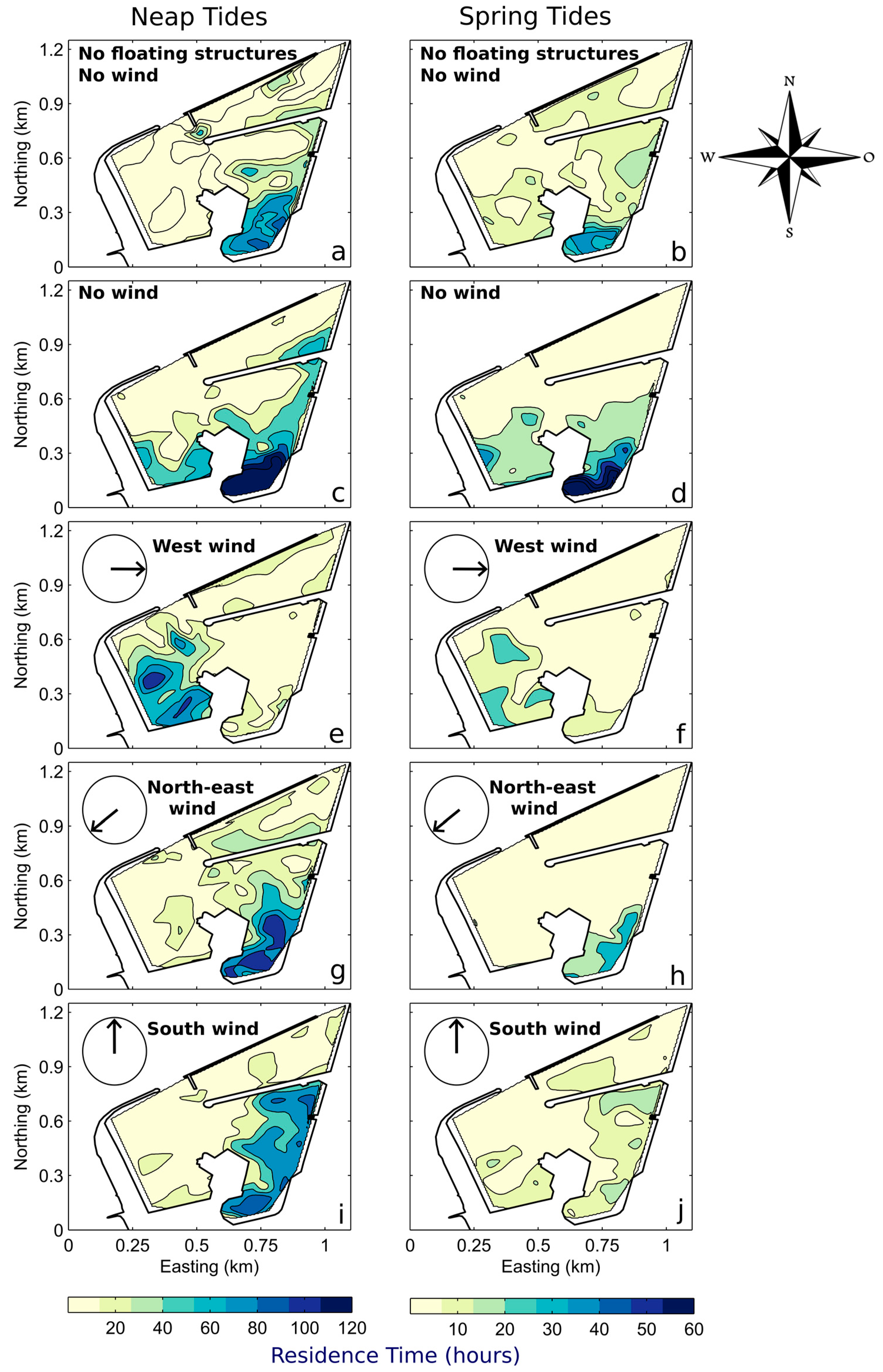
4.2. Residence Time
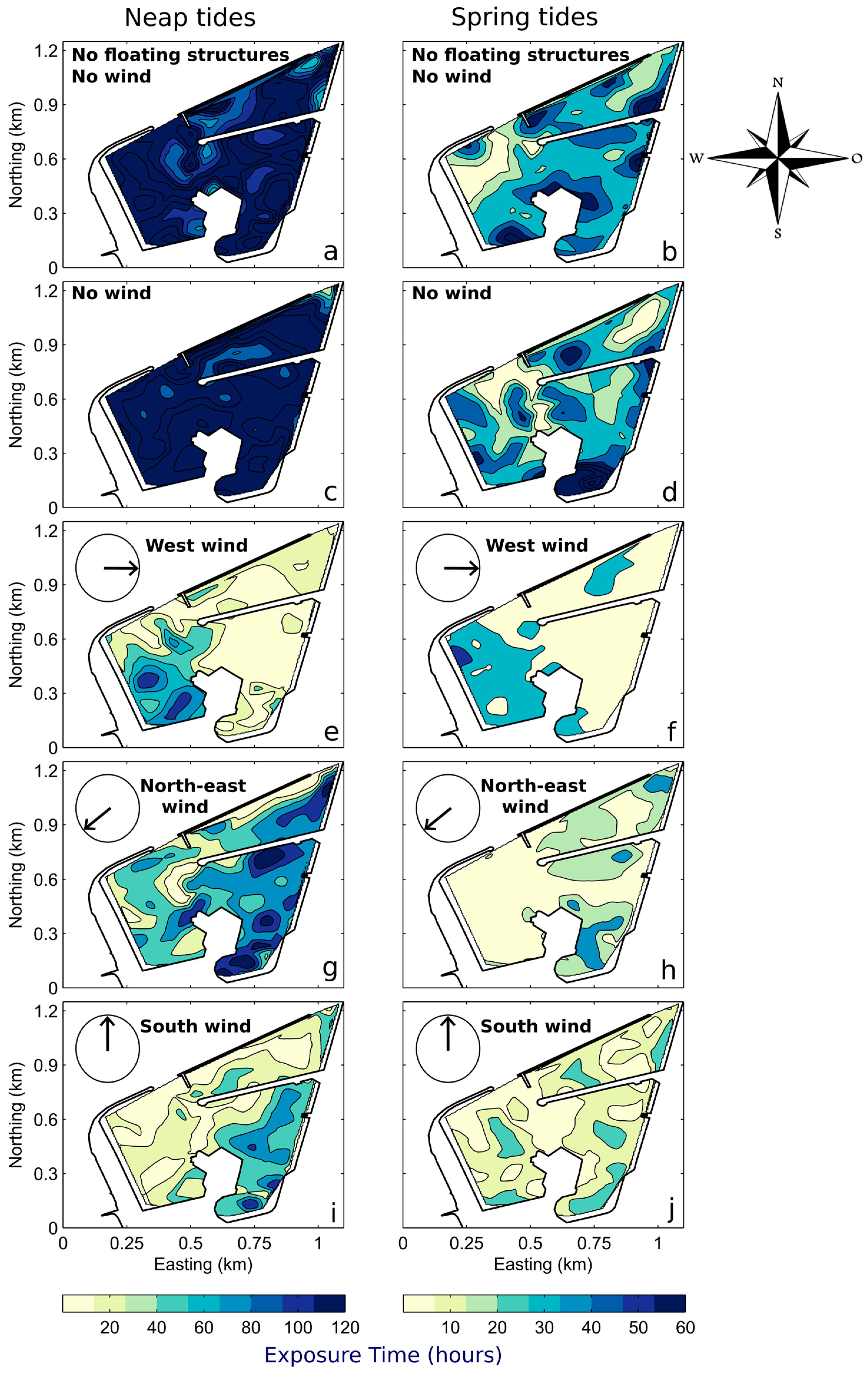
4.3. Exposure Time
4.4. Characterisation of the Return-Flow
5. Discussion
5.1. Assessment of the Main Drivers of the Water Renewal
5.1.1. Tidal Influence
5.1.2. Wind Influence
5.1.3. Influence of Floating Structures
5.1.4. Influence of the Tidal Phase
5.2. Comparison of Water Transport Timescales
5.3. Impact of the Return-Flow in the Water Renewal
6. Conclusions and Perspectives
- The marina displays a strong horizontal variability of the renewal. The most confined waters are located in the south of the SE basin, while the NE basin is generally the most renewed.
- Both the tide and wind substantially impact the water renewal of the marina. The transition from spring to neap tides significantly decreases the water renewal while the presence of the wind enhances it, in particular for west and south directions.
- In the marina, physical processes responsible for the water renewal are generally more affected by the tidal phase than in its surrounding bay.
- The influence of the wind is less significant in the marina than in the system bay-marina.
- The water renewal is relatively homogeneous over the water column even with the effect of the wind (data not shown).
- As shown by the study of RT, the Floating Structures (FS) particularly decreased the water renewal in the most sheltered parts (SE basin), but they also generally increase it in the most exposed parts of the marina (NE basin). The study of both ET and IFT showed that they do not alter the circulation significantly outside the marina and thus, slightly affect the return-flow in the marina.
- The return-flow has been consistently represented both in a Lagrangian and Eulerian way. Its information is a relevant indication of the circulation processes occurring outside the marina. Without wind, return-flow in the marina is amplified by neap tides that generate significant trapping of the water masses at the scale of the bay. Both ET and IFT results showed that return-flow was a key parameter in the dynamics of water renewal in the marina.
- The return-flow is very sensitive to the wind action and the trapping processes happening in the bay can be drastically reduced. Wind enhances the dispersion of water masses away from the bay and thus decreases the return-flow in the marina. Powerful west winds (15 ms−1), typical of winter conditions, can generate a near-zero return-flow.
- Without wind, return-flow is amplified by neap tides that generates significant trapping.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ondiviela, B.; Gómez, A.G.; Puente, A.; Juanes, J.J. A pragmatic approach to define the ecological potential of water bodies heavily modified by the presence of ports. Envion Sci. Policy 2013, 33, 320–331. [Google Scholar] [CrossRef]
- Gómez, A.G.; Ondiviela, B.; Fernández, M.; Juanes, J.J. Atlas of susceptibility to pollution in marinas. Application to the Spanish coast. Mar. Pollut. Bull. 2017, 114, 239–246. [Google Scholar] [CrossRef] [PubMed]
- Mestres, M.; Sierra, J.P.; Mösso, C.; Sánchez-Arcilla, A. Sources of contamination and modelled pollutant trajectories in a Mediterranean harbour (Tarragona, Spain). Mar. Pollut. Bull. 2010, 60, 898–907. [Google Scholar] [CrossRef] [PubMed]
- Grifoll, M.; Jordà, G.; Borja, Á.; Espino, M. A new risk assessment method for water quality degradation in harbour domains, using hydrodynamic models. Mar. Pollut. Bull. 2010, 60, 69–78. [Google Scholar] [CrossRef] [PubMed]
- Fatoki, O.S.; Mathabatha, S. An assessment of heavy metal pollution in the East London and Port Elizabeth harbours. Water SA 2001, 27, 233–240. [Google Scholar] [CrossRef]
- Bonamano, S.; Madonia, A.; Piazzolla, D.; Paladini de Mendoza, F.; Piermattei, V.; Scanu, S.; Marcelli, M. Development of a Predictive Tool to Support Environmentally Sustainable Management in Port Basins. Water 2017, 9, 898. [Google Scholar] [CrossRef]
- Mali, M.; De Serio, F.; Dell’Anna, M.M.; Mastrorilli, P.; Damiani, L.; Mossa, M. Enhancing the performance of hazard indexes in assessing hot spots of harbour areas by considering hydrodynamic processes. Ecol. Indic. 2017, 73, 38–45. [Google Scholar] [CrossRef]
- Bolin, B.; Rodhe, H. A note on the concepts of age distribution and transit time in natural reservoirs. Tellus 1973, 25, 58–62. [Google Scholar] [CrossRef]
- Takeoka, H. Fundamental concepts of exchange and transport time scales in a coastal sea. Cont. Shelf Res. 1984, 3, 311–326. [Google Scholar] [CrossRef]
- Zimmerman, J.T.F. Estuarine Residence Times. In Hydrodynamics of Estuaries, 1st ed.; Kjerfve, B., Ed.; CRC Press: Boca Raton, FL, USA, 1988; p. 171. [Google Scholar]
- Monsen, N.E.; Cloern, J.E.; Lucas, L.V.; Monismith, S.G. A comment on the use of flushing time, residence time, and age as transport time scales. Limnol. Oceanogr. 2002, 47, 1545–1553. [Google Scholar] [CrossRef]
- Delhez, E.J.M.; Heeming, A.W.; Deleersnijder, E. Residence time in a semi-enclosed domain from the solution of an adjoint problem. Estuar. Coast. Shelf Sci. 2004, 61, 691–702. [Google Scholar] [CrossRef]
- Deleersnijder, E.; Delhez, E. Timescale and tracer-based methods for understanding the results of complex marine models. Estuar. Coast. Shelf Sci. 2007, 74, 5–7. [Google Scholar] [CrossRef]
- Oliveira, A.; Baptista, A.M. Diagnostic modeling of residence times in estuaries. Water Resour. Res. 1997, 3, 1935–1946. [Google Scholar] [CrossRef]
- Abdelrhman, M.A. Simplified modeling of flushing and residence times in 42 embayments in New England, USA, with special attention to Greenwich Bay, Rhode Island. Estuar. Coast. Shelf Sci. 2005, 62, 339–351. [Google Scholar] [CrossRef]
- Cucco, A.; Umgiesser, G. Modeling the Venice Lagoon residence time. Ecol. Model. 2006, 193, 34–51. [Google Scholar] [CrossRef]
- Sánchez-Arcilla, A.; Espino, M.; Grifoll, M.; Mösso, C.; Sierra, J.P.; Mestres, M.; Spyroupoulu, S.; Hernáez, M.; Ojanguren, A.; Sotillo, M.G.; et al. Quay design and operational oceanography. The case of bilbao harbour. Coast. Eng. Proc. 2011, 1, 51. [Google Scholar] [CrossRef]
- Breitwieser, M.; Dubillot, E.; Barbarin, M.; Churlaud, C.; Huet, V.; Muttin, F.; Thomas, H. Assessment of the biological quality of port areas: A case study on the three harbours of La Rochelle: The marina, the fishing harbour and the seaport. PLoS ONE 2018, 13, e0198255. [Google Scholar] [CrossRef] [PubMed]
- Huguet, J.R.; Brenon, I.; Coulombier, T. Influence of floating structures on the tide and wind-driven hydrodynamics of a highly populated marina. J. Waterw. Port Coast. Ocean Eng. 2019. In press. [Google Scholar]
- Sanford, L.P.; Boicourt, W.C.; Rives, S.R. Model for Estimating Tidal Flushing of Small Embayments. J. Waterw. Port Coast. Ocean Eng. 1992, 118, 635–654. [Google Scholar] [CrossRef]
- Chaumillon, E.; Weber, N. Spatial variability of modern incised valleys on the French Atlantic coast: Comparison between the Charente (Pertuis d’Antioche) and the Lay-Sèvre (Pertuis Breton) incised valleys. In Incised Valleys in Time and Space, 1st ed.; Dalrymple, R.A., Leckie, D.A., Tillman, R.A., Eds.; SEPM Special Publications: Tarsa, OK, USA, 2006; pp. 57–85. [Google Scholar]
- Bertin, X.; Bruneau, N.; Breilh, J.F.; Fortunato, A.B.; Karpytchev, M. Importance of wave age and resonance in storm surges; the case Xynthia, Bay of Biscay. Ocean Model. 2012, 42, 16–30. [Google Scholar] [CrossRef]
- Le Cann, B. Barotropic tidal dynamics of the Bay of Biscay shelf: Observations, numerical modelling and physical interpretation. Cont. Shelf Res. 1990, 10, 723–758. [Google Scholar] [CrossRef]
- Bertin, X. Morphodynamique Séculaire, Architecture Interne et Modélisation d’un Système Baie/Embouchure tidale: Le Pertuis de Maumusson et la Baie de Marennes-Oléron. Ph.D. Thesis, La Rochelle University, La Rochelle, France, 2005. [Google Scholar]
- Dodet, G.; Bertin, X.; Tabord, R. Wave climate variability in the North-East Atlantic Ocean over the last six decades. Ocean Model. 2010, 31, 120–131. [Google Scholar] [CrossRef]
- Hervouet, J.M. Hydrodynamics of Free Surface Flows: Modelling with the Finite Element Method; John Wiley and Sons: Hobken, NJ, USA, 2007; p. 360. [Google Scholar]
- Viero, P.D.; Defina, A. Renewal time scales in tidal basins: Climbing the Tower of Babel. In Sustainable Hydraulics in the Era of Global Chang, 1st ed.; Taylor & Francis Group: London, UK, 2016; p. 216. [Google Scholar]
- Donea, J.; Giuliani, S.; Halleux, J.P. An arbitrary Lagrangian-Eulerian finite element method for transient dynamic fluid-structure interactions. Comput. Methods Appl. Mech. Eng. 1982, 33, 689–723. [Google Scholar] [CrossRef]
- SHOM. Information Géographique Maritime et Littorale de Référence. Available online: https://data.shom.fr/ (accessed on 2 July 2019).
- AVISO+ Satellite Altimetry Data. Available online: https://www.aviso.altimetry.fr/en/data/products/auxiliary-products/global-tide-fes.html (accessed on 2 July 2019).
- Choi, K.W.; Lee, J.H.W. Numerical Determination of Flushing Time for Stratified Water Bodies. J. Mar. Syst. 2004, 50, 263–281. [Google Scholar] [CrossRef]
- Grifoll, M.; Del Campo, A.; Espino, M.; Mader, J.; González, M.; Borja, Á. Water renewal and risk assessment of water pollution in semi-enclosed domains: Application to Bilbao Harbour (Bay of Biscay). J. Mar. Syst. 2013, 109–110, 241–251. [Google Scholar] [CrossRef]
- Plus, M.; Dumas, F.; Stanisière, J.Y.; Maurer, D. Hydrodynamic characterization of the Archachon Bay, using model-derived descriptors. Cont. Shelf Res. 2009, 29, 1008–1013. [Google Scholar] [CrossRef]
- Wang, C.F.; Hsu, M.H.; Kuo, A.Y. Residence time of the Danshuei River estuary, Taiwan. Estuar. Coast. Shelf Sci. 2004, 60, 381–393. [Google Scholar] [CrossRef]
- Andrejev, O.; Myrberg, K.; Lundberg, P.A. Age and renewal time of water masses in a semi-enclosed basin—application to the Gulf of Finland. Tellus A Dyn. Meteorol. Oceanogr. 2004, 56, 548–558. [Google Scholar]
- Fugate, D.C. Estimation of Residence Time in a Shallow Back Barrier Lagoon, Hog Island Bay, Virginia, USA. In Proceedings of the Ninth International Conference on Estuarine and Coastal Modelling, Charleston, SC, USA, 31 October–2 November 2005. [Google Scholar]
- Thomann, R.V.; Mueller, J.A. Principles of Surface Water Quality Modeling and Control; Harper & Row, Publishers: New York, NY, USA, 1987; p. 656. [Google Scholar]
- Zimmerman, J.T.F. Mixing and flushing of tidal embayments in the Western Dutch wadden Sea, Part I: Distribution of salinity and calculation of mixing time scales. Neth. J. Sea Res. 1976, 10, 149–191. [Google Scholar] [CrossRef]
- Dronkers, J.; Zimmerman, J.T.F. Some principles of mixing in tidal lagoons. Oceanol. Acta 1982, 4, 107–118. [Google Scholar]
- Cucco, A.; Umgiesser, G.; Ferrarin, C.; Perilli, A.; Canu, D.M.; Solidoro, C. Eulerian and lagrangian transport timescales of a tidal active coastal basin. Ecol. Model. 2009, 220, 913–922. [Google Scholar] [CrossRef]
- Spivakovskaya, D.; Heemink, A.W.; Deleersnijder, E. Lagrangian modelling of multi-dimensional advection-diffusion with space-varying diffusivities: Theory and idealized test cases. Ocean Dyn. 2007, 57, 189–203. [Google Scholar] [CrossRef][Green Version]
- Delhez, E.J.M.; Brye, B.; Brauwere, A.; Deleersnijder, E. Residence time vs influence time. J. Mar. Syst. 2014, 132, 185–195. [Google Scholar] [CrossRef]
- Wolanski, E. Estuarine Ecohydrology, 1st ed.; Elsevier Science: Atlanta, GA, USA, 2007; p. 168. [Google Scholar]
- Brauwere, A.; Brye, B.; Blaise, S.; Deleersnijder, E. Residence time, exposure time and connectivity in the Scheldt Estuary. J. Mar. Syst. 2011, 84, 85–95. [Google Scholar] [CrossRef]
- Delhez, E. On the concept of exposure time. Cont. Shelf Res. 2013, 71, 27–36. [Google Scholar] [CrossRef]
- Andutta, F.P.; Helfer, F.; Miranda, L.B.; Deleersnjider, E.; Thomas, C.; Lemckert, C. An assessment of transport timescales and return coefficient in adjacent tropical estuaries. Cont. Shelf Res. 2013, 124, 49–62. [Google Scholar] [CrossRef][Green Version]
- Barcena, J.F.; Garcià, A.; Gómez, A.G.; Àlvarez, C.; Juanes, J.A.; Revilla, A. Spatial and temporal flushing time approach in estuaries influenced by river and tide. An application in Suances Estuary (Northern Spain). Estuar. Coast. Shelf Sci. 2012, 112, 40–51. [Google Scholar] [CrossRef]
- Wijeratne, S.; Rydberg, L. Modelling and observations of tidal wave propagation circulation and residence times in Puttalam Lagoon, Sri Lanka. Estuar. Coast. Shelf Sci. 2007, 74, 697–708. [Google Scholar] [CrossRef]
- Ferrarin, C.; Zaggia, L.; Paschini, E.; Scirocco, T.; Lorenzetti, G.; Bajo, M.; Penna, P.; Francavilla, M.; D’Adamo, R.; Guerzoni, S. Hydrological Regime and Renewal Capacity of the Micro-tidal Lesina Lagoon, Italy. Estuar. Coasts 2013, 37, 79–93. [Google Scholar] [CrossRef]
- Yuan, D.; Lin, B.; Falconer, R.A. A modelling study of residence time in a macro-tidal estuary. Estuar. Coast. Shelf Sci. 2007, 71, 401–411. [Google Scholar] [CrossRef]
- Cavalcante, G.H.; Kjverfve, B.; Feary, D.A. Examination of residence time and its relevance to water quality whithin a coastal mega-structure: The Palm Jumeirah Lagoon. J. Hydrol. 2012, 468–469, 111–119. [Google Scholar] [CrossRef]
- Lisi, I.; Taramelli, A.; Di Risio, M.; Cappucci, S.; Gabellini, M. Flushing efficiency of Augusta Harbour (Italy). J. Coast. Res. 2009, 56, 841–845. [Google Scholar]
- Schwartz, R.A.; Imberger, J. Flushing behaviour of a coastal marina. Coast. Eng. Proc. 1988, 2626–2640. [Google Scholar] [CrossRef]
- Grifoll, M.; Espino, M.; Gonzàlez, M.; Ferrer, L.; Sánchez-Arcilla, A. Spatial residence time description for water discharges in harbours. In Proceedings of the 4th Internation Conference on Marine Waste Water Disposal and Marine Environment, Antalya, Turkey, 6–10 November 2006. [Google Scholar]
- Orfila, A.; Jordi, A.; Basterretxea, G.; Vizoso, G.; Marbà, N.; Duarte, C.M.; Werner, F.J.; Tintoré, J. Residence time and Posidonia oceanica in Cabrera Archipelago National park, Spain. Cont. Shelf Res. 2005, 25, 1339–1352. [Google Scholar] [CrossRef][Green Version]
- Geyer, W.R. Influence of Wind on Dynamics and Flushing of Shallow Estuaries. Estuar. Coast. Shelf Sci. 1997, 44, 713–722. [Google Scholar] [CrossRef]
- Canu, D.M.; Solidoro, C.; Umgiesser, G.; Cucco, A.; Ferrarin, C. Assessing confinement in coastal lagoons. Mar. Pollut. Bull. 2012, 64, 2391–2398. [Google Scholar] [CrossRef]
- Du, J.; Shen, J. Water residence time in Chesapeake Bay for 1982–2012. J. Mar. Syst. 2016, 164, 101–111. [Google Scholar] [CrossRef]
- Umgiesser, G.; Zemlys, P.; Erturk, A.; Razinkova-Baziukas, A.; Mežine, J.; Ferrarin, C. Seasonal renewal time variability in the Curonian Lagoon caused by atmospheric and hydrographical forcing. Ocean Sci. 2016, 12, 391–402. [Google Scholar] [CrossRef]
- Warner, J.C.; Geyer, R.W.; Arango, H.G. Using a composite grid approach in a complex coastal domain to estimate estuarine residence time. Comput. Geosci. 2010, 36, 921–935. [Google Scholar] [CrossRef]
- Babu, M.T.; Vethamony, P.; Desa, E. Modelling tide-driven currents and residual eddies in the Gulf of Kachchh and their seasonal variability: A marine environmental planning perspective. Ecol. Model. 2005, 184, 299–312. [Google Scholar] [CrossRef]
- Ridderkinhof, H.; Zimmerman, J.T.F.; Philippart, M.E. Tidal exchange between the north sea and Dutch Wadden Sea and mixing timescales of the tidal basins. Neth. J. Sea Res. 1990, 25, 331–350. [Google Scholar] [CrossRef]
- Aref, H. Stirring by chaotic advection. J. Fluid Mech. 1984, 143, 1–21. [Google Scholar] [CrossRef]
- Arega, F.; Badr, A.W. Numerical age and residence-time mapping for a small tidal creek: Case study. J. Waterw. Port Coast. Ocean Eng. 2010, 136, 226–237. [Google Scholar] [CrossRef]
- Arega, F. Hydrodynamic modeling and characterizing of Lagrangian flows in the West Scott Creek wetlands system, South Carolina. J. Hydro Environ. Res. 2013, 7, 50–60. [Google Scholar] [CrossRef]
- Dyer, K.R. Estuaries: A Physical Introduction; John Wiley and Sons: New York, NY, USA, 1973. [Google Scholar]
- Callaway, R.J. Flushing study of South Beach Marina, Oregon. J. Waterw. Port Coast. Ocean Eng. 1981, 102, 47–58. [Google Scholar]
- Arega, F.; Armstrong, S.; Badr, A.W. Modeling of residence time in the East Scott Creek Estuary, South Carolina, USA. J. Hydro Environ. Res. 2008, 2, 99–108. [Google Scholar] [CrossRef]
- Zhang, C. Water renewal timescales in an ecological reconstructed lagoon in China. J. Hydroinform. 2013, 15, 991–1001. [Google Scholar]
- Gillibrand, P.A. Calculating Exchange Times in a Scottish Fjord Using a Two-dimensional, Laterally-integrated Numerical model. Estuar. Coast. Shelf Sci. 2001, 53, 437–449. [Google Scholar] [CrossRef]
- Ascione Kenov, I.; Muttin, F.; Campbell, R.; Fernandes, R.; Campuzano, F.; Machado, F.; Franz, G.; Neves, R. Water fluxes and renewal rates at Pertuis d’Antioche/Marennes-Oléron Bay, France. Estuar. Coast. Shelf Sci. 2015, 167, 32–44. [Google Scholar] [CrossRef]
- Bialik, R.J.; Karpiński, M. On the effect of the window size on the assessment of particle diffusion. J. Hydraul. Res. 2018, 56, 560–566. [Google Scholar] [CrossRef]
- Huang, W.; Spaulding, M. Modelling residence-time response to freshwater input in Apalachicola Bay, Florida, USA. Hydrol. Process. 2002, 16, 3051–3064. [Google Scholar] [CrossRef]
- Asselin, S.; Spaulding, M.L. Flushing Times for Providence River Based on Tracer Experiments. Estuaries 1993, 16, 830–839. [Google Scholar] [CrossRef]
- Malhadas, M.S.; Neves, R.J.; Leitão, P.C.; Silva, A. Influence of tide and waves on water renewal in Obidos Lagoon, Portugal. Ocean Dyn. 2010, 60, 41–55. [Google Scholar] [CrossRef]
- Azevedo, A.; Oliveira, A.; Fortunato, A.B.; Bertin, X. Application of an Eulerian-Lagrangian oil spill modeling system to the Prestige accident: Trajectory analysis. J. Coast. Res. 2009, 56, 777–781. [Google Scholar]
- Delpey, M.T.; Ardhuin, F.; Otherguy, P.; Jouon, A. Effects of waves on coastal water dispersion in a small estuarine bay. J. Geophys. Res. 2014, 119, 70–86. [Google Scholar] [CrossRef]
- Guo, W.; Wu, G.; Liang, B.; Xu, T.; Chen, X.; Yang, Z.; Xie, M.; Jiang, M. The influence of surface wave on water exchange in the Bohai Sea. Cont. Shelf Res. 2016, 118, 128–142. [Google Scholar] [CrossRef]
- Malhadas, M.S.; Silva, A.; Leitão, P.C.; Neves, R. Effect of the Bathymetric Changes on the Hydrodynamic and Residence Time in Obidos Lagoon (Portugal). J. Coast. Res. 2009, 56, 549–553. [Google Scholar]

| Conditions | Scenarios | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| a | c | e | g | i | k | b | d | f | h | j | l | ||
| Tides | Neap | ● | ● | ● | ● | ● | ● | ||||||
| Spring | ● | ● | ● | ● | ● | ● | |||||||
| Wind | West 7.5 ms−1 | ● | ● | ||||||||||
| North-East 7.5 ms−1 | ● | ● | |||||||||||
| South 7.5 ms−1 | ● | ● | |||||||||||
| West 15 ms−1 | ● | ● | |||||||||||
| With floating structures (FS) | ● | ● | ● | ● | ● | ● | ● | ● | ● | ● | |||
| Deployment Date | Number of Drifters per Release | Number of Releases | Location | Duration | Weather-Marine Conditions |
|---|---|---|---|---|---|
| 9 May 2017 | 3 | 5 | 46°08′54.1″ N 1°10′08.0″ W | Over one flood tide | Spring tides with strong south winds |
| 17 May 2017 | 3 | 5 | 46°08′54.1″ N 1°10′08.0″ W | Over one flood tide | Neap tides with calm weather |
| 14 June 2017 | 5 | 1 | 46°08′16.2″ N 1°10′46.4″ W | One ebb tide | Neap tides with calm weather |
| 26 June 2017 | 5 | 1 | 46°08′15.8″ N 1°10′46.6″ W | One ebb tide | Spring tides with calm weather |
| 15 January 2019 | 3 | 1 | 46°08′39.2″ N 1°10′52.7″ W | 24 h | Neap tides with mixed winds |
| Deployment Date | RMSD (ms−1) | R2 | ||
|---|---|---|---|---|
| U | V | U | V | |
| 9 May 2017 | 0.19 | 0.15 | 0.69 | 0.75 |
| 17 May 2017 | 0.17 | 0.14 | 0.71 | 0.82 |
| 14 June 2017 | 0.01 | 0.04 | 0.91 | 0.80 |
| 26 June 2017 | 0.04 | 0.06 | 0.88 | 0.69 |
| 15 January 2019 | 0.11 | 0.17 | 0.76 | 0.72 |
| Parameters | Weather–Marine Scenarios | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Neap Tides | Spring Tides | ||||||||||||
| a | c | e | g | i | k | b | d | f | h | j | l | ||
| IFT | Mean | 88.9 | 89.3 | 27.3 | 43.6 | 35.4 | 25.6 | 22.5 | 23.6 | 13.5 | 19.4 | 12.5 | 10.1 |
| SSD | 22.7 | 30.2 | 12.1 | 19.1 | 13.5 | 13 | 6.2 | 11.1 | 3.7 | 4.9 | 3.1 | 5.6 | |
| TPSD | 6.8 | 4.3 | 3.6 | 1.6 | 3.9 | 3.3 | 4.2 | 3.7 | 2.8 | 2.6 | 2 | 2.9 | |
| RT | Mean | 31.1 | 37 | 24.9 | 33.9 | 26.4 | 23.9 | 13.3 | 15.4 | 9.3 | 10.3 | 8.1 | 9.6 |
| SSD | 28.3 | 42.9 | 26.8 | 31.2 | 26.7 | 27.9 | 12.7 | 20.6 | 7.5 | 10.6 | 5.1 | 15.4 | |
| TPSD | 4.6 | 7.1 | 3.1 | 2.5 | 4.1 | 3.8 | 4.3 | 5.3 | 3.6 | 1.6 | 2.5 | 2.8 | |
| ET | Mean | 124.1 | 129.3 | 35.5 | 69.9 | 41.3 | 27.5 | 34.5 | 34.8 | 19.3 | 19.5 | 14.2 | 11.8 |
| SSD | 24.1 | 25.7 | 27.2 | 36.2 | 27.7 | 27.6 | 17.4 | 21.2 | 14.9 | 13.1 | 9.1 | 16.7 | |
| TPSD | 5 | 6.4 | 3.4 | 1.3 | 6.4 | 6 | 6.1 | 8 | 3.9 | 0.3 | 2.3 | 5.1 | |
| RFF | Mean | 0.72 | 0.73 | 0.12 | 0.45 | 0.32 | 0.06 | 0.64 | 0.66 | 0.41 | 0.59 | 0.36 | 0.19 |
| SSD | 0.02 | 0.01 | 0.11 | 0.02 | 0.08 | 0.10 | 0.07 | 0.05 | 0.11 | 0.10 | 0.10 | 0.11 | |
| TPSD | 0.05 | 0.03 | 0.03 | 0.01 | 0.01 | 0.01 | 0.04 | 0.03 | 0.01 | 0.01 | 0.02 | 0.02 | |
| RC | Mean | 0.75 | 0.73 | 0.19 | 0.51 | 0.36 | 0.09 | 0.61 | 0.56 | 0.47 | 0.50 | 0.43 | 0.08 |
| SSD | 0.03 | 0.04 | 0.05 | 0.04 | 0.05 | 0.11 | 0.09 | 0.06 | 0.13 | 0.06 | 0.08 | 0.1 | |
| TPSD | 0.06 | 0.07 | 0.04 | 0.03 | 0.01 | 0.01 | 0.06 | 0.05 | 0.02 | 0.01 | 0.03 | 0.03 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huguet, J.-R.; Brenon, I.; Coulombier, T. Characterisation of the Water Renewal in a Macro-Tidal Marina Using Several Transport Timescales. Water 2019, 11, 2050. https://doi.org/10.3390/w11102050
Huguet J-R, Brenon I, Coulombier T. Characterisation of the Water Renewal in a Macro-Tidal Marina Using Several Transport Timescales. Water. 2019; 11(10):2050. https://doi.org/10.3390/w11102050
Chicago/Turabian StyleHuguet, Jean-Rémy, Isabelle Brenon, and Thibault Coulombier. 2019. "Characterisation of the Water Renewal in a Macro-Tidal Marina Using Several Transport Timescales" Water 11, no. 10: 2050. https://doi.org/10.3390/w11102050
APA StyleHuguet, J.-R., Brenon, I., & Coulombier, T. (2019). Characterisation of the Water Renewal in a Macro-Tidal Marina Using Several Transport Timescales. Water, 11(10), 2050. https://doi.org/10.3390/w11102050





