Spatio–Temporal Evolution of Electric Field, Magnetic Field and Thermal Infrared Remote Sensing Associated with the 2021 Mw7.3 Maduo Earthquake in China
Abstract
1. Introduction
2. Data Selection and Processing
2.1. Thermal Infrared Remote Sensing Data
- (1)
- Our main concerns revolved around the reconstructed signal and the error of the original signal. We used the db8 wavelet basis in the Daubechies (dbN) wavelet system to wavelet transform the data. To achieve this, we experimented with various wavelet bases to decompose and reconstruct the signal. The characteristic of the dbN wavelet system is that it can divide the frequency band better with the increase in the order. However, as the calculation greatly increases, the real–time performance deteriorates. Conversely, a dbN wavelet system with too small an order (such as Db3) divides the frequency band roughly [40]. We also considered the dbN wavelet system calculation, so we selected the db8 wavelet basis. It could exclude, as far as possible, the Earth’s annual, daily, and basic temperature fields, temperature variations caused by hot or cold air currents and rain clouds, and minor temperature variations caused by other factors. Cold or hot air currents and rain clouds caused by temperature changes generally occur on short time scales (hours to days). They include high–frequency information and could be removed by a second–order wavelet transform based on the db8 wavelet basis. The Earth’s basic and annual temperature fields (notable annual variation information) could also be removed by applying the seven–order wavelet transform based on the db8 wavelet. They include low–frequency information. Since the second–order wavelet scale subtracted the seven–order wavelet scale, the information in the middle frequency band could be retained. The information in the high and low frequencies was omitted, and this step was equivalent to band–pass filtering.
- (2)
- We carried out a Fourier transform process to data within a moving window (window length n = 64 days, step m = 1 day). We obtained each power spectrum by windowing the start time of the data and the time series data for each pixel. In this way, the time–frequency spatial data were obtained. The similarities and differences between the thermal power spectra of the aseismic and seismic periods were analyzed using frequency and amplitude.
- (3)
- The power spectrum frequencies of each pixel were calculated from relative amplitudes using Equations (1) and (2) to generate spatial data with relative time–frequency variations.
2.2. Electric Field Data
- (1)
- Our observational study of orbital data passing near the epicenter exhibited an enhancement of the proton cyclotron frequency at ~600 Hz. In order to ensure the credibility of the data, we divided the frequency bands of the power spectral density into 371 Hz–500 Hz and 700 Hz–871 Hz [42,43], as shown in Figure 1.
- (2)
- We constructed the statistical background of observation (80° E–120° E; 12° N–52° N). The CSES has a revisit period of 5 days. The distance of its neighbor track is ~4.73° in longitude [41]. Thereby, in order to ensure that every grid exhibited data, we used a sliding step of 4° in longitude and 1° in latitude with at least one week of data in the region based on our tests. There were 10 × 40 grids in the region with a precision longitude 4° × latitude 1°.
- (3)
- We calculated the PSD data in the ELF band from 1 January 2021 to 12 December 2022. We got the median matrix β as the background and the standard variance matrix σ as the background perturbation. Two groups of 10 × 40 matrices could be obtained.
- (4)
- We selected data every 7–days to calculate the median to get the median matrix α as the real–time data. Finally, we used Equation (3) to extract perturbation amplitude θ.
2.3. Magnetic Field Data
2.4. Research Area
3. Results
3.1. Spatio–Temporal Evolution of Thermal Infrared Anomalies
3.2. Spatio–Temporal Evolution of Electric Field Anomalies
3.3. Spatio–Temporal Evolution of Magnetic Field Anomalies
4. Discussion
5. Conclusions
- (1)
- We selected the TBB data to be the thermal infrared remote sensing data observed by the FY–2G satellite. The TBB radiation began to increase notably along the northern fault of the epicenter ~1.5 months prior to the occurrence of the earthquake. It spread and increased along the southeastern side of the fault gradually. It achieved its maximum intensity on 17 May, and then began to decrease gradually. The Maduo earthquake occurred as the anomalies decreased. The anomalies lasted for ~2 months.
- (2)
- We selected the PSD data in the ELF frequency band detected by the EFD payload in the nighttime without the space magnetic environment perturbations. It was observed that the PSD in the 371 Hz–500 Hz and 700 Hz–871 Hz bands exhibited anomaly perturbations in the vicinity of the epicenter and its magnetic conjugate area on 17 May, with particularly notable anomaly perturbations in the magnetic conjugate area. We observed that anomaly perturbations began to occur ~1 month prior to the occurrence of the earthquake. The earthquake occurred as the anomalies decreased, and this trend continued post–earthquake. Among them, the vertical component perturbations were more notable as the dominant component, and the 700 Hz–871 Hz perturbations were more notable as the dominant frequency band.
- (3)
- We selected the magnetic field –east–west component vector data detected by the HPM payload, as well as the ion velocity Vx data recorded by the PAP payload for the study. The most notable magnetic field anomaly perturbations were exhibited in the vicinity of the Maduo epicenter and its magnetic conjugate area on 16 May, one week prior to the earthquake, and on 22 May, the occurrence date of the earthquake. In addition, magnetic field anomaly perturbations were also exhibited near the 2021 Ms6.4 Yangbi earthquake on 21 May. Both the magnetic –east–west component data and the Vx data exhibited anomaly perturbations near the epicenter and the magnetic conjugate area on 11 May and 16 May. However, the magnitudes of their perturbations were not consistent across the observations.
- (4)
- The anomaly perturbations observed in both thermal infrared TBB data and CSES electric and magnetic field data occur within a consistent perturbation time period and spatial proximity, suggesting a potential presence of LAIC relationships. There may be both chemical and electromagnetic wave propagation models involved in the LAIC mechanism related to the Maduo earthquake. It can be approximately hypothesized that three channels in the LAIC models may have varying degrees of influence before and after the earthquake, suggesting that there may be not more than one LAIC mechanism for the preparation and occurrence of an earthquake. In the future, we will integrate data from a broader range of sources and altitudes to investigate different types of earthquakes more comprehensively.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CSES | China Seismo–Electromagnetic Satellite |
| FY–2G | Chinese stationary meteorological satellites Feng Yun–2G |
| PSD | Power spectrum density |
| ELF | Extremely low frequency |
| TBB | Temperature of brightness blackbody |
| Vx | The ion velocity (velocity direction matches the direction of the satellite flight velocity) |
| LAIC | “lithosphere—atmosphere—ionosphere” coupling |
| DC | Direct current |
| UTC | Universal time coordinated |
| LT | Local time |
| USGS | United States Geological Survey |
| ULF | Ultralow–frequency |
| VLF | Very low frequency |
| OLR | Outgoing longwave radiation |
| MIB | Medium–wave infrared brightness |
| EFD | Electric field detector |
| HPM | High–precision magnetometer |
| CDSM | Coupled dark–state magnetometer |
| FGMs | Fluxgate sensors |
| PAP | Plasma analyzer package |
References
- Hayakawa, M. Electromagnetic phenomena associated with earthquakes: A frontier in terrestrial electromagnetic noise environment. Recent Res. Dev. Geophys. 2004, 6, 81–112. [Google Scholar]
- Pulinets, S.; Ouzounov, D. Lithosphere–Atmosphere–Ionosphere Coupling (LAIC) model:An unified concept for earthquake precursors validation. J. Asian Earth Sci. 2011, 41, 371–382. [Google Scholar] [CrossRef]
- Zhang, X.M.; Shen, X.H. The development in seismo–ionospheric coupling mechanism. Prog. Earthq. Sci. 2022, 52, 193–202. [Google Scholar]
- Zhao, S.F.; Shen, X.H.; Liao, L.; Zeren, Z. A lithosphere–atmosphere–ionosphere coupling model for ELF electromagnetic waves radiated from seismic sources and its possibility observed by the CSES. Sci. China Tech. Sci. 2021, 64, 2551–2559. [Google Scholar] [CrossRef]
- Nayak, K.; López-Urías, C.; Romero-Andrade, R.; Sharma, G.; Guzmán-Acevedo, G.M.; Trejo-Soto, M.E. Ionospheric Total Electron Content (TEC) Anomalies as Earthquake Precursors: Unveiling the Geophysical Connection Leading to the 2023 Moroccan 6.8 Mw Earthquake. Geosciences 2023, 13, 319. [Google Scholar] [CrossRef]
- Sharma, G.; Nayak, K.; Romero-Andrade, R.; Aslam, M.A.M.; Sarma, K.K.; Aggarwal, S.P. Low Ionosphere Density Above the Earthquake Epicentre Region of Mw 7.2, El Mayor–Cucapah Earthquake Evident from Dense CORS Data. J. Indian Soc. Remote Sens. 2024, 52, 543–555. [Google Scholar] [CrossRef]
- Tachema, A. Identifying Pre–Seismic Ionospheric Disturbances Using Space Geodesy: A Case Study of the 2011 Lorca Earthquake (Mw 5.1). Spain. Earth Sci. Inform. 2024, 17, 2055–2071. [Google Scholar] [CrossRef]
- Ouyang, X.Y.; Parrot, M.; Bortnik, J. ULF Wave Activity Observed in the Nighttime Ionosphere above and Some Hours before Strong Earthquakes. J. Geophys. Res. Space Phys. 2020, 125, e2020JA028396. [Google Scholar] [CrossRef]
- Huang, J.; Zhang, F.; Li, Z.; Shen, X.; Yang, B.; Li, W.; Zeren, Z.; Lu, H.; Tan, Q. Disturbance identification of electric field data observed by the CSES–01 satellite before earthquakes. Sci. China Earth Sci. 2023, 66, 1814–1824. [Google Scholar] [CrossRef]
- Li, Z.; Chen, Z.; Huang, J.; Li, X.; Han, Y.; Yang, X.; Li, Z. Study on VLF Electric Field Anomalies Caused by Seismic Activity on the Western Coast of the Pacific Rim. Atmosphere 2023, 14, 1676. [Google Scholar] [CrossRef]
- Yang, M.; Zhang, X.; Ouyang, X.; Liu, J.; Qian, G.; Li, T.; Shen, X. Polarization Method–Based Research on Magnetic Field Data Associated with Earthquakes in Northeast Asia Recorded by the China Seismo–Electromagnetic Satellite. Atmosphere 2023, 14, 1555. [Google Scholar] [CrossRef]
- Zhima, Z.; Hu, Y.; Piersanti, M.; Shen, X.; De Santis, A.; Yan, R.; Yang, Y.; Zhao, S.; Zhang, Z.; Wang, Q. The seismic electromagnetic emissions during the 2010 Mw 7.8 Northern Sumatra Earthquake revealed by DEMETER satellite. Front. Earth Sci. 2020, 8, 459. [Google Scholar] [CrossRef]
- Qian, G.; Zeren, Z.; Zhang, X.M.; Shen, X.H. Spatio–temporal evolution of electromagnetic field pre–and post–earthquakes. Acta Seismol. Sin. 2016, 38, 259–271+329. [Google Scholar]
- Shalimova, S.L.; Nesterovc, I.A.; Vorontsovc, A.M. On the GPS–based ionospheric perturbation after the Tohoku earthquake of March 11, 2011. Phys. Solid Earth 2017, 53, 262–273. [Google Scholar] [CrossRef]
- Akhoondzadeh, M.; De Santis, A.; Marchetti, D.; Piscini, A.; Cianchini, G. Multi precursors analysis associated with the powerful ecuador (MW = 7.8) earthquake of 16 April 2016 using Swarm satellites data in conjunction with other multi–platform satellite and ground data. Adv. Space Res. 2018, 61, 248–263. [Google Scholar] [CrossRef]
- Zahra, S.; Masoud, M.H. Application of the T2–Hotelling test for investigating ionospheric anomalies before large earthquakes. J. Atmos. Sol. Terr. Phys. 2019, 185, 7–21. [Google Scholar]
- Kuo, C.L.; Lee, L.C.; Huba, J.D. An improved coupling model for the lithosphere–atmosphere–ionosphere system. J. Geophys. Res. Space Phys. 2014, 119, 3189–3205. [Google Scholar] [CrossRef]
- Ouzounov, D.; Bryant, N.; Logan, T.; Pulinets, S.; Taylor, P. Satellite thermal IR phenomena associated with some of the major earthquakes in 1999–2003. Phys. Chem. Earth. 2006, 31, 154–163. [Google Scholar] [CrossRef]
- Salman, A.; Egan, W.G.; Tronin, A.A. Infrared remote sensing of seismic disturbances. In Polarization and Remote Sensing; SPIE: San Diego, CA, USA, 1992; pp. 208–218. [Google Scholar]
- Gorny, V.I.; Salman, A.G.; Tronin, A.A.; Shlin, B.V. The Earth’s outgoing IR radiation as an indicator of seismic activity. Proc. Acad. Sci. USSR 1988, 301, 67–69. [Google Scholar]
- Qiang, Z.; Xu, X.-D.; Dian, C.-G. Thermal infrared anomaly—Precursor of impending earthquakes. Chin. Sci. Bull. 1991, 36, 319–323. [Google Scholar] [CrossRef]
- Tronin, A.; Hayakawa, M.; Molchanov, O.A. Thermal IR satellite data application for earthquake research in Japan and China. J. Geodyn. 2002, 33, 519–534. [Google Scholar] [CrossRef]
- Tramutoli, V.; Cuomo, V.; Filizzola, C.; Pergola, N.; Pietrapertosa, C. Assessing the potential of thermal infrared satellite surveys for monitoring seismically active areas. The case of Kocaeli (_ Izmit) earthquake, August 17th, 1999. Remote Sens. Environ. 2005, 96, 409–426. [Google Scholar] [CrossRef]
- Freund, F. Charge generation and propagation in rocks. J. Geodyn. 2002, 33, 545–572. [Google Scholar] [CrossRef]
- Pulinets, S.; Ouzounov, D.; Karelin, A.; Boyarchuk, K.; Pokhmelnykh, L. The physical nature of thermal anomalies observed before strong earthquakes. Phys. Chem. Earth 2006, 31, 143–153. [Google Scholar] [CrossRef]
- Liu, X.; Wu, L.; Zhang, Y.; Mao, W. Localized Enhancement of Infrared Radiation Temperature of Rock Compressively Sheared to Fracturing Sliding: Features and Significance. Front. Earth Sci. 2021, 9, 756369. [Google Scholar] [CrossRef]
- Qi, Y.; Wu, L.; Ding, Y.; Liu, Y.; Chen, S.; Wang, X.; Mao, W. Extraction and Discrimination of MBT Anomalies Possibly Associated with the Mw 7.3 Maduo (Qinghai, China) Earthquake on 21 May 2021. Remote Sens. 2021, 13, 4726. [Google Scholar] [CrossRef]
- Satti, M.S.; Ehsan, M.; Abbas, A.; Shah, M.; de Oliveira-Júnior, J.F.; Naqvi, N.A. Atmospheric and ionospheric precursors associated with Mw6.5 earthquakes from multiple satellites. J. Atmos. Sol. Terr. Phys. 2022, 227, 105802. [Google Scholar] [CrossRef]
- Jing, F.; Zhang, L.; Singh, R.P. Pronounced Changes in Thermal Signals Associated with the Madoi (China) M 7.3 Earthquake from Passive Microwave and Infrared Satellite Data. Remote Sens. 2022, 14, 2539. [Google Scholar] [CrossRef]
- Zhong, M.J.; Shan, X.J.; Zhang, X.M.; Qu, C.Y.; Guo, X.; Jiao, Z.H. Thermal infrared and ionospheric anomalies of the 2017 Mw6.5 jiuzhaigou earthquake. Remote Sens. 2020, 12, 2843. [Google Scholar] [CrossRef]
- De Santis, A.; Perrone, L.; Calcara, M.; Campuzano, S.; Cianchini, G.; D’arcangelo, S.; Di Mauro, D.; Marchetti, D.; Nardi, A.; Orlando, M.; et al. A comprehensive multiparametric and multilayer approach to study the preparation phase of large earthquakes from ground to space: The case study of the June 15 2019, M7.2 Kermadec Islands (New Zealand) earthquake. Remote Sens. Environ. 2022, 283, 113325. [Google Scholar] [CrossRef]
- Wang, Y.; Ma, W.; Zhao, B.; Yue, C.; Zhu, P.; Yu, C.; Yao, L. Responses to the Preparation of the 2021 M7.4 Madoi Earthquake in the Lithosphere–Atmosphere–Ionosphere System. Atmosphere 2023, 14, 1315. [Google Scholar] [CrossRef]
- Chen, X.F.; Wu, Z.L.; Shi, Y.L.; Zhang, P.Z.; Shao, Z.G. Deepening the research on the mechanism and prediction of strong continental earthquakes. Chin. Sci. Bull. 2022, 67, 1347–1351. [Google Scholar] [CrossRef]
- Zhang, P.Z.; Shen, Z.; Wang, M.; Gan, W.J.; Burgmann, R.; Molnar, P. Continuous deformation of the Tibetan Plateau from global positioning system data. Geology 2004, 32, 809–812. [Google Scholar] [CrossRef]
- Xu, R.; Sarah, D.S. Present–day kinematics of the eastern Tibetan Plateau and Sichuan Basin: Implications for lower crustal rheology. J. Geophys. Res. Solid Earth 2016, 121, 3846–3866. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, Y.S.; Wei, C.X. Comparative Study on Thermal Infrared Anomaly Characteristics of Several Moderate and Strong Earthquakes in Yunnan Province. Imaging Sci. Photochem. 2019, 37, 215–226. [Google Scholar]
- Zhang, Y.; Kang, C.L.; Ma, W.Y.; Yao, Q. The change in outgoing longwave radiation before the Ludian Ms6.5 earthquake based on tidal force niche cycles. Seismol. Geomagn. Obs. Res. 2016, 37, 68–74. [Google Scholar]
- Wei, C.X.; Zhang, Y.S.; Wang, Y. Time–frequency analysis of influence of MW9.1 earthquake on regional thermal radiation background field. Acta Seismol. Sin. 2018, 40, 205–214. [Google Scholar]
- Guo, X.; Zou, R.; Zhang, X.; Wang, Y. Analysis of long wave radiation anomalies of several strong earthquakes in mainland China. China Earthq. Eng. J. 2019, 41, 1221–1227+1250. [Google Scholar]
- Zhu, X.; Jie, D.; Gao, Q.; Zhang, Y.C. On the application of analyzing power system harmonics using Db8. J. Electr. Power Sci. Technol. 2011, 26, 67–71. [Google Scholar] [CrossRef]
- Shen, X.H.; Zhang, X.; Yuan, S.G.; Wang, L.W.; Cao, J.B.; Huang, J.P.; Zhu, X.H.; Piergiorgio, P.; Dai, J.P. The state–of–the–art of the China Seismo–Electromagnetic Satellite mission. Sci. China Technol. Sci. 2018, 61, 634–642. [Google Scholar] [CrossRef]
- Zeren, Z.M.; Shen, X.H.; Cao, J.B.; Zhang, X.M.; Huang, J.P.; Liu, J.; Ouyang, X.Y.; Zhao, S.F. Statistical analysis of ELF/VLF magnetic field disturbances before major earthquakes. Chin. J. Geophys. 2012, 55, 3699–3708. (In Chinese) [Google Scholar]
- Hu, Y.; Zhima, Z.; Huang, J.; Zhao, S.; Guo, F.; Wang, Q.; Shen, X. Algorithms and implementation of wave vector analysis tool for the electromagnetic waves recorded by the CSES satellite. Chin. J. Geophys. 2020, 63, 1751–1765. [Google Scholar]
- Zhou, B.; Yang, Y.; Zhang, Y.; Gou, X.; Cheng, B.; Wang, J.; Li, L. Magnetic field data processing methods of the China SeismoElectromagnetic Satellite. Earth Planet. Phys. 2018, 2, 455–461. [Google Scholar] [CrossRef]
- Pollinger, A.; Lammegger, R.; Magnes, W.; Hagen, C.; Ellmeier, M.; Jernej, I.; Leichtfried, M.; Kürbisch, C.; Maierhofer, R.; Wallner, R.; et al. Coupled dark state magnetometer for the China Seismo–Electromagnetic Satellite. Meas. Sci. Technol. 2018, 29, 095103. [Google Scholar] [CrossRef]
- Hu, Y.; Zhima, Z.; Fu, H.; Cao, J.; Piersanti, M.; Wang, T.; Yang, D.; Sun, X.; Lv, F.; Lu, C.; et al. A large–scale magnetospheric line radiation event in the upper ionosphere recorded by the China–SeismoElectromagnetic Satellite. J. Geophys. Res. Space Phys. 2023, 128, e2022JA030743. [Google Scholar] [CrossRef]
- De Santis, A.; Balasis, G.; Pavón-Carrasco, F.J.; Cianchini, G.; Mandea, M. Potential earthquake precursory pattern from space: The 2015 Nepal event as seen by magnetic Swarm satellites. Earth Planet. Sci. Lett. 2017, 461, 119–126. [Google Scholar] [CrossRef]
- Marchetti, D.; De Santis, A.; D’Arcangelo, S.; Poggio, F.; Jin, S.; Piscini, A. Magnetic field and electron density anomalies from swarm satellites preceding the major earthquakes of the 2016–2017 Amatrice–Norcia (Central Italy) seismic sequence. Pure Appl. Geophys. 2019, 177, 305–319. [Google Scholar] [CrossRef]
- Ouyang, X.Y.; Shen, X. Typical interferences of ULF electric field waveform data observed by DEMETER. Seismol. Geomagn. Obs. Res. 2015, 36, 19–25. [Google Scholar]
- Zhang, P.; Deng, Q.; Zhang, G.; Ma, J.; Gan, W.; Min, W.; Mao, F.; Wang, Q. Active tectonic blocks and strong earthquakes in the continent of China. Sci. China Ser. D Earth Sci. 2003, 46 (Suppl. S2), 13–24. [Google Scholar] [CrossRef]
- Deng, Q.D.; Gao, X.; Chen, G.H.; Hu, Y. Recent tectonic activity of Bayankala fault–block and the Kunlun–Wenchuan earthquake series of the Tibetan Plateau. Earth Sci. Front. 2010, 17, 163–178. (In Chinese) [Google Scholar]
- Pan, J.W.; Bai, M.K.; Li, C.; Liu, F.; Li, H.; Liu, D.; Chevalier, M.L.; Wu, K.; Wang, P.; Lu, H.; et al. Coseismic surface rupture and seismogenic structure of the 2021–05–22 Maduo (Qinghai) MS7.4. Earthq. Acta Geol. Sin. 2021, 95, 1655–1670. (In Chinese) [Google Scholar] [CrossRef]
- Wang, W.; Fang, L.; Wu, J.; Tu, H.; Chen, L.; Lai, G.; Zhang, L. Aftershock sequence relocation of the 2021 MS7.4Maduo Earthquake, Qinghai, China. Sci. China Earth Sci. 2021, 64, 1371–1380. [Google Scholar] [CrossRef]
- Deng, Q.; Zhang, P.; Ran, Y.; Yang, X.; Min, W.; Chu, Q. Basic characteristics of active tectonics of China. Sci. China Ser. D Earth Sci. 2002, 46, 356–372. [Google Scholar] [CrossRef]
- Xu, X.; Yu, G.; Ma, W.; Klinger, Y.; Tapponnier, P. Rupture behavior and deformation localization of the Kunlunshan earthquake (MW7.8) and their tectonic implications. Sci. China Ser. D Earth Sci. 2008, 51, 1361–1374. [Google Scholar] [CrossRef]
- Wu, L.X.; Lu, J.C.; Mao, W.F.; Hu, J.; Zhou, Z.; Li, Z.W.; Qi, Y.; Yao, R.B. Sectional fault–inclination–change based numerical simulation of tectonic stress evolution on the seismogenic fault of Madoi earthquake. Chin. J. Geophys. 2022, 65, 3844–3857. (In Chinese) [Google Scholar] [CrossRef]
- Dobrovolsky, I.P.; Zubkov, S.I.; Miachkin, V.I. Estimation of the size of earthquake preparation zones. Pure Appl. Geophys. 1979, 117, 1025–1044. [Google Scholar] [CrossRef]
- Guo, W.Y.; Shan, X.J.; Qu, C.Y. Correlation between infrared anomalous and earthquakes in Tarim basin. Arid. Land Geogr. 2006, 29, 736–741. (In Chinese) [Google Scholar]
- Wu, L.X.; Liu, S.J.; Chen, Y.H.; Ma, B.D.; Li, L.L. Satellite thermal infrared anomaly and cloud anomaly before Wenchuan Earthquake. Sci. Technol. Rev. 2008, 26, 32–36. [Google Scholar]
- Zhang, L.F.; Guo, X.; Zhang, X.; Tu, H.W. Anomaly of thermal infrared brightness temperature and basin effect before Jiuzhaigou MS7.0 earthquake in 2017. Acta Seismol. Sin. 2018, 40, 797–808. [Google Scholar]
- Cicerone, R.D.; Ebel, J.E.; Britton, J. A systematic compilation of earthquake precursors. Tectonophysics 2009, 476, 371–396. [Google Scholar] [CrossRef]
- Li, J.; Zhou, L.Q.; Long, H.Y.; Nie, X.H.; Guo, Y. Spatial–temporal characteristics of the focal mechanism consistency parameter in Tianshan (within chinese territory) seismic zone. Seismol. Geol. Chin. 2015, 37, 792–803. [Google Scholar]
- Hongtao, W.; Zuji, Q. Reasearch on earthquake prediction using satellite thermal infrared anomaly. Adv. Earth Sci. Chin. 1995, 10, 537–541. [Google Scholar]
- Liu, D.; Zeren, Z.; Shen, X.; Zhao, S.; Yan, R.; Wang, X.; Liu, C.; Guan, Y.; Zhu, X.; Miao, Y.; et al. Typical ionospheric disturbances revealed by the plasma analyzer package onboard the China Seismo–Electromagnetic Satellite. Adv. Space Res. 2021, 68, 3796–3805. [Google Scholar] [CrossRef]
- Huang, Q. Rethinking earthquake–related DC–ULF electromagnetic phenomena: Towards a physics–based approach. Nat. Hazards Earth Syst. Sci. 2011, 11, 2941–2949. [Google Scholar] [CrossRef]
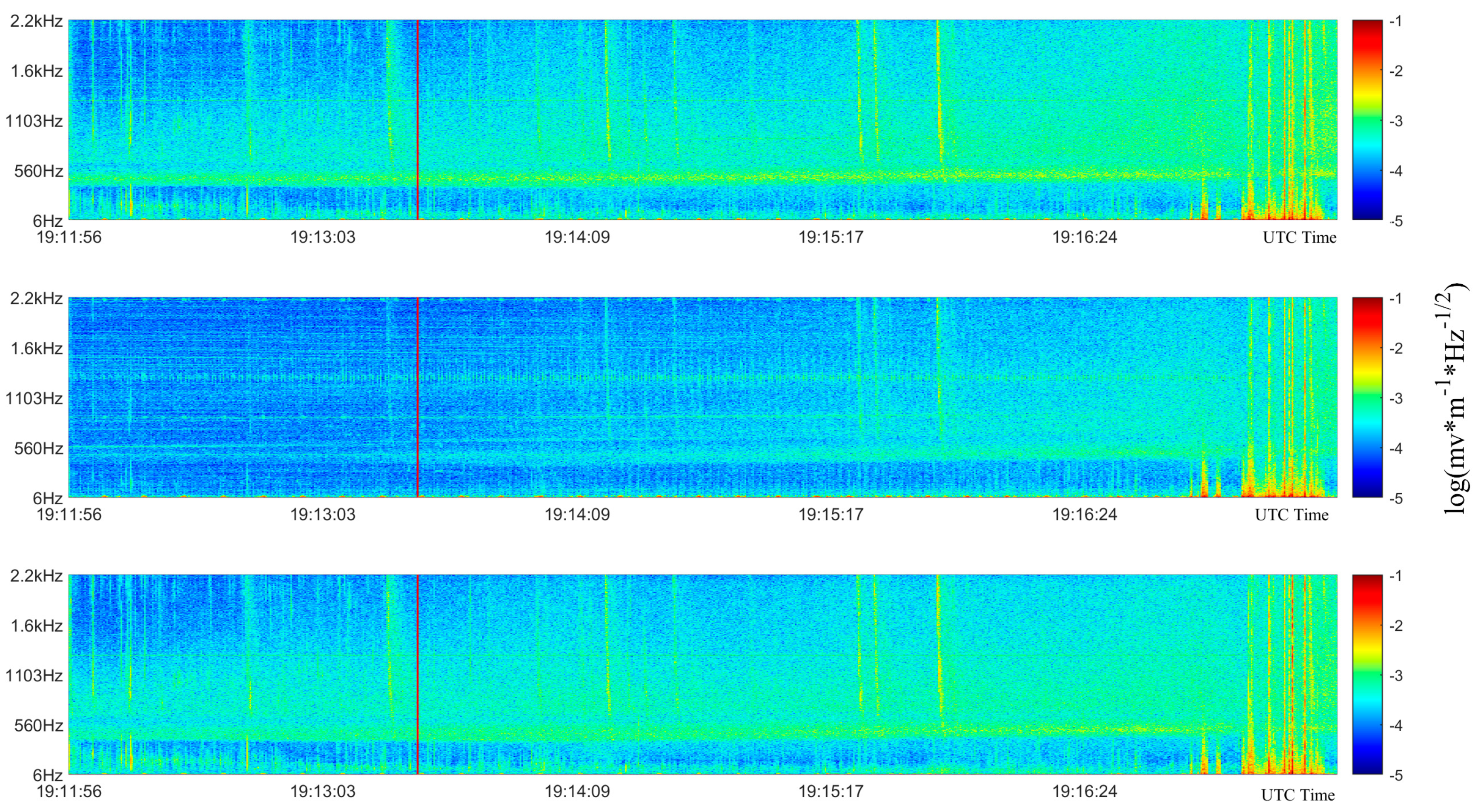
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).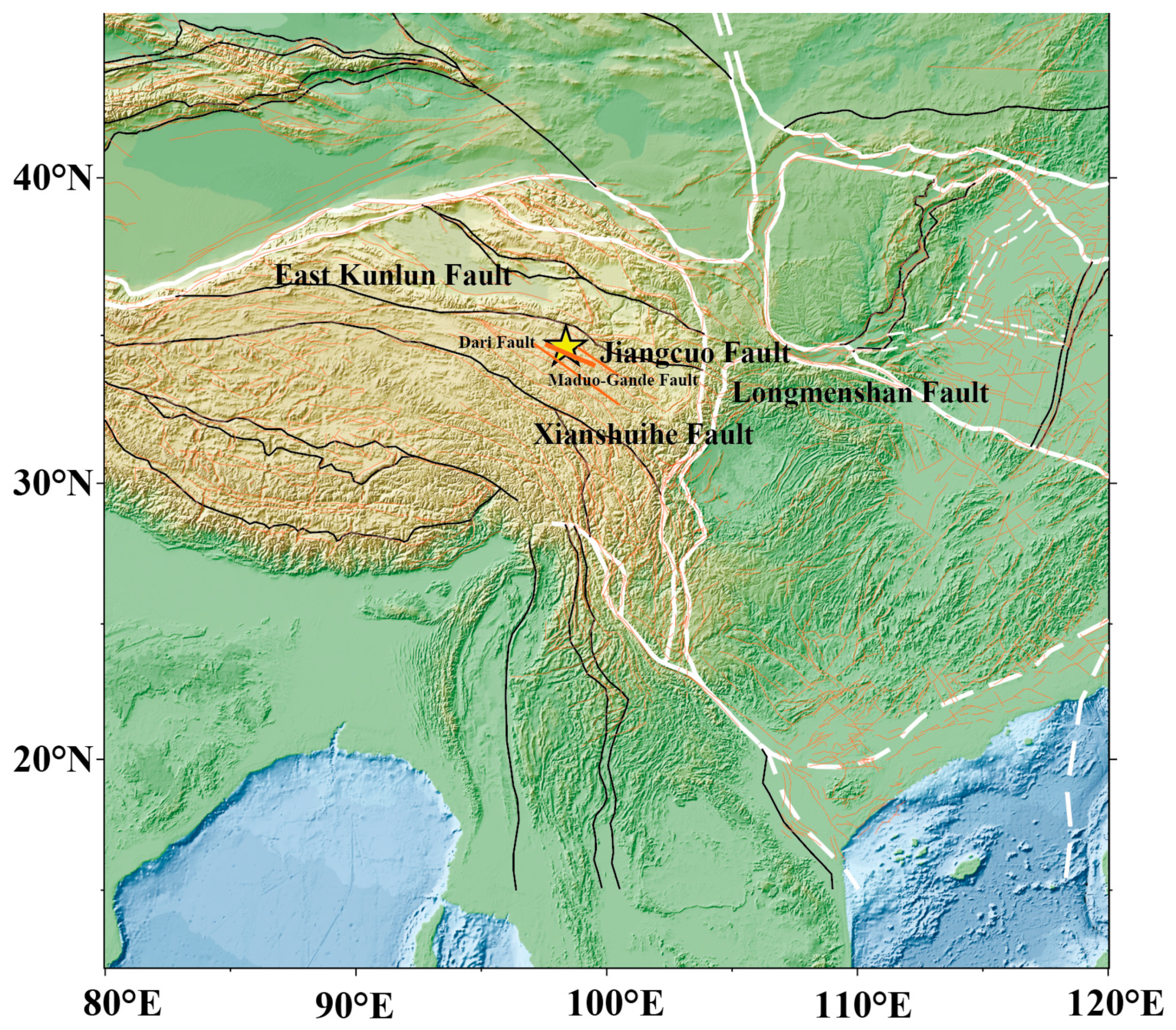
 : epicenter, the black lines are faults).
: epicenter, the black lines are faults).
 : epicenter, the black lines are faults).
: epicenter, the black lines are faults).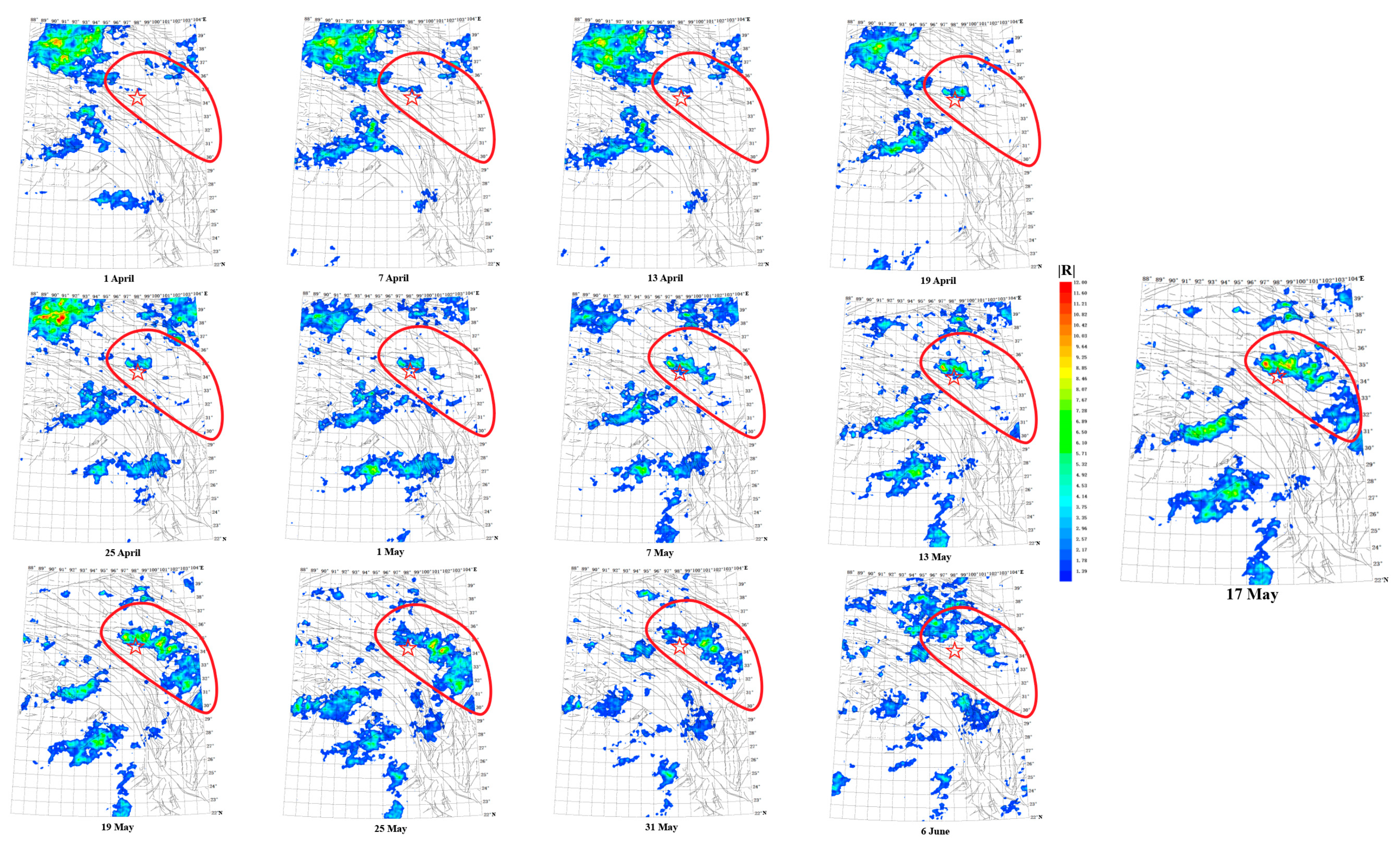
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).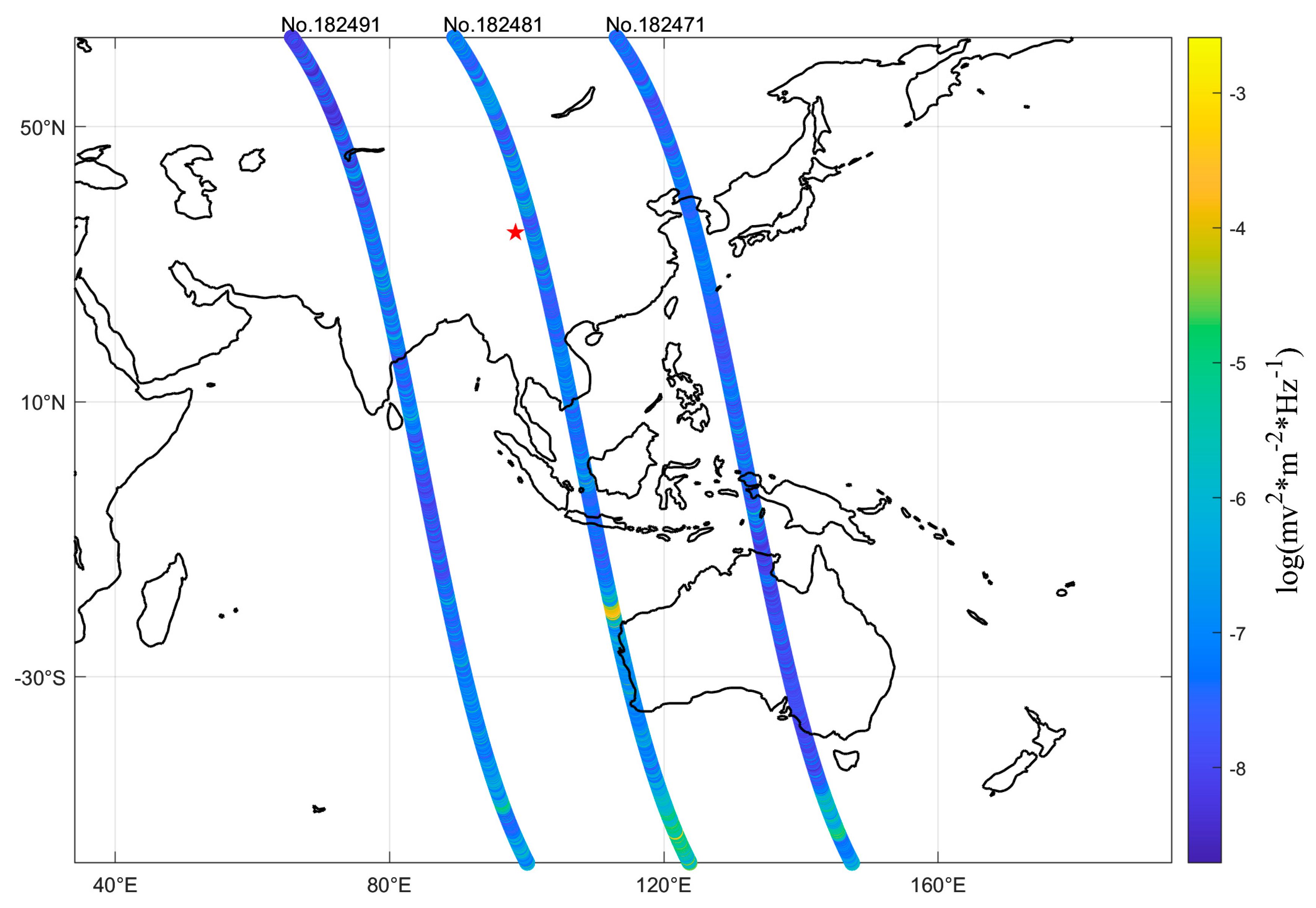
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).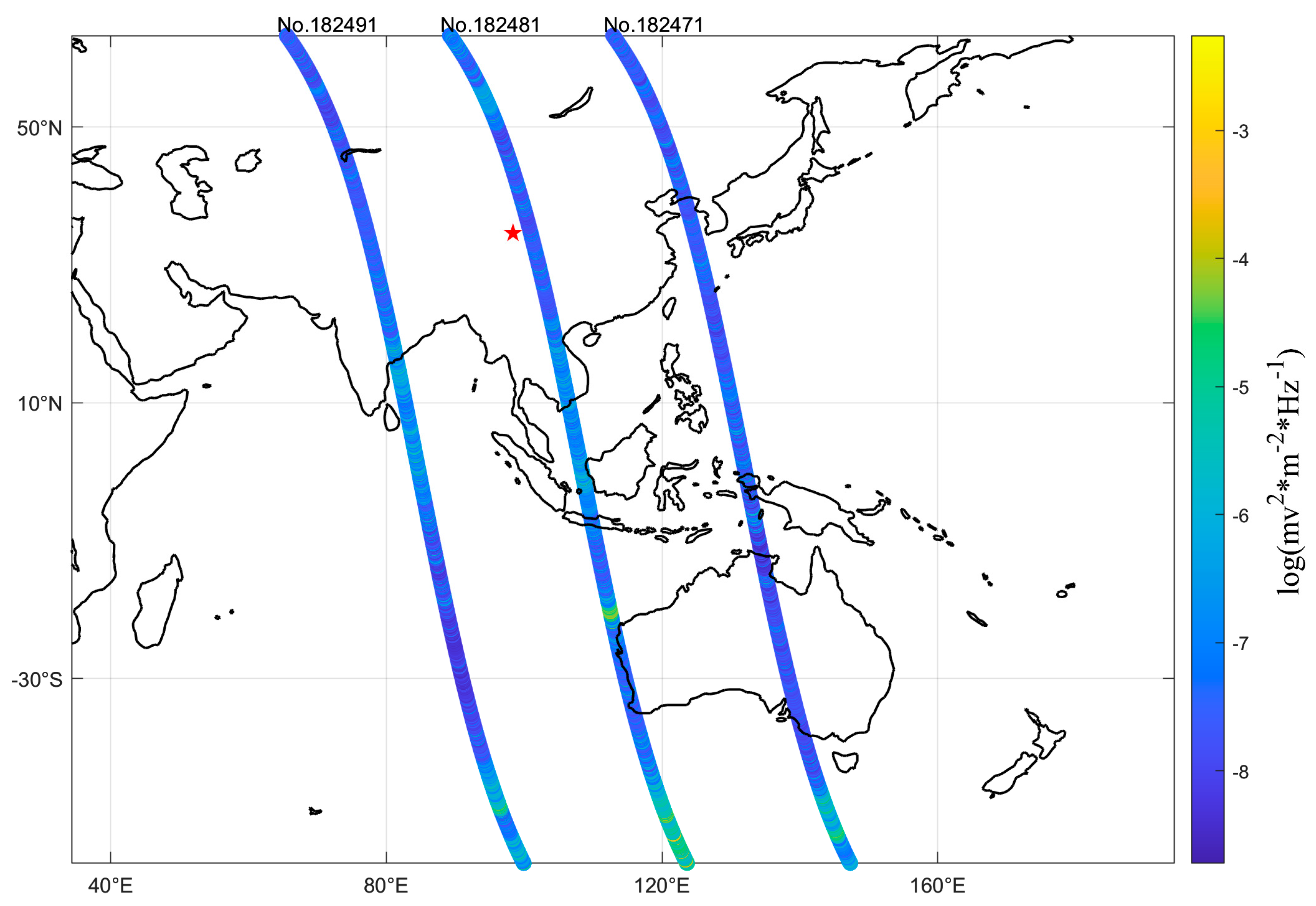
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).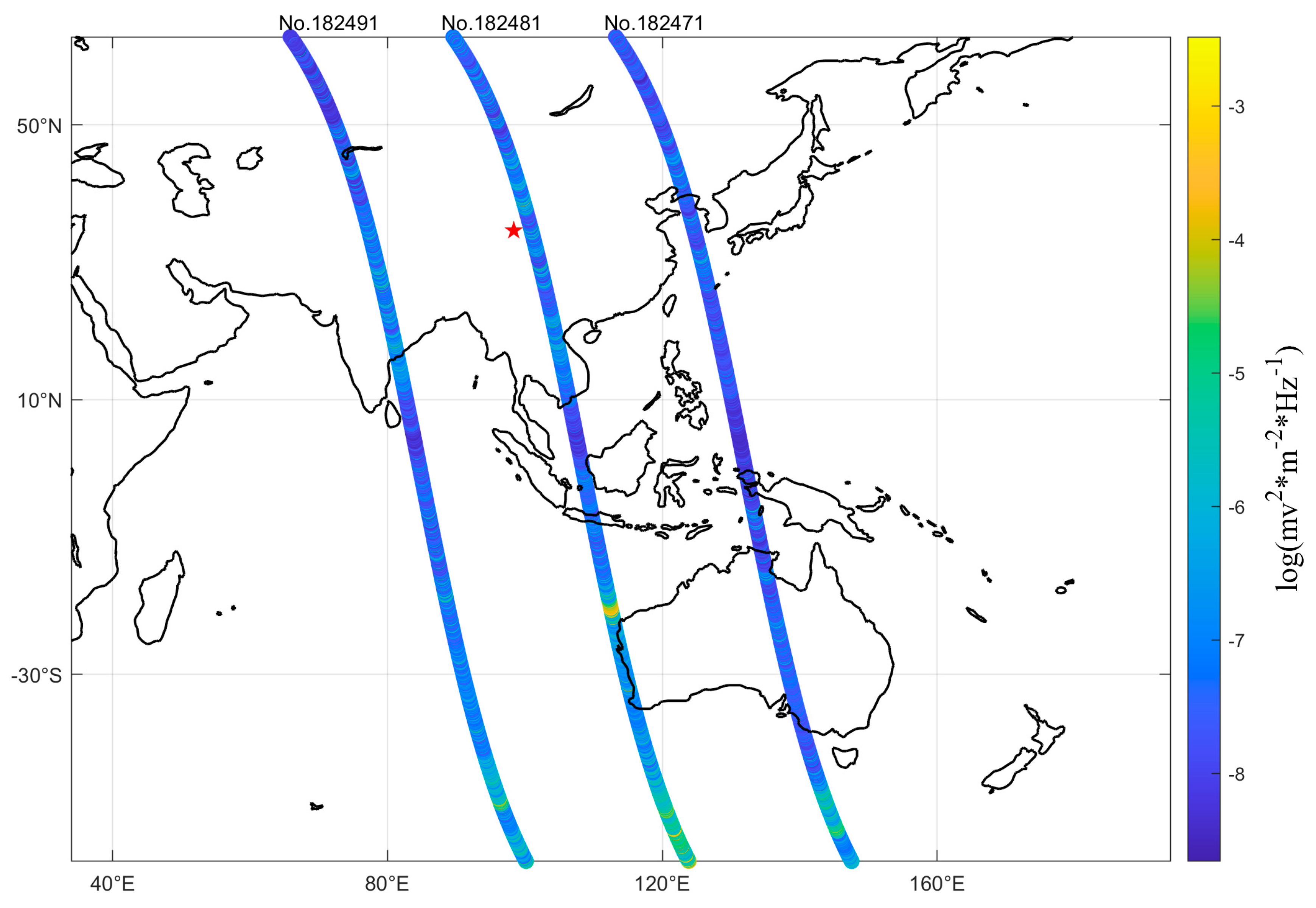
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).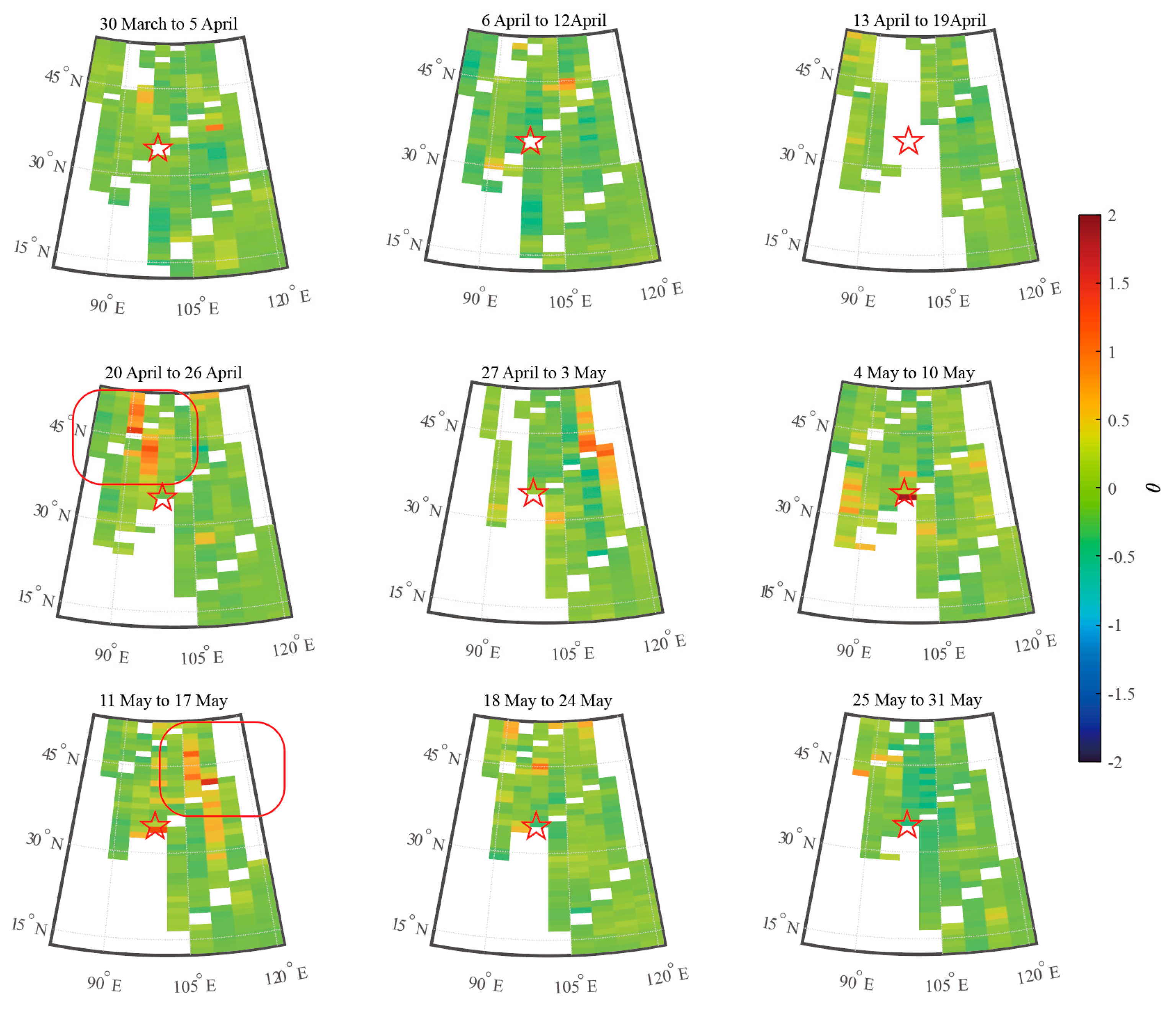
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).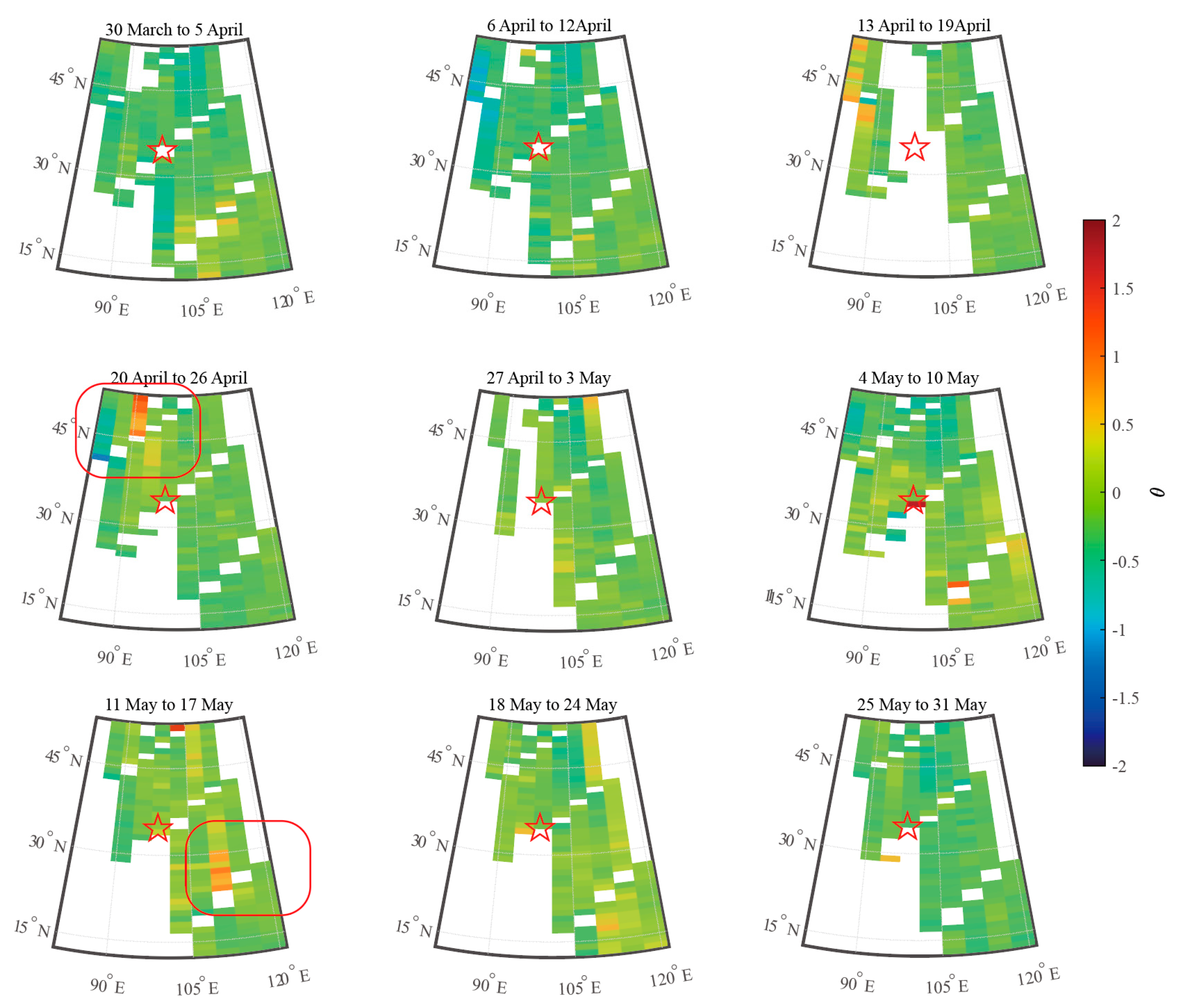
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).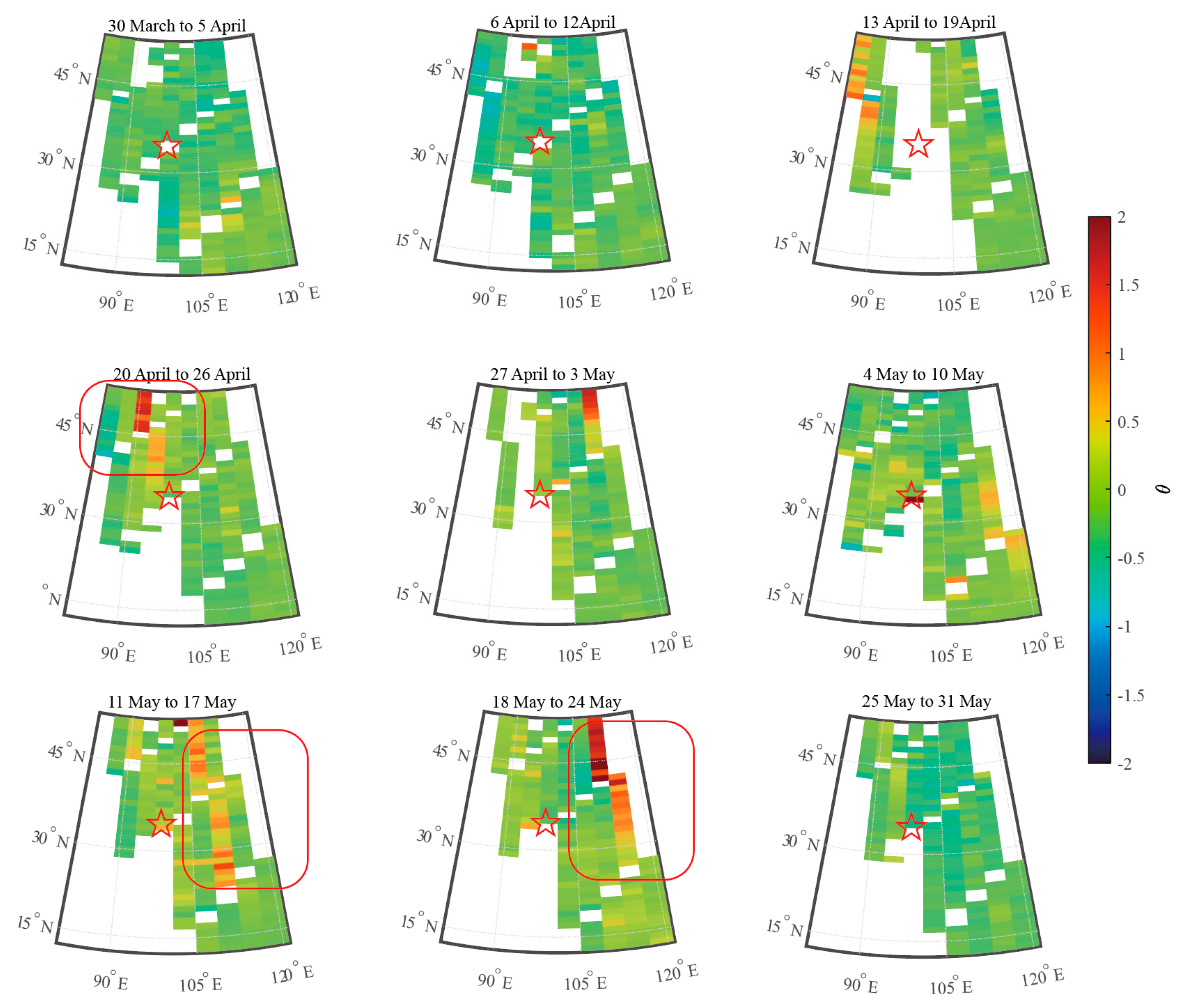
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).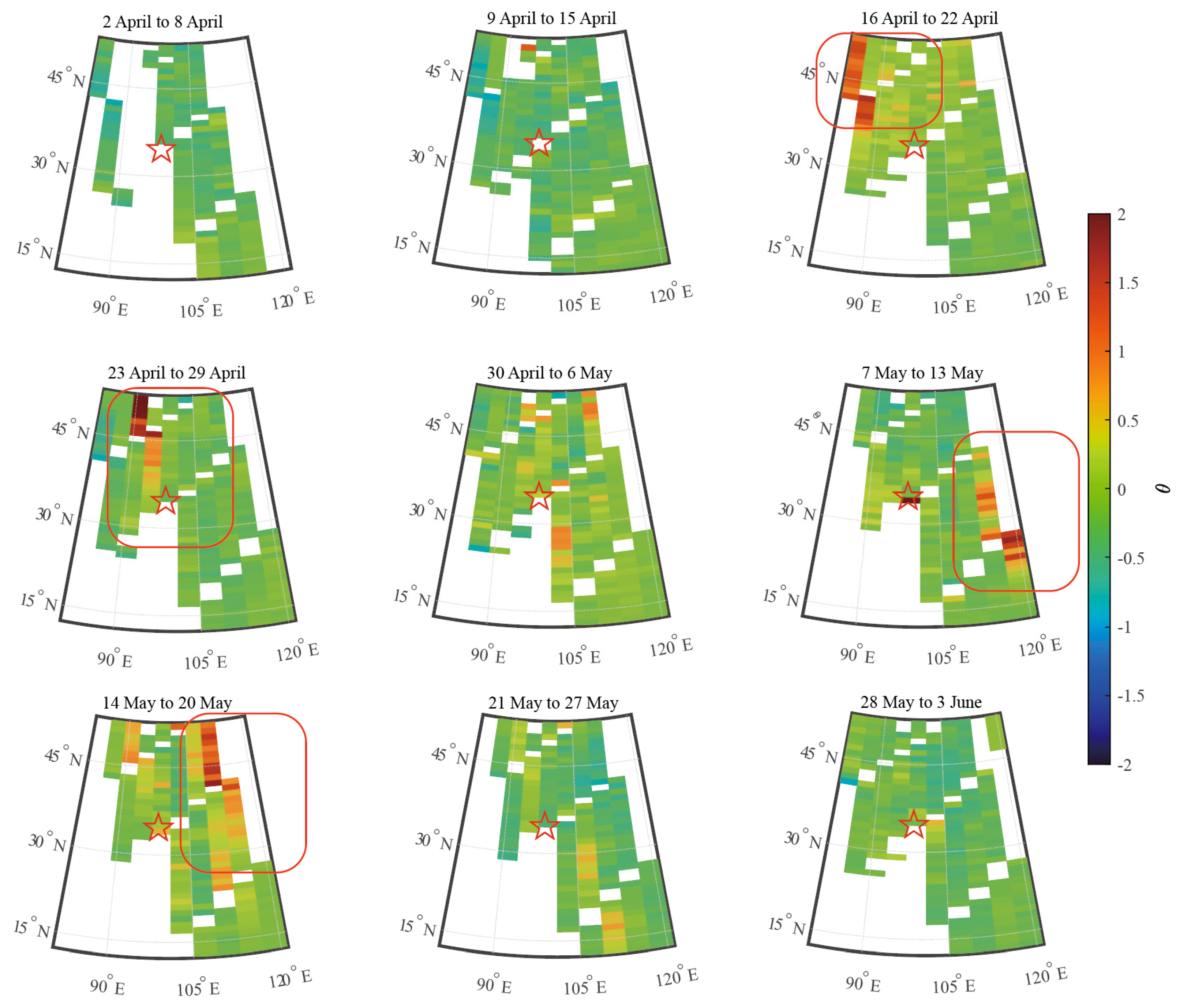
 : epicenter).
: epicenter).
 : epicenter).
: epicenter).
 : epicenter, the sets of red dashed lines are the latitudes of the epicenters and the magnetic conjugate region for the 2021 Yangbi Ms6.4 earthquake and the 2021 Maduo Mw7.3 earthquake, the two pentagrams are the epicenters of the two earthquakes).
: epicenter, the sets of red dashed lines are the latitudes of the epicenters and the magnetic conjugate region for the 2021 Yangbi Ms6.4 earthquake and the 2021 Maduo Mw7.3 earthquake, the two pentagrams are the epicenters of the two earthquakes).
 : epicenter, the sets of red dashed lines are the latitudes of the epicenters and the magnetic conjugate region for the 2021 Yangbi Ms6.4 earthquake and the 2021 Maduo Mw7.3 earthquake, the two pentagrams are the epicenters of the two earthquakes).
: epicenter, the sets of red dashed lines are the latitudes of the epicenters and the magnetic conjugate region for the 2021 Yangbi Ms6.4 earthquake and the 2021 Maduo Mw7.3 earthquake, the two pentagrams are the epicenters of the two earthquakes).
 : epicenter, the sets of red dashed lines are the latitude of the epicenters and the magnetic conjugate region for the 2021 Yangbi Ms6.4 earthquake and the 2021 Maduo Mw7.3 earthquake. The two pentagrams are the epicenters of the two earthquakes).
: epicenter, the sets of red dashed lines are the latitude of the epicenters and the magnetic conjugate region for the 2021 Yangbi Ms6.4 earthquake and the 2021 Maduo Mw7.3 earthquake. The two pentagrams are the epicenters of the two earthquakes).
 : epicenter, the sets of red dashed lines are the latitude of the epicenters and the magnetic conjugate region for the 2021 Yangbi Ms6.4 earthquake and the 2021 Maduo Mw7.3 earthquake. The two pentagrams are the epicenters of the two earthquakes).
: epicenter, the sets of red dashed lines are the latitude of the epicenters and the magnetic conjugate region for the 2021 Yangbi Ms6.4 earthquake and the 2021 Maduo Mw7.3 earthquake. The two pentagrams are the epicenters of the two earthquakes).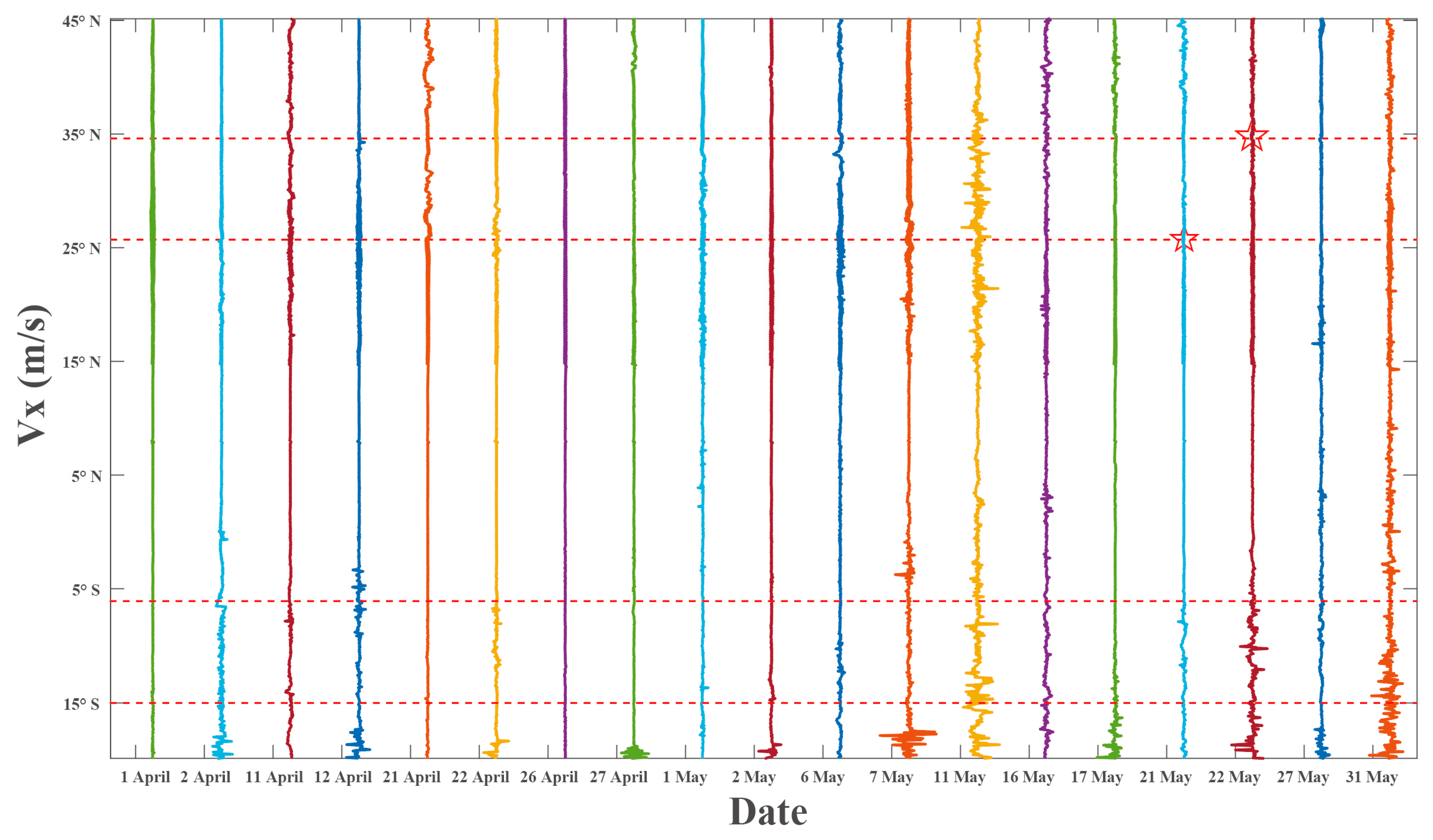
| Magnitude (Ms) | Date | Longitude (°E) | Latitude (°N) | Depth (km) | Location |
|---|---|---|---|---|---|
| 6.1 | 19 March 2021 | 92.75 | 31.94 | 10 | Biru |
| 6.4 | 21 May 2021 | 99.88 | 25.70 | 10 | Yangbi |
| 7.4 | 22 May 2021 | 98.37 | 34.61 | 17 | Maduo |
| 6.0 | 16 September 2021 | 105.34 | 29.20 | 10 | Luxian |
| 6.9 | 8 January 2022 | 101.25 | 37.77 | 10 | Menyuan |
| 6.0 | 26 March 2022 | 97.33 | 38.50 | 10 | Delingha |
| 6.1 | 1 June 2022 | 102.94 | 30.37 | 17 | Yaan |
| 6.0 | 10 June 2022 | 101.82 | 32.25 | 13 | Maerkang |
| 6.8 | 5 September 2022 | 102.8 | 29.59 | 16 | Luding |
| 6.2 | 18 December 2023 | 102.79 | 35.7 | 10 | Jishishan |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, M.; Zhang, X.; Zhong, M.; Guo, Y.; Qian, G.; Liu, J.; Yuan, C.; Li, Z.; Wang, S.; Zhai, L.; et al. Spatio–Temporal Evolution of Electric Field, Magnetic Field and Thermal Infrared Remote Sensing Associated with the 2021 Mw7.3 Maduo Earthquake in China. Atmosphere 2024, 15, 770. https://doi.org/10.3390/atmos15070770
Yang M, Zhang X, Zhong M, Guo Y, Qian G, Liu J, Yuan C, Li Z, Wang S, Zhai L, et al. Spatio–Temporal Evolution of Electric Field, Magnetic Field and Thermal Infrared Remote Sensing Associated with the 2021 Mw7.3 Maduo Earthquake in China. Atmosphere. 2024; 15(7):770. https://doi.org/10.3390/atmos15070770
Chicago/Turabian StyleYang, Muping, Xuemin Zhang, Meijiao Zhong, Yufan Guo, Geng Qian, Jiang Liu, Chao Yuan, Zihao Li, Shuting Wang, Lina Zhai, and et al. 2024. "Spatio–Temporal Evolution of Electric Field, Magnetic Field and Thermal Infrared Remote Sensing Associated with the 2021 Mw7.3 Maduo Earthquake in China" Atmosphere 15, no. 7: 770. https://doi.org/10.3390/atmos15070770
APA StyleYang, M., Zhang, X., Zhong, M., Guo, Y., Qian, G., Liu, J., Yuan, C., Li, Z., Wang, S., Zhai, L., Li, T., & Shen, X. (2024). Spatio–Temporal Evolution of Electric Field, Magnetic Field and Thermal Infrared Remote Sensing Associated with the 2021 Mw7.3 Maduo Earthquake in China. Atmosphere, 15(7), 770. https://doi.org/10.3390/atmos15070770







